Evaluation of a Prototype Broadband Water-Vapour Profiling Differential Absorption Lidar at Cardington, UK
Abstract
:1. Introduction
2. BB-DIAL Description and Campaign Setting
2.1. Instrument Description
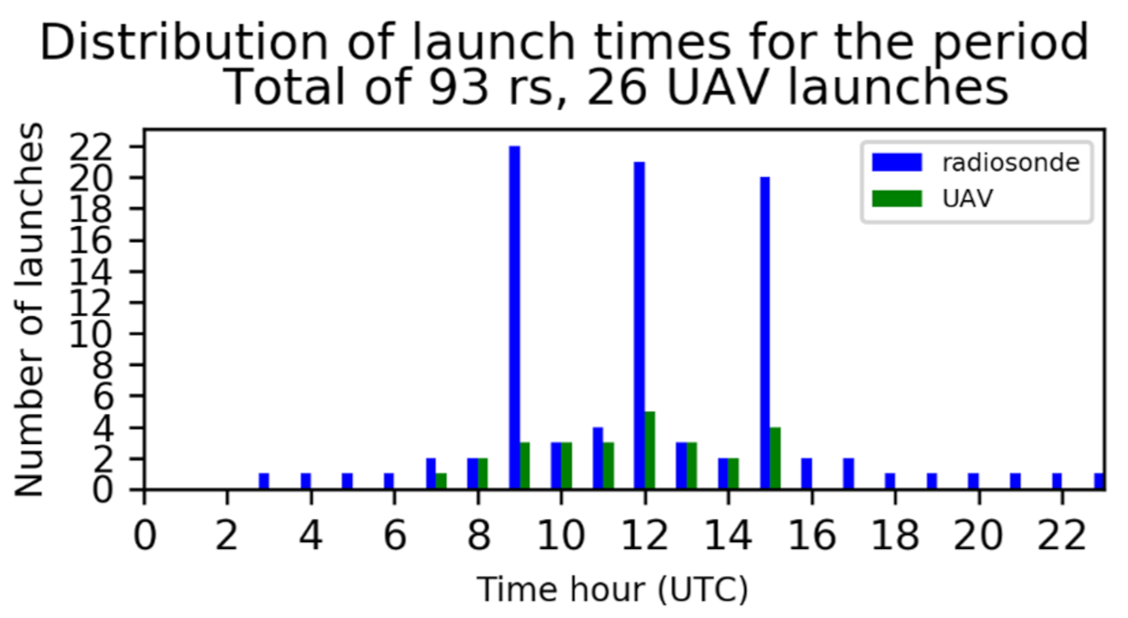
2.2. Campaign Setting
3. Data Analysis
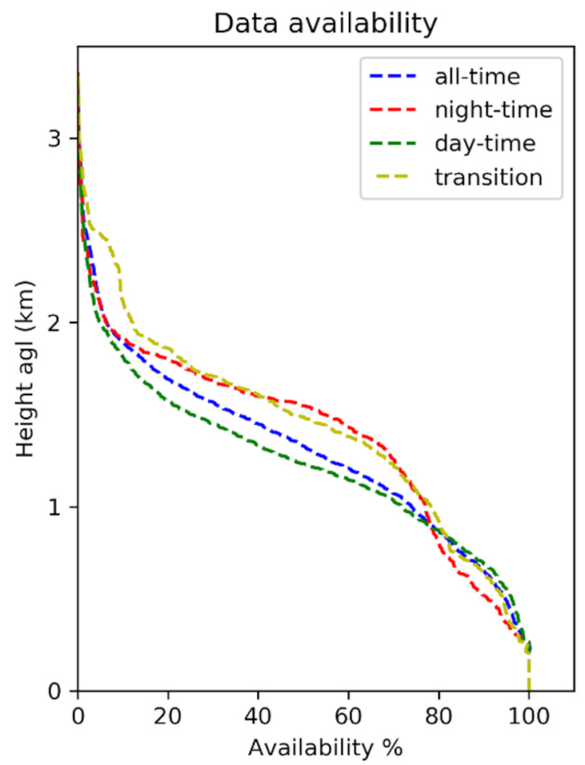
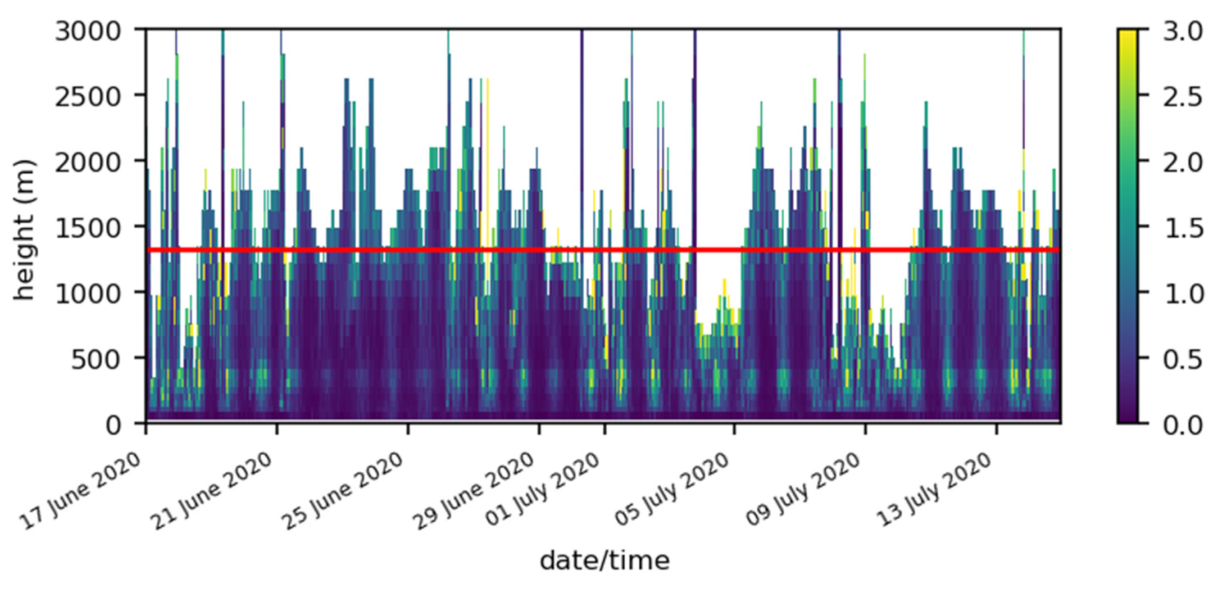
3.1. Data Availability
3.2. Comparison of Water Vapour Mixing Ratio
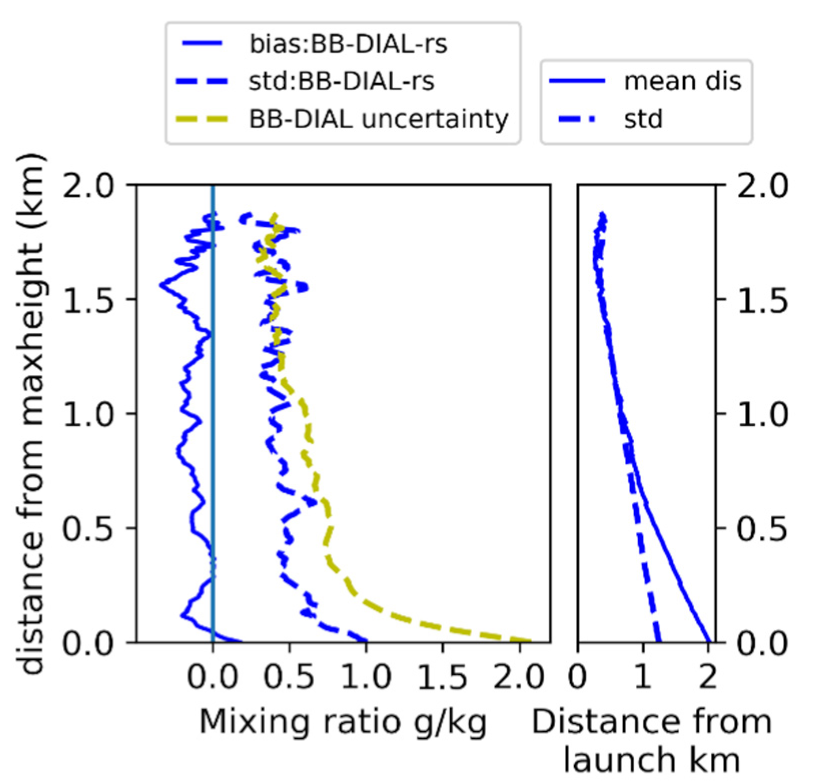
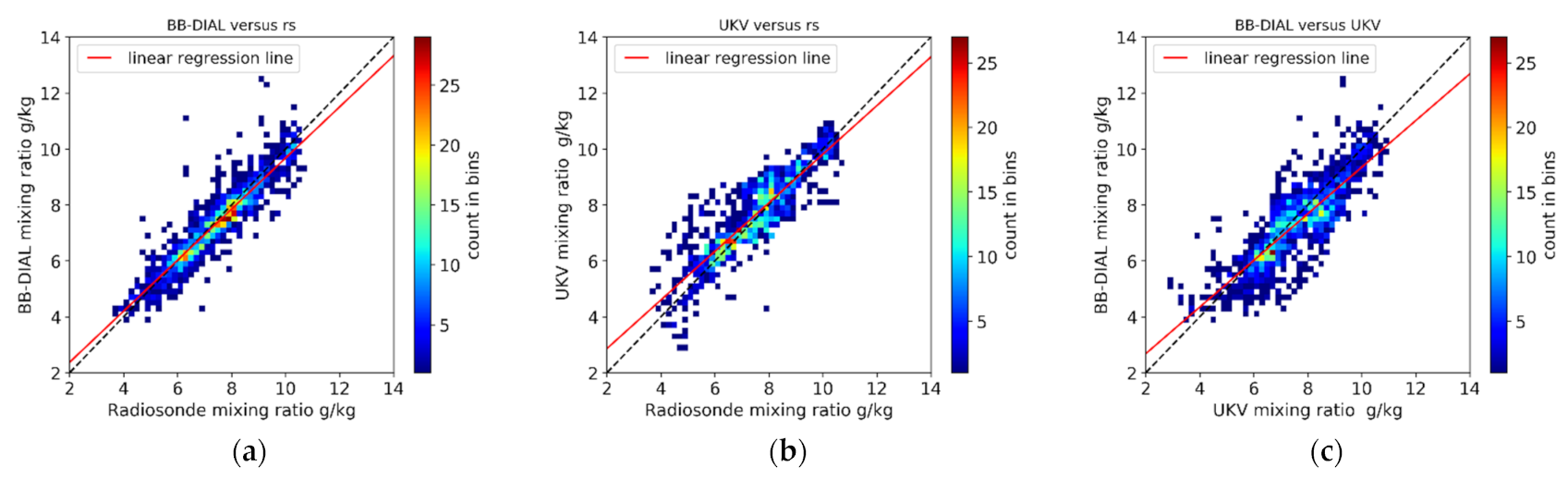
3.2.1. BB-DIAL versus Radiosonde
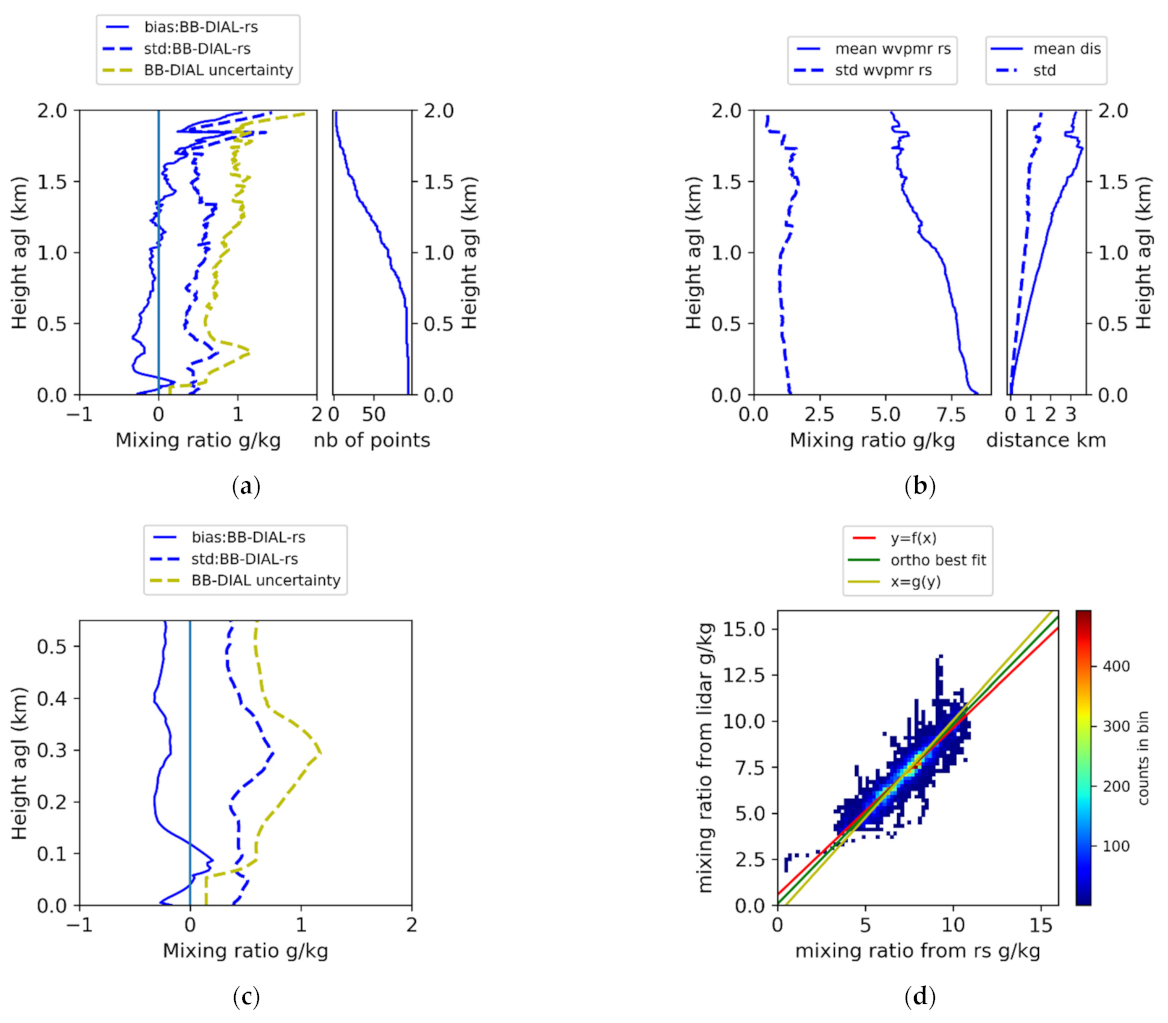
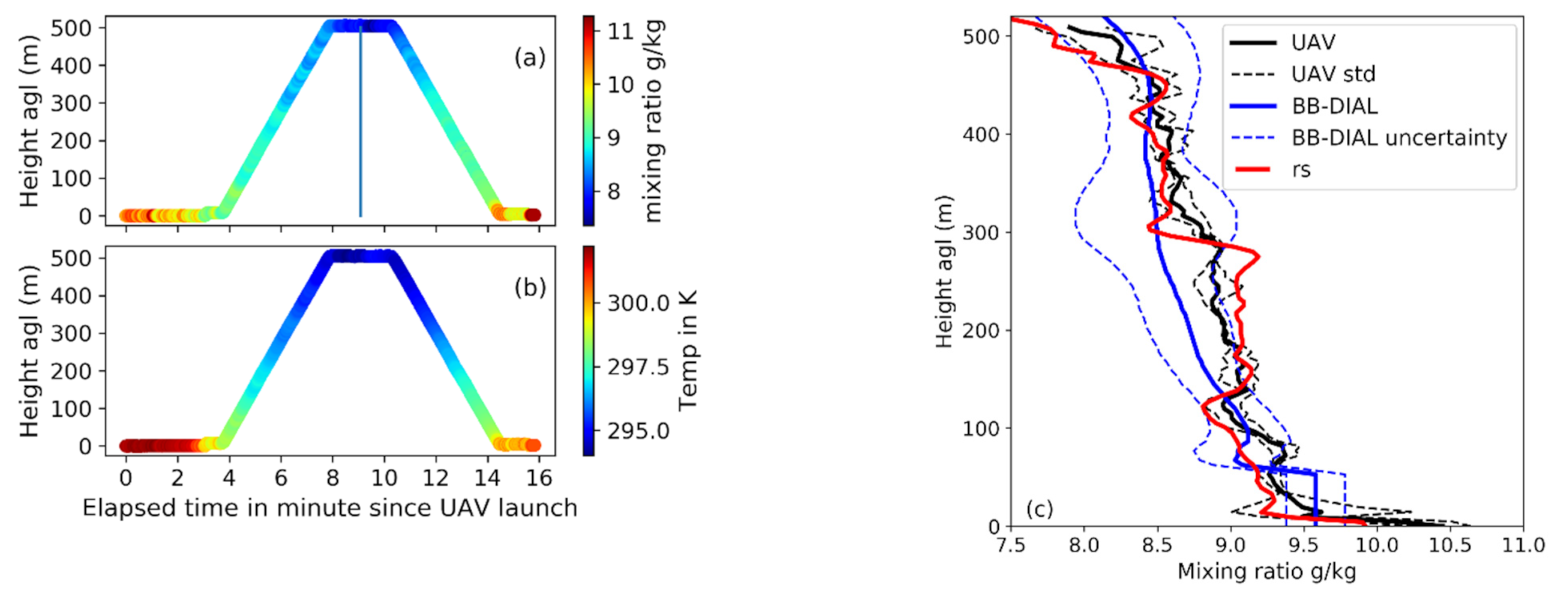
3.2.2. BB-DIAL versus UAV
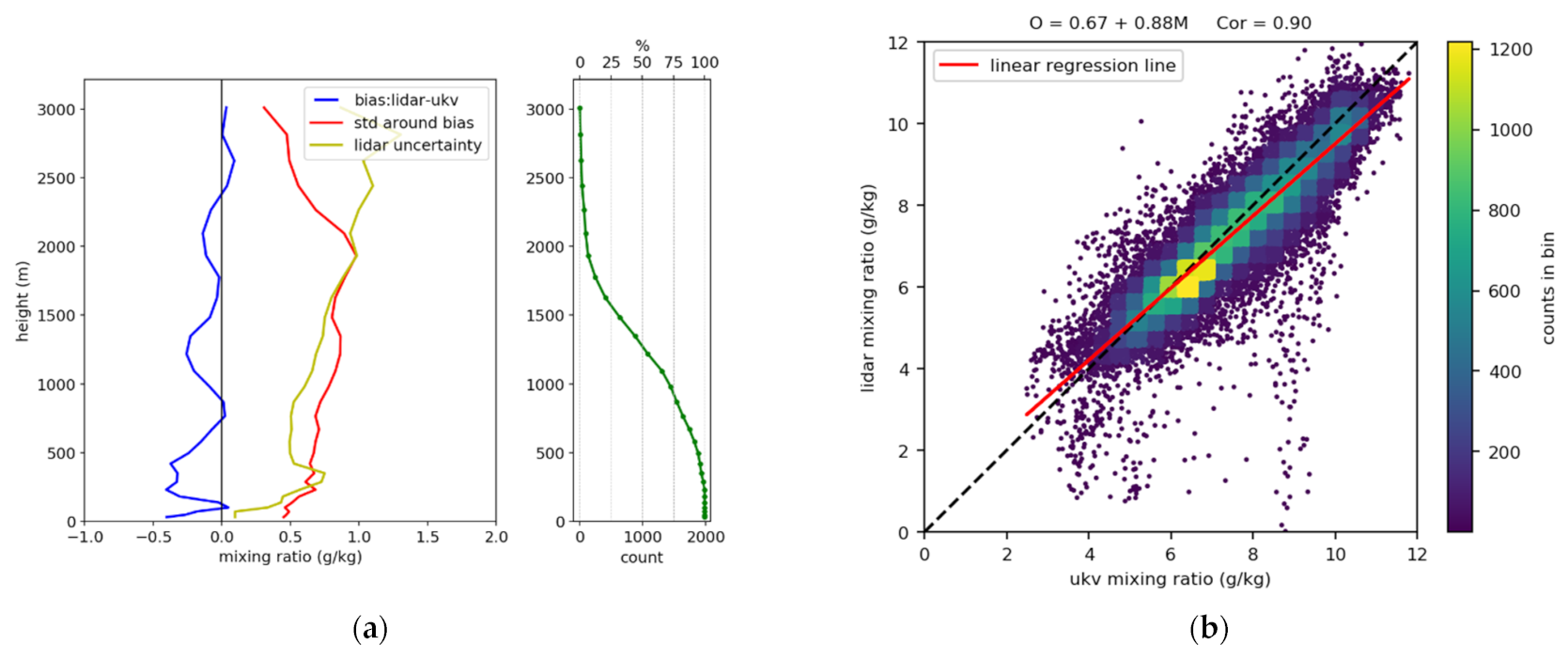
3.2.3. BB-DIAL versus UKV
3.2.4. Assessment of BB-DIAL and NWP Model versus Radiosondes
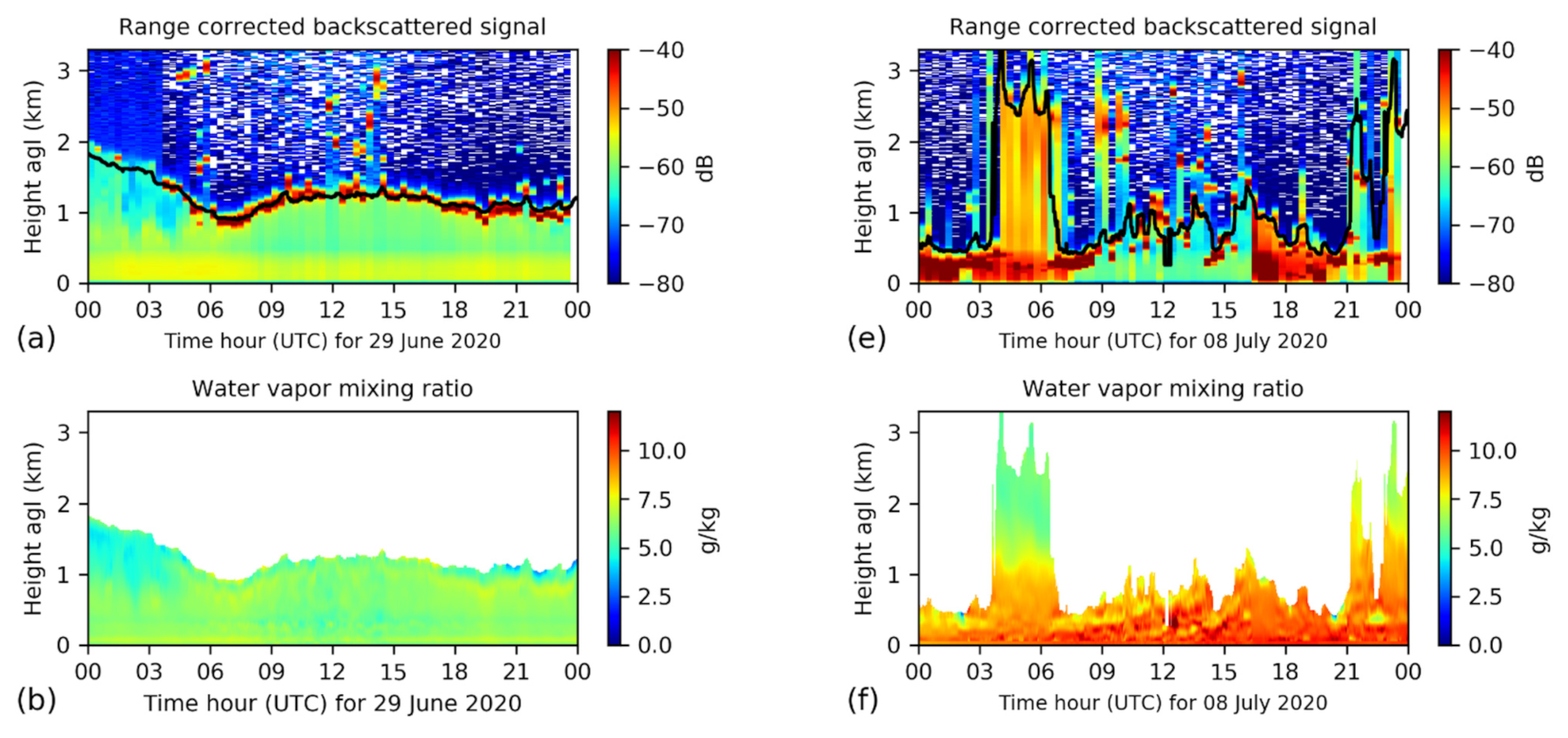
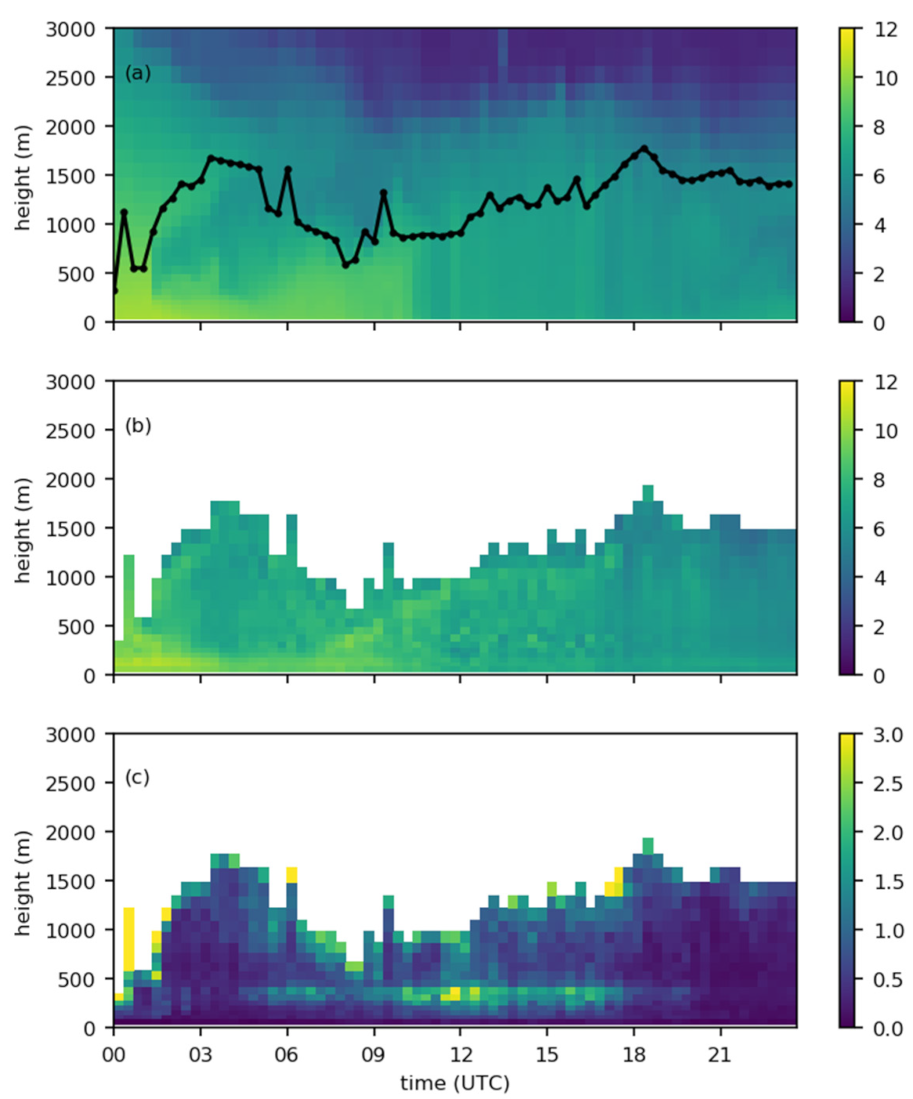
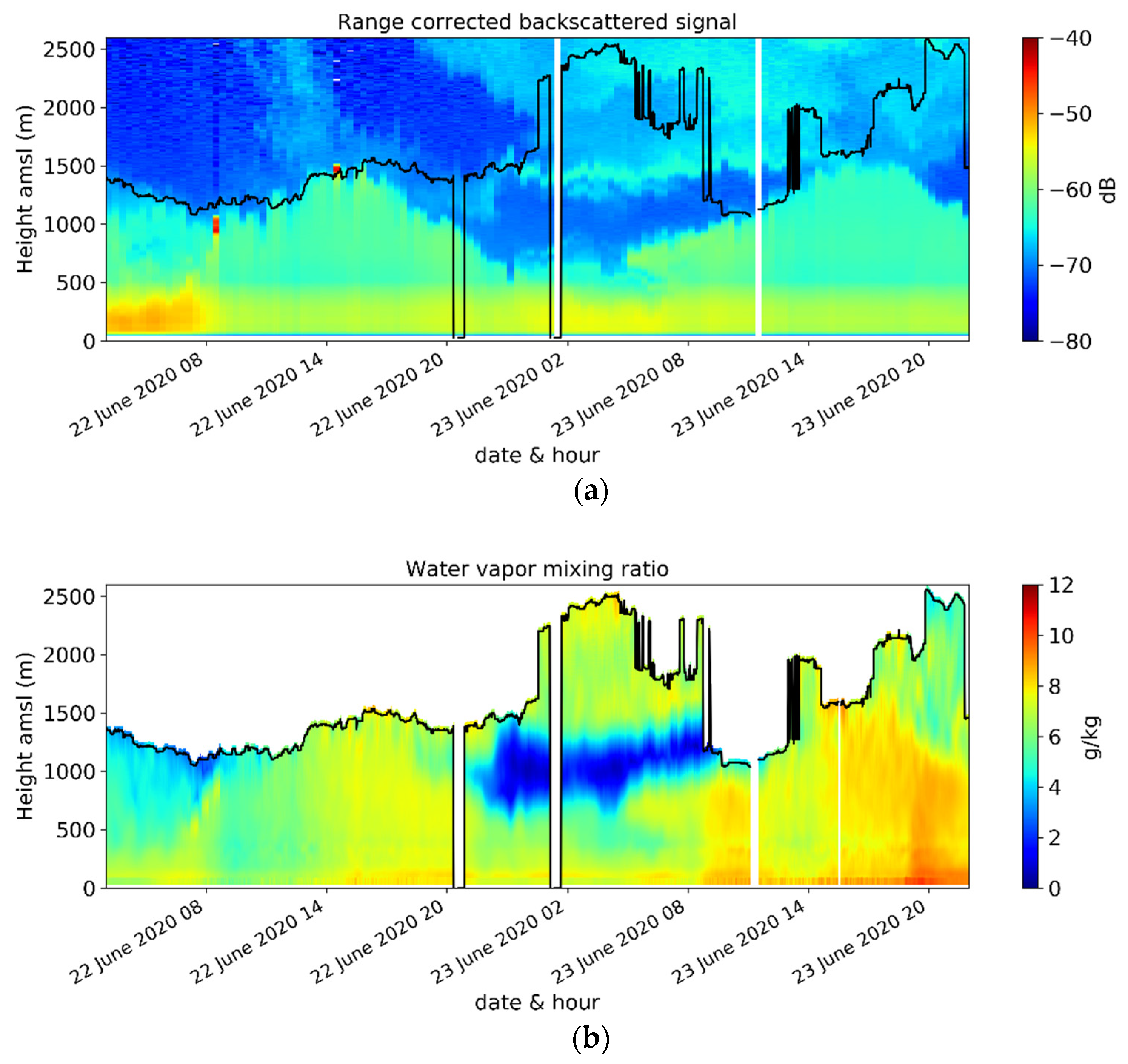
3.2.5. Capture of a Dry Layer in between Two More Moist Layers
4. BB-DIAL Data Quality Issues Identified
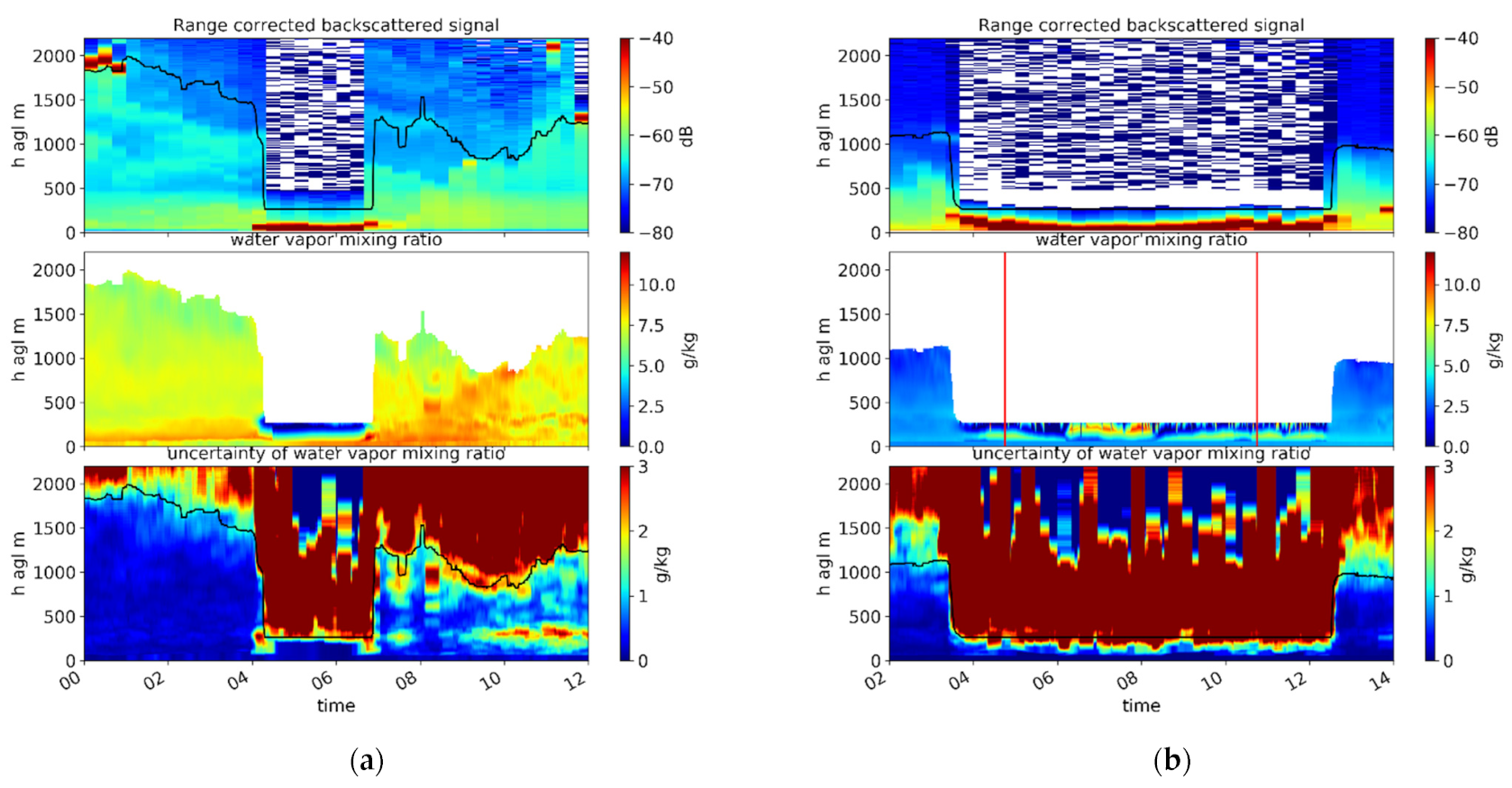
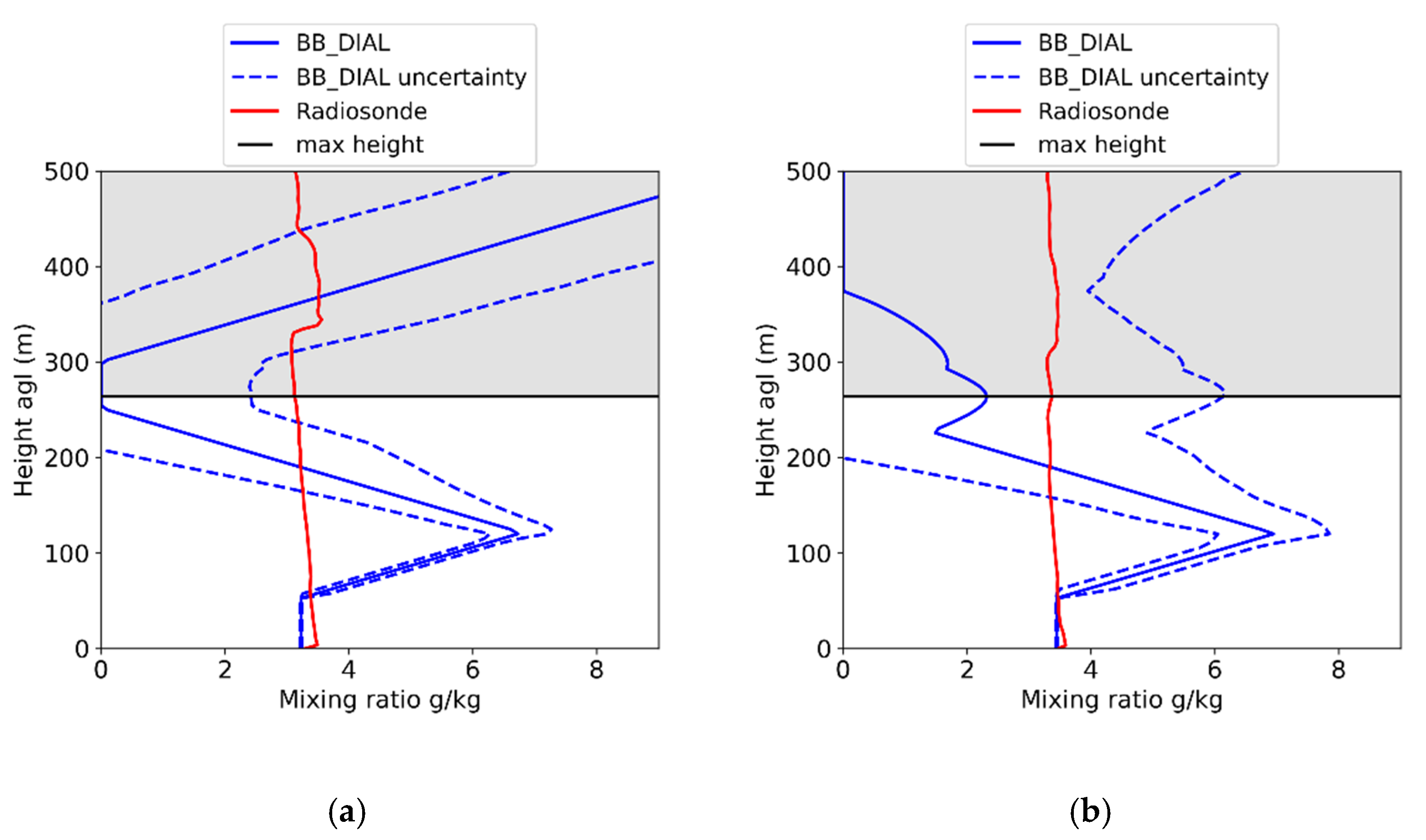
4.1. Lowest 500 m
4.2. Spurious Oscillations
5. Discussion and Concluding Remarks
- The BB-DIAL data below 500 m show a variable bias with altitude and a large error against the radiosonde, the UKV and the UAV. The method of blending data from the near and far field overlap region could be explored as a means of mitigating this problem.
- The mixing ratio profiles frequently show oscillations, in particular when there is little change in the mixing ratio in the vertical. Such oscillations are relatively well captured by an increase of the reported BB-DIAL uncertainty but are nevertheless spurious features. Increasing the signal to noise ratio is something that could be explored in order to reduce this oscillation.
- The very dry layer reported above fog or thick cloud is not realistic. Utilising an automatic gain control is a possible solution to this issue.
- It should be clear that the lowest 50 m are surface instrument measurements, rather than based on information from the BB-DIAL. This is something that should be flagged in the data to ensure correct interpretation of the information.
- The instrument reports measurements every 4.8 m and every minute for smoother presentation but in practise, the resolution is much coarser, actually very close to the UKV model resolution for the vertical. It would be usefully to get the real vertical resolution at least in the form of metadata information.
- Data are averaged every 20 min. With a 10-min time step the UKV 4D-Var assimilation would probably benefit from a shorter averaging period. There is clearly a trade-off between accuracy and capturing variability in rapidly evolving situations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stone, E.K.; Pearce, G. A Network of Mode-S Receivers for Routine Acquisition of Aircraft-Derived Meteorological Data. J. Atmos. Ocean. Technol. 2016, 33, 757–768. [Google Scholar] [CrossRef]
- De Haan, S. High-resolution wind and temperature observations from aircraft tracked by Mode-S air traffic control radar. J. Geophys. Res. Space Phys. 2011, 116, D10111. [Google Scholar] [CrossRef]
- Wulfmeyer, V.; Hardesty, R.M.; Turner, D.; Behrendt, A.; Cadeddu, M.P.; Di Girolamo, P.; Schlüssel, P.; Van Baelen, J.; Zus, F. A review of the remote sensing of lower tropospheric thermodynamic profiles and its indispensable role for the understanding and the simulation of water and energy cycles. Rev. Geophys. 2015, 53, 819–895. [Google Scholar] [CrossRef]
- Milan, M.; MacPherson, B.; Tubbs, R.; Dow, G.; Inverarity, G.; Mittermaier, M.; Halloran, G.; Kelly, G.; Li, D.; Maycock, A.; et al. Hourly 4D-Var in the Met Office UKV operational forecast model. Q. J. R. Meteorol. Soc. 2019, 146, 1281–1301. [Google Scholar] [CrossRef]
- Hoover, B.T.; Santek, D.A.; Daloz, A.-S.; Zhong, Y.; Dworak, R.; Petersen, R.A.; Collard, A. Forecast Impact of Assimilating Aircraft WVSS-II Water Vapor Mixing Ratio Observations in the Global Data Assimilation System (GDAS); UW SSEC Publication 16.02.H1/Project Report; University of Wisconsin: Wisconsin, MW, USA, 2016; 38p, Available online: http://library.ssec.wisc.edu/research_Resources/publications/pdfs/SSECPUBS/SSEC_Publication_No_16_02_H1.pdf (accessed on 20 October 2021).
- Petersen, R.A.; Cronce, L.; Mamrosh, R.; Baker, R.; Pauley, P. On the Impact and Future Benefits of AMDAR Observations in Operational Forecasting: Part II: Water Vapor Observations. Bull. Am. Meteorol. Soc. 2016, 97, 2117–2133. [Google Scholar] [CrossRef]
- Reen, B.P.; Dumais, R.E. Assimilation of Aircraft Observations in High-Resolution Mesoscale Modeling. Adv. Meteorol. 2018, 2018, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Bianco, L.; Friedrich, K.; Wilczak, J.M.; Hazen, D.; Wolfe, D.; Delgado, R.; Oncley, S.P.; Lundquist, J.K. Assessing the accuracy of microwave radiometers and radio acoustic sounding systems for wind energy applications. Atmos. Meas. Tech. 2017, 10, 1707–1721. [Google Scholar] [CrossRef] [Green Version]
- Leuenberger, D.; Haefele, A.; Omanovic, N.; Fengler, M.; Martucci, G.; Calpini, B.; Fuhrer, O.; Rossa, A. Improving High-Impact Numerical Weather Prediction with Lidar and Drone Observations. Bull. Am. Meteorol. Soc. 2020, 101, E1036–E1051. [Google Scholar] [CrossRef] [Green Version]
- Aviation Technique for Tracking Humidity through Aircraft Signals Wins Top European Award. 2019. Available online: https://www.meteorologicaltechnologyinternational.com/news/aviation/technique-for-tracking-humidity-through-aircraft-signals-wins-top-european-award.html (accessed on 20 October 2021).
- Brenot, H.; Rohm, W.; Kačmařík, M.; Möller, G.; Sá, A.; Tondaś, D.; Rapant, L.; Biondi, R.; Manning, T.; Champollion, C. Cross-Comparison and Methodological Improvement in GPS Tomography. Remote Sens. 2019, 12, 30. [Google Scholar] [CrossRef] [Green Version]
- Whiteman, D.N.; Demoz, B.; Rush, K.; Schwemmer, G.; Gentry, B.; Di Girolamo, P.; Comer, J.; Veselovskii, I.; Evans, K.; Melfi, S.H.; et al. Raman Lidar Measurements during the International H2O Project. Part I: Instrumentation and Analysis Techniques. J. Atmos. Ocean. Technol. 2006, 23, 157–169. [Google Scholar] [CrossRef] [Green Version]
- Chazette, P.; Marnas, F.; Totems, J. The mobile Water vapor Aerosol Raman LIdar and its implication in the framework of the HyMeX and ChArMEx programs: Application to a dust transport process. Atmos. Meas. Tech. 2014, 7, 1629–1647. [Google Scholar] [CrossRef] [Green Version]
- Flamant, C.; Chazette, P.; Caumont, O.; Di Girolamo, P.; Behrendt, A.; Sicard, M.; Totems, J.; Lange, D.; Fourrié, N.; Brousseau, P.; et al. A network of water vapor Raman lidars for improving heavy precipitation forecasting in southern France: Introducing the WaLiNeAs initiative. Bull. Atmos. Sci. Technol. 2021, 2, 1–21. [Google Scholar] [CrossRef]
- Spuler, S.M.; Hayman, M.; Stillwell, R.A.; Carnes, J.; Bernatsky, T.; Repasky, K.S. MicroPulse DIAL (MPD)–A diode-laser-based lidar architecture for quantitative atmospheric profiling. Atmos. Meas. Tech. 2021, 14, 4593–4616. [Google Scholar] [CrossRef]
- Newsom, R.K.; Turner, D.; Lehtinen, R.; Münkel, C.; Kallio, J.; Roininen, R. Evaluation of a Compact Broadband Differential Absorption Lidar for Routine Water Vapor Profiling in the Atmospheric Boundary Layer. J. Atmos. Ocean. Technol. 2020, 37, 47–65. [Google Scholar] [CrossRef]
- Mariani, Z.; Hicks-Jalali, S.; Strawbridge, K.; Gwozdecky, J.; Crawford, R.; Casati, B.; Lemay, F.; Lehtinen, R.; Tuominen, P. Evaluation of Arctic Water Vapor Profile Observations from a Differential Absorption Lidar. Remote Sens. 2021, 13, 551. [Google Scholar] [CrossRef]
- Yeung, W.L.; Chan, P.W.; Lehtinen, R.; Roininen, R.; Münkel, C.; Chiu, Y.Y. Observations of subtropical weather by a prototype water vapour LiDAR at Hong Kong Observatory. Weather 2020, 75, 244–251. [Google Scholar] [CrossRef]
- Roininen, R.; Münkel, C. Results from continuous atmospheric boundary layer humidity profiling with a compact BB-DIAL instrument. In Proceedings of the Eighth Symposium on Lidar Atmospheric Applications, Seattle, WA, USA, 26 January 2017; Available online: https://ams.confex.com/ams/97Annual/webprogram/Paper301717.html (accessed on 20 October 2021).
- Mariani, Z.; Stanton, N.; Whiteway, J.; Lehtinen, R. Toronto Water Vapor Lidar Inter-Comparison Campaign. Remote Sens. 2020, 12, 3165. [Google Scholar] [CrossRef]
- Rawlins, F.; Ballard, S.P.; Bovis, K.J.; Clayton, A.M.; Li, D.; Inverarity, G.W.; Lorenc, A.C.; Payne, T.J. The Met Office global four-dimensional variational data assimilation scheme. Q. J. R. Meteorol. Soc. 2007, 133, 347–362. [Google Scholar] [CrossRef]
- Rothman, L.S.; Gordon, I.E.; Barbe, A.; Benner, D.C.; Bernath, P.E.; Birk, M.; Boudon, V.; Brown, L.R.; Campargue, A.; Champion, J.P.; et al. The HITRAN 2008 molecular spectroscopic database. J. Quant. Spectrosc. Radiat. Transf. 2009, 110, 533–572. [Google Scholar] [CrossRef] [Green Version]
- HYT271 Sensor. Available online: https://www.ist-ag.com/sites/default/files/downloads/hyt271.pdf (accessed on 20 October 2021).
- NTC Type FP0. Available online: https://www.mouser.co.uk/pdfDocs/AAS-920-267D-Thermometrics-NTC-TypeFP07-041216-web.pdf (accessed on 20 October 2021).
- BMP 280. Available online: https://www.best-microcontroller-projects.com/support-files/bst-bmp280-ds001-18.pdf (accessed on 20 October 2021).
- World Meteorological Organization (WMO); Oakley, T.; Vömel, H.; Wei, L. Proceedings of the WMO Intercomparison of High-Quality Radiosonde Systems, Yangjiang, China, 12 July–3 August 2010; WMO: Geneva, Switzerland, 2011; Available online: https://library.wmo.int/index.php?lvl=notice_display&id=15531#.YL90v0zTWF5 (accessed on 20 October 2021).
- Sun, B.; Calbet, X.; Reale, A.; Schroeder, S.; Bali, M.; Smith, R.; Pettey, M. Accuracy of Vaisala RS41 and RS92 Upper Tropospheric Humidity Compared to Satellite Hyperspectral Infrared Measurements. Remote Sens. 2021, 13, 173. [Google Scholar] [CrossRef]
- Browning, K.A.; Blyth, A.M.; Clark, P.; Corsmeier, U.; Morcrette, C.; Agnew, J.L.; Ballard, S.P.; Bamber, D.; Barthlott, C.; Bennett, L.; et al. The Convective Storm Initiation Project. Bull. Am. Meteorol. Soc. 2007, 88, 1939–1956. [Google Scholar] [CrossRef]
- Desroziers, G.; Berre, L.; Chapnik, B.; Poli, P. Diagnosis of observation, background and analysis-error statistics in observation space. Q. J. R. Meteorol. Soc. 2005, 131, 3385–3396. [Google Scholar] [CrossRef]











| Specific Humidity | Uncer Tainty | Horizontal Resolution | Vertical Resolution | Obsvation Cycle | Timeliness | Coverage | |
|---|---|---|---|---|---|---|---|
| FT | goal | 2% | 2 km | 0.3 km | 15 min | 15 min | global |
| breakthrough | 5% | 10 km | 0.4 km | 60 min | 30 min | “ ” | |
| threshold | 10% | 30 km | 1 km | 6 h | 2 h | “ ” | |
| PBL | goal | 2% | 0.5 km | 0.1 km | 15 min | 15 min | “ ” |
| breakthrough | 5% | 5 km | 0.2 km | 60 min | 30 min | “ ” | |
| threshold | 10% | 20 km | 1 km | 6 h | 2 h | “ ” | |
| Bedfordshire | Maximum Temperature | Minimum Temperature | Mean Temperature | Rainfall | Sunshine | Rain Days (>1 mm) | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| °C | °C | °C | °C | °C | °C | mn | % | h | % | Days | Days | |
| Actual | 1981–2010 Anomaly | Actual | 1981–2010 Anomaly | Actual | 1981–2010 Anomaly | Actual | 1981–2010 Anomaly | Actual | 1981–2010 Anomaly | Actual | 1981–2010 Anomaly | |
| 20 June | 21 | 1.3 | 10.6 | 0.9 | 15.7 | 1.1 | 55.5 | 107 | 201.7 | 109 | 10.5 | 1.4 |
| 20 July | 21.7 | −0.7 | 11.9 | 0 | 16.8 | −0.3 | 59.7 | 119 | 177.8 | 89 | 9.9 | 1.4 |
| Number of Points | Correlation | Bias (BB-DIAL-Radiosonde) | RMS | Std Dev. |
|---|---|---|---|---|
| 23071 | 0.93 | 0.10 g/kg | 0.53 g/kg | 0.52 g/kg |
| No. of Points: 1454 | BB-DIAL-Radiosonde | UKV-Radiosonde | BB-DIAL-UKV |
|---|---|---|---|
| Correlation | 0.92 | 0.88 | 0.83 |
| Bias | −0.099 g/kg | +0.149 g/kg | −0.248 g/kg |
| RMS | 0.55 g/kg | 0.69 g/kg | 0.83 g/kg |
| Standard deviation | 0.54 g/kg | 0.67 g/kg | 0.79 g/kg |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaffard, C.; Li, Z.; Harrison, D.; Lehtinen, R.; Roininen, R. Evaluation of a Prototype Broadband Water-Vapour Profiling Differential Absorption Lidar at Cardington, UK. Atmosphere 2021, 12, 1521. https://doi.org/10.3390/atmos12111521
Gaffard C, Li Z, Harrison D, Lehtinen R, Roininen R. Evaluation of a Prototype Broadband Water-Vapour Profiling Differential Absorption Lidar at Cardington, UK. Atmosphere. 2021; 12(11):1521. https://doi.org/10.3390/atmos12111521
Chicago/Turabian StyleGaffard, Catherine, Zhihong Li, Dawn Harrison, Raisa Lehtinen, and Reijo Roininen. 2021. "Evaluation of a Prototype Broadband Water-Vapour Profiling Differential Absorption Lidar at Cardington, UK" Atmosphere 12, no. 11: 1521. https://doi.org/10.3390/atmos12111521
APA StyleGaffard, C., Li, Z., Harrison, D., Lehtinen, R., & Roininen, R. (2021). Evaluation of a Prototype Broadband Water-Vapour Profiling Differential Absorption Lidar at Cardington, UK. Atmosphere, 12(11), 1521. https://doi.org/10.3390/atmos12111521





