A Climatological Study of the Mechanisms Controlling the Seasonal Meridional Migration of the Atlantic Warm Pool in an OGCM
Abstract
:1. Introduction
2. Data and Methods
2.1. Data
2.1.1. Observations
2.1.2. Numerical Model
2.2. Methodology
2.2.1. Choice of the Meridional AWP Boundaries
2.2.2. AWP Migration Velocity Equation
3. Results and Discussion
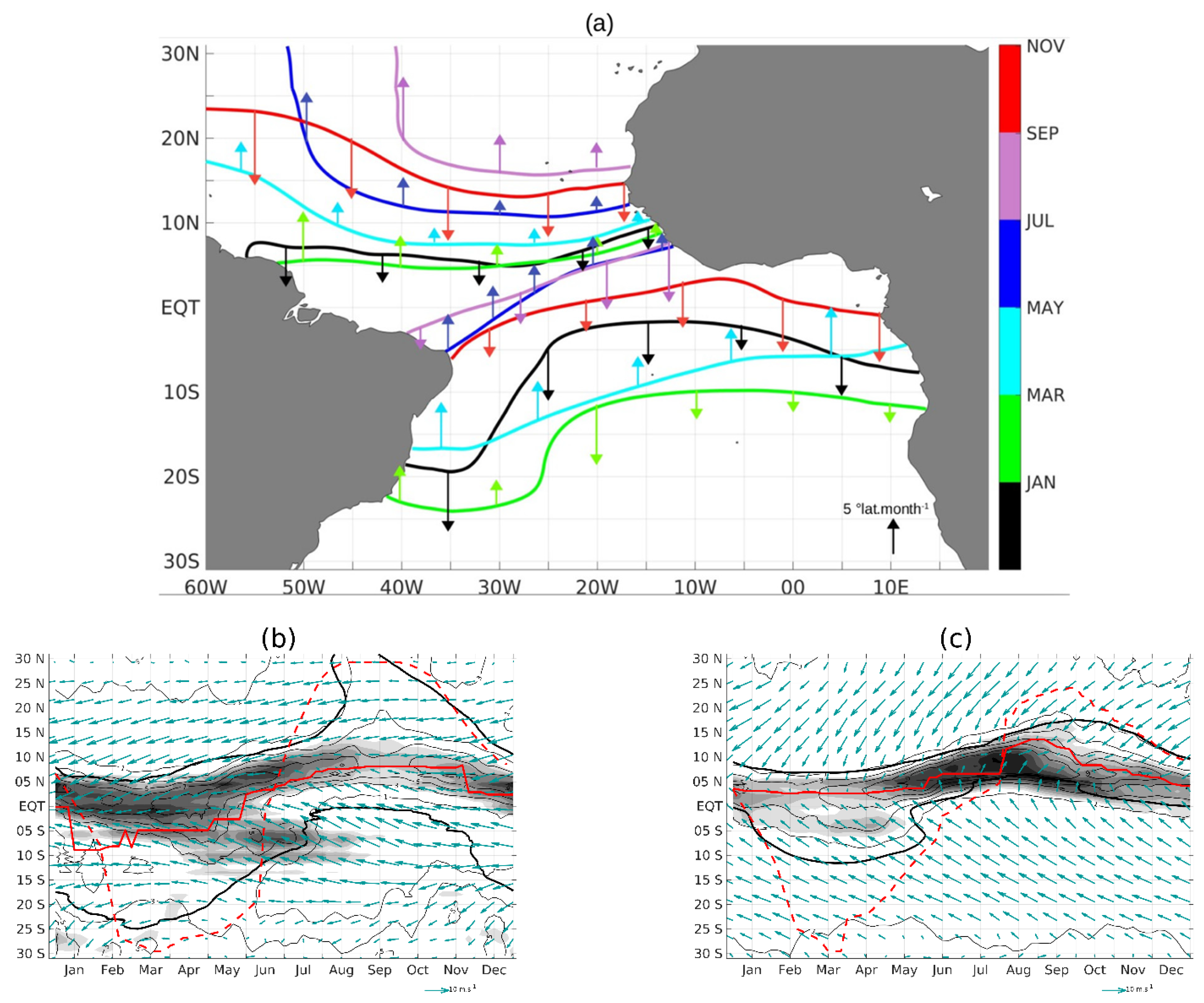
3.1. Description of the ITCZ and AWP Migration
3.2. Validation of the Meridional Migration of the AWP Boundaries Simulated by the OGCM and the Linearized Speed Equation
3.2.1. Validation of the AWP Migration Simulated by the OGCM
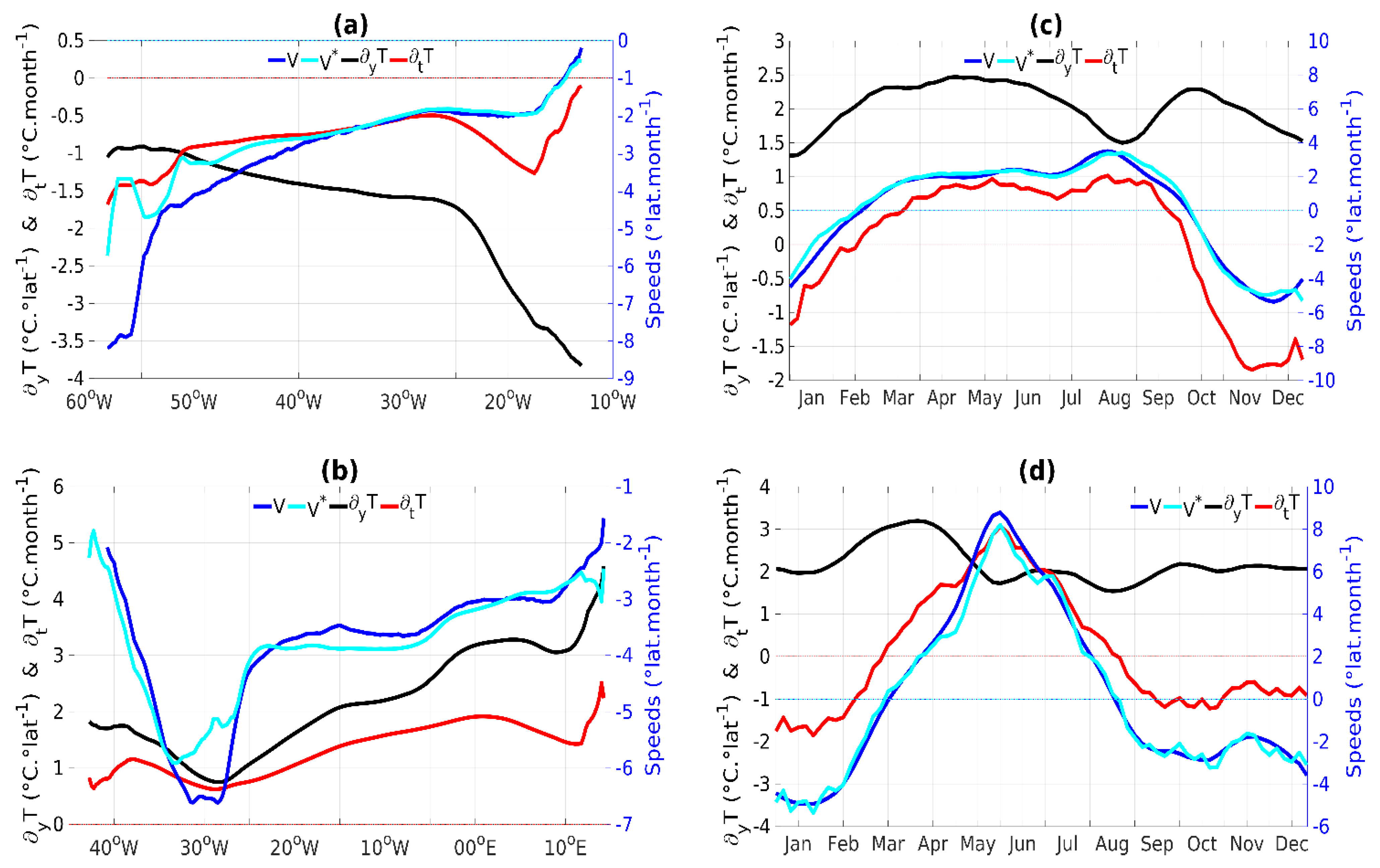
3.2.2. Validation of the Linearized Velocity Equation
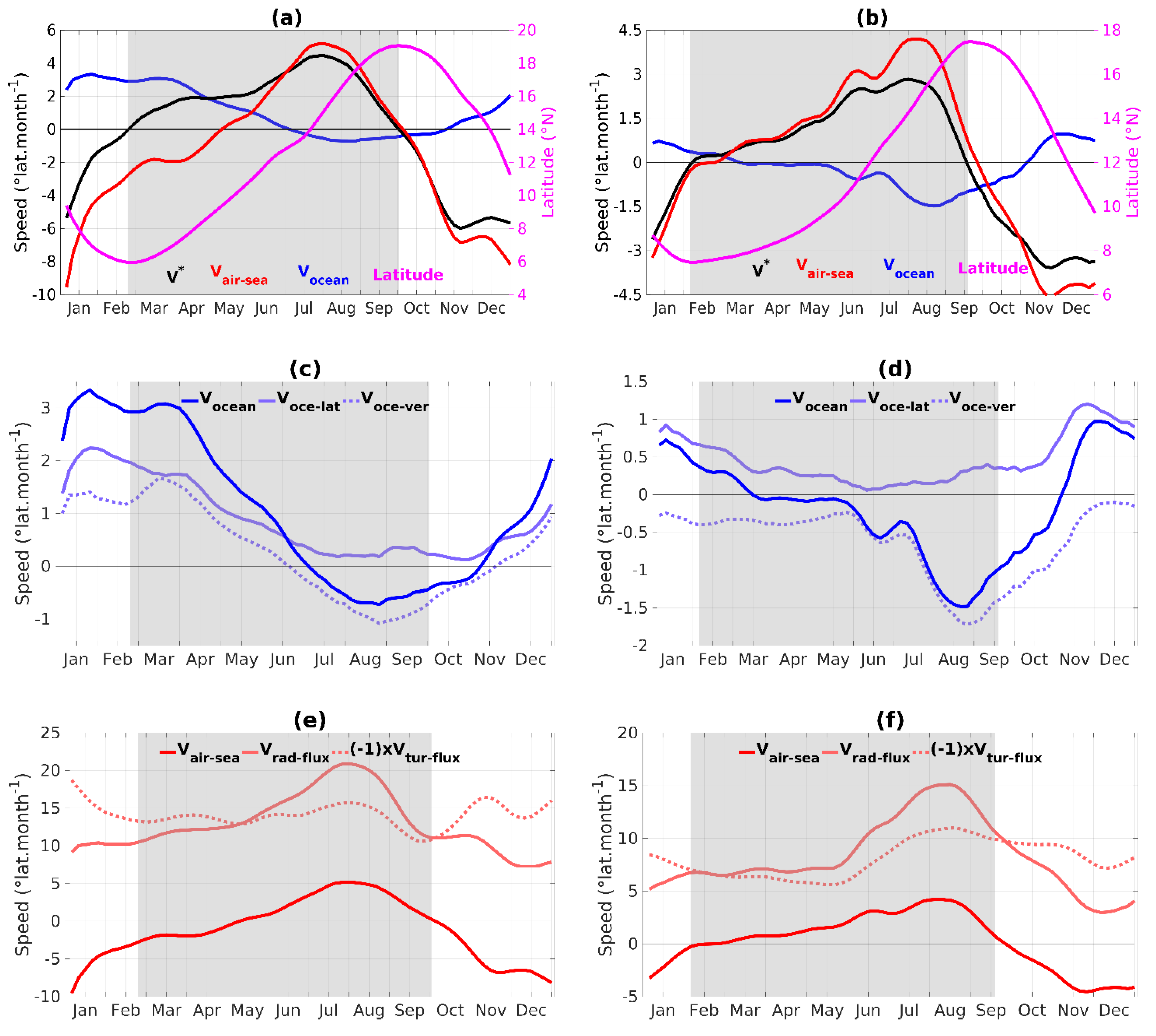
3.3. Processes Controlling the AWP Meridional Migration Speed
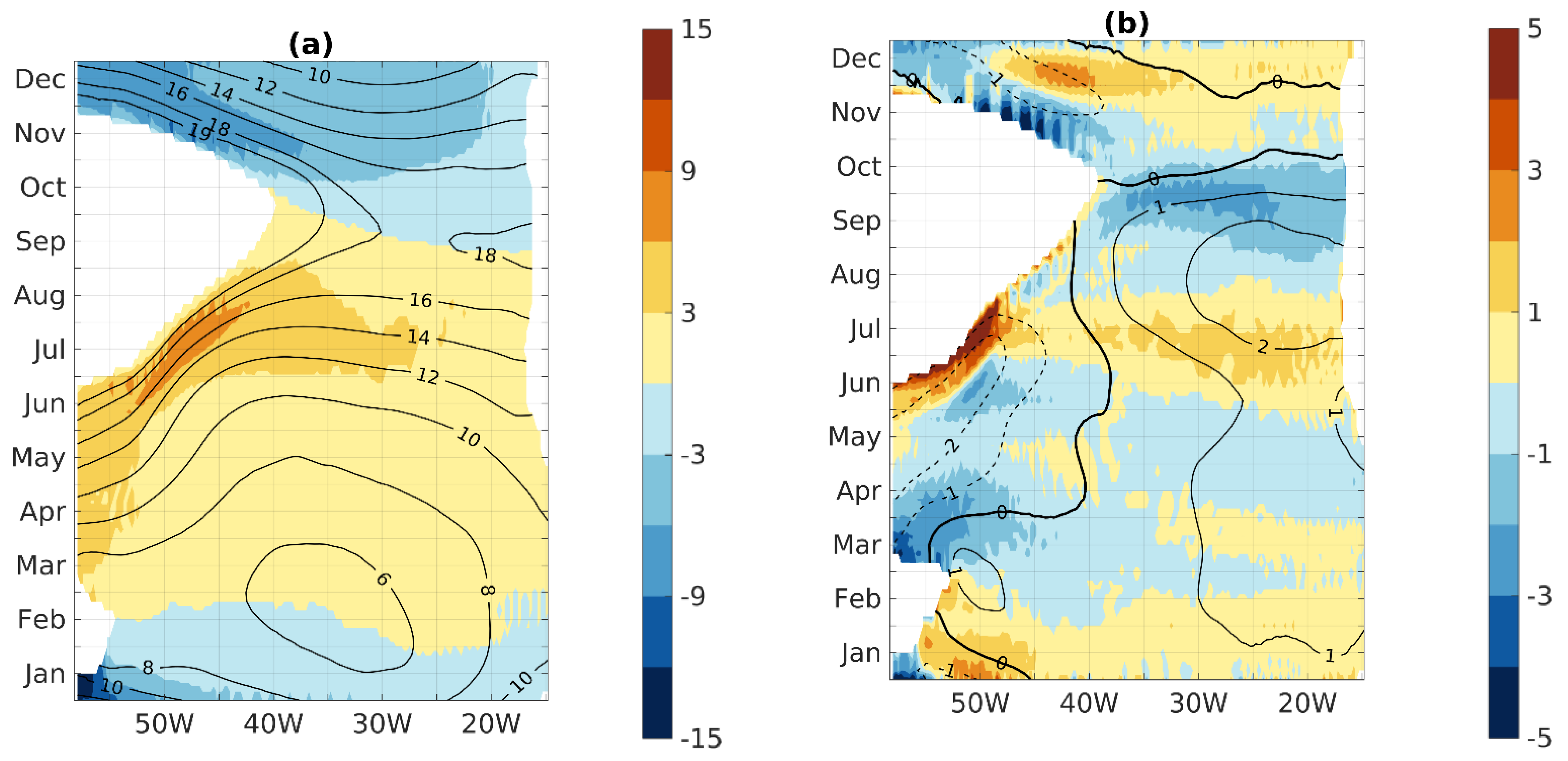
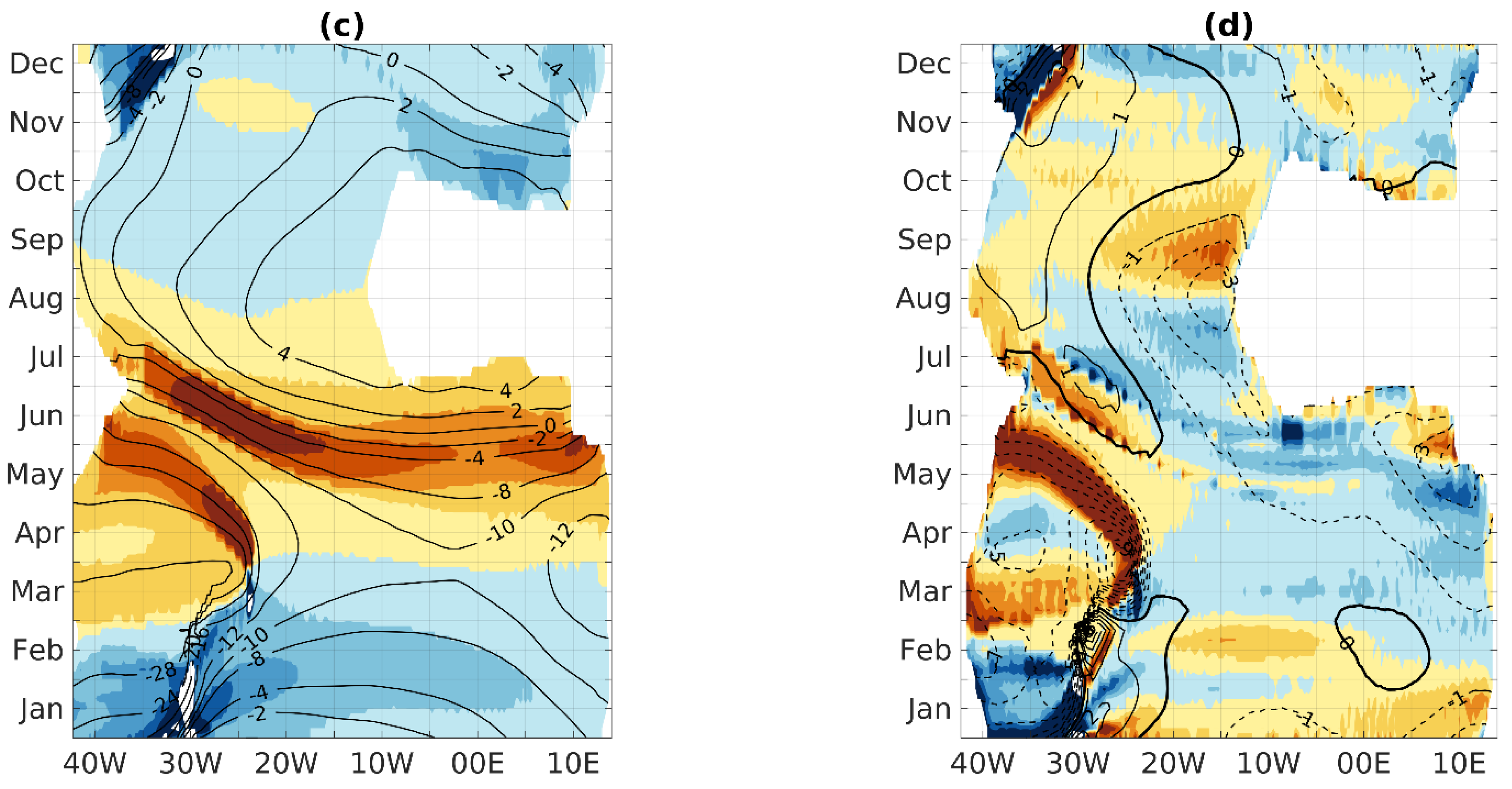
3.3.1. Respective Role of the Meridional SST Gradients and the SST Time-Derivative
3.3.2. Role of Heat Fluxes in the Mixed Layer
Seasonal Migration of the Northern Boundary
Seasonal Migration of the Southern Boundary
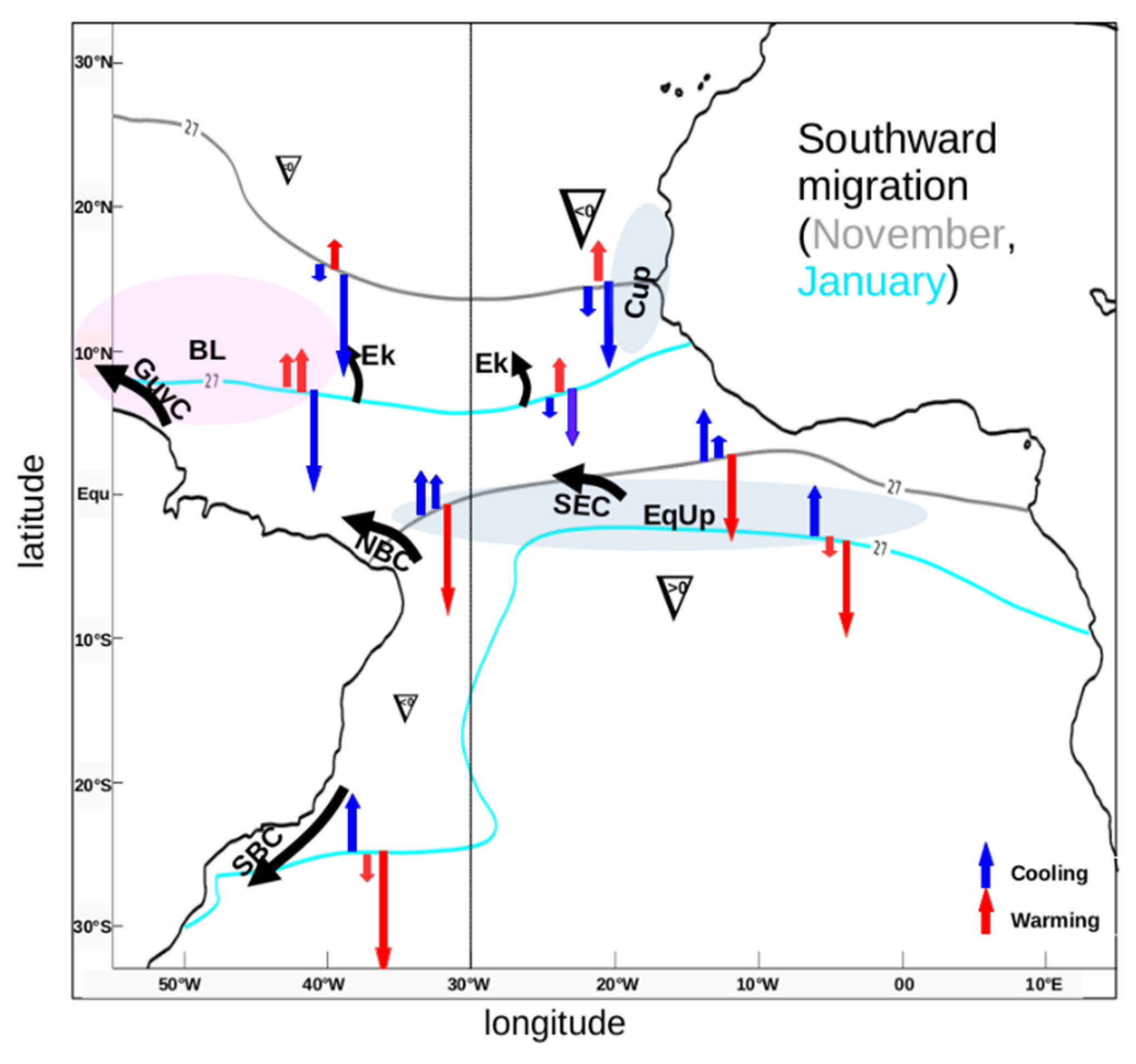
4. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ninomiya, K. Similarity and Difference between the South Atlantic Convergence Zone and the Baiu Frontal Zone Simulated by an AGCM. J. Meteo. Soc. Jpn. 2007, 85, 277–299. [Google Scholar] [CrossRef] [Green Version]
- Villela, R.J. The south atlantic convergence zone: A critical view and overview. Rev. Do Inst. Geológico São Paulo 2017, 38, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Schneider, T.; Bischoff, T.; Haug, G.H. Migrations and dynamics of the inertropical convergence zone. Nature 2014, 513. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.T.; Xie, X. Double intertropical convergence zones—A new look using scatterometer. Geophys. Res. Lett. 2002, 29, 2072. [Google Scholar] [CrossRef] [Green Version]
- Tomaziello, A.C.N.; Carvalho, L.M.V.; Gandu, A.W. Intraseasonal variability of the Atlantic Intertropical Convergence Zone during austral summer and winter. Clim. Dyn. 2016. [Google Scholar] [CrossRef]
- Yu, L.; Jin, X.; Weller, R.A. Role of Net Surface Heat Flux in Seasonal Variations of Sea Surface Temperature in the Tro Atlantic Ocean. J. Clim. 2006, 19, 6153–6169. [Google Scholar] [CrossRef]
- Fasullo, J.; Webster, P.J. Warm Pool SST Variability in Relation to the Surface Energy Balance. J. Clim. 1998, 12, 1292–1305. [Google Scholar] [CrossRef]
- Rajendran, K.; Gadgil, S.; Surendran, S. Monsoon season local control on precipitation over warm tropical oceans. Meterol. Atmos. Phys. 2019, 131, 1451–1465. [Google Scholar] [CrossRef]
- Graham, N.E.; Barnett, T.P. Sea surface Temperature, Surface Wind Divergence, and Convection over Tropical Oceans. Report 30 October 1987. Available online: http://www.sciencemag.org/ (accessed on 28 November 2014).
- He, J.; Johnson, N.C.; Vecchi, G.A.; Kirtman, B.; Wittenberg, A.T.; Sturm, S. Precipitation Sensitivity to Local Variations in Tropical Sea Surface Temperature. J. Clim. 2018, 31, 9225–9238. [Google Scholar] [CrossRef] [Green Version]
- Oueslati, B.; Bellon, G. The double ITCZ bias in CMIP5 models: Interaction between SST, large-scale circulation and precipitation. Clim Dyn. 2015, 44, 585–607. [Google Scholar] [CrossRef]
- Waliser, D.E.; Graham, N.E. Convective Cloud Systems and Warm-Pool Sea Surface Temperatures: Coupled Interactions and Self-Regulation. J. Geophys. Res. 1993, 98, 12881–12893. [Google Scholar] [CrossRef]
- Okumura, Y.; Xie, S.P. Interaction of the Atlantic Equatorial Cold Tongue and the African Monsoon. J. Clim. 2004, 17, 3589–3602. [Google Scholar] [CrossRef] [Green Version]
- Lin, B.; Wielicki, B.A.; Minnis, P.; Lin, C.; Xu, K.M.; Hu, Y. The effect of Environmental Conditions on Tropical Deep Convective Systems Observed from the TRMM Satellite. J. Clim. 2006, 19, 5745–5761. [Google Scholar] [CrossRef] [Green Version]
- Xie, S.P.; Carton, J.A. Tropical Atlantic Variability: Patterns, Mechanisms, and Impacts. In Geophysical Monograph; AGU: Washington, DC, USA, 2004. [Google Scholar]
- Cintra, M.M.; Lentini, C.A.D.; Servain, J.; Araujo, M.; Marone, E. Physical processes that drive the seasonal evolution of the Southwestern Tropical Atlantic Warm Pool. Dyn. Atmos. 2015, 72, 1–11. [Google Scholar] [CrossRef]
- Kim, S.T.; Yu, J.-Y.; Lu, M.-M. The distinct behaviors of Pacific and Indian Ocean warm pool properties on seasonal and interannual time scales. J. Geophys. Res. 2012, 117, D05128. [Google Scholar] [CrossRef] [Green Version]
- Shi, W.; Xiao, Z.; AI, Y. The behavior of deep convective clouds over the warm pool and connection to the Walker circulation. Sci. China Earth Sci. 2018, 61, 1605–1621. [Google Scholar] [CrossRef]
- Wang, C.; Enfield, D.B. The tropical Western Hemisphere warm pool. Geophys. Res. Lett. 2001, 28, 1635–1638. [Google Scholar] [CrossRef]
- Gill, A.E. Some simple solutions for heat-induced tropical circulation. Quart. J. Met. Soc. 1980, 106, 447–462. [Google Scholar] [CrossRef]
- Biasutti, M.; Battisti, D.S.; Sarachick, E.S. Mechanisms Controlling the Annual Cycle of Precipitation in the Tropical Atlantic Sector in an Atmospheric GCM. J. Clim. 2004, 17, 4708–4723. [Google Scholar] [CrossRef] [Green Version]
- Back, L.E.; Bretherthon, C.S. On the Relationship between SST Gradients, Boundary Layer Winds, and Convergence over Tropical Oceans. J. Clim. 2009, 22, 4182–4196. [Google Scholar] [CrossRef]
- Diakhaté, M.; Lazar, A.; de Coëtlogon, G.; Gaye, A.T. Do SST gradients drive the monthly climatological surface wind convergence over the tropical Atlantic? Int. J. Climatol. 2018. [Google Scholar] [CrossRef]
- Stevens, B.; Duan, J.J.; Mc Williams, J.C.; Münnich, M.; Neelin, D. Entrainment, Rayleigh friction, and boundary layer winds over tropical Pacific. J. Clim. 2002, 648, 30–44. [Google Scholar] [CrossRef]
- Waliser, D.E.; Jiang, X. Intertropical Convergence Zone. Atmos. Sci. 2015, 6, 121–131. [Google Scholar] [CrossRef]
- Giannini, A.; Saravanan, R.; Chang, P. Dynamics of the boreal summer African monsoon in the NSIPP1 atmospheric model. Clim. Dyn. 2005, 25, 517–535. [Google Scholar] [CrossRef]
- Sultan, B.; Janicot, S. Abrupt shift of the ITCZ over West Africa and intra-seasonal variability. Geophys. Res. Lett. 2000, 27, 3353–3356. [Google Scholar] [CrossRef]
- Sultan, B.; Janicot, S. The West African Monsoon Dynamics. Part II: The « Preonset » and « Onset » of the Summer Monsoon. J. Clim. 2003, 16, 3407–3427. [Google Scholar] [CrossRef]
- Hastenrath, S. Circulation and teleconnection mechanisms of Northeast Brazil droughts. Prog. Oceanogr. 2006, 407–415. [Google Scholar] [CrossRef]
- Hounsou-gbo, G.A.; Araujo, M.; Bourlès, B.; Veleda, D.; Servain, J. Tropical Atlantic Contributions to Strong Rainfall Variability Along the Northeast Brazilian Coast. Adv. Meteorol. 2015. [Google Scholar] [CrossRef] [Green Version]
- Nobre, P.; Shukla, J. Variations of Sea Surface Temperature, Wind Stress, and Rainfall over the Tropical Atlantic and South America. J. Clim. 1996, 9, 2464–2479. [Google Scholar] [CrossRef]
- Donders, T.H.; de Boer, H.J.; Finsinger, W.; Grimm, E.C.; Dekker, S.C.; Reichart, G.J.; Wagner-Cremer, F. Impact of the Atlantic Warm Pool on precipitation and temperature in Florida during North Atlantic cold spells. Clim. Dyn. 2011, 36, 109–118. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Wang, C.; Lee, S.K.; Enfield, D. Inhomogeneous influence of the Atlantic warm pool on United States precipitation. Atmos. Sci. Let. 2015, 16, 63–69. [Google Scholar] [CrossRef]
- Misra, V.; Groenen, D.; Bhardwaj, A.; Mishra, A. The warm pool variability of the tropical northeast Pacific. Int. J. Climatol. 2016, 36, 4625–4637. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, L.; Lee, S.K. Response of Freshwater Flux and Sea Surface Salinity to Variability of the Atlantic Warm Pool. J. Clim. 2013, 26, 1249–1267. [Google Scholar] [CrossRef]
- Wang, C.; Enfield, D.B.; Lee, S.K.; Landsea, C.W. Influences of the Atlantic Warm Pool on Western Hemisphere Summer Rainfall and Atlantic Hurricanes. J. Clim. 2006, 19, 3011–3028. [Google Scholar] [CrossRef]
- Philander, S.G.H.; Gu, D.; Halpern, D.; Lambert, G.; Lau, N.C.; Li, T.; Pacanowski, R.C. Why the ITCZ Is Mostly North of the Equator. J. Clim. 1996, 9, 2958–2972. [Google Scholar] [CrossRef] [Green Version]
- Frierson, D.M.W.; Hwang, Y.T. Extratropical Influence on ITCZ Shifts in Slab Ocean Simulations of Global Warming. J. Clim. 2012, 25, 720–733. [Google Scholar] [CrossRef] [Green Version]
- Kang, S.; Frierson, D.M.; Held, I.M. The Tropical Response to Extratropical Thermal Forcing in an Idealized GCM: The Importance of Radiative Feedbacks and Convective Parameterization. J. Atmos. Sci. 2009. [Google Scholar] [CrossRef] [Green Version]
- Clement, A.C.; Seager, R.; Murtugudde, R. Why Are There Tropical Warm Pools? J. Clim. 2005, 18, 5294–5311. [Google Scholar] [CrossRef]
- Jouanno, J.; Marin, F.; Penhoat, Y.; Sheinbaum, J.; Molines, J.M. Seasonal heat balance in the upper 100 m of the equatorial Atlantic Ocean. J. Ceophys. Res. 2011, 116, C09003. [Google Scholar] [CrossRef] [Green Version]
- Peter, A.C.; Le Hénaff, M.; du Penhoat, Y.; Menkes, C.E.; Marin, F.; Vialard, J.; Caniaux, G.; Lazar, A. A model study of the seasonal mixed layer heat budget in the equatorial Atlantic. J. Geophys. Res. 2006, 111, C0014. [Google Scholar] [CrossRef] [Green Version]
- Misra, V.; Stroman, A.; DiNapoli, S. The rendition of the Atlantic Warm Pool in the reanalyses. Clim. Dyn. 2013, 41, 517–532. [Google Scholar] [CrossRef]
- Wade, M.; Caniaux, G.; du Penhoat, Y.; Dengler, M.; Giordani, H.; Hummels, R. A one-dimensional modeling study of the diurnal cycle in the equatorial Atlantic at the PIRATA buoys during the EGEEE-3 campaign. Oce. Dyn. 2010, 61, 1–20. [Google Scholar] [CrossRef]
- Huffman, G.J.; Bolvin, D.T. Version 1.2 GPCP One-Degree Daily Precipitation Data Set Documentation. NASA Goddard Space Flight Cent. Rep. 2013, 27. Available online: hftp://meso.gsfc.nasa.gov/pub/1dd-v1.2/1DD_v1.2_doc.pdf (accessed on 28 November 2014).
- Reynolds, R.W.; Smith, T.M.; Liu, C.; Chelton, D.B.; Casey, K.S.; Schlax, M.G. Daily High-Resolution-Blended Analyses for Sea Surface Temperature. J. Clim. 2007, 20, 5473–5496. [Google Scholar] [CrossRef]
- Praveen Kumar, B.; Vialard, J.; Lengaigne, M.; Murty, V.S.N.; McPhaden, M.J. TropFlux: Air-sea fluxes for the global tropical oceans-description and evaluation. Clim. Dyn. 2012, 38, 1521–1543. [Google Scholar] [CrossRef]
- Brodeau, L.; Barnier, B.; Tréguier, A.; Penduff, T.; Gulev, S. An ERA40-based atmospheric forcing for global ocean circulations models. Ocean. Model. 2010, 31, 88–104. [Google Scholar] [CrossRef] [Green Version]
- Madec, G. NEMO Ocean Engine. In Note Pôle Modèle; Inst. Pierre-Simon Laplace: Paris, France, 2008; Volume 77. [Google Scholar]
- Roullet, G.; Madec, G. Salt conservation, free surface, and varying levels: A new formulation for ocean general circulation models. J. Geophys. Res. 2000, 105, 23927–23942. [Google Scholar] [CrossRef]
- Faye, S.; Lazar, A.; Sow, B.A.; Gaye, A.T. A model study of the seasonality of sea surface temperature and circulation in the Atlantic North-eastern Tropical Upwelling System. Front. Phys. 2015, 3, 76. [Google Scholar] [CrossRef] [Green Version]
- Molines, J.M.; Barnier, B.; Penduff, T.; Brodeau, L.; Treguier, A.M.; Theetten, S.; Madec, G. Definition of the Interannual Experiment Orca025-g70, 1958 2004. Technical Report; Laboratire des Ecoulements Geophysiques et Industriels, CNRS UMR 5519: Grenoble, France, 2007. [Google Scholar]
- Large, W.G.; Yeager, S.G. Diurnal to Decadal Global Forcing For Ocean and Sea-Ice Models: The Data Sets and Flux Climatologies. Natl. Cent. Atmos. Res. 2004, 11, 324–336. [Google Scholar]
- Zhang, Y.; Rossow, W.B.; Lacis, A.A.; Oinas, V.; Mishchenko, M.I. Calculation of radiative fluxes from the surface to top of atmosphere based on ISCCP and other global data sets: Refinements of the radiative transfer model and the input data. J. Geophys. Res. 2004, 109, D19105. [Google Scholar] [CrossRef] [Green Version]
- Vialard, J.; Menkes, C.; Boulanger, J.P.; Delecluse, P.; Guilyardi, M.; McPhaden, M.J.; Madec, G. A Model Study of Oceanic Mechanisms Affecting Equatorial Pacific Sea Surface Temperature during the 1997-98 El Nino. J. Phys. Oce. 2001, 31, 1649–1675. [Google Scholar] [CrossRef] [Green Version]
- Carella, G.; Morris, A.K.R.; Pascal, R.W.; Yelland, M.J.; Berry, D.I.; Morak-Bozzo, S.; Merchant, C.J.; Kent, E.C. Measurements and models of the temperature change of water samples in sea-surface temperature buckets. Q. J. R. Meteorol. Soc. 2017, 143, 2198–2209. [Google Scholar] [CrossRef] [Green Version]
- Cayan, D.R. Latent and Sensible Heat Flux Anomalies over the Northern Oceans: Driving the Sea Surface Temperature. J. Phys. Oceanogr. 1992, 22, 859–881. [Google Scholar] [CrossRef]
- De Boyer Montégut, C.; Vialard, J.; Shenoi, S.S.; Shankar, D.; Durand, F.; Ethé, C.; Madec, G. Simulated Seasonal and Interannual Variability of the Mixed Layer Heat Budget in the Northern Indian Ocean. J. Clim. 2007, 20, 3249–3268. [Google Scholar] [CrossRef]
- Doi, T.; Vecchi, G.A.; Rosati, A.J.; Delworth, T.L. Biases in the Atlantic ITCZ in Seasonal-Interannual Variations for a Coarse and a High-Resolution Coupled Climate Model. J. Clim. 2012, 25, 5494–5511. [Google Scholar] [CrossRef]
- Carton, J.A.; Cao, X.; Giese, B.S.; da Silva, A.M. Decadal and interannual SST variability in the tropical Atlantic Ocean. J. Phys. Oceanogr. 1996, 26, 1165–1175. [Google Scholar] [CrossRef] [Green Version]
- Richter, I.; Xie, S.P.; Morioka, Y.; Doi, T.; Taguchi, B.; Behera, S. Phase locking of equatorial Atlantic variability through the seasonal migration of the ITCZ. Clim. Dyn. 2016, 48, 3615–3629. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wane, D.; Lazar, A.; Wade, M.; Gaye, A.T. A Climatological Study of the Mechanisms Controlling the Seasonal Meridional Migration of the Atlantic Warm Pool in an OGCM. Atmosphere 2021, 12, 1224. https://doi.org/10.3390/atmos12091224
Wane D, Lazar A, Wade M, Gaye AT. A Climatological Study of the Mechanisms Controlling the Seasonal Meridional Migration of the Atlantic Warm Pool in an OGCM. Atmosphere. 2021; 12(9):1224. https://doi.org/10.3390/atmos12091224
Chicago/Turabian StyleWane, Dahirou, Alban Lazar, Malick Wade, and Amadou Thierno Gaye. 2021. "A Climatological Study of the Mechanisms Controlling the Seasonal Meridional Migration of the Atlantic Warm Pool in an OGCM" Atmosphere 12, no. 9: 1224. https://doi.org/10.3390/atmos12091224
APA StyleWane, D., Lazar, A., Wade, M., & Gaye, A. T. (2021). A Climatological Study of the Mechanisms Controlling the Seasonal Meridional Migration of the Atlantic Warm Pool in an OGCM. Atmosphere, 12(9), 1224. https://doi.org/10.3390/atmos12091224






