1. Introduction
Cities are areas of highly concentrated human activities that induce large amounts of air pollutants emitted by anthropic activities, such as traffic, industry and residential activities. Cities are also heterogeneous environments, and the presence of high buildings represents obstacles that reduce air flow inside streets and limit the dispersion of pollutants emitted within [
1,
2,
3,
4]. These two processes lead to poor air quality, thus, imposing a risk for human health. In addition, the growing number of people living in cities increases the vulnerability, and massive urbanization has negative consequences on the environment and human health especially during pollution peaks [
5,
6,
7,
8,
9]. In the case of a street canyon, air recirculates inside the canyon, as shown by Harman et al. [
10] for the wind perpendicular to the street.
Pollutants emitted in the street—by traffic for example—tend to accumulate on the leeward side of the street, and the concentrations at this location may be much higher than the background concentrations [
11,
12,
13]. Knowing that people spend most of their time indoors, the vertical distribution of pollutant concentrations is also a key research topic. People are not only exposed to air pollution at street pedestrian level but also to air pollution inside buildings, which may be influenced by outdoor pollution and the height levels of the building [
14,
15,
16].
Models are powerful tools to study air flow and pollutant concentration because the conditions can be fixed and the simulations are easily replicable, thus, allowing the analysis of different processes influencing the concentrations. Currently, chemistry-transport models (CTMs) are widely used at the meso-scale to understand processes, interpret observations and forecast the evolution of pollutant concentrations [
17,
18,
19,
20]. Informed by emissions inventories, meteorological, initial and boundary conditions, these models use numerical techniques to simulate pollutant transport, chemical transformation in the atmosphere and compute air concentrations and deposition fluxes.
In CTMs, concentrations are averaged over mesh cells with a horizontal resolution ranging from kilometers to hundreds of kilometers. Due to their coarse spatial resolution, CTMs are not able to capture the high pollutant concentrations observed in streets, and thus city-scale models, such as simplified street-network or street-in-grid models are used to represent the street level concentrations [
21,
22,
23,
24,
25,
26].
Street models use parametrizations to represent the dispersion of pollutants at the street level over a neighborhood or a city. The streets are not discretized finely; however, concentrations are assumed to be homogeneous in each street segment, as in the Model of Urban Network of Intersecting Canyons and Highways (MUNICH) [
24,
25], or in part of the street (lee side versus wind side, for example, as in the OSPM (Operational Street Pollution Model) [
21].
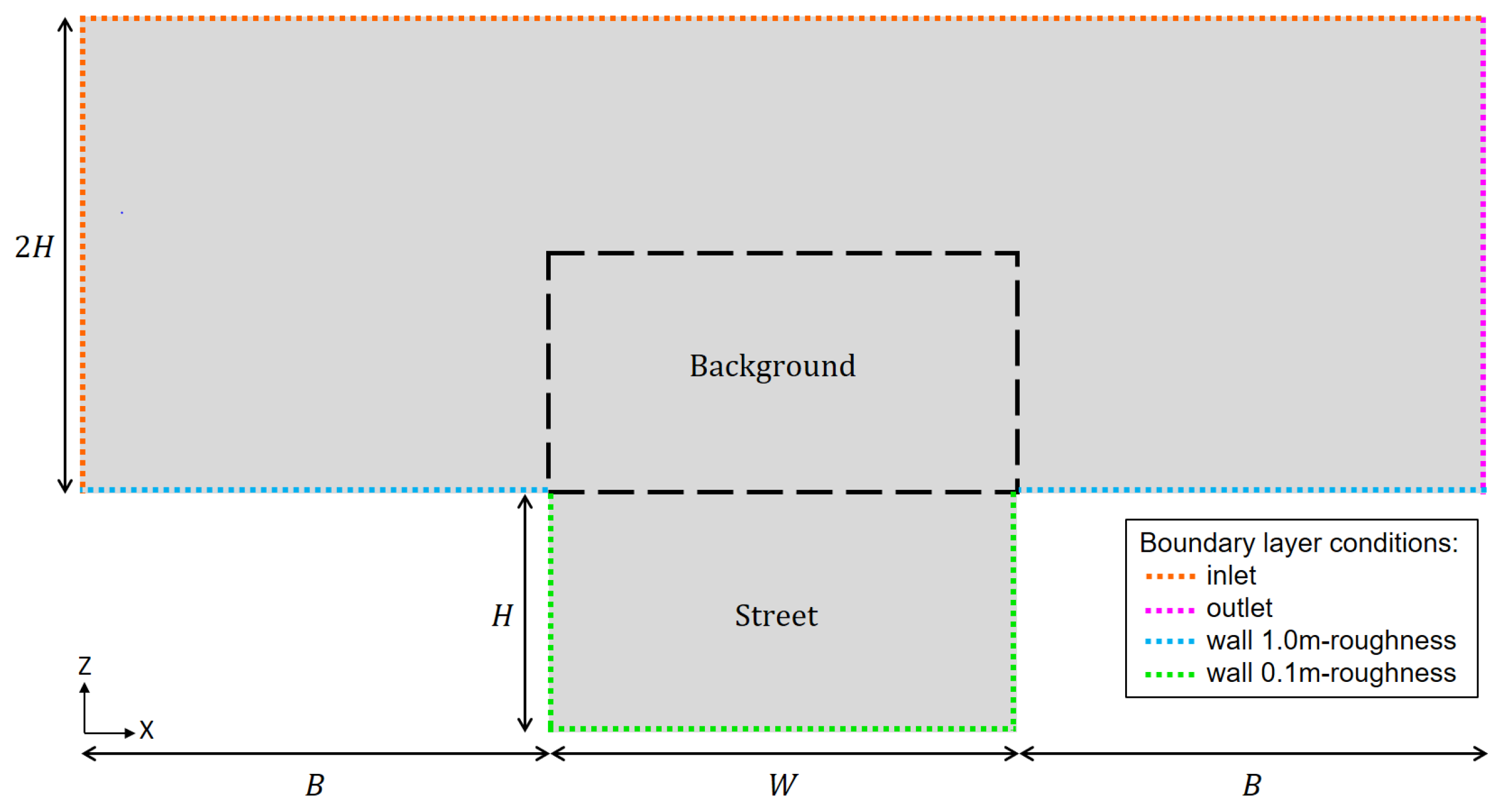
To study wind fields and pollutant dispersion at a local scale (100 m to 1 km), Computational Fluid Dynamics (CFD) are commonly used [
27,
28,
29]. The simulation domain is composed of grid cells with a resolution ranging from centimeters to meters. To solve air flow in two or three dimensions, several turbulence schemes are used, such as
and Large Eddy Simulation (LES). This type of model allows capturing the complex street micro-meteorology; however, the computational cost is high to study pollutant dispersion at city scale as this requires billions of grids.
As concentrations are assumed to be homogeneous in each street segment, the computational cost associated with MUNICH is low compared to CFD, allowing simulations over a whole city [
25]. To model the dispersion of pollutants, the street-network model MUNICH separates air flow into two components related to transfer velocities. First, the horizontal wind speed is assumed to follow the street direction and to be homogeneous across the street. Second, the vertical transfer velocity between the street and the background domain is calculated at the roof level.
These horizontal and vertical air flows depend on the canyon geometry, wind angle, above-street flow and atmospheric stability [
30]. Background concentrations above the roughness sublayer can be provided by 3D CTMs, such as Polair3D from the Polyphemus air-quality modeling platform [
25,
26,
31].
The objective of this study is to evaluate the parametrizations that are currently used in MUNICH for the horizontal and vertical transfers as well as to develop a new parametrization as accurate as the existing ones but with a simpler formulation. It is an adaptation of a parametrization originally designed to model flows in sparse and dense vegetated canopies, depending on the leaf area or spacing between trees. The parametrizations are evaluated by comparisons to Computational Fluid Dynamics (CFD) simulations in street canyons.
They are performed with Code_Saturne [
32], an open-source code that can represent air and pollutant flows in a street canyon [
33]. “It solves the Navier–Stokes equations for 2D, 2D-axisymmetric and 3D flows, steady or unsteady, laminar or turbulent, incompressible or weakly compressible, isothermal or not, with scalars transport” if required (
https://www.code-saturne.org/, accessed on 17 December 2021).
This code has been adapted to atmospheric flows and can simulate the humid atmosphere with radiative heat transfer (not used here). Code_Saturne has been evaluated with the results of the Mock Urban Setting Test (MUST). This is a near full-scale experiment conducted in Utah’s West Desert area based on the release of a neutral gas in a field of regularly spaced shipping containers [
33,
34]. In addition, Code_Saturne was used to simulate thermal effect and pollutant dispersion in a real urban neighborhood of Toulouse, France [
35] and micro-scale heterogeneities of turbulent variables for different wind directions over a complex semi-urban area located near Paris [
36].
In these different studies, the simulated air flow agreed well with the measurements, demonstrating that Code_Saturne was able to accurately simulate airflow and pollutant dispersion over complex urban sites, as well as 2D simplified street canyons. In the present study, Code_Saturne version 6.0 is used. To compare the parametrizations, several simulations are performed with Code_Saturne to consider different street sizes and wind directions. Therefore, the set-up of the model is simplified, and 2D streets are considered to be consistent with the street-network-model approach.
The structure of the paper is as follows. The models MUNICH and Code_Saturne are detailed in
Section 2. Then, a new parametrization for horizontal and vertical transfers based on Code_Saturne simulations and Wang [
37,
38] is proposed for MUNICH and is compared with existing MUNICH parametrizations in
Section 3. Our conclusions are provided in
Section 4.
4. Conclusions
A parametrization that was originally developed for flow in sparse and dense vegetated canopies was adapted to represent the flow in street canyons in the Model of Urban Network of Intersecting Canyons and Highways (MUNICH) based on CFD simulations performed with the Code_Saturne code. The different MUNICH flow parametrizations and Code_Saturne simulations were compared by spatially averaging the wind speed and the passive tracer concentration in the street and the background domains.
The newly adapted parametrization is based on an analytical resolution of the momentum equation within sparse and dense vegetated canopies developed by Wang [
37,
38]. Assuming a homogeneous canopy, the vertical transfer coefficient profile is proportional to the distance from the ground and depends on the canopy features (the height and frontal area density). The vertical wind speed profile is also a function of the canopy features; this converges to a logarithmic profile in the no-canopy scenario and tends to an exponential profile when the frontal area density of obstacles increases.
The Wang [
37,
38] equations were adapted to street canyons by modifying the value of two parameters, one involved in the characteristic length calculation and the other one in the wind speed attenuation coefficient. The street canyon aspect ratio was multiplied by a function of the wind angle to consider the variation of the wind speed in the street with this wind angle (only for horizontal transfers). The modified parameters were determined to maximize the agreement with Code_Saturne simulations: for the average wind speed in the street, the normalized mean absolute error ranged from 1.0% to 1.9%, and the normalized mean bias ranged from −1.0% to −1.9%. For the vertical transfer coefficient, the relative deviation ranged from −4.1% to 2.8%.
Compared to other MUNICH parametrizations, this work added a dependence on the wind angle for the horizontal wind speed in the street. The formulation of the wind speed and vertical transfer coefficient is general and valid for a wide range of street–canyon and wind characteristics. Furthermore, it is simple enough to be easily modified to take new features into account. For example, in further work, the tree effect on air flow in street canyons will be parametrized to consider both building and tree effects on the horizontal wind speed and vertical transfer coefficient. In addition, this parametrization developed for pollutant dispersion could also be used in urban climate models to compute heat and water vapor transfers.