Precursory Signs of Large Forbush Decreases in Relation to Cosmic Rays Equatorial Anisotropy Variation

Abstract

Forbush decreases are usually characterized by increased values of cosmic ray anisotropy. The precursory signs, i.e., pre-increases and especially pre-decreases of the cosmic ray intensity, are highly anisotropic phenomena that ordinarily forewarn of such events. Two Cosmic Ray Groups from the National and Kapodistrian University of Athens (NKUA) and the Pushkov Institute of Terrestrial Magnetism, Ionosphere, and Radiowave Propagation of the Russian Academy of Sciences (IZMIRAN) have been investigating the existence of precursory signs preceding Forbush decreases in relation to different solar phenomena, interplanetary parameters, and geomagnetic conditions. In this study, large Forbush decreases (magnitude > 5%) accompanied by geomagnetic storms (i.e., geomagnetic index Dst < −100 nT and 5 ≤ Kp-index ≤ 9) and characterized by an equatorial anisotropy 1 h before the onset of the event (A_xyb, %) less than 0.8% were examined regarding precursors. In total, 50 events with the aforementioned features were selected and analyzed from the IZMIRAN’s Forbush Effects and Interplanetary Disturbances database concerning the time period from 1969 until 2023. The Ring of Stations method, which depicts the cosmic ray variations for various asymptotic longitudes in relation to time, was applied on each event. The results revealed that clear signs of pre-decreases were not present for the majority of the events. Since particularly strong events were considered, most of them still showed some precursory signs, albeit mainly weak. Despite this, the value of A_xyb = 0.8% proves to be a good threshold for the manual selection of FDs with well-expressed precursors.

1. Introduction

The Earth is bombarded by cosmic rays, i.e., relativistic charged particles that originate from extraterrestrial sources [1,2,3,4]. Specifically, galactic cosmic rays are composed of protons, α-particles (7–10%), and heavier nuclei (1%) [5,6,7,8], come from unknown sources outside the solar system [9], and the solar activity determines their flux in the heliosphere [10]. Furthermore, solar cosmic rays are related to solar flares [11,12] and coronal mass ejections [13] and mainly consist of protons (>89%), α-particles (10%), and heavier nuclei (<1%). The typical energy values of these particles are a few hundred MeV/nucleon, but sometimes reach GeV/nucleon [4,14]. A strong dependence on solar activity is also observed, since at the maximum of solar activity and during the descending phase of the solar cycle, an increased flux of low-energy particles is recorded [10].

One of the most important phenomena of galactic cosmic rays is the Forbush effect or Forbush decrease (FD). Decreases in the recording rate of cosmic rays, which last about a week, were first observed by Forbush in 1937 [15,16,17] using ionization chambers [18]. In particular, Ref. [16] analyzed the measurements from ionization chambers distributed around the Earth for 17 consecutive months to conclude that these intensity variations observed at stations around the world were related to each other. Furthermore, these intensity variations with time were irrespective of atmospheric effects. Initially, it was supposed that these variations were the direct or indirect result of geomagnetic variations, such as disturbances of the geomagnetic field during geomagnetic storms. That is why it was assumed that their origin was terrestrial [19].

Later on, in the early 1950s, it was concluded that these temporal variations were not owing to geomagnetic field disturbances alone [18]. Specifically, Refs. [20,21,22,23,24] recorded cosmic ray intensity (CRI) decreases at the geomagnetic pole. Ref. [23] continued his accurate research using neutron monitors to study the temporal intensity variations in a part of the lower-energy primary particles spectrum. Finally, he showed that the source of these variations was not related to geomagnetic field variations [19] but to the interplanetary medium [25,26] and, specifically, to solar activity phenomena [27,28]. Moreover, he singled out the sharp, non-periodic CRI decreases in magnitude greater than 6%, which would later be defined as FDs. These initial findings are considered to have laid the foundation for the later development of the field of cosmic ray modulation.

Over the years, many definitions have been proposed for describing FDs. The definition as ‘a cosmic ray variation during a geomagnetic storm’ mentioned in [29] is considered to be out of date, since FDs are neither always accompanied by geomagnetic storms nor are always recorded near the Earth. Moreover, the Glossary of Solar-Terrestrial Terms NOAA (https://www.ngdc.noaa.gov/stp/glossary/glossary.html, accessed on 12 May 2024) defines FDs as ‘the sudden variation of about 10% in the galactic cosmic rays intensity, as recorded by the neutron monitors’. However, this also is not a precise definition, since an FD is a phenomenon that evolves gradually and usually has a magnitude of less than 10%, in some rare cases. In addition, this definition assumes that these phenomena can only be observed with neutron monitors, which is not accurate since other detectors are capable of recording FDs, i.e., ionization chambers, ground-based or underground muon detectors, as well as other detectors used in the study of cosmic rays in space. Essentially, the FD could be described as a cosmic ray storm, during which the galactic cosmic ray flux becomes anisotropic. Therefore, the FD is a cosmic ray storm as well as a heliospheric event [30]. According to their origin, FDs could be defined as ‘the effect of coronal mass ejections (CMEs and ICMEs) and/or fast-moving solar wind streams from coronal holes on cosmic rays’ [31,32,33,34]. After having mentioned all the above, the most contemporary definitions describe FDs as a short and abrupt decrease in the galactic CRI that is accompanied by a relatively slow recovery (lasting almost one week) [35,36,37,38].

One of the main subjects when studying FDs is the existence of precursory signs preceding the main phase of the CRI decrease. Refs. [39,40] were the first to introduce and study the decreases and/or increases in the CRI before the evolution of an FD. Nowadays, the investigation of precursory signs has developed into an essential chapter of space weather research [41,42,43,44], etc. and an important contributor to the forewarning of space weather phenomena [45,46,47,48].

Responsible for the occurrence of precursors are the kinetic interactions between cosmic ray particles and the approaching interplanetary shock [42,49]. More specifically, the pre-decrease is recorded when cosmic ray particles exit the FD zone along the magnetic field lines. This happens because the Earth and the cosmic ray-depleted region behind the shock front are magnetically connected, i.e., the “loss cone” effect [42,50]. On the other hand, cosmic ray particles reflect from the approaching interplanetary disturbance, and furthermore, their acceleration at the front of the approaching shock results in pre-increase recording [51,52]. The two precursory signs, i.e., pre-decreases and/or pre-increases in the CRI, differ regarding the mechanism that creates them, but coexist in the area in front of the shock wave [50]. Both types of precursors can last from some hours up to one day before the main phase of the decrease.

The study of precursory signs has concerned many scientific groups around the world. The Athens Cosmic Ray Group of the National and Kapodistrian University of Athens (NKUA) and the Cosmic Ray Group of the Pushkov Institute of Terrestrial Magnetism, Ionosphere, and Radio Wave Propagation (IZMIRAN) of the Russian Academy of Sciences are conducting an ongoing investigation through which different solar parameters, interplanetary conditions, and geomagnetic disturbances are examined in relation to their effectiveness concerning the existence of precursors. The Ring of Stations method is used for studying the precursory signs of FDs. A more detailed description of the method used is included in the next section (see Section 2). These findings are collected and expressed in the form of different criteria.

Moreover, two more attempts in monitoring precursory signs preceding FDs are described herein. First, the global network of neutron monitors called ‘Spaceship Earth’ of the Bartol Research Institute is mentioned. Ref. [53] proposed to set up this neutron monitor network, since neutron monitor stations located at high latitudes provide an excellent opportunity to study cosmic ray events. As a result, a multinational cooperation (USA, Russia, Australia, Canada) took place (https://neutronm.bartol.udel.edu/catch/sse1.html, accessed on 12 May 2024). This network includes 11 stations (Inuvik—Canada, Fort Smith—Canada, Peawanuck—Canada, Nain—Canada, Mawson—Antarctica, Apatity—Russia, Norilsk—Russia, Tixie Bay—Russia, Cape Schmidt—Russia, Thule—Greenland, McMurdo—Antarctica) which are located at high latitudes on four continents and are distinguished by the most favorable directional sensitivity.

The Bartol Research Institute runs an efficient precursory signs monitoring system (http://neutronm.bartol.udel.edu/, accessed on 12 May 2024). It is based on the cosmic rays distribution regarding pitch angle, and even though it uses a limited number of neutron monitor stations, they are located uniformly in regard to longitude. Amongst other interplanetary parameters, the hourly average of the CRI measured by one Spaceship Earth station in relation to the cosmic ray density (for neutron monitor data) or a single directional channel in relation to the cosmic rays density (for muon detector data) is depicted with a circle. The red color describes intensity deficit, while the blue color describes intensity excess. The deficit or excess’s magnitude corresponds to the size of the circle. The vertical and horizontal axes display the pitch angle of the station or the directional channel and the time (last 3 days), respectively (https://neutronm.bartol.udel.edu/spaceweather/welcome.html, accessed on 12 May 2024).

However, this system can potentially have two drawbacks, since a possible operational failure of one of the stations can be responsible for an almost 50° gap in the scan of the celestial sphere, and the uninterrupted interplanetary magnetic field data flow is essential, since these data are used for pitch angle calculations [54].

The search for precursory signs with muon detectors has also been developed and rather successfully [43,52,55,56]. Two investigations related to precursory signs of cosmic rays were conducted using ground-based detectors. As mentioned in [57], 14 major geomagnetic storms were studied using the neutron monitor network. These storms were listed by [58] and were characterized by Kp 8– or greater. Another 22 large geomagnetic storms were studied using data from ground-based muon detectors [55], i.e., a large storm is defined as one characterized by Kp 7– or greater [59]. It should be noted here that neutron monitors as well as muon detectors record the secondary particles’ intensity produced when primary cosmic rays, mainly protons, interact with atoms in the atmosphere of the Earth. The typical energy of the primary cosmic rays that will produce the secondary particles (which will, in turn, be modulated by FDs) is ∼10 GeV and ∼30 GeV for neutron monitors and muon detectors, respectively [42].

It was concluded that the majority of large or major geomagnetic storms are accompanied by precursors. In 68% of events (15 out of 22 large storms) registered by muon detectors and in 79% of events (11 out of 14 major storms) registered by neutron monitors, the precursory signs were clear. As reported by [55], if the muon detectors investigation included only the largest storms (with a Kp value of 8 or greater), then the corresponding percent increases to 89%. It was noted that the precursor signs preceded the sudden storm commencement (SSC) usually by a few to 12 h. This time interval is sufficient to be used in space weather forewarning. Moreover, there is a difference between the duration of the precursory signs provided by neutron monitors and muon detectors. Thus, precursory signs are recorded 8 and 4 h prior to the main event for muon detectors and neutron monitors, respectively [42].

It is known that cosmic rays present stability on a galactic scale and show signs of isotropy in their spatial distribution [60,61]. The mean statistical value of the equatorial component of the first harmonic of cosmic ray anisotropy, A_xy, is less than 0.6% [62], and more precisely, as mentioned in [63], this value is almost 0.51–0.53% during quiet conditions, i.e., ambient solar wind. However, disturbances created in the Sun and moving through interplanetary space may affect cosmic rays. An increase in the solar wind speed, the interplanetary magnetic field, or the cosmic ray density gradient may result in CRI variations and enhanced anisotropies, i.e., an increase in the value of A_xy and significant variations in the vector’s direction. Moreover, the anisotropy 1 h before the FD onset, A_xyb, increases to almost 0.7%, but may jump to ~1% for large (>4%) FDs [64].

As it is understood, the precursory signs preceding FDs are a highly anisotropic phenomenon. FDs with an A_xyb greater than 1.2%, regardless of their magnitude, were examined for precursors in [62] and provided clear and irrefutable results. Yet, because this value exceeded the expected A_xyb before an FD by far, it was imperative to study precursory signs regarding smaller values of anisotropy. Indeed, in the studies that followed, the threshold value for A_xyb was set at 0.8%. Precursors were evident in large FDs (magnitude > 5%), accompanied by geomagnetic storms (geomagnetic index Dst < −100 nT and 5 ≤ Kp-index ≤ 9), and characterized by A_xyb greater than 0.8% [65].

In this work, large FDs (i.e., magnitude > 5%) with an equatorial component of the first harmonic of cosmic ray anisotropy 1 h before the development of the event (A_xyb, %) less than 0.8% that are accompanied by geomagnetic storms (i.e., geomagnetic index Dst < −100 nT and 5 ≤ Kp-index ≤ 9) are being examined for precursory signs. These events cover the period from 1969 until 2023.

2. Data and Method

The events under study were selected from the IZMIRAN’s Forbush Effects and Interplanetary Disturbances (FEID) database [66], which can be found online (https://tools.izmiran.ru/feid, accessed on 12 May 2024), and met the conditions that were mentioned above. Cosmic ray variation parameters in this database are computed using the Global Survey Method (GSM) [67] for the 10 GV rigidity particles with the neutron monitor network data. For example, the zero spherical harmonic or isotropic part of the cosmic ray flux and the first spherical harmonic (i.e., density and anisotropy, respectively), both calculated in percentage, are provided by this method. The aforementioned anisotropy is the hourly equatorial component A_xy of the cosmic ray anisotropy, i.e., the diurnal anisotropy A_xy = $\sqrt{{\mathrm{A}}_{\mathrm{x}}^2+{\mathrm{A}}_{\mathrm{y}}^2}$, where A_x and A_y are components of the cosmic ray anisotropy vector.

For the analysis and description of each event, a significant number of data, inserted in the FEID, were required. For example, data on solar flares, i.e., type, class, location, time (ftp://ftp.swpc.noaa.gov/pub/indices/events/, accessed on 12 May 2024), interplanetary conditions (solar wind speed and interplanetary magnetic field) and geomagnetic activity (geomagnetic indices Dst, Ap, and Kp) provided by the OMNI database (http://omniweb.gsfc.nasa.gov, accessed on 12 May 2024), SSCs (http://isgi.unistra.fr/data_download.php, accessed on 12 May 2024), etc. were assessed.

The FEID is an important tool in the study of cosmic ray phenomena since it includes all CRI variations taking place from 1957 (the beginning of neutron monitors operation) onwards. It provides the opportunity for retrospective studies of almost seven solar cycles (beginning from solar cycle 19 until the current solar cycle 25).

For the monitoring of the precursory signs of FDs, the Ring of Stations method (RSM) was applied (https://tools.izmiran.ru/ros, accessed on 12 May 2024). This method uses hourly CRI data derived from the neutron monitor stations located in different parts of the world that meet certain criteria. In particular, stations must have a geomagnetic cut-off rigidity R_i less than 4 GV, a coupling coefficient for the North–South component of the cosmic ray anisotropy $\left|C_{10}^i\right|$ less than 0.55, and, finally, be located in altitude h_i less than 1200 m. In total, the method derives data from 36 neutron monitor stations, which are presented in detail in [50]. Nevertheless, due to operational issues that the stations can sometimes have, this number may vary occasionally.

Furthermore, the hourly values of the CRI variations at each station are calculated in regard to a quiet period. They are, then, plotted for various asymptotic longitudes in relation to time. It should be mentioned that each neutron monitor station records particles from certain asymptotic directions. The sufficient number of neutron monitor stations that are used by this method ensures as much as possible the most complete scanning of the celestial sphere.

3. Results

In this study, large FDs (with magnitude > 5%), accompanied by geomagnetic storms (geomagnetic index Dst < −100 nT and 5 ≤ Kp-index ≤ 9) and characterized by A_xyb less than 0.8%, were investigated regarding precursory signs. In total, 50 events covering the period from 1969 to 2023 were examined. These events are presented in

Table 1. The FDs under examination and the corresponding magnitude and equatorial component of the first harmonic of cosmic ray anisotropy 1 h before the shock arrival.

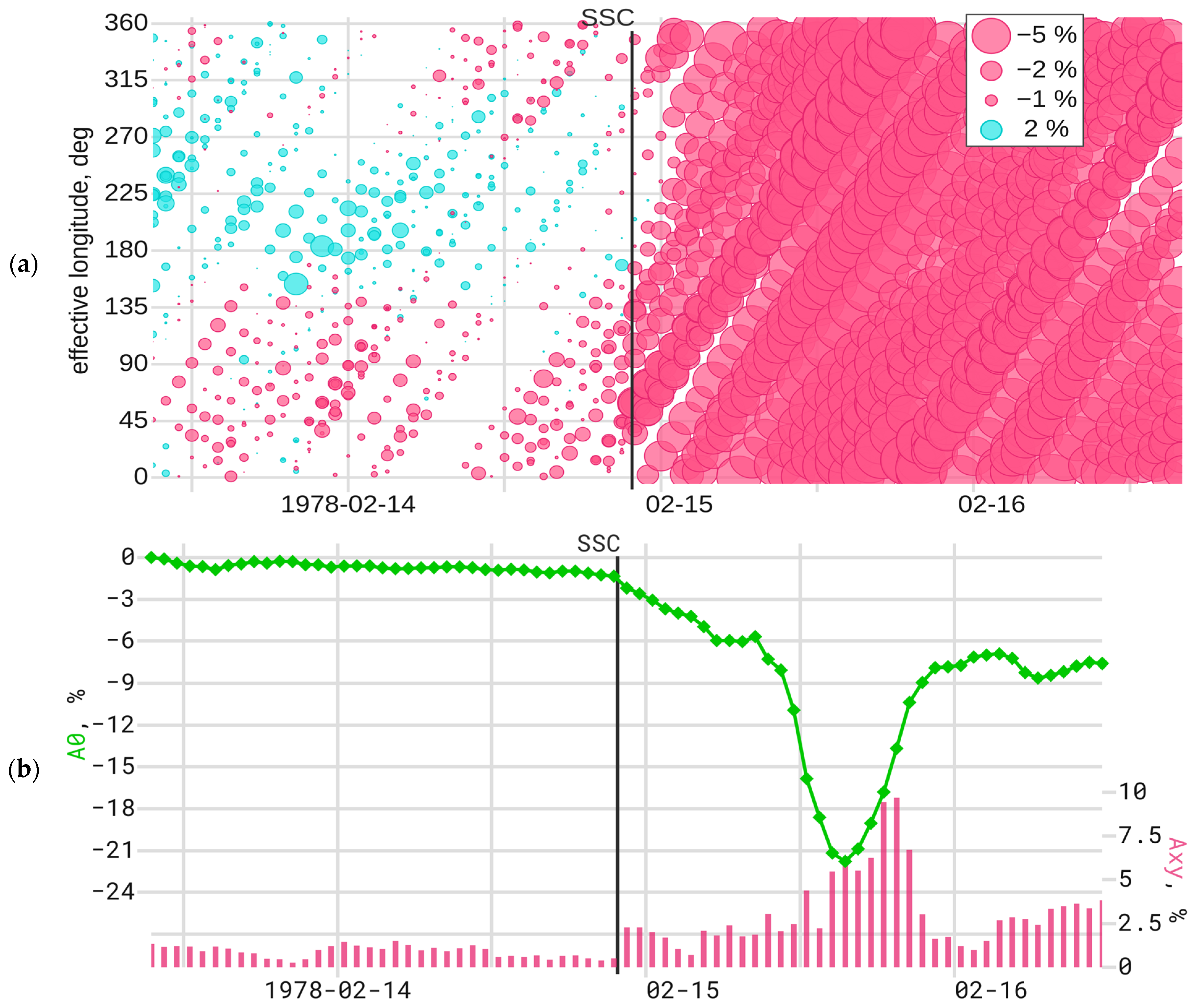
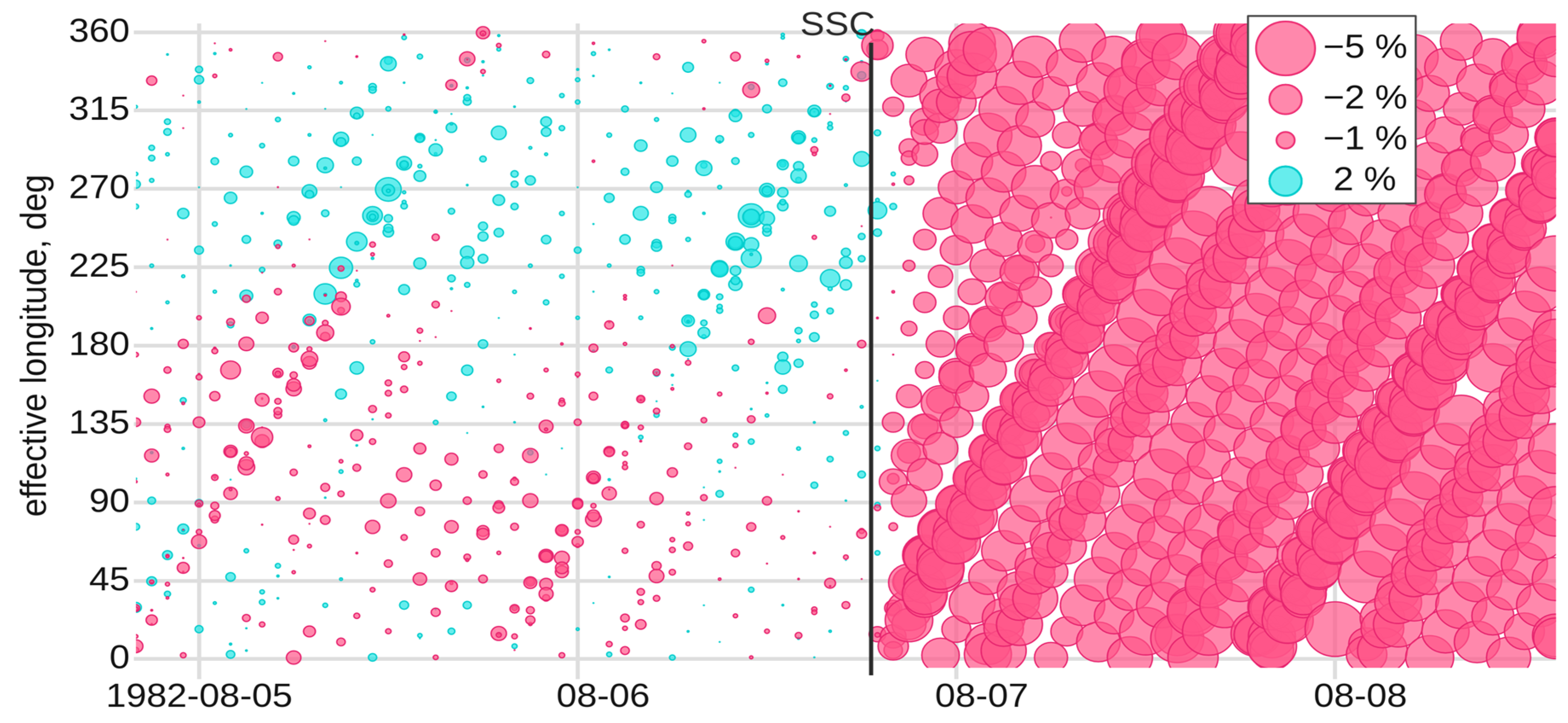
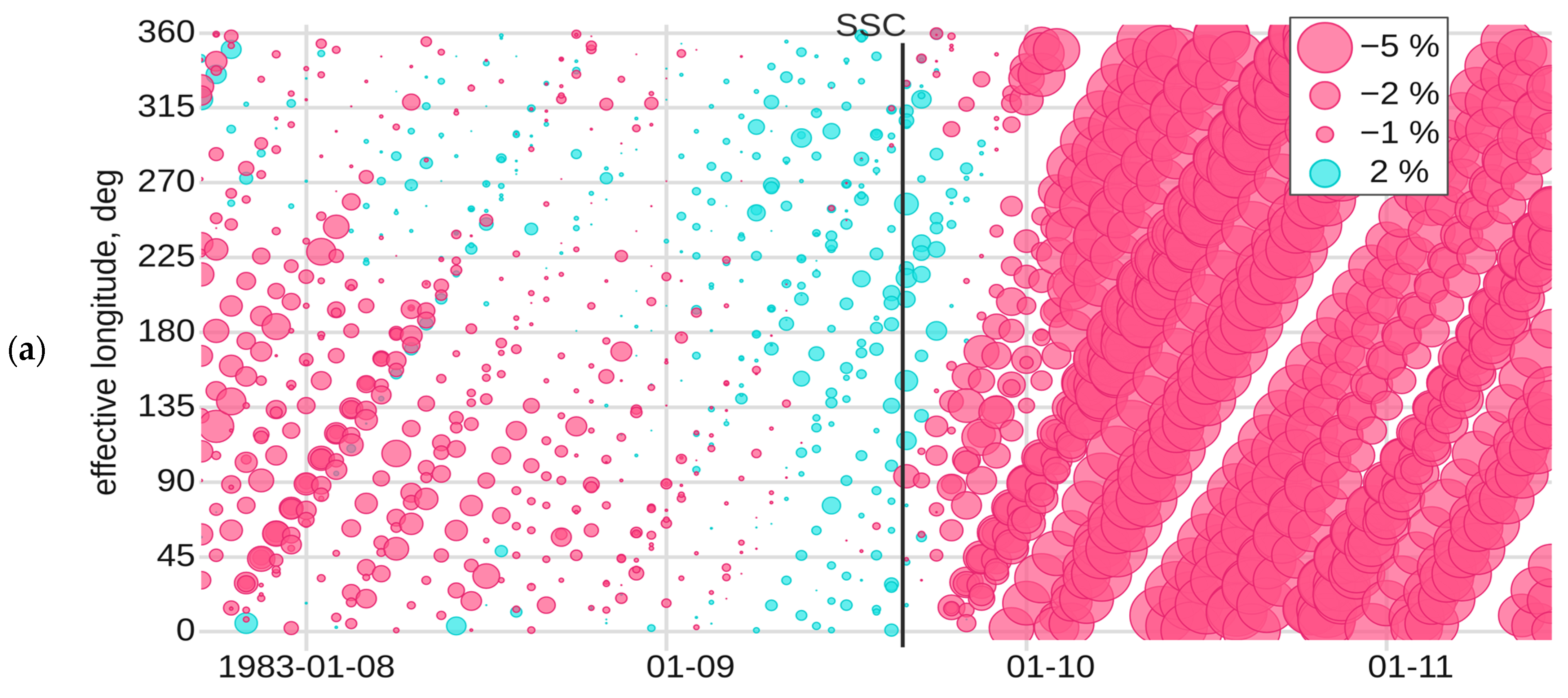
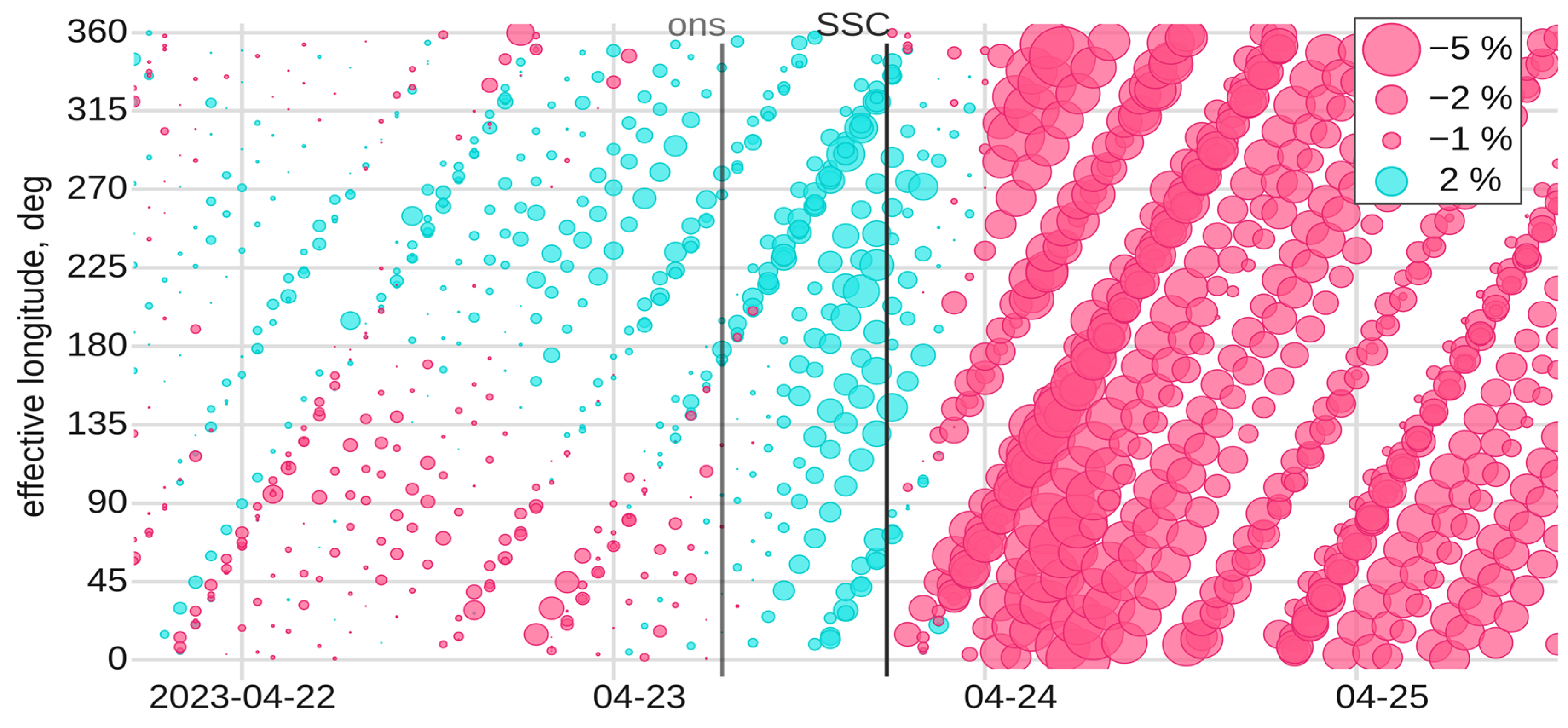
a/a	Forbush Decrease	Magnitude, %	Axyb, %
1	23 March 1969	9.2	0.79
2	14 May 1969	8.7	0.65
3	29 September 1969	5.7	0.59
4	24 July 1970	5.6	0.43
5	14 December 1970	5.8	0.67
6	17 June 1972	7.9	0.44
7	14 February 1978	20.9	0.38
8	24 April 1979	5.3	0.67
9	19 December 1980	8	0.33
10	25 July 1981	8.8	0.51
11	10 October 1981	6.1	0.61
12	13 October 1981	8	0.54
13	1 March 1982	7.2	0.64
14	6 August 1982	6.1	0.42
15	5 September 1982	5.3	0.63
16	21 September 1982	8.5	0.57
17	24 November 1982	7.4	0.45
18	9 January 1983	9.4	0.32
19	21 February 1988	8.7	0.41
20	13 March 1989	20.9	0.11
21	8 June 1989	6	0.30
22	18 September 1989	8.2	0.69
23	17 November 1989	5.9	0.08
24	12 March 1990	7	0.63
25	20 March 1990	5.6	0.18
26	28 July 1990	5.3	0.52
27	24 March 1991	22.1	0.60
28	4 June 1991	8.8	0.71
29	18 August 1991	8.6	0.38
30	8 February 1992	5.8	0.32
31	20 February 1992	5.1	0.23
32	9 May 1992	10.5	0.32
33	22 August 1992	6.3	0.50
34	18 February 1999	6.1	0.27
35	6 November 2000	8.7	0.37
36	24 November 2001	9.8	0.67
37	17 April 2002	7	0.63
38	23 May 2002	7.5	0.34
39	18 August 2002	5.1	0.76
40	29 May 2003	6.9	0.47
41	22 January 2004	9.4	0.35
42	7 November 2004	8.6	0.51
43	9 November 2004	8.6	0.41
44	11 September 2005	13.2	0.41
45	14 December 2006	9.6	0.74
46	26 September 2011	5.1	0.22
47	14 July 2012	9.6	0.53
48	17 March 2015	5.6	0.43
49	7 September 2017	7.7	0.42
50	23 April 2023	7.2	0.16

, along with their magnitude (%) and the equatorial component of the first harmonic of cosmic ray anisotropy 1 h before the shock arrival (%). As it is noticed, some events break the rule that dictates increased anisotropy during a large disturbance. For instance, the FD on 13 March 1989 had a magnitude of 20.9% but the A_xyb was only 0.11%. Another example of a large event (magnitude of 20.9%) with a small A_xyb (0.38%) was the FD on 14 February 1978. This is most likely associated with the characteristics of the solar sources, the geometry of particle acceleration, and the particles’ asymptotic directions of arrival. It should be noted that around half of the events in question happened in 24 h or less after another FD. Though this situation is to be expected in periods of high solar activity, it makes precursors and anisotropy study in general much harder, because a phenomenon which may be regarded as a precursor of an event that may as well turn out to be related to the development of the previous one.

Figure 1a shows the CRI variations for various asymptotic longitudes with time for the FDs on 14 February 1978. In these plots, which were derived from the RSM, magenta-colored circles depict CRI decreases and blue colored circles depict CRI increases (both estimated with respect to an undisturbed base period). Each neutron monitor station’s recording for 24 h is demonstrated diagonally (i.e., line of circles). The circle’s size depends on the size of the variation; the vertical line denotes the FDs’ onset. The asymptotic longitude (in ^o) and the time are marked in the vertical and horizontal axes, respectively. Additionally, for the event on 14 February 1978, data on cosmic ray variations calculated by the GSM are presented (Figure 1b): green curve (A0, left scale)—cosmic ray density variations; pink bars (A_xy, right scale)—changes in hourly values of the equatorial component of cosmic ray vector anisotropy. The A_xyb parameter used to select events in this study is the bar before the event start hour (vertical line).

The event in Figure 1a happened on 14 February 1978, and as was already mentioned, while being of great magnitude (20.9%), it shows a small A_xyb (0.38%), and indeed does not show any clear precursory signs, which is an unexpected behavior for an event of such size. The second event on 6 August 1982 (A_f = 6%, Dst_min = −155 nT, Kp_max = 8–, and A_xyb = 0.42%) is a good example of the A_xyb criterion. The A_xy is quite low before this event, and no clear precursors are visible in the picture (Figure 2). Though the weak increase between longitudes 180° and 320° may be related to the shock wave, it is most likely just related to background solar diurnal anisotropy variation.

Note that sometimes anisotropy may be visible on the RSM pictures, while the value of A_xy computed with GSM is very low. This can be explained by the nature of both methods: while RSM uses count variations directly, the GSM uses more stations and fits a spherical model to their data, which inevitably leads to some smoothing effect.

However, due to the nature of the sample, which includes large FDs, many of them are expected to at least have a pre-increase. Since pre-increase is caused by particle acceleration on the interplanetary shockwave, it thus depends on the shockwave speed, and for the discussed sample of events the average solar wind speed is very high (740 km/s). Two FDs that still show precursory signs while having relatively low A_xyb are presented in Figure 3.

The precursory signs for the FD on 9 January 1983 (A_f = 9.4%, Dst_min = −213 nT, Kp_max = 8+, and A_xyb = 0.32%) indicate a pre-increase on all longitudes, lasting about 8 h (Figure 3a). For the FD on 24 November 2001 (A_f = 9.8%, Dst_min = −221 nT, Kp_max = 8.33, and A_xyb = 0.67%), a pre-increase is also noticed in the longitudinal zone 90°–270°, lasting for almost 6 h (Figure 3b). As can be concluded, by the size of the circles, these precursory signs are relatively weak and last a few hours until the development of the main phase of the FD.

Another situation where the A_xyb criterion does not work as expected is observed in the relatively recent event of 23 April 2023, shown in Figure 4. A very significant pre-increase is observable as blue circles on all longitudes. This effect may be explained by the cosmic ray particles being trapped in a compressing space between two shockwaves, the second one moving faster than the first. Such a large and almost isotropic increase led to very low values of A_xyb.

4. Conclusions and Discussion

Cosmic rays are an important part of space weather. In particular, the study of precursory signs of Forbush decreases can be useful for forecasting large interplanetary disturbances, causing significant geomagnetic storms. These in turn cause changes in the upper atmosphere and other effects, which can be harmful to satellites and other technology [68].

To be able to forewarn of FD events, the NKUA and the IZMIRAN’s Cosmic Ray Groups, in this study, examined whether or not precursory signs were present before the development of large FDs (magnitude > 5%), accompanied by geomagnetic storms (Dst-index < −100 nT and 5 ≤ Kp-index ≤ 9), and characterized by an equatorial component of the first harmonic of cosmic ray anisotropy 1 h before the shock arrival less than 0.8%. In total, 50 events, which occurred from 1969 until 2023, were analyzed.

Events with an equatorial component of the first harmonic of cosmic ray anisotropy 1 h before the shock arrival greater than 0.8% have also been examined and analyzed in a different study [65]. However, this does not affect the novelty of this particular work. The two studies are complementary and not repetitive or even contradictory. The threshold (0.8%) of anisotropy is fully investigated (for greater or lesser values) and, as is shown, the chosen threshold is correctly chosen since the results differ according to this.

It is expected for large events to have great values of anisotropy 1 h before the shock arrival. However, herein, large events with small values of anisotropy 1 h before the shock arrival are studied. This on its own is a very unique situation, indicating specific environmental conditions. Moreover, the fact that the selected events presented signs of a pre-increase and not clear signs of pre-decrease is an important result which helps us understand the physical mechanisms related to these events and evaluate the methods used to analyze them.

The results of this work are summarized as follows:

(1) Sixteen out of the 50 events under study show some signs of a pre-increase, due to the fast-moving interplanetary shock waves. These are the events on 24 April 1979, 19 December 1980, 5 September 1982, 9 January 1983, 13 March 1989, 8 June 1989, 12 March 1990, 28 July 1990, 4 June 1991, 20 February 1992, 9 May 1992, 24 November 2001, 17 April 2002, 29 May 2003, 14 December 2006, and 14 July 2012;

(2) For the majority of the events under study, clear signs of pre-decrease were not observed. Only three events out of 50 showed signs of pre-decrease (7 November 2004, 11 September 2005, and 26 September 2011);

(3) Only two events (1 March 1982 and 22 January 2004) have signs of pre-decrease and pre-increase;

(4) The Ring of Stations method is more sensitive to precursor-like anomalies, which explains some inconsistencies with the Global Survey Method 10 GV anisotropy approximations;

(5) Significant FDs often appear consecutively, which makes the study of the precursors harder;

(6) Setting the threshold value of Axyb at 0.8% helps to select events with clearly expressed precursors, but ideally more criteria should be used along with it.