Functional Relationship between Soil Slurry Transfer and Deposition in Urban Sewer Conduits
Abstract
:1. Introduction
- The flow of a mixture of fluid and soil slurry in urban sewer conduits was considered, similar to simulating the mixing and flowing of a large amount of soil slurry and runoff.
- In conditions of turbulent flow, it was assumed that flow in the conduit consisted of fluid and solid phases.
- For accurate flow analysis, incidental simulations were excluded, and a method for reducing the time needed to analyze a short conduit was considered.
- Soil flowing in a sewer conduit consists of particles of different sizes. For modeling, it is necessary to consider this distribution of particle size. In this study, it was assumed that the soil slurry had a uniform particle size distribution.
2. Numerical Method
2.1. Governing Equations and Mathematical Model
2.2. Turbulence Model
2.3. Setup and Boundary Conditions
2.4. Model Validation
3. Numerical Modeling
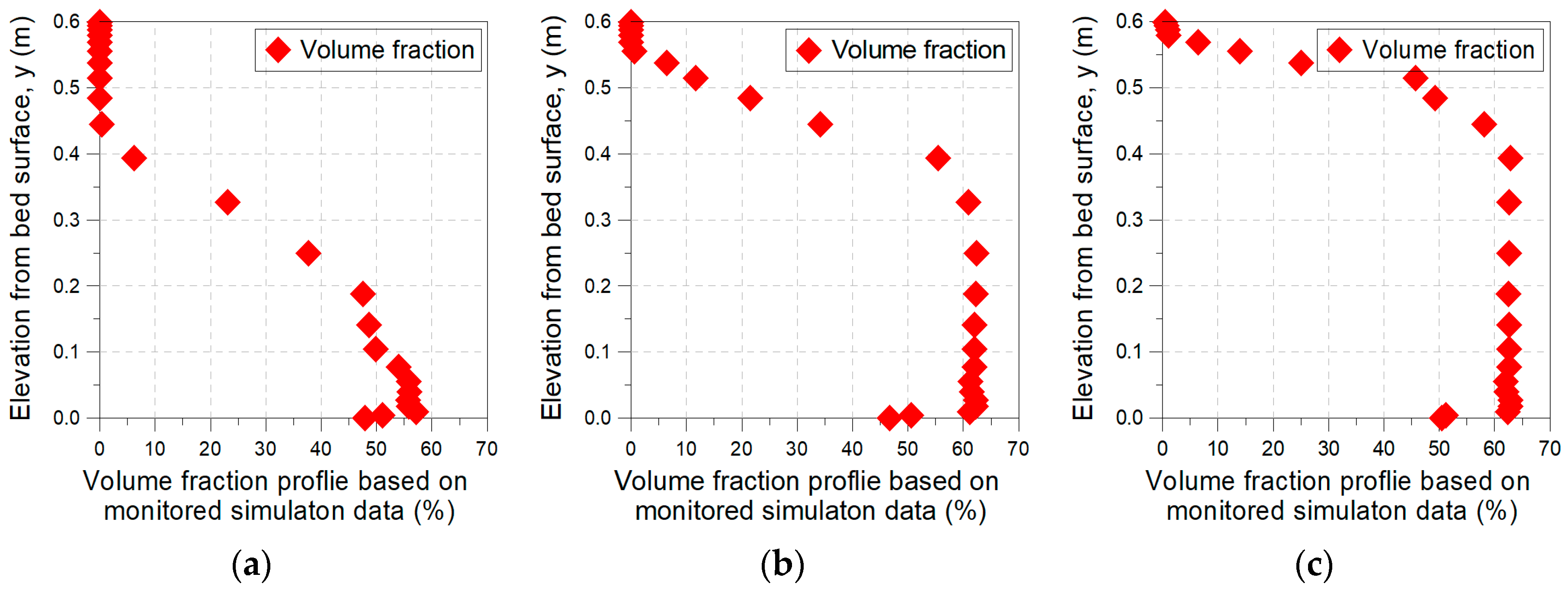
3.1. Analysis of Flow Characteristics in Conduit Depending on Inlet Flow Velocity
3.2. Analysis of Flow Characteristics of Conduit Depending on Inlet Volume Fraction
4. Functional Relationship of Soil Slurry Transfer Deposition in Urban Sewer Conduits
4.1. Calculation and Review of Limiting Tractive Force
4.2. Functional Relationship of Transfer Deposition Due to Soil Slurry Particles
5. Conclusions
- Particle size is the basic criterion for soil slurry transfer in calculating the limiting tractive force, but its influence is little to negligible if particle size is greater or less than a particular value. Nevertheless, small particles flowing into urban sewer conduits are an important variable, and thus conduits must be designed considering their discharge capacity.
- A turbulence model was applied to calculate average flow velocity, shear flow velocity, and shear stress. In a conduit with sedimentation, the distribution of flow velocity was weakened overall by a similar drag-based volume fraction, and a slant in the flow velocity in the conduit increased due to deposition. The shear flow velocity and turbulent stress were large when a large value was calculated around the boundary of the bottom of the conduit. Therefore, it was estimated by applying an overall inclination.
- Based on the results, the authors proposed a functional relationship between the limiting tractive and particle size. This relationship can be used as criterion to judge the transfer deposition of soil slurry in a conduit, and can be applied to urban sewer conduits, unlike previous studies. If the phenomena in urban sewer conduits are measured for comparison in an improved study, a more reasonable research method can be devised.
- The results of this study can help overcome inaccuracy in simulating particles of small diameters in one-dimensional models, which are used to estimate the flow of sediment in urban sewer conduits. In future work, it will be necessary to further investigate the deposition rate of soil in conduits and the pattern of cohesion of each particle.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chebbo, G.; Gromaire, M.C.; Ahyerre, M.; Garnaud, S. Production and transport of urban wet weather pollution in combined sewer systems: The “Marais” experimental urban catchment in Paris. Urban Water 2001, 3, 3–15. [Google Scholar] [CrossRef]
- Gromaire, M.C.; Garnaud, S.; Saad, M.; Chebbo, G. Contribution of different sources to the pollution of wet weather flows in combined sewers. Water Res. 2001, 35, 521–533. [Google Scholar] [CrossRef]
- Ab. Ghani, A.; Md. Azamathulla, H. Gene-expression programming for sediment transport in sewer pipe systems. J. Pipeline Syst. Eng. Pract. 2010, 2, 102–106. [Google Scholar] [CrossRef]
- Azamathulla, H.M.; Ghani, A.A.; Fei, S.Y. ANFIS-based approach for predicting sediment transport in clean sewer. Appl. Soft Comput. 2012, 12, 1227–1230. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shu, A.P.; Wang, L.; Zhang, X.; Ou, G.Q.; Wang, S. Study on the formation and initial transport for non-homogeneous debris flow. Water 2017, 9, 253. [Google Scholar] [CrossRef]
- Lee, H.Y.; Lin, Y.T.; Yunyou, J.; Wenwang, H. On three-dimensional continuous saltating process of sediment particles near the channel bed. J. Hydraul. Res. 2006, 44, 374–389. [Google Scholar] [CrossRef]
- Li, X.; Wei, X. Analysis of the relationship between soil erosion risk and surplus floodwater during flood season. J. Hydrol. Eng. 2013, 19, 1294–1311. [Google Scholar] [CrossRef]
- Meyer-Peter, E.; Müller, R. Formulas for bed-load transport; appendix 2. In Proceedings of the 2nd Meeting of the International Association for Hydraulic Structures Research (IAHSR), Delft, The Netherlands, 7 June 1948; IAHR: Stockholm, Sweden, 1948. [Google Scholar]
- Song, Z.; Liu, Q.; Hu, Z.; Li, H.; Xiong, J. Assessment of sediment impact on the risk of river diversion during dam construction: A simulation-based project study on the Jing River, China. Water 2018, 10, 217. [Google Scholar] [CrossRef]
- Ribberink, J.S. Bed-load transport for steady flows and unsteady oscillatory flows. Coast. Eng. 1998, 34, 59–82. [Google Scholar] [CrossRef]
- Ungar, J.E.; Haff, P.K. Steady-state saltation in air. Sedimentology 1987, 34, 289–299. [Google Scholar] [CrossRef]
- Joshi, S.; Xu, Y.J. Bedload and suspended load transport in the 140-km reach downstream of the Mississippi River avulsion to the Atchafalaya River. Water 2017, 9, 716. [Google Scholar] [CrossRef]
- Shields, A. Application of Similarity Principles, and Turbulence Research to Bed-Load Movement; California Institute of Technology: Pasadena, CA, USA, 1936. [Google Scholar]
- Julien, P.Y. Erosion and Sedimentation; Cambridge University Press: New York, NY, USA, 1995. [Google Scholar]
- Brownlie, W.R. Prediction of Flow Depth and Sediment Discharge in Open Channels; W. M. Keck Laboratory of Hydraulics and Water Resources, California Institute of Technology: Pasadena, CA, USA, 1981. [Google Scholar]
- Eaton, J.K.; Johnston, J.P. A review of research on subsonic turbulent flow reattachment. AIAA J. 1981, 19, 1093–1100. [Google Scholar] [CrossRef]
- Agelinchaab, M.; Tachie, M.F. PIV study of separated and reattached open channel flow over surface mounted blocks. J. Fluids Eng. 2008, 130, 1–9. [Google Scholar] [CrossRef]
- Brevis, W.; García-Villalba, M.; Niño, Y. Experimental and large eddy simulation study of the flow developed by a sequence of lateral obstacles. Environ. Fluid Mech. 2014, 14, 873–893. [Google Scholar] [CrossRef]
- Georgoulas, A.N.; Kopasakis, K.I.; Angelidis, P.B.; Kotsovinos, N.E. Numerical investigation of continuous, high-density turbidity currents response, in the variation of fundamental flow controlling parameters. Comput. Fluids 2012, 60, 21–35. [Google Scholar] [CrossRef] [Green Version]
- Chuanjian, M.A.N.; Jungsun, O.H. Stochastic particle based models for suspended particle movement in surface flows. Int. J. Sediment Res. 2014, 29, 195–207. [Google Scholar]
- Pang, A.L.J.; Skote, M.; Lim, S.Y.; Gullman-Strand, J.; Morgan, N. A numerical approach for determining equilibrium scour depth around a mono-pile due to steady currents. Appl. Ocean Res. 2016, 57, 114–124. [Google Scholar] [CrossRef]
- Coker, E.H.; Van Peursem, D. The Erosion of horizontal sand slurry pipelines resulting from inter-particle collision. Wear 2017, 400–401, 74–81. [Google Scholar] [CrossRef]
- Song, Y.H.; Yun, R.; Lee, E.H.; Lee, J.H. Predicting sedimentation in urban sewer conduits. Water 2018, 10, 462. [Google Scholar] [CrossRef]
- ANSYS. ANSYS Fluent 12.1 Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2010. [Google Scholar]
- Newton, C.H.; Behnia, M. Numerical calculation of turbulent stratified gas–liquid pipe flows. Int. J. Multiph. Flow 2000, 26, 327–337. [Google Scholar] [CrossRef]
- Ghorai, S.; Nigam, K.D.P. CFD modeling of flow profiles and interfacial phenomena in two-phase flow in pipes. Chem. Eng. Process. Process Intensif. 2006, 45, 55–65. [Google Scholar] [CrossRef]
- De Schepper, S.C.; Heynderickx, G.J.; Marin, G.B. CFD modeling of all gas-liquid and vapor-liquid flow regimes predicted by the Baker chart. Chem. Eng. J. 2008, 138, 349–357. [Google Scholar] [CrossRef]
- Bhramara, P.; Rao, V.D.; Sharma, K.V.; Reddy, T.K.K. CFD analysis of two phase flow in a horizontal pipe-prediction of pressure drop. Momentum 2009, 10, 476–482. [Google Scholar]
- Nezu, I.; Nakagawa, H.; Jirka, G.H. Turbulence in open-channel flows. J. Hydraul. Eng. 1994, 120, 1235–1237. [Google Scholar] [CrossRef]
- Durán, O.; Andreotti, B.; Claudin, P. Turbulent and viscous sediment transport—A numerical study. Adv. Geosci. 2014, 37, 73–80. [Google Scholar] [CrossRef]
- Guo, J.; Mohebbi, A.; Zhai, Y.; Clark, S.P. Turbulent velocity distribution with dip phenomenon in conic open channels. J. Hydraul. Res. 2015, 53, 73–82. [Google Scholar] [CrossRef]
- Park, C.W.; Hong, C.H. User Guide of ANSYS Workbench; Intervision: Seoul, Korea, 2008. [Google Scholar]
- Anjum, N.; Ghani, U.; Ahmed Pasha, G.; Latif, A.; Sultan, T.; Ali, S. To investigate the flow structure of discontinuous vegetation patches of two vertically different layers in an open channel. Water 2018, 10, 75. [Google Scholar] [CrossRef]
- Török, G.T.; Baranya, S.; Rüther, N. 3D CFD modeling of local scouring, bed armoring and sediment deposition. Water 2017, 9, 56. [Google Scholar] [CrossRef]
- Fan, F.; Liang, B.; Li, Y.; Bai, Y.; Zhu, Y.; Zhu, Z. Numerical investigation of the influence of water jumping on the local scour beneath a pipeline under steady flow. Water 2017, 9, 642. [Google Scholar] [CrossRef]
- Loth, E.; Kailasanath, K.; Löhner, R. Supersonic flow over an axisymmetric backward-facing step. J. Spacecr. Rocket. 1992, 29, 352–359. [Google Scholar] [CrossRef]
- Sahu, J.; Heavey, K.R. Numerical investigation of supersonic base flow with base bleed. J. Spacecr. Rocket. 1997, 34, 62–69. [Google Scholar] [CrossRef]
- Jalali, P. Theory and Modelling of Multiphase Flows; Lecture Material; Lappeenranta University of Technology: Lappeenranta, Finland, 2010. [Google Scholar]
- Wallis, G.B. One-Dimensional Two-Phase Flow; McGraw-Hill Book Company: New York, NY, USA, 1969. [Google Scholar]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Pergamon Press: New York, NY, USA, 1983; pp. 96–116. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education: London, UK, 2007. [Google Scholar]
- Prandtl, L. Bericht uber Untersuchungen zur ausgebildeten Turbulenz. Z. Angew. Math. Mech. 1925, 5, 136–139. [Google Scholar]
- Nabil, T.; El-Sawaf, I.; El-Nahhas, K. Sand-water slurry flow modelling in a horizontal pipeline by computational fluid dynamics technique. Int. Water Technol. J. 2014, 4, 13. [Google Scholar]
- Matousek, V. Flow Mechanism of Soil–Water Mixtures in Pipelines. Ph.D. Thesis, Delft University, Delft, The Netherlands, 15 December 1997. [Google Scholar]
- Raudkivi, A.J. Loose Boundary Hydraulics, 4th ed.; CRC Press: Rotterdam, The Netherlands, 1998. [Google Scholar]
- O’Brien, J.S.; Julien, P.Y. Laboratory analysis of mudflow properties. J. Hydraul. Eng. 1988, 114, 877–887. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Strain accumulation in sand due to drained uniaxial cyclic loading. In Cyclic Behaviour of Soils and Liquefaction Phenomena; Taylor & Francis Group: London, UK, 2004; pp. 233–246. [Google Scholar]
- Nezu, I. Experimental Study on Secondary Currents in Open Channel Flows. In Proceedings of the 21st IAHR Congress, Melbourne, Australia, 13–18 August 1985. [Google Scholar]
- Schlichting, H.; Gersten, K.; Krause, E.; Oertel, H.; Mayes, K. Boundary-Layer Theory; McGraw-Hill Book Company: New York, NY, USA, 1955. [Google Scholar]
- Maa, J.P.Y. The bed shear stress of an annular sea-bed flume. In Estuarine Water Quality Management; Springer: Berlin/Heidelberg, Germany, 1990. [Google Scholar]
- Yalin, M.S.; Karahan, E. Inception of sediment transport. J. Hydraul. Div. 1979, 105, 1433–1443. [Google Scholar]
- Smith, D.A.; Cheung, K.F. Initiation of motion of calcareous sand. J. Hydraul. Eng. 2004, 130, 467–472. [Google Scholar] [CrossRef]
- Guo, J. Hunter rouse and shields diagram. In Advances in Hydraulics and Water Engineering; World Scientific Publishing Co.: Singapore, 2002; Volumes I & II, pp. 1096–1098. [Google Scholar]
- Syamlal, M.; O’Brien, T.J. The Derivation of a Drag Coefficient Formula from Velocity-Voidage Correlations; Technical Note; US Department of Energy, Office of Fossil Energy, NETL: Morgantown, WV, USA, 1987. [Google Scholar]
- Wen, C.Y.; Yu, Y.H. A generalized method for predicting the minimum fluidization velocity. AIChE J. 1966, 12, 610–612. [Google Scholar] [CrossRef]
- Parker, G.; Toro-Escobar, C.M.; Ramey, M.; Beck, S. Effect of floodwater extraction on mountain stream morphology. J. Hydraul. Eng. 2003, 129, 885–895. [Google Scholar] [CrossRef]
- Karahan, H.; Gurarslan, G.; Geem, Z.W. Parameter estimation of the nonlinear Muskingum flood-routing model using a hybrid harmony search algorithm. J. Hydrol. Eng. 2012, 18, 352–360. [Google Scholar] [CrossRef]
- Hyndman, R.J.; Koehler, A.B. Another look at measures of forecast accuracy. Int. J. Forecast. 2006, 22, 679–688. [Google Scholar] [CrossRef] [Green Version]
- Willmott, C.J.; Matsuura, K. On the use of dimensioned measures of error to evaluate the performance of spatial interpolators. Int. J. Geogr. Inf. Sci. 2006, 20, 89–102. [Google Scholar] [CrossRef]
- Pontius, R.G.; Thontteh, O.; Chen, H. Components of information for multiple resolution comparison between maps that share a real variable. Environ. Ecol. Stat. 2008, 15, 111–142. [Google Scholar] [CrossRef]

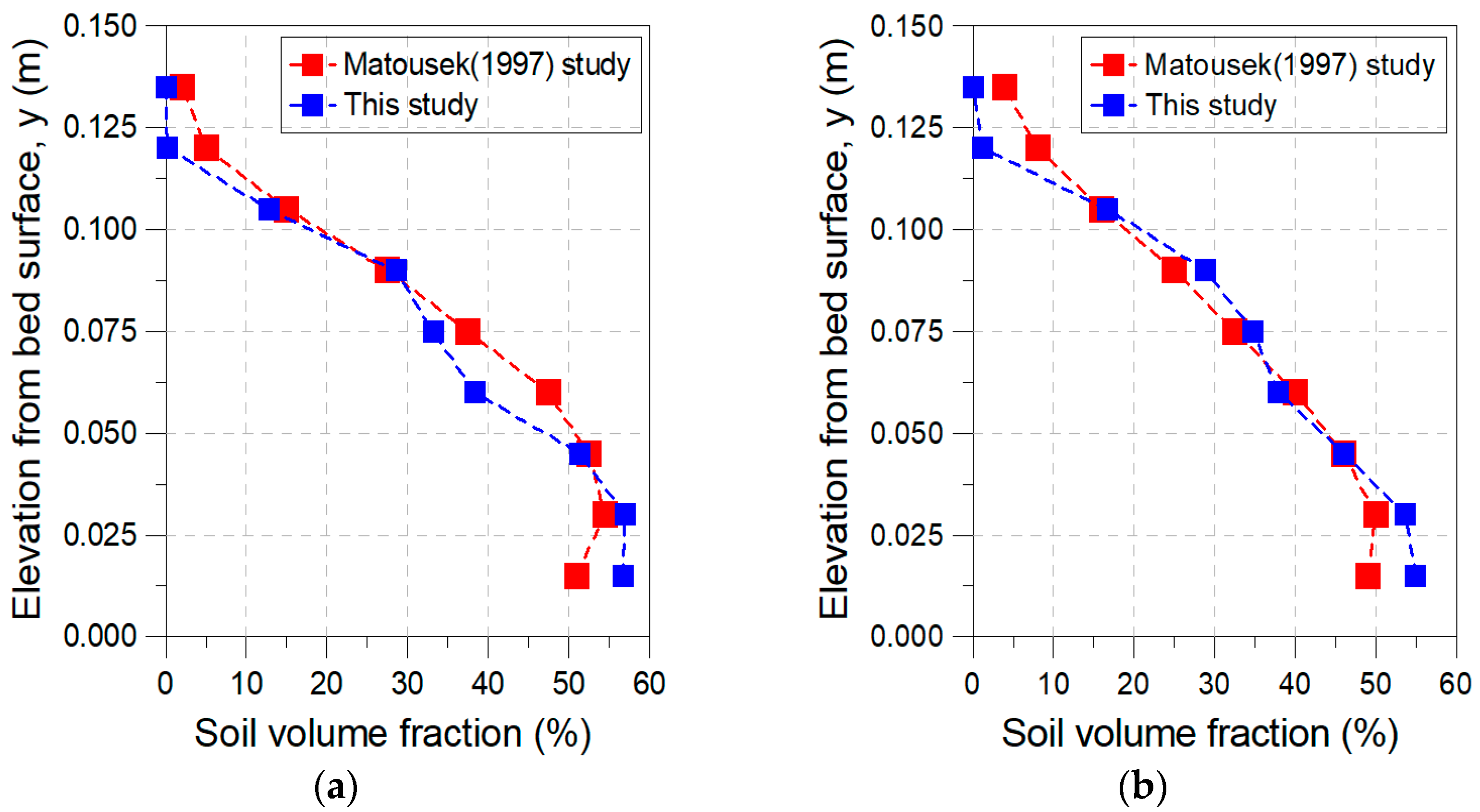
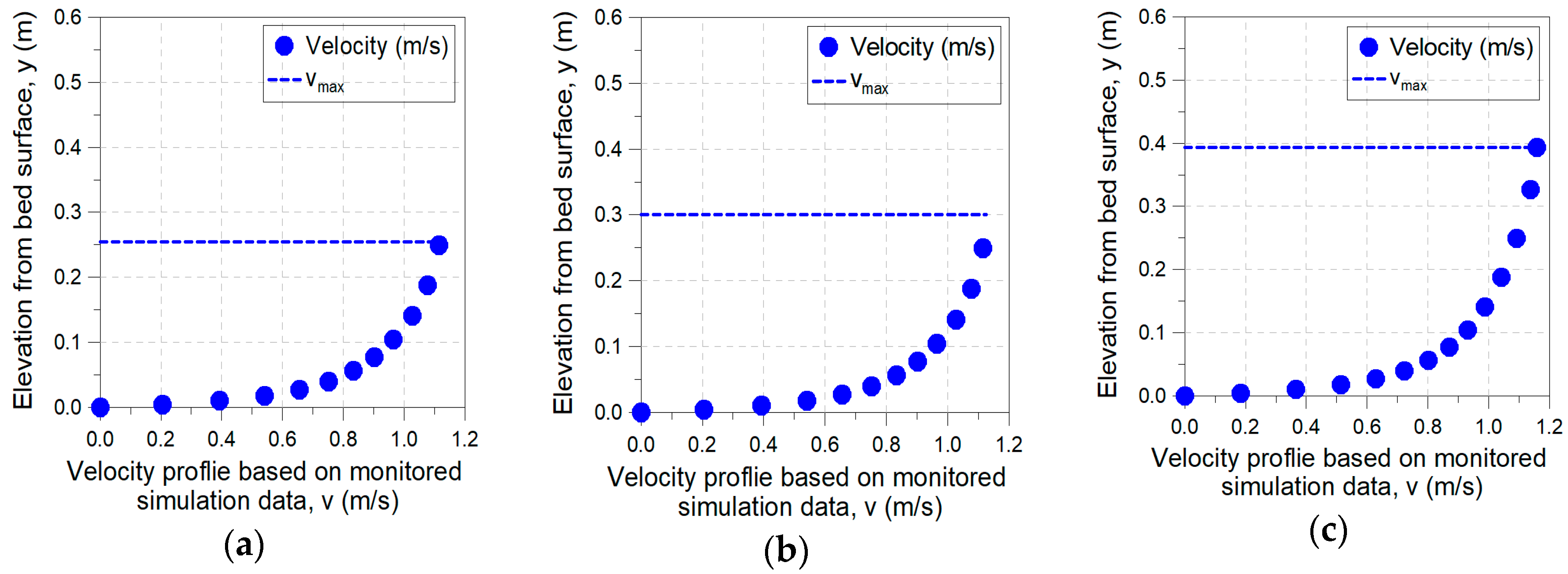
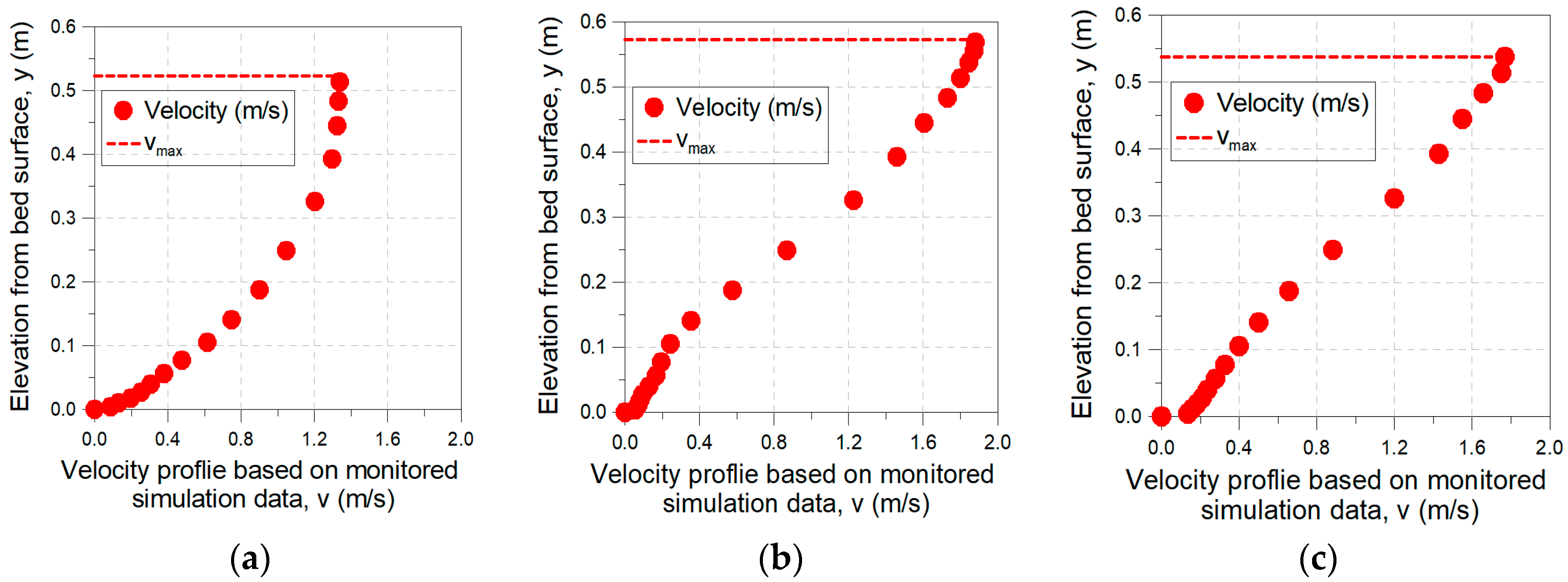
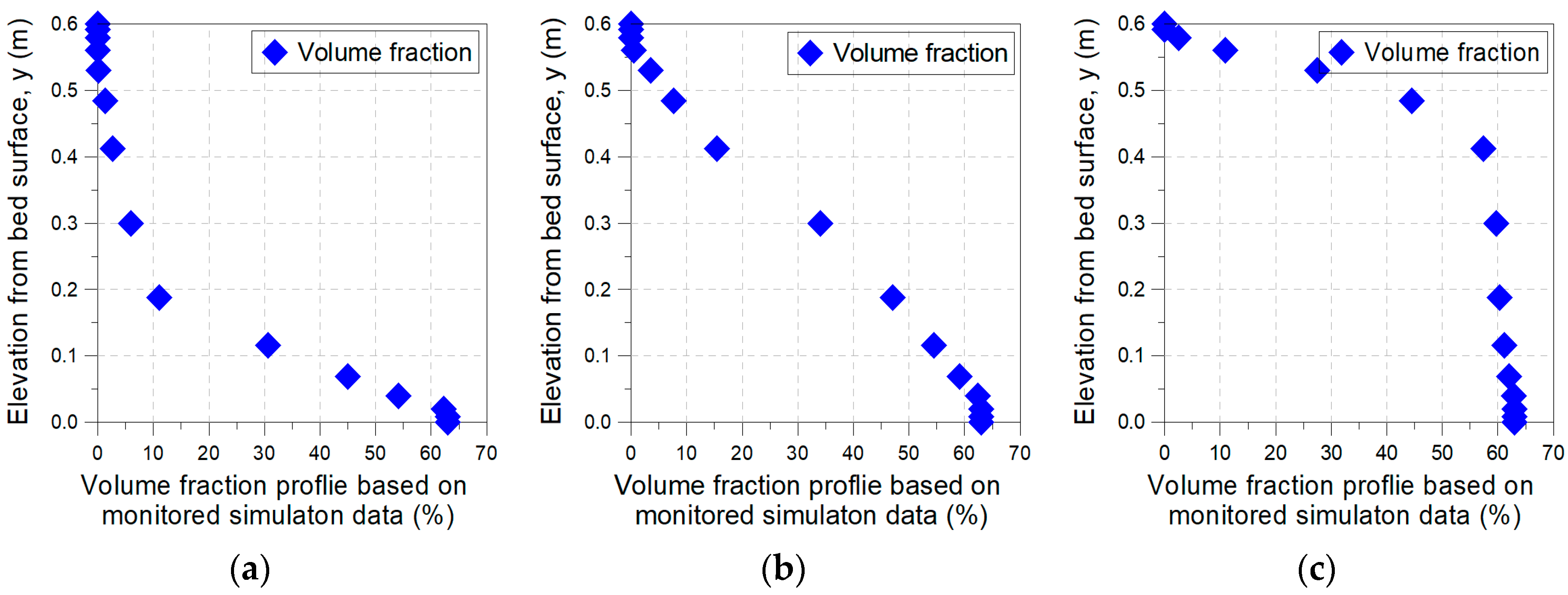

| Parameter | |||||
|---|---|---|---|---|---|
| Value | 0.09 | 1.44 | 1.92 | 1.0 | 1.3 |
| Classification | Boundary Conditions |
|---|---|
| Multiphase flow | Fluid (water)-Solid (soil) |
| Applied models and flow conditions | Euler–Euler model Standard k-ε model Turbulent flow Unsteady and turbulent |
| Inlet conditions | Inlet velocity Inlet volume fraction Soil diameter |
| Outlet condition | Free fall |
| Wall condition | Non-slip |
| Convergence | 0.001 |
| Parameter | Units | Value |
|---|---|---|
| Conduit specification | m | 0.6 (D) × 10 (L) |
| Mesh specification | grid | 140,000 |
| Inlet velocity condition | m/s | 1.0, 2.0, 3.0 |
| Inlet volume fraction condition | % | 10, 30, 50 |
| Fluid density | kg/m3 | 998.2 |
| Fluid kinematic viscosity | Pa·s | 0.001003 |
| Soil density | kg/m3 | 2,650 |
| Soil diameter | mm | 0.5, 1.0, 3.0, 5.0, 7.0, 15.0, 20.0 |
| d (mm) | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 1.0 m/s | 2.0 m/s | 3.0 m/s | |||||||
| 10% v/f | 30% v/f | 50% v/f | 10% v/f | 30% v/f | 50% v/f | 10% v/f | 30% v/f | 50% v/f | |
| 0.5 | 0.030 | 0.060 | 0.056 | 0.035 | 0.040 | 0.041 | 0.284 | 0.308 | 0.307 |
| 1.0 | 0.036 | 0.036 | 0.029 | 0.074 | 0.730 | 0.068 | 0.224 | 0.259 | 0.243 |
| 3.0 | 0.061 | 0.037 | 0.041 | 0.033 | 0.029 | 0.028 | 0.086 | 0.098 | 0.102 |
| 5.0 | 0.046 | 0.051 | 0.056 | 0.056 | 0.032 | 0.056 | 0.027 | 0.036 | 0.034 |
| 7.0 | 0.047 | 0.047 | 0.036 | 0.045 | 0.047 | 0.041 | 0.035 | 0.055 | 0.056 |
| 15.0 | 0.052 | 0.052 | 0.060 | 0.038 | 0.045 | 0.048 | 0.042 | 0.042 | 0.051 |
| 20.0 | 0.048 | 0.060 | 0.054 | 0.053 | 0.048 | 0.051 | 0.049 | 0.055 | 0.056 |
| (mm) | (non-dimensional) | Relationship Equation |
|---|---|---|
| ≤ 0.5 | ≤ 9.4 | |
| 0.5 < ≤ 1.0 | 9.4 < ≤ 18.8 | |
| 1.0 < ≤ 3.0 | 18.8 < ≤ 56.4 | |
| 3.0 < ≤ 5.0 | 56.4 < ≤ 94.0 | |
| 5.0 < ≤ 7.0 | 94.0 < ≤ 131.6 | |
| 7.0 < ≤ 15.0 | 131.6 < ≤ 282.1 | |
| 15.0 < ≤ 20.0 | 282.1 < ≤ 376.1 | |
| 20.0 < | 376.1 < |
| (mm) | (non-dimensional) | Shields and Brownlie | This Study |
|---|---|---|---|
| 0.5 | 9.4 | 0.0334 | 0.1468 |
| 1.0 | 18.8 | 0.0298 | 0.0600 |
| 3.0 | 56.4 | 0.0419 | 0.0395 |
| 5.0 | 94.0 | 0.0485 | 0.0438 |
| 7.0 | 131.6 | 0.0535 | 0.0450 |
| 15.0 | 282.1 | 0.0556 | 0.0478 |
| 20.0 | 376.1 | 0.0556 | 0.0530 |
| SSQ | 0.0020 | ||
| RMSE | 0.0446 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.H.; Lee, E.H.; Lee, J.H. Functional Relationship between Soil Slurry Transfer and Deposition in Urban Sewer Conduits. Water 2018, 10, 825. https://doi.org/10.3390/w10070825
Song YH, Lee EH, Lee JH. Functional Relationship between Soil Slurry Transfer and Deposition in Urban Sewer Conduits. Water. 2018; 10(7):825. https://doi.org/10.3390/w10070825
Chicago/Turabian StyleSong, Yang Ho, Eui Hoon Lee, and Jung Ho Lee. 2018. "Functional Relationship between Soil Slurry Transfer and Deposition in Urban Sewer Conduits" Water 10, no. 7: 825. https://doi.org/10.3390/w10070825
APA StyleSong, Y. H., Lee, E. H., & Lee, J. H. (2018). Functional Relationship between Soil Slurry Transfer and Deposition in Urban Sewer Conduits. Water, 10(7), 825. https://doi.org/10.3390/w10070825







