An Experimental Study on Mechanisms for Sediment Transformation Due to Riverbank Collapse
Abstract
:1. Introduction
2. Methodology
2.1. Experiments
2.1.1. Experimental Setup
2.1.2. Experimental Testing Conditions
2.1.3. Experimental Procedure
2.2. Data Processing
2.2.1. Estimation of Riverbank Collapse Volume
2.2.2. Estimation of Velocity for Incipient Sediment Motion
2.2.3. Estimation of Sediment Load Ratio
- -
- Firstly, it is essential to assume a series of d0 values (The value of d0 is from 0.050 mm to 0.070 mm, and d0 should be taken every 0.002 mm, for example, 0.050 mm, 0.052 mm, ..., 0.070 mm). For each value of d0, the corresponding X can be obtained through Figure 5b;
- -
- Consequently, because the parameters (Sv, γf, γm, γs, γw, g, R, and ν) are the basic data and can be obtained through experimental conditions, Svc and Svf can be obtained through Equations (10) and (11);
- -
- Then ω0 can be obtained by applying Equation (9);
- -
- Jl can be obtained from Equation (13), Js can be obtained from Equation (14);
- -
- J = Jl + Js can be obtained from Equation (2);
3. Results
3.1. Dynamic Characteristics of the Bank Collapse Process
3.1.1. Collapse Process of Cohesive Riverbanks
3.1.2. Velocity Distribution
3.1.3. Pore Water Pressure
3.2. Quantitative Analysis of the Sediments Transformation
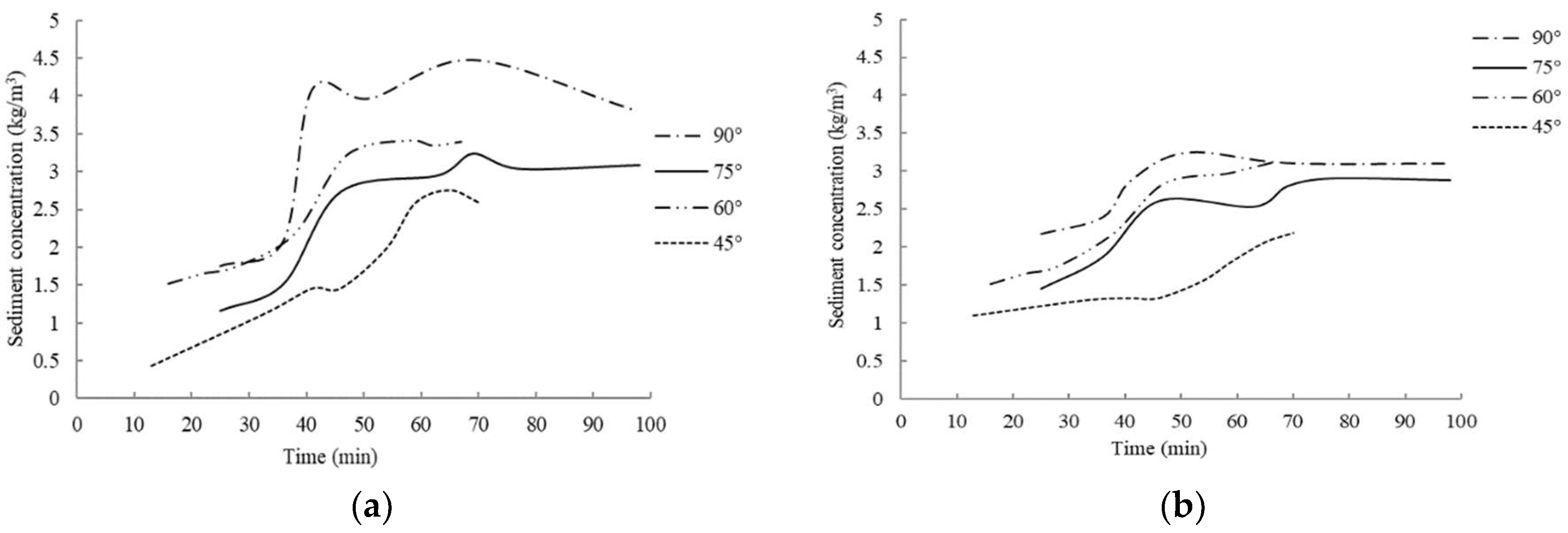
3.2.1. Sediment Concentration Variation
3.2.2. Sediment Transformation from Bank Collapsed Materials to River Sediment
3.2.3. Sediment Transformation from Bed Load to Suspended Load
4. Conclusions and Discussion
- (1)
- Experimental tests conducted have enabled the characterization of the riverbank collapse process within four different slopes simulated. This phenomenon can be subdivided into multiple steps: (i) the foot of the bank is frequently affected by the washing effect of the water flowing within the river; (ii) small fragments start to fall down along the bank and a groove is usually gradually formed on the bank toe. The groove becomes deeper and deeper as the water flow continues to wash the bank. Due to the strong bonding effect, the cohesive soil in the upper part of the groove is suspended. When the gravity of the suspended soil is greater than its anti-sliding force and exceeds its stable slope, cracks form along the bank slope. The cracks deepen until the collapse occurs and part of the bank falls in blocks inside the river. When the bank slope is steep, its collapse surface is almost flat, which is consistent with the collapse observed at Dengkou River section [37].
- (2)
- Topographic surveys were completed to characterize the riverbank collapse under different slope scenarios and the bank collapse magnitude is directly correlated with the water flow rate; in fact, the greater is the flow rate, the faster the collapse occurs and faster is the time for the collapse to initiate. Moreover, the pore water pressure monitored during the occurrence of the riverbank collapse, is an indicator of how quickly this phenomenon arises (e.g., for gentle slopes, the internal pore water pressure of soil fluctuates for a longer duration, particles interact more frequently, and the shear resistance seems to be stronger than the shear resistance of steep slopes).
- (3)
- The process of sediment transformation from bank collapsed materials to river sediment was analyzed through monitoring the sediment concentration at typical sections and the tailgate. It was found that the change of sediment concentration with time is basically consistent regardless of the initial bank slope. At the beginning, the sediment concentration increases slowly, and then rises dramatically. The rapid rise of the curve corresponds with the occurrence of the collapse along the riverbank. When the high amount of debris enters the river, it mixes with the water, increasing the sediment concentration along the stream and reaching the maximum value recorded. Consequently, it gradually decreases and becomes stable. The rapid increase of sediment concentration due to collapse is between 35 and 45 min after the start of the experiment, but for the tailgate section, the maximum sediment concentration is between 40 and 50 min. The maximum sediment concentration of the tailgate section arrives later than that of section #3. This is due to the fact that when collapsed soil enters into the water, the water requires time to carry it downstream. Additionally, it was observed that the maximum sediment concentration of the tailgate is smaller than that of section #3. That is due to the typical sediments transport process within river.
- (4)
- When the sediment enters the river, part of it is carried downstream by the current, and the remaining part deposits at the foot of the bank. The deposited sediment, under the action of water flowing, is further activated and then transformed into bed load and suspended load, which is transported downstream as well within the water. In terms of quantity, about 7–10% of the sediment typically deposits locally, and the rest continues to move downstream with the water flowing, part of which (70–77%) forms suspended sediment and another part (15–20%) forms bed load.
Author Contributions
Funding
Conflicts of Interest
References
- Yao, Z.Y.; Ta, W.Q.; Jia, X.P.; Xiao, J.H. Bank erosion and accretion along the Ningxia-Inner Mongolia reaches of the Yellow River from 1958 to 2008. Geomorphology 2011, 1–2, 99–106. [Google Scholar] [CrossRef]
- Shu, A.; Gao, J.; Duan, G.S.; Zhang, X. Cluster analysis for factor classification and riverbank collapse along the desert wide valley reach of the upper Yellow River. J. Tsinghua Univ. (Sci. Tech.) 2014, 8, 1044–1048. (In Chinese) [Google Scholar] [CrossRef]
- Ta, W.Q.; Xiao, H.L.; Dong, Z.B. Long-term morphodynamic changes of a desert reach of the Yellow River following upstream large reservoirs’ operation. Geomorphology 2008, 97, 249–259. [Google Scholar] [CrossRef]
- Yu, M.H.; Wei, H.Y.; Liang, Y.J.; Hu, C.W. Study on the stability of non-cohesive riverbank. Int. J. Sediment. Res. 2010, 4, 391–398. [Google Scholar] [CrossRef]
- Xia, J.Q.; Wang, G.Q.; Zhang, H.W.; Fang, H.W. A review of the research on lateral widenning mechanisms of alluvial rivers and their simulation approaches. J. Sediment. Res. 2001, 6, 71–78. (In Chinese) [Google Scholar] [CrossRef]
- Thorne, C.R. ASCE task committee on hydraulic, bank mechanics, and modeling of river width adjustment. River width adjustment. I: Processes and mechanisms. J. Hydraul. Eng. 1998, 9, 881–902. [Google Scholar] [CrossRef]
- Buffington, J.M.; Montgomery, D.R. A systematic analysis of eight decades of incipient motion studies, with special reference to gravel-bedded rivers. J. Water Resour. Res. 1997, 8, 1993–2029. [Google Scholar] [CrossRef]
- Hooke, J.M. An analysis of the processes of riverbank erosion. J. Hydrol. 1979, 1–2, 39–62. [Google Scholar] [CrossRef]
- Lohnes, R.A.; Handy, R.L. Slope Angles in Friable Loess. J. Geol. 1968, 3, 247–258. [Google Scholar] [CrossRef]
- Osman, A.M.; Thorne, C.R. Riverbank Stability Analysis. I: Theory. J. Hydraul. Eng. 1988, 2, 134–150. [Google Scholar] [CrossRef]
- Simon, A.; Wolfe, W.J.; Molinas, A. Mass wasting algorithms in an alluvial channel model. In Proceedings of the 5th Federal Interagency Sedimentation Conference, Las Vegas, NV, USA, 18–21 March 1991; pp. 22–29. [Google Scholar]
- Taylor, D.W. Fundamentals of Soil Mechanics. Soil Sci. 1948, 2, 161. [Google Scholar] [CrossRef]
- Millar, R.G.; Quick, M.C. Discussion: Development and Testing of Riverbank-Stability Analysis. J. Hydraul. Eng. 1997, 11, 1051–1053. [Google Scholar] [CrossRef]
- Darby, S.E.; Thorne, C.R. Development and Testing of Riverbank-Stability Analysis. J. Hydraul. Eng. 1996, 8, 443–454. [Google Scholar] [CrossRef]
- Rinaldi, M.; Casagli, N. Stability of streambanks formed in partially saturated soils and effects of negative pore water pressures: The Sieve River (Italy). Geomorphology 1999, 4, 253–277. [Google Scholar] [CrossRef]
- Simon, A.; Curini, A.; Darby, S.E.; Langendoen, E.J. Bank and near-bank processes in an incised channel. Geomorphology 2000, 3–4, 193–217. [Google Scholar] [CrossRef]
- Huang, B.S.; Bai, Y.C.; Wan, Y.C. Model for dilapidation mechanism of riverbank. J. Hydraul. Eng. 2002, 9, 49–54. (In Chinese) [Google Scholar] [CrossRef]
- Wang, Y.G.; Kuang, S.F. Critical height of bank collapse. J. Hydraul. Eng. 2007, 10, 1158–1165. (In Chinese) [Google Scholar] [CrossRef]
- Xia, J.Q.; Wu, B.S.; Wang, Y.P.; Zhao, S.G. An analysis of soil composition and mechanical properties of riverbanks in a braided reach of the Lower Yellow River. Chin. Sci. Bull. 2008, 15, 2400–2409. [Google Scholar] [CrossRef]
- Thorne, C.R.; Tovey, N.K. Stability of composite riverbanks. Earth Surf. Proc. Land. 1981, 5, 469–484. [Google Scholar] [CrossRef]
- Hagerty, D.J. Piping/Sapping Erosion. I: Basic Considerations. J. Hydraul. Eng. 1991, 8, 991–1008. [Google Scholar] [CrossRef]
- Xia, J.Q.; Zong, Q.L.; Xu, Q.X.; Deng, C.Y. Soil properties and erosion mechanisms of composite riverbanks in Lower Jingjiang Reach. Adv. Water Sci. 2013, 6, 810–820. (In Chinese) [Google Scholar] [CrossRef]
- Nagata, N.; Hosoda, T.; Muramoto, Y. Numerical Analysis of River Channel Processes with Bank Erosion. J. Hydraul. Eng. 2000, 4, 243–252. [Google Scholar] [CrossRef]
- Darby, S.E.; Delbono, I. A model of equilibrium bed topography for meander bends with erodible banks. Earth Surf. Proc. Landf. 2002, 10, 1057–1085. [Google Scholar] [CrossRef]
- Simon, A.; Pollenbankhead, N.; Mahacek, V.; Langendoen, E. Quantifying reductions of mass-failure frequency and sediment loadings from streambanks using toe protection and other means: Lake Tahoe, United States. J. Am. Water Resour. Assoc. 2009, 1, 170–186. [Google Scholar] [CrossRef]
- Darby, S.E.; Trieu, H.Q.; Carling, P.A.; Sarkkula, J.; Koponen, J.; Kummu, M.; Conlan, I.; Leyland, J. A physically based model to predict hydraulic erosion of fine-grained riverbanks: The role of form roughness in limiting erosion. J. Geophys. Res. Earth Surf. 2010, 115, F04003. [Google Scholar] [CrossRef]
- Jia, D.D.; Shao, X.J.; Wang, H.; Zhou, G. Three-dimensional modeling of bank erosion and morphological changes in the Shishou bend of the middle Yangtze River. Adv. Water Res. 2010, 3, 348–360. [Google Scholar] [CrossRef]
- Nardi, L.; Rinaldi, M.; Solari, L. An experimental investigation on mass failures occurring in a riverbank composed of sandy gravel. Geomorphology 2012, 9, 56–59. [Google Scholar] [CrossRef]
- Xiao, Y.; Shao, X.J.; Zhou, G.; Zhou, J.Y. 2D mathematical modeling of fluvial processes considering influences of vegetation and bank erosion. J. Hydraul. Eng. 2012, 6, 149–153. (In Chinese) [Google Scholar]
- Xia, J.Q.; Wang, G.Q.; Wu, B.S. Evolution of Wandering Rivers and Its Numerical Simulation; Deng, Q., Zhang, J., Li, L., Eds.; China Water Conservancy and Hydropower Press: Beijing, China, 2005; pp. 182–188. ISBN 9787508426259. [Google Scholar]
- Jia, D.D.; Shao, X.J.; Wang, H.; Zhou, G. 3D mathematical modeling for fluvial process considering bank erosion. Adv. Water Sci. 2009, 3, 311–317. (In Chinese) [Google Scholar] [CrossRef]
- Yu, M.H.; Shen, K.; Wu, S.B.; Wei, H.Y. An experimental study of interaction between bank collapse and riverbed evolution. Adv. Water Sci. 2013, 5, 675–682. (In Chinese) [Google Scholar] [CrossRef]
- Yu, M.H.; Guo, X. Experimental study on the interaction between the hydraulic transport of failed bank soil and near-bank bed evolution. Adv. Water Sci. 2014, 5, 677–683. (In Chinese) [Google Scholar] [CrossRef]
- Wu, S.B.; Yu, M.H. Experimental study on bank failure process and interaction with riverbed deformation due to fluvial hydraulic force. J. Hydraul. Eng. 2014, 6, 649–657. (In Chinese) [Google Scholar] [CrossRef]
- Deng, S.S.; Xia, J.Q.; Zhou, M.R.; Li, J. Conceptual model of bank retreat processes in the Upper Jingjiang Reach. Chin. Sci. Bull. 2016, 33, 3606–3615. (In Chinese) [Google Scholar] [CrossRef]
- Yu, M.H.; Chen, X.; Wei, H.Y.; Hu, C.W.; Wu, S.B. Experiment of the influence of different near-bank riverbed compositions on bank failure. Adv. Water Sci. 2016, 2, 176–185. (In Chinese) [Google Scholar] [CrossRef]
- Duan, G.S.; Shu, A.; Matteo, R.; Wang, S.; Zhu, F.Y. Collapsing mechanisms of the typical cohesive riverbank along the Ningxia-Inner Mongolia catchment. Water 2018, 9, 1272. [Google Scholar] [CrossRef]
- Xue, Y. Basic Course of AutoCAD 2010; Tsinghua University Press: Beijing, China, 2009; pp. 113–135. ISBN 9787302207504. (In Chinese) [Google Scholar]
- Dong, Y.H. Review on 10 groups of demarcating grain sizes of river sediment. J. Yangtze Sci. Res. Inst. 2018, 2, 1–7. (In Chinese) [Google Scholar] [CrossRef]
- Qian, N.; Wan, Z.H. Sediment Motion Mechanics; Yang, J., Ed.; Science Press: Beijing, China, 1983; pp. 265–268. ISBN 9787030112606. [Google Scholar]
- Qian, N.; Zhang, R.J.; Zhou, Z.D. Riverbed Evolution; Zhu, S., Ed.; Science Press: Beijing, China, 1987; pp. 258–269. ISBN 13031-3498. [Google Scholar]
- Shu, A.; Wang, L.; Yang, K.; Fei, X.J. Investigation on movement characteristics for non-homogeneous and solid-liquid two-phase debris flow. Chin. Sci. Bull. 2010, 31, 3006–3012. (In Chinese) [Google Scholar] [CrossRef]
- Shu, A.; Zhang, Z.D.; Wang, L.; Fei, X.J. Method for determining the critical grain size of viscous debris flow based on energy dissipation principle. J. Hydraul. Eng. 2008, 3, 257–263. (In Chinese) [Google Scholar] [CrossRef]
- Fei, X.J. A model for calculating viscosity of sediment carrying flow in the middle and lower Yellow River. J. Sediment. Res. 1991, 2, 1–13. (In Chinese) [Google Scholar] [CrossRef]


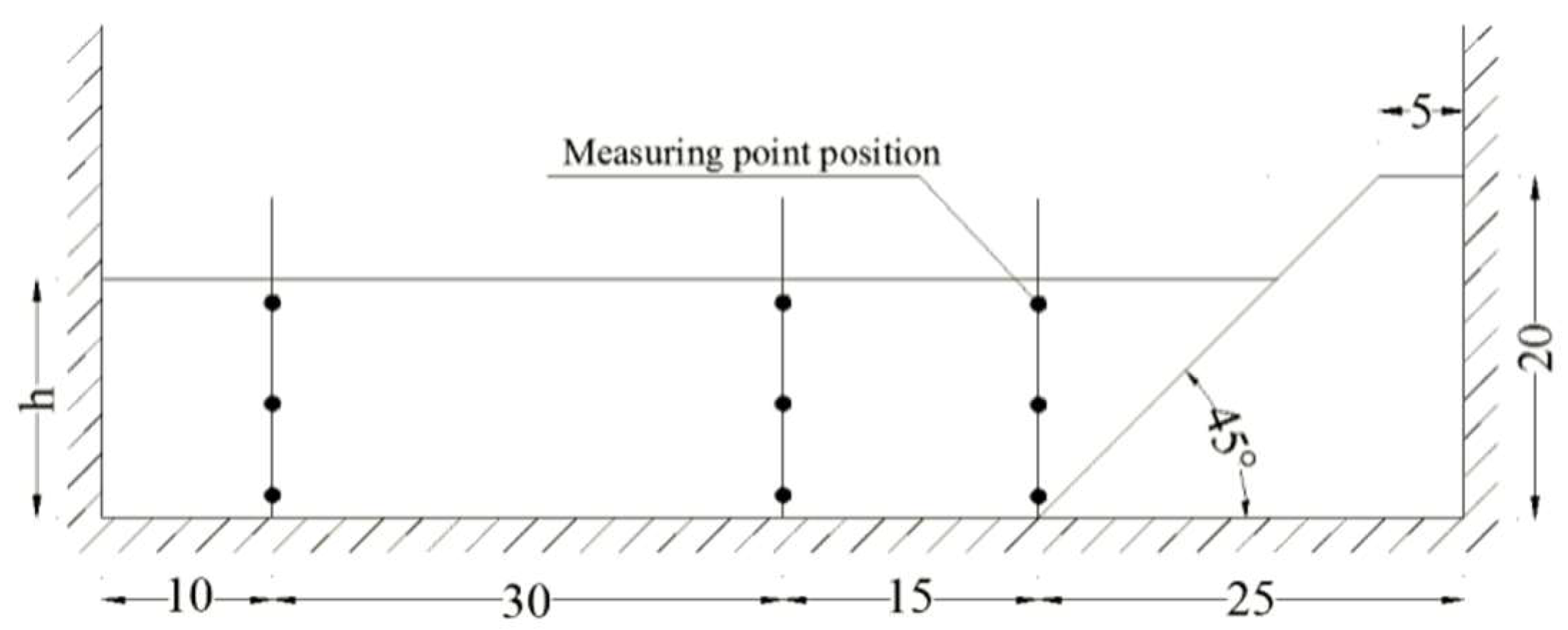
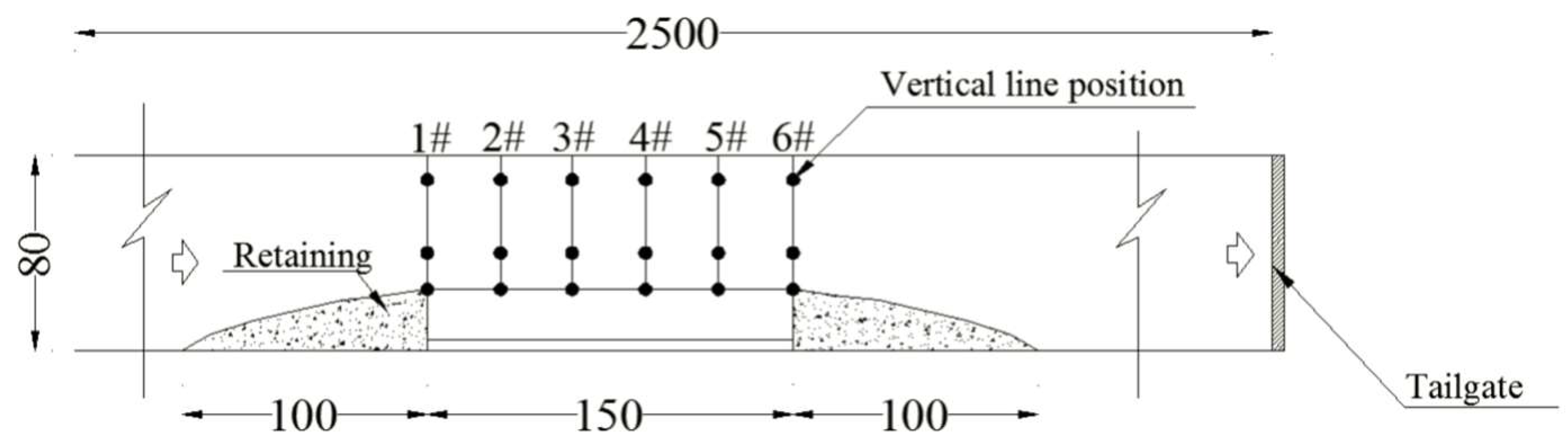






| Group | Slope Degree (°) | Bank Morphology | Flux (L/s) | Water Discharge Time (min) | Water Level (cm) | ||
|---|---|---|---|---|---|---|---|
| Bank Top Width (cm) | Bank Height (cm) | Bank Toe Width (cm) | |||||
| No.1 | 45 | 5 | 20 | 25 | 45 | 40 | 11.2 |
| 60 | 30 | 13.2 | |||||
| No.2 | 60 | 13.45 | 20 | 25 | 45 | 40 | 11.5 |
| 60 | 30 | 12.8 | |||||
| No.3 | 75 | 19.64 | 20 | 25 | 45 | 40 | 11.8 |
| 60 | 30 | 13.9 | |||||
| No.4 | 90 | 25 | 20 | 25 | 45 | 40 | 11.25 |
| 60 | 30 | 13 | |||||
| Group | Soil Position | Moisture Content (%) | Unit Weight (g/cm3) | Cohesion (kPa) | Friction Angle (°) |
|---|---|---|---|---|---|
| No.1 | Bank toe | 15.50 | 1.83 | 14.77 | 19.46 |
| Bank top | 15.33 | 1.82 | 14.45 | 19.19 | |
| No.2 | Bank toe | 15.50 | 2.01 | 14.77 | 19.46 |
| Bank top | 15.33 | 1.76 | 14.45 | 19.19 | |
| No.3 | Bank toe | 14.92 | 1.66 | 13.66 | 18.54 |
| Bank top | 14.54 | 1.58 | 12.93 | 17.92 | |
| No.4 | Bank toe | 14.98 | 1.80 | 13.77 | 18.63 |
| Bank top | 15.80 | 1.61 | 15.37 | 19.96 |
| Group | Slope Gradient (°) | Flow Rate (L/s) | Average Water Level (cm) | Average Flow Rate (m/s) | Incipience Motion Particle Size (Lower Limits) (μm) | Incipience Motion Particle Size (Upper Limits) (mm) | Incipience Motion Percentage (%) |
|---|---|---|---|---|---|---|---|
| No.1 | 45 | 45 | 12.40 | 0.62 | 9.25 | 7.29 | 89.85 |
| 60 | 13.25 | 0.70 | 7.23 | 7.84 | 91.46 | ||
| No.2 | 60 | 45 | 11.50 | 0.69 | 7.46 | 8.95 | 91.06 |
| 60 | 12.80 | 0.74 | 6.38 | 10.42 | 92.92 | ||
| No.3 | 75 | 45 | 11.80 | 0.65 | 8.48 | 8.88 | 90.56 |
| 60 | 13.90 | 0.72 | 6.88 | 10.63 | 92.06 | ||
| No.4 | 90 | 45 | 11.25 | 0.65 | 8.20 | 9.52 | 90.81 |
| 60 | 13.00 | 0.77 | 5.98 | 11.10 | 93.60 |
| Group | Slope Gradient (°) | Collapse Time (min) | Collapse Amount (kg) | Average Collapse Magnitude (g/s) |
|---|---|---|---|---|
| No.1 | 45 | 29 | 38.87 | 9.25 |
| No.2 | 60 | 27 | 41.58 | 9.90 |
| No.3 | 75 | 23 | 62.45 | 14.87 |
| No.4 | 90 | 16 | 82.43 | 19.63 |
| Group | Slope Degree (°) | Flow Rate (L/s) | Critical Particle Size (mm) |
|---|---|---|---|
| No.1 | 45 | 45 | 0.06 |
| 60 | 0.062 | ||
| No.2 | 60 | 45 | 0.064 |
| 60 | 0.062 | ||
| No.3 | 75 | 45 | 0.068 |
| 60 | 0.066 | ||
| No.4 | 90 | 45 | 0.068 |
| 60 | 0.068 |
| Group | Bank Slope | Flow Rate (L/s) | Deposited Sediment (%) | Suspended Sediment Percentage (%) | Bed Load Percentage (%) |
|---|---|---|---|---|---|
| No.1 | 45° | 45 | 10.15 | 70.35 | 19.50 |
| 60 | 8.54 | 71.61 | 19.85 | ||
| No.2 | 60° | 45 | 8.94 | 75.76 | 15.30 |
| 60 | 7.08 | 77.31 | 15.61 | ||
| No.3 | 75° | 45 | 9.44 | 70.91 | 19.65 |
| 60 | 7.94 | 72.08 | 19.98 | ||
| No.4 | 90° | 45 | 8.39 | 73.38 | 18.23 |
| 60 | 9.06 | 74.16 | 16.78 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shu, A.; Duan, G.; Rubinato, M.; Tian, L.; Wang, M.; Wang, S. An Experimental Study on Mechanisms for Sediment Transformation Due to Riverbank Collapse. Water 2019, 11, 529. https://doi.org/10.3390/w11030529
Shu A, Duan G, Rubinato M, Tian L, Wang M, Wang S. An Experimental Study on Mechanisms for Sediment Transformation Due to Riverbank Collapse. Water. 2019; 11(3):529. https://doi.org/10.3390/w11030529
Chicago/Turabian StyleShu, Anping, Guosheng Duan, Matteo Rubinato, Lu Tian, Mengyao Wang, and Shu Wang. 2019. "An Experimental Study on Mechanisms for Sediment Transformation Due to Riverbank Collapse" Water 11, no. 3: 529. https://doi.org/10.3390/w11030529
APA StyleShu, A., Duan, G., Rubinato, M., Tian, L., Wang, M., & Wang, S. (2019). An Experimental Study on Mechanisms for Sediment Transformation Due to Riverbank Collapse. Water, 11(3), 529. https://doi.org/10.3390/w11030529






