Effects of Temperature Change on the Soil Water Characteristic Curve and a Prediction Model for the Mu Us Bottomland, Northern China
Abstract
:1. Introduction
2. Materials and Methods
2.1. Soil-Water Characteristic Curve Model at Different Temperatures
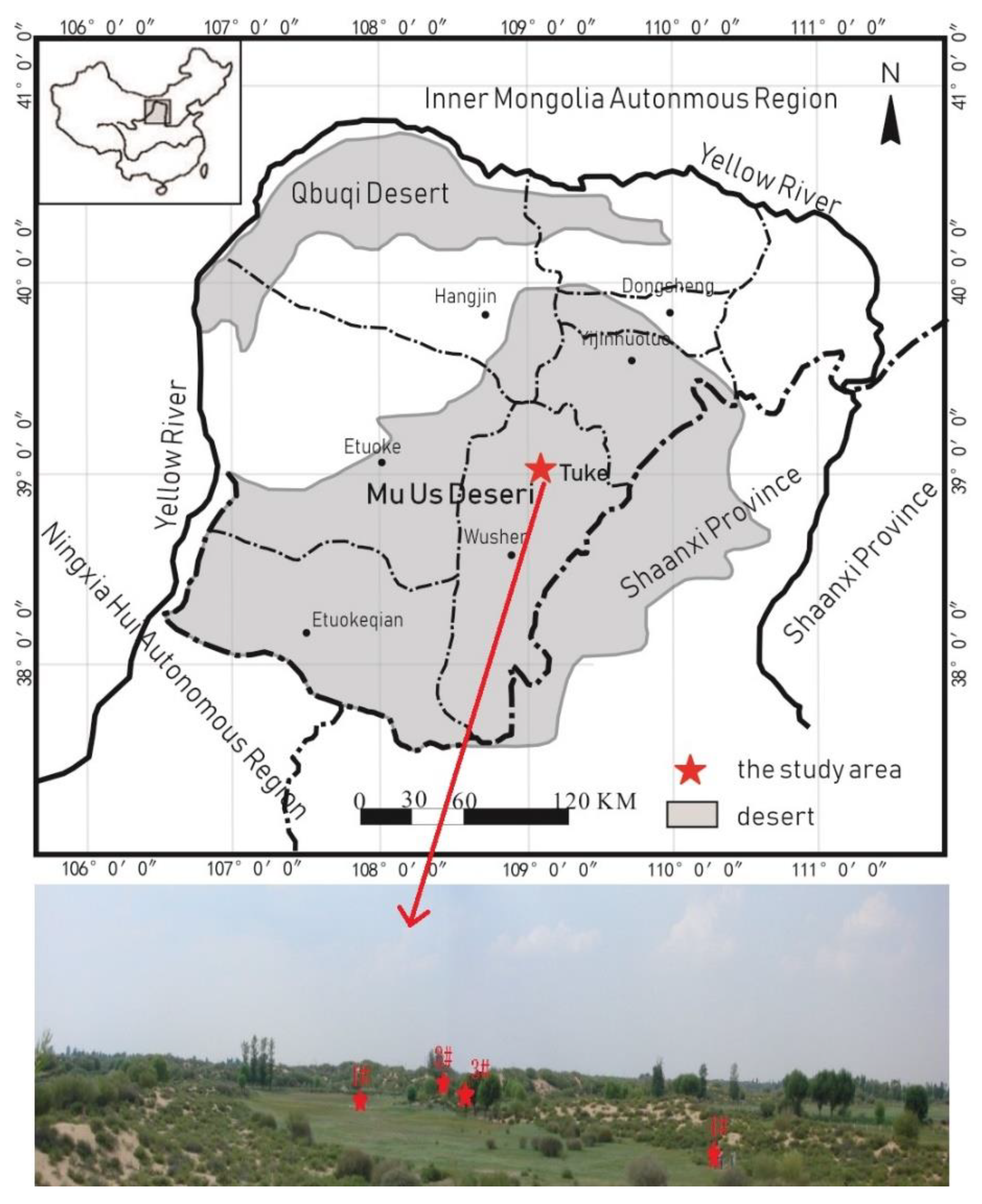
2.2. Sample Collection
2.3. Experimental Setup
2.3.1. Soil Column Experiment
2.3.2. Ku-pF Experiment
3. Results and Discussion
3.1. Results from the Two Devices without Any Temperature Effect
3.2. Results of Soil Column Experiments at Different Temperatures
3.3. Results of Ku-pF Experiments at Different Temperatures
3.4. Prediction Model for the Soil-Water Characteristic Curve (SWCC) at Different Temperatures and Error Analysis
3.5. Effect of Temperature on the Unsaturated Hydraulic Conductivity of Undisturbed Soil
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Shao, M.A.; Horton, R. Integral method for estimating soil hydraulic properties. Soil Sci. Soc. Am. J. 1998, 62, 585–592. [Google Scholar] [CrossRef]
- Chahal, R.S. Effect of temperature and trapped air on the energy status of water in porous media. Soil Sci. 1964, 98, 107–112. [Google Scholar] [CrossRef]
- Chahal, R.S. Effect of temperature and trapped air on matric suction. Soil Sci. 1965, 100, 262–266. [Google Scholar] [CrossRef]
- Haridasan, M.; Jensen, R.D. Effect of Temperature on Pressure Headwater Content Relationship and Conductivity of Two Soils. Soil Sci. Soc. Am. J. 1972, 36, 861–865. [Google Scholar] [CrossRef]
- Hopmans, J.; Dane, J.H. Effect of temperature-dependent hydraulic properties on soil water movement. Soil Sci. Soc. Am. J. 1985, 49, 51–58. [Google Scholar] [CrossRef]
- Li, P.; Li, T.L.; Wang, H.; Liang, Y. Soil-water characteristic curve and permeability perdiction on Childs & Collis-Geroge model of unsaturated loess. Rock Soil Mech. 2013, 34, 184–189. (In Chinese) [Google Scholar]
- Luo, P.P.; He, B.; Takara, K.; Xiong, Y.E.; Nover, D.; Duan, W.; Fukushi, K. Historical Assessment of Chinese and Japanese Flood Management Policies and Implications for Managing Future Floods. Environ. Sci. Policy 2015, 48, 265–277. [Google Scholar] [CrossRef]
- Luo, P.P.; Mu, D.R.; Xue, H.; Ngo-Duc, T.; Dang-Dinh, K.; Takara, K.; Nover, D.; Schladow, G. Flood inundation assessment for the Hanoi Central Area, Vietnam under historical and extreme rainfall conditions. Sci. Rep. 2018, 8. [Google Scholar] [CrossRef]
- Luo, P.P.; Zhou, M.M.; Deng, H.Z.; Lyu, J.Q.; Cao, W.Q.; Takara, K.; Nover, D.; Schladow, S.G. Impact of forest maintenance on water shortages: Hydrologic modeling and effects of climate change. Sci. Total Environ. 2018, 615, 1355–1363. [Google Scholar] [CrossRef]
- Luo, P.P.; He, A.B.; Duan, W.; Takara, K.; Nover, D. Impact assessment of rainfall scenarios and land-use change on hydrologic response using synthetic Area IDF curves. J. Flood Risk Manag. 2015, 11, S84–S97. [Google Scholar] [CrossRef]
- Gardner, R. Relation of temperature to moisture tension of soil. Soil Sci. 1955, 79, 257–265. [Google Scholar] [CrossRef]
- Nimmo, J.R.; Miller, E.E. The temperature isothermal moisture vs potential characteristics dependence of soils. Soil Sci. Soc. Am. J. 1986, 50, 1105–1113. [Google Scholar] [CrossRef]
- Philip, J.R.; Vries, D.A.D. Moisture movement in porous materials at temperature gradients. Trans. Am. Geophys. Union 1957, 38, 222–232. [Google Scholar] [CrossRef]
- Wang, T.H.; Lu, J.; Yue, C.K. Soil-water characteristic curve for unsaturated loess considering temperature and density effect. Rock Soil Mech. 2008, 29, 1–5. [Google Scholar] [CrossRef]
- Tong, F.G. Numerical Modeling of Coupled Thermo-Hydro-Mechanical Processes of Geological Porous Media; Royal Institute of Technology: Stockholm, Sweden, 2010. [Google Scholar]
- Wilkinson, G.E.; Klute, A. The temperature effect on the equilibrium energy status of water held by porous media. Soil Sci. Soc. Am. J. 1962, 26, 326–329. [Google Scholar] [CrossRef]
- Hopmans, J.W.; Dane, J.H. Temperature dependence of soil hydraulic properties. Soil Sci. Soc. Am. J. 1986, 50, 4–9. [Google Scholar] [CrossRef]
- Constantz, J. Comparison of isothermal and isobaric water retention paths in nonswelling porous materials. Water Resour. Res. 1991, 7, 3165–3170. [Google Scholar] [CrossRef]
- Liu, H.H.; Dane, J.H. Reconciliation between Measured and Theoretical Temperature Effects on Soil Water Retention Curves. Soil Sci. Soc. Am. J. 1993, 57, 1202–1207. [Google Scholar] [CrossRef]
- She, H.Y.; Sleep, B.E. The effect of temperature on capillary pressure-saturation relationships for air-water and perchloroethylene-water systems. Water Resour. Res. 1998, 34, 2587–2597. [Google Scholar] [CrossRef]
- Bachmann, J.; Horton, R.; Grant, S.A.; Van der Ploeg, R.R. Temperature Dependence of Water Retention Curves for Wettable and Water-Repellent Soils. Soil Sci. Soc. Am. J. 2002, 66, 44–52. [Google Scholar] [CrossRef]
- Jacinto, A.C.; Villar, M.V.; Gómez-Espina, R.; Ledesma, A. Adaptation of Van Genuchten expression to the effects of temperature and density for compacted bentonites. Appl. Clay Sci. 2009, 42, 575–582. [Google Scholar] [CrossRef]
- Grant, S.A.; Salehzadeh, A. Calculation of Temperature Effects on Wetting Coefficients of Porous Solids and Their Capillary Pressure Functions. Water Resour. Res. 1996, 32, 261–270. [Google Scholar] [CrossRef]
- Remero, E.; Gens, A.; Lloret, A. Temperature effects of the hydraulic behavior of an unsaturated clay. Geotech. Geol. Eng. 2001, 19, 311–332. [Google Scholar] [CrossRef]
- Villar, M.V.; Lloret, A. Influence of temperature on the hydro-mechanical behavior of a compacted bentonite. Appl. Clay Sci. 2004, 26, 337–350. [Google Scholar] [CrossRef]
- Villar, M.V.; Roberto, G.E. Retention curves of two bentonites at high temperatures. In Experimental Unsaturated Soil Mechanics; Springer: Berlin/Heidelberg, Germany, 2007; Volume 112, pp. 267–274. [Google Scholar] [CrossRef]
- Tang, A.M.; Cui, Y.J. Controlling suction by the vapour equilibrium technique at different temperatures and its application in determining the water retention properties of MX-80 clay. Can. Geotech. J. 2005, 42, 287–296. [Google Scholar] [CrossRef]
- Brooks, R.H.; Corey, A.J. Hydraulic Properties of Porous Media; Colorado State University: Fort Collins, CO, USA, 1964. [Google Scholar]
- Gardner, W.R.; Hillel, D.; Benyamini, Y. Post-Irrigation Movement of Soil Water: 1. Redistribution. Water Resour. Res. 1970, 6, 851–861. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Russo, D. Determining soil hydraulic properties by parameter estimation: On the selection of a model for the hydraulic properties. Water Resour. Res. 1988, 24, 453–459. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Xing, A.Q. Equations for the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 521–532. [Google Scholar] [CrossRef]
- Xu, S.H.; Zhang, J.B.; Liu, J.L. Suitability of models describing soil water retention curve. Acta Pedol. Sin. 2002, 39, 498–504. [Google Scholar] [CrossRef]
- Ma, C.; Hueckel, T. Stress and pore pressure in saturated clay subjected to heat from radioactive waste: A numerical simulation. Can. Geotech. J. 1992, 29, 1087–1094. [Google Scholar] [CrossRef]
- Grant, S.A. Extension of temperature effects model for capillary pressure saturation relations. Water Resour. Res. 2003, 39, 1003. [Google Scholar] [CrossRef]
- Sun, J.M. Origin of eolian sand mobilization during the past 2300 years in the Mu Us Desert. China Quat. Res. 2000, 53, 78–88. [Google Scholar] [CrossRef]
- Li, J.T. Negative Pressure Transducer of the Vadose Zone. Chinese Patent No. 201220063548, 2006. Available online: http://www1.soopat.com/Patent/201220063548 (accessed on 12 October 2018).
- Li, J.T. Vadose Zone Parameter Tester. Chinese Patent No. 201210044373, 2012. Available online: http://www1.soopat.com/Home/Result?SearchWord=201210044373 (accessed on 12 October 2018).
- Jürgen, P.; UGT GmbH; Jens, B. Ku-pF Apparatus Operating Instructions; Umwelt-Geräte-Technik: Freising, Germany, 2013; Available online: https://www.ugt-online.de/home/ (accessed on 20 April 2019).
- Schneider, M.; Goss, K.U. Temperature dependence of the water retention curve for dry soils. Water Resour. Res. 2011, 47, 5. [Google Scholar] [CrossRef]
- Arifin, Y.F.; Agus, S.S.; Schanz, T. Temperature effects on suction characteristic curve of bentonite-sand mixtures. In Proceedings of the 4th International Conference on Unsaturated Soils, Carefree, AZ, USA, 2–6 April 2006; pp. 1314–1325. [Google Scholar] [CrossRef]
- Gao, K. Study on the Effect of Temperature on Soil Water Characteristic Curve for Unsaturated Soils; Three Gorges University: Yichang, China, 2014. (In Chinese) [Google Scholar]
- Zhang, Y.P.; Bai, J.L.; Zhang, J.C. A study of the temperature effect on soil water potential. Acta Pedol. Sin. 1990, 454–458. [Google Scholar]
- Constantz, J. Temperature dependence of unsaturated hydraulic conductivity of two soils. Soil Sci. Soc. Am. J. 1982, 46, 466–470. [Google Scholar] [CrossRef]
- Gao, H.B.; Shao, M.A. Effect of temperature changes on soil hydraulic properties. Soil Tillage Res. 2015, 153, 145–154. [Google Scholar] [CrossRef]





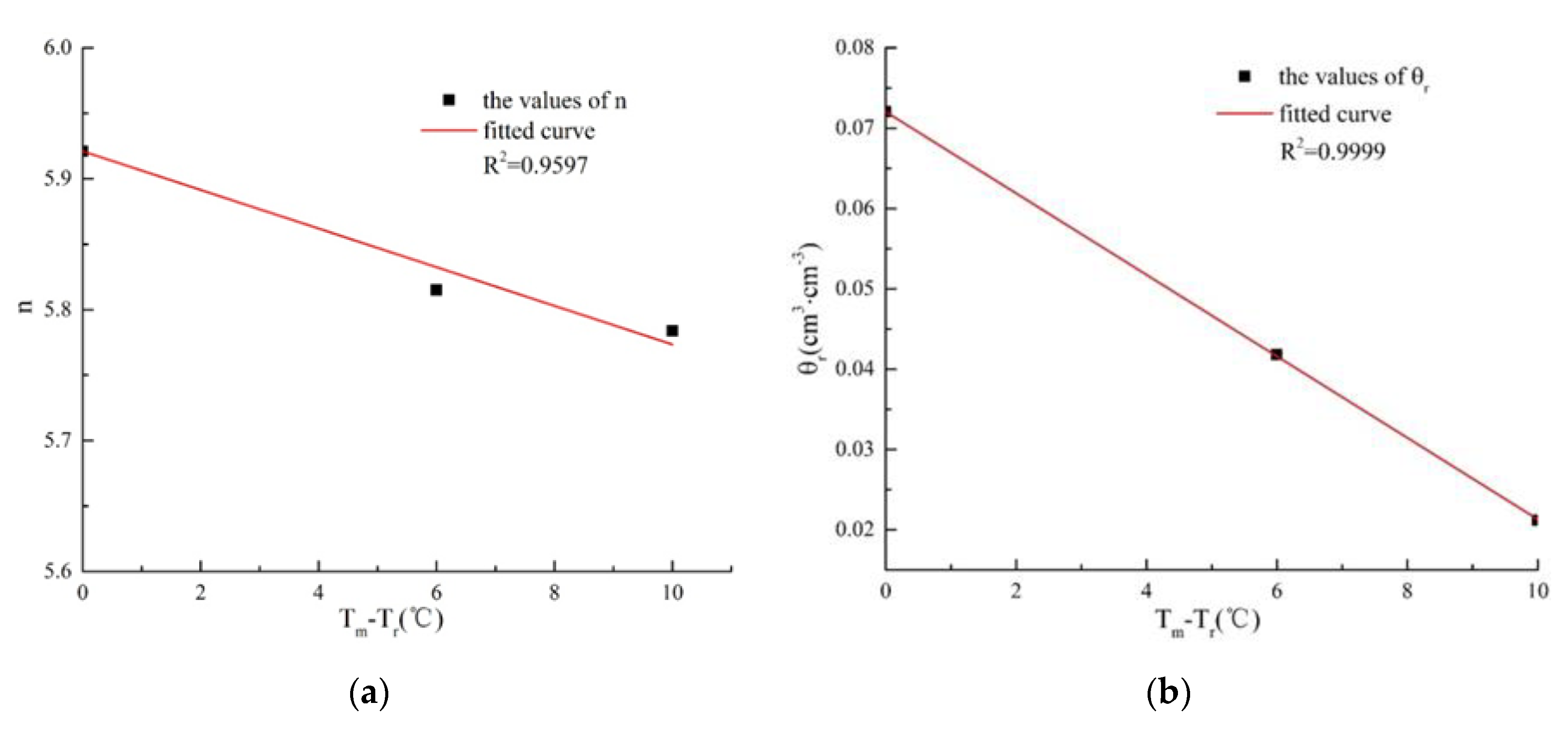


| Temperature (°C) | θr | θs | α | n | R2 | RMSE |
|---|---|---|---|---|---|---|
| 13 | 0.1097 | 0.3661 | 0.0313 | 10.65 | 0.9956 | 0.002493 |
| 18 | 0.1143 | 0.351 | 0.0307 | 10.72 | 0.998 | 0.002193 |
| 23 | 0.1077 | 0.3508 | 0.0311 | 9.31 | 0.9953 | 0.002364 |
| 27 | 0.1073 | 0.3437 | 0.0306 | 9.62 | 0.9962 | 0.002365 |
| 30 | 0.1012 | 0.3455 | 0.0312 | 8.189 | 0.9963 | 0.001904 |
| Temperature (°C) | θr | θs | α | n | R2 | RMSE |
|---|---|---|---|---|---|---|
| 13 | 0.0739 | 0.3993 | 0.0409 | 3.798 | 0.9760 | 0.0139 |
| 18 | 0.0433 | 0.3585 | 0.0237 | 8.736 | 0.9143 | 0.0324 |
| 23 | 0.0219 | 0.4028 | 0.01656 | 5.739 | 0.9861 | 0.0128 |
| T (°C) | θr | θs | α | n | R2 | RMSE |
|---|---|---|---|---|---|---|
| 13 | 0.07203 | 0.3919 | 0.03282 | 5.921 | 0.9755 | 0.006541 |
| 18 | 0.0418 | 0.3684 | 0.0287 | 5.815 | 0.9872 | 0.009596 |
| 23 | 0.02118 | 0.3642 | 0.01595 | 5.784 | 0.9829 | 0.01275 |
| Parameters | n0 | θs0 | θr0 | β | κn | λs | λr |
|---|---|---|---|---|---|---|---|
| 13 °C | 5.921 | 0.3919 | 0.072 | 1.41286 | −0.01475 | −0.00307 | −0.00507 |
| R2 | 0.8028 | 0.9597 | 0.9219 | 0.9999 |
| T (°C) | 13 | 18 | 23 | 28 | Scaling Factor | |
|---|---|---|---|---|---|---|
| θ (%) | ||||||
| 0.1 | 0.03 | 0.17 | 0.33 | 0.52 | 0.228 | |
| 0.15 | 0.22 | 0.58 | 0.94 | 1.28 | 0.394 | |
| 0.2 | 1.62 | 2.98 | 3.68 | 4.05 | 0.559 | |
| 0.3 | 5.48 | 9.41 | 10.46 | 10.74 | 0.891 | |
| 0.35 | 10.34 | 15.16 | 17.07 | 17.82 | 1.057 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiao, X.; Ma, S.; Pan, G.; Liu, G. Effects of Temperature Change on the Soil Water Characteristic Curve and a Prediction Model for the Mu Us Bottomland, Northern China. Water 2019, 11, 1235. https://doi.org/10.3390/w11061235
Qiao X, Ma S, Pan G, Liu G. Effects of Temperature Change on the Soil Water Characteristic Curve and a Prediction Model for the Mu Us Bottomland, Northern China. Water. 2019; 11(6):1235. https://doi.org/10.3390/w11061235
Chicago/Turabian StyleQiao, Xiaoying, Shaoyang Ma, Guixing Pan, and Guanglu Liu. 2019. "Effects of Temperature Change on the Soil Water Characteristic Curve and a Prediction Model for the Mu Us Bottomland, Northern China" Water 11, no. 6: 1235. https://doi.org/10.3390/w11061235




