A Calibrated, Watershed-Specific SCS-CN Method: Application to Wangjiaqiao Watershed in the Three Gorges Area, China
Abstract
1. Introduction
2. The Proposed Calibrated Watershed-Specific - Method
- Perform bootstrap, BCa procedure and normality test in SPSS (version 18.0 or an equivalent statistics software) for .
- Check the normality test results of to see whether it is normally distributed or not:
- (a)
- If yes, refer to the mean BCa confidence interval for S optimization.
- (b)
- Otherwise, refer to the median BCa confidence interval for S optimization.
- Check the normality test results of to see whether it is normally distributed or not:
- (a)
- If yes, refer to the mean BCa confidence interval for optimization.
- (b)
- Otherwise, refer to the median BCa confidence interval for optimization.
- Substitute the and value into Equation (1) to form a calibrated SCS runoff model.
- Given and , compute with Equation (8).
- Given and , compute with Equation (8).
- Correlate and to form a S correlation equation.
- Substitute the S correlation equation into Equation (4) to derive .
3. Application to Wangjiaqiao Watershed in the Three Gorges Area, China
3.1. Study Site and Rainfall-Runoff Dataset
3.2. Runoff Model Assessment
3.3. Results and Discussion
3.3.1. Inferential Statistics Assessment to Obtain Optimum and S
3.3.2. Watershed-Specific S Correlation Equation and for Wangjiaqiao Watershed in China
3.3.3. Asymptotic of Wangjiaqiao Watershed
3.3.4. Residual Modeling and the Corrected Equation
3.3.5. Comparison of Runoff Prediction Models
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| SCS-CN | Soil Conservation Service Curve Number |
| NEH | National Engineering Handbook |
| Curve Number | |
| Initial Abstraction Ratio | |
| Q | Runoff Depth |
| S | Maximum potential water retention amount |
| P | The rainfall depth |
| BCa | Bias corrected and accelerated |
| S value of different | |
| Conjugate Curve Number | |
| Runoff prediction differences | |
| E | Nash-Sutcliffe index |
| Model residual sum of square errors | |
| Overall model prediction error | |
| CI | Confidence Interval |
| SPSS | IBM statistical software SPSS |
| Adj | Adjusted |
| AFM | Asymptotic fitting method |
References
- Wang, Z.; Jiao, J.; Rayburg, S.; Wang, Q.; Su, Y. Soil erosion resistance of “Grain for Green” vegetation types under extreme rainfall conditions on the Loess Plateau, China. Catena 2016, 141, 109–116. [Google Scholar] [CrossRef]
- Zhou, J.; Fu, B.; Gao, G.; Lü, Y.; Liu, Y.; Lü, N.; Wang, S. Effects of precipitation and restoration vegetation on soil erosion in a semi-arid environment in the Loess Plateau, China. Catena 2016, 137, 1–11. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, X.P.; Abla, M.; Lu, D.; Yan, R.; Ren, Q.F.; Ren, Z.Y.; Yang, Y.H.; Zhao, W.H.; Lin, P.F. Effects of vegetation and rainfall types on surface runoff and soil erosion on steep slopes on the Loess Plateau, China. Catena 2018, 170, 141–149. [Google Scholar] [CrossRef]
- Merritt, W.S.; Letcher, R.A.; Jakeman, A.J. A review of erosion and sediment transport models. Environ. Model. Softw. 2003, 18, 761–799. [Google Scholar] [CrossRef]
- Beven, K. Changing ideas in hydrology—The case of physically based models. J. Hydrol. 1989, 105, 157–172. [Google Scholar] [CrossRef]
- Ferro, V.; Minacapilli, M. Sediment delivery processes at basin scale. Hydrol. Sci. J. 1995, 40, 703–717. [Google Scholar] [CrossRef]
- Loague, K.; Freeze, R.A. Comparison of rainfall-runoff modeling techniques on small upland catchments. Water Resour. Res. 1985, 21, 229–248. [Google Scholar] [CrossRef]
- Perrin, C.; Michel, C.; Andreassian, V. Does a large number of parameters enhance model performance? Comparative assessment of common catchment model structures on 429 catchments. J. Hydrol. 2001, 242, 275–301. [Google Scholar] [CrossRef]
- Steefel, C.I.; Van Cappellan, P. Reactive transport modeling of natural systems. J. Hydrol. 1998, 209, 1–7. [Google Scholar]
- Kleissen, F.; Beck, M.; Wheater, H. The identifiability of conceptual hydrochemical models. Water Resour. Res. 1990, 26, 2979–2992. [Google Scholar] [CrossRef]
- SCS National Engineering Handbook (SCS NEH). Section 4, Hydrology, Chapter 21. In National Engineering Handbook; SCS: Washington, DC, USA, 1965. [Google Scholar]
- Shi, P.J.; Yuan, Y.; Zheng, J.; Wang, J.A.; Ge, Y.; Qiu, G.Y. The effect of land use/cover change on surface runoff in Shenzhen region, China. Catena 2007, 69, 31–35. [Google Scholar] [CrossRef]
- Shi, Z.H.; Chen, L.D.; Fang, N.F.; Qin, D.F.; Cai, C.F. Research on the SCS-CN initial abstraction ratio using rainfall- runoff event analysis in the Three Gorges Area, China. Catena 2009, 77, 1–7. [Google Scholar] [CrossRef]
- Jain, M.K.; Mishra, S.K.; Babu, S.; Singh, V.P. Enhanced runoff curve number model incorporating storm duration and a non-linear Ia-S relation. J. Hydrol. Eng. ASCE 2006, 11, 631–635. [Google Scholar] [CrossRef]
- Ponce, V.M.; Hawkins, R.H. Runoff curve number: Has it reached maturity? J. Hydrol. Eng. 1996, 1, 11–19. [Google Scholar] [CrossRef]
- Mishra, S.K.; Singh, V.P. Long-term hydrological simulation based on the soil conservation service curve number. Hydrol. Process 2004, 18, 1291–1313. [Google Scholar] [CrossRef]
- Baltas, E.A.; Dervos, N.A.; Mimikou, M.A. Technical note: Determination of the SCS initial abstraction ratio in an experimental watershed in Greece. Hydrol. Earth Syst. Sci. 2007, 11, 1825–1829. [Google Scholar] [CrossRef]
- Hawkins, R.H.; Ward, T.J.; Woodward, D.E.; Van Mullem, J.A. Curve Number Hydrology: State of Practice; American Society of Civil Engineers: Reston, VI, USA, 2009. [Google Scholar]
- Fu, S.; Zhang, G.; Wang, N.; Luo, L. Initial abstraction ratio in the SCS-CN method in the Loess Plateau of China. Trans. ASABE 2011, 54, 163–169. [Google Scholar] [CrossRef]
- D’Asaro, F.; Grillone, G. Empirical investigation of curve number method parameters in the Mediterranean area. J. Hydrol. Eng 2012, 17, 1141–1152. [Google Scholar] [CrossRef]
- D’Asaro, F.; Grillone, G.; Hawkins, R.H. Curve number: Empirical evaluation and comparison with curve number handbook tables in Sicily. J. Hydrol. Eng. 2014, 19. [Google Scholar] [CrossRef]
- Singh, P.K.; Mishra, S.K.; Berndtsson, R.; Jain, M.K.; Pandey, R.P. Development of a modified SMA based MSCS-CN model for runoff estimation. Water Resour. Manag. 2015, 29, 4111–4127. [Google Scholar] [CrossRef]
- Ross, C.; Prihodko, L.; Anchang, J.; Kumar, S.; Ji, W.; Hanan, N. HYSOGs250m, global gridded hydrologic soil groups for curve-number-based runoff modeling. Sci. Data 2018, 5, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Jaafar, H.; Ahmad, F.; El Beyrouthy, N. GCN250, new global gridded curve numbers for hydrologic modeling and design. Sci. Data 2019, 6, 1–8. [Google Scholar] [CrossRef] [PubMed]
- National Engineering Handbook (NEH). Part 630 Hydrology. In National Engineering Handbook; USDA: Washington, DC, USA, 2004. [Google Scholar]
- Efron, B.; Tibshirani, R. An Introduction to the Bootstrap; Chapman & Hall/CRC: Boca Raton, FL, USA, 1994; ISBN 978-0-412-04231-7. [Google Scholar]
- Davison, A.C.; Hinkley, D.V. Bootstrap methods and their application. In Cambridge Series in Statistical and Probabilistic Mathematics; Cambridge University Press: Cambridge, UK, 1997; ISBN 0-521-57391-2. [Google Scholar]
- Efron, B. Large-Scale Inference: Empirical Bayes Methods for Estimation, Testing and Prediction; Institute of Mathematical Statistics Monographs, Cambridge University Press: Cambridge, UK, 2010; ISBN 978-0-521-19249-1. [Google Scholar]
- Rochoxicz, J.A., Jr. Bootstrapping Analysis, Inferential Statistics and EXCEL. Spreadsheets Educ. 2011, 4, 4. [Google Scholar]
- Schneider, L.E.; McCuen, R.H. Statistical guidelines for curve number generation. J. Irrig. Drain. Eng. 2005, 131, 282–290. [Google Scholar] [CrossRef]
- Andy, F. Discovering Statistics Using IBM SPSS, 4th ed.; SAGE Publications: Thousand Oaks, CA, USA, 2013; pp. 595–609. [Google Scholar]
- Nash, J.; Sutcliffe, J. River flow forecasting through conceptual models part 1 - A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Moriasi, D.N.; Arnold, J.G.; Van Liew, M.W.; Bingner, R.L.; Harmel, R.D.; Veith, T.L. Model evaluation guidelines for systematic quantification of accuracy in watershed simulations. Trans. ASABE 2007, 50, 885–900. [Google Scholar] [CrossRef]
- Hawkins, R.H. Asymptotic determination of runoff curve numbers from data. J. Irrig. Drain. Eng. 1993, 119, 334–345. [Google Scholar] [CrossRef]
- Soulis, K.X.; Valiantzas, J.D.; Dercas, N.; Londra, P.A. Investigation of the direct runoff generation mechanism for the analysis of the SCS-CN method applicability to a partial area experimental watershed. Hydrol. Earth Syst. Sci. 2009, 13, 605–615. [Google Scholar] [CrossRef]
- Soulis, K.X.; Valiantzas, J.D. Identification of the SCS-CN parameter spatial distribution using rainfall-runoff data in heterogeneous watersheds. Water Resour. Manag. 2013, 27, 1737–1749. [Google Scholar] [CrossRef]
- Mishra, S.K.; Singh, V.P. SCS-CN method. Part-I: Derivation of SCS-CN based models. Acta Geophy. Pol. 2002, 50, 457–477. [Google Scholar]
- Mishra, S.K.; Singh, V.P. Soil Conservation Service Curve Number (SCS-CN) Methodology; Kluwer: Dordrecht, The Netherlands, 2003; ISBN 1-4020-1132-6. [Google Scholar]
- Sahu, R.K.; Mishra, S.K.; Eldho, T.I.; Jain, M.K. An advanced soil moisture accounting procedure for SCS curve number method. Hydrol. Process. 2007, 21, 2872–2881. [Google Scholar] [CrossRef]
- Kim, N.W.; Lee, J. Temporally weighted average curve number method for daily runoff simulation. Hydrol. Process. 2008, 22, 4936–4948. [Google Scholar] [CrossRef]
- Wang, S.; Kang, S.; Zhang, L.; Li, F. Simulation of an agricultural watershed using an improved curve number method in SWAT. Trans. ASABE 2008, 51, 1323–1339. [Google Scholar] [CrossRef]
- Ajmal, M.; Moon, G.; Ahn, J.; Kim, T. Investigation of SCS-CN and its inspired modified models for runoff estimation in South Korean watersheds. J. Hydro-Environ. Res. 2015, 9, 592–603. [Google Scholar] [CrossRef]
- Ajmal, M.; Waseem, M.; Wi, S.; Kim, T. Evolution of a parsimonious rainfall–runoff model using soil moisture proxies. J. Hydrol. 2015, 530, 623–633. [Google Scholar] [CrossRef]
- Ajmal, M.; Khan, T.A.; Kim, T.W. A CN-based ensembled hydrological model for enhanced watershed runoff prediction. Water 2016, 8, 20. [Google Scholar] [CrossRef]
- Arnbjerg-Nielsen, K.; Harremoes, P. Prediction of hydrological reduction factor and initial loss in urban surface runoff from small ungauged catchments. Atmos. Res. 1996, 42, 137–147. [Google Scholar] [CrossRef]



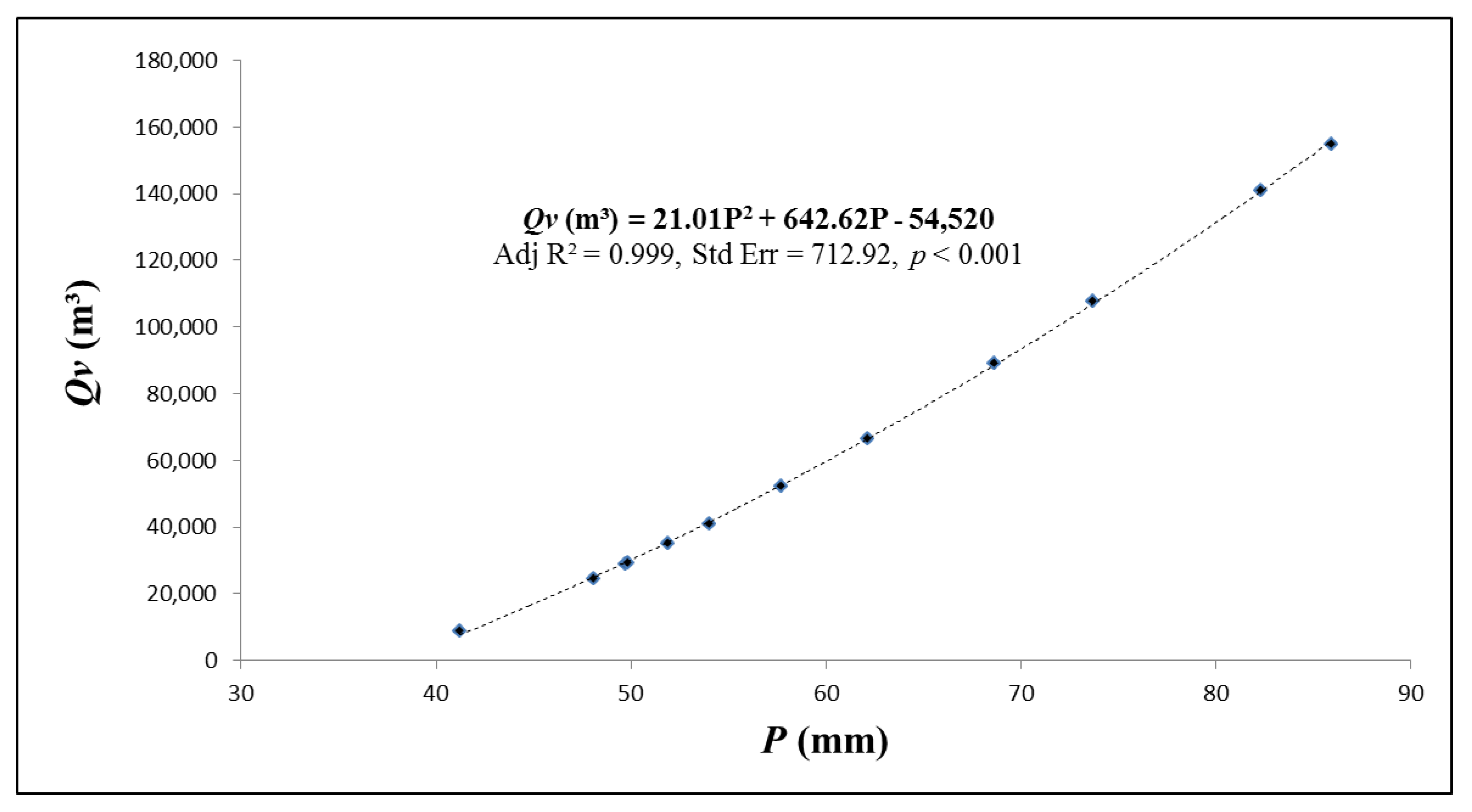
| No. | Storm | Rainfall | Direct Runoff | Initial Abstraction | Retention | Ratio |
|---|---|---|---|---|---|---|
| Event | P (mm) | Q (mm) | (mm) | S (mm) | ||
| 1 | 03/05/1994 | 11.2 | 0.36 | 4.6 | 114.1 | 0.040 |
| 2 | 14/06/1995 | 11.9 | 0.16 | 6.5 | 176.9 | 0.037 |
| 3 | 24/04/1994 | 14.2 | 0.23 | 8.6 | 130.7 | 0.066 |
| 4 | 12/05/1995 | 19.0 | 0.01 | 16.8 | 481.8 | 0.032 |
| 5 | 16/04/1995 | 19.8 | 0.47 | 9.9 | 200.0 | 0.050 |
| 6 | 10/09/1995 | 22.0 | 0.03 | 18.1 | 503.1 | 0.036 |
| 7 | 01/06/1995 | 23.6 | 0.29 | 14.8 | 258.7 | 0.057 |
| 8 | 17/10/1995 | 24.1 | 0.48 | 13.6 | 218.0 | 0.062 |
| 9 | 09/05/1994 | 26.5 | 0.65 | 13.1 | 262.8 | 0.050 |
| 10 | 19/05/1995 | 27.1 | 1.78 | 10.0 | 146.9 | 0.068 |
| 11 | 19/06/1996 | 28.3 | 2.18 | 13.4 | 86.9 | 0.154 |
| 12 | 20/06/1995 | 30.8 | 4.02 | 8.3 | 103.4 | 0.080 |
| 13 | 29/07/1996 | 31.7 | 0.92 | 10.7 | 458.3 | 0.023 |
| 14 | 23/06/1996 | 32.3 | 1.27 | 6.1 | 514.7 | 0.012 |
| 15 | 28/06/1996 | 32.4 | 1.75 | 14.6 | 163.3 | 0.089 |
| 16 | 14/05/1996 | 36.1 | 3.45 | 8.9 | 187.2 | 0.048 |
| 17 | 18/04/1994 | 38.1 | 3.72 | 10.4 | 178.6 | 0.058 |
| 18 | 19/10/1995 | 41.2 | 1.75 | 8.6 | 574.0 | 0.015 |
| 19 | 26/08/1994 | 48.1 | 1.08 | 19.0 | 755.2 | 0.025 |
| 20 | 04/06/1994 | 49.7 | 2.88 | 9.3 | 525.7 | 0.018 |
| 21 | 04/11/1996 | 49.8 | 4.15 | 6.7 | 405.1 | 0.017 |
| 22 | 07/07/1995 | 51.9 | 11.25 | 12.1 | 100.9 | 0.120 |
| 23 | 02/07/1996 | 54.0 | 2.86 | 7.4 | 714.0 | 0.010 |
| 24 | 02/05/1996 | 57.7 | 5.34 | 21.6 | 207.8 | 0.104 |
| 25 | 03/06/1996 | 62.1 | 3.23 | 18.5 | 544.6 | 0.034 |
| 26 | 07/06/1994 | 68.6 | 11.87 | 11.3 | 219.2 | 0.052 |
| 27 | 09/04/1994 | 73.7 | 9.94 | 14.1 | 297.6 | 0.047 |
| 28 | 18/09/1996 | 82.3 | 15.70 | 16.7 | 208.6 | 0.082 |
| 29 | 03/07/1996 | 85.9 | 21.31 | 7.8 | 208.1 | 0.037 |
| Wangjiaqiao Datasets | Descriptive Statistics of | BCa 99% Confidence Interval | Descriptive Statistics of S | BCa 99% Confidence Interval | ||
|---|---|---|---|---|---|---|
| Lower | Upper | Lower | Upper | |||
| Mean | 0.053 | 0.069 | 0.384 | 308.477 | 230.413 | 395.527 |
| Median | 0.048 | 0.035 | 0.062 | 219.188 | 178.561 | 458.348 |
| Skewness | 1.251 | 0.268 | 1.846 | 0.867 | −0.160 | 2.275 |
| Kurtosis | 1.884 | −0.786 | 4.830 | −0.389 | −1.833 | 6.030 |
| Std. Deviation | 0.034 | 0.021 | 0.044 | 192.843 | 137.400 | 232.351 |
| Model Parameters and Statistics | AFM Model | Calibrated SCS-CN Model Equation (12) | Conventional SCS-CN Model Equation (3) | Corrected SCS-CN Model Equation (15) | Linear Regression Model |
|---|---|---|---|---|---|
| p value | - | <0.001 | Not Significant | Adjusted | <0.001 |
| 0.200 | 0.043 | 0.200 | 0.200 | - | |
| S (mm) | 136.19 | 260.08 | 100.80 | - | - |
| (mm) | 27.238 | 11.190 | 20.16 | - | 4.623 |
| E | 0.799 | 0.825 | 0.482 | 0.826 | 0.725 |
| 152.251 | 133.044 | 393.126 | 131.960 | 208.462 | |
| −0.624 | 0.056 | 1.586 | 0.055 | −0.008 | |
| 65.096 | 72.284 | 71.590 | - | - | |
| Residual Mean | −0.624 | 0.056 | n/a | 0.055 | −0.008 |
| BCa 99% CI | |||||
| Range of | [−1.631, 0.283] | [−0.898, 0.961] | n/a | [−0.896, 0.970] | [−1.225, 1.124] |
| Mean Residual | |||||
| Residual Median | n/a | n/a | 0.140 | n/a | n/a |
| BCa 99% CI | |||||
| Range of | n/a | n/a | [−0.420, 3.850] | n/a | n/a |
| Median Residual | |||||
| Standard | |||||
| Deviation of | 2.244 | 2.179 | 3.381 | 2.171 | 2.728 |
| Model error | |||||
| Variance (Residual) | 5.035 | 4.748 | 11.429 | 4.715 | 7.445 |
| Range | 11.350 | 10.844 | 12.740 | 10.770 | 12.987 |
| p value | |||||
| of Shapiro-Wilk | 0.197 | 0.111 | 0.012 | 0.121 | 0.597 |
| Test |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ling, L.; Yusop, Z.; Yap, W.-S.; Tan, W.L.; Chow, M.F.; Ling, J.L. A Calibrated, Watershed-Specific SCS-CN Method: Application to Wangjiaqiao Watershed in the Three Gorges Area, China. Water 2020, 12, 60. https://doi.org/10.3390/w12010060
Ling L, Yusop Z, Yap W-S, Tan WL, Chow MF, Ling JL. A Calibrated, Watershed-Specific SCS-CN Method: Application to Wangjiaqiao Watershed in the Three Gorges Area, China. Water. 2020; 12(1):60. https://doi.org/10.3390/w12010060
Chicago/Turabian StyleLing, Lloyd, Zulkifli Yusop, Wun-She Yap, Wei Lun Tan, Ming Fai Chow, and Joan Lucille Ling. 2020. "A Calibrated, Watershed-Specific SCS-CN Method: Application to Wangjiaqiao Watershed in the Three Gorges Area, China" Water 12, no. 1: 60. https://doi.org/10.3390/w12010060
APA StyleLing, L., Yusop, Z., Yap, W.-S., Tan, W. L., Chow, M. F., & Ling, J. L. (2020). A Calibrated, Watershed-Specific SCS-CN Method: Application to Wangjiaqiao Watershed in the Three Gorges Area, China. Water, 12(1), 60. https://doi.org/10.3390/w12010060






