Multivariate Analysis and Geochemical Signatures of Shallow Groundwater in the Main Urban Area of Chongqing, Southwestern China
Abstract
1. Introduction
2. Study Area
3. Materials and Methods
3.1. Groundwater Sampling and Analytical Techniques
3.2. Multivariate Statistical Analysis
3.3. Geochemical Modeling
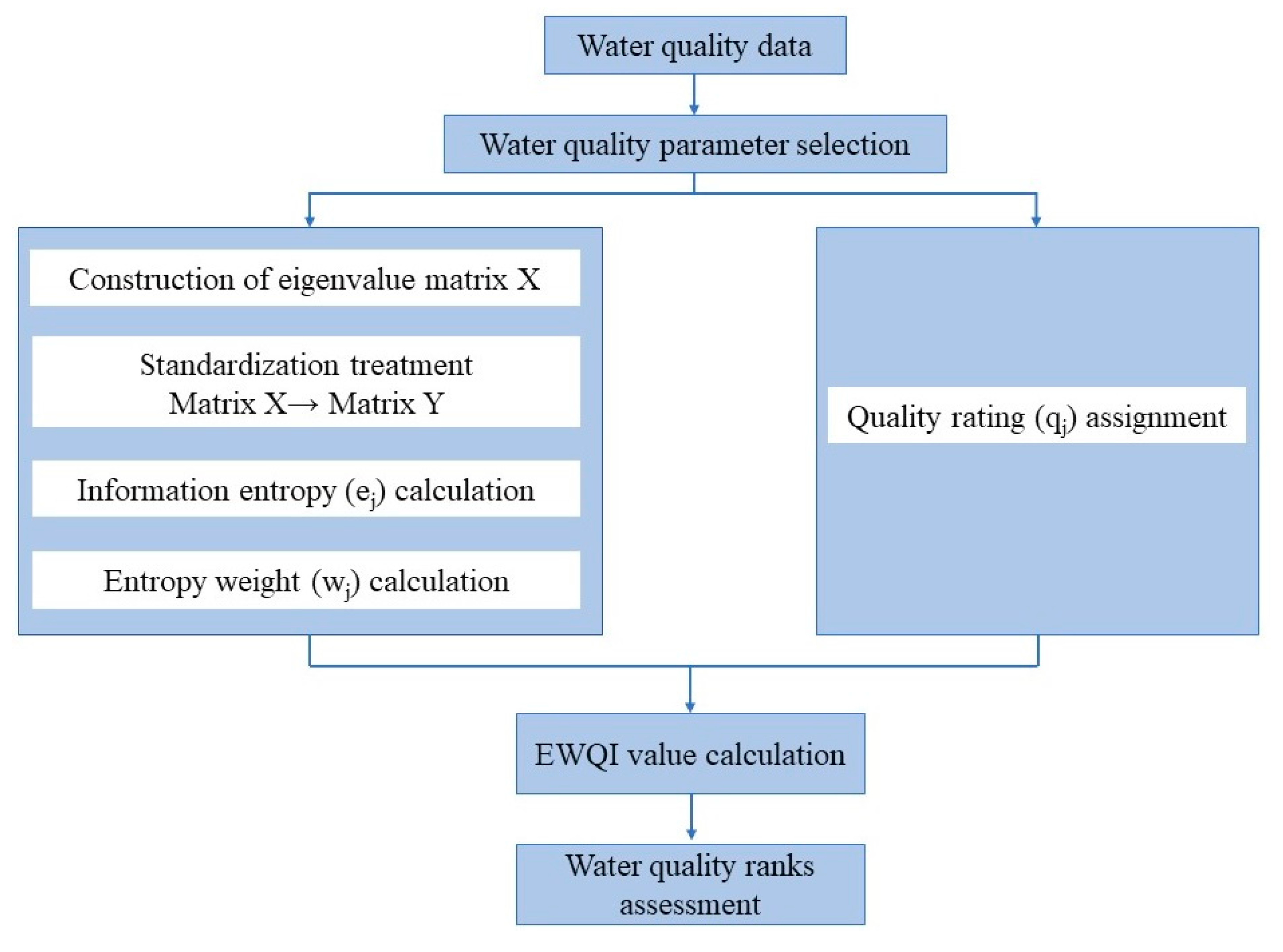
3.4. Entropy-Weighted Water Quality Index (EWQI)
4. Results and Discussion
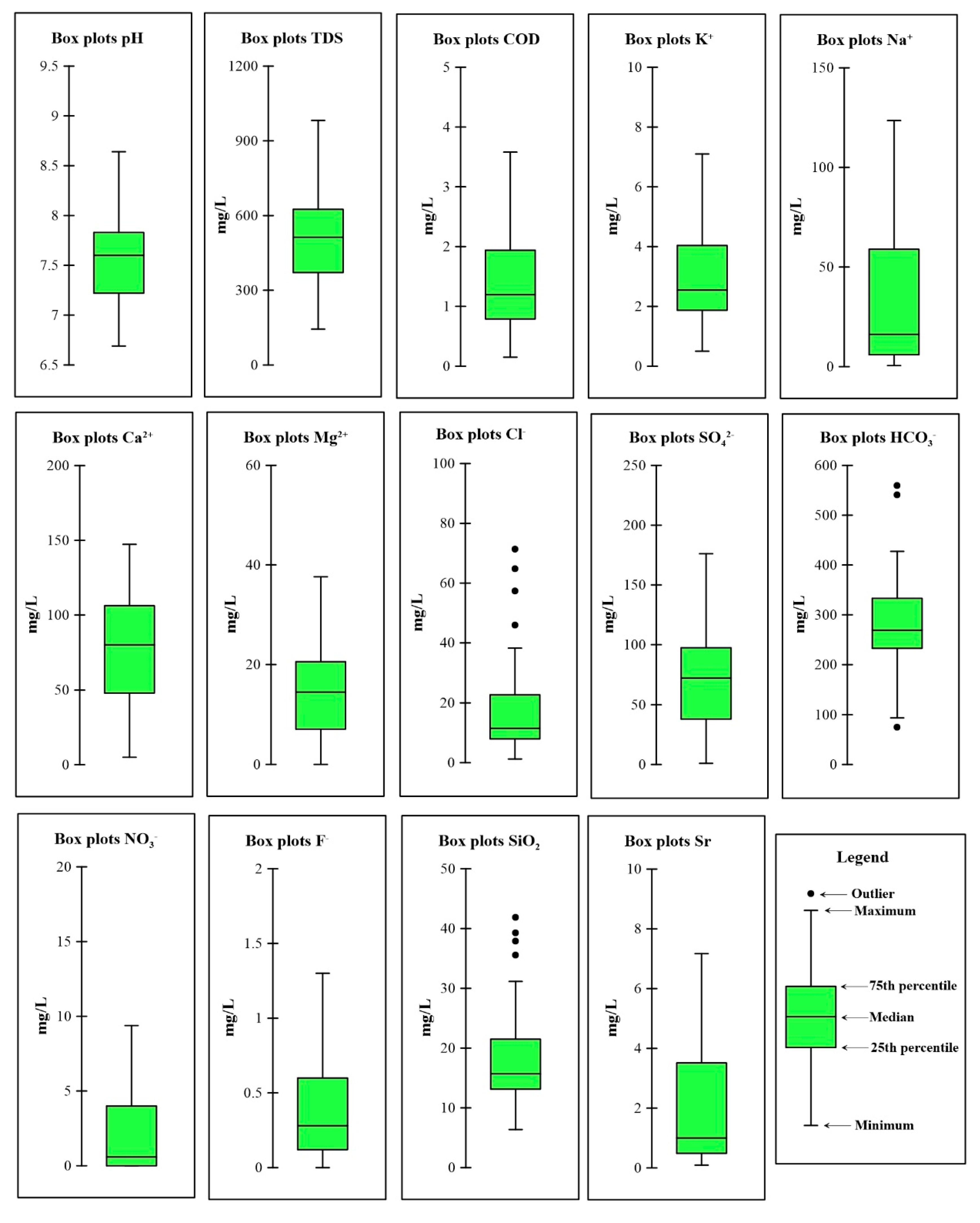
4.1. Statistical Results of Hydrochemical Parameters
4.2. Hydrochemical Type
4.3. Mechanism Controlling Groundwater Hydrogeochemistry
4.3.1. Principal Component Analysis
4.3.2. Gibbs Diagram
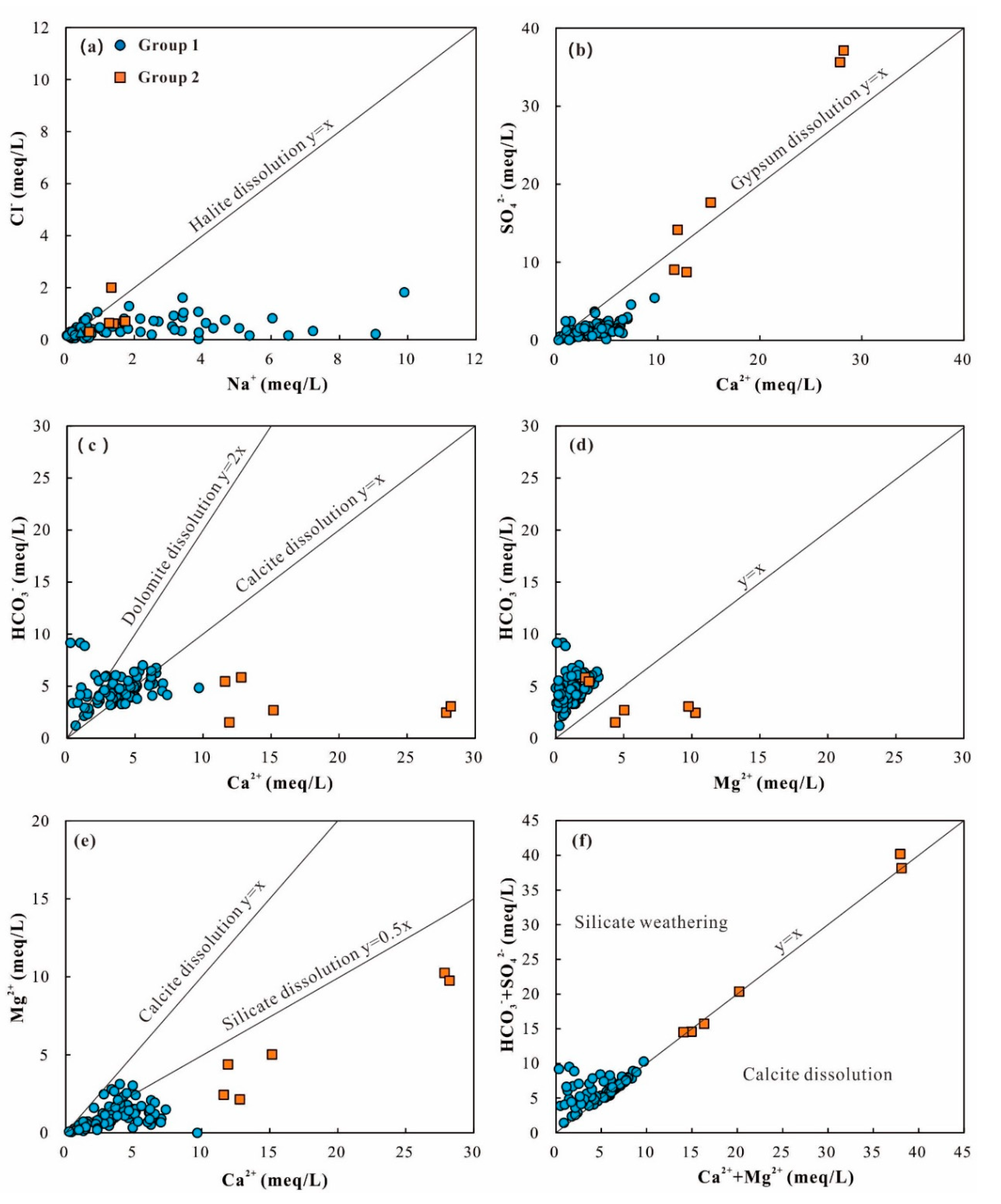
4.3.3. Hydrogeochemical Process
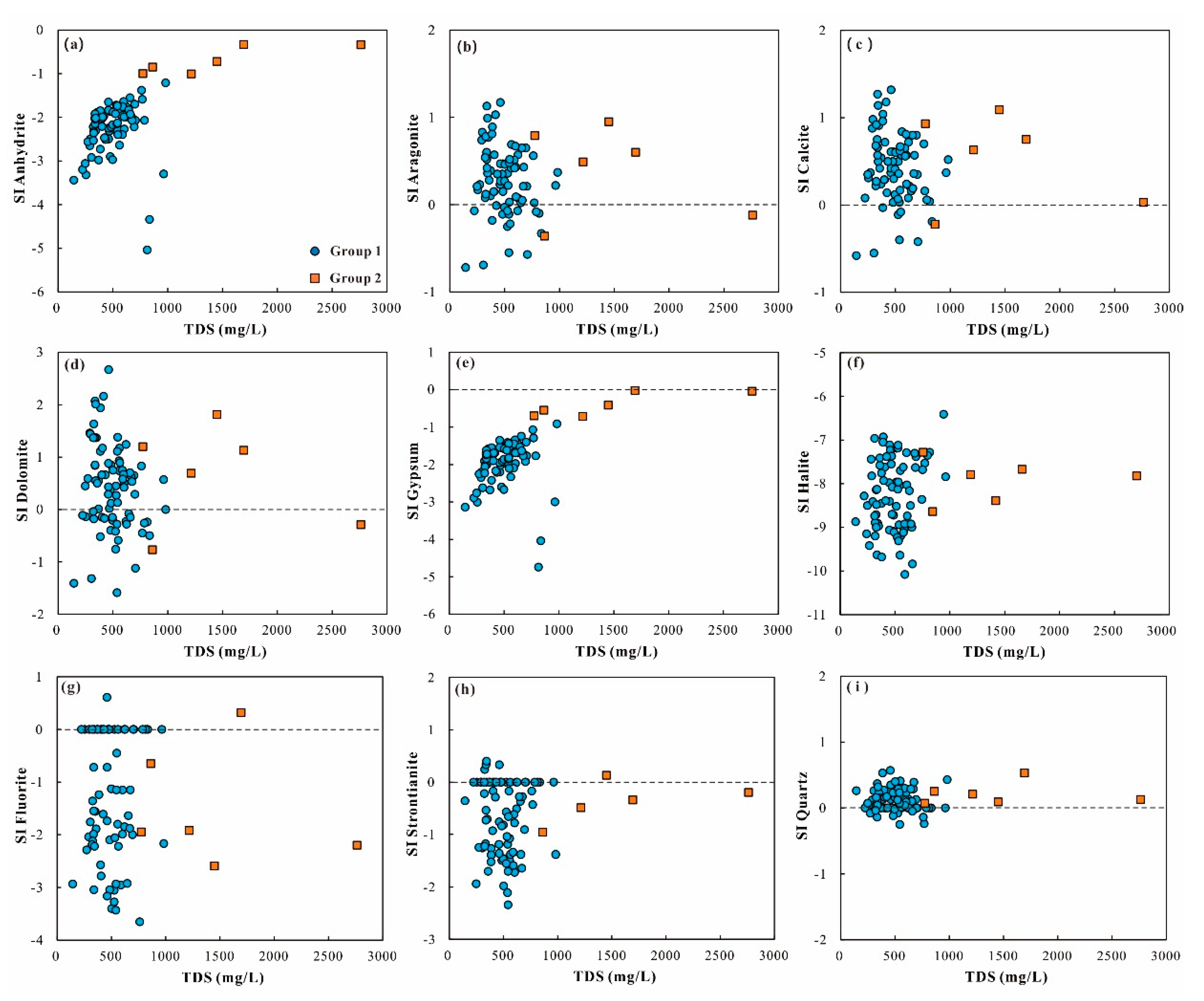
4.3.4. Saturation Indices
4.4. Groundwater Quality Classification and Its Spatial Distribution
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gu, X.; Xiao, Y.; Yin, S.; Hao, Q.; Liu, H.; Hao, Z.; Meng, G.; Pei, Q.; Yan, H. Hydrogeochemical Characterization and Quality Assessment of Groundwater in a Long-Term Reclaimed Water Irrigation Area, North China Plain. Water 2018, 10, 1209. [Google Scholar] [CrossRef]
- Xiao, Y.; Gu, X.; Yin, S.; Pan, X.; Shao, J.; Cui, Y. Investigation of Geochemical Characteristics and Controlling Processes of Groundwater in a Typical Long-Term Reclaimed Water Use Area. Water 2017, 9, 800. [Google Scholar] [CrossRef]
- Xiong, G.-Y.; Chen, G.-Q.; Xu, X.-Y.; Liu, W.-Q.; Fu, T.-F.; Khokiattiwong, S.; Kornkanitnan, N.; Ali Seddique, A.; Shi, X.-F.; Liu, S.-F.; et al. A comparative study on hydrochemical evolution and quality of groundwater in coastal areas of Thailand and Bangladesh. J. Asian Earth Sci. 2020, 195, 104336. [Google Scholar] [CrossRef]
- Stopelli, E.; Duyen, V.T.; Mai, T.T.; Trang, P.T.K.; Viet, P.H.; Lightfoot, A.; Kipfer, R.; Schneider, M.; Eiche, E.; Kontny, A.; et al. Spatial and temporal evolution of groundwater arsenic contamination in the Red River delta, Vietnam: Interplay of mobilisation and retardation processes. Sci. Total Environ. 2020, 717, 137143. [Google Scholar] [CrossRef]
- Liu, F.; Zhao, Z.; Yang, L.; Ma, Y.; Xu, Y.; Gong, L.; Liu, H. Geochemical characterization of shallow groundwater using multivariate statistical analysis and geochemical modeling in an irrigated region along the upper Yellow River, Northwestern China. J. Geochem. Explor. 2020, 215, 106565. [Google Scholar] [CrossRef]
- Liu, F.; Wang, S.; Yeh, T.-C.J.; Zhen, P.; Wang, L.; Shi, L. Using multivariate statistical techniques and geochemical modelling to identify factors controlling the evolution of groundwater chemistry in a typical transitional area between Taihang Mountains and North China Plain. Hydrol. Process. 2020, 34, 1888–1905. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, M.; Li, X.; Qi, J.; Zhang, Q.; Guo, J.; Yu, L.; Zhao, R. Hydrochemical Characteristics and Multivariate Statistical Analysis of Natural Water System: A Case Study in Kangding County, Southwestern China. Water 2018, 10, 80. [Google Scholar] [CrossRef]
- Bam, E.K.P.; Bansah, S. Groundwater chemistry and isotopes reveal vulnerability of granitic aquifer in the White Volta River watershed, West Africa. Appl. Geochem. 2020, 119, 104662. [Google Scholar] [CrossRef]
- Ruiz-Pico, Á.; Pérez-Cuenca, Á.; Serrano-Agila, R.; Maza-Criollo, D.; Leiva-Piedra, J.; Salazar-Campos, J. Hydrochemical characterization of groundwater in the Loja Basin (Ecuador). Appl. Geochem. 2019, 104, 1–9. [Google Scholar] [CrossRef]
- Swift Bird, K.; Navarre-Sitchler, A.; Singha, K. Hydrogeological controls of arsenic and uranium dissolution into groundwater of the Pine Ridge Reservation, South Dakota. Appl. Geochem. 2020, 114, 104522. [Google Scholar] [CrossRef]
- Zhang, Q.; Xu, P.; Qian, H.; Yang, F. Hydrogeochemistry and fluoride contamination in Jiaokou Irrigation District, Central China: Assessment based on multivariate statistical approach and human health risk. Sci. Total Environ. 2020, 741, 140460. [Google Scholar] [CrossRef]
- Jia, H.; Howard, K.; Qian, H. Use of multiple isotopic and chemical tracers to identify sources of nitrate in shallow groundwaters along the northern slope of the Qinling Mountains, China. Appl. Geochem. 2020, 113, 104512. [Google Scholar] [CrossRef]
- Barzegar, R.; Asghari Moghaddam, A.; Tziritis, E.; Adamowski, J.; Bou Nassar, J.; Noori, M.; Aalami, M.T.; Kazemian, N. Exploring the hydrogeochemical evolution of cold and thermal waters in the Sarein-Nir area, Iran using stable isotopes (δ18O and δD), geothermometry and multivariate statistical approaches. Geothermics 2020, 85, 101815. [Google Scholar] [CrossRef]
- Singh, C.K.; Kumar, A.; Shashtri, S.; Kumar, A.; Kumar, P.; Mallick, J. Multivariate statistical analysis and geochemical modeling for geochemical assessment of groundwater of Delhi, India. J. Geochem. Explor. 2017, 175, 59–71. [Google Scholar] [CrossRef]
- Kazakis, N.; Mattas, C.; Pavlou, A.; Patrikaki, O.; Voudouris, K. Multivariate statistical analysis for the assessment of groundwater quality under different hydrogeological regimes. Environ. Earth Sci. 2017, 76, 349. [Google Scholar] [CrossRef]
- Tziritis, E.P.; Datta, P.S.; Barzegar, R. Characterization and Assessment of Groundwater Resources in a Complex Hydrological Basin of Central Greece (Kopaida basin) with the Joint Use of Hydrogeochemical Analysis, Multivariate Statistics and Stable Isotopes. Aquat. Geochem. 2017, 23, 271–298. [Google Scholar] [CrossRef]
- Liu, P.; Hoth, N.; Drebenstedt, C.; Sun, Y.; Xu, Z. Hydro-geochemical paths of multi-layer groundwater system in coal mining regions—Using multivariate statistics and geochemical modeling approaches. Sci. Total Environ. 2017, 601, 1–14. [Google Scholar] [CrossRef]
- Gil-Márquez, J.M.; Barberá, J.A.; Andreo, B.; Mudarra, M. Hydrological and geochemical processes constraining groundwater salinity in wetland areas related to evaporitic (karst) systems. A case study from Southern Spain. J. Hydrol. 2017, 544, 538–554. [Google Scholar] [CrossRef]
- Sappa, G.; Ergul, S.; Ferranti, F. Geochemical modeling and multivariate statistical evaluation of trace elements in arsenic contaminated groundwater systems of Viterbo Area, (Central Italy). SpringerPlus 2014, 3, 237. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, P.; Xue, L.; Dong, Z.; Li, D. Solute geochemistry and groundwater quality for drinking and irrigation purposes: A case study in Xinle City, North China. Geochemistry 2020, 125609. [Google Scholar] [CrossRef]
- Maity, S.; Biswas, R.; Sarkar, A. Comparative valuation of groundwater quality parameters in Bhojpur, Bihar for arsenic risk assessment. Chemosphere 2020, 259, 127398. [Google Scholar] [CrossRef]
- Lee, K.-J.; Yun, S.-T.; Yu, S.; Kim, K.-H.; Lee, J.-H.; Lee, S.-H. The combined use of self-organizing map technique and fuzzy c-means clustering to evaluate urban groundwater quality in Seoul metropolitan city, South Korea. J. Hydrol. 2019, 569, 685–697. [Google Scholar] [CrossRef]
- Yang, P.; Luo, D.; Hong, A.; Ham, B.; Xie, S.; Ming, X.; Wang, Z.; Pang, Z. Hydrogeochemistry and geothermometry of the carbonate-evaporite aquifers controlled by deep-seated faults using major ions and environmental isotopes. J. Hydrol. 2019, 579, 124116. [Google Scholar] [CrossRef]
- Yang, P.; Dan, L.; Groves, C.; Xie, S. Geochemistry and genesis of geothermal well water from a carbonate-evaporite aquifer in Chongqing, SW China. Environ. Earth Sci. 2019, 78, 1–14. [Google Scholar] [CrossRef]
- Yang, P.; Cheng, Q.; Xie, S.; Wang, J.; Chang, L.; Yu, Q.; Zhan, Z.; Chen, F. Hydrogeochemistry and geothermometry of deep thermal water in the carbonate formation in the main urban area of Chongqing, China. J. Hydrol. 2017, 549, 50–61. [Google Scholar] [CrossRef]
- Ta, M.; Zhou, X.; Guo, J.; Wang, X.; Wang, Y.; Xu, Y. The Evolution and Sources of Major Ions in Hot Springs in the Triassic Carbonates of Chongqing, China. Water 2020, 12, 1194. [Google Scholar] [CrossRef]
- Ta, M.; Zhou, X.; Guo, J.; Wang, Y.; Wang, X.; Xu, Y. Hydrogeochemical characteristics and formation of the hot springs occurring in the plunging ends of an anticline in Chongqing, Eastern Sichuan Basin, China. Environ. Earth Sci. 2019, 78, 468. [Google Scholar] [CrossRef]
- Xiao, Q.; Jiang, Y.; Shen, L.; Yuan, D. Origin of calcium sulfate-type water in the Triassic carbonate thermal water system in Chongqing, China: A chemical and isotopic reconnaissance. Appl. Geochem. 2018, 89, 49–58. [Google Scholar] [CrossRef]
- Xie, G.-W.; Yang, P.-H.; Sheng, T.; Deng, S.-J.; Hong, A.-H. Comparison of the Geochemical Characteristics of Karst Springs of a Vertically Zoned Climate Region under Human Activity: A Case of Shuifang Spring and Bitan Spring in the Jinfo Mountain Area, Chongqing. Huan Jing Ke Xue Huanjing Kexue 2019, 40, 3078–3088. [Google Scholar]
- Zheng, D.; Liu, Y.; Luo, L.; Shahid, M.Z.; Hou, D. Spatial variation and health risk assessment of fluoride in drinking water in the Chongqing urban areas, China. Environ. Geochem. Health 2020. [Google Scholar] [CrossRef]
- Pu, J.; Yuan, D.; Xiao, Q.; Zhao, H. Hydrogeochemical characteristics in karst subterranean streams: A case history from Chongqing, China. Carbonates Evaporites 2015, 30, 307–319. [Google Scholar] [CrossRef]
- Pu, J.; Yuan, D.; Zhang, C.; Zhao, H. Hydrogeochemistry and possible sulfate sources in karst groundwater in Chongqing, China. Environ. Earth Sci. 2013, 68, 159–168. [Google Scholar] [CrossRef]
- Pu, J.; Yuan, D.; Zhang, C.; Zhao, H. Tracing the sources of strontium in karst groundwater in Chongqing, China: A combined hydrogeochemical approach and strontium isotope. Environ. Earth Sci. 2012, 67, 2371–2381. [Google Scholar] [CrossRef]
- Yan, D.-P.; Zhou, M.-F.; Song, H.-L.; Wang, X.-W.; Malpas, J. Origin and tectonic significance of a Mesozoic multi-layer over-thrust system within the Yangtze Block (South China). Tectonophysics 2003, 361, 239–254. [Google Scholar] [CrossRef]
- Szczygieł, J.; Golicz, M.; Hercman, H.; Lynch, E. Geological constraints on cave development in the plateau-gorge karst of South China (Wulong, Chongqing). Geomorphology 2018, 304, 50–63. [Google Scholar] [CrossRef]
- Chen, K.-L.; Wu, H.-N.; Cheng, W.-C.; Zhang, Z.; Chen, J. Geological characteristics of strata in Chongqing, China, and mitigation of the environmental impacts of tunneling-induced geo-hazards. Environ. Earth Sci. 2016, 76, 10. [Google Scholar] [CrossRef]
- Kaiser, H.F. The varimax criterion for analytic rotation in factor analysis. Psychometrika 1958, 23, 187–200. [Google Scholar] [CrossRef]
- Parkhurst, D.L.; Appelo, C. Description of Input and Examples for PHREEQC Version 3: A Computer Program for Speciation, Batch-Reaction, One-Dimensional Transport, and Inverse Geochemical Calculations; 2328-7055; US Geological Survey: Reston, VA, USA, 2013. [Google Scholar]
- Gao, Y.; Qian, H.; Ren, W.; Wang, H.; Liu, F.; Yang, F. Hydrogeochemical characterization and quality assessment of groundwater based on integrated-weight water quality index in a concentrated urban area. J. Clean. Prod. 2020, 260, 121006. [Google Scholar] [CrossRef]
- WHO. Guidelines for Drinking-Water Quality, 4th ed.; World Health Organization: Geneva, Switzerland, 2011. [Google Scholar]
- Xiao, Y.; Yin, S.; Hao, Q.; Gu, X.; Pei, Q.; Zhang, Y. Hydrogeochemical appraisal of groundwater quality and health risk in a near-suburb area of North China. J. Water Supply Res. Technol. AQUA 2020, 69, 55–69. [Google Scholar] [CrossRef]
- Adimalla, N.; Qian, H.; Li, P. Entropy water quality index and probabilistic health risk assessment from geochemistry of groundwaters in hard rock terrain of Nanganur County, South India. Geochemistry 2019, 125544. [Google Scholar] [CrossRef]





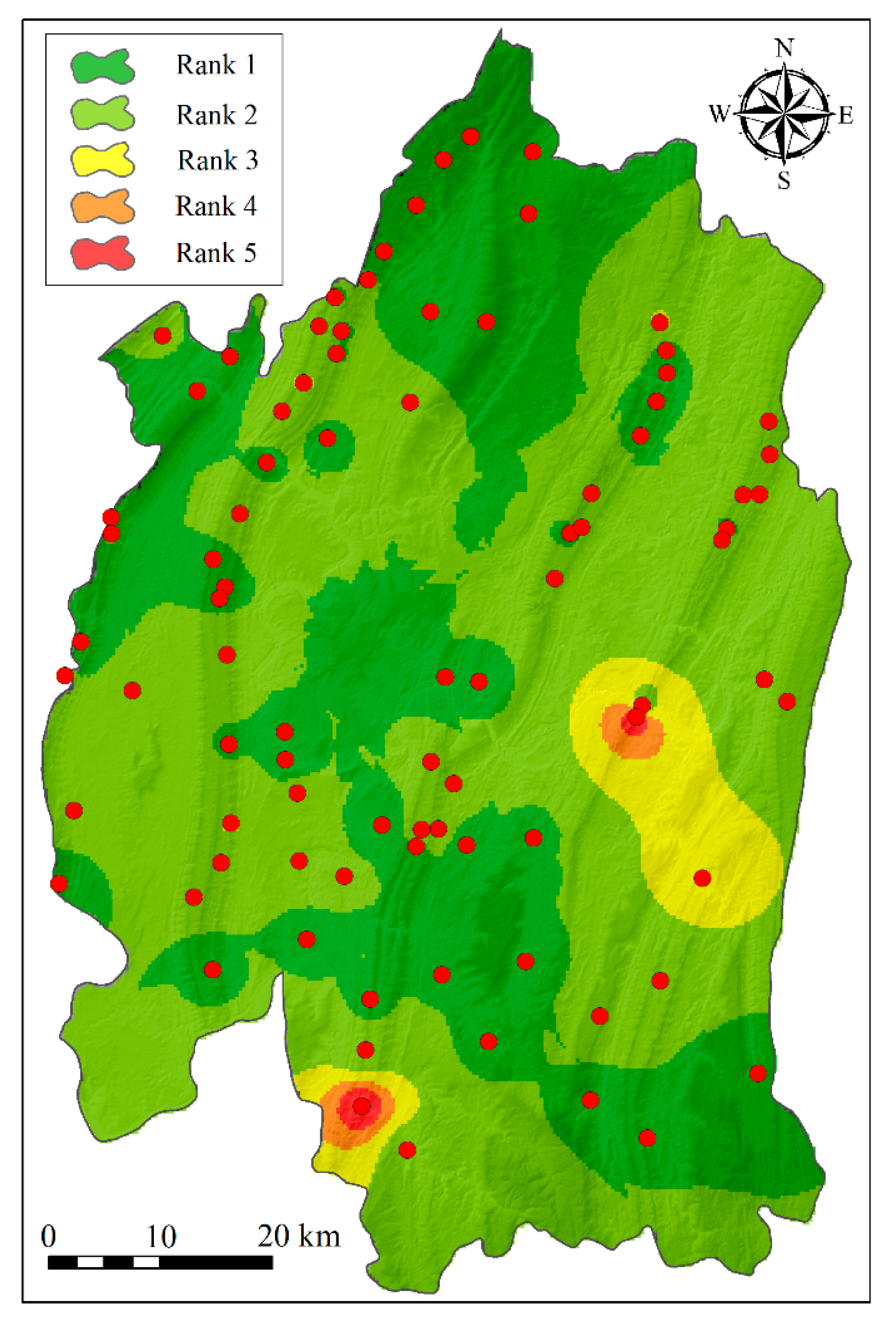
| Rank | EWQI | Water Quality |
|---|---|---|
| 1 | <50 | Excellent |
| 2 | 50–100 | Good |
| 3 | 100–150 | Medium |
| 4 | 150–200 | Poor |
| 5 | >200 | Extremely poor |
| Parameters | Units | Max | Min | Mean | Median | Standard Deviation |
|---|---|---|---|---|---|---|
| pH | 8.6 | 6.7 | 7.6 | 7.6 | 0.45 | |
| COD | mg/L | 24.21 | 0.15 | 2.79 | 1.23 | 4.71 |
| TDS | mg/L | 2761.92 | 143.90 | 555.66 | 483.70 | 364.83 |
| Ca | mg/L | 564.41 | 5.01 | 95.77 | 79.76 | 93.63 |
| Mg | mg/L | 123.08 | 0.00 | 18.13 | 14.52 | 20.38 |
| Na | mg/L | 227.64 | 0.52 | 38.27 | 14.79 | 49.24 |
| K | mg/L | 11.35 | 0.50 | 3.29 | 2.51 | 2.31 |
| HCO3 | mg/L | 559.37 | 75.07 | 279.20 | 263.97 | 91.44 |
| SO4 | mg/L | 1782.33 | 1.00 | 134.89 | 66.25 | 299.49 |
| Cl | mg/L | 64.84 | 1.24 | 15.32 | 10.97 | 11.93 |
| NO3 | mg/L | 39.97 | 0.14 | 5.26 | 2.57 | 7.46 |
| F | mg/L | 3.60 | 0.00 | 0.51 | 0.28 | 0.63 |
| Scaled Coordinates | Principe Components | |||
|---|---|---|---|---|
| 1 | 2 | 3 | 4 | |
| pH | −0.49 | 0.30 | 0.55 | −0.24 |
| COD | −0.30 | 0.47 | 0.23 | 0.15 |
| TDS | 0.67 | 0.34 | −0.33 | −0.13 |
| K | 0.64 | 0.07 | 0.41 | −0.02 |
| Na | −0.27 | 0.87 | −0.10 | −0.07 |
| Ca | 0.94 | −0.18 | 0.03 | 0.02 |
| Mg | 0.83 | −0.15 | 0.05 | 0.08 |
| Cl | 0.39 | 0.55 | −0.38 | 0.05 |
| SO4 | 0.84 | 0.12 | 0.32 | −0.07 |
| HCO3 | 0.03 | 0.07 | −0.75 | −0.39 |
| SiO2 | −0.02 | 0.66 | −0.01 | 0.22 |
| NO3 | 0.08 | −0.04 | −0.20 | 0.89 |
| F | 0.68 | 0.13 | 0.12 | 0.08 |
| Sr | 0.54 | 0.21 | 0.16 | −0.20 |
| Eigenvalue | 4.03 | 2.57 | 1.54 | 1.16 |
| Explained variance (%) | 28.76 | 18.39 | 10.97 | 8.26 |
| Cumulative % of variance | 28.76 | 47.15 | 58.12 | 66.38 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, S.; Tang, Z.; Wang, J.; Wu, J.; Yang, C.; Kang, W.; Huang, X. Multivariate Analysis and Geochemical Signatures of Shallow Groundwater in the Main Urban Area of Chongqing, Southwestern China. Water 2020, 12, 2833. https://doi.org/10.3390/w12102833
Chen S, Tang Z, Wang J, Wu J, Yang C, Kang W, Huang X. Multivariate Analysis and Geochemical Signatures of Shallow Groundwater in the Main Urban Area of Chongqing, Southwestern China. Water. 2020; 12(10):2833. https://doi.org/10.3390/w12102833
Chicago/Turabian StyleChen, Si, Zhonghua Tang, Jian Wang, Jialing Wu, Chang Yang, Wulue Kang, and Xun Huang. 2020. "Multivariate Analysis and Geochemical Signatures of Shallow Groundwater in the Main Urban Area of Chongqing, Southwestern China" Water 12, no. 10: 2833. https://doi.org/10.3390/w12102833
APA StyleChen, S., Tang, Z., Wang, J., Wu, J., Yang, C., Kang, W., & Huang, X. (2020). Multivariate Analysis and Geochemical Signatures of Shallow Groundwater in the Main Urban Area of Chongqing, Southwestern China. Water, 12(10), 2833. https://doi.org/10.3390/w12102833





