Variable Seepage Meter Efficiency in High-Permeability Settings
Abstract
:1. Introduction
- where
- ES is the multiplier to compensate for seepage meter undermeasurement (unitless) and is the inverse of seepage meter efficiency;
- ∆V is the change in volume of water contained in the seepage bag (m3);
- ∆t is the duration of seepage bag connection to the seepage cylinder (commonly minute or day);
- A is the inside area of the seepage cylinder, equal to the area of the sediment bed being measured (m2).
1.1. Sources of Inefficiency
- where
- ΔP is pressure drop over the length of the tubing (kg/m/s2);
- µ is dynamic viscosity determined at the water temperature (kg/m/s);
- L is the tubing length (m);
- Q is the volumetric seepage rate (m3/s);
- R is the radius of the tubing (m).
1.2. Reported Seepage Meter Efficiency
1.3. Purpose and Scope
2. Materials and Methods
2.1. Seepage Bag Efficiency Tests
2.2. Simulation of K Versus Seepage Meter Efficiency
3. Results
3.1. Efficiency Results from Seepage Calibration Tank Measurements
3.1.1. Bag Efficiency Related to Bag Fullness
3.1.2. Efficiency Related to Seepage Velocity
3.1.3. Efficiency Related to Bag Connection Duration
3.1.4. Efficiency Related to Upward vs. Downward Seepage
3.1.5. Inertial Effects of Shutting Down Seepage Prior to a Measurement
3.1.6. Seepage Cylinder Installation Depth
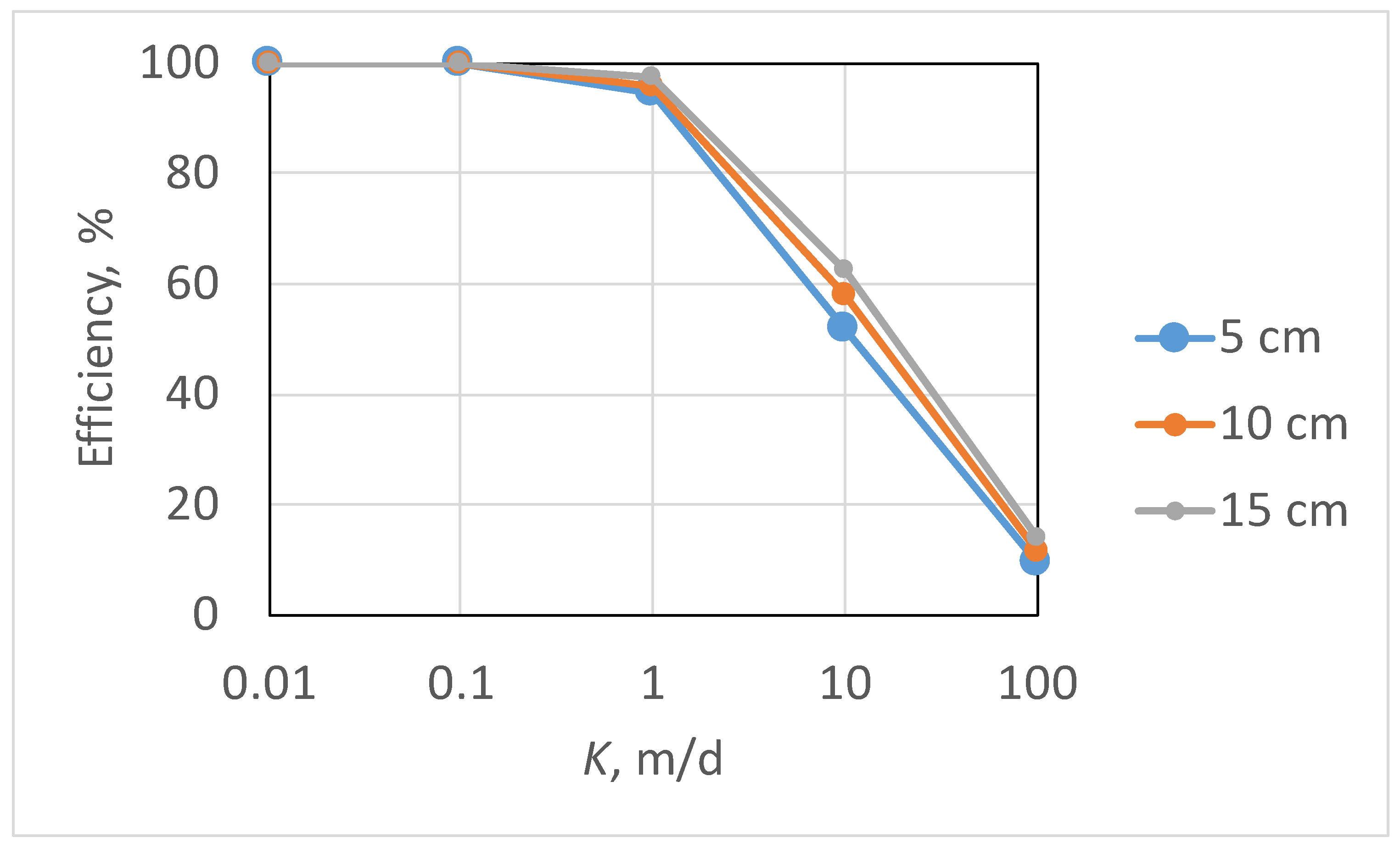
3.2. Influence of Hydraulic Conductivity on Meter Efficiency
4. Discussion
4.1. Recommended Practices to Maximize Efficiency and Consistency
- Operate seepage bags in the mid-range of their capacity;
- Use shorter rather than longer bag connection times;
- Maintain continuous flow through the meter prior, during, and after bag attachment;
- Use thin-wall, pliable seepage bags and avoid using high-capacity seepage bags;
- Press the seepage cylinder more deeply into the sediment where possible;
- Avoid routing water through sharp bends in the seepage-measurement device;
- Use a meter that does not require inflation or deflation of a seepage bag.
4.1.1. Operate Seepage Bags from about 1/5 to 2/3 Full
4.1.2. Use Shorter rather than Longer Bag Connection Times in Highly Permeable Sediments
4.1.3. Maintain Continuous Flow through the Seepage Cylinder during Bag Attachment and Removal
4.1.4. Use Thin-Wall Seepage Bags and Avoid Using a High-Capacity Seepage Bag
4.1.5. Install the Seepage Cylinder Deeper into the Bed Sediment
4.1.6. Avoid Sharp Bends that Can Create Inertial Effects
4.1.7. Replace the Bag with another Flow-Measurement Device
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rosenberry, D.O.; Duque, C.; Lee, D.R. History and evolution of seepage meters for quantifying flow between groundwater and surface water: Part 1—Freshwater settings. Earth Sci. Rev. 2020, 204, 103167. [Google Scholar] [CrossRef]
- Rosenberry, D.O.; Menheer, M.A. A System for Calibrating Seepage Meters Used to Measure Flow Between Ground Water and Surface Water; Scientific-Investigations Report 2006-5053; U.S. Geological Survey: Reston, VA, USA, 2006; 21p.
- Belanger, T.V.; Montgomery, M.T. Seepage meter errors. Limnol. Oceanogr. 1992, 37, 1787–1795. [Google Scholar] [CrossRef]
- Bouwer, H.; Rice, R.C. Seepage meters in seepage and recharge studies. J. Irrig. Drain. Div. 1963, 89, 17–42. [Google Scholar]
- Cherkauer, D.A.; McBride, J.M. A remotely operated seepage meter for use in large lakes and rivers. Ground Water 1988, 26, 165–171. [Google Scholar] [CrossRef]
- Zamora, C. Estimating Water Fluxes Across the Sediment-Water Interface in the Lower Merced River, California; Scientific-Investigations Report 2007-5216; U.S. Geological Survey: Reston, VA, USA, 2007; 47p.
- Dorrance, D.W. Streaming Potential and Seepage Meter Studies at Upper Lake Mary Near Flagstaff, Arizona. Master’s Thesis, University of Arizona, Tucson, AZ, USA, 1989. [Google Scholar]
- John, P.H.; Lock, M.A. The spatial distribution of groundwater discharge into the littoral zone of a New Zealand lake. J. Hydrol. 1977, 33, 391–395. [Google Scholar] [CrossRef]
- Lee, D.R. A device for measuring seepage flux in lakes and estuaries. Limnol. Oceanogr. 1977, 22, 140–147. [Google Scholar] [CrossRef]
- Lee, D.R.; Cherry, J.A. A field exercise on groundwater flow using seepage meters and mini-piezometers. J. Geol. Educ. 1978, 27, 6–20. [Google Scholar] [CrossRef]
- Fellows, C.R.; Brezonik, P.L. Seepage flow into Florida lakes. Water Resour. Bull. 1980, 16, 635–641. [Google Scholar] [CrossRef]
- Erickson, D.R. A Study of Littoral Groundwater Seepage at Williams Lake, Minnesota Using Seepage Meters and Wells. Master’s Thesis, University of Minnesota, Minneapolis, MN, USA, 1981. [Google Scholar]
- Rosenberry, D.O.; Morin, R.H. Use of an electromagnetic seepage meter to investigate temporal variability in lake seepage. Ground Water 2004, 42, 68–77. [Google Scholar] [CrossRef]
- Rosenberry, D.O. A seepage meter designed for use in flowing water. J. Hydrol. 2008, 359, 118–130. [Google Scholar] [CrossRef]
- Murdoch, L.C.; Kelly, S.E. Factors affecting the performance of conventional seepage meters. Water Resour. Res. 2003, 39. [Google Scholar] [CrossRef]
- Shinn, E.A.; Reich, C.D.; Hickey, T.D. Seepage meters and Bernoulli’s Revenge. Estuaries 2002, 25, 126–132. [Google Scholar] [CrossRef]
- Sebestyen, S.D.; Schneider, R.L. Dynamic temporal patterns of nearshore seepage flux in a headwater Adirondack lake. J. Hydrol. 2001, 247, 137–150. [Google Scholar] [CrossRef]
- Libelo, E.L.; MacIntyre, W.G. Effects of surface-water movement on seepage-meter measurements of flow through the sediment-water interface. Appl. Hydrogeol. 1994, 2, 49–54. [Google Scholar] [CrossRef]
- Koopmans, D.; Berg, P. An alternative to traditional seepage meters: Dye displacement. Water Resour. Res. 2011, 47, W01506. [Google Scholar] [CrossRef]
- Fryar, A.E.; Wallin, E.J.; Brown, D.L. Spatial and temporal variability in seepage between a contaminated aquifer and tributaries to the Ohio River. Ground Water Monit. Remediat. 2000, 20, 129–146. [Google Scholar] [CrossRef]
- Isiorho, S.A.; Meyer, J.H. The effects of bag type and meter size on seepage meter measurements. Ground Water 1999, 37, 411–413. [Google Scholar] [CrossRef]
- Lock, M.A.; John, P.H. The measurement of groundwater discharge into a lake by direct method. Int. Revue Gesamten Hydrobiol. 1978, 63, 271–275. [Google Scholar] [CrossRef]
- Brodie, R.S.; Baskaran, S.; Ransley, T.; Spring, J. Seepage meter: Progressing a simple method of directly measuring water flow between surface water and groundwater systems. Aust. J. Earth Sci. 2009, 56, 3–11. [Google Scholar] [CrossRef]
- Russoniello, C.J.; Michael, H.A. Investigation of Seepage Meter Measurements in Steady Flow and Wave Conditions. Groundwater 2015, 53, 959–966. [Google Scholar] [CrossRef]
- Birck, M.D. Temporal Variability of Riverbed Hydraulic Conductivity at an Induced Infiltration Site, Southwest Ohio. Master’s Thesis, Miami University, Oxford, UK, 2006. [Google Scholar]
- Rosenberry, D.O. Integrating seepage heterogeneity with the use of ganged seepage meters. Limnol. Oceanogr. Methods 2005, 3, 131–142. [Google Scholar] [CrossRef]
- Asbury, C.E. The Role of Groundwater Seepage in Sediment Chemistry and Nutrient Budgets in Mirror Lake, New Hampshire. Ph.D. Thesis, Cornell University, Ithaca, NY, USA, 1990. [Google Scholar]
- Kennedy, C.D.; Murdoch, L.C.; Genereux, D.P.; Corbett, D.R.; Stone, K.; Pham, P.; Mitasova, H. Comparison of Darcian flux calculations and seepage meter measurements in a sandy streambed in North Carolina, United States. Water Resour. Res. 2010, 46. [Google Scholar] [CrossRef]
- Solder, J.E.; Gilmore, T.E.; Genereux, D.P.; Solomon, D.K. A tube seepage meter for in situ measurement of seepage rate and groundwater sampling. Groundwater 2016, 54, 588–595. [Google Scholar] [CrossRef] [PubMed]
- Rosenberry, D.O.; Pitlick, J. Effects of sediment transport and seepage direction on hydraulic properties at the sediment-water interface of hyporheic settings. J. Hydrol. 2009, 373, 377–391. [Google Scholar] [CrossRef]
- Tryon, M.; Brown, K.; Dorman, L.; Sauter, A. A new benthic aqueous flux meter for very low to moderate discharge rates. Deep Sea Res. I 2001, 48, 2121–2146. [Google Scholar] [CrossRef]
- Amaral, E.J.; Pryor, W.A. Depositional environment of the St. Peter Sandstone deduced by textural analysis. J. Sediment. Res. 1977, 47, 32–52. [Google Scholar] [CrossRef]
- Benson, M.E.; Wilson, A.B. Frac Sand in the United States—A Geological and Industry Overview; Open-File Report 2015–1107; U.S. Geological Survey: Reston, VA, USA, 2015; 78p.
- Bouwer, H.; Rice, R.C. A slug test for determining hydraulic conductivity of unconfined aquifers with completely or partially penetrating wells. Water Resour. Res. 1976, 12, 423–428. [Google Scholar] [CrossRef] [Green Version]
- Bouwer, H. The Bouwer and Rice slug test—An update. Ground Water 1989, 27, 304–309. [Google Scholar] [CrossRef]
- Chen, X. Measurement of streambed hydraulic conductivity and its anisotropy. Environ. Geol. 2000, 39, 1317–1324. [Google Scholar] [CrossRef]
- Landon, M.K.; Rus, D.L.; Harvey, F.E. Comparison of instream methods for measuring hydraulic conductivity in sandy streambeds. Ground Water 2001, 39, 870–885. [Google Scholar] [CrossRef] [Green Version]
- Toran, L.; Nyquist, J.; Rosenberry, D.; Gagliano, M.; Mitchell, N.; Mikochik, J. Geophysical and hydrologic studies of lake seepage variability. Groundwater 2015, 53, 841–850. [Google Scholar] [CrossRef] [PubMed]
- Hare, D.K.; Briggs, M.A.; Rosenberry, D.O.; Boutt, D.F.; Lane, J.W. A comparison of thermal infrared to fiber-optic distributed temperature sensing for evaluation of groundwater discharge to surface water. J. Hydrol. 2015, 530, 153–166. [Google Scholar] [CrossRef] [Green Version]
- Kidmose, J.; Nilsson, B.; Engesgaard, P.; Frandsen, M.; Karan, S.; Landkildehus, F.; Søndergaard, M.; Jeppesen, E. Focused groundwater discharge of phosphorus to a eutrophic seepage lake (Lake Væng, Denmark): Implications for lake ecological state and restoration. Hydrogeol. J. 2013, 21, 1787–1802. [Google Scholar] [CrossRef]
- Rosenberry, D.O.; Briggs, M.A.; Delin, G.; Hare, D.K. Combined use of thermal methods and seepage meters to efficiently locate, quantify, and monitor focused groundwater discharge to a sand-bed stream. Water Resour. Res. 2016, 52, 4486–4503. [Google Scholar] [CrossRef] [Green Version]
- Rosenberry, D.O.; Pitlick, J. Local-scale spatial and temporal variability of seepage and hydraulic conductivity in a shallow gravel-bed river. Hydrol. Process. 2009, 23, 3306–3318. [Google Scholar] [CrossRef]
- Waldrop, W.R.; Swarzenski, P.W. A new tool for quantifying flux rates between surface water and ground water. In Coastal Hydrology and Processes; Singh, V.P., Xu, Y.J., Eds.; Water Resources Publications, LLC: Highlands Ranch, CO, USA, 2006; pp. 305–312. [Google Scholar]
- Harbaugh, A.W.; Langevin, C.D.; Hughes, J.D.; Niswonger, R.N.; Konikow, L.F. MODFLOW-2005 Version 1.12.00, the U.S. Geological Survey Modular Groundwater Model; U.S. Geological Survey: Reston, VA, USA, 2017.
- Rosenberry, D.O.; Lewandowski, J.; Meinikmann, K.; Nützmann, G. Groundwater—The disregarded component in lake water and nutrient budgets. Part 1: Effects of groundwater on hydrology. Hydrol. Process. 2015, 29, 2895–2921. [Google Scholar] [CrossRef]
- Rosenberry, D.O.; Nieto-López, J.M.; Webb, R.M.; Müller, S. Seepage Meter Efficiency in Highly Permeable Settings Source Data (2020); U.S. Geological Survey: Reston, VA, USA, 2020. [CrossRef]
- Webb, R.M.; Rosenberry, D.O. MODFLOW 2005 and MODPATH 5 Model Data Sets Used to Evaluate Seepage-Meter Efficiency in High-Permeability Settings; U.S. Geological Survey: Reston, VA, USA, 2020. [CrossRef]
- Shaw, R.D.; Prepas, E.E. Anomalous, short-term influx of water into seepage meters. Limnol. Oceanogr. 1989, 34, 1343–1351. [Google Scholar] [CrossRef]
- Blanchfield, P.J.; Ridgway, M.S. Use of seepage meters to measure groundwater flow at brook trout redds. Trans. Am. Fish Soc. 1996, 125, 813–818. [Google Scholar] [CrossRef]
- Attanayake, M.P.; Waller, D.H. Use of seepage meters in a groundwater-lake interaction study in a fractured rock basin—A case study. Can. J. Civil Eng. 1988, 15, 984–989. [Google Scholar] [CrossRef]
- Lien, B.K. Development and Demonstration of a Bidirectional Advective Flux Meter for Sediment-Water Interface; EPA/600/R-06/122; U.S. Environmental Protection Agency: Cincinnatti, OH, USA, 2006; 87p.
- Menheer, M.A. Development of a Benthic-Flux Chamber for Measurement of Ground-Water Seepage and Water Sampling for Mercury Analysis at the Sediment-Water Interface; Scientific Investigations Report 2004-5298; U.S. Geological Survey: Reston, VA, USA, 2004; 14p.
- Sholkovitz, E.; Herbold, C.; Charette, M. An automated dye-dilution based seepage meter for the time-series measurement of submarine groundwater discharge. Limnol. Oceanogr. Methods 2003, 1, 16–28. [Google Scholar] [CrossRef] [Green Version]
- Taniguchi, M.; Iwakawa, H. Measurements of submarine groundwater discharge rates by a continuous heat-type automated seepage meter in Osaka Bay, Japan. J. Groundw. Hydrol. 2001, 43, 271–277. [Google Scholar] [CrossRef]
- Paulsen, R.J.; Smith, C.F.; O’Rourke, D.; Wong, T. Development and evaluation of an ultrasonic ground water seepage meter. Ground Water 2001, 39, 904–911. [Google Scholar] [CrossRef]
- Krupa, S.L.; Belanger, T.V.; Heck, H.H.; Brock, J.T.; Jones, B.J. Krupaseep—The next generation seepage meter. J. Coast. Res. 1998, 26, 210–213. [Google Scholar]
- Solomon, D.K.; Humphrey, E.; Gilmore, T.E.; Genereux, D.P.; Zlotnik, V. An automated seepage meter for streams and lakes. Water Resour. Res. 2020, 56, e2019WR026983. [Google Scholar] [CrossRef] [Green Version]






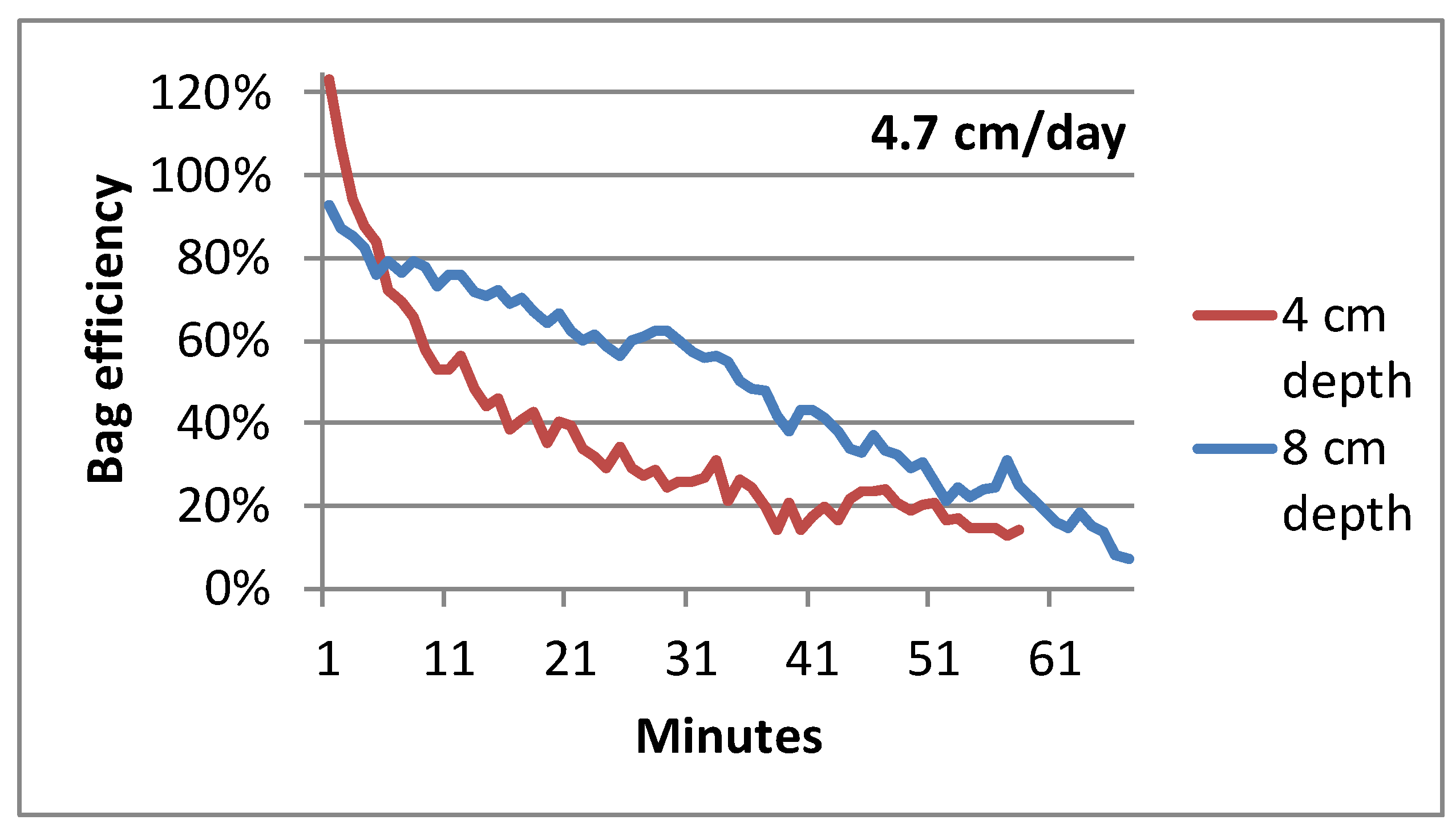


| Seepage Rate, cm/d | Bag-Averaged Efficiency, % | Average Efficiency, % | Average Efficiency for First 3 Min, % |
|---|---|---|---|
| 2.1 | 18 | 18 | 81 |
| 4.7 | 51 | 51 | 59 |
| 6.8 | 68, 73, 62, 51 | 64 | 79 |
| 7.9 | 67, 49, 46 | 53 | 74 |
| 13.5 | 78, 79, 75 | 77 | 80 |
| 38.7 | 88, 86, 87 | 87 | 87 |
| Percent Bag Fullness | Continuous Flow, mL/min | Stopped Flow, mL/min | Stopped ÷ Continuous |
|---|---|---|---|
| 75–100 | −15.02 | −11.93 | 79% |
| 25–75 | −12.00 | −7.31 | 61% |
| 25–75 | −12.67 | −7.54 | 60% |
| 25–75 | −12.00 | −4.09 | 34% |
| 0–25 | −5.09 | −3.11 | 61% |
| Average | −11.36 | −6.80 | 59% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rosenberry, D.O.; Nieto López, J.M.; Webb, R.M.T.; Müller, S. Variable Seepage Meter Efficiency in High-Permeability Settings. Water 2020, 12, 3267. https://doi.org/10.3390/w12113267
Rosenberry DO, Nieto López JM, Webb RMT, Müller S. Variable Seepage Meter Efficiency in High-Permeability Settings. Water. 2020; 12(11):3267. https://doi.org/10.3390/w12113267
Chicago/Turabian StyleRosenberry, Donald O., José Manuel Nieto López, Richard M. T. Webb, and Sascha Müller. 2020. "Variable Seepage Meter Efficiency in High-Permeability Settings" Water 12, no. 11: 3267. https://doi.org/10.3390/w12113267
APA StyleRosenberry, D. O., Nieto López, J. M., Webb, R. M. T., & Müller, S. (2020). Variable Seepage Meter Efficiency in High-Permeability Settings. Water, 12(11), 3267. https://doi.org/10.3390/w12113267






