Ice Regime of the Kozłowa Góra Reservoir (Southern Poland) as an Indicator of Changes of the Thermal Conditions of Ambient Air
Abstract
:1. Introduction
2. Materials and Methods
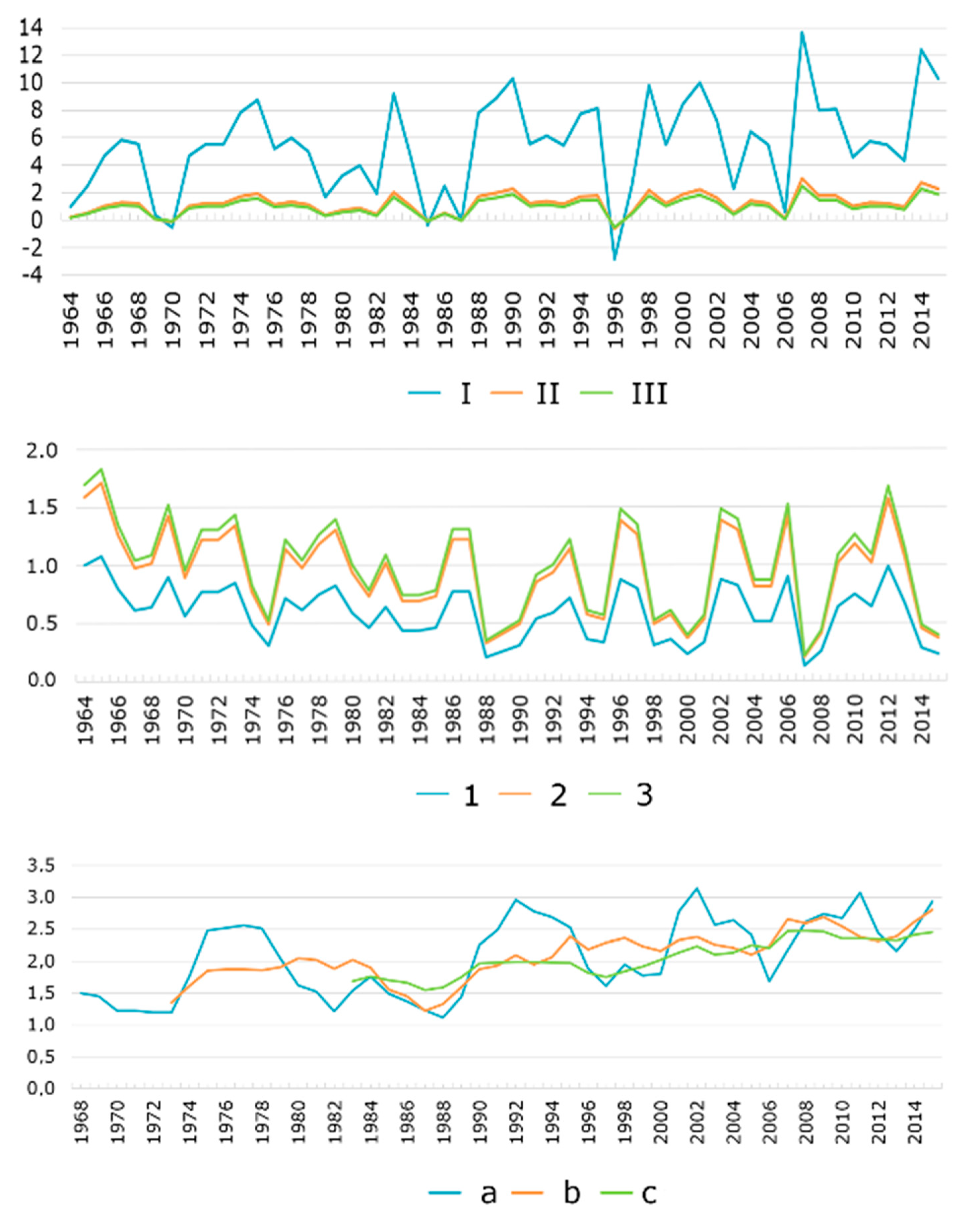
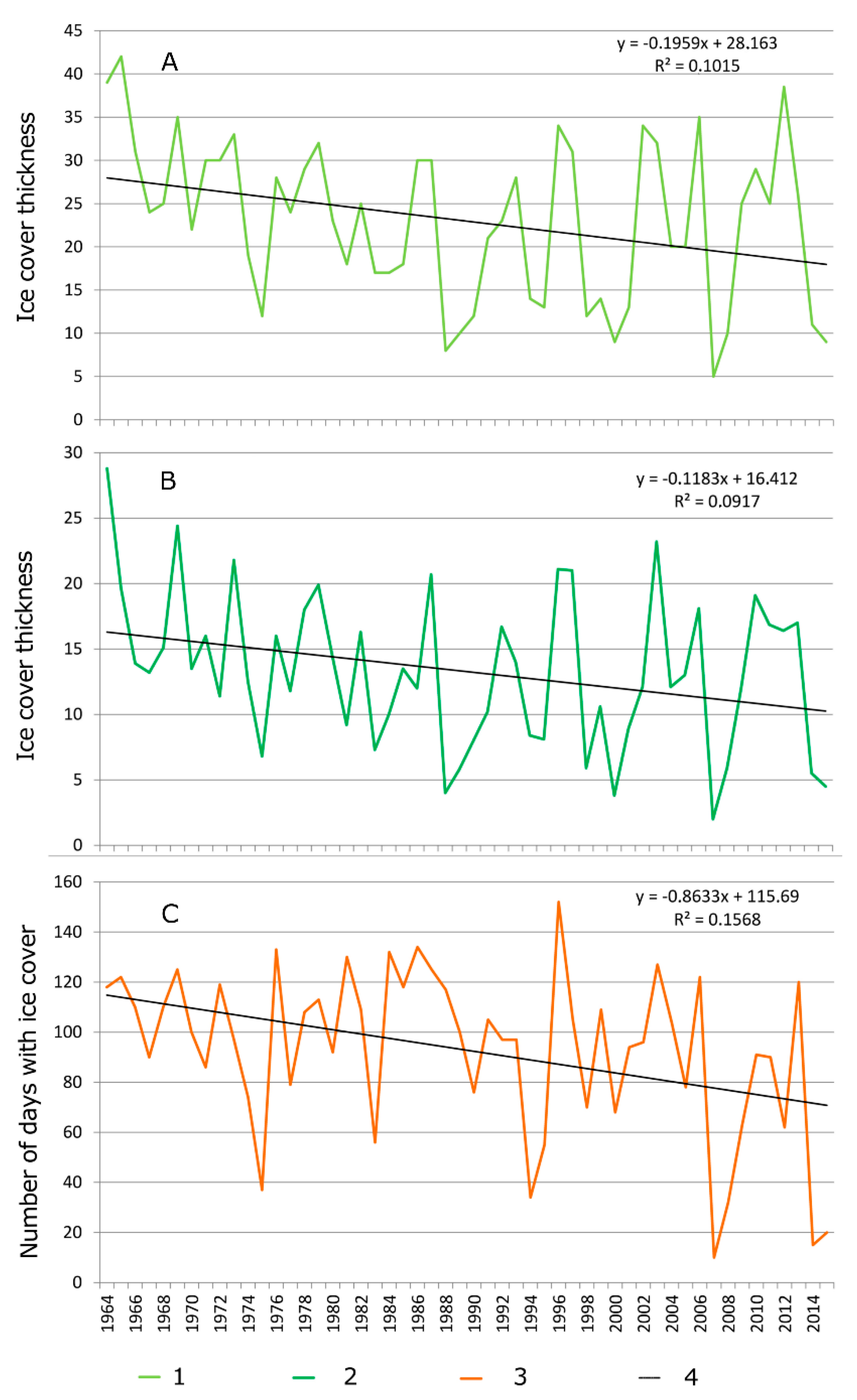
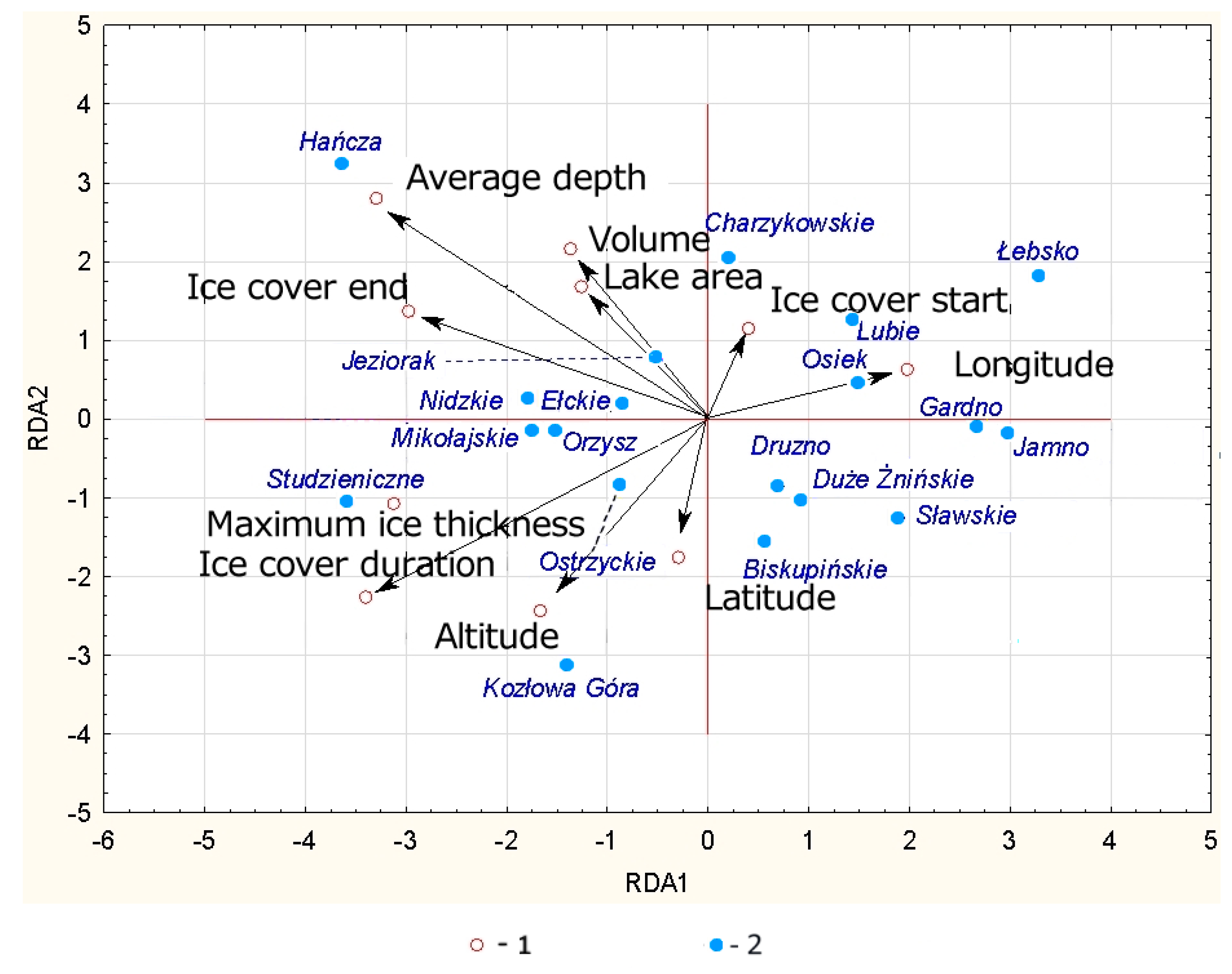
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Choiński, A. Physical Limnology of Poland; Adam Mickiewicz University: Poznań, Poland, 2007. [Google Scholar]
- Leppäranta, M. Freezing of Lakes and the Evolution of their Ice Cover; Springer Science and Business Media: Berlin, Germany, 2015; pp. 1–301. [Google Scholar]
- Magnuson, J.J.; Robertson, D.M.; Benson, B.J.; Wynne, R.H.; Livingstone, D.M.; Arai, T.; Assel, R.A.; Barry, R.G.; Card, V.; Kuusisto, E.; et al. Historical Trends in Lake and River Ice Cover in the Northern Hemisphere. Science 2000, 289, 1743–1746. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Marszelewski, W.; Skowron, R. Ice cover as an indicator of winter air temperature changes: Case study of the Polish Lowland lakes. Hydrol. Sci. J. 2006, 51, 336–349. [Google Scholar] [CrossRef]
- Sporka, F.; Livingstone, D.M.; Stuchlik, E.; Turek, J.; Galas, J. Water temperatures and ice cover in lakes of the Tatra Mountains. Biologia 2006, 61, 77–90. [Google Scholar] [CrossRef] [Green Version]
- Brown, L.C.; Duguay, C.R. A comparison of simulated and measured lake ice thickness using a Shallow Water Ice Profiler. Hydrol. Process. 2011, 25, 2932–2941. [Google Scholar] [CrossRef]
- Karetnikov, S.G.; Naumenko, M.A. Lake Ladoga ice phenology: Mean condition and extremes during the last 65 years. Hydrol. Process. 2011, 25, 2859–2867. [Google Scholar] [CrossRef]
- Pociask-Karteczka, J.; Choiński, A. Recent trends in ice cover duration for Lake Morskie Oko (Tatra Mountains, East-Central Europe). Hydrol. Res. 2012, 43, 500–506. [Google Scholar] [CrossRef] [Green Version]
- Choiński, A.; Ptak, M.; Skowron, R.; Strzelczak, A. Changes in ice phenology on polish lakes from 1961 to 2010 related to location and morphometry. Limnologica 2015, 53, 42–49. [Google Scholar] [CrossRef]
- Hewitt, B.A.; Lopez, L.S.; Gaibisels, K.M.; Murdoch, A.D.; Higgins, S.N.; Magnuson, J.J.; Paterson, A.M.; Rusak, J.A.; Yao, H.; Sharma, S. Historical Trends, Drivers, and Future Projections of Ice Phenology in Small North Temperate Lakes in the Laurentian Great Lakes Region. Water 2018, 10, 70. [Google Scholar] [CrossRef] [Green Version]
- Lopez, L.S.; Hewitt, B.A.; Sharma, S. Reaching a breaking point: How is climate change influencing the timing of ice breakup in lakes across the northern hemisphere? Limnol. Oceanogr. 2019, 64, 2621–2631. [Google Scholar] [CrossRef]
- Gao, S.; Stefan, H.G. Multiple Linear Regression for Lake Ice and Lake Temperature Characteristics. J. Cold Reg. Eng. 1999, 13, 59–77. [Google Scholar] [CrossRef]
- Leppäranta, M.; Reinart, A.; Erm, A.; Arst, H.; Hussainov, M.; Sipelgas, L. Investigation of Ice and Water Properties and Under-ice Light Fields in Fresh and Brackish Water Bodies. Hydrol. Res. 2003, 34, 245–266. [Google Scholar] [CrossRef] [Green Version]
- Prowse, T.D.; Stephenson, R.L. The relationship between winter lake cover, radiation receipts and the oxygen deficit in temperate lakes. Atmos. Ocean 1986, 24, 386–403. [Google Scholar] [CrossRef] [Green Version]
- Shuter, B.J.; Finstad, A.G.; Helland, I.P.; Zweimuller, I.; Hölker, F. The role of winter phenology in shaping the ecology of freshwater fish and their sensitivities to climate change. Aquat. Sci. 2012, 74, 637–657. [Google Scholar] [CrossRef]
- Bryan, M.L.; Marcus, M.G. Physical Characteristics of Near-Shore Ice Ridges. Arctic 1972, 25, 182–192. [Google Scholar] [CrossRef]
- Cox, G.F. A preliminary investigation of thermal ice pressures. Cold Reg. Sci. Technol. 1984, 9, 221–229. [Google Scholar] [CrossRef]
- Banach, M. Morphodynamics of the Włocławek Reservoir coastal zone. Geogr. Stud. 1994, 161, 1–181. [Google Scholar]
- Fang, X.; Stefan, H.G. Potential climate warming effects on ice covers of small lakes in the contiguous U.S. Cold Reg. Sci. Technol. 1998, 27, 119–140. [Google Scholar] [CrossRef]
- Palecki, M.A.; Barry, R.G. Freeze-up and Break-up of Lakes as an Index of Temperature Changes during the Transition Seasons: A Case Study for Finland. J. Clim. Appl. Meteorol. 1986, 25, 893–902. [Google Scholar] [CrossRef]
- Kuusisto, E. An analysis of the longest ice observation series made on Finnish lakes. Aqua Fenn. 1987, 17, 123–132. [Google Scholar]
- Assel, R.A.; Robertson, D.M. Changes in winter air temperatures near Lake Michigan, 1851-1993, as determined from regional lake-ice records. Limnol. Oceanogr. 1995, 40, 165–176. [Google Scholar] [CrossRef]
- Livingstone, D.M. Break-up Dates of Alpine Lakes As Proxy Data for Local and Regional Mean Surface Air Temperatures. Clim. Chang. 1997, 37, 407–439. [Google Scholar] [CrossRef] [Green Version]
- Livingstone, D.M. Impact of Secular Climate Change on the Thermal Structure of a Large Temperate Central European Lake. Clim. Chang. 2003, 57, 205–225. [Google Scholar] [CrossRef]
- Kuusisto, E.; Elo, A.-R. Lake and river ice variables as climate indicators in Northern Europe. Verh. Int. Ver. Limnol. 2000, 27, 2761–2764. [Google Scholar] [CrossRef]
- Hodgkins, G.A.; James, I.C.; Huntington, T.G. Historical changes in lake ice-out dates as indicators of climate change in New England, 1850–2000. Int. J. Clim. 2002, 22, 1819–1827. [Google Scholar] [CrossRef]
- Girjatowicz, J.P. Ice conditions in coastal lakes of the southern Baltic Sea. Ann. Limnol. Int. J. Limnol. 2003, 39, 317–331. [Google Scholar] [CrossRef] [Green Version]
- Futter, M. Patterns and trends in Southern Ontario lake ice phenology. Environ. Monit. Assess. 2003, 88, 431–444. [Google Scholar] [CrossRef]
- Johnson, S.L.; Stefan, H.G. Indicators of Climate Warming in Minnesota: Lake ICE Covers and Snowmelt Runoff. Clim. Chang. 2006, 75, 421–453. [Google Scholar] [CrossRef]
- Jensen, O.P.; Benson, B.J.; Magnuson, J.J.; Card, V.M.; Futter, M.; Soranno, P.A.; Stewart, K.M. Spatial analysis of ice phenology trends across the Laurentian Great Lakes region during a recent warming period. Limnol. Oceanogr. 2007, 52, 2013–2026. [Google Scholar] [CrossRef] [Green Version]
- Adrian, R.; Reilly, C.M.O.; Zagarese, H.; Baines, S.B.; Hessen, D.O.; Keller, W.; Livingstone, D.M.; Sommaruga, R.; Straile, D.; Van Donk, E.; et al. Lakes as sentinels of climate change. Limnol. Oceanogr. 2009, 54, 2283–2297. [Google Scholar] [CrossRef]
- Skowron, R. Changeability of the Ice Cover on the Lakes of Northern Poland in the Light of Climatic Changes. Bull. Geogr. Phys. Geogr. Ser. 2009, 1, 103–123. [Google Scholar] [CrossRef] [Green Version]
- Sziwa, R.; Jańczak, J. Extreme values of ice cover thickness and ice phenomena duration on lakes in Poland. Limnol. Rev. 2009, 9, 111–119. [Google Scholar]
- Choiński, A.; Kolendowicz, L.; Pociask-Karteczka, J.; Sobkowiak, L. Changes in lake ice cover on the Morskie Oko lake in Poland (1971–2007). Adv. Clim. Chang. Res. 2010, 1, 71–75. [Google Scholar] [CrossRef]
- Weyhenmeyer, G.A.; Livingstone, D.M.; Meili, M.; Jensen, O.; Benson, B.; Magnuson, J.J. Large geographical differences in the sensitivity of ice-covered lakes and rivers in the Northern Hemisphere to temperature changes. Glob. Chang. Biol. 2010, 17, 268–275. [Google Scholar] [CrossRef]
- Benson, B.J.; Magnuson, J.J.; Jensen, O.P.; Card, V.M.; Hodgkins, G.; Korhonen, J.; Livingstone, D.M.; Stewart, K.M.; Weyhenmeyer, G.A.; Granin, N.G. Extreme events, trends, and variability in Northern Hemisphere lake-ice phenology (1855–2005). Clim. Chang. 2012, 112, 299–323. [Google Scholar] [CrossRef]
- Livingstone, D.M. Ice break-up on southern Lake Baikal and its relationship to local and regional air temperatures in Siberia and to the North Atlantic Oscillation. Limnol. Oceanogr. 1999, 44, 1486–1497. [Google Scholar] [CrossRef] [Green Version]
- Yoo, J.; D’Odorico, P. Trends and fluctuations in the dates of ice break-up of lakes and rivers in Northern Europe: The effect of the North Atlantic Oscillation. J. Hydrol. 2002, 268, 100–112. [Google Scholar] [CrossRef]
- Borowiak, D.; Barańczuk, J. Secular fluctuations of ice phenomena in Upper Radunia Lake, Kashubian Lakeland. Limnol. Rev. 2004, 4, 17–24. [Google Scholar]
- George, D.G. The Impact of the North Atlantic Oscillation on the development of ice on Lake Windermere. Clim. Chang. 2007, 81, 455–468. [Google Scholar] [CrossRef]
- Ghanbari, R.N.; Bravo, H.R.; Magnuson, J.J.; Hyzer, W.G.; Benson, B.J. Coherence between lake ice cover, local climate and teleconnections (Lake Mendota, Wisconsin). J. Hydrol. 2009, 374, 282–293. [Google Scholar] [CrossRef]
- Mudelsee, M. A proxy record of winter temperatures since 1836 from ice freeze-up/breakup in lake Näsijärvi, Finland. Clim. Dyn. 2011, 38, 1413–1420. [Google Scholar] [CrossRef] [Green Version]
- Sharma, S.; Magnuson, J.J. Oscillatory dynamics do not mask linear trends in the timing of ice breakup for Northern Hemispher lakes from 1855 to 2004. Clim. Chang. 2014, 124, 835–847. [Google Scholar] [CrossRef]
- Sánchez-López, G.; Hernández, A.; Pla-Rabes, S.; Toro, M.; Granados, I.; Sigro, J.; Trigo, R.; Rubio-Inglés, M.J.; Camarero, L.; Valero-Garcés, B.; et al. The effects of the NAO on the ice phenology of Spanish alpine lakes. Clim. Chang. 2015, 130, 101–113. [Google Scholar] [CrossRef] [Green Version]
- Sharma, S.; Magnuson, J.J.; Batt, R.D.; Winslow, L.A.; Korhonen, J.; Aono, Y. Direct observations of ice seasonality reveal changes in climate over the past 320–570 years. Sci. Rep. 2016, 6, 25061. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ptak, M.; Tomczyk, A.M.; Wrzesiński, D.; Bednorz, E. Effect of teleconnection patterns on ice conditions in lakes in lowland Poland. Theor. Appl. Clim. 2019, 138, 1961–1969. [Google Scholar] [CrossRef] [Green Version]
- Anders, I.; Stagl, J.; Auer, I.; Pavlik, D. Climate Change in Central and Eastern Europe. In Advances in Global Change Research; Springer Science and Business Media: Berlin, Germany, 2013; Volume 58, pp. 17–30. [Google Scholar]
- Jones, P.D.; Osborn, T.J.; Briffa, K.R. The Evolution of Climate Over the Last Millennium. Science 2001, 292, 662–667. [Google Scholar] [CrossRef] [Green Version]
- Degirmendzic, J.; Rozuchowski, K.; Zmudzka, E. Changes of air temperature and precipitation in Poland in the period 1951–2000 and their relationship to atmospheric circulation. Int. J. Climatol. 2004, 24, 291–310. [Google Scholar] [CrossRef]
- Rzetala, M. Ice cover development in a small water body in an undrained depression. In International Multidiscyplinary Scientific Geoconferences, Proceedings of the 14th GeoConference on Water Resources, Albena, Bulgaria, 17–26 June 2014; STEF92 Technology Ltd.: Sofia, Bulgaria, 2014; pp. 397–404. [Google Scholar]
- Sharma, S.; Blagrave, K.; Magnuson, J.J.; O’Reilly, C.M.; Oliver, S.; Batt, R.D.; Magee, M.R.; Straile, D.; Weyhenmeyer, G.A.; Winslow, L.A.; et al. Widespread loss of lake ice around the Northern Hemisphere in a warming world. Nat. Clim. Chang. 2019, 9, 227–231. [Google Scholar] [CrossRef]
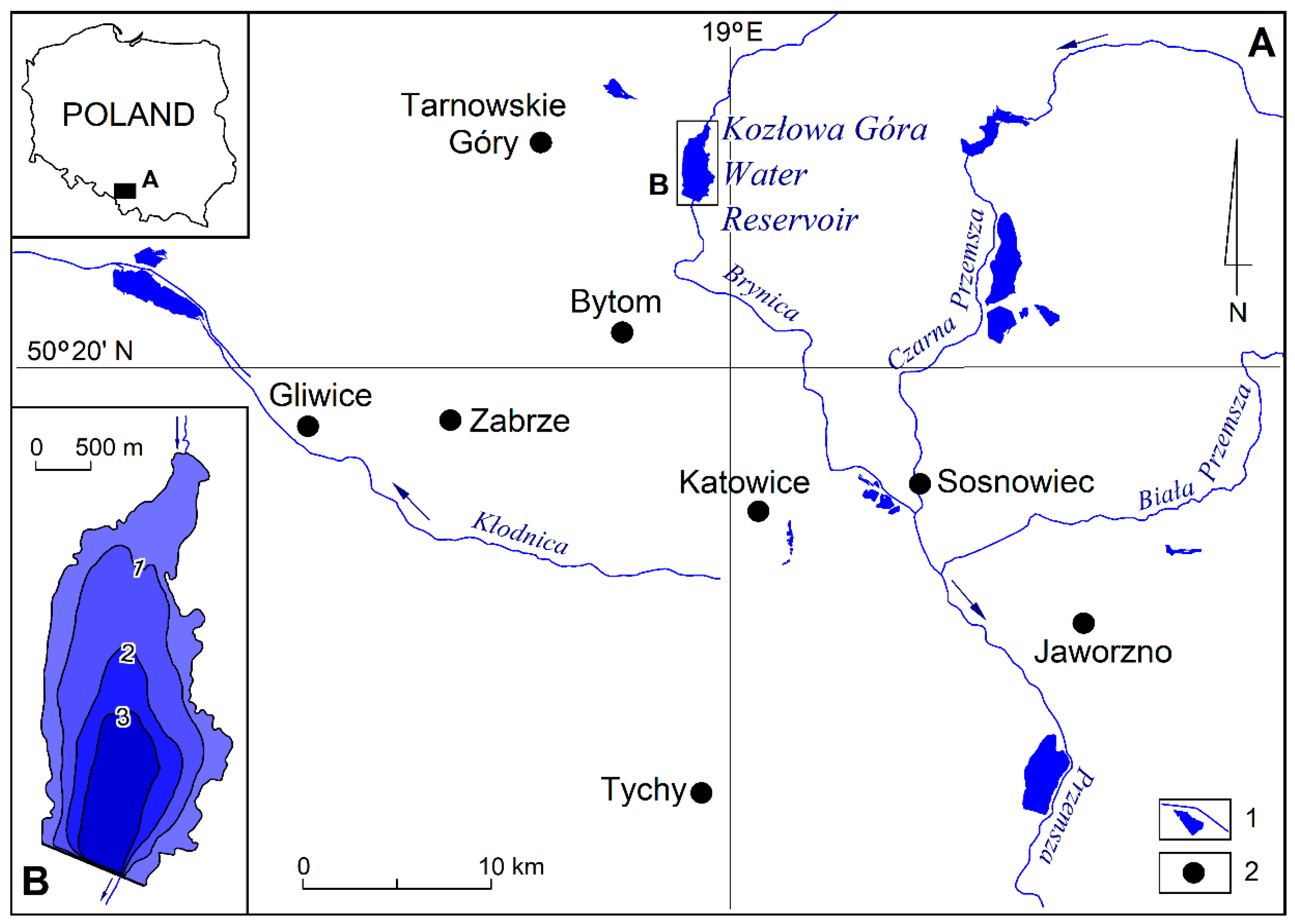
- Rzetala, M.; Jaguś, A. New lake district in Europe: Origin and hydrochemical characteristics. Water Environ. J. 2011, 26, 108–117. [Google Scholar] [CrossRef]
- Jagus, A.; Rzetala, M. Kozłowa Góra Water Reservoir—Functioning and Protection against a Background of Geographical and Limnological Characteristics; Polish Geographical Society—Hydrological Commission: Warsaw, Poland, 2003. [Google Scholar]
- Statistical Yearbook of Poland; Statistics Poland: Warsaw, Poland, 1964–2015.
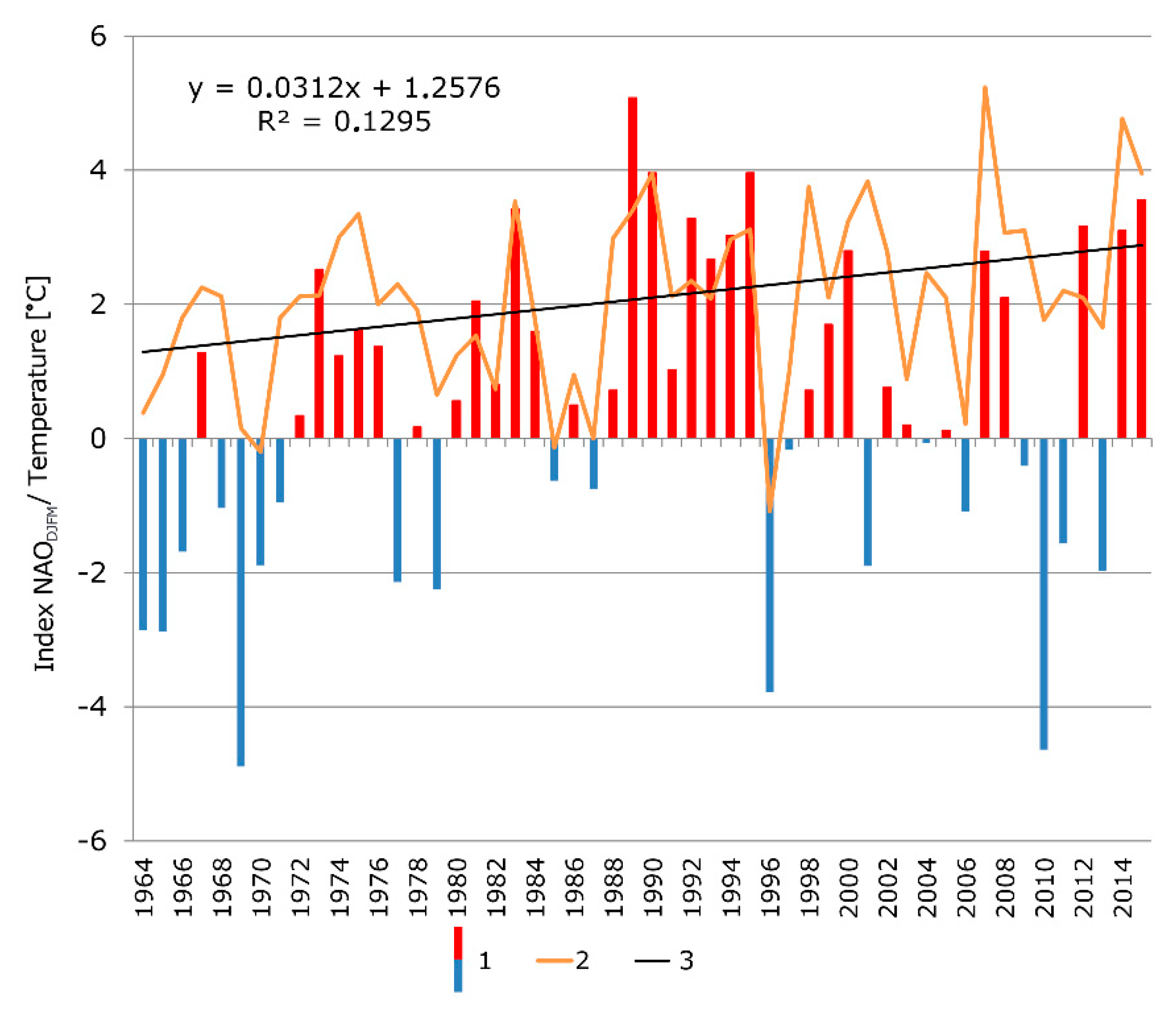
- Hurrell, J.W. Decadal Trends in the North Atlantic Oscillation: Regional Temperatures and Precipitation. Science 1995, 269, 676–679. [Google Scholar] [CrossRef] [Green Version]
- Hurrell Station-Based DJFM NAO Index. Available online: https://climatedataguide.ucar.edu/sites/default/files/nao_station_djfm.txt (accessed on 20 December 2018).
- Kocherlakota, K.; Kocherlakota, S.; Krzanowski, W.J. Principles of Multivariate Analysis, A User’s Perspective; Oxford University Press: Oxford, UK, 2000; pp. 1–608. [Google Scholar]
- Misztal, M. On the Use of Redundancy Analysis to Study the Property Crime in Poland. Acta Univ. Lodz. Folia Oeconomica 2018, 6, 99–109. [Google Scholar] [CrossRef]
- StatSoft Inc. STATISTICA (Data Analysis Software System). Version 10. 2011. Available online: www.statsoft.com (accessed on 20 December 2018).
- Mishra, V.; Cherkauer, K.A.; Bowling, L.C.; Huber, M. Lake Ice phenology of small lakes: Impacts of climate variability in the Great Lakes region. Glob. Planet. Chang. 2011, 76, 166–185. [Google Scholar] [CrossRef]
- Mishra, V.; Cherkauer, K.A.; Bowling, L.C. Changing thermal dynamics of lakes in the Great Lakes region: Role of ice cover feedbacks. Glob. Planet. Chang. 2011, 75, 155–172. [Google Scholar] [CrossRef]
- Williams, G.P. Correlation of freezing and break up with weather conditions. Can. Geotech. J. 1965, 2, 313–326. [Google Scholar] [CrossRef]
- Stefan, H.G.; Fang, X. Simulated climate change effects on ice and snow covers on lakes in a temperate region. Cold Reg. Sci. Technol. 1997, 25, 137–152. [Google Scholar] [CrossRef]
- Assel, R.; Cronk, K.; Norton, D. Recent Trends in Laurentian Great Lakes Ice Cover. Clim. Chang. 2003, 57, 185–204. [Google Scholar] [CrossRef]
- Williams, G.; Layman, K.L.; Stefan, H.G. Dependence of lake ice covers on climatic, geographic and bathymetric variables. Cold Reg. Sci. Technol. 2004, 40, 145–164. [Google Scholar] [CrossRef]
- Leppäranta, M. Modelling the formation and decay of lake ice. In The Impact of Climate Change on European Lakes; George, G., Ed.; Springer: Dordrecht, The Netherland, 2009. [Google Scholar]
- Bernhardt, J.; Engelhardt, C.; Kirilin, G.; Matschullat, J. Lake ice phenology in Berlin-Brandenburg from 1947–2007: Observations and model hindcasts. Clim. Chang. 2011, 112, 791–817. [Google Scholar] [CrossRef]
- Kirilin, G.; Leppäranta, M.; Terzhevik, A.; Granin, N.; Bernhardt, J.; Engelhardt, C.; Efremova, T.; Golosov, S.; Palshin, N.; Sherstyankin, P.; et al. Physics of seasonally ice-covered lakes: A review. Aquat. Sci. 2012, 74, 659–682. [Google Scholar] [CrossRef]
- Korhonen, J. Long-term changes in lake ice cover in Finland. Hydrol. Res. 2006, 37, 347–363. [Google Scholar] [CrossRef]
- Barańczuk, J.; Bajkiewicz-Grabowska, E.; Barańczuk, K.; Staszek, W. The ice regime of Lake Raduńskie Górne (Kashubian Lakeland, Northern Poland). Limnol. Rev. 2017, 17, 61–70. [Google Scholar] [CrossRef] [Green Version]
- Girjatowicz, J.P. The influence of the North Atlantic Oscillation on ice conditions in coastal lakes of the Southern Baltic Sea. Ann. Limnol. Int. J. Limnol. 2003, 39, 71–80. [Google Scholar] [CrossRef] [Green Version]
- Nowak, B.; Nowak, D.; Ptak, M. Variability and course of occurrence of ice cover on selected lakes of the Gnieźnieńskie Lakeland (Central Poland) in the period 1976–2015. In Proceedings of the E3S Web of Conferences 44, 10th Conference on Interdisciplinary Problems in Environmental Protection and Engineering EKO-DOK, Polanica-Zdrój, Poland, 16–18 April 2018. [Google Scholar]
- Wrzesiński, D.; Choiński, A.; Ptak, M.; Skowron, R. Effect of the North Atlantic Oscillation on the Pattern of Lake Ice Phenology in Poland. Acta Geophys. 2015, 63, 1664–1684. [Google Scholar] [CrossRef] [Green Version]
| Item | Index NAO Annual | Index NAODJFM | Index NAONDJ | Index NAODJF | Index NAOJFM | Index NAOFMA | Index NAOMAM |
|---|---|---|---|---|---|---|---|
| Date of ice cover formation | −0.011 p = 0.937 | 0.131 p = 0.354 | 0.433 p = 0.001 | 0.132 p = 0.353 | 0.055 p = 0.699 | −0.004 p = 0.976 | 0.063 p = 0.656 |
| Date of ice cover break up | −0.419 p = 0.002 | −0.559 p = 0.000 | −0.360 p = 0.009 | −0.502 p = 0.002 | −0.542 p = 0.000 | −0.476 p = 0.000 | −0.302 p = 0.029 |
| Number of days with ice cover | −0.334 p = 0.016 | −0.520 p = 0.000 | −0.568 p = 0.000 | −0.492 p = 0.000 | −0.466 p = 0.001 | −0.378 p = 0.006 | −0.288 p = 0.039 |
| Average ice thickness [cm] | −0.454 p = 0.001 | −0.638 p = 0.000 | −0.539 p = 0.000 | −0.610 p = 0.000 | −0.574 p = 0.000 | −0.420 p = 0.002 | −0.335 p = 0.002 |
| Maximum ice thickness [cm] | −0.394 p = 0.004 | −0.587 p = 0.000 | −0.426 p = 0.002 | −0.555 p = 0.000 | −0.520 p = 0.001 | −0.381 p = 0.006 | −0.198 p = 0.159 |
| Item | Average Air Temperature (XI–IV) | Average Air Temperature (XII–III) |
|---|---|---|
| Ice cover formation date | 0.336 p = 0.015 | 0.264 p = 0.058 |
| Ice cover break up date | −0.722 p = 0.000 | −0.664 p = 0.000 |
| Number of days with ice cover | −0.771 p = 0.000 | −0.687 p = 0.000 |
| Average ice thickness [cm] | −0.776 p = 0.000 | −0.809 p = 0.000 |
| Maximum ice thickness [cm] | −0.709 p = 0.000 | 0.728 p = 0.000 |
| Item | Average Air Temperature | ||||
|---|---|---|---|---|---|
| XI | XI–XII | XII | XII–I | I | |
| Date of formation of ice cover | 0.403 p = 0.004 | 0.474 p = 0.000 | 0.350 p = 0.015 | 0.376 p = 0.006 | 0.307 p = 0.034 |
| Item | Average Air Temperature | ||||
|---|---|---|---|---|---|
| II | II–III | III | III–IV | IV | |
| Date of break-up of ice cover | −0.606 p = 0.000 | −0.676 p = 0.000 | −0.610 p = 0.000 | −0.471 p = 0.000 | −0.333 p = 0.071 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Solarski, M.; Rzętała, M. Ice Regime of the Kozłowa Góra Reservoir (Southern Poland) as an Indicator of Changes of the Thermal Conditions of Ambient Air. Water 2020, 12, 2435. https://doi.org/10.3390/w12092435
Solarski M, Rzętała M. Ice Regime of the Kozłowa Góra Reservoir (Southern Poland) as an Indicator of Changes of the Thermal Conditions of Ambient Air. Water. 2020; 12(9):2435. https://doi.org/10.3390/w12092435
Chicago/Turabian StyleSolarski, Maksymilian, and Mariusz Rzętała. 2020. "Ice Regime of the Kozłowa Góra Reservoir (Southern Poland) as an Indicator of Changes of the Thermal Conditions of Ambient Air" Water 12, no. 9: 2435. https://doi.org/10.3390/w12092435
APA StyleSolarski, M., & Rzętała, M. (2020). Ice Regime of the Kozłowa Góra Reservoir (Southern Poland) as an Indicator of Changes of the Thermal Conditions of Ambient Air. Water, 12(9), 2435. https://doi.org/10.3390/w12092435






