Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water
Abstract
:1. Introduction
2. Model Equations and Mathematical Evaluation
3. PNP Equations Solution for the Stern Model
3.1. Boundary Conditions
3.2. Linearized Solution of the PNP Equation
Validation of the Electric Circuit Models for Long Ducts
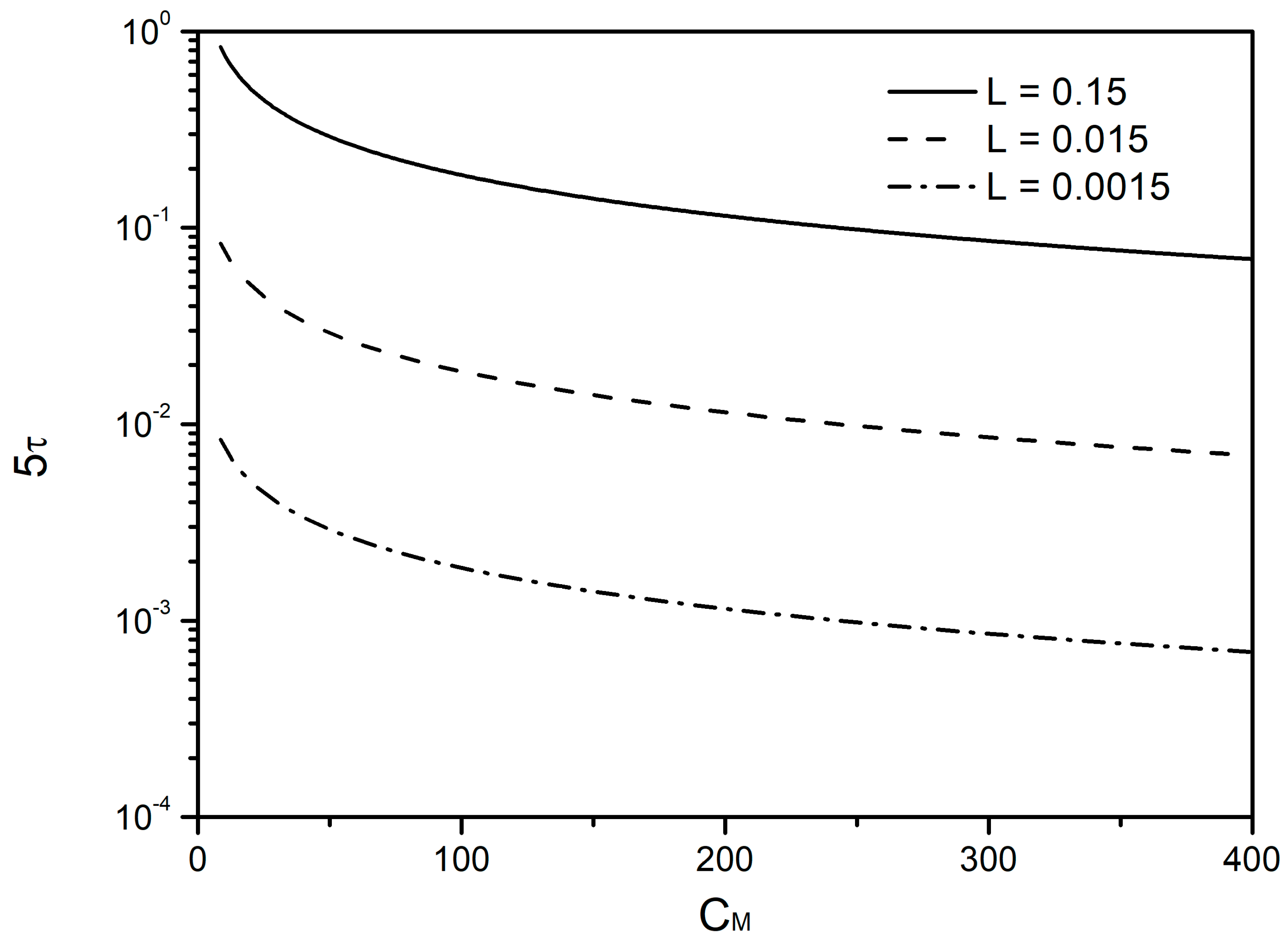
3.3. Long Time Behavior
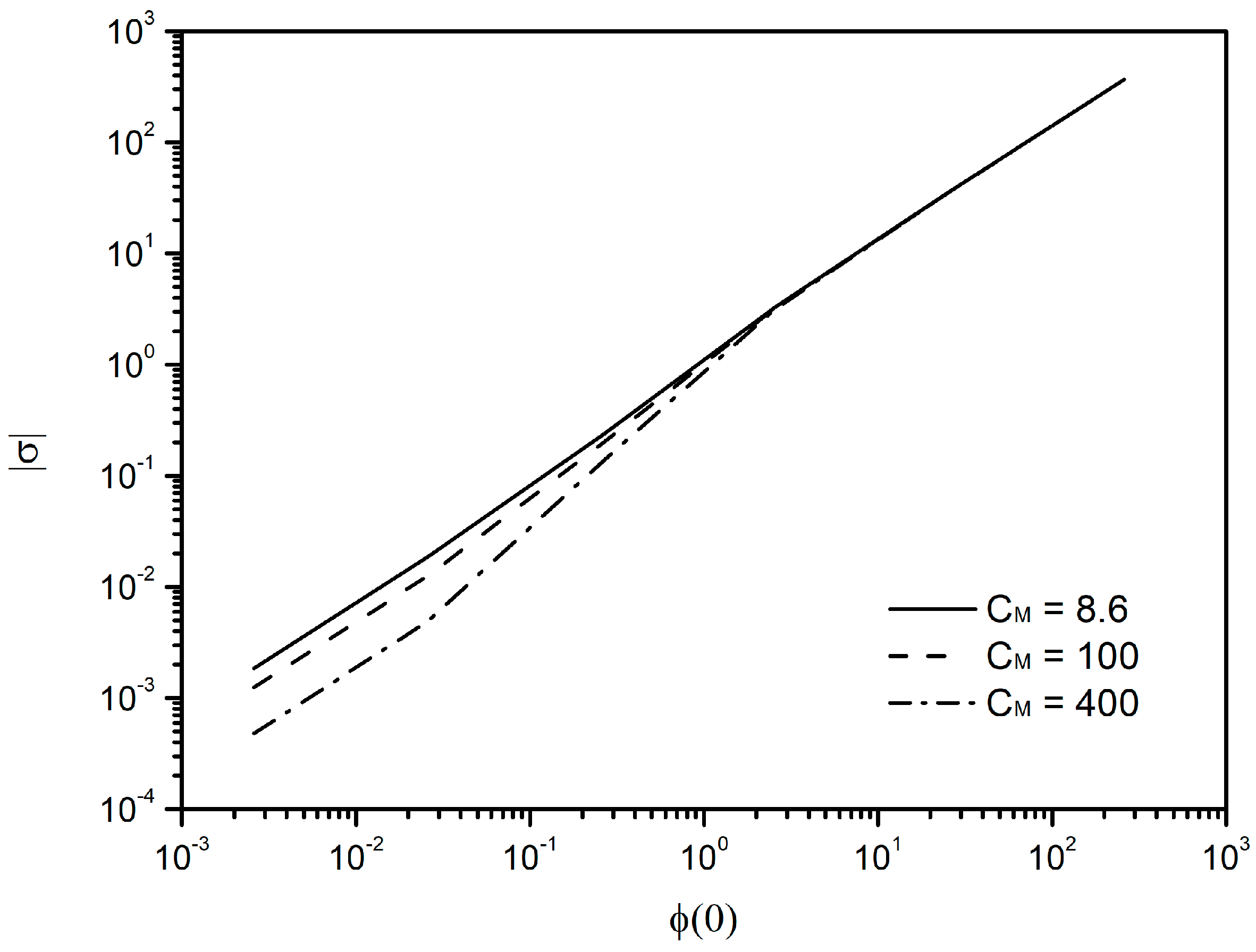
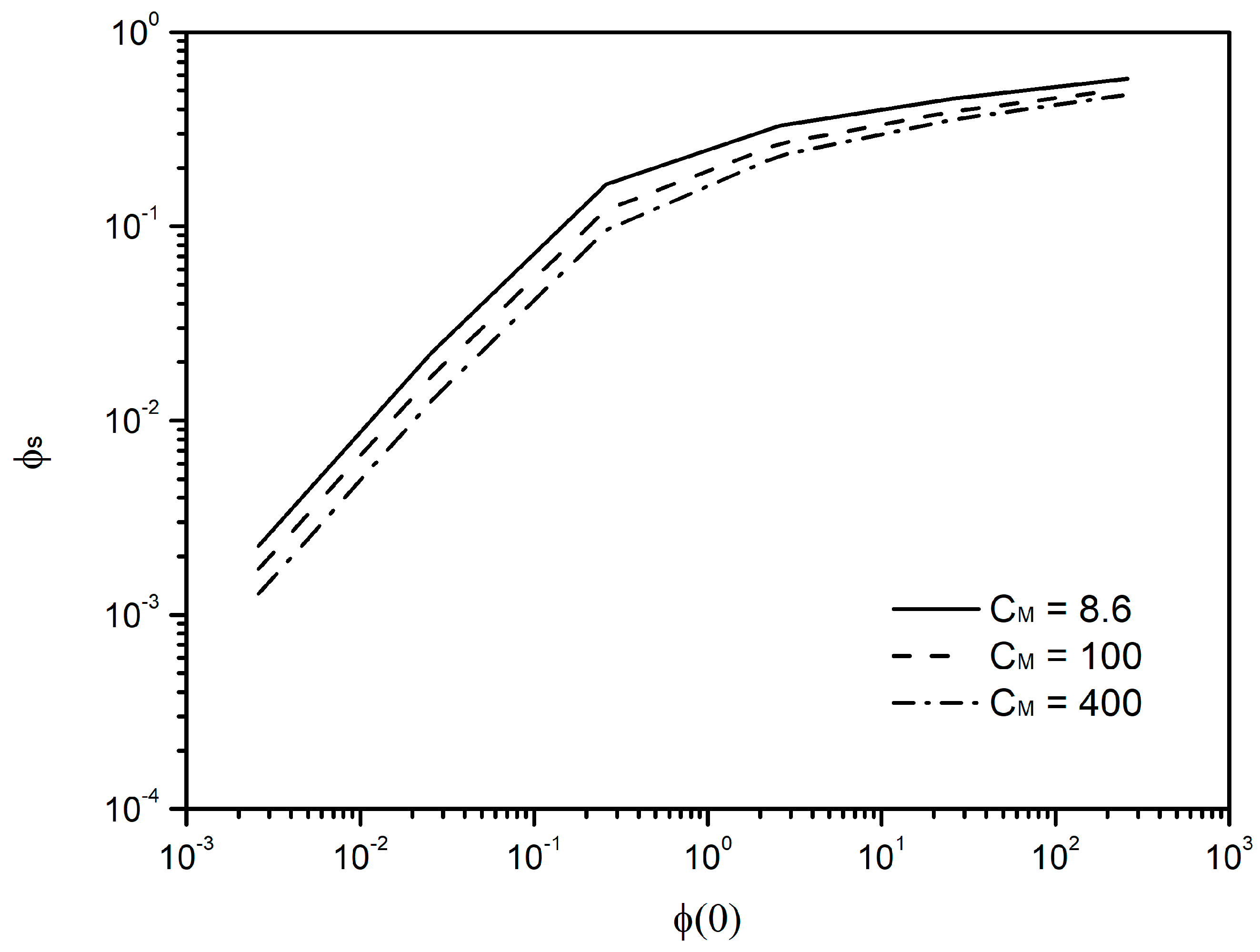
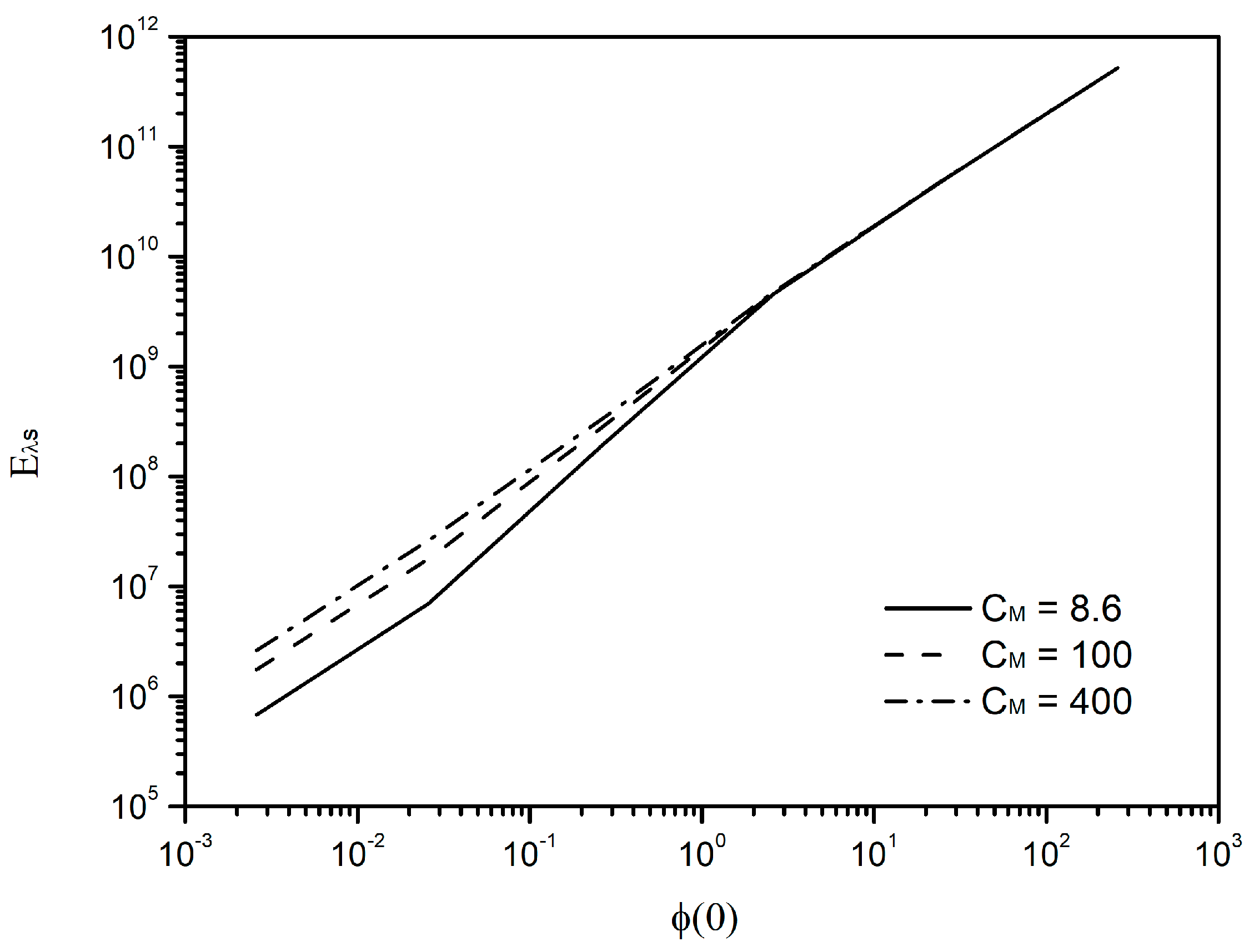
4. Exact Evaluation of the Electric Field and the Surface Charge Density
5. Nonlinear PNP Solution
6. Comparison with Experimental Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Concentration, mole/m3 | |
| μ | Mobility, |
| D | Diffusion coefficient, /s |
| J | Ionic flux, |
| i | Current density, |
| T | Absolute temperature, K |
| z | Number of overflow protons or electrons |
| E | Electric field intensity V/m |
| y | y-axis coordinate, m |
| L | Width of the duct, m |
| σ | Electric charge surface density C/m2 |
| C | Capacity, F |
| Greek symbols | |
| electric permittivity, F/m | |
| φ | Electric potential, V |
| ρ | Charge density, |
| υ | Velocity, m/s |
| ν | Dynamic viscosity, Pa s |
| σ | Surface charge density, C/m2 |
| Subscripts | |
| y | Along y-axis |
| bef | Before |
| after | After |
| Constants | |
Appendix A. General Theory of Ion Movement
Appendix B. The RC Models—Time Scale
Appendix B.1. Gouy-Chapman Model
Appendix B.2. Stern’s Model
- The compact part that is simulated by a Helmholtz capacitor of effective width of the order of molecule that is almost constant. The term effective is due to the possibility that the solvent (i.e., the water here) may not be considered in this region as a continuous media, and the permittivity ε is considered to be similar to the fluid’s bulk. Thus, the width of the compact part may be considered the size of the ionic radius, i.e., [51], while in the present calculations, it is assumed to be equal to . Thus, the capacity of the compact part per unit area is given by .
- The diffuse layer that is simulated by a Gouy–Chapman-type capacitor with effective width of the diffuse part , and the known capacity per area unit is given by .
Appendix C. Solution of the Linear PNP Equation
References
- Setiawan, A.A.; Zhao, Y.; Nayar, C.V. Design, Economic analysis and environmental considerations of mini-grid hybrid power system with reverse osmosis desalination plant for remote areas. Renew. Energy 2009, 34, 374–383. [Google Scholar] [CrossRef]
- Cipollina, A.; Micale, G. Coupling sustainable energy with membrane distillation processes for seawater desalination. In Proceedings of the 2010 1st International Nuclear & Renewable Energy Conference (INREC), Amman, Jordan, 21–24 March 2010. [Google Scholar]
- García-Rodríguez, L. Renewable energy applications in desalination: State of the art. Sol. Energy 2003, 75, 381–393. [Google Scholar] [CrossRef]
- Wilf, M.; Awerbuch, L.; Bartels, C.; Mickley, M.; Pearce, G.; Voutchkov, N. The Guidebook to Membrane Desalination Technology. Reverse Osmosis, Nanofiltration and Hybrid Systems. Processes, Design, Applications; Balaban Desalination Publications: L’Aquila, Italy, 2011. [Google Scholar]
- Guoling, R.; Houjun, F. Technical Progress in Seawater Desalination Technology at Home and Abroad. China Water Wastewater 2008, 20, 86–90. [Google Scholar]
- Mathioulakis, E.; Belessiotis, V.; Delyannis, E. Desalination by using alternative energy: Review and state-of-the-art. Desalination 2007, 203, 346–365. [Google Scholar] [CrossRef]
- Mohamed, E.; Papadakis, G.; Mathioulakis, E.; Belessiotis, V. The effect of hydraulic energy recovery in a small sea water reverse osmosis desalination system; experimental and economical evaluation. Desalination 2005, 184, 241–246. [Google Scholar] [CrossRef]
- Mohamed, E.; Papadakis, G.; Mathioulakis, E.; Belessiotis, V. An experimental comparative study of the technical and economic performance of a small reverse osmosis desalination system equipped with an hydraulic energy recovery unit. Desalination 2006, 194, 239–250. [Google Scholar] [CrossRef]
- Mohamed, E.S.; Papadakis, G.; Mathioulakis, E.; Belessiotis, V. A direct coupled photovoltaic seawater reverse osmosis desalination system toward battery based systems—A technical and economical experimental comparative study. Desalination 2008, 221, 17–22. [Google Scholar] [CrossRef]
- Li, N.; Fane, A.; Winston, W.H.; Matsuura, T. Advanced Membrane Technology and Applications; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Mezher, T.; Fath, H.; Abbas, Z.; Khalid, A. Techno-economic assessment and environmental impacts of desalination technologies. Desalination 2011, 266, 263–273. [Google Scholar] [CrossRef]
- Maerzke, K.A.; Siepmann, J.I. Effects of an Applied Electric Field on the Vapor−Liquid Equilibria of Water, Methanol, and Dimethyl Ether. J. Phys. Chem. B 2010, 114, 4261–4270. [Google Scholar] [CrossRef] [PubMed]
- Bakker, H.J. Structural Dynamics of Aqueous Salt Solutions. Chem. Rev. 2008, 108, 1456–1473. [Google Scholar] [CrossRef] [PubMed]
- Morro, A.; Drouot, R.; Maugin, G.A. Thermodynamics of Polyelectrolyte Solutions in an Electric Field. J. Non-Equilib. Thermodyn. 1985, 10, 131–144. [Google Scholar] [CrossRef]
- Wright, M. An Introduction to Aqueous Electrolyte Solutions; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Gordon, M.J.; Huang, X.; Pentoney, S.L.; Zare, R.N. Capillary Electrophoresis. Science 1988, 242, 224–228. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Strathmann, H. Ion-Exchange Membrane Separation Processes; Elsevier: Amsterdam, The Netherlands, 2004; Volume 9. [Google Scholar]
- Ganzi, C.; Egozy, Y.; Giuffrida, A.; Jha, A. High purity water by electro-deionization. Ultrapure Water J. 1987, 4, 43–50. [Google Scholar]
- Tironi, I.; Sperb, R.; Smith, P.; van Gunsteren, W. A generalized reaction field method for molecular dynamics simulations. J. Chem. Phys. 1995, 102, 5451–5459. [Google Scholar] [CrossRef]
- Berendsen, H.; Postma, J.; van Gunsteren, W. Intermolecular Forces; Pullman, B., Ed.; Reidel Publishing Co.: Dordrecht, The Netherlands, 1981; pp. 331–342. [Google Scholar]
- Wulfsberg, G. Principles of Descriptive Inorganic Chemistry; University Science Books: South Orange, NJ, USA, 1991; pp. 23–31. [Google Scholar]
- Rasaiah, J.; Lynden-Bell, R. Computer simulation studies of the structure and dynamics of ions and non–polar solutes in water. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2001, 359, 1545–1574. [Google Scholar] [CrossRef]
- Belch, A.C.; Berkowitz, M.; McCammon, J.A. Solvation structure of a sodium chloride ion pair in water. J. Am. Chem. Soc. 1986, 108, 1755–1761. [Google Scholar] [CrossRef]
- Das, A.; Tembe, B. The pervasive solvent-separated sodium chloride ion pair in water-DMSO mixtures. Proc. Indian Acad. Sci. (Chem. Sci.) 1999, 111, 353–360. [Google Scholar]
- Murad, S. The role of external electric fields in enhancing ion mobility, drift velocity, and drift–diffusion rates in aqueous electrolyte solutions. J. Chem. Phys. 2011, 134, 114504. [Google Scholar] [CrossRef] [PubMed]
- Sheikholeslami, M.; Sheremet, M.A.; Shafee, A.; Li, Z. CVFEM approach for EHD flow of nanofluid through porous medium within a wavy chamber under the impacts of radiation and moving walls. J. Therm. Anal. Calorim. 2019, 138, 573–581. [Google Scholar] [CrossRef]
- Sofos, F.; Karakasidis, T.E.; Spetsiotis, D. Molecular dynamics simulations of ion separation in nano-channel water flows using an electric field. Mol. Simul. 2019, 45, 1395–1402. [Google Scholar] [CrossRef]
- Suss, M.E.; Porada, S.; Sun, X.; Biesheuvel, P.; Yoon, J.; Presser, V. Water desalination via capacitive deionization: What is it and what can we expect from it? Energy Environ. Sci. 2015, 8, 2296–2319. [Google Scholar] [CrossRef] [Green Version]
- Azamat, J. Functionalized Graphene Nanosheet as a Membrane for Water Desalination Using Applied Electric Fields: Insights from Molecular Dynamics Simulations. J. Phys. Chem. C 2016, 120, 23883–23891. [Google Scholar] [CrossRef]
- Drahushuk, L.W.; Strano, M.S. Mechanisms of Gas Permeation through Single Layer Graphene Membranes. Langmuir 2012, 28, 16671–16678. [Google Scholar] [CrossRef]
- Sun, C.; Boutilier, M.S.H.; Au, H.; Poesio, P.; Bai, B.; Karnik, R.; Hadjiconstantinou, N.G. Mechanisms of Molecular Permeation through Nanoporous Graphene Membranes. Langmuir 2014, 30, 675–682. [Google Scholar] [CrossRef] [PubMed]
- Cohen-Tanugi, D.; Grossman, J.C. Water Desalination across Nanoporous Graphene. Nano Lett. 2012, 12, 3602–3608. [Google Scholar] [CrossRef]
- Humplik, T.; Lee, J.; O’Hern, S.C.; A Fellman, B.; Baig, M.A.; Hassan, S.F.; Atieh, M.; Rahman, F.; Laoui, T.; Karnik, R.; et al. Nanostructured materials for water desalination. Nanotechnology 2011, 22, 292001. [Google Scholar] [CrossRef] [PubMed]
- Suk, M.E.; Aluru, N. Water Transport through Ultrathin Graphene. J. Phys. Chem. Lett. 2010, 1, 1590–1594. [Google Scholar] [CrossRef]
- Wang, E.; Karnik, R. Water Desalination: Graphene Cleans Up Water. Nat. Nanotechnol. 2012, 7, 552–554. [Google Scholar] [CrossRef] [PubMed]
- Ninos, G.; Bartzis, V.; Merlemis, N.; Sarris, I. Uncertainty quantification imlementations in human hemodynamic. Comput. Methods Programs Biomed. 2021, 203, 106021. [Google Scholar] [CrossRef] [PubMed]
- Bartzis, V.; Sarris, I.E. A theoretical model for salt ion drift due to electric field suitable to sea water desalination. Desalination 2020, 473, 114163. [Google Scholar] [CrossRef]
- Oren, Y. Capacitive deionization (CDI) for desalination and water treatment past, present and future (a review). Desalination 2008, 228, 10–29. [Google Scholar] [CrossRef]
- Choia, J.; Dorjib, P.; Shonb, H.; Honga, S. Applications of capacitive deionization: Desalination, softening, selective removal, and energy efficiency. Desalination 2019, 449, 118–130. [Google Scholar] [CrossRef]
- Minhas, M.; Jande, Y.; Kim, W. Hybrid Reverse Osmosis-Capacitive Deionization versus Two-Stage Reverse Osmosis: A Comparative Analysis. Chem. Eng. Technol. 2014, 37, 1137–1145. [Google Scholar] [CrossRef]
- Peters, P.B.; van Roij, R.; Bazant, M.; Biesheuvel, P. Analysis of electrolyte transport through charged nanopores. Phys. Rev. E 2016, 93, 053108. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Biesheuvel, P.M.; Bazant, M. Analysis of ionic conductance of carbon nanotubes. Phys. Rev. E 2016, 94, 050601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tsouris, C.; Mayes, R.; Kiggans, J.; Sharma, K.; Yiacoumi, S.; DePaoli, D.; Dai, S. Mesoporous carbon for capacitive deionization of saline water. Envirom. Sci. Tech. 2011, 45, 10243–10249. [Google Scholar] [CrossRef] [PubMed]
- He, F.; Biesheuvel, P.M.; Bazant, M.; Hatton, T.A. Theory of water treatment by capacitive deionization with redox active porous electrodes. Water Res. 2018, 132, 282. [Google Scholar] [CrossRef] [PubMed]
- Guyes, E.N.; Shocron, A.N.; Simakovski, A.; Biesheuvel, P.M.; Suss, M. A one dimensional model for water desalination by flow-through electrode capacitive deionization. Desalination 2017, 415, 8. [Google Scholar] [CrossRef] [Green Version]
- Bartzis, V.; Sarris, I.E. Electric field distribution and diffuse layer thickness study due to salt ion movement in water desalination. Desalination 2020, 490, 114549. [Google Scholar] [CrossRef]
- Atkins, P.; Paola, J. Atkin’s Physical Chemistry, 8th ed.; Oxford University Press: New York, NY, USA, 2006; Volume 5, pp. 136–169. [Google Scholar]
- Mortimer, R.G. Physical Chemistry, 3rd ed.; Elsevier: Amsterdam, The Netherlands, 2008; Volume 8, pp. 351–378. [Google Scholar]
- Brett, C.A.; Arett, A. Electrochemistry Principles, Methods, and Applications; Oxford University Press: New York, NY, USA, 1994. [Google Scholar]
- Debye, P.; Hückel, E. The theory of electrolytes. I. Lowering of freezing point and related phenomena. Phys. Z. 1923, 24, 185–206. [Google Scholar]
- Bonnefont, A.; Argoul, F.; Bazant, M. Analysis of diffuse-layer effects on time-dependent interfacial kinetics. J. Electroanal. Chem. 2001, 500, 52–61. [Google Scholar] [CrossRef]
- Bazant, M.; Thornton, K.; Ajdari, A. Diffuse-charge dynamics in electrochemical systems. Phys. Rev. E 2004, 70, 021506. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Biesheuvel, P.M.; Dykstra, J.E. The difference between Faradaic and Nonfaradaic processes in Electrochemistry. arXiv 2021, arXiv:1809.02930. [Google Scholar]
- Golovnev, A.; Trimper, S. Exact solution of the Poisson–Nernst–Planck equations in the linear regime. J. Chem. Phys. 2009, 131, 114903. [Google Scholar] [CrossRef] [PubMed]
- Nekrasov, S.A. Calculating the electrostatic field in the bulk of an aqueous solution. Russ. J. Phys. Chem. A 2012, 86, 1732–1735. [Google Scholar] [CrossRef]
- Han, J.-H.; Muralidhar, R.; Waser, R.; Bazant, M.Z. Resistive Switching in Aqueous Nanopores by Shock Electrodeposition. Electrochim. Acta 2016, 222, 370–375. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bartzis, V.; Sarris, I.E. Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water. Water 2021, 13, 2185. https://doi.org/10.3390/w13162185
Bartzis V, Sarris IE. Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water. Water. 2021; 13(16):2185. https://doi.org/10.3390/w13162185
Chicago/Turabian StyleBartzis, Vasileios, and Ioannis E. Sarris. 2021. "Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water" Water 13, no. 16: 2185. https://doi.org/10.3390/w13162185
APA StyleBartzis, V., & Sarris, I. E. (2021). Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water. Water, 13(16), 2185. https://doi.org/10.3390/w13162185






