Numerical Study of Three-Dimensional Surface Jets Emerging from a Fishway Entrance Slot
Abstract
:1. Introduction
- Orifice geometries of the considered fishways consist of vertical slots [16], which differ from nozzles geometrically and with regard to their hydraulic properties. The local head drop induces shear at the slot margins and forces the jet to submerge at the water surface (Figure 1). In addition, the vena contracta effect causes velocities to increase in and downstream of the slot [11].
- Flow in fishway pools is turbulent [17,18] so that flow approaching the slot is inhomogeneous and highly turbulent, whereas flow approaching a nozzle is approximately homogeneous with a low turbulence level [10,11,12,13]. Furthermore, [19] show that different velocity distribution and turbulence intensities impact jet propagation. These differences may influence the propagation of the jet.
- Tailrace geometry, specifically solid boundaries, may influence jet propagation. Wall effects are common near fishway entrances because of the proximity of a nearby river bank. Wall jets create different propagation characteristics compared to unbounded free jets. For two-dimensional wall jets, propagation length increases because turbulent mixing is suppressed at the walls (e.g., [20]). However, fishway entrances are not located immediately next to a lateral wall but with a small offset distance that is usually in the order of one slot width, so that fish may approach the slot from either side [21]. The Coanda effect forces the jet to attach to the wall and the jet follows the pattern of the wall instead of its initial direction [22]. The distance between the slot and the lateral wall denominated as offset determines the attachment point and consequently influences the propagation characteristics of the jet [23,24,25].
2. Material and Methods
2.1. Numerical Methods
2.2. Simulation Setup
- Channel geometry (ch, Figure 2a) for comparison with previous studies;
- Slot geometry with a homogeneous approach flow (sh, Figure 2b) for investigating the effect of a slot;
- Slot geometry with an inhomogeneous approach flow (si, Figure 2c wall not included in the model domain) for identifying the effect of approach flow in combination with a slot;
- Slot geometry with an inhomogeneous approach flow and lateral wall in the tailrace (siw, Figure 2c wall included in the model domain) for determination of the effect of river bank in the tailwater.
2.3. Boundary Conditions
- inflow boundary of ch and sh: inlet velocity boundary with a flowrate of 0.74 m3/s; turbulence intensity = 0;
- inflow boundaries for si and siw: two inlet velocity boundaries with a flowrate of 0.37 m3/s each; turbulence intensity = 0;
- outflow boundary: pressure outlet boundary with a fixed water surface of 1.2 m and a water density of 1000 kg/m3;
- wall boundaries except the slot geometry and the walls of the entrance pool: slip boundary conditions without friction for velocity; and
- wall boundary in the entrance pool and at the slot: fixed value of velocity = 0 m/s and a wall function with wall roughness m.
2.4. Additional Physical Scale Model Investigations
3. Results
3.1. Approach Flow Velocity Distributions
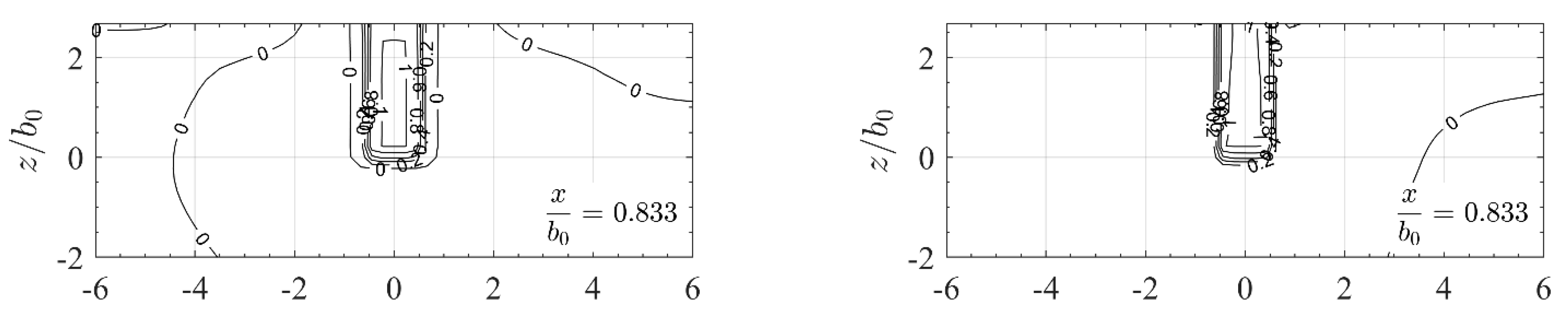
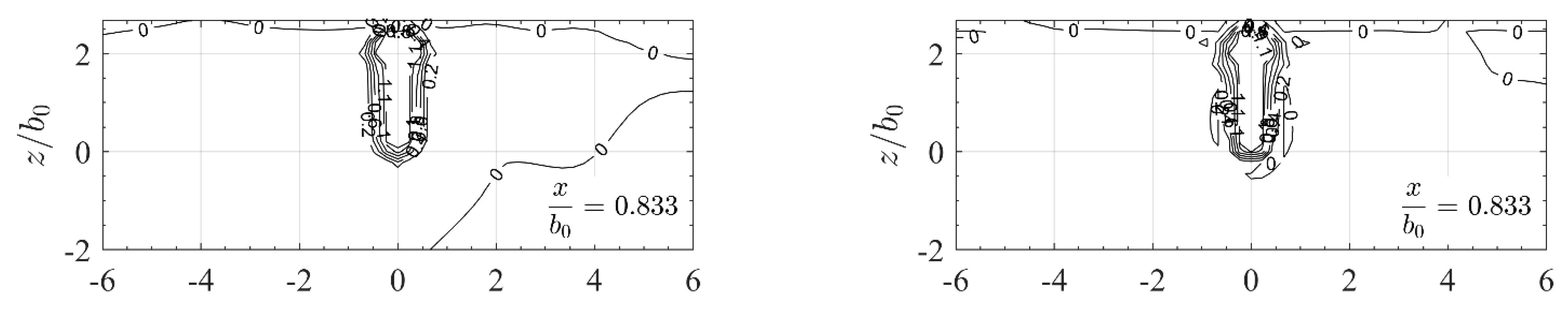
3.2. Qualitative Assessment of the Jet Velocity Fields
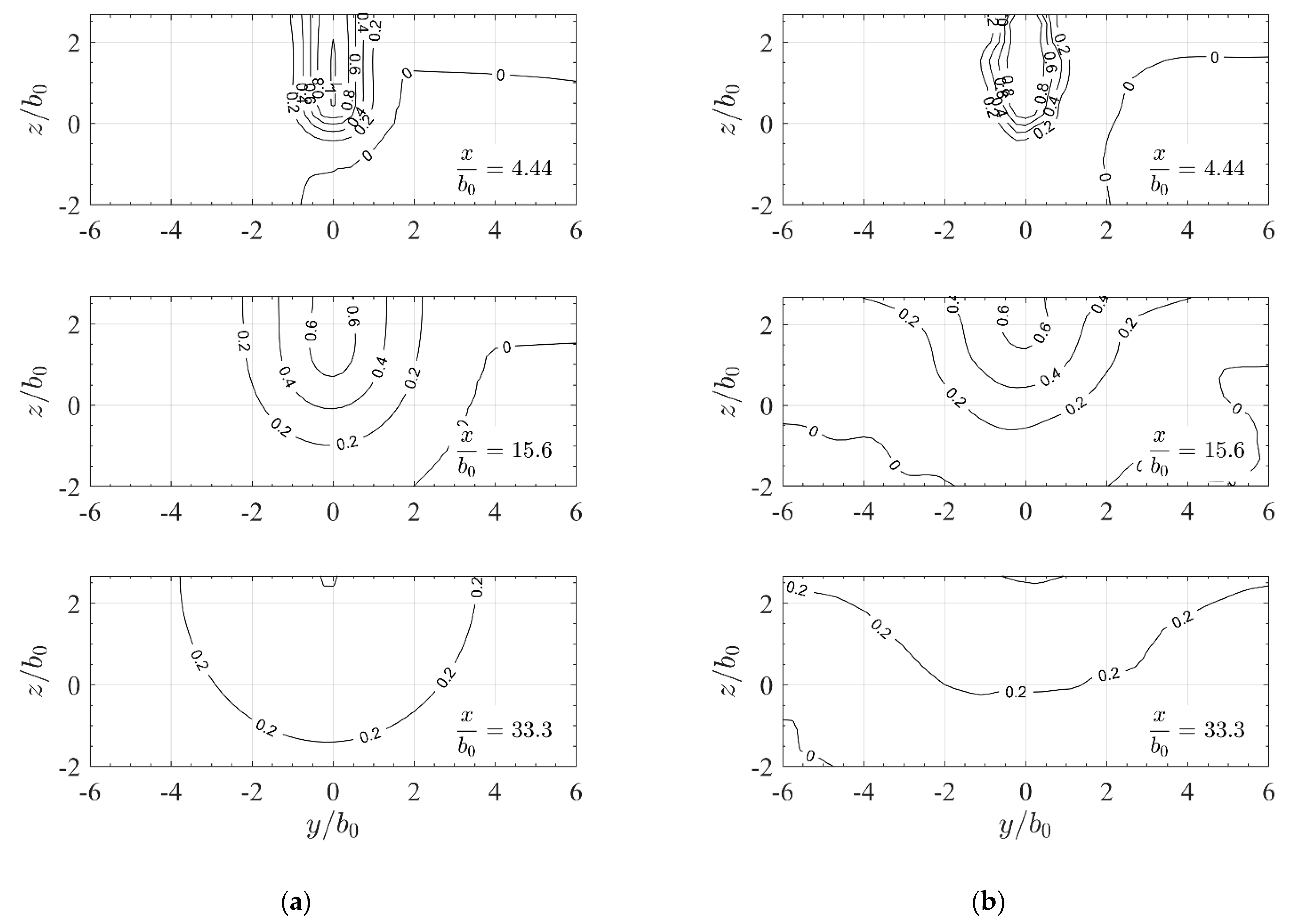
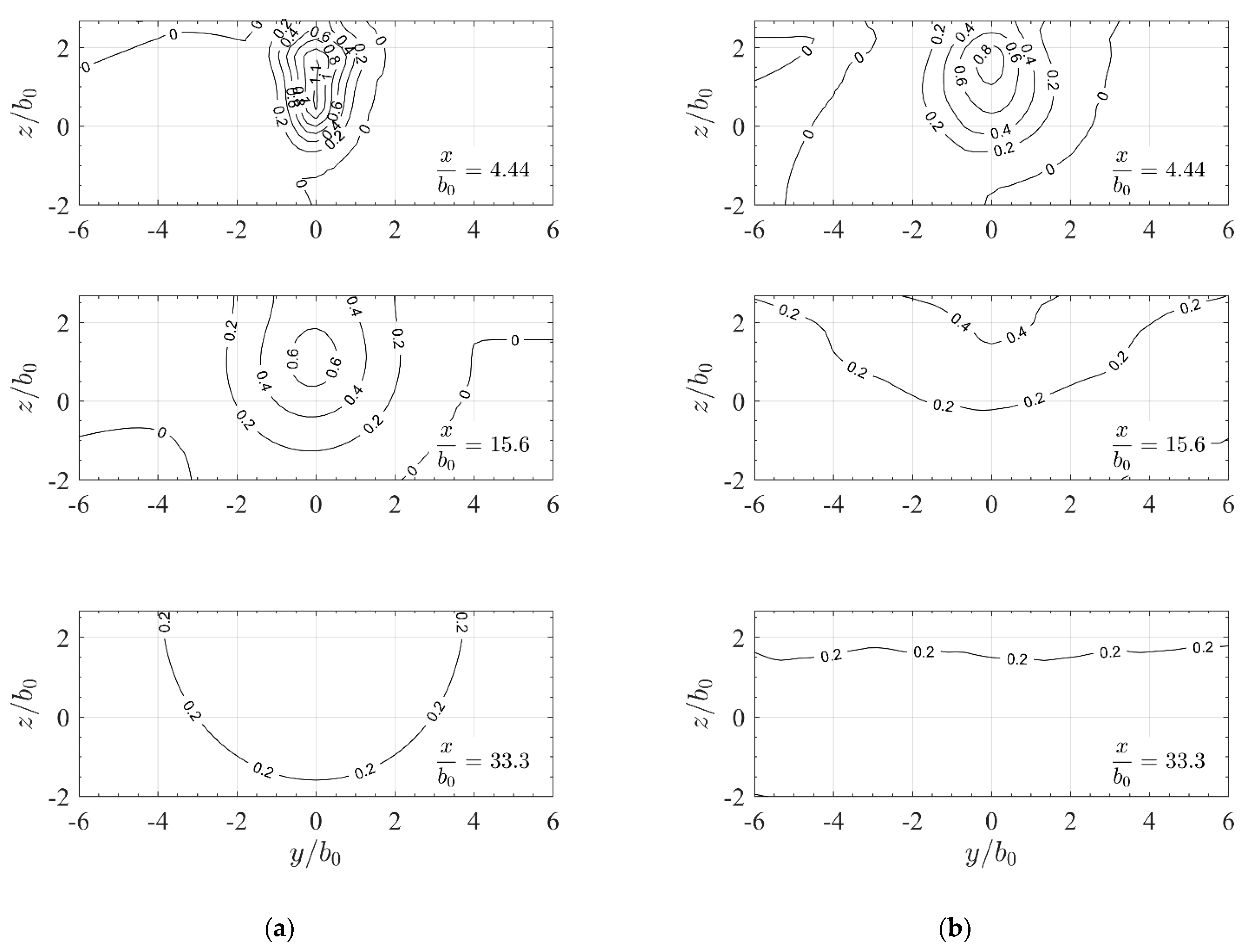
3.3. Influence of Slot and Approach Flow on Free Jet Propagation
3.3.1. Velocity Fields
3.3.2. Jet Half-Width Growth
3.3.3. Self-Similarity
3.3.4. Velocity Decay
3.4. Influence of a Lateral Wall on Jet Propagation
4. Discussion
5. Conclusions
- The impact of a slot with a homogeneous approach flow on jet propagation is limited to the near-field downstream of the orifice.
- Inhomogeneous approach flow of fishways in combination with an entrance slot may reduce propagation length of attraction flow about 50% because of increased lateral spreading.
- A lateral wall in the tailrace enhances the propagation length for a slot set-up with an inhomogeneous approach flow so that length reduction is compensated and propagation of attraction flow is 20% farther downstream than that of a jet with a homogeneous approach flow without a wall.
- Existing analytical methods cannot readily be applied for fishway attraction flow assessment in the presence of a slot and an inhomogeneous approach flow.
- Our results may be applied to provide useful correction factors to account for the inhomogeneous approach flow in combination with slot geometry and the river bank effect for determination of the fishway attraction flow [5].
- DES models resolved the effects of a slot and inhomogeneous, highly turbulent approach flow on jet propagation.
- RANS simulations performed for jets with inhomogeneous and turbulent approach flow overestimated jet propagation in the tailrace.
- The RANS model yielded a more reliable propagation assessment in the presence of a wall or for a homogeneous approach flow.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Larinier, M. Fishways—General considerations. Bull. Fr. Pêche Piscic. 2002, 364, 21–27. [Google Scholar] [CrossRef] [Green Version]
- Williams, J.G.; Armstrong, G.S.; Katopodis, C.; Larinier, M.; Travade, F. Thinking like a fish: A key ingredient for development of effective fish passage facilities at river obstructions. River Res. Appl. 2012, 28, 407–417. [Google Scholar] [CrossRef] [Green Version]
- Musall, M.; Oberle, P.; Nestmann, F.; Fust, A. 3-D-Strömungssimulation zur Bewertung der Leitströmung eines Umgehungsgerinnes am Hochrheinkraftwerk Ryburg-Schwörstadt. Wasserwirtschaft 2008, 98, 37–42. [Google Scholar] [CrossRef]
- Gisen, D.C.; Weichert, R.B.; Nestler, J.M. Optimizing attraction flow for upstream fish passage at a hydropower dam employing 3D Detached-Eddy Simulation. Ecol. Eng. 2017, 100, 344–353. [Google Scholar] [CrossRef] [Green Version]
- Heneka, P.; Zinkhahn, M.; Schütz, C.; Weichert, R.B. A parametric approach for determining fishway attraction flow at hydropower dams. Water 2021, 13, 743. [Google Scholar] [CrossRef]
- Sforza, P.M.; Steiger, M.H.; Trentacoste, N. Studies on three-dimensional viscous jets. AIAA J. 1966, 4, 800–806. [Google Scholar] [CrossRef]
- Kraatz, W. Ausbreitungs- und Mischvorgänge in Strömungen. Ph.D. Thesis, Technische Universität Dresden, Dresden, Germany, 1975. [Google Scholar]
- Demissie, M. Diffusion of Three-Dimensional Slot Jets with Deep and Shallow Submergence. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 1980. [Google Scholar]
- Seo, I.W.; Kwon, S.J. Experimental Investigation of Three-Dimensional Nonbuoyant Rectangular Jets. J. Eng. Mech. 2005, 131, 733–746. [Google Scholar] [CrossRef]
- Quinn, W.R.; Militzer, J. Experimental and numerical study of a turbulent free square jet. Phys. Fluids 1988, 31, 1017–1025. [Google Scholar] [CrossRef]
- Quinn, W.R. Upstream nozzle shaping effects on near field flow in round turbulent free jets. Eur. J. Mech B/Fluids 2006, 25, 279–301. [Google Scholar] [CrossRef]
- Faghani, E.; Maddahian, R.; Faghani, P.; Farhanieh, B. Numerical investigation of turbulent free jet flows issuing from rectangular nozzles: The influence of small aspect ratio. Arch. Appl. Mech. 2010, 80, 727–745. [Google Scholar] [CrossRef]
- Mi, J.; Nathan, G.J. Statistical Properties of Turbulent Free Jets Issuing from Nine Differently-Shaped Nozzles. Flow Turbulence Combust. 2010, 84, 583–606. [Google Scholar] [CrossRef]
- Rajaratnam, N.; Humphries, J.A. Turbulent non-buoyant surface jets. J. Hydraul. Res. 1984, 22, 103–115. [Google Scholar] [CrossRef]
- Gholamreza-Kashi, S.; Martinuzzi, R.J.; Baddour, R.E. Mean Flow Field of a Nonbuoyant Rectangular Surface Jet. J. Hydraul. Eng. 2007, 133, 234–239. [Google Scholar] [CrossRef]
- DWA. Merkblatt DWA-M 509: Fischaufstiegsanlagen und Fischpassierbare Bauwerke—Gestaltung, Bemessung, Qualitätssicherung; DWA-Regelwerk: Hennef, Germany, 2014. [Google Scholar]
- Puertas, J.; Pena, L.; Teijeiro, T. Experimental Approach to the Hydraulics of Vertical Slot Fishways. J. Hydraul. Eng. 2004, 130, 10–23. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.; Zhu, D.Z.; Thiem, J.D.; Cooke, S.J.; Katopodis, C. Field and numerical assessment of turning pool hydraulics in a vertical slot fishway. Ecol. Eng. 2014, 63, 88–101. [Google Scholar] [CrossRef]
- Faghani, E.; Saemi, S.D.; Maddahian, R.; Farhanieh, B. On the effect of inflow conditions in simulation of a turbulent round jet. Arch. Appl. Mech. 2011, 81, 1439–1453. [Google Scholar] [CrossRef]
- Rajaratnam, N. Turbulent Jets; Elsevier Scientific Pub. Co.: Amsterdam, The Netherlands; New York, NY, USA, 1976; ISBN 0-444-41372-3. [Google Scholar]
- Sagnes, P.; Statzner, B. Hydrodynamic abilities of riverine fish: A functional link between morphology and velocity use. Aquat. Living Resour. 2009, 22, 79–91. [Google Scholar] [CrossRef] [Green Version]
- Miozzi, M.; Lalli, F.; Romano, G.P. Experimental investigation of a free-surface turbulent jet with Coanda effect. Exp. Fluids 2010, 49, 341–353. [Google Scholar] [CrossRef]
- Agelin-Chaab, M.; Tachie, M.F. Characteristics of Turbulent Three-Dimensional Offset Jets. J. Fluids Eng. 2011, 133, 2919. [Google Scholar] [CrossRef]
- Gao, N.; Ewing, D. Experimental investigation of planar offset attaching jets with small offset distances. Exp. Fluids 2007, 42, 941–954. [Google Scholar] [CrossRef]
- Dey, S.; Ravi Kishore, G.; Castro-Orgaz, O.; Ali, S.Z. Hydrodynamics of submerged turbulent plane offset jets. Phys. Fluids 2017, 29, 65112. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Schulze, L.; Thorenz, C. The Multiphase Capabilities of the CFD Toolbox OpenFOAM for Hydraulic Engineering Applications. In Proceedings of the 11th International Conference on Hydroscience & Engineering, ICHE, Hamburg, Germany, 28 September–2 October 2014; Lehfeldt, R., Kopmann, R., Eds.; Bundesanstalt für Wasserbau: Karlsruhe, Germany, 2014; pp. 1007–1014. ISBN 978-3-939230-32-8. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten Years of Industrial Experience with the SST Turbulence Model. In Turbulence, Heat and Mass Transfer 4: Proceedings of the Fourth International Symposium on Turbulence, Heat and Mass Transfer, Antalya, Turkey, 12–17 October 2003; Hanjalić, K., Nagano, Y., Tummers, M.J., Eds.; Begell House: New York, NY, USA, 2003; ISBN 1567001963. [Google Scholar]
- Rodi, W. Turbulence Modeling and Simulation in Hydraulics: A Historical Review. J. Hydraul. Eng. 2017, 143. [Google Scholar] [CrossRef]
- Besbes, S.; Mhiri, H.; Le Palec, G.; Bournot, P. Numerical and experimental study of two turbulent opposed plane jets. Heat Mass Transf. 2003, 39, 675–686. [Google Scholar] [CrossRef]
- Tomac, M.N.; Gregory, J.W. Oscillation characteristics of mutually impinging dual jets in a mixing chamber. Phys. Fluids 2018, 30, 117102. [Google Scholar] [CrossRef] [Green Version]
- Rajaratnam, N.; Katopodis, C.; Solanki, S. New designs for vertical slot fishways. Can. J. Civ. Eng. 1992, 19, 402–414. [Google Scholar] [CrossRef]
- Bombač, M.; Četina, M.; Novak, G. Study on flow characteristics in vertical slot fishways regarding slot layout optimization. Ecol. Eng. 2017, 107, 126–136. [Google Scholar] [CrossRef]
- Schmieder, H. Experimentelle Untersuchung von Oberflächennahen Freistrahlen bei Variation der Einlassgeometrie eines Fischpasses. Bachelor’s Thesis, Karlsruhe Institute of Technology, Karlsruhe, Germany, 2018. [Google Scholar]
- Wygnanski, I.; Fiedler, H. Some measurements in the self-preserving jet. J. Fluid Mech. 1969, 38, 577–612. [Google Scholar] [CrossRef] [Green Version]
- Sokoray-Varga, B. Detecting Flow Events in Turbulent Flow of Vertical-Slot Fish Passes. Ph.D. Thesis, Karlsruher Institut für Technologie, Karlsruhe, Germany, 2016. [Google Scholar]
- Ostermann, F.; Woszidlo, R.; Nayeri, C.N.; Paschereit, C.O. Properties of a sweeping jet emitted from a fluidic oscillator. J. Fluid Mech. 2018, 857, 216–238. [Google Scholar] [CrossRef] [Green Version]
- Walker, D.T. On the origin of the ‘surface current’ in turbulent free-surface flows. J. Fluid Mech. 1997, 339, 275–285. [Google Scholar] [CrossRef]
- Yan, Z.; Zhong, Y.; Lin, W.E.; Savory, E.; You, Y. Evaluation of RANS and LES turbulence models for simulating a steady 2-D plane wall jet. Eng. Comput. 2018, 35, 211–234. [Google Scholar] [CrossRef]










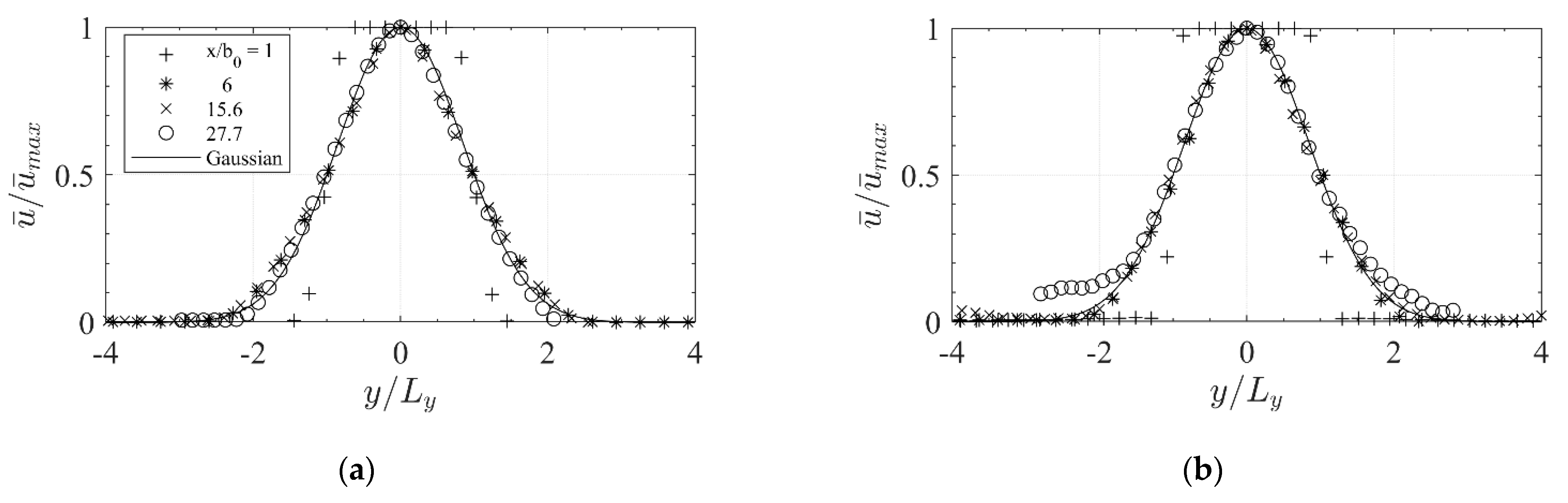
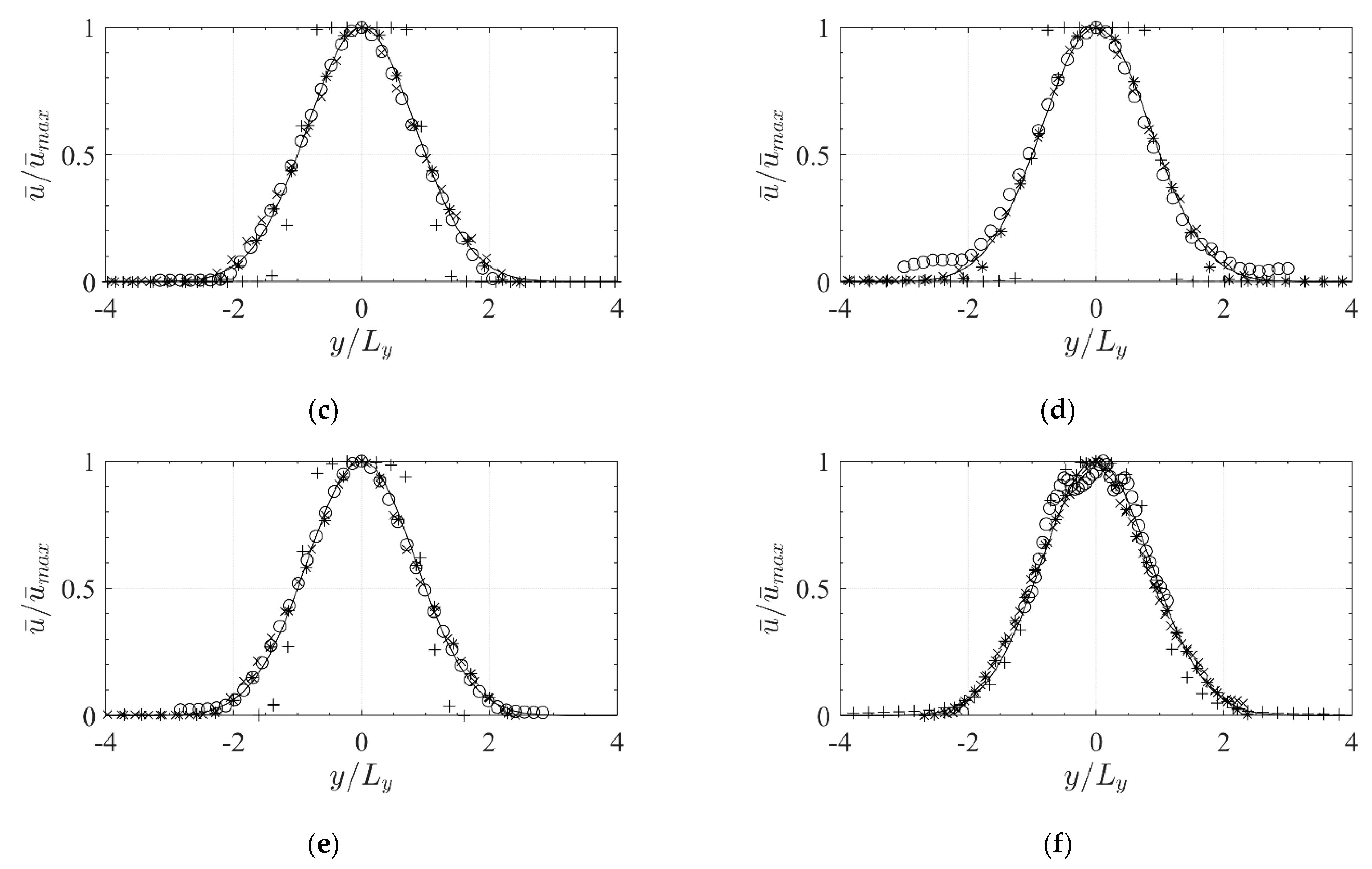

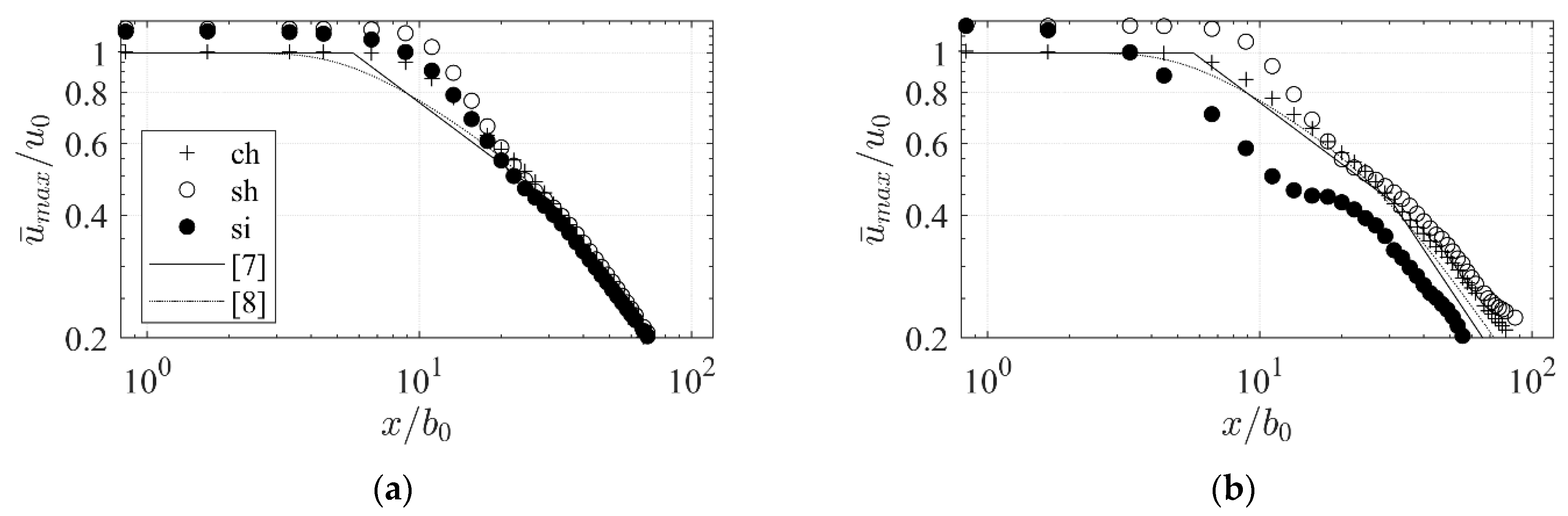


| Configuration | Case Name | Tailrace Condition | Orifice Geometry | Turbulence Model | Approach Flow |
|---|---|---|---|---|---|
| ch | ch-rans | Surface free jet | channel | RANS | homogeneous |
| ch | ch-des | Surface free jet | channel | DES | homogeneous |
| sh | sh-rans | Surface free jet | slot | RANS | homogeneous |
| sh | sh-des | Surface free jet | slot | DES | homogeneous |
| si | si-rans | Surface free jet | slot | RANS | inhomogeneous |
| si | si-des | Surface free jet | slot | DES | inhomogeneous |
| siw | siw-rans | Surface wall jet | slot | RANS | inhomogeneous |
| siw | siw-des | Surface wall jet | slot | DES | inhomogeneous |
| Paper | Jet Type | |
|---|---|---|
| Miozzi et al. [22] | Plane surface jet | 0.10 |
| Kashi [15] | Rectangular surface jet | 0.12 |
| Rajaratnam and Humphries [14] | Circular or rectangular surface jet | 0.09 |
| Present study | Rectangular surface channel jet (ch) | 0.10 |
| Present study | Rectangular surface slot jet (sh) | 0.10 |
| Present study | Rectangular surface slot jet (si) | 0.25 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahl, L.; Heneka, P.; Henning, M.; Weichert, R.B. Numerical Study of Three-Dimensional Surface Jets Emerging from a Fishway Entrance Slot. Water 2021, 13, 1079. https://doi.org/10.3390/w13081079
Mahl L, Heneka P, Henning M, Weichert RB. Numerical Study of Three-Dimensional Surface Jets Emerging from a Fishway Entrance Slot. Water. 2021; 13(8):1079. https://doi.org/10.3390/w13081079
Chicago/Turabian StyleMahl, Lena, Patrick Heneka, Martin Henning, and Roman B. Weichert. 2021. "Numerical Study of Three-Dimensional Surface Jets Emerging from a Fishway Entrance Slot" Water 13, no. 8: 1079. https://doi.org/10.3390/w13081079
APA StyleMahl, L., Heneka, P., Henning, M., & Weichert, R. B. (2021). Numerical Study of Three-Dimensional Surface Jets Emerging from a Fishway Entrance Slot. Water, 13(8), 1079. https://doi.org/10.3390/w13081079






