3D Printing Technique for Experimental Modeling of Hydraulic Structures: Exemplary Scaled Weir Models
Abstract
:1. Introduction
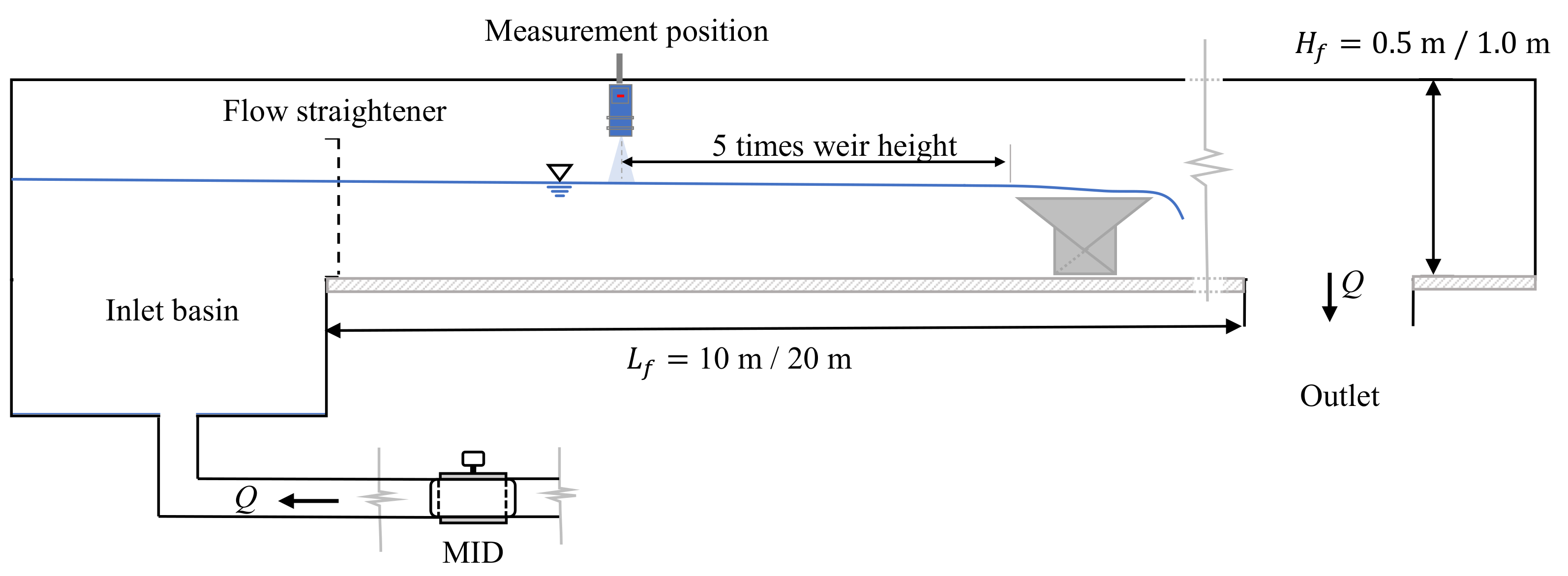
2. Experimental Setup and Methods
3. Comparison between 3D Printing and Conventional Fabrication Process
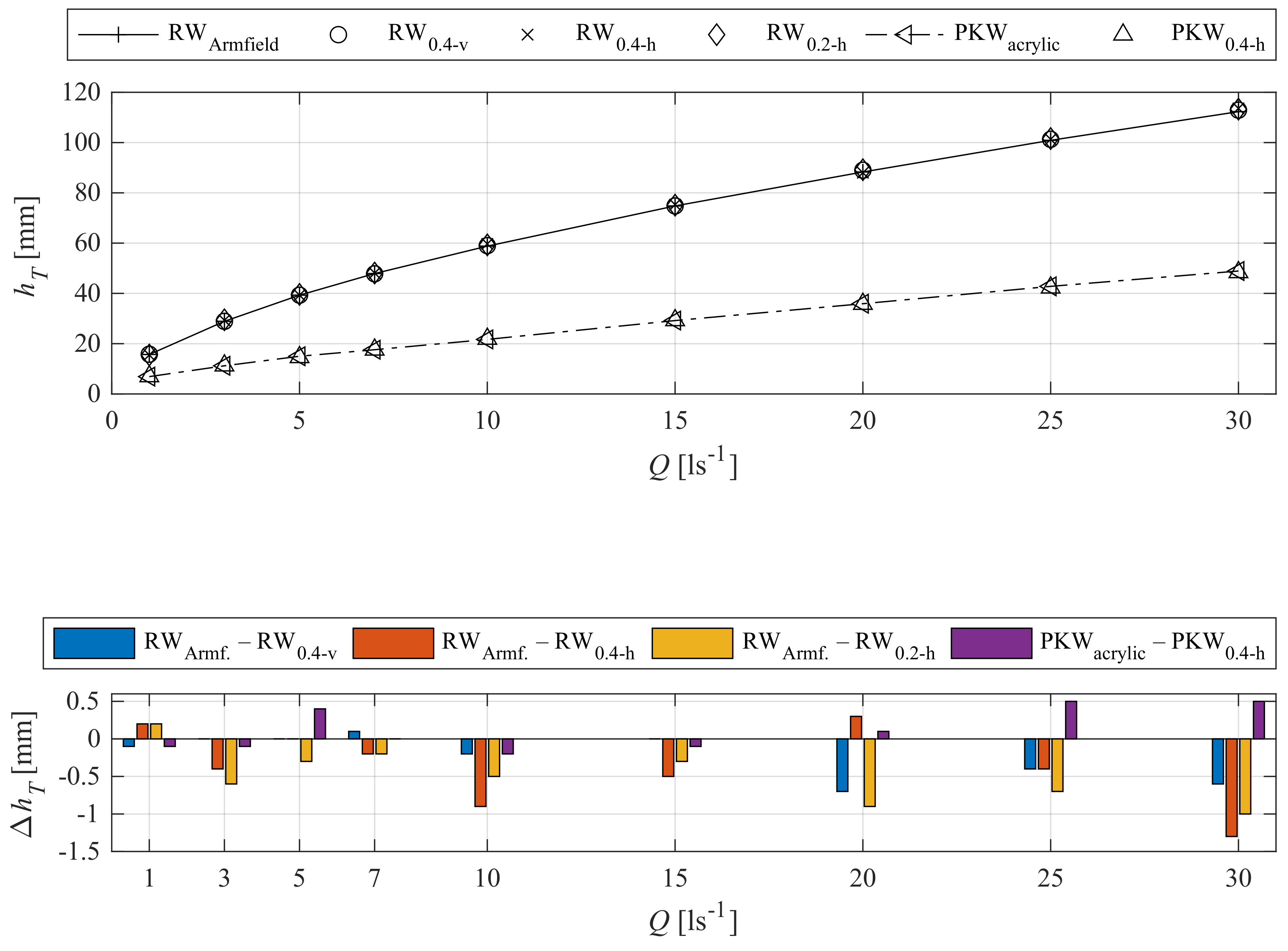
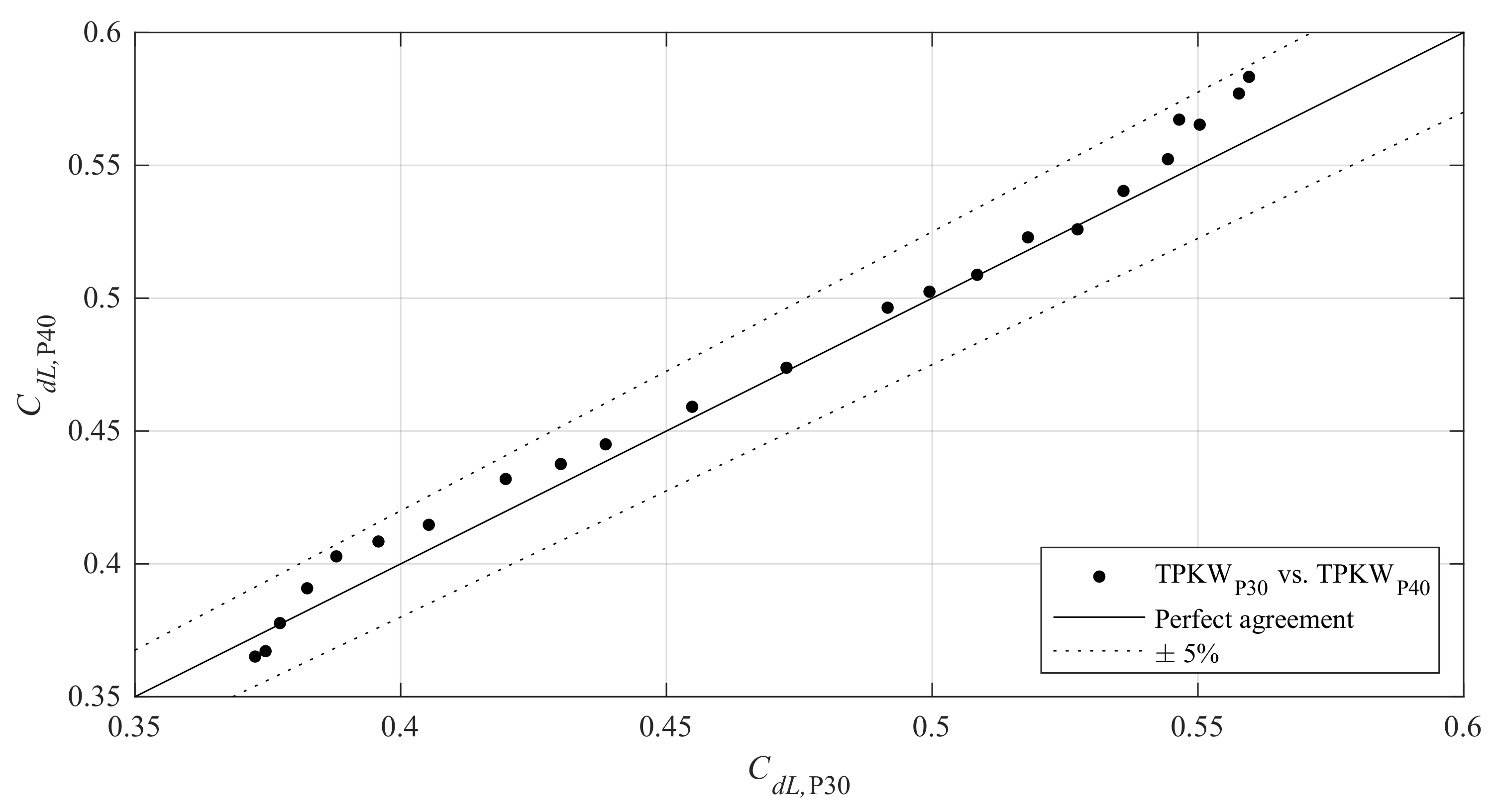
4. Exemplary Experimental Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Notations
| B | model length (m) |
| discharge coefficient related to total crest length (m) | |
| g | gravity acceleration (m s) |
| H | model height (m) |
| flume depth (m) | |
| 3D plotter printing domain (m) | |
| upstream total head (m) | |
| L | total crest length of tested weirs (m) |
| flume length (m) | |
| 3D plotter printing domain (m) | |
| P | weir height (m) |
| Q | discharge (l s) |
| regular linear weirs | |
| reference regular linear weir model fabricated by the company Armfield | |
| regular linear weirs 3D printed with horizontal layers and 0.2 mm layer height | |
| regular linear weirs 3D printed with horizontal layers and 0.4 mm layer height | |
| regular linear weirs 3D printed with vertical layers and 0.4 mm layer height | |
| piano key weir with rectangular plan form geometry | |
| reference piano key weir model fabricated conventionally with acrylic glass | |
| piano key weir model 3D printed with horizontal layers and 0.4 mm layer height | |
| piano key weir with trapezoidal plan form geometry | |
| 3D printed trapezoidal piano key weir with 30 cm weir height | |
| 3D printed trapezoidal piano key weir with 40 cm weir height | |
| W | model width (m) |
| flume width (m) | |
| piano key weir inlet key width (m) | |
| piano key weir outlet key width (m) | |
| 3D plotter printing domain (m) |
References
- Bung, D.B. Laboratory Models of Free-Surface Flows. In Rivers—Physical, Fluvial and Environmental Processes; Rowiński, P., Radecki-Pawlik, A., Eds.; GeoPlanet: Earth and Planetary Sciences; Springer: Cham, Switzerland; Heidelberg, Germany; New York, NY, USA; Dordrecht, The Netherlands; London, UK, 2015; ISBN 978-3-319-17718-2. [Google Scholar] [CrossRef]
- Erpicum, S.; Crookston, B.M.; Bombardelli, F.; Bung, D.B.; Felder, S.; Mulligan, S.; Oertel, M.; Palermo, M. Hydraulic structures engineering: An evolving science in a changing world. WIREs Water 2019, 8, e1505. [Google Scholar] [CrossRef]
- USBR. Hydraulic Laboratory Techniques, A Guide for Applying Engineering Knowledge to Hydraulic Studies Based on 50 Years of Research and Testing Experience; United States Bureau of Reclamation (USBR), Department of the Interior, Water and Power Resources Service: Denver, CO, USA, 1980. [Google Scholar]
- Crookston, B.M.; Erpicum, S.; Tullis, B.P.; Laugier, F. Hydraulics of Labyrinth and Piano Key Weirs: 100 Years of Prototype Structures, Advancements, and Future Research Needs. ASCE J. Hydraul. Eng. 2019, 145, 02519004. [Google Scholar] [CrossRef]
- Gibson, I.; Rosen, D.; Stucker, B. Additive Manufacturing Technologies: 3D Printing, Rapid Prototyping, and Direct Digital Manufacturing, 2nd ed.; Springer: New York, NY, USA, 2015; ISBN 978-1-4939-2112-6. [Google Scholar]
- Jacobs, P. Rapid prototyping and manufacturing: Fundamentals of stereolithography. In Society of Manufacturing Engineers, 1st ed.; Society of Manufacturing Engineers: Dearborn, MI, USA, 1992; ISBN 0-87263-425-6. [Google Scholar]
- Rülke, C.M. Audi Expands Polymer 3D Printing in Production. Audi Mediacenter. 2019. Available online: https://www.audi-mediacenter.com/en/press-releases/audi-expands-polymer-3d-printing-in-production-11466 (accessed on 24 August 2021).
- Jones, R.; Haufe, P.; Sells, E.; Iravani, P.; Olliver, V.; Palmer, C.; Bowyer, A. RepRap—The Replicating Rapid Prototyper, Robotica; Cambridge University Press: Cambridge, UK, 2011; Volume 29, pp. 177–191. [Google Scholar]
- Bowyer, A. 3D printing and humanity’s first imperfect replicator. 3D Print. Addit. Manuf. 2014, 1, 4–5. [Google Scholar] [CrossRef]
- Bertin, S.; Friedrich, H.; Delmas, P.; Chan, E.; Gimelfarb, G. DEM quality assessment with a 3D printed gravel bed applied to stereo photogrammetry. Photogramm. Rec. 2019, 29, 241–246. [Google Scholar] [CrossRef]
- Hodge, R.A.; Hoey, T.B. A Froude-scaled model of a bedrock-alluvial channel reach: 1. Hydraulics. JGR Earth Surf. 2016, 121, 1578–1596. [Google Scholar] [CrossRef] [Green Version]
- Mazen, A.; McClanahan, B.; Weaver, J.M. Factors affecting ultimate tensile strength and impact toughness of 3D printed parts using fractional factorial design. Int. J. Adv. Manuf. Technol. 2022, 119, 2639–2651. [Google Scholar] [CrossRef]
- O’Brien, J.H.; Evers, J.; Tempelman, E. Surface roughness of 3D printed materials: Comparing physical model measurements and human perception. Mater. Today Commun. 2019, 19, 300–305. [Google Scholar] [CrossRef]
- Fuad, J.S. Quantifying the Surface Roughness of a 3D Printed Model; The University of New South Wales Canberra at the Australian Defence Force Academy: Campbell, ACT, Australia, 2019. [Google Scholar]
- Oertel, M. Piano key weir research: State-of-the-art and future challenges. In Proceedings of the 7th International Symposium on Hydraulic Structures, Aachen, Germany, 15–18 May 2018; pp. 1–8. [Google Scholar]
- Shen, X.; Oertel, M. Comparative study of nonsymmetrical trapezoidal and rectangular Piano Key Weirs with varying key width ratios. ASCE J. Hydraul. Eng. 2021, 147, 04021045. [Google Scholar] [CrossRef]
- Pfister, M.; Boillat, J.L.; Schleiss, A.; Laugier, F.; Leite Ribeiro, M. Piano Key Weirs as efficient spillway structure. In Proceedings of the 24th Congress of CIGB—ICOLD, EPFL, Kyoto, Japan, 2–8 June 2012. [Google Scholar]
- Oertel, M. Size-scale effects of an a-type piano key weir. In Proceedings of the 38th IAHR World Congress–Water: Connecting the World, Panama City, Panama, 1–6 September 2019; pp. 1930–1939. [Google Scholar]
- Tullis, B.P.; Crookston, B.M.; Young, N. Scale effects in free-flow nonlinear weir head-discharge relationships. ASCE J. Hydraul. Eng. 2020, 146, 04019056. [Google Scholar] [CrossRef]

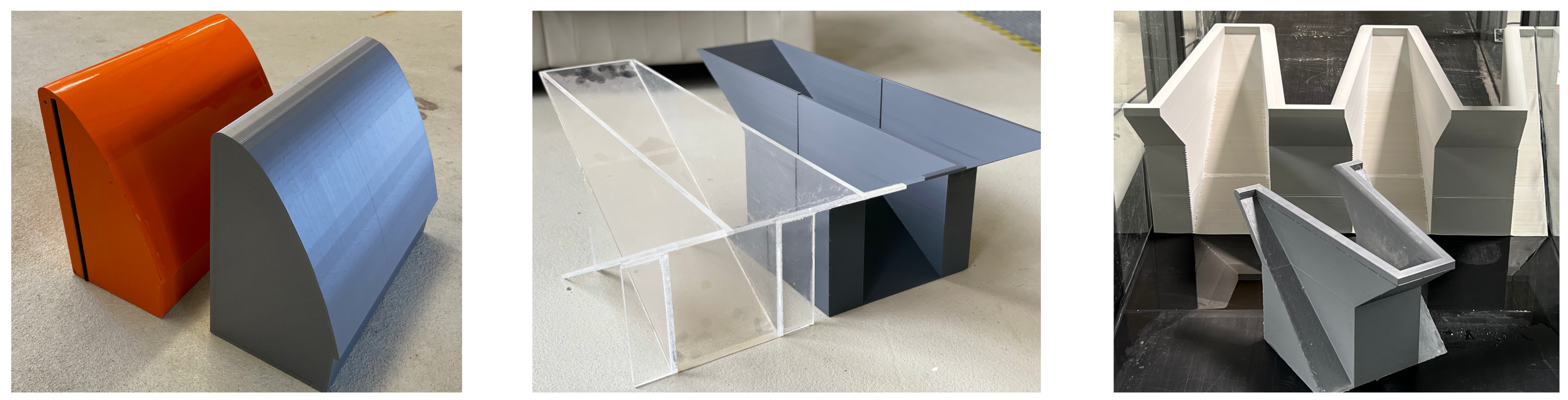





| Model | Layer Orientation | Material | Layer Height (mm) | P (mm) | L * (mm) | B (mm) | W (mm) | / (mm) |
|---|---|---|---|---|---|---|---|---|
| Regular Weir models (RW) | ||||||||
| RW | - | GRP ** | - | 239 | 300 | 133 | 300 | - |
| RW | horizontal | PLA | 0.2 | 239 | 300 | 133 | 300 | - |
| RW | horizontal | PLA | 0.4 | 239 | 300 | 133 | 300 | - |
| RW | vertical | PLA | 0.4 | 239 | 300 | 133 | 300 | - |
| Rectangular Piano Key Weir models (RPKW) | ||||||||
| RPKW | - | Acrylic glass | - | 315 | 1858 | 789 | 300 | 1.5 |
| RPKW | horizontal | PLA | 0.4 | 315 | 1858 | 789 | 300 | 1.5 |
| Trapezoidal Piano Key Weir models (TPKW) | ||||||||
| TPKW | horizontal | PLA | 0.4 | 300 | 1103 | 480 | 300 | 1.0 |
| TPKW | horizontal | PLA | 0.6 | 400 | 1356 | 640 | 1000 | 1.0 |
| Model | Material | Layer Height | Infill Density | Model Weight | Cost (Euro) | Time (Hours) |
|---|---|---|---|---|---|---|
| Regular linear weir models (RW) | ||||||
| RW | PLA | 0.2 mm | 15% | 1.6 kg | ∼40 | 120 |
| RW | PLA | 0.4 mm | 15% | 1.8 kg | ∼45 | 42 |
| RW | PLA | 0.4 mm | 15% | 1.8 kg | ∼45 | 40 |
| Rectangular Piano Key Weir models (RPKW) | ||||||
| RPKW | acrylic glass | - | - | 8 kg | ∼300 | 180 |
| RPKW | PLA | 0.4 mm | 15% | 4.6 kg | ∼115 | 96 |
| Trapezoidal Piano Key Weir models (TPKW) | ||||||
| TPKW | PLA | 0.4 mm | 15% | 2 kg | ∼50 | 50 |
| TPKW | PLA | 0.6 mm | 15% | 24 kg | ∼750 | 192 |
| Advantages | Disadvantages |
|---|---|
|
|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oertel, M.; Shen, X. 3D Printing Technique for Experimental Modeling of Hydraulic Structures: Exemplary Scaled Weir Models. Water 2022, 14, 2153. https://doi.org/10.3390/w14142153
Oertel M, Shen X. 3D Printing Technique for Experimental Modeling of Hydraulic Structures: Exemplary Scaled Weir Models. Water. 2022; 14(14):2153. https://doi.org/10.3390/w14142153
Chicago/Turabian StyleOertel, Mario, and Xiaoyang Shen. 2022. "3D Printing Technique for Experimental Modeling of Hydraulic Structures: Exemplary Scaled Weir Models" Water 14, no. 14: 2153. https://doi.org/10.3390/w14142153
APA StyleOertel, M., & Shen, X. (2022). 3D Printing Technique for Experimental Modeling of Hydraulic Structures: Exemplary Scaled Weir Models. Water, 14(14), 2153. https://doi.org/10.3390/w14142153






