Conceptual Model Based on Groundwater Dynamics in the Northern Croatian Dinaric Region at the Transition from the Deep Karst and Fluviokarst
Abstract
:1. Introduction
2. Materials and Methods
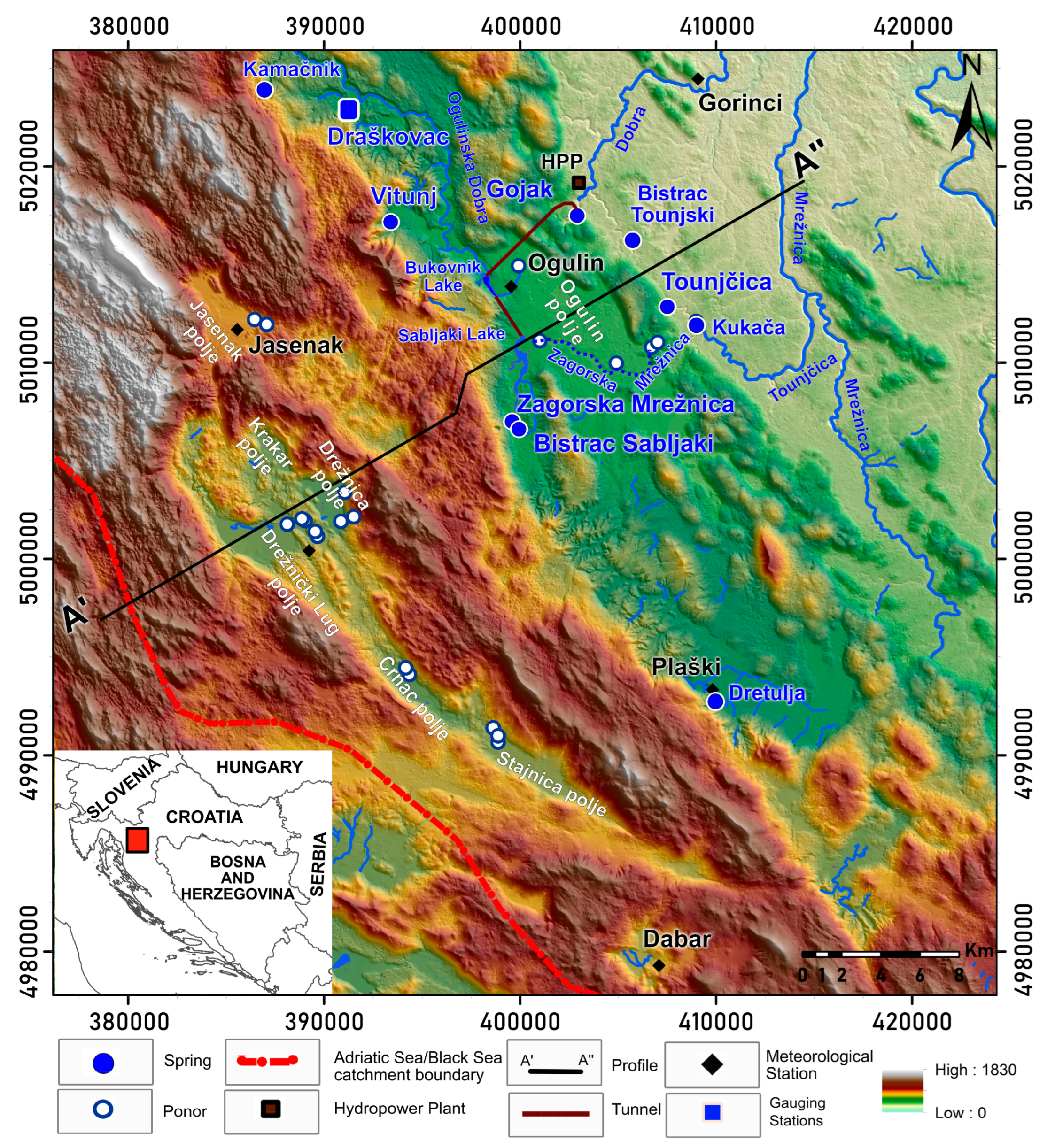
2.1. Setting
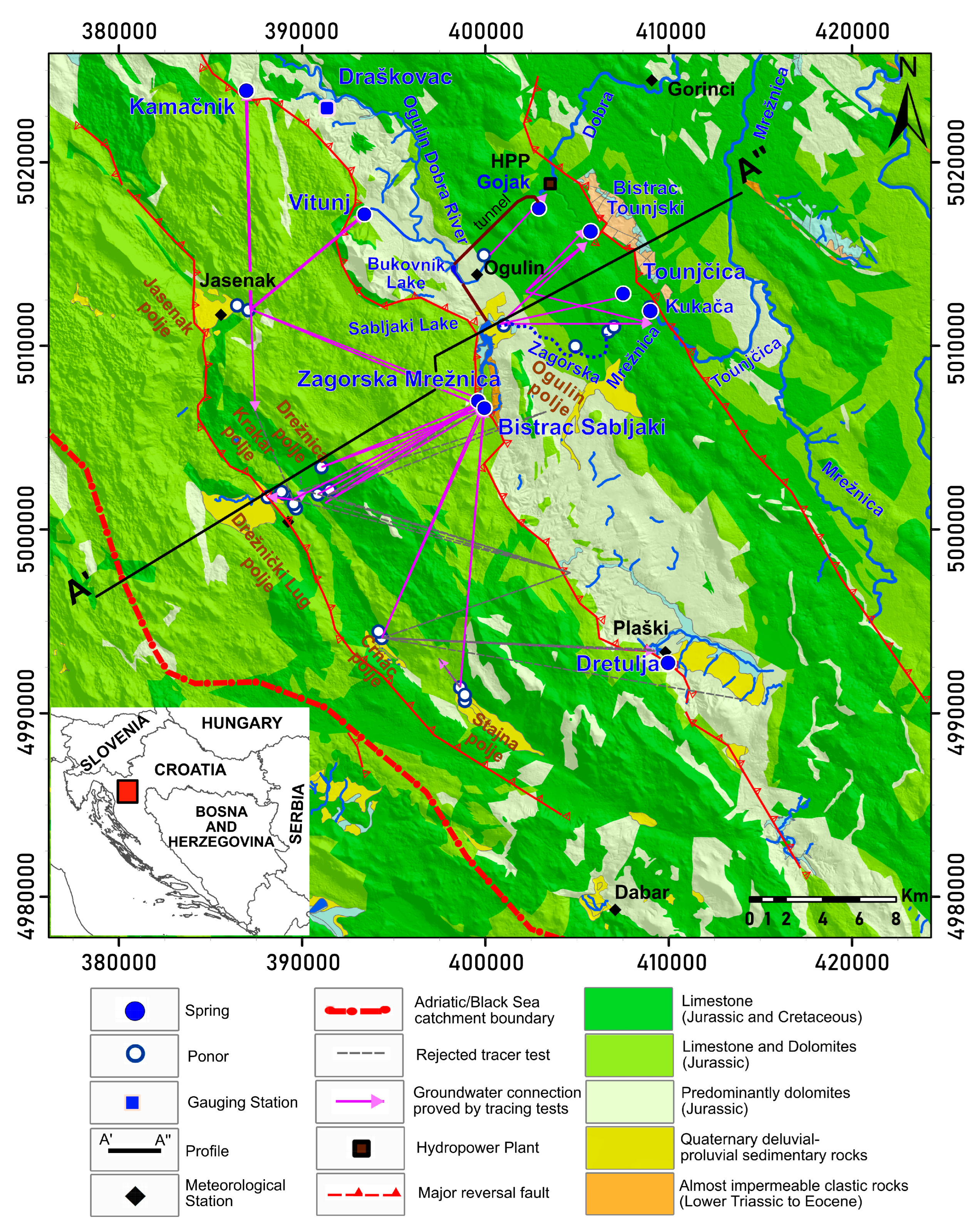
2.2. Hydrogeological Characteristics
2.3. Methods and Data
3. Results and Discussion
3.1. Hydrochemical of Groundwater
3.2. Hidrogeological Conceptual Model of Karst System
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ford, D.; Williams, P.D. Karst Hydrogeology and Geomorphology; John Wiley & Sons: Chichester, UK, 2007; p. 562. [Google Scholar]
- Goldscheider, N.; Drew, D. Methods in Karst Hydrogeology. In IAH International Contributions to Hydrogeology, 1st ed.; Taylor & Francis: London, UK, 2007; Volume 26, p. 264. [Google Scholar]
- Fiorillo, F. The Recession of Spring Hydrographs, Focused on Karst Aquifers. Water Resour. Manag. 2014, 28, 1781–1805. [Google Scholar] [CrossRef]
- Torresan, F.; Fabbri, P.; Piccinini, L.; Dalla Libera, N.; Pola, M.; Zampieri, D. Defining the hydrogeological behavior of karst springs through an integrated analysis: A case study in the Berici Mountains area (Vicenza, NE Italy). Hydrogeol. J. 2020, 28, 1229–1247. [Google Scholar] [CrossRef]
- Fiorillo, F.; Doglioni, A. The relation between karst spring discharge and rainfall by crosscorrelation analysis (Campania, Southern Italy). Hydrogeol. J. 2010, 18, 1881–1895. [Google Scholar] [CrossRef]
- Kovács, A.; Perrochet, P. A quantitative approach to spring hydrograph decomposition. J. Hydrol. 2008, 352, 16–29. [Google Scholar] [CrossRef]
- Panagopoulos, G.; Lambrakis, N. The contribution of time series analysis to the study of the hydrodynamic characteristics of the karst systems: Application on two typical karst aquifers of Greece (Trifilia, Almyros Crete). J. Hydrol. 2006, 329, 368–376. [Google Scholar] [CrossRef]
- Krešić, N. Quantitative Solutions in Hydrogeology and Ground-Water Modeling; Lewis Publishers: New York, NY, USA, 1997; 461p. [Google Scholar]
- Boussinesq, M.J. Essai sur la Theories des eax Courantes; Memoires Presentes par Divers Savants a l’Academie des Sciences de l’Institut National de France; Imprimerie Nationale: Paris, France, 1877; Volume XXIII. [Google Scholar]
- Boussinesq, M.J. Recherches the´oriques sur l’e´coulement des nappes d’eau infiltre´es dans le sol et sur le debit des sources. J. Mathématiques Pures Appliquées 1904, 10, 5–78. [Google Scholar]
- Maillet, E. Mécanique et Physique du Globe: Essai D’hydraulique Souterraine et Fluviale [Mechanics and Physiques of the World: An Essay of Subterranean and Fluviatile Hydraulics]; Hermann: Paris, France, 1905. [Google Scholar]
- Schoeller, H. Hydrodynamique dans le Karst. Hydrogeologie des roches fissure´es. In Actes du Colloque de Dubrovnik; IAHS-UNESCO: Paris, France, 1965. [Google Scholar]
- Atkinson, T.C. Diffuse Flow and Conduit Flow in Limestone Terrain in the Mendip Hills, Somerset, UK. J. Hydrol. 1977, 35, 93–110. [Google Scholar] [CrossRef]
- Malik, P. Assessment of regional karstification degree and groundwater sensitivity to pollution using hydrograph analysis in the Velka Fatra Mountains. Slovakia. Environ. Geol. 2007, 51, 707–711. [Google Scholar] [CrossRef]
- Krešić, N.; Bonacci, O. Spring discharge hydrograph. In Groundwater Hydrology of Springs: Engineering, Theory, Management, and Sustainability; Elsevier: Amsterdam, The Netherlands, 2010; Chapter 4; pp. 129–163. [Google Scholar] [CrossRef]
- Posavec, K.; Bačani, A.; Nakić, Z. A visual basic spreadsheet macro for recession curve analysis. Groundwater 2006, 44, 764–767. [Google Scholar] [CrossRef]
- Posavec, K.; Parlov, J.; Nakić, Z. Fully Automated Objective-Based Method for Master Recession Curve Separation. Groundwater 2010, 48, 598–603. [Google Scholar] [CrossRef]
- Posavec, K.; Giacopetti, M.; Materazzi, M.; Birk, S. Method and Excel VBA Algorithm for Modeling Master Recession Curve Using Trigonometry Approach. Groundwater 2017, 55, 891–898. [Google Scholar] [CrossRef] [PubMed]
- Kovács, A. Quantitative classification of carbonate aquifers based on hydrodynamic behaviour. Hydrogeol. J. 2017, 29, 33–52. [Google Scholar] [CrossRef]
- Bailly-Comte, V.; Ladouche, B.; Charlier, J.B.; Hakoun, V.; Maréchal, J.C. XLKarst, an Excel tool for time series analysis, spring recession curve analysis and classification of karst aquifers. Hydrogeol. J. 2023, 31, 240–2415. [Google Scholar] [CrossRef]
- Padilla, A.; Pulido-Bosch, A.; Mangin, A. Relative Importance of Baseflow and Quickflow from Hydrographs of Karst Spring. Groundwater 1994, 32, 267–277. [Google Scholar] [CrossRef]
- Krešić, N.; Stevanović, Z. Groundwater Hydrology of Springs: Engineering, Theory, Management, and Sustainability; Butterworth-Heinemann: Oxford, UK, 2010; 573p. [Google Scholar]
- Malík, P.; Vojtková, S. Use of recession-curve analysis for estimation of karstification degree and its application in assessing overflow/underflow conditions in closely spaced karstic springs. Environ. Earth Sci. 2012, 65, 2245–2257. [Google Scholar] [CrossRef]
- Kovács, A.; Perrochet, P.; Király, L.; Jeannin, P.Y. A quantitative method for the characterization of karst aquifers based on spring hydrograph analysis. J. Hydrol. 2005, 303, 152–164. [Google Scholar] [CrossRef]
- Kovačič, G. Hydrogeological study of the Malenščica karst spring (SW Slovenia) by means of a time series analysis. Acta Carsologica 2010, 39, 201–215. [Google Scholar] [CrossRef]
- Malík, P. Evaluating Discharge Regimes of Karst Aquifer. In Karst Aquifers—Characterization and Engineering. Professional Practice in Earth Sciences; Stevanović, Z., Ed.; Springer: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Verma, R.K.; Murthy, S.; Verma, S.; Kumar Mishra, S. Design flow duration curves for environmental flows estimation in Damodar River Basin, India. Appl. Water Sci. 2017, 7, 1283–1293. [Google Scholar] [CrossRef]
- Mangin, A. Pour une meilleure connaissance des systèmes hydrologiques a partir des analyses correlatoire et spectrale. J. Hydrol. 1984, 67, 25–43. [Google Scholar] [CrossRef]
- Chung, S.Y.; Senapathi, V.; Sekar, S.; Kim, T.H. Time series analyses of hydrological parameter variations and their correlations at a coastal area in Busan, South Korea. Hydrogeol. J. 2018, 26, 1875–1885. [Google Scholar] [CrossRef]
- Jemcov, I.; Petrič, M. Measured precipitation vs. effective infiltration and their influence on the assessment of karst systems based on results of the time series analysis. J. Hydrol. 2009, 379, 304–314. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, X.; Chen, X.; Shi, P. Quantifying time lag of epikarstspring hydrograph response to rainfall using correlation and spectral analyses. Hydrogeol. J. 2013, 21, 1619–1631. [Google Scholar] [CrossRef]
- Paiva, I.; Cunha, L. Characterization of the hydrodynamic functioning of the Degracias-Sicó Karst Aquifer, Portugal. Hydrogeol. J. 2020, 28, 2613–2629. [Google Scholar] [CrossRef]
- Stroj, A.; Briški, M.; Oštrić, M. Study of Groundwater Flow Properties in a Karst System by Coupled Analysis of Diverse Environmental Tracers and Discharge Dynamics. Water 2020, 12, 2442. [Google Scholar] [CrossRef]
- Pahernik, M. Prostorna gustoća ponikava na području Republike Hrvatske. Hrvat. Geogr. Glas. 2012, 74, 5–26. [Google Scholar] [CrossRef]
- Selak, A.; Boljat, I.; Reberski, J.L.; Terzić, J.; Čenčur, B.C. Impact of land use on Karst water resources—A case study of the Kupa (Kolpa) transboundary river catchment. Water 2020, 12, 3226. [Google Scholar] [CrossRef]
- Bahun, S. Geološka osnova hidrogeoloških odnosa krškog područja između Slunja i Vrbovskog (Geological basis of the hydrogeological relations in the karst area between Slunj and Vrbovsko). Geološki Vjesn. 1968, 21, 19–82. [Google Scholar]
- Lukač Reberski, J.; Kapelj, S.; Terzić, J. An estimation of groundwater type and origin of the complex karst catchment using hydrological and hydrogeochemical parameters: A case study of the Gacka river springs. Geol. Croat. 2009, 62, 157–178. [Google Scholar] [CrossRef]
- Lukač Reberski, J.; Marković, T.; Nakić, Z. Definition of the river Gacka springs subcatchment areas on the basis of hydrogeological parameters. Geol. Croat. 2013, 66, 39–53. [Google Scholar] [CrossRef]
- Biondić, B.; Biondić, R.; Kapelj, S. Karst groundwater protection in the Kupa River catchment area and sustainable development. Environ. Geol. 2006, 49, 828–839. [Google Scholar] [CrossRef]
- Pavlić, K.; Parlov, J. Crosscorrelation and Cross-Spectral Analysis of the Hydrographs in the Northern Part of the Dinaric Karst of Croatia. Geosciences 2019, 9, 86. [Google Scholar] [CrossRef]
- Terzić, J.; Stroj, A.; Frangen, T. Hydrogeological investigation of karst system properties by common use of diverse methods: A case study of Lička Jesenica springs in Dinaric karst of Croatia. Hydrol. Process 2012, 26, 3302–3311. [Google Scholar] [CrossRef]
- Buljan, R.; Pavlić, K.; Terzić, J.; Perković, D. A Conceptual Model of Groundwater Dynamics in the Catchment Area of the Zagorska Mrežnica Spring, the Karst Massif of Kapela Mountain. Water 2019, 11, 1983. [Google Scholar] [CrossRef]
- Bonacci, O.; Andrić, I. Impact of an inter-basin water transfer and reservoir operation on a karst open streamflow hydrological regime: An example from the Dinaric karst (Croatia). Hydrol. Process 2010, 24, 3852–3863. [Google Scholar] [CrossRef]
- HGI-CGS Arhive; Croatian Geological Survey: Zagreb, Croatia, 1948.
- Gajić-Čapka, M.; Perćec Tadić, M.; Patarcic, M. Digitalna godisnja oborinska karta Hrvatske. (A Digital Annual Precipitation Map of Croatia-m Croatian). Hrvat. Meteorološki Časopis (Croat. Meteorol. J.) 2003, 38, 21–33. [Google Scholar]
- Zaninović, K.; Srnec, L.; Perčec-Tadić, M. Digitalna godišnja temperaturna karta Hrvatske [A Digital Annual Temperature Map of Croatia—In Croatian]. Hrvat. Meteorološki Časopis (Croat. Meteorol. J.) 2004, 39, 51–58. [Google Scholar]
- Korbar, T. Orogenic evolution of the External Dinarides in the NE Adriatic region: A model constrained by tectonostratigraphy of Upper Cretaceous to Paleogene carbonates. Earth-Sci. Rev. 2009, 96, 296–312. [Google Scholar] [CrossRef]
- Tari, V. Evolution of the northern and western Dinarides: A tectonostratigraphic approach. Eur. Geosci. Union Stephan. Mueller Spec. Publ. 2002, 1, 223–236. [Google Scholar] [CrossRef]
- Schmid, S.M.; Bernoulli, D.; Fügenschuh, B.; Matenco, L.; Schefer, S.; Schuster, R.; Tischler, M.; Ustaszewski, K. The Alpine-Carpathian-Dinaridic orogenic system: Correlation and evolution of tectonic units. Swiss J. Geosci. 2008, 101, 139–183. [Google Scholar] [CrossRef]
- Vlahović, I.; Tišljar, J.; Velić, I.; Matičec, D. Evolution of the Adriatic Carbonate Platform: Palaeogeography, main events and depositional dynamics. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2005, 220, 333–360. [Google Scholar] [CrossRef]
- Prelogović, E.; Aljinović, B.; Bahun, S. New data on structural relationships in the Northern Dalmatian Dinaride Area. Geol. Croat. 1995, 48, 167–176. [Google Scholar]
- Velić, I.; Sokač, B. Basic Geological Map of the Republic of Croatia 1:100.000; Sheet Ogulin; Croatian Geological Survey: Zagreb, Croatia, 1982. [Google Scholar]
- Šušnjar, M.; Bukovac, J.; Nikler, L.; Crnolatac, I.; Milan, A.; Šikic, D.; Griman, I.; Vulić, Ž.; Blašković, M. Basic Geological Map of the Republic of Croatia 1:100.000; Sheet Crikvenica; Croatian Geological Survey: Zagreb, Croatia, 1970. [Google Scholar]
- Bukovac, J.; Šušnjar, M.; Poljak, M.; Čakalo, M. Basic Geological Map of the Republic of Croatia 1:100.000; Sheet Črnomelj; Croatian Geological Survey: Zagreb, Croatia, 1983. [Google Scholar]
- Stroj, A.; Paar, D. Water and air dynamics within a deep vadose zone of a karst massif: Observations from the Lukina jama–Trojama cave system (−1,431 m) in Dinaric karst (Croatia). Hydrol. Process 2019, 33, 551–561. [Google Scholar] [CrossRef]
- Bočić, N.; Pahernik, M.; Mihevc, A. Geomorphological significance of the palaeodrainage network on a karst plateau: The Una–Korana plateau, Dinaric karst, Croatia. Geomorphology 2015, 247, 55–65. [Google Scholar] [CrossRef]
- Kovačević, A. Hydrogeological Features of Karlovac County. Master’s Thesis, University of Zagreb, Zagreb, Croatia, 2006. [Google Scholar]
- Karlović, I.; Pavlić, K.; Posavec, K.; Marković, T. Analysis of the hydraulic connection of the Plitvica stream and the groundwater of the Varaždin alluvial aquifer. Geofizika 2021, 38, 15–35. [Google Scholar] [CrossRef]
- Baedke, S.J.; Krothe, N.C. Derivation of effective hydraulic parameters of a Karst Aquifer from discharge hydrograph analysis. Water Resour. Res. 2001, 37, 13–19. [Google Scholar] [CrossRef]
- Milanović, P. Water regime in deep karst—Case study of the Ombla Spring drainage area. In Karst Hydrology and Water Resources, Proceedings of the U.S.-Yugoslavian Symposium, Dubrovnik, Croatia, 2–7 June 1975; Yevjevich, V., Ed.; Water Resources Publication: Littleton, CO, USA, 1981; pp. 165–191. [Google Scholar]
- Tallaksen, L.M. A review of baseflow recession analysis. J. Hydrol. 1995, 165, 349–370. [Google Scholar] [CrossRef]
- Urumović, K.; Duić, Ž.; Hlevnjak, B. Hydrogeological significance of recession coefficients at the example of Istrian springs. Rud.-Geološko-Naft. Zb. 2009, 21, 25–34. [Google Scholar]
- Trauth, M.H. MATLAB Recipes for Earth Sciences; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Liu, L.; Chen, X.; Xu, G.; Shu, L. Use of hydrologic time-series data for identification of hydrodynamic function and behavior in a karstic water system in China. Hydrogeol. J. 2011, 19, 1577–1585. [Google Scholar] [CrossRef]
- Taylor, C.J.; Green, E.A. Hydrogeologic characterization and methods used in the investigation of karst hydrology. In Field Techniques for Estimating Water Fluxes Between Surface Water and Groundwater; Techniques and Methods 4–D2; U.S. Geological Survey: Reston, VA, USA, 2008; pp. 75–114. [Google Scholar]
- Guo, Y.; Wang, F.; Qin, J.D.; Zhao, F.Z.; Gan, F.P.; Yan, B.K.; Bai, J.; Muhammed, H. Hydrodynamic characteristics of a typical karst spring system based on time series analysis in northern China. China Geol. 2021, 4, 433–445. [Google Scholar] [CrossRef]
- Kuhta, M.; Stroj, A.; Brkić, Ž. Hydrodynamic characteristics of Mt. Biokovo foothill springs in Croatia. Geol. Croat. 2012, 65, 41–52. [Google Scholar] [CrossRef]
- Boljat, I.; Terzić, J.; Duić, Ž.; Lukač Reberski, J.; Selak, A.; Briški, M. Tracing Hydrological Processes: Insights from Hydrochemical and Isotopic Investigations in the Northern Part of Croatian Dinaric Karst. Geol. Croat. 2024; in progress. [Google Scholar]
- Herak, M.; Bahun, S.; Magdalenić, A. Pozitivni i negativni utjecaji na razvoj krša u Hrvatskoj (Positive and negative influences on the development of the Karst in Croatia). Jugosl. Akad. Znan. I Umjet. Odjel Za Prir. Nauk. 1969, 6, 45–71. [Google Scholar]

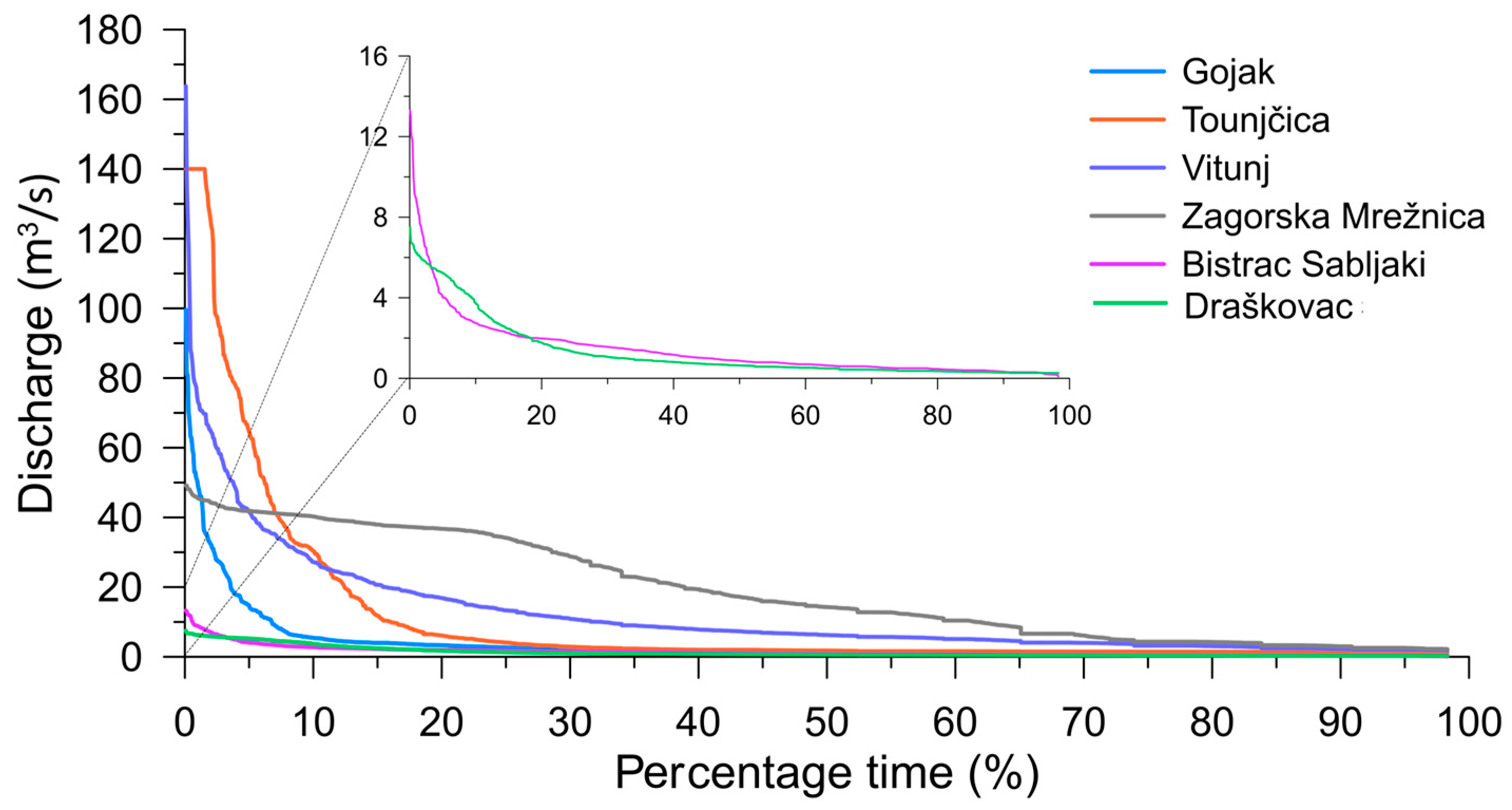


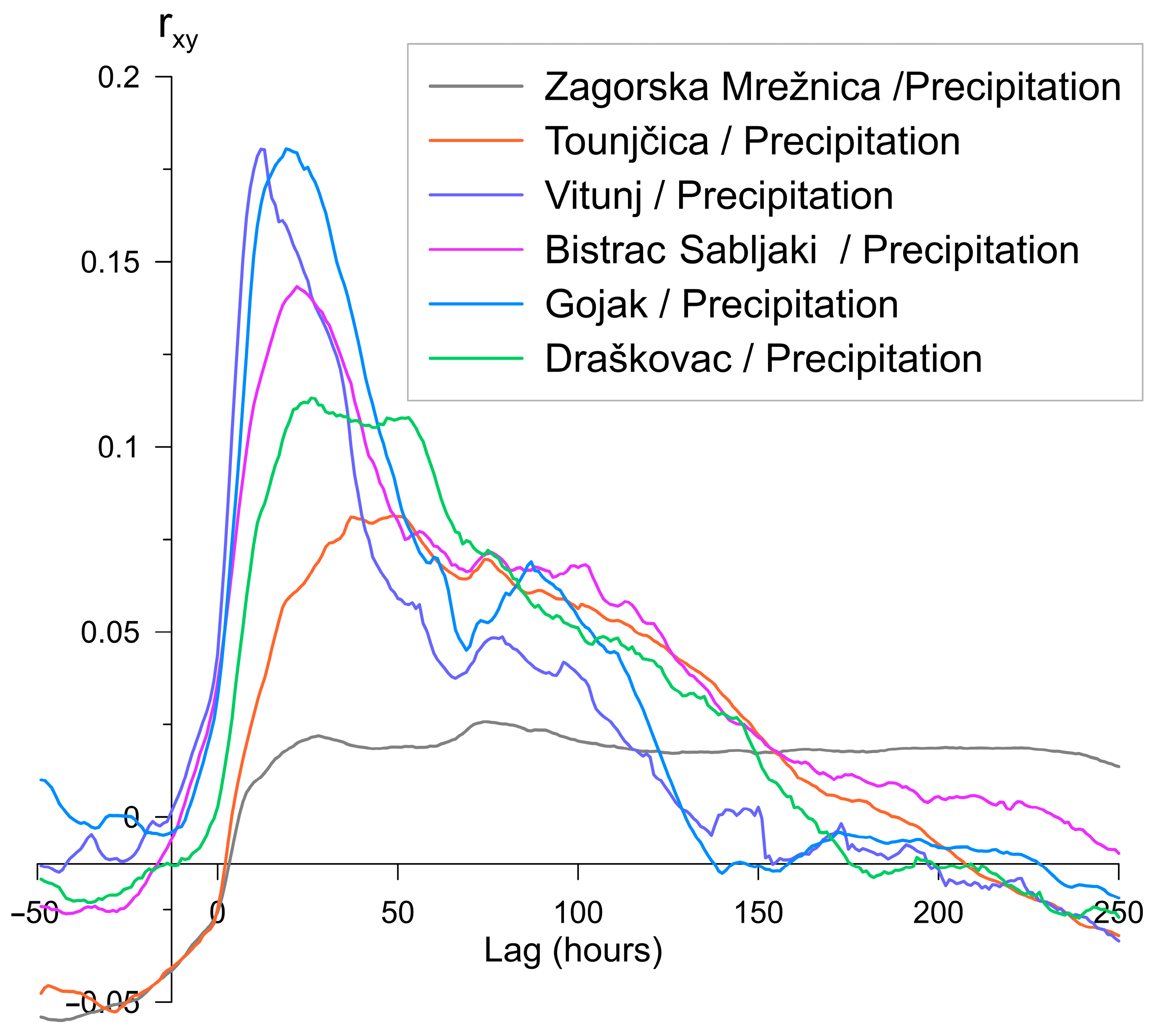
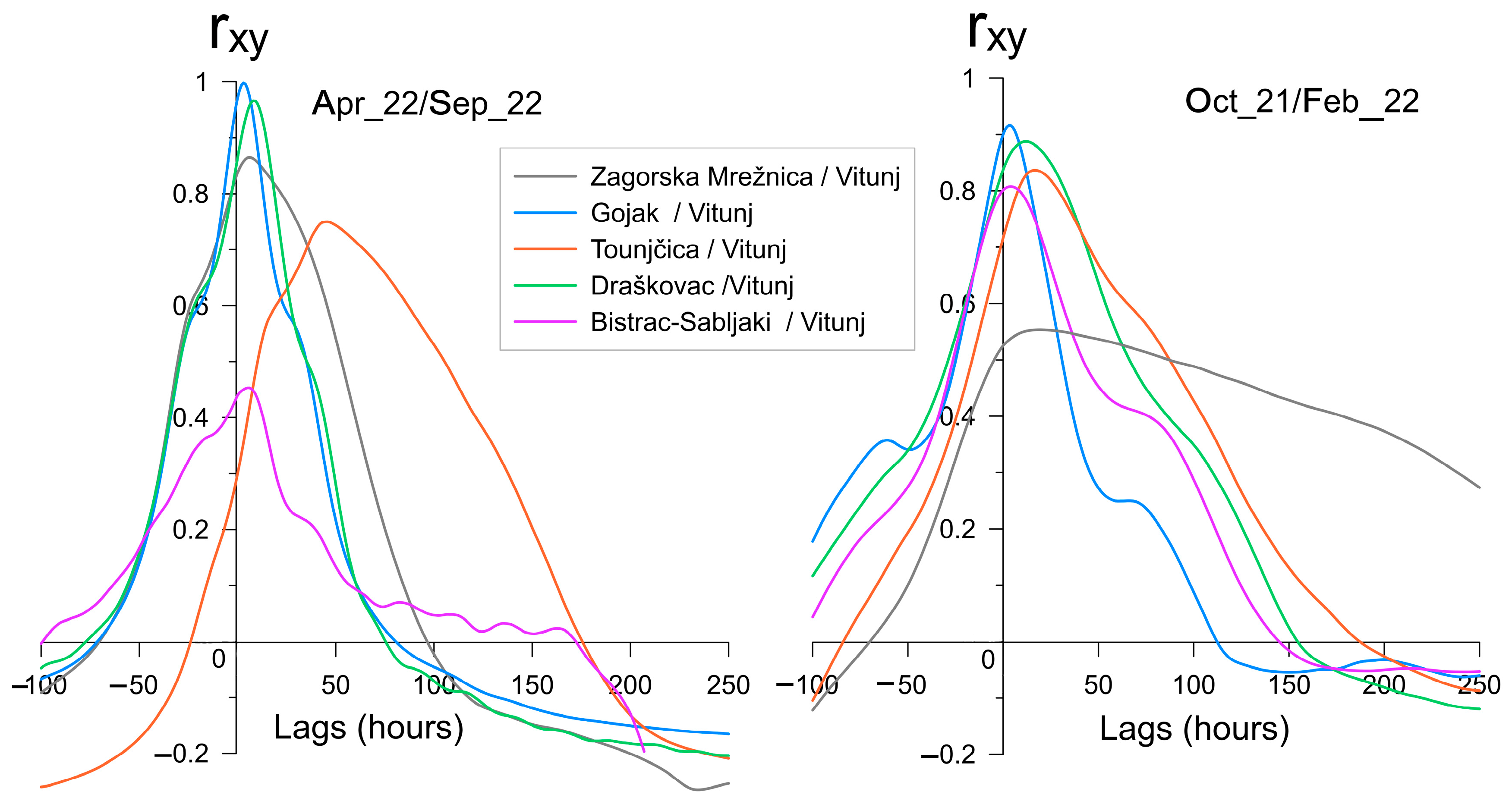
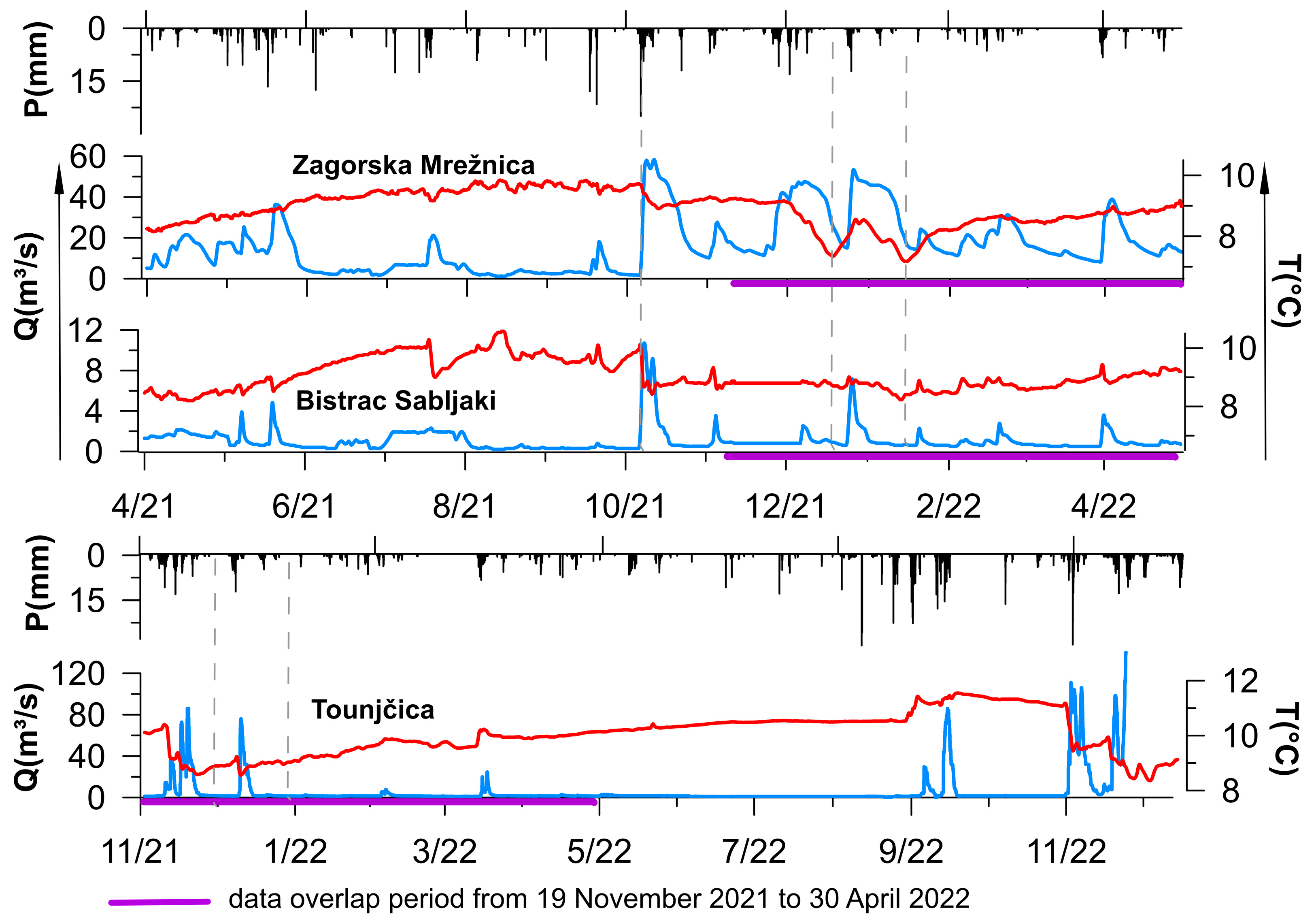
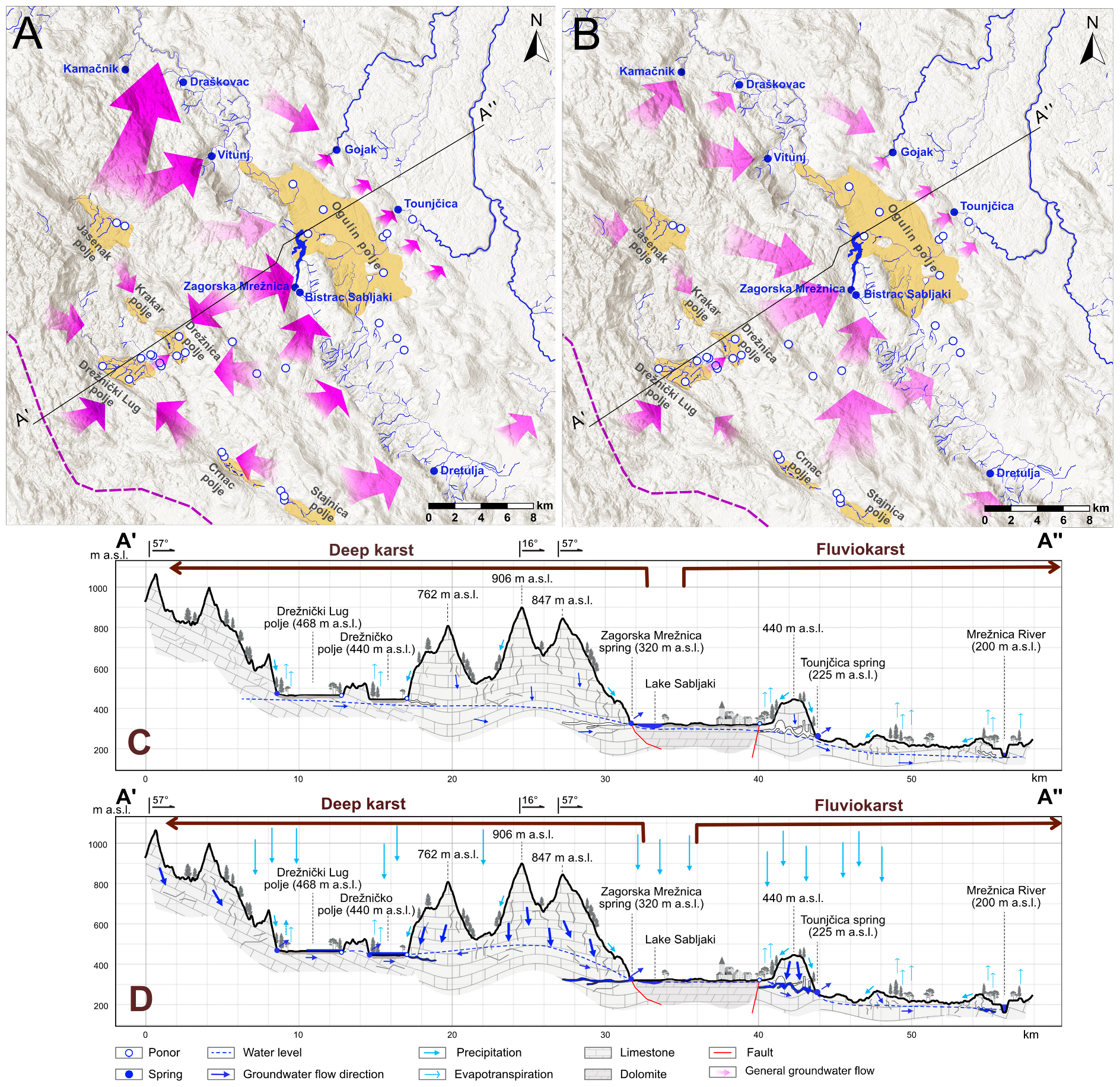
| Vitunj (m3/s) | Zagorska Mrežnica (m3/s) | Bistrac Sabljaki (m3/s) | Gojak (m3/s) | Draškovac (m3/s) | Tounjčica (m3/s) | |
|---|---|---|---|---|---|---|
| QMin | 0.30 | 0.30 | 0.10 | 0.30 | 0.10 | 0.20 |
| QMax | 163.78 | 49.15 | 13.30 | 99.58 | 7.50 | 140.00 |
| Qavg | 9.07 | 14.16 | 1.39 | 3.13 | 1.06 | 7.98 |
| Qmedian | 4.27 | 7.41 | 0.85 | 1.50 | 0.51 | 0.54 |
| Ϭ sd | 14.07 | 14.54 | 1.57 | 7.22 | 1.40 | 21.75 |
| QMin:Qmed:QMax | 1:14:546 | 1:25:164 | 1:9:133 | 1:5:332 | 1:5:75 | 1:3:700 |
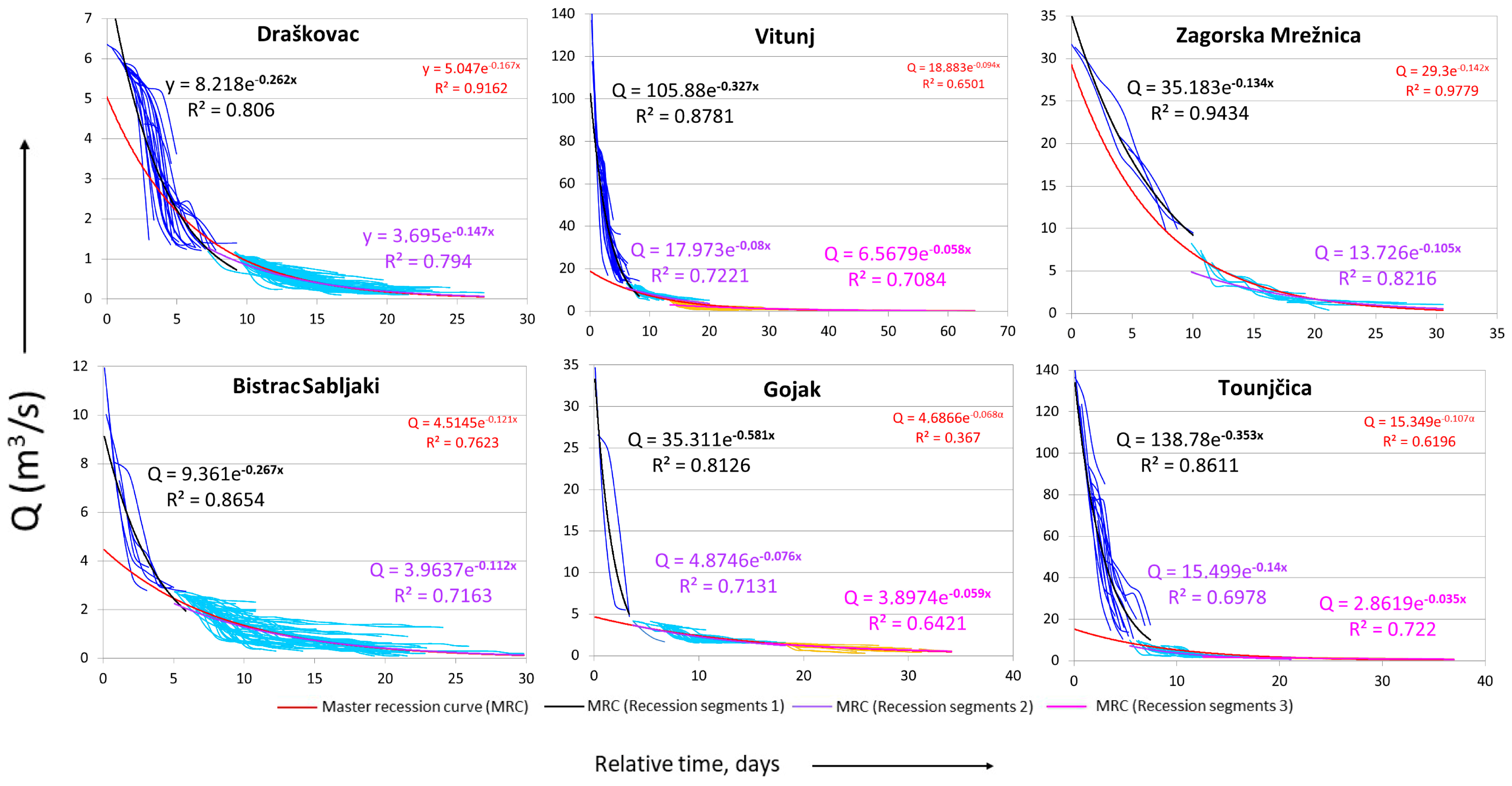
| Springs | Recession Segment 1 Curve | Recession Segment 2 Curve | Recession Segment 3 Curve | Master Recession Curve |
|---|---|---|---|---|
| Vitunj | ||||
| Zagorska Mrežnica | / | |||
| Bistrac Sabljaki | / | |||
| Draškovac | / | |||
| Tounjčica | ||||
| Gojak |
| Zagorska Mrežnica | Bistrac Sabljaki | Tounjčica | |
|---|---|---|---|
| MIN | 7.17 | 8.20 | 8.36 |
| MAX | 9.85 | 10.58 | 11.55 |
| Mean | 8.96 | 8.85 | 10.09 |
| Average | 8.90 | 9.06 | 10.03 |
| SD | 0.59 | 0.52 | 0.78 |
| CV | 6.60% | 5.92% | 7.72% |
| Sampled Spring | Date | Ca2+ (mg/L) | Mg2+ (mg/L) | Na+ (mg/L) | HCO3− (mg/L) | Cl− (mg/L) | NO3− (mg/L) | SO42− (mg/L) |
|---|---|---|---|---|---|---|---|---|
| Draškovac | 10 July 2019 * | 58.20 | 23.64 | 1.52 | 254 | 2.41 | 6.08 | 6.09 |
| 12 February 2020 ** | 58.55 | 22.76 | 0.67 | 272 | 1.87 | 5.66 | 5.42 | |
| 28 January 2021 *** | 52.49 | 16.26 | 0.98 | 256 | 1.80 | 3.50 | 1.99 | |
| Vitunj | 10 July 2019 * | 55.75 | 7.1 | 0.63 | 166 | 1.22 | 4.7 | 5.69 |
| 12 February 2020 ** | 55.01 | 5.77 | 0.73 | 199 | 1.44 | 5.24 | 5.18 | |
| 28 January 2021 *** | 51.37 | 20.93 | 0.44 | 221 | 1.04 | 1.35 | 2.23 | |
| Zagorska Mrežnica | 10 July 2019 * | 73.90 | 8.01 | 1.76 | 262 | 2.74 | 4.77 | 6.76 |
| 12 February 2020 ** | 71.02 | 9.41 | 1.45 | 254 | 2.04 | 4.53 | 6.47 | |
| 28 January 2021 *** | 65.80 | 5.88 | 2.12 | 257 | 3.17 | 2.19 | 1.98 | |
| Bistrac Sabljaki | 10 July 2019 * | 64.57 | 21.69 | 2.55 | 325 | 3.97 | 5.59 | 8.30 |
| 12 February 2020 ** | 67.62 | 19.13 | 3.14 | 290 | 4.02 | 4.73 | 7.09 | |
| 28 January 2021 *** | 63.00 | 14.39 | 2.81 | 268 | 4.74 | 2.79 | 2.74 | |
| Tounjčica | 10 July 2019 * | 65.61 | 7.86 | 1.41 | 181 | 2.21 | 7.45 | 6.65 |
| 12 February 2020 ** | 73.89 | 8.35 | 1.68 | 264 | 2.29 | 5.64 | 5.95 | |
| 28 January 2021 *** | 66.86 | 7.87 | 1.99 | 243 | 3.12 | 3.07 | 2.51 | |
| Gojak | 10 July 2019 * | 68.41 | 11.04 | 3.27 | 183 | 3.98 | 5.71 | 7.20 |
| 12 February 2020 ** | 59.93 | 14.12 | 4.54 | 239 | 5.64 | 6.45 | 6.74 | |
| 28 January 2021 *** | 60.66 | 10.27 | 5.23 | 233 | 7.74 | 4.87 | 3.54 |
| Location of Tracing Test | Injection Location | Spring of the Tracer Detection | Calculate Flow Rate (cm/s) | Emergence of Tracer (h) | Water Conditions |
|---|---|---|---|---|---|
| Jasenak polje | South ponor, 1972 | Kamačnik Vitunj | 2.4 1.6 | 130 144 | High water 100 L/s |
| South ponor, 1986 | Zagorska Mrežnica Bistrac | 0.86 0.85 | 448 456 | Low water 12.8 L/s | |
| Drežnica polje | Kolovoz, 1972 | Pećina Bistrac | 1.6 1.9 | 162 146 | High water 6 m3/s |
| Potočak, 1980 | Zagorska Mrežnica | 1.73 | 167 | Low water 17.3 L/s | |
| Pražića jaruga, 1985 | Zagorska Mrežnica Bistrac | 2.9 2.9 | 88 88 | High water 3–4 m3/s | |
| Zrnića, 1981 | Zagorska Mrežnica Pećina | 3.4 | 80 | End of the water wave | |
| Bosanić, 2013 | - | Flood | |||
| Drežnički Lug | Pećina Maravić Dragi, 1980 | Pećina Sušik | 0.55 | 43 | Low water |
| Stajnica polje | Jaruga-Jezerane, 2002 | Rokina Bezdani | 2.36 | 24 | Medium water |
| Jezerane ponor, 1988 | Zagorska Mrežnica Bistrac Sabljaki | 1.36 1.21 | 320 344 | 5 L/s | |
| Jaruga-Jezerane, 2000 | Zagorska Mrežnica Bistrac | 1.53 1.38 | 284 308 | 80–100 L/s | |
| Crnac polje | 1972 | Dretulja | 2.3 | 182 | High water |
| 1985 | Zagorska Mrežnica Bistrac | 2.3 2.16 | 160 173 | Low water 150–200 L/s | |
| Ogulin polje | Đula ponor, 1948 | Gojak | 5.68 | 23.45 | 2 m3/s |
| Zagorska Mrežnica watercours, 1948 | Bistrac Sabljaki Tounjčica Kukača | 10.2 5.5 11.7 | 18.45 32.55 18.25 | 9.37 m3/s | |
| Zagorska Mrežnica watercourse, 1948 | Bistrac Sabljaki Tounjčica Kukača | 11.76 6.97 6.17 | 15.98 28.45 37.25 | 13.20 m3/s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boljat, I.; Terzić, J.; Duić, Ž.; Lukač Reberski, J.; Selak, A. Conceptual Model Based on Groundwater Dynamics in the Northern Croatian Dinaric Region at the Transition from the Deep Karst and Fluviokarst. Water 2024, 16, 1630. https://doi.org/10.3390/w16111630
Boljat I, Terzić J, Duić Ž, Lukač Reberski J, Selak A. Conceptual Model Based on Groundwater Dynamics in the Northern Croatian Dinaric Region at the Transition from the Deep Karst and Fluviokarst. Water. 2024; 16(11):1630. https://doi.org/10.3390/w16111630
Chicago/Turabian StyleBoljat, Ivana, Josip Terzić, Željko Duić, Jasmina Lukač Reberski, and Ana Selak. 2024. "Conceptual Model Based on Groundwater Dynamics in the Northern Croatian Dinaric Region at the Transition from the Deep Karst and Fluviokarst" Water 16, no. 11: 1630. https://doi.org/10.3390/w16111630
APA StyleBoljat, I., Terzić, J., Duić, Ž., Lukač Reberski, J., & Selak, A. (2024). Conceptual Model Based on Groundwater Dynamics in the Northern Croatian Dinaric Region at the Transition from the Deep Karst and Fluviokarst. Water, 16(11), 1630. https://doi.org/10.3390/w16111630






