A Quasi-Steady Model for Estimating the Rate of Frost Heave When Subjected to Overburden Pressure
Abstract
:1. Introduction
2. Frost Heave Model
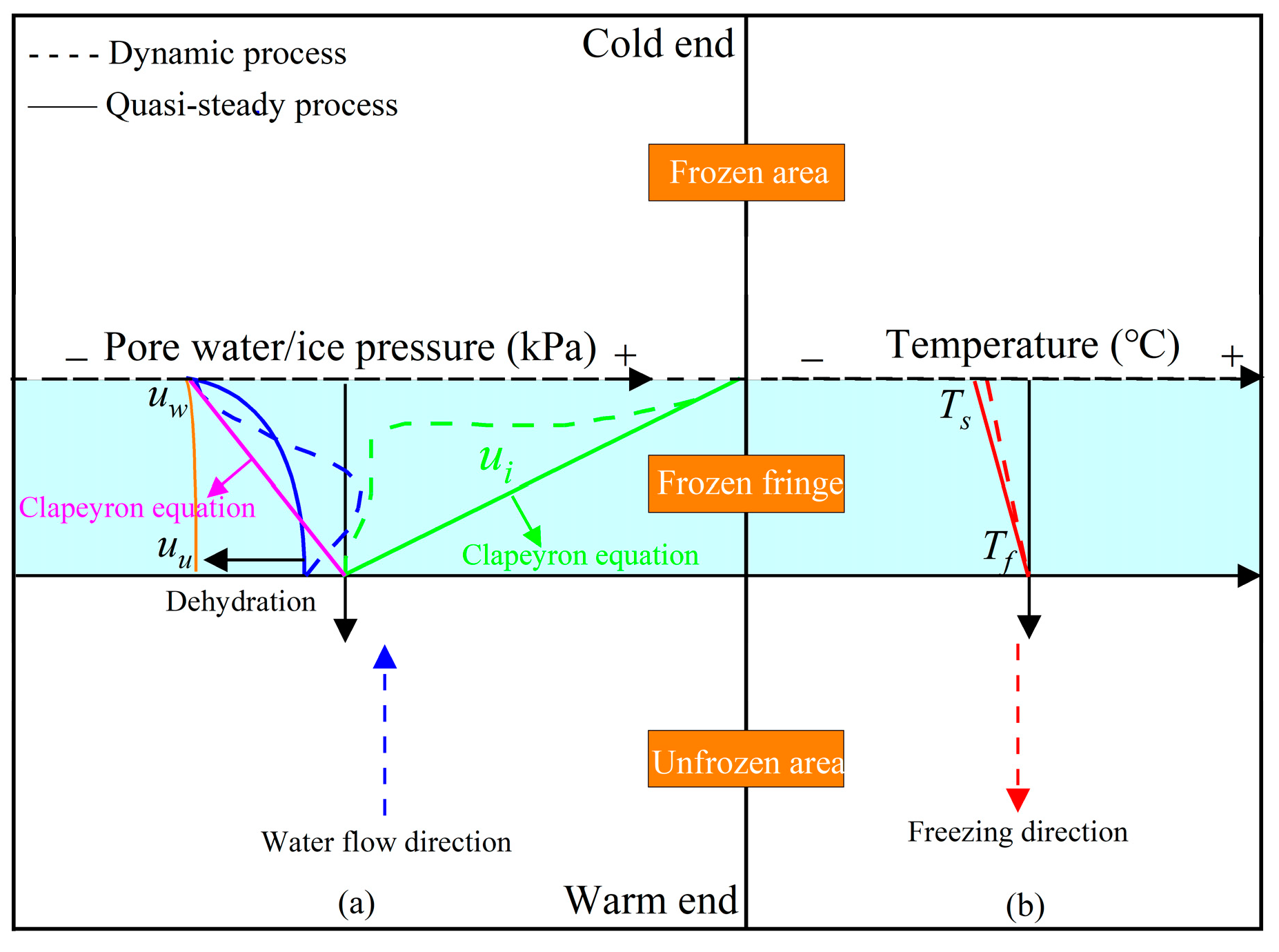
2.1. Physical Model
2.2. Derivation of the Mathematical Model
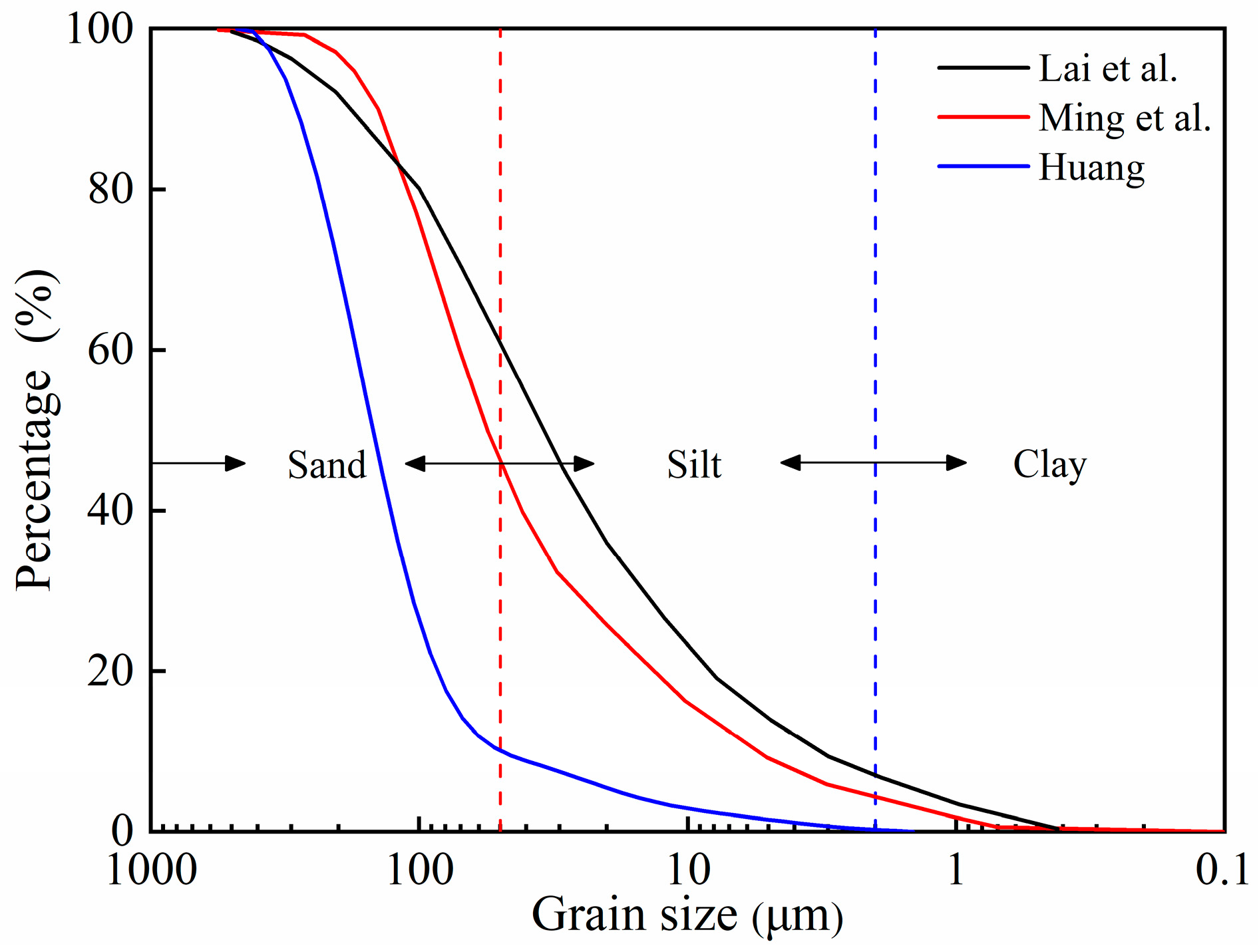
2.3. Soil Sample
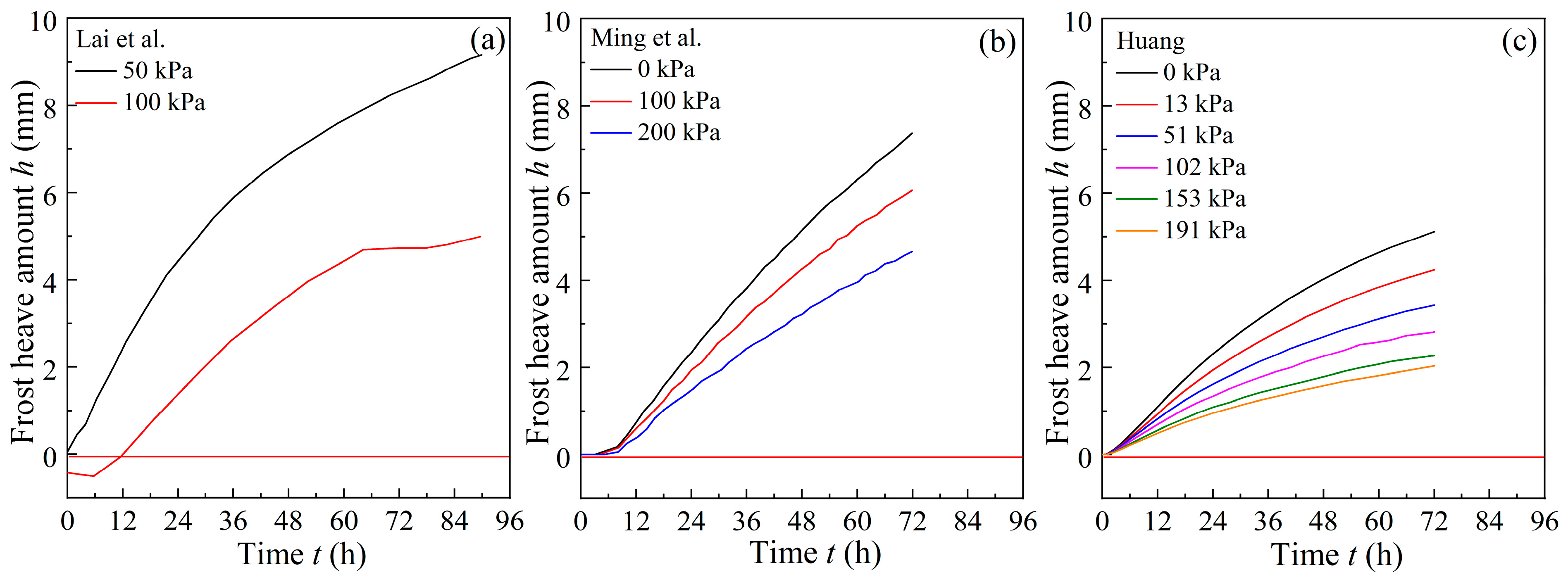
2.4. Frost Heave
3. Results and Discussion
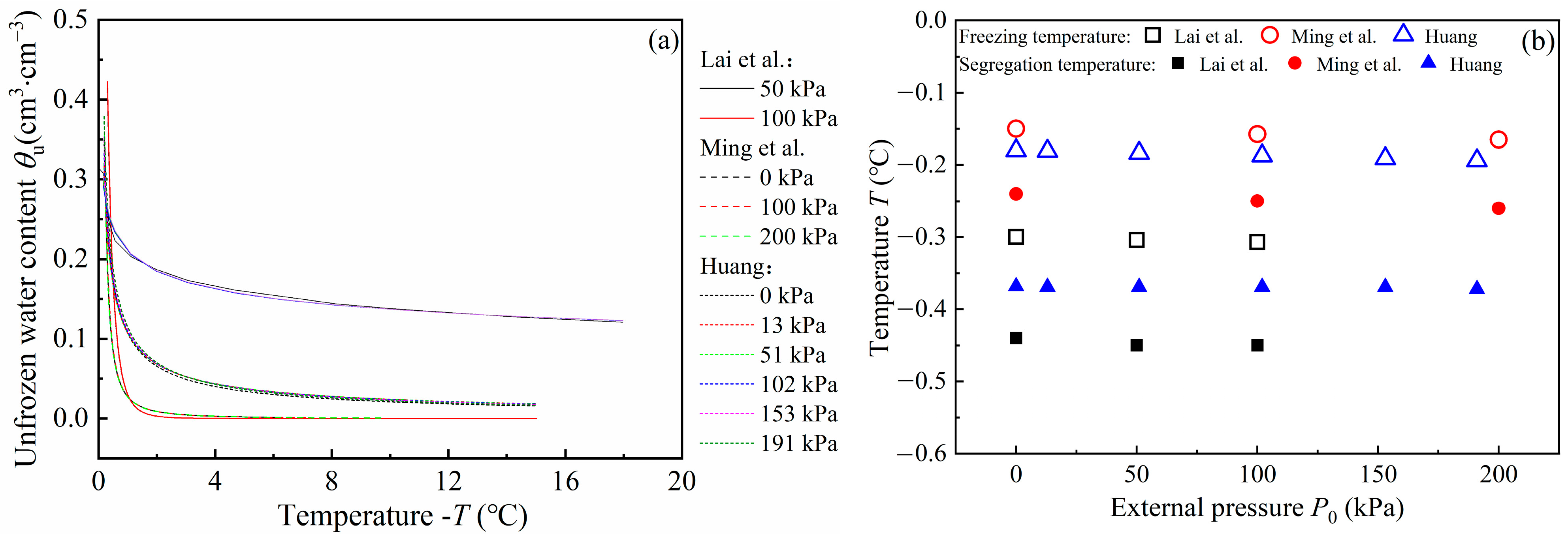
3.1. Parameters of the Model
3.1.1. Characteristic Curve of Soil Freezing and the Freezing Temperature under External Pressure
3.1.2. Pore Water Pressure and Hydraulic Conductivity at the Top of the Frozen Fringe
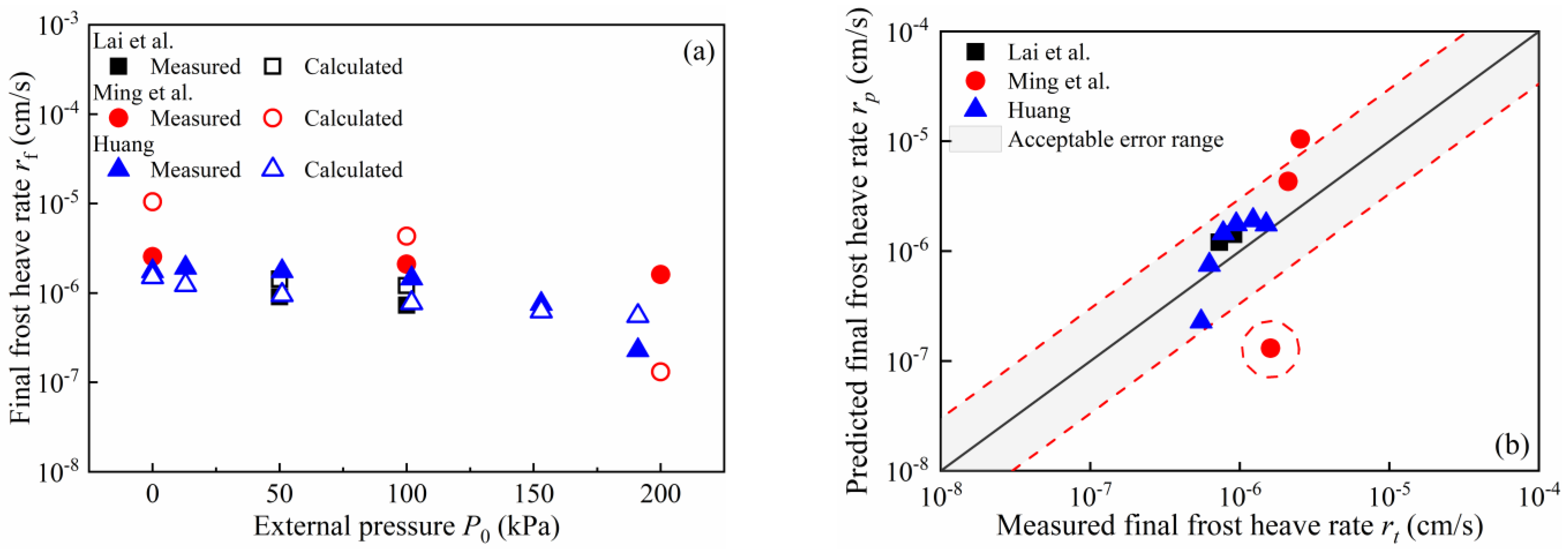
3.2. Model Verification
4. Conclusions
- (1)
- The proposed model suggested that the soil water potential gradient of the frozen fringe is the primary force propelling water movement. The difference in soil water potential is defined as uw-uu, where uw can be calculated using the Clapeyron equation, and uu can be determined with the soil water characteristic curve. Additionally, the model suggested that, when uw = uu, the water movement stops, leading to the end of frost heave.
- (2)
- To assess and examine the proposed model, three existing frost-heaving experiments were studied. The findings revealed that the predicted frost heave rates for the soil samples closely matched the experimental data overall, demonstrating the model’s validity.
- (3)
- The model is easy to apply as long as the SFCC, the soil particle size distribution, the overburden, the freezing point, the saturated hydraulic conductivity, and the saturation water content are provided.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Yang, Z.H.; Dutta, U.; Xiong, F.; Biswas, N.; Benz, H. Seasonal frost effects on the dynamic behavior of a twenty-story office building. Cold Reg. Sci. Technol. 2008, 51, 76–84. [Google Scholar] [CrossRef]
- Wang, Y.P.; Jin, H.J.; Li, G.Y. Investigation of the freeze–thaw states of foundation soils in permafrost areas along the China–Russia Crude Oil Pipeline (CRCOP) route using ground-penetrating radar (GPR). Cold Reg. Sci. Technol. 2016, 126, 10–21. [Google Scholar] [CrossRef]
- Kanie, S.; Akagawa, S.; Kim, K.; Mikami, T. Estimation method of frost heaving for chilled gas pipeline buried in frost susceptible soil. Cold Reg. Eng. 2006, 20, 1–12. [Google Scholar] [CrossRef]
- Kraatz, S.; Jacobs, J.M.; Miller, H.J. Spatial and temporal freeze-thaw variations in Alaskan roads. Cold Reg. Sci. Technol. 2019, 157, 149–162. [Google Scholar] [CrossRef]
- Wu, X.Y.; Niu, F.J.; Lin, Z.J.; Luo, J.; Zheng, H.; Shao, Z.J. Delamination frost heave in embankment of high speed railway in high altitude and seasonal frozen region. Cold Reg. Sci. Technol. 2018, 153, 25–32. [Google Scholar] [CrossRef]
- Qin, Z.P.; Lai, Y.M.; Tian, Y.; Yu, F. Frost-heaving mechanical model for concrete face slabs of earthen dams in cold regions. Cold Reg. Sci. Technol. 2019, 161, 91–98. [Google Scholar] [CrossRef]
- Taber, S. Frost heaving. J. Geol. 1929, 37, 428–461. [Google Scholar] [CrossRef]
- Taber, S. The mechanics of frost heaving. J. Geol. 1930, 38, 303–317. [Google Scholar] [CrossRef]
- Xu, X.Z.; Wang, J.C.; Zhang, L.X. Frozen Soil Physics; Science Press: Beijing, China, 2010. [Google Scholar]
- She, W.; Wei, L.S.; Zhao, G.T.; Yang, G.T. New insights into the frost heave behavior of coarse grained soils for high-speed railway roadbed: Clustering effect of fines. Cold Reg. Sci. Technol. 2019, 167, 102863. [Google Scholar] [CrossRef]
- Bronfenbrener, L.; Bronfenbrener, R. Modeling frost heave in freezing soils. Cold Reg. Sci. Technol. 2010, 61, 43–64. [Google Scholar] [CrossRef]
- Everett, D.H. The thermodynamics of frost damage to porous solids. Trans. Faraday Soc. 1961, 57, 1541–1551. [Google Scholar] [CrossRef]
- Gilpin, R.R. A model for the prediction of ice lensing and frost heave in soils. Water Resour. Res. 1980, 16, 918–930. [Google Scholar] [CrossRef]
- Nixon, J.F. Discrete ice lens theory for frost heave in soils. Can. Geotech. J. 1991, 28, 843–859. [Google Scholar] [CrossRef]
- O’Neill, K.; Miller, R.D. Exploration of a rigid ice model of frost heave. Water Resour. Res. 1985, 21, 281–296. [Google Scholar] [CrossRef]
- Nixon, J.F. Discrete ice lens theory for frost heave beneath pipelines. Can. Geotech. J. 1992, 29, 487–497. [Google Scholar] [CrossRef]
- Zhou, J.Z.; Li, D.Q. Numerical analysis of coupled water, heat and stress in saturated freezing soil. Cold Reg. Sci. Technol. 2012, 72, 43–49. [Google Scholar] [CrossRef]
- Ming, F.; Zhang, Y.; Li, D.Q. Experimental and theoretical investigations into the formation of ice lenses in deformable porous media. Geosci. J. 2016, 20, 667–679. [Google Scholar] [CrossRef]
- Konrad, J.M.; Morgenstern, N.R. The segregation potential of a freezing soil. Can. Geotech. J. 1981, 18, 482–491. [Google Scholar] [CrossRef]
- Konrad, J.M.; Shen, M. 2-D frost heave action model using segregation potential of soils. Cold Reg. Sci. Technol. 1996, 24, 263–278. [Google Scholar] [CrossRef]
- Konrad, J.M. Estimation of the segregation potential of fine-grained soils using the frost heave response of two reference soils. Can. Geotech. J. 2005, 42, 38–50. [Google Scholar] [CrossRef]
- Nixon, J.F. Field frost heave predictions using the segregation potential concept. Can. Geotech. J. 1982, 19, 526–529. [Google Scholar] [CrossRef]
- Ma, W.; Zhang, L.; Yang, C. Discussion of the applicability of the generalized Clausius–Clapeyron equation and the frozen fringe process. Earth-Sci. Rev. 2015, 142, 47–59. [Google Scholar] [CrossRef]
- Kurylyk, B.L.; Watanabe, K. The mathematical representation of freezing and thawing processes in variablysaturated, non-deformable soils. Adv. Water Resour. 2013, 60, 160–177. [Google Scholar] [CrossRef]
- Lai, Y.M.; Pei, W.S.; Zhang, M.Y.; Zhou, J.Z. Study on theory model of hydro-thermal–mechanical interaction process in saturated freezing silty soil. Int. J. Heat Mass Transf. 2014, 78, 805–819. [Google Scholar] [CrossRef]
- Edlefsen, N.E.; Anderson, A.B.C. Thermodynamics of soil moisture. Hilgardia 1943, 12, 117–123. [Google Scholar] [CrossRef]
- Ming, F.; Li, D.Q. A model of migration potential for moisture migration during soil freezing. Cold Reg. Sci. Technol. 2016, 124, 87–94. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Sheng, Y.; Huang, L.; Huang, X.B.; He, B.B. Application of the segregation potential model to freezing soil in a closed system. Water 2020, 12, 2418. [Google Scholar] [CrossRef]
- Huang, L. Study on Mechanical Characteristics in the Process of Interaction between Pipe and Frozen Soil under Frost Heaving Conditions; University of Chinese Academy of Sciences: Lanzhou, China, 2019. [Google Scholar]
- Ming, F.; Chen, L.; Li, D.Q.; Wei, X.B. Estimation of hydraulic conductivity of saturated frozen soil from the soil freezing characteristic curve. Sci. Total Environ. 2020, 698, 134132. [Google Scholar] [CrossRef]
- Ji, Y.; Zhou, G.; Zhao, X.; Wang, J.; Wang, T.; Lai, Z.; Mo, P. On the frost heaving-induced pressure response and its dropping power-law behaviors of freezing soils under various restraints. Cold Reg. Sci. Technol. 2017, 142, 25–33. [Google Scholar] [CrossRef]
- Sheng, D.; Zhang, S.; Niu, F.; Cheng, G. A potential new frost heave mechanism in high-speed railway embankments. Géotechnique 2014, 64, 144–154. [Google Scholar] [CrossRef]
- Lei, Z.D.; Yang, S.X.; Xie, S.C. Soil Hydrodynamics; Tsinghua University Press: Beijing, China, 1988. [Google Scholar]
- Hoekstra, P. Moisture movement in soils under temperature gradients with the cold-side temperature below freezing. Water Resour. Res. 1966, 2, 241–250. [Google Scholar] [CrossRef]
- Mageau, D.W.; Morgenstern, N.R. Observations on moisture migration in frozen soils. Can. Geotech. J. 1980, 17, 54–60. [Google Scholar] [CrossRef]
- Thomas, H.R.; Cleall, P.; Li, Y.C.; Harris, C.; Kern-Luetschg, M. Modelling of cryogenic processes in permafrost and seasonally frozen soils. Géotechnique 2009, 59, 173–184. [Google Scholar] [CrossRef]
- Black, P.B. Application of the Clapeyron Equation to Water and Ice in Porous Media; American Society for Testing and Materials: Philadelphia, PA, USA, 1995. [Google Scholar]
- Henry, K.S. A Review of the Thermodynamics of Frost Heave; Defense Technical Information Center: Fort Belvoir, VA, USA, 2000. [Google Scholar]
- Campbell, G.S. Soil Physics with Basic: Transport Models for Soil-Plant Systems; Elsevier: Amsterdam, The Netherlands, 1985; Volume 14. [Google Scholar]
- Zhou, J.Z.; Wei, C.F.; Lai, Y.M.; Wei, H.Z.; Tian, H.H. Application of the generalized clapeyron equation to freezing point depression and unfrozen water content. Water Resour. Res. 2018, 54, 9412–9431. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, X.Y. A model for predicting the hydraulic conductivity of warm saturated frozen soil. Build. Sci. 2020, 179, 106939. [Google Scholar] [CrossRef]


| Soil Type | W (%) | ρd (g/cm3) | k0 (m/s) | L (cm) | D (cm) |
|---|---|---|---|---|---|
| S1 [25] | 20.59 | 1.96 | 2.15 × 10−8 | 10 | 10 |
| S2 [18] | 16.17 | 1.84 | 2.10 × 10−8 | 10 | 10 |
| S3 [29] | 35.10 | 1.49 | 1.20 × 10−10 | 11 | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Zhang, X. A Quasi-Steady Model for Estimating the Rate of Frost Heave When Subjected to Overburden Pressure. Water 2024, 16, 2542. https://doi.org/10.3390/w16172542
Chen L, Zhang X. A Quasi-Steady Model for Estimating the Rate of Frost Heave When Subjected to Overburden Pressure. Water. 2024; 16(17):2542. https://doi.org/10.3390/w16172542
Chicago/Turabian StyleChen, Lei, and Xiyan Zhang. 2024. "A Quasi-Steady Model for Estimating the Rate of Frost Heave When Subjected to Overburden Pressure" Water 16, no. 17: 2542. https://doi.org/10.3390/w16172542
APA StyleChen, L., & Zhang, X. (2024). A Quasi-Steady Model for Estimating the Rate of Frost Heave When Subjected to Overburden Pressure. Water, 16(17), 2542. https://doi.org/10.3390/w16172542