1. Introduction
Fairy circles are remarkable bare circular gaps in a background of uniform grass. They are common within the pro-Namib Desert in areas with 50–100 mm of annual precipitation and in the Pilbara region in Western Australia, where the average precipitation is 330 mm but with ten times more evaporation (
Figure 1; [
1,
2,
3] and references therein). Their formation is still under debate both in Australia and Namibia. In the Australian fairy circles, the water transport is by the overland flow of surface water from the center of the gap to the periphery due to the low infiltrability of the physical soil crust at the bare soil. In contrast, in the sandy environment of Namibia, the water transport is below the surface due to lateral soil diffusion from the center to the edges [
4,
5,
6]. Thus, both in Namibia and Australia, the bare soil patches in the fairy circles are a source of extra water available for the vegetation in the matrix. These vegetation patterns can survive drier conditions than the uniform vegetation and they are very important for the ecological system in these harsh environments [
4]. A recent work, based on remote sensing analysis [
7], claimed that FC-like patterns are globally distributed, and discovered 263 new sites of vegetation gaps, but more detailed field work is needed to understand the formative mechanisms (physical and ecological) at these new sites and to distinguish between FC-like patterns (vegetation gaps) to proper FCs [
8].
The aim of the current work is to study the physical characteristics of fairy circles in one of the driest places in the Namib Desert and the role of aeolian interaction in their formation and persistence. Unlike the fairy circles in the other areas of the Namib Desert that have a unique hexagonal spatial order [
9], the fairy circles near Sesriem are more random, lacking the typical spatial order observed at the famous, more densely covered FC sites (
Figure 1).
In this extreme climate, the grass in the matrix, which is the area between the circles, is very sparse and the fairy circles resemble vegetation rings (
Figure 2). Most of the fairy circles in the study site were decorated by small megaripples and their effect on the ecohydrology of fairy circles is still unknown. Normal aeolian (impact) ripples develop due to an instability of an initially flat bed of cohesionless sand that is mobilized into a hopping motion called saltation. These very common ripples emerge on relatively fine desert sands with typically unimodal and well-sorted grain size distributions and form rather parallel wave trains. They can be characterized as effectively two-dimensional bedforms, displaying only small crest modulations transverse to the wind direction. In contrast, so-called megaripples are larger bedforms that develop from poorly sorted sands and their crests exhibit greater sinuosity due to transverse instability that increases with the ripple’s age and is further controlled by wind intensity and direction [
10].
Two main theories have been proposed to explain fairy circle development. According to the termite hypothesis, the termites eat the grass’ roots in the Namibian fairy circles (NFCs), whereas the hard pavement created by the termites prevent grass growth in the Australian fairy circles (AFCs; [
11,
12,
13,
14]). The self-organizing theory views the fairy circles as one of the various vegetation patterns that form in water-limited systems due to competition between plants for water [
15,
16]. According to this hypothesis, patterns form due to the instability of the uniform vegetation state to non-uniform perturbations (known as Turing instability or finite wavelength instability) that include short positive feedbacks and long-range negative feedbacks. This generic mechanism, which is also known as scale-dependent feedback [
17], can explain the formation of spots, stripes (labyrinths on flat terrains), and gaps depending on the amount of rainfall.
According to a new model, these FC ‘ring-like’ patterns are the last viable patterns before collapse to the bare soil state occurs [
18]. This new model takes into account phenotypic changes in the root structure of plants coupled with pattern-forming feedback between the two soil layers. It accounts for the lack of the predicted stripe and spot patterns in Namibia along the rainfall gradient by taking into account the phenotypic plastically feedback, namely that plants can grow longer roots to reach high water content in deeper layers [
18]. According to this new model, the final pattern before tipping to the bare soil is a bare matrix with peripheral rings similar to the pattern observed in the study site (
Figure 2).
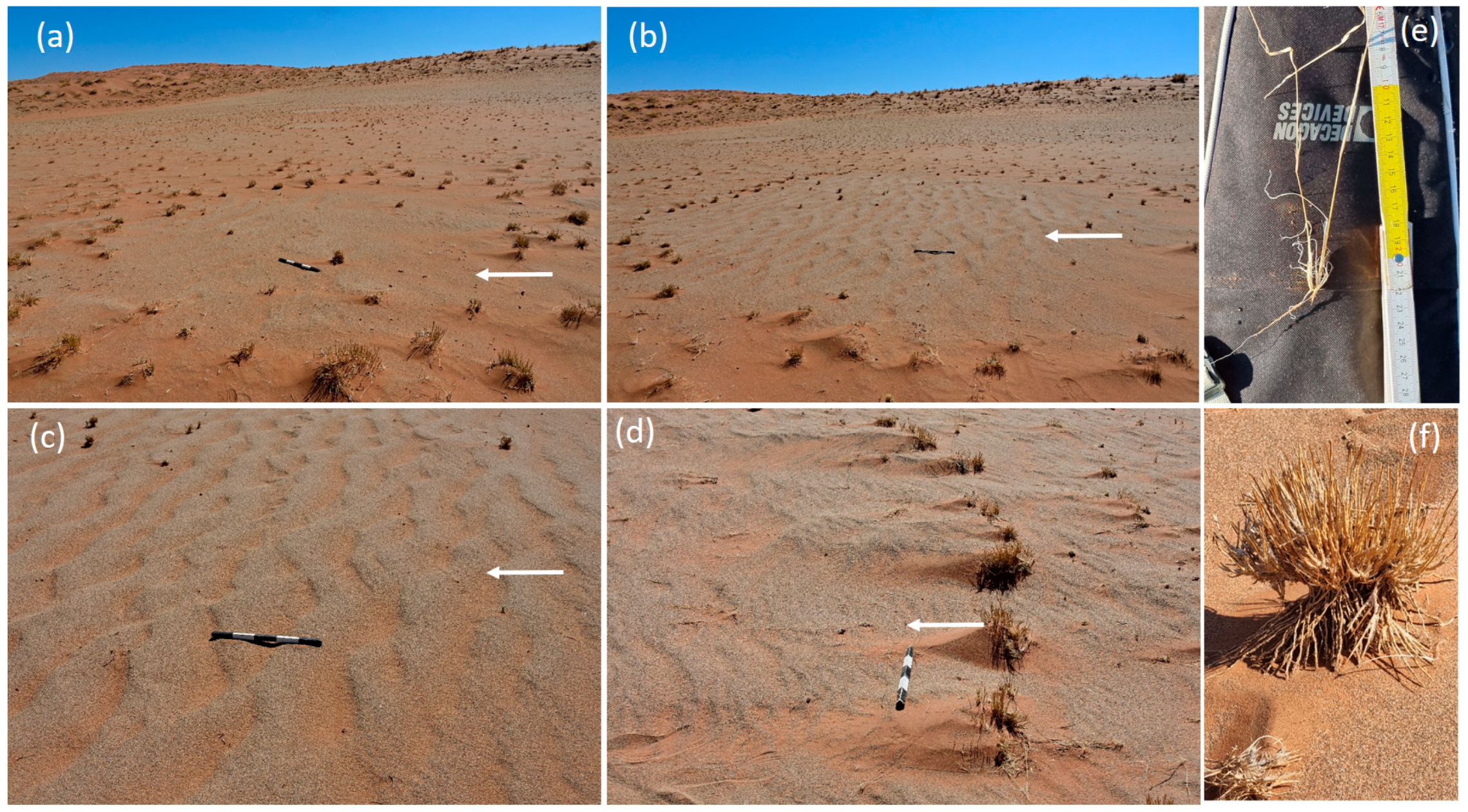
Figure 2.
The fairy circles near Sesriem Namibia (latitude 24°36.5104′ S, longitude 15°40.4276′ E) look more like vegetation rings in the dry season, as the vegetation in the matrix is very sparse (panels (
a,
b)) due to the lower precipitation rate in the year before our measurements. The interior is predominantly covered by small megaripples (panel (
c); average wavelength ~30 cm), whereas the plants at the periphery form small nebkhas (
d) due to the accumulation of fine sand behind the plants that act as barriers for sand transport. The prevailing wind direction is from the east, indicated by the white arrows. Note the difference between the greyish coarse grains (mode 900 µm) and the reddish fine sand (mode 230 µm). The red color is caused by iron oxide [
19]. The length of the scale bar is 50 cm. (
e) Dead seedling from the center of a fairy circle that appears to have died due to a lack of water and not due to root harvesting termites. (
f)
Stipagrostis ciliata grass with exposed roots due to wind erosion at the edge of the fairy circle.
Figure 2.
The fairy circles near Sesriem Namibia (latitude 24°36.5104′ S, longitude 15°40.4276′ E) look more like vegetation rings in the dry season, as the vegetation in the matrix is very sparse (panels (
a,
b)) due to the lower precipitation rate in the year before our measurements. The interior is predominantly covered by small megaripples (panel (
c); average wavelength ~30 cm), whereas the plants at the periphery form small nebkhas (
d) due to the accumulation of fine sand behind the plants that act as barriers for sand transport. The prevailing wind direction is from the east, indicated by the white arrows. Note the difference between the greyish coarse grains (mode 900 µm) and the reddish fine sand (mode 230 µm). The red color is caused by iron oxide [
19]. The length of the scale bar is 50 cm. (
e) Dead seedling from the center of a fairy circle that appears to have died due to a lack of water and not due to root harvesting termites. (
f)
Stipagrostis ciliata grass with exposed roots due to wind erosion at the edge of the fairy circle.
![Land 13 00197 g002]()
3. Results
Figure 5 shows two fairy circles, FC
8 covered with sand megaripples and FC
9 covered with normal ripples composed mainly of fine sand, and the corresponding soil water content in the cross-sections. In both fairy circles, FC
8 and FC
9, the upper 12 cm is very dry (~1%), whereas at a depth of 20 cm, the soil water content is about four times higher.
Figure 6 shows that the megaripples in the interior of the fairy circle are a source of heterogeneity in the soil water content at 20 cm due to small difference between the crest and the troughs. As the crest is higher than the trough, the probe in the trough can reach slightly deeper and wetter soil.
Figure 7 shows the results of the soil water content in the cross-section for two fairy circles, FC
1 and FC
5 (
Figure 7a). The soil water content at 20 cm is again about four times larger than the soil water content at 12 cm. This behavior is robust for all 10 of the fairy circles, as shown in
Figure 7b and
Figure S2. The values of the normalized
index for the two depths is shown in
Figure 7c. For FC
9 the values are quite low, and are higher for the soil depth of 12 cm than 20 cm. The calculated hydraulic conductivity (
k) in the center of the fairy circles is shown in
Figure 7d. The values are larger than the typical values of loose dry sand (0.15 ± 0.04 cm/s).
The different modes,
and
, inside and outside the fairy circles, are shown in
Figure 8a,b. The grain size distribution of FC
8 that is covered by megaripples and of FC
9 that is covered by normal ripples is shown in
Figure 9 and the photos of the typical coarse and fine grains are shown in
Figure 10 with the grain size distribution of the silt fraction. Normally, the pores between the monodisperse coarse grains are larger than those between the monodisperse finer grains, enabling water to percolate quickly to the deeper soil layers.
Generally, due to the megaripple formation, the sand at the upper layers inside the fairy circles is coarser than the sand outside the fairy circles. In , there is a significant difference between the inside and outside environments for most of the fairy circles (FC2, FC4, FC5, FC6, FC8, FC10). In those fairy circles, the is much coarser (~800 µm) inside the circle compared with the soil outside the circles (~200 µm). This trend is also found for , which is much larger inside (~970 µm) the circles than outside (~400 µm). The megaripple crest inside the fairy circles contains coarse grains in the range of 1000–1200 µm, except for the circles FC7 and FC9, for which the interior is not covered by megaripples.
As one of our major findings
Figure 8c shows that there is a linear trend between the average SWC at 20 cm and the fairy circle size (the FC diameter). The larger the fairy circle size, the higher is SWC. A similar trend also exists with
inside the fairy circle, as shown in
Figure 8d.
Interestingly, there is also a correlation between the FC size and the median grain diameter,
(of sand from the FCs interior), as shown in
Figure 11a. The coarser the grain, the larger the SWC is (
Figure 8d). The negative correlation between dw12 and the FC size (
Figure 11b) may be seen as an indication that SWC can diffuse laterally, which would support the vegetation at the periphery. The soil water diffusion coefficient,
, is also larger for the coarser grains. A numerical simulation of a model for the Namibian FC showed that the FC size increases with
[
13], in agreement with our new finding. The full correlation relations analyses between the various measurement variables are shown in
Figure S4.
4. Discussion
The fairy circles studied in this work are located at the extreme part of the Namib desert, where rainfall is low (79 mm annual average), with high inter-annual variability. Possibly because of the small limited area at the foot of the big dunes, they are not spatially periodic and lack the characteristic hexagonal ordering of fairy circles seen in other sites in Namibia like the Giribes plains or the Namib Rand Nature Reserve [
2,
9].
In very dry years (e.g., 2023), the vegetation in the matrix is very sparse and the fairy circles are more similar to vegetation rings, but in rainy years (e.g., 2011 with 180 mm and 2012 with 134 mm) the vegetation in the matrix recovers and the fairy circles become vegetation gaps, similar to the known fairy circles in the more humid sites in Namibia, with a visible perennial belt [
11].
Most of the fairy circles in our studied area are covered with megaripples with a wavelength in the range of 20–30 cm and a height of 2 cm, which is relatively small compared to other known megaripples, e.g., [
22,
23]. Megaripples feature a characteristic bimodal grain size distribution, shaped by the bimodal transport process and the prevailing wind shear stress. The coarse peak corresponds to the grains that move only by reptation induced by saltating fine grains, found in the fine-grain peak [
22]. However, the non-trivial interaction of the vegetation pattern with the sand transport makes megaripples in FCs a special case, potentially different from those in larger bare megaripple fields, without interfering vegetation. In particular, the trapping of fine saltating grains by the vegetation, as is well established for so-called nebkhas (
Figure 2d), may be responsible for systematic deviations between the GSDs inside and outside the FCs, as shown by Ravi et al. [
24] for FCs in Mirabib, Namibia, and other studies [
25], and in
Figure 9 (featuring the GSDs for two FCs). The observed absence of a clear fine-grain peak in the GSDs inside the FCs is thus most likely due to the fine, saltating grains being trapped with a higher rate by the vegetation outside and at the perimeter of the FCs than inside the FCs, leading to a large-scale lateral sorting. The induced under-saturated saltation flux leads to erosive conditions inside the FCs, favoring the formation of megaripples.
By taking into account both GSDs outside and inside of each FC, we arguably obtain a more complete picture of the transported grains that contribute to megaripple formation inside the FCs. In particular, we can then exploit that the margins of the coarse-grain (reptation) peak of the megaripples are sensitive to the prevailing wind conditions. This enables us to estimate the prevailing wind conditions, following the method proposed in [
22]. Applying it to the GSDs of FCs covered by megaripples, the mean wind velocity at a 10 m height evaluates to
m/s, which compares well to the mean wind speed near the measurement site of about 4 m/s (
https://globalwindatlas.info/en, accessed on 22 November 2023). A slight upward deviation of the inferred value as compared to the yearly average resonates with the usually stronger-than-average winds in the months prior to our measurements (
https://globalwindatlas.info/en, accessed on 22 November 2023). Hence, altogether, the GSD analysis seems consistent with the predominant wind conditions and the notion of megaripple formation inside the FCs, yet it also hints at the special character of the grain sorting and megaripple formation inside the FCs compared to larger vegetation-free megaripple fields. The preliminary results by Yizhaq et al. (unpublished;
Figure S5), based on the model of Manukyan and Prigozhin 2009 [
26], appear to be consistent with our observations.
In addition to the spatially varying deposition rate due to the vegetation at the FCs’ periphery and matrix, the location of the study site in the deposition zone of a dune (
Figure 3b) may induce further spatial irregularities in the GSDs. It can be qualitatively stated that the absence of megaripples is observed in the two FCs (7 and 9) with the closest upwind distance to the nearby dune. More extensive field measurements might yield a more systematic correlation between the presence or absence of megaripples and the distance to an upwind dunes, which act as sources of fine, saltating grains. In any case, our present observation is consistent with the sorting model of Lämmel et al. 2018 [
27], which requires a low saturation of the wind with fine grains to allow for megaripple formation, and with the common knowledge that megaripple formation is strongly impeded in natural dune sands [
10,
23].
It seems plausible that the presence of megaripples may increase rainwater infiltration into the soil and thus indirectly increase the lateral diffusion of soil water to the periphery [
5]. Such a correlation is supported by the linear relation between the average SWC at 20 cm (
Figure 8d) and the median grain diameter inside the fairy circles. Moreover, the trapping of fine particles and the formation of small nebkahs around the grasses along the FC perimeter (
Figure 2d) increases the soil water retention at the surface, which helps the vegetation to survive in this extreme environment. Altogether, the larger fairy circles thus seem to be more efficient in deep water harvesting both due to their larger area and their coarser sands (
Figure 8c and
Figure 11a), which may help support the vegetation circles along their perimeter. Consistently, we find the larger fairy circles in the studied site to be more resistant to prolonged droughts than the smaller ones that can diminish in drought years, as shown in
Figure 12.
We also note that the distinction between the FCs and vegetation rings and our finding of megaripple formation in the former, seems significant. Namely, in contrast to fairy circles, the interior of small vegetation rings covered by fine grains has been found to enhance the growth of biocrust compared to fringes, thereby decreasing the infiltration into the center, for
Asphodelus ramosus rings in the Northern Negev, Israel ([
20];
Figure S6).
The dry and coarser topsoil inside the fairy circle (
Figure S7) may prevent the recolonization of new seedlings inside the fairy circles [
6]. Indeed, we found a few dead seedlings (
Figure 2f and
Figure 13) without any sign of damage caused by termites and with short root lengths (~10 cm), hence, too short to reach the deeper soil layers with a higher water content [
6]. Although there is a reservoir of soil water in the deeper layers, the upper layer remained very dry (SWC at around 2%), well below the wilting point [
28], thereby stabilizing the bare patches, especially during prolonged droughts.
The lack of the typical hexagonal order of fairy circles in the study site remains unclear and can be possibly attributed to the relatively limited area available for fairy circle growth in the foot of the large dunes. Further studies in this site located in the extreme edge of the climatic strip of Namibian fairy circles can help to solve the ongoing debate about the origin of fairy circles [
2,
8,
18,
27,
29,
30,
31,
32,
33,
34].