Mathematical Analysis on an Asymmetrical Wavy Motion of Blood under the Influence Entropy Generation with Convective Boundary Conditions

Abstract

In this article, we discuss the entropy generation on the asymmetric peristaltic propulsion of non-Newtonian fluid with convective boundary conditions. The Williamson fluid model is considered for the analysis of flow properties. The current fluid model has the ability to reveal Newtonian and non-Newtonian behavior. The present model is formulated via momentum, entropy, and energy equations, under the approximation of small Reynolds number and long wavelength of the peristaltic wave. A regular perturbation scheme is employed to obtain the series solutions up to third-order approximation. All the leading parameters are discussed with the help of graphs for entropy and temperature profiles. The irreversibility process is also discussed with the help of Bejan number. Streamlines are plotted to examine the trapping phenomena. Results obtained provide an excellent benchmark for further study on the entropy production with mass transfer and peristaltic pumping mechanism.

1. Introduction

In our daily life, living organisms require energy to do physical work and keep the body temperature under the influence of heat exchange to the environment, as well as to generate, replace, and propagate molecules to the relevant constituents. Such type of energy comes from the oxidation process of organic substances i.e., amino acids, fats, and carbohydrates fed to the organisms. As compared to the other heat engines (i.e., in which the chemical energy gets transformed to the thermal energy, and then is transformed to mechanical work), living organisms can transform the nutrient’s chemical energy into work. It happens due to the oxidation of nutrients located internally in the organisms i.e., metabolism, pass through different steps, which helps to hold some energy from ATP (adenosine triphosphate). The ATP utilized entirely by all beings for the direct transformation of mechanical energy and also actively supports other biological reactions [1]. In recent years, various authors [2,3,4,5,6] have examined the heat production of mammals via calorimetry, and presented that for the given nutrients, both combustion and animal metabolism expends the same amount of oxygen. According to previous research [7], it is found that living things can produce thermal energy via fat, and combustion of carbohydrates in the living body, and is identical to the oxidation of heat of these elements. As a result, the amount of nutrients digested by a living being, and hence its input energy, can be determined by the chemical composition of food intake and the measurements of breathing i.e., CO${}_2$ and O${}_2$.

Hershey [8] and Hershey and Wang [9] examined the entropy production during the lifespan of a human being. They found that when the human body is in a state of rest, mostly the output energy due to the nutrient’s metabolism occurs as a heat. They also reinforced the calorimeter to examine the heat transfer rate to the environment and verified that with the help of BMR (basal metabolic). According to their results, it was found that entropy generated over a lifespan was 10,678 KJ/kg·K for females, and 10,025 KJ/kg·K for males. Rahman [10] discussed the entropy generation for forced and free convection using a new mathematical model. He discussed forced and free convection at distinct mass influx, outflux (i.e., waste, air, food, and water, etc.), level of physical activity, clothing effects, and airspeeds. His results are similar to those from Hershey [8] and Aoki [11] but one order of magnitude higher in general. Annamalai and Puri [12] utilized the first law of thermodynamics to achieve the metabolic scaling for a biological system. They also used the second law of thermodynamics to determine the entropy generation in humans and prognosticate the lifespan of 77 years by assuming the maximal entropy generation as 10,000 KJ/kg·K. Bejan [13,14] introduced a constructal design principle and presented optimal geometric types scales to the power of their associated size and showed that different natural structures (i.e., lightening, river deltas, tree branches, and vascularized tissue) are periodic in nature. Rashidi et al. [15] discussed the entropy generation with magnetic effects and slip boundary conditions propagating among an infinite porous disk having variable features. According to their results, they observed that the disk is an essential root of entropy generation. Komurgoz et al. [16] examined the entropy generation through an inclined porous channel with magnetic effects. According to their results, they found that maximal entropy production can be gained in the absence of porosity and magnetic field.

Blood is an essential part of the human body, which comprises 7% of the total body weight. The leading role of blood is molecular oxygen for cellular metabolism and carry the nutrients as well as a significant role in thermoregulatory. Blood performs as a non-Newtonian fluid. The blood viscosity changes due to the shear rate. The viscosity of the blood can be analyzed by the hematocrit, plasma (constitute 54.3% of the whole blood) viscosity, and the mechanical features of red blood cells (constitute 45% of the whole blood). The human blood is a heterogeneous solution that contain multiple kinds of cells (known as corpuscles or formed elements), which consist of leukocytes, thrombocytes, and erythrocytes. In view of such importance, different authors discussed the entropy generation in blood. For instance, Rashidi et al. [17] discussed the magnetic effects on the blood flow propagating through a porous medium with a filtration and control process. Akbar et al. [18] examined the thermal conductivity on the peristaltic propulsion of H${}_2$+Cu nanofluids with entropy production. Rashidi et al. [19] obtained the series solution for the entropy generation of the blood flow of a nanofluid in the presence of a magnetic field. Endoscopic effect and entropy production on peristaltic nanofluid flow, having a thermal conductivity of 2 HO were investigated by Akbar et al. [20]. Abbas et al. [21] presented a detailed analysis of the peristaltic flow with nanofluids and entropy production through a finite channel with compliant walls. Bhatti et al. [22] considered the Casson blood flow to examine the entropy process with peristaltic movement under the uniform magnetic field. Ranjit and Shit [23] examined the entropy production on the electroosmotic flow under uniform magnetic field with peristaltic pumping. More studies on the blood flow and entropy generation can be found from the references [24,25,26,27,28].

According to the above survey, it is found that less attention has been given to the entropy production asymmetric peristaltic propulsion of blood flow with heat transfer. Therefore, in the present analysis, we discuss the entropy generation with convection on the asymmetric propulsion of the peristaltic blood of nonlinear Williamson fluid. An assumption of long peristaltic wavelength is taken into account and Reynolds number is considered to be very small (Re ≈ 0). A regular perturbation method is used to obtain series solutions. The novelty of all the leading parameters is discussed and illustrated. The trapping mechanism is also examined to determine the nonlinear asymmetric peristaltic motion.

2. Governing Equations

In this section we analyze the incompressible peristaltic propulsion of Williamson fluid in a two-dimensional channel with a width $\overline{d_1}+\overline{d_2}$. The flow is initialized by a sinusoidal wave propagating with a constant speed c along the layout of channel (see Figure 1). The addition here is the extra equations of energy and entropy generation. It is assumed that the temperature at the upper wall of the channel is $T_1$ and lower wall has the temperature $T_0$ such that $T_0<T_1.$ It depicts the physical reasoning that heat will transfer from lower to upper wall. The wall surfaces are suggested as:

$$\begin{array}{c}\hfill \overline{Y}=\left\{\begin{array}{c}{H}_i=\overline{d_i}+\overline{a_i}cos\left[2\pi \omega \right],i=1,\\ H_j=-\overline{d_j}-\overline{b_i}cos\left[2\pi \omega +\varphi \right],j=2,\end{array}\right.\end{array}$$

(1)

and

$$\begin{array}{c}\hfill \omega ={\displaystyle \frac{\overline{X}-c\overline{t}}{\lambda }},\end{array}$$

(2)

where $\overline{a_1}$ and $\overline{b_1}$ are the wave amplitudes, $\lambda$ the wave length, $\overline{t}$ the time, c the velocity of the propagation, and $\overline{X}$ is the direction of wave propagation. The phase difference $\varphi$ has the range $0\le \varphi \le \pi$ i.e., waves out of phase $\varphi =0$ associated to the symmetric channel, and $\varphi =\pi$ associated to the waves are in phase. Moreover, $\overline{a_i}$, $\overline{b_i}$, $\overline{d_i}$, $\overline{d_j}$, and $\varphi$ satisfy the condition:

$${\overline{a_i}}^2+{\overline{b_i}}^2+2\overline{a_i}\overline{b_i}cos\varphi \le {\left(\overline{d_i}+\overline{d_j}\right)}^2.$$

(3)

The equations of momentum in component forms are described as:

$$\rho \left[D_{\overline{t}}+\overline{U}{D}_{\overline{X}}+\overline{V}{D}_{\overline{Y}}\right]\overline{U}=-D_{\overline{X}}P+D_{\overline{X}}{\overline{S}}_{\overline{XX}}+D_{\overline{Y}}{\overline{S}}_{\overline{XY}},$$

(4)

where $D_{\overline{t}}=\frac{\partial }{\partial \overline{t}},D_{\overline{X}}=\frac{\partial }{\partial \overline{X}},D_{\overline{Y}}=\frac{\partial }{\partial \overline{Y}}$.

$$\rho \left[D_{\overline{t}}+\overline{U}{D}_{\overline{X}}+\overline{V}{D}_{\overline{Y}}\right]\overline{V}=D_{\overline{Y}}P+D_{\overline{X}}{\overline{S}}_{\overline{YX}}+D_{\overline{Y}}{\overline{S}}_{\overline{YY}}.$$

(5)

The stress tensor for the Williamson fluid model reads as:

$$\overline{\mathbf{S}}=\left[{\overline{\mu }}_{\infty }-({\overline{\mu }}_{\infty }-{\overline{\mu }}_0){\left(1-\overline{\Gamma }\stackrel{.}{\gamma }\right)}^{-1}\right]\stackrel{.}{\gamma },$$

(6)

where ${\overline{\mu }}_{\infty },{\overline{\mu }}_0$ the infinite and zero shear rate viscosity, $\overline{\Gamma }$ the time constant, and $\stackrel{.}{\gamma }$ reads as:

$$\begin{array}{c}\hfill \stackrel{.}{\gamma }={\left({\displaystyle \frac{1}{2}}\sum _m\sum _n{\stackrel{.}{\gamma }}_{mn}{\stackrel{.}{\gamma }}_{nm}\right)}^{\frac{1}{2}}={\left({\displaystyle \frac{1}{2}}{S}_t\right)}^{\frac{1}{2}},\end{array}$$

(7)

where $S_t$ is the second invariant strain tensor. For the present flow problem, we considered ${\overline{\mu }}_{\infty }=0$ (the infinite shear rate viscosity is very small as compared to zero shear rate viscosity) and $\overline{\Gamma }\stackrel{.}{\gamma }<i$ i.e., $i=1$. Then, Equation (6) takes the following form:

$$\overline{\mathbf{S}}={\overline{\mu }}_0{\left(1-\overline{\Gamma }\stackrel{.}{\gamma }\right)}^{-1}\stackrel{.}{\gamma }.$$

(8)

The energy equation to represent the heat exchange in the channel is as stated below. The law of conservation of energy in the dimensional mathematical pattern is given by:

$$S_h\left[D_{\overline{t}}+\overline{U}{D}_{\overline{X}}+\overline{V}{D}_{\overline{Y}}\right]T=\frac{K}{\rho }\left[D_{\overline{XX}}T+D_{\overline{YY}}T\right]+\frac{{\overline{S}}_{\overline{XY}}}{\rho }{D}_{\overline{Y}}\overline{U}.$$

(9)

In the above equation, $S_h$ is the specific heat coefficient, K the thermal conductivity, and $\rho$ the density of the governing fluid.

Introducing wave frame coordinates transformations with propagation velocity c away from the fixed frame read as:

$$\{\overline{x}+c\overline{t},\overline{u}+c,\overline{y},\overline{v},\overline{P}\left(\overline{x}\right)\}=\{\overline{X},\overline{U},\overline{Y},\overline{V},\overline{P}\left(\overline{X},t\right)\}$$

(10)

Defining the dimensionless quantities as:

$$\begin{array}{cc}\hfill & x=\frac{\overline{x}}{\lambda },y=\frac{\overline{y}}{\overline{d_i}},u=\frac{\overline{u}}{\lambda },v=\frac{\overline{v}}{\lambda },S_{xx}=\frac{\lambda }{{\overline{\mu }}_{0}c}{\overline{S}}_{\overline{XX}},S_{xy}=\frac{\overline{d_i}}{{\overline{\mu }}_{0}c}{\overline{S}}_{\overline{XY}},S_{yy}=\frac{\overline{d_i}}{{\overline{\mu }}_{0}c}{\overline{S}}_{\overline{YY}},\hfill \\ \hfill & \theta =\frac{T-T_0}{T_i-T_0},p=\frac{{\overline{d_i}}^2}{c\lambda {\overline{\mu }}_0}P,\stackrel{.}{\gamma }=\frac{\overline{\stackrel{.}{\gamma }}\overline{d_1}}{c},a=\frac{\overline{a_i}}{\overline{d_i}},b=\frac{\overline{b_i}}{\overline{d_i}},d=\frac{\overline{d_j}}{\overline{d_i}},h_{i,j}=\frac{H_{i,j}}{\overline{d_i}},\hfill \end{array}$$

(11)

where $\theta$ is the dimensionless temperature profile.

By invoking the above transformations in Equations (4)–(6), we arrive at (after ignoring the bars):

$$\mathrm{Re}\left[\delta u{D}_{x}u+v{D}_{y}u\right]=-D_{x}p+D_x{S}_{xx}+D_y{S}_{xy},$$

(12)

$$\mathrm{Re}\delta \left[\delta u{D}_{x}v+v{D}_{y}v\right]=-D_{y}p+D_y{S}_{yy}+{\delta }^2{D}_x{S}_{yx},$$

(13)

$$P_r\mathrm{Re}\left[\delta u{D}_x\theta +\delta v{D}_y\theta \right]=\left[-{\delta }^2{D}_{xx}\theta +D_{yy}\theta \right]+B_r{S}_{xy}{D}_{y}u\theta ,$$

(14)

and

$$S_{xy}=-\left(1+We\stackrel{·}{\gamma }\right)\left(D_{y}u+\delta D_{x}v\right),$$

(15)

where,

$$\begin{array}{cc}\hfill \delta & =\frac{\overline{d_1}}{\lambda },\mathrm{Re}=\frac{\rho c\overline{d_1}}{{\mu }_0},We=\frac{\Gamma c}{\overline{d_i}},B_r=P_r{E}_c,P_r=\frac{v{S}_h\rho }{K},E_c=\frac{c^2}{S_h\left(T_i-T_0\right)}.\hfill \end{array}$$

(16)

In the above equation, $We$ the Weissenberg number, $E_c$ is the Eckert number, $P_r$ the Prandlt number, $\mathrm{Re}$ the Reynolds number, and $B_r$ the Brinkman number. Under the assumptions of long wavelength and low Reynolds numbers ($\delta \approx 1$, $\mathrm{Re}$$\approx 0)$, Equations (12)–(14) take the form:

$$D_{x}p=D_y\left[\left(1+We{D}_{y}u\right)D_{y}u\right],$$

(17)

$$D_{y}p=0,$$

(18)

$$D_{yy}\theta =-B_r\left[{\left(D_{y}u\right)}^2+We{\left(D_{y}u\right)}^3\right].$$

(19)

This equation implies that $p\ne p\left(y\right)$ so $\partial p/\partial x$ can be written as $dp/dx.$ At $We=0$, the above equation turns into viscous fluid flow. The associated no slip and convective boundary conditions selected for the problem read as:

$$\begin{array}{cc}\hfill & u=-1,{\theta }^{\prime }-B_i\theta =-B_i\mathrm{at}y=h_i\left(x\right)=1+acos2x\pi ,\hfill \\ \hfill & u=-1,\theta =0\mathrm{at}y=h_j\left(x\right)=-d-bcos\left(\varphi +2\pi x\right).\hfill \end{array}$$

(20)

where $B_i$ is the Biot number.

3. Entropy Generation Analysis

According to the theory of thermodynamics, the physical process can be divided in to two types: Irreversible and reversible process. The characterization of such kind of procedures is associated with the change of entropy. Particularly, we say that the process is reversible if there is no change in the entropy, whereas, if the change occurs i.e., entropy is not zero, it shows that the process is irreversible. Therefore, the production of entropy is the measure of the irreversibility of a process. All the processes that arise in nature are irreversible and this reveals a significant obstacle in the study of that process.

The entropy generation in the dimensional form can be defined as:

$$\begin{array}{c}\hfill S_{gen}^{\prime }={\displaystyle \frac{K}{T_0^2}}{\left(D_{\overline{Y}}T\right)}^2+{\displaystyle \frac{{\overline{S}}_{\overline{XY}}}{T_0}}{D}_{\overline{Y}}\overline{U}.\end{array}$$

(21)

Here we define some new dimensionless quantities in addition to those used above:

$$\begin{array}{c}\hfill S_g^{\prime }=\frac{K\left(T_i-T_0\right)}{T_0^2\overline{d_i^2}},\Delta =\frac{T_0}{K\left(T_i-T_0\right)}.\end{array}$$

(22)

Using Equation (22) in Equation (21), we get the dimensionless form of entropy generation:

$$\begin{array}{c}\hfill N={\displaystyle \frac{S_{gen}^{\prime }}{S_g^{\prime }}}={\left(D_y\theta \right)}^2+\Delta B_r\left[-1+We{D}_{y}u\right]{\left(D_{y}u\right)}^2.\end{array}$$

(23)

In the above expression, $\Delta$ shows the entropy production characteristics and temperature difference parameter. Equation (23) is divided into two parts. The first is due to the finite temperature difference whereas the second part defines the fluid frictional irreversibility.

The Bejan number is describe as the entropy production ratio because of heat transfer irreversibility to the total entropy production:

$$\begin{array}{c}\hfill Be={\displaystyle \frac{{\left(D_y\theta \right)}^2}{{\left(D_y\theta \right)}^2+\Delta B_r\left[-1+We{D}_{y}u\right]{\left(D_{y}u\right)}^2}}.\end{array}$$

(24)

Bejan number lies between 0 to 1. $Be<1$ represents that the total entropy production dominates the total entropy production due to heat transfer. $Be=1$ represents when the total entropy production is equal to entropy production due to heat transfer irreversibility.

4. Series Solution

Since Equation (17) is non linear, its exact solution may not be possible, therefore, we employ the regular perturbation method to find the solution. For perturbation solution, we expand $u,$ F and $dp/dx$ as:

$$\begin{array}{c}\hfill u=\sum _{n=0}^{\infty }W{e}^n{u}_n,\end{array}$$

(25)

$$\begin{array}{c}\hfill F=\sum _{n=0}^{\infty }W{e}^n{F}_n,\end{array}$$

(26)

$$\begin{array}{c}\hfill {\displaystyle \frac{dp}{dx}}=\sum _{n=0}^{\infty }W{e}^n{\displaystyle \frac{d{p}_n}{dx}},\end{array}$$

(27)

Substituting above expression in Equation (17) and their boundary conditions in Equation (20) and comparing the coefficients of powers of $We$ we get the zeroth and first order systems which can be manipulated easily by a mathematical computing tool Mathematica and are conclusively stated as:

$$\begin{array}{cc}\hfill u=& \frac{1}{2!}\left[-2+C_1{h}_1{h}_2-C_1{C}_{3}y+C_1{y}^2\right]+\frac{1}{3!}We\left[C_2{h}_1{h}_2+C_1^2{C}_3{h}_1{h}_2\right.\hfill \\ & \left.-C_2\left(C_3+y\right)y+C_1^2\left(h_1^2-4h_1{h}_2-h_2^2\right)y+C_1^2(3C_3-2y)y\right]+O\left(W{e}^2\right),\hfill \end{array}$$

(28)

$$\begin{array}{cc}\hfill {\displaystyle \frac{dp}{dx}}& =12C_1+12We{C}_2+O\left(W{e}^2\right),\hfill \end{array}$$

(29)

where the constant are defined as:

$$\begin{array}{c}\hfill C_1=\frac{12\left(1+d-h_1+h_2-Q\right)}{{\left(h_1-h_2\right)}^3},\end{array}$$

(30)

$$\begin{array}{c}\hfill C_2={\displaystyle \frac{36\left(1+d-Q\right)}{{\left(h_1-h_2\right)}^3}},\end{array}$$

(31)

$$\begin{array}{c}\hfill C_3=h_1+h_2,\end{array}$$

(32)

$$\begin{array}{c}\hfill C_4=C_3^2+h_1{h}_2,\end{array}$$

(33)

$$\begin{array}{c}\hfill C_5=17C_3^2+4h_1{h}_2,\end{array}$$

(34)

$$\begin{array}{c}\hfill C_6=7C_3^2+2h_1{h}_2.\end{array}$$

(35)

The solution for velocity u obtained by above perturbation method can be used in Equation (19). The final solution for $\theta$ can be obtained by integrating Equation (19) along with their associated boundary conditions (See Equation (20)) and can be written as:

$$\begin{array}{c}\hfill \theta ={\theta }_1+{\theta }_{2}y+{\theta }_3{y}^2+{\theta }_4{y}^3+{\theta }_5{y}^4+{\theta }_6{y}^5+{\theta }_7{y}^6+{\theta }_8{y}^7+{\theta }_9{y}^8,\end{array}$$

(36)

where constants of integration ${\theta }_1$ and ${\theta }_2$ can be evaluated by using boundary conditions defined in Equation (20) and the expression obtained are very large and therefore are not presented here. The remaining constants are defined as:

$$\begin{array}{cc}\hfill {\theta }_3& ={\displaystyle \frac{B_r\sqrt{C_3}}{432}}{\left[3{\displaystyle \frac{d{p}_0}{dx}}+\left(C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+3{\displaystyle \frac{d{p}_1}{dx}}\right)We\right]}^2\hfill \\ \hfill & \times \left[-6+3C_{3}We{\displaystyle \frac{d{p}_0}{dx}}+C_3\left(C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+3{\displaystyle \frac{d{p}_1}{dx}}\right)W{e}^2\right],\hfill \end{array}$$

(37)

$$\begin{array}{cc}\hfill {\theta }_4& =-{\displaystyle \frac{B_r{C}_3}{72}}\left({\displaystyle \frac{d{p}_0}{dx}}+C_{3}We{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+{\displaystyle \frac{d{p}_1}{dx}}\right)\hfill \\ \hfill & \times \left[3{\displaystyle \frac{d{p}_0}{dx}}+\left(C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+3{\displaystyle \frac{d{p}_1}{dx}}\right)We\right]\hfill \\ \hfill & \times \left[-4+3C_{3}We{\displaystyle \frac{d{p}_0}{dx}}+C_3\left(C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+3{\displaystyle \frac{d{p}_1}{dx}}\right)W{e}^2\right],\hfill \end{array}$$

(38)

$$\begin{array}{cc}\hfill {\theta }_5& ={\displaystyle \frac{B_r}{12}}\left[-12{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2-6{\displaystyle \frac{d{p}_0}{dx}}\left(3C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+4{\displaystyle \frac{d{p}_1}{dx}}\right)\right.\hfill \\ \hfill & +\left((7h_1+5h_2)(5h_1+7h_2){\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+18C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2{\displaystyle \frac{d{p}_1}{dx}}-12{\left({\displaystyle \frac{d{p}_1}{dx}}\right)}^2\right)W{e}^2\hfill \\ \hfill & +6{\displaystyle \frac{d{p}_0}{dx}}\left(6C_3{C}_4{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+C_5{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2{\displaystyle \frac{d{p}_1}{dx}}+9C_3{\left({\displaystyle \frac{d{p}_1}{dx}}\right)}^2\right)\hfill \\ \hfill & \left.+C_3\left(C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+3{\displaystyle \frac{d{p}_1}{dx}}\right)\left(C_6{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+15C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2{\displaystyle \frac{d{p}_1}{dx}}+6{\left({\displaystyle \frac{d{p}_1}{dx}}\right)}^2\right)W{e}^4\right],\hfill \end{array}$$

(39)

$$\begin{array}{cc}\hfill {\theta }_6& =-{\displaystyle \frac{B_{r}We}{20}}\left({\displaystyle \frac{d{p}_0}{dx}}+C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+We{\displaystyle \frac{d{p}_1}{dx}}\right)\hfill \\ \hfill & \times \left[-{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+{\displaystyle \frac{d{p}_0}{dx}}We\left(5C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+2{\displaystyle \frac{d{p}_1}{dx}}\right)We\right.\hfill \\ \hfill & +\left.\left(2C_4{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+5C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2{\displaystyle \frac{d{p}_1}{dx}}+{\left({\displaystyle \frac{d{p}_1}{dx}}\right)}^2\right)W{e}^2\right],\hfill \end{array}$$

(40)

$$\begin{array}{cc}\hfill {\theta }_7& ={\displaystyle \frac{B_{r}W{e}^2}{60}}{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2\left[4{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+3{\displaystyle \frac{d{p}_0}{dx}}\left(5C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+4{\displaystyle \frac{d{p}_1}{dx}}\right)We\right.\hfill \\ \hfill & +\left.\left(C_6{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4+15C_3{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2{\displaystyle \frac{d{p}_1}{dx}}+6{\left({\displaystyle \frac{d{p}_1}{dx}}\right)}^2\right)W{e}^2\right],\hfill \end{array}$$

(41)

$$\begin{array}{cc}\hfill {\theta }_8& =-{\displaystyle \frac{B_{r}W{e}^3}{14}}{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^4\left[{\displaystyle \frac{d{p}_0}{dx}}+C_{3}We{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^2+We{\displaystyle \frac{d{p}_1}{dx}}\right],\hfill \end{array}$$

(42)

$$\begin{array}{cc}\hfill {\theta }_9& ={\displaystyle \frac{1}{56}}{B}_{r}W{e}^4{\left({\displaystyle \frac{d{p}_0}{dx}}\right)}^6.\hfill \end{array}$$

(43)

The dimensionless mean flow reads as:

$$\begin{array}{c}\hfill F=d+1-Q.\end{array}$$

(44)

and

$$\begin{array}{c}\hfill F=\stackrel{h_1}{\underset{h_2}{\int }}u\mathrm{d}y.\end{array}$$

(45)

The expression for entropy generation and Bejan number can be easily obtained by incorporating value of u and $\theta$ in Equation (24).

5. Discussion

In this section, we present our results by varying the quantities under the variation of several factors. Figures of temperature profile $\theta$, entropy generation coefficient N, and streamlines are illustrated below. Figure 2, Figure 3, Figure 4 and Figure 5 reflect the behavior of $\theta$ for some useful parameters. Entropy generation graphs are given in Figure 6, Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11. The streamlines conducting the flow samples are depicted in Figure 12 and Figure 13.

Figure 2 shows the impact of parameters a and b on temperature profile $\theta .$ It can be observed from this plot that temperature is getting increased for both parameters from the lower wall to the upper wall. Figure 3 shows the mechanism of the Biot number and Brinkman number. Biot number is an important mechanism to determine the heat transfer. It can be visualized from this figure that an enhancement in Biot number tends to boost the temperature profile while the contrary behavior has been observed with the Brinkman number. Brinkman number is the product of Eckert and Prandtl numbers $B_r=P_r{E}_c$, or it is the ratio of the heat generated by viscous dissipation and propagation of heat by molecular conduction, such as, the ratio of the viscous heat production to extrinsic heating. Therefore, the enhancement of Brinkman’s number tends to increase the temperature profile. It can be seen in Figure 4 that the volumetric flow rate significantly enhances the temperature profile. It can also be noticed that the temperature profile has a lower magnitude for smaller values of d whereas the behavior is converse for higher values. It can be viewed from Figure 5 that the Weissenberg number causes a remarkable resistance for higher values. By enhancing the Weissenberg number, the elastic forces are more dominant, which diminishes the temperature profile. However, the phase difference $\varphi$ also produces a significant resistance in the temperature profile.

Figure 6, Figure 7, Figure 8 and Figure 9 are presented for entropy profiles against the leading parameters. It can be viewed from Figure 6 that an increment in a and b tends to boost the entropy profile whereas the entropy profile is increasing along the whole channel. Figure 7 shows that by increasing the Brinkman number, the entropy profile rises, and it decreases by increasing the Weissenberg number. However, the entropy remains positive and growing along the entire channel. It is seen from Figure 8 that the Biot number enhances the entropy profile. It can be seen that at the lower wall, the entropy profile is maximum and minimum at the upper wall, whereas it is uniform in the middle of the channel. The entropy profile for various values of $\Delta$ is presented in Figure 9. It is noticed in this figure that the entropy profile is uniform, and no change occurs in the middle of the channel i.e., $y\in (0,0.5)$. Although it shows a decreasing pattern, but it rises along the upper wall of the channel and remains positive.

Figure 10 and Figure 11 are plotted for the Bejan number profile against the governing parameters. It is observed from Figure 10 that the Bejan number profile diminishes for higher values of the Brinkman number and shows a converse behavior for the Weissenberg number. In Figure 11, we can see that the phase difference shows versatile behavior for higher values on the Bejan number profile. When Bejan number rises, then the phase difference’s effects are negligible for the domain $y\in (0,1.3)$, while when the Bejan number is small, it decreases in a similar area.

The most interesting and useful phenomena of peristaltic motion are trapping, which is plotted in Figure 12 and Figure 13 via streamlines. It was found that by enhancing the phase difference parameter, the effects are negligible on the trapping bolus despite the fact that an unusual movement in the magnitude of the bolus is noticed. Furthermore, we can see in Figure 13 that an increment in the Weissenberg number profile tends to diminish the width of the trapping bolus. The number of boluses disappeared more quickly in the lower region as compared with the upper one.

6. Conclusions

In this study, we analyzed the entropy generation on the asymmetric peristaltic propulsion of non-Newtonian fluid with convective boundary conditions. The Williamson fluid model was considered to examine the entropy profile. The mathematical modeling was performed under the approximation of small Reynolds number and long wavelength of the peristaltic wave. A regular perturbation method was employed to get the series solutions up to third-order approximation. The significant results of the governing flow problem are summarized below:

(i)

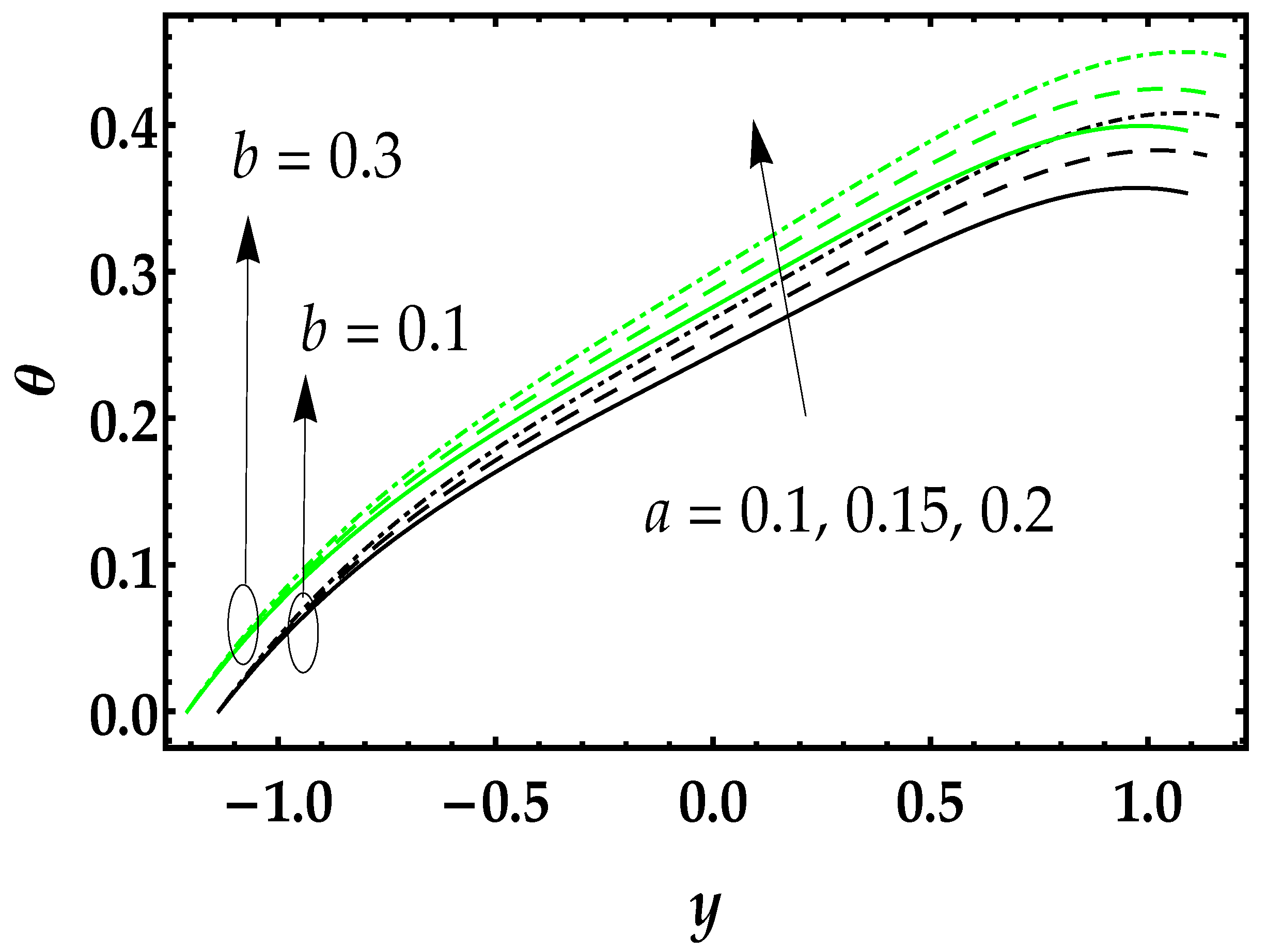
It was noticed that the temperature profile revealed an increasing behavior by increasing the amplitude in the upper and lower region;

(ii)

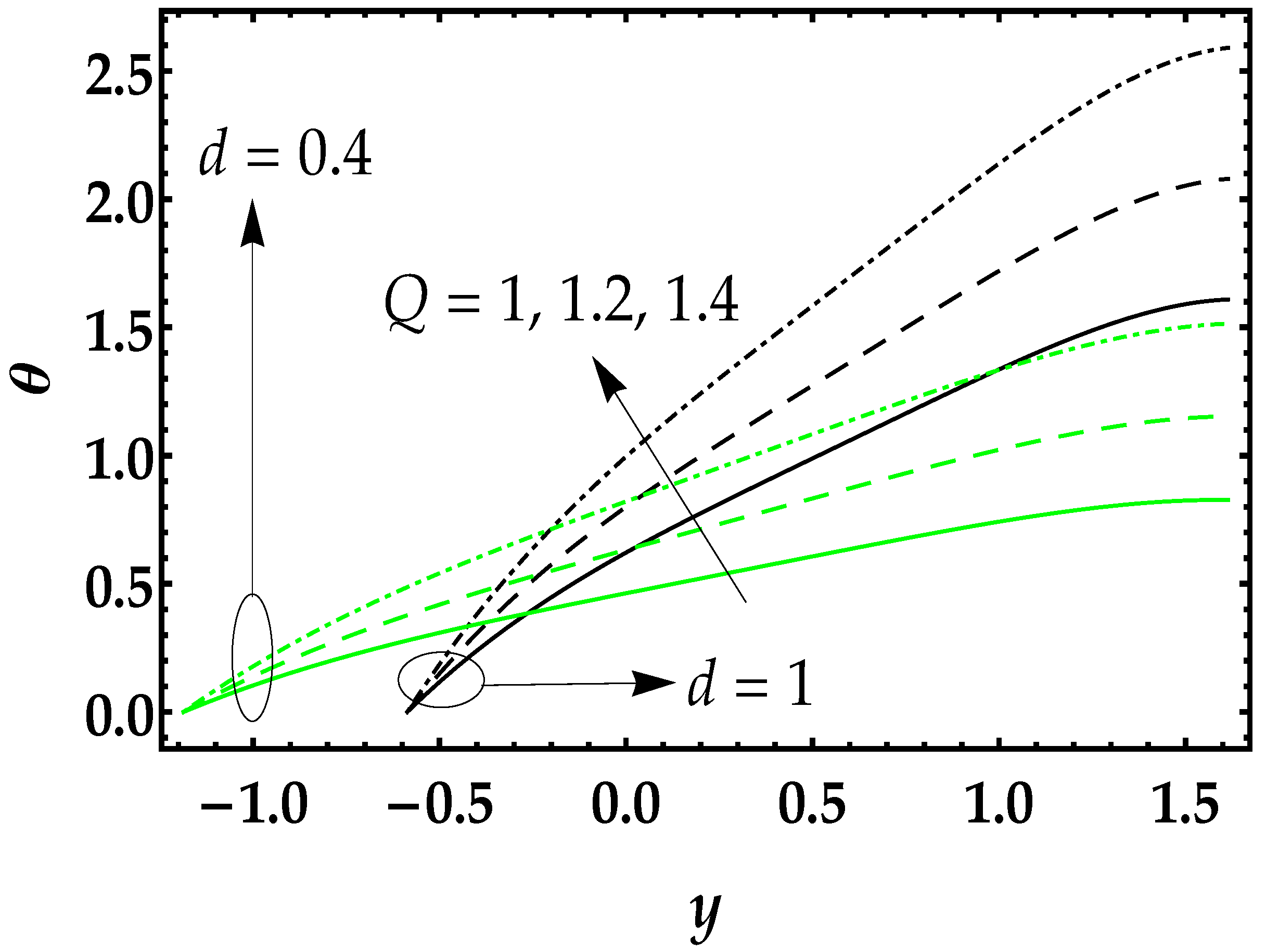
The Biot number and Brinkman number significantly enhanced the temperature profile, whereas the behavior is converse for the phase difference parameter and Weissenberg number;

(iii)

Entropy profile represented an increment profile for higher values of Brinkmann number and Biot number, and a decrement behavior for the Weissenberg number;

(iv)

The Weissenberg number boosedt the Bejan number profile, whereas it decreased due to the Biot number and Brinkman number;

(v)

Trapping mechanism showed that the phase difference parameter affected the magnitude of the trapped bolus, while the Weissenberg number not only affected the magnitude of the trapped bolus and the number of trapped boluses reduced in the lower region;

(vi)

The non-Newtonian results in the present study could be reduced to Newtonian fluid flow by taking $We=0.$

The present results provide an excellent benchmark for further study on the entropy production with mass transfer and peristaltic pumping mechanism. The mass transfer phenomena with magnetic and porosity effects that were not covered in this paper is a topic for future research.