Symmetries in Teleportation Assisted by N-Channels under Indefinite Causal Order and Post-Measurement
Abstract
:1. Introduction
1.1. Background of Indefinite Causal Order in Communication
1.2. Approaches to Teleportation under Causal Order Schemes
2. Teleportation Algorithm as a Quantum Channel and -Redundant Teleportation Problem
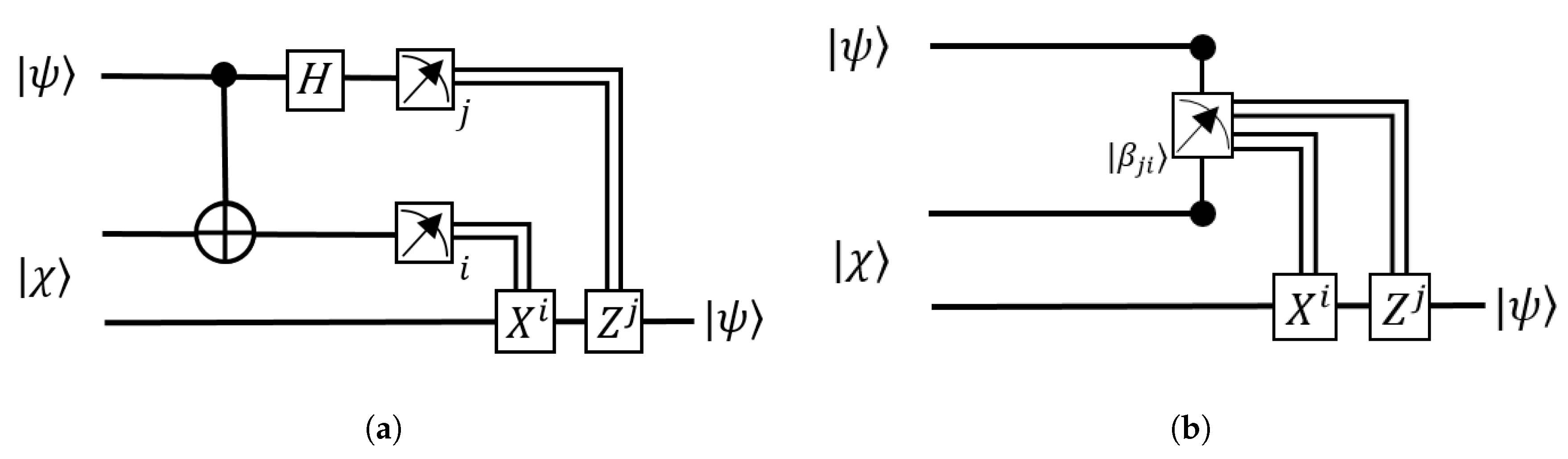
2.1. Quantum Teleportation as a Quantum Channel
2.2. N-Redundant Quantum Teleportation
3. Quantum Teleportation Assisted by Indefinite Causal Order with Channels
4. Analysis of Quantum Teleportation Assisted by the First Indefinite Causal Orders
4.1. Teleportation with Teleportation Channels in an Indefinite Causal Order Superposition
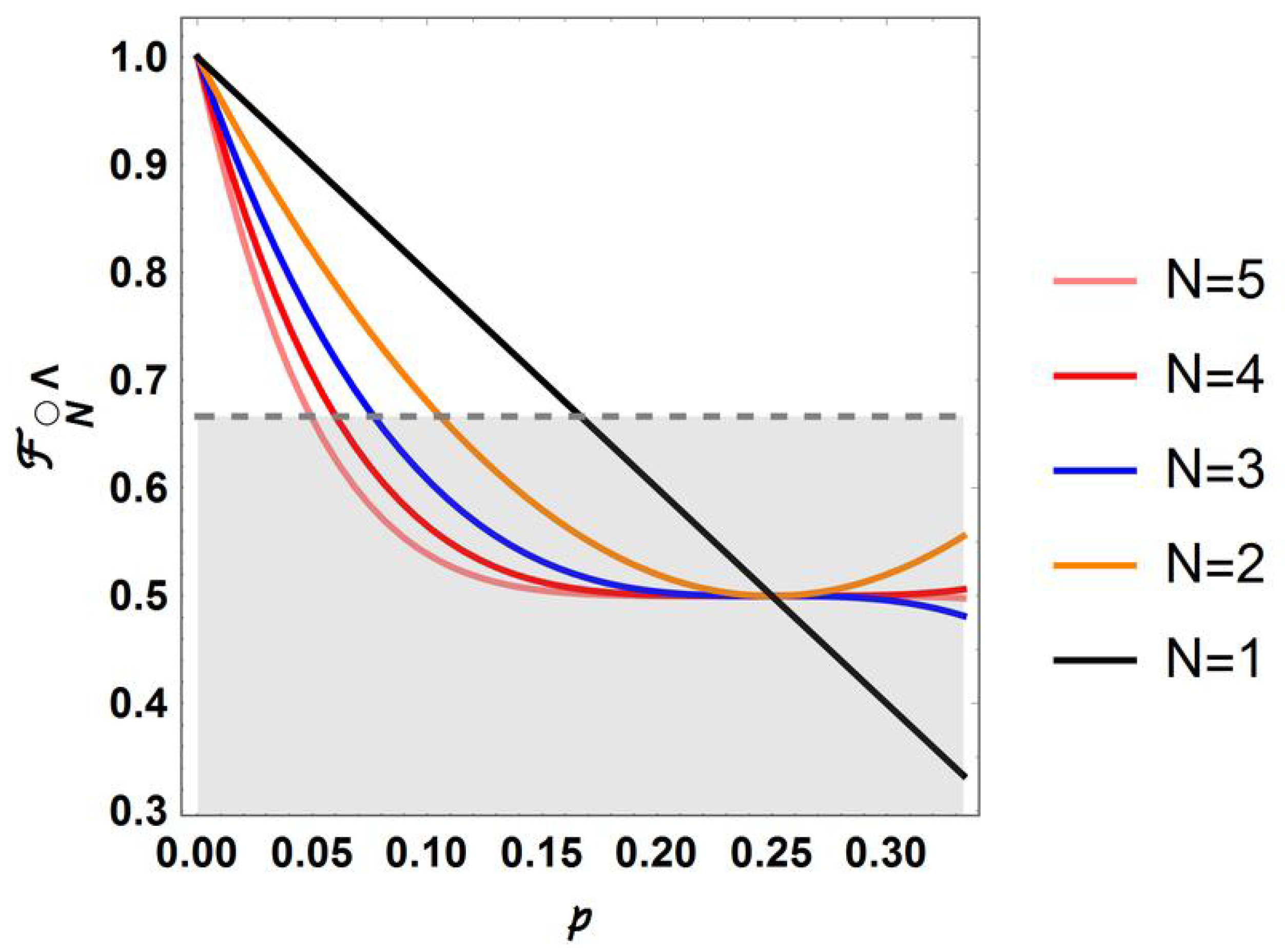
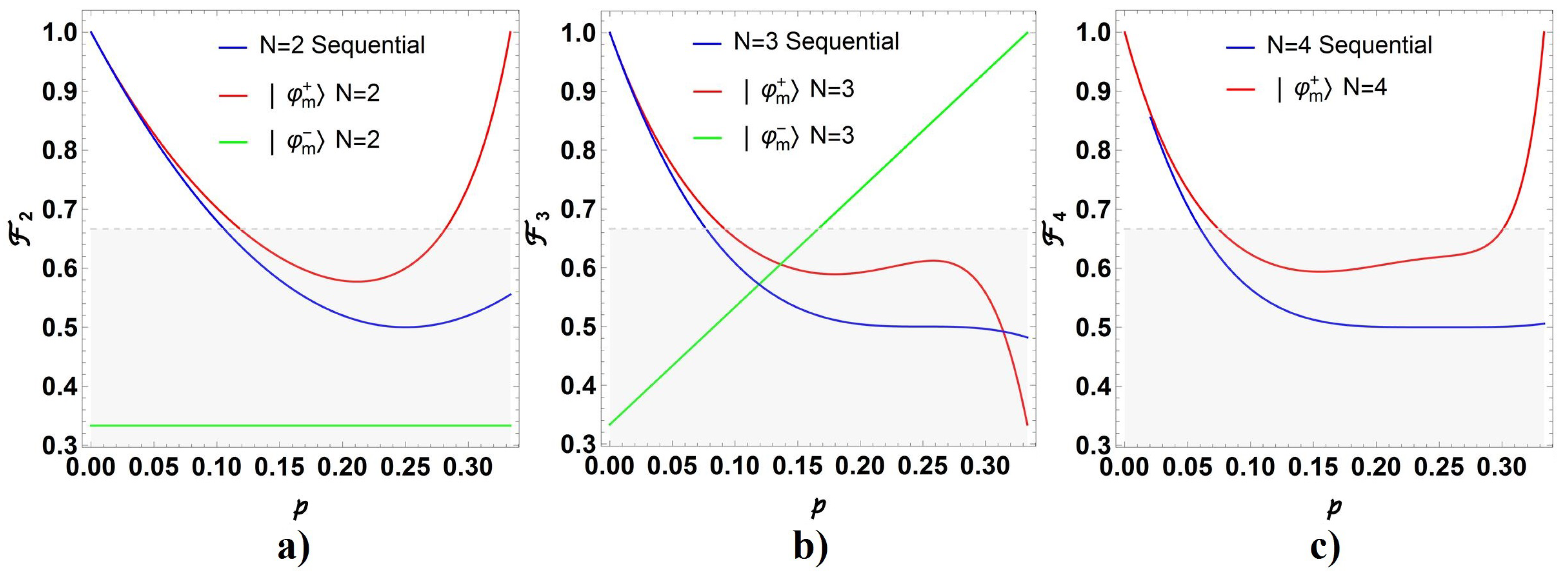
4.2. Teleportation with an Increasing Number of Teleportation Channels in an iNdefinite Causal Order Superposition
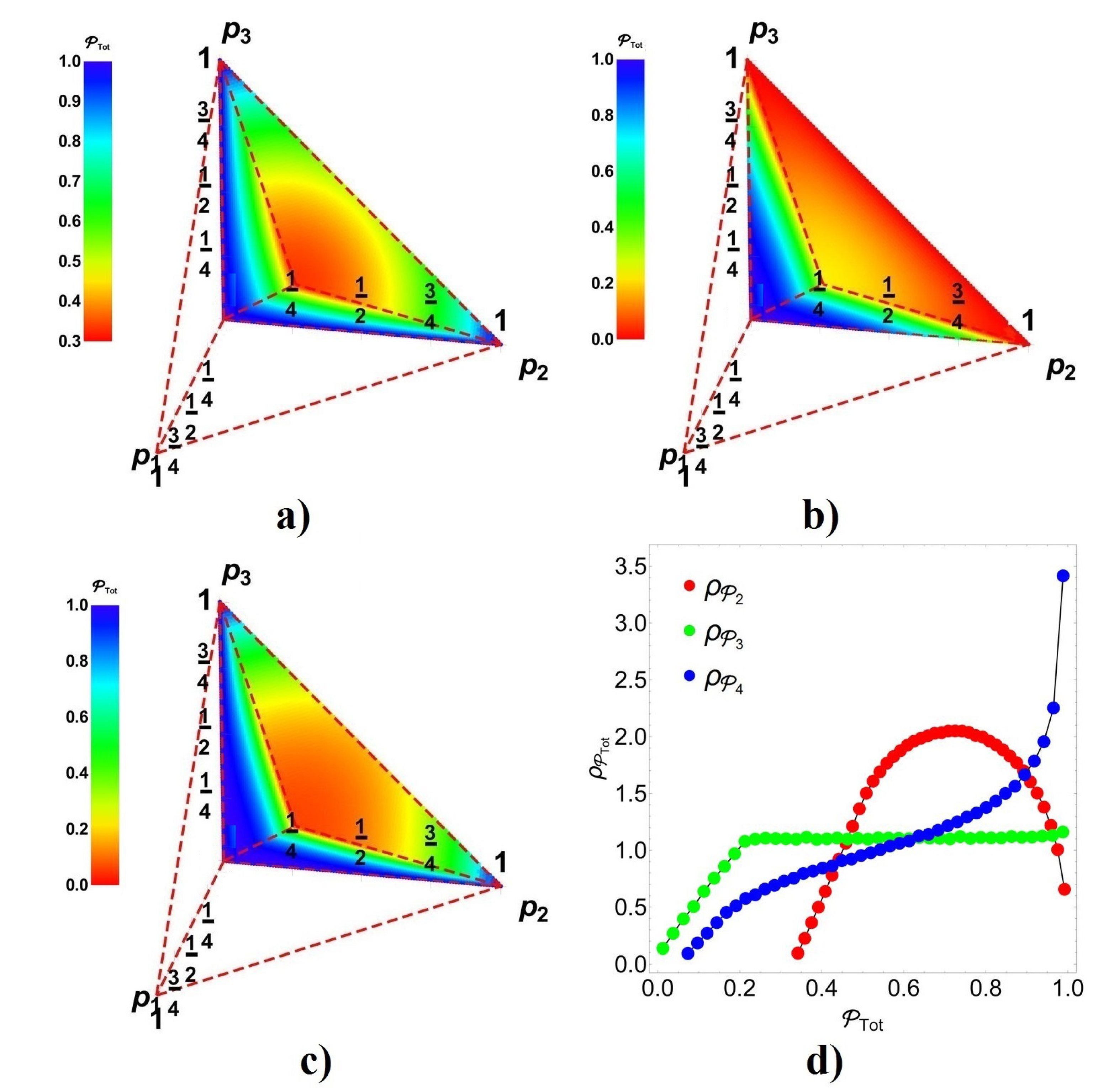
4.2.1. Case
4.2.2. Case ,
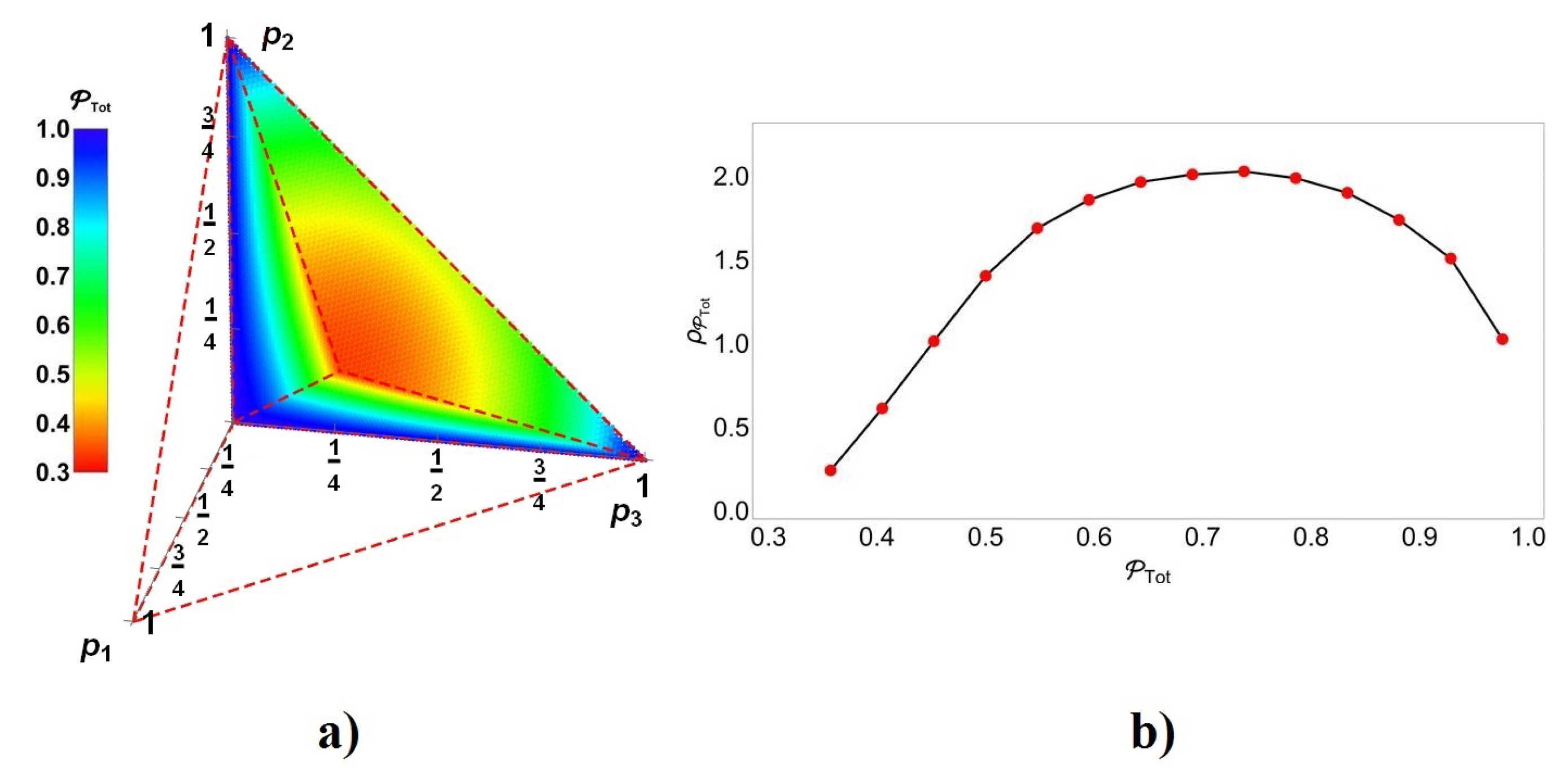
4.3. Notable Behavior on the Frontal Face of Parametric Region: Case
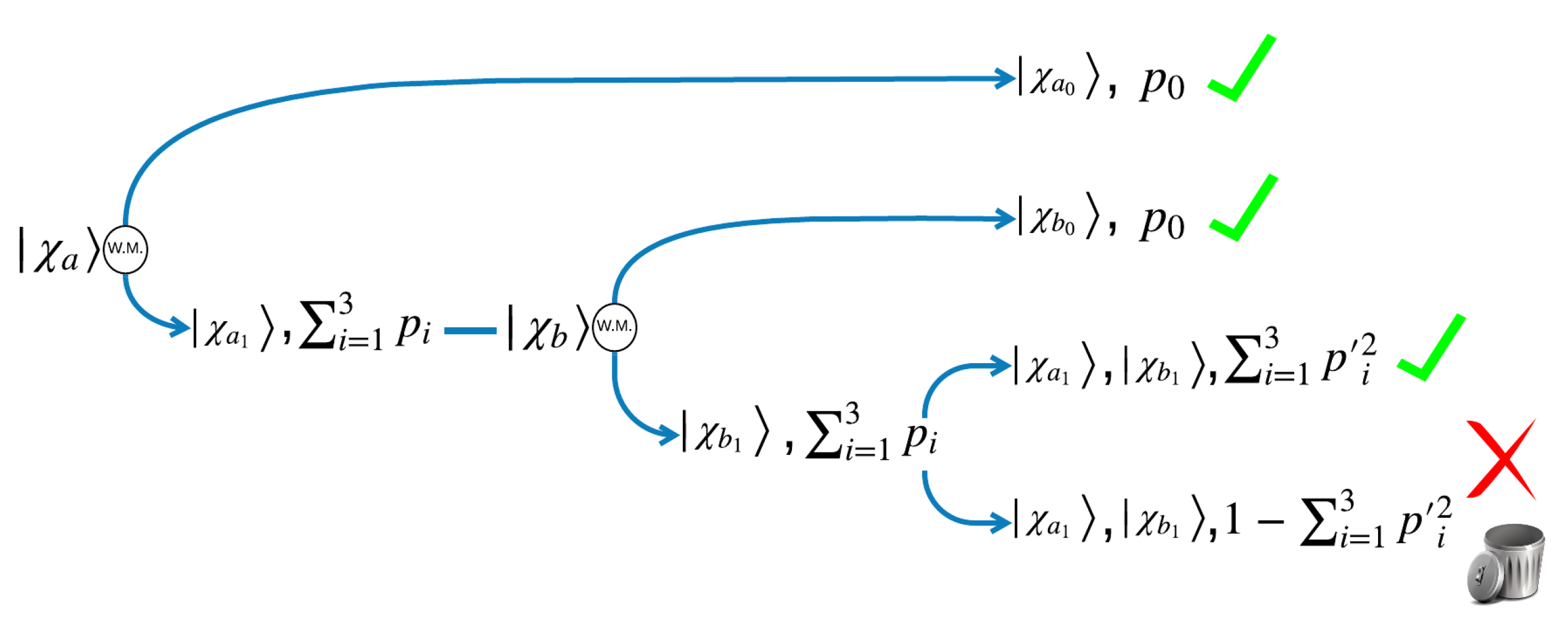
5. An Alternative Procedure Introducing Weak Measurement
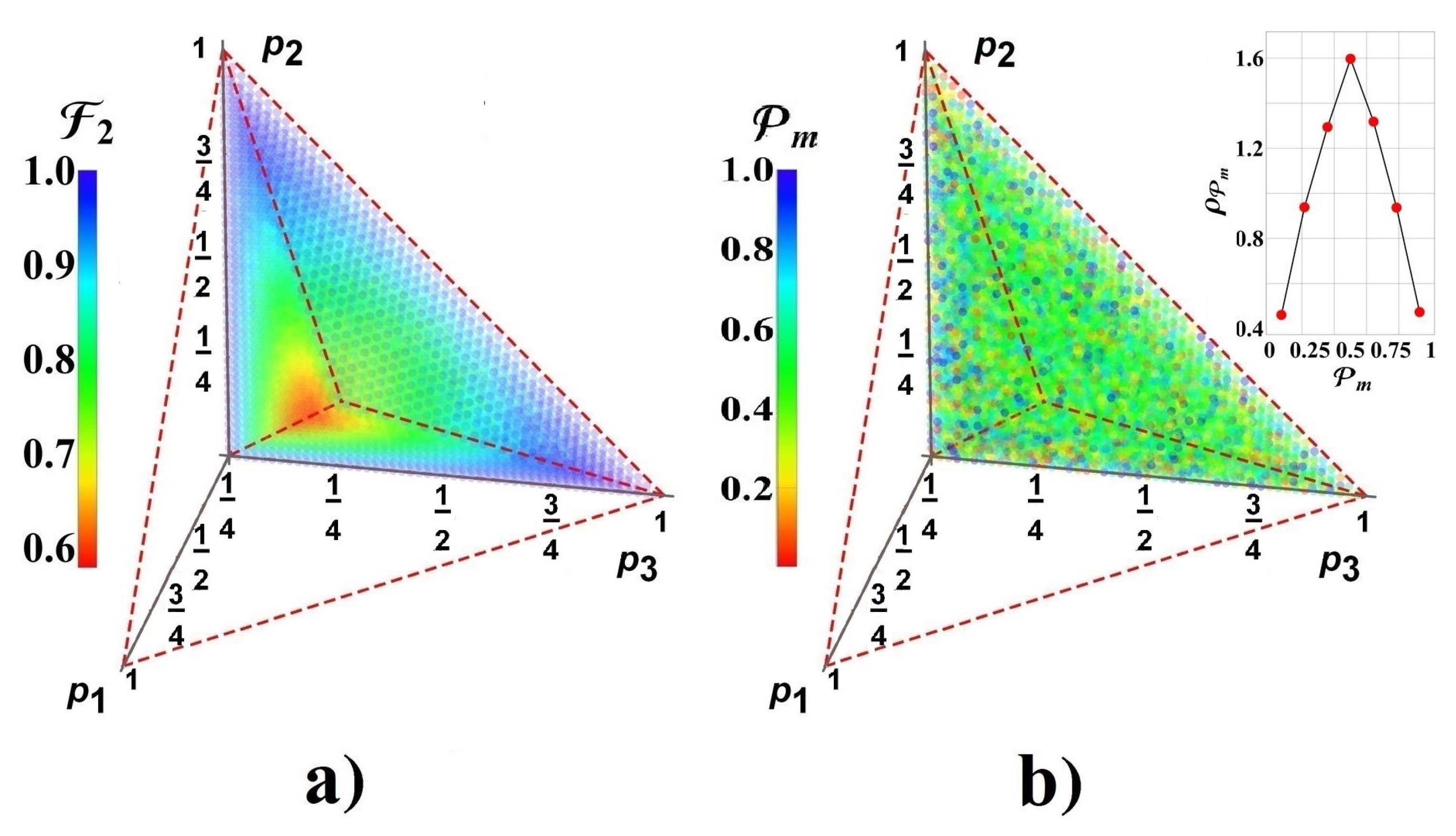
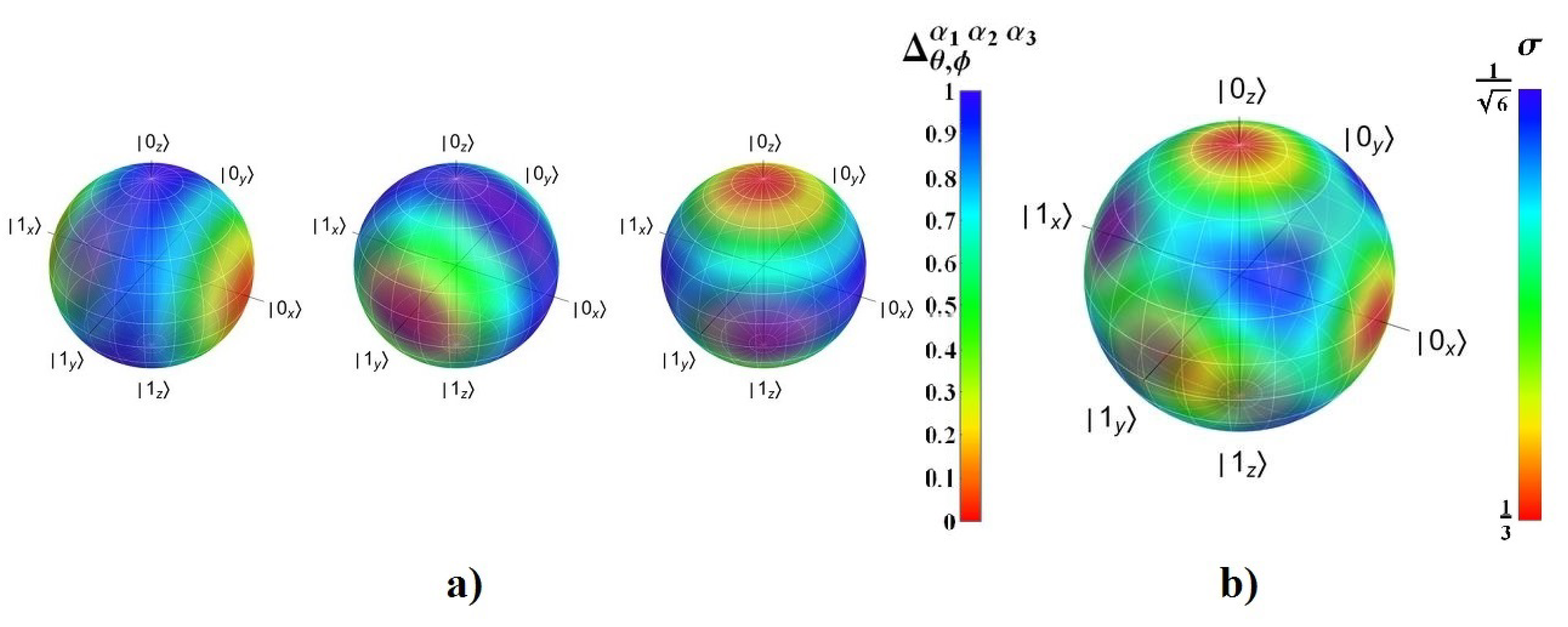
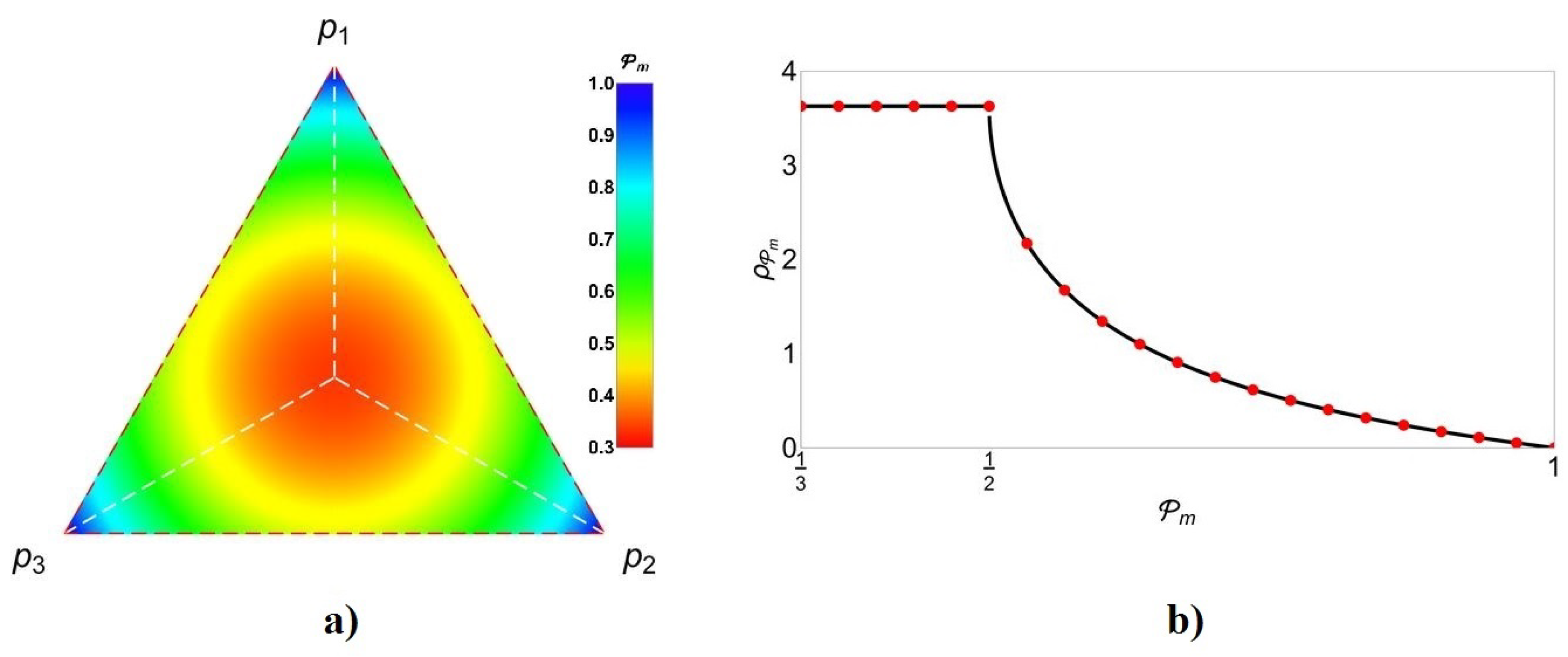
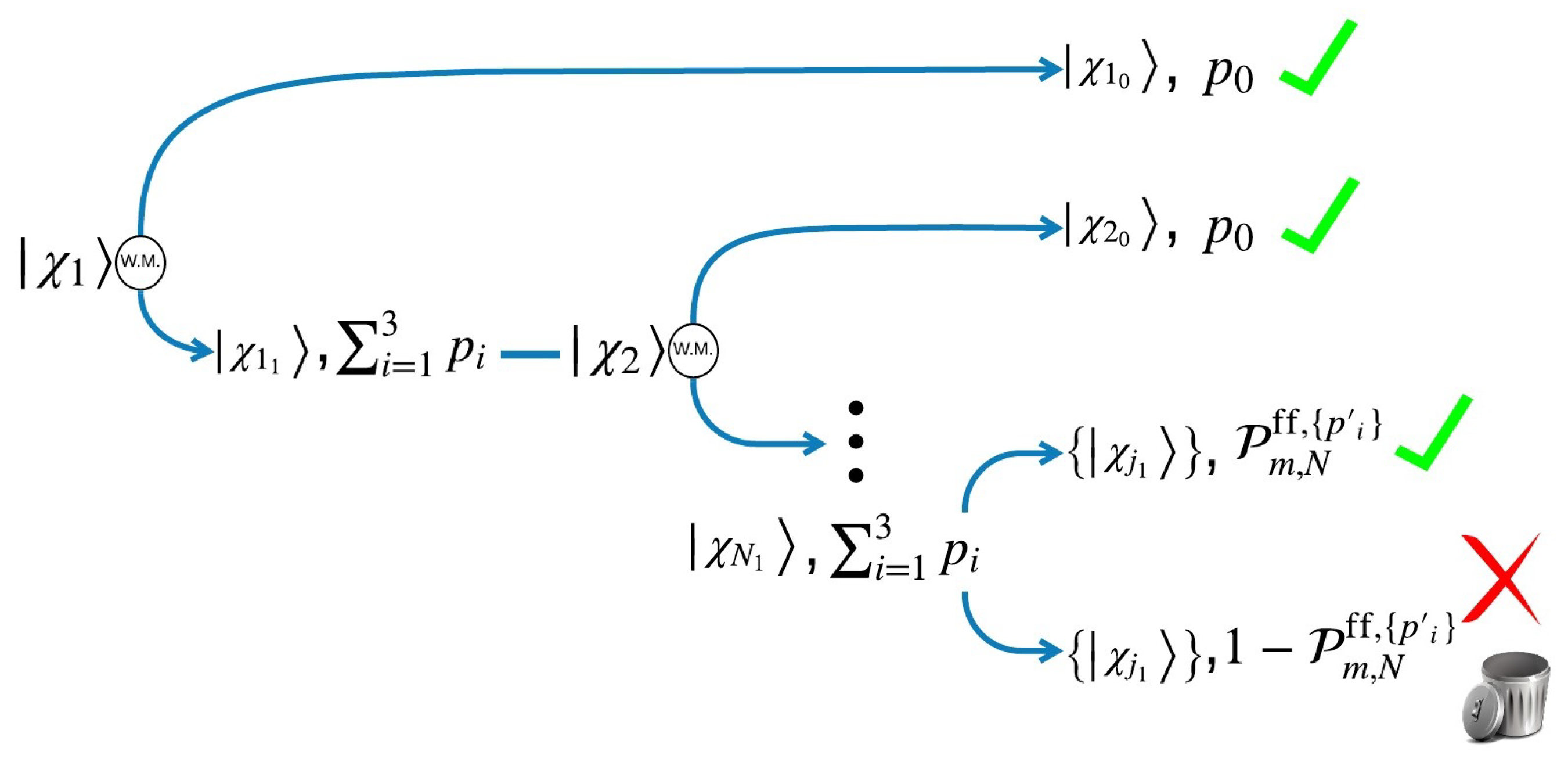
5.1. General Case for Assisted by a Weak Measurement
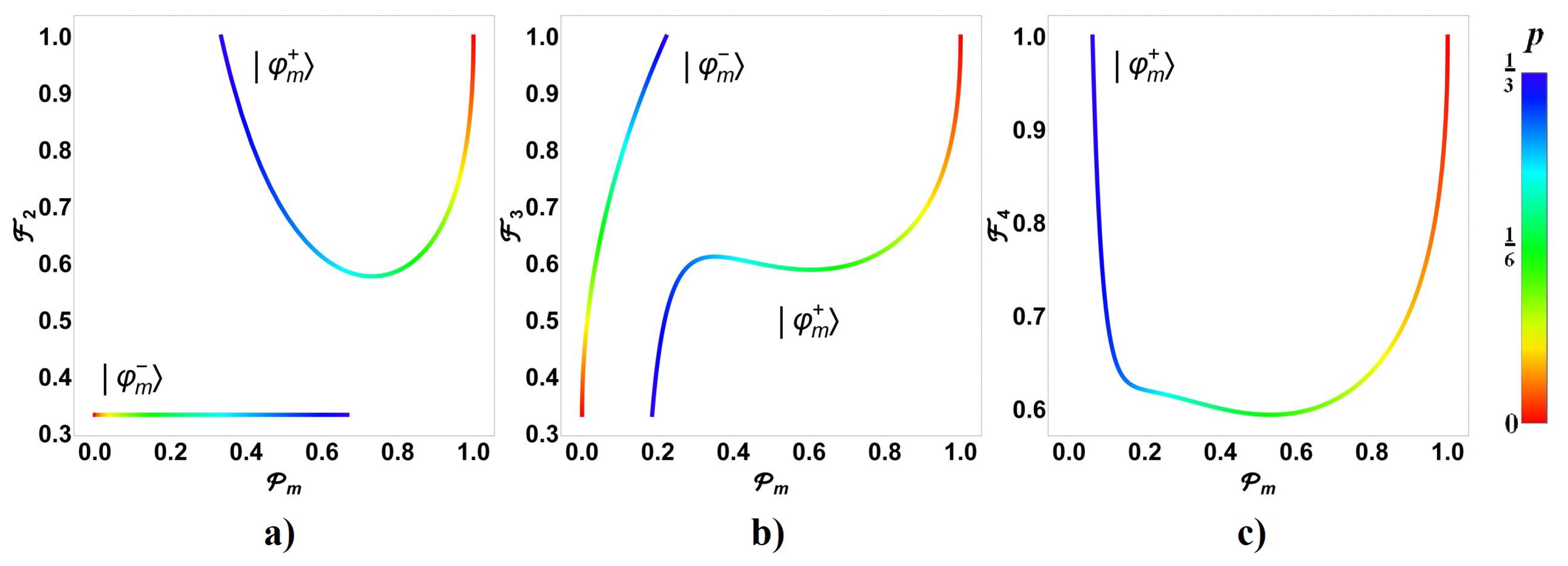
5.2. Cases for Assisted by a Weak Measurement
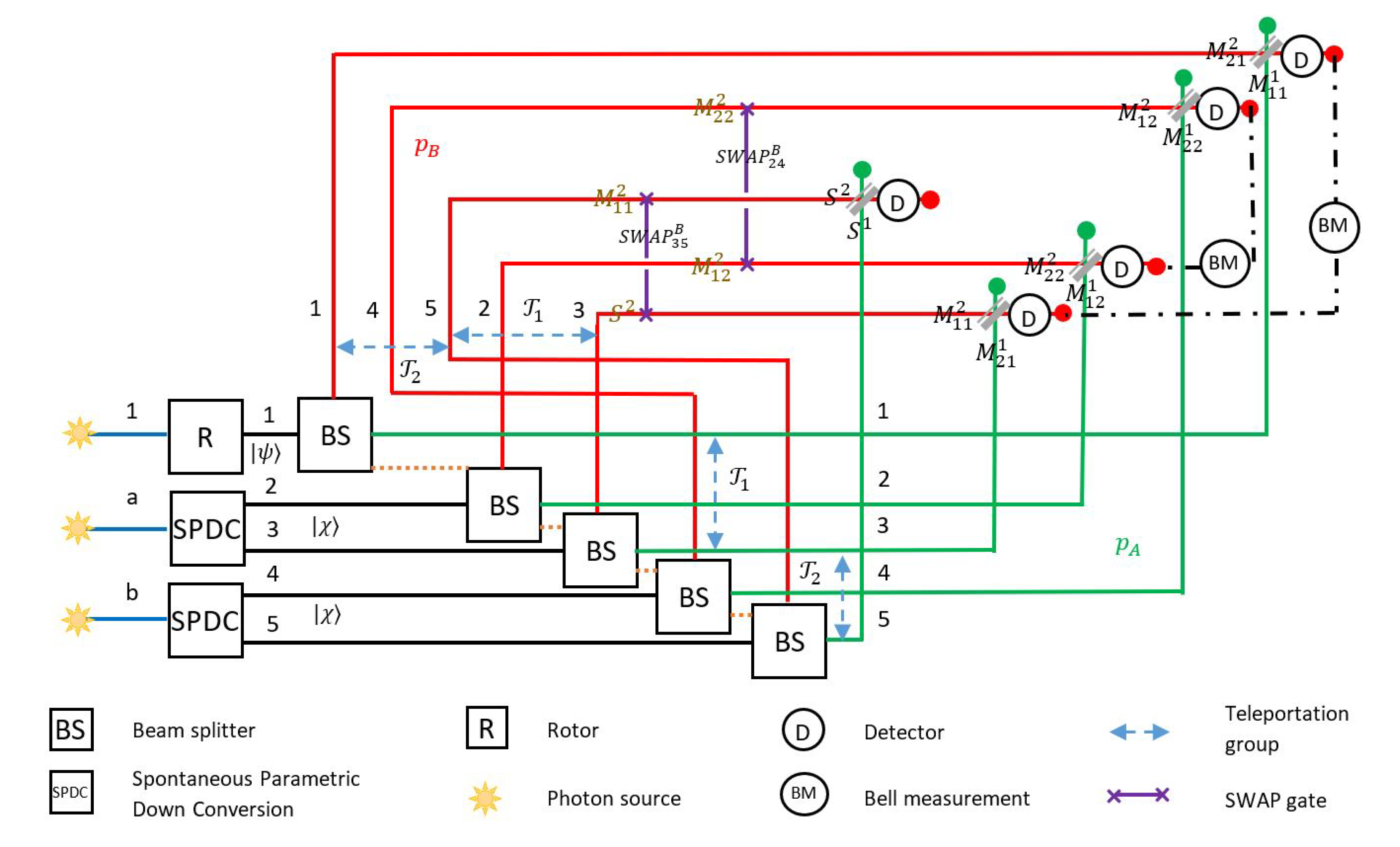
6. Experimental Deployment of Teleportation with Indefinite Causal Order
6.1. Implementation of Weak Measurement to Project
6.2. An Insight View about Teleportation Implementing Indefinite Causal Orders Experimentally with Light
- If for all cases with a global successful probability of .
- If for the cases and with a global successful probability of .
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Formulas for and for the Case
Appendix B. Formulas for and for the Case
References
- Ebler, C.; Salek, S.; Chiribella, G. Enhanced Communication with the Assistance of Indefinite Causal Order. Phys. Rev. Lett. 2017, 120, 120502. [Google Scholar]
- Chiribella, G.; Banik, M.; Bhattacharya, S.S.; Guha, T.; Alimuddin, M.; Roy, A.; Saha, S.; Agrawal, S.; Kar, G. Indefinite causal order enables perfect quantum communication with zero capacity channel. arXiv 2018, arXiv:1810.10457v2. [Google Scholar]
- Goswami, K.; Cao, Y.; Paz-Silva, G.A.; Romero, J.; White, A. Communicating via ignorance. arXiv 2019, arXiv:1807.07383v3. [Google Scholar]
- Procopio, L.M.; Moqanaki, A.; Araújo, M.; Costa, F.; Calafell, I.A.; Dowd, E.G.; Hamel, D.R.; Rozema, L.A.; Brukner, C.; Walther, P. Experimental superposition of orders of quantum gates. Nat. Commun. 2015, 6, 7913. [Google Scholar]
- Procopio, L.M.; Delgado, F.; Enríquez, M.; Belabas, N.; Levenson, J.A. Communication enhancement through quantum coherent control of N channels in an indefinite causal-order scenario. Entropy 2019, 21, 1012. [Google Scholar]
- Procopio, L.M.; Delgado, F.; Enríquez, M.; Belabas, N.; Levenson, J. A Sending classical information via three noisy channels in superposition of causal orders. Phys. Rev. A 2020, 101, 012346. [Google Scholar]
- Mukhopadhyay, C.; Pati, A.K. Superposition of causal order enables perfect quantum teleportation with very noisy singlets. J. Phys. Commun. 2020. [Google Scholar] [CrossRef]
- Delgado, F.; Cardoso, F. Performance characterization of Pauli channels assisted by indefinite causal order and post-measurement. Quantum Inf. Comput. 2020, 20, 1261–1280. [Google Scholar]
- Bennett, C.H.; Wiesner, S.J. Communication via One- and Two-Particle Operators on Einstein-Podolsky-Rosen States. Phys. Rev. Lett. 1992, 69, 2881–2884. [Google Scholar]
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an Unknown Quantum State via Dual Classical and Einstein-Podolsky-Rosen Channels. Phys. Rev. Lett. 1993, 70, 1895. [Google Scholar]
- Delgado, F. Teleportation based on control of anisotropic Ising interaction in three dimensions. JPCS 2015, 624, 012006. [Google Scholar]
- Yan, F.; Yang, L. Economical teleportation of multiparticle quantum state. Il Nuovo C. B 2003, 118, 79. [Google Scholar]
- Metcalf, B.J.; Spring, J.B.; Humphreys, P.C.; Thomas-Peter, N.; Barbieri, M.; Kolthammer, W.S.; Jin, X.-M.; Langford, N.K.; Kundys, F.; Gates, J.C.; et al. Quantum teleportation on a photonic chip. Nat. Photonics 2014, 8, 770. [Google Scholar]
- Nielsen, M.A.; Knill, E.; Laflamme, R. Complete quantum teleportation using nuclear magnetic resonance. Nature 1998, 396, 52–55. [Google Scholar]
- Delgado, F. Teleportation algorithm settled in a resonant cavity using non-local gates. In Quantum Information and Measurement; OSA Technical Digest: Rome, Italy, 2019. [Google Scholar]
- Bouwmeester, D.; Pan, J.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar]
- Ursin, R.; Jennewein, T.; Aspelmeyer, M.; Kaltenbaek, R.; Lindenthal, M.; Walther, P.; Zeilinger, A. Quantum teleportation across the Danube. Nature 2004, 430, 849. [Google Scholar]
- Ma, X.S.; Herbst, T.; Scheidl, T.; Wang, D.; Kropatschek, S.; Naylor, W.; Wittmann, B.; Mech, A.; Kofler, J.; Anisimova, E.; et al. Quantum teleportation over 143 kilometres using active feed-forward. Nature 2012, 489, 269–273. [Google Scholar]
- Luo, Y.H.; Zhong, H.S.; Erhard, M.; Wang, X.L.; Peng, L.C.; Krenn, M.; Jiang, X.; Li, L.; Liu, N.-L.; Lu, C.-Y.; et al. Quantum Teleportation in High Dimensions. Phys. Rev. Lett. 2019, 123, 070505. [Google Scholar]
- Caleffi, M.; Cacciapuoti, A.S. Quantum Switch for the Quantum Internet: Noiseless Communications through Noisy Channels. IEEE J. Sel. Areas Commun. 2020, 38, 575. [Google Scholar]
- Yin, J.; Ren, J.G.; Lu, H.; Cao, Y.; Yong, H.L.; Wu, Y.P.; Liu, C.; Liao, S.K.; Zhou, F.; Jiang, Y.; et al. Quantum teleportation and entanglement distribution over 100-kilometre free-space channels. Nature 2012, 488, 185–188. [Google Scholar]
- Cardoso, C.; Delgado, F. Featuring causal order in teleportation of two quantum teleportation channels. J. Phys. Conf. Ser. 2020, 1540, 012024. [Google Scholar]
- Bowen, G.; Bose, S. Teleportation as a Depolarizing Quantum Channel, Relative Entropy, and Classical Capacity. Phys. Rev. Lett. 2001, 87, 267901. [Google Scholar] [PubMed] [Green Version]
- Petz, D. Quantum Information Theory and Quantum Statistics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Schmidt-Kaler, F.; Häffner, H.; Riebe, M.; Gulde, S.; Lancaster, G.P.; Deuschle, T.; Becher, C.; Roos, C.F.; Eschner, J.; Blatt, R. Realization of the Cirac–Zoller controlled-NOT quantum gate. Nature 2003, 422, 408–411. [Google Scholar] [PubMed]
- Maslov, D. Basic circuit compilation techniques for an ion-trap quantum machine. New J. Phys. 2017, 19, 023035. [Google Scholar]
- Lopes, J.-H.; Soares, W.-C.; Bertúlio de Lima, B.; Caetano, D.P.; Askery, C. Linear optical CNOT gate with orbital angular momentum and polarization. Quantum Inf. Process. 2019, 18, 256. [Google Scholar]
- Sleator, T.; Weinfurter, H. Elementary gates for quantum computation. Phys. Rev. Lett. 1995, 74, 4087. [Google Scholar]
- Monz, T.; Kim, K.; Hänsel, W.; Riebe, M.; Villar, A.-S.; Schindler, P.; Chwalla, M.; Hennrich, M.; Blatt, R. Realization of the Quantum Toffoli Gate with Trapped Ions. Phys. Rev. Lett. 2009, 102, 040501. [Google Scholar]
- Huang, H.L.; Bao, W.S.; Li, T.; Li, F.G.; Fu, X.Q.; Zhang, S.; Zhang, H.L.; Wang, X. Deterministic linear optical quantum Toffoli gate. Phys. Lett. A 2017, 381, 2673–2676. [Google Scholar]
- Boschi, D.; Branca, S.; De Martini, F.; Hardy, L.; Popescu, S. Experimental Realization of Teleporting an Unknown Pure Quantum State via Dual Classical and Einstein-Podolsky-Rosen Channels. Phys. Rev. Lett. 1998, 80, 1121. [Google Scholar]
- Boyd, R. Nonlinear Optics; Academic Press: Rochester, NY, USA, 2018. [Google Scholar]
- Bass, M.; DeCusatis, C.; Enoch, J.; Lakshminarayanan, V.; Li, G.; MacDonald, C.; Mahajan, V.; Van Stryland, E. Handbook of Optics, 3rd ed.; McGraw-Hill Professiona: New York, NY, USA, 2009; Volume 1, pp. 3.46–3.61. [Google Scholar]
- Erhard, M.; Malik, M.; Krenn, M.; Zeilinger, A. Experimental Greenberger–Horne–Zeilinger entanglement beyond qubits. Nat. Photonics 2018, 12, 759–764. [Google Scholar]
- Zhu, M.; Ye, L. Implementation of swap gate and Fredkin gate using linear optical elements. Int. J. Quantum Inf. 2013, 11, 1350031. [Google Scholar]
- Ono, T.; Okamoto, R.; Tanida1, M.; Hofmann, H.; Takeuchi, S. Implementation of a quantum controlled-SWAP gate with photonic circuits. Sci. Rep. 2017, 7, 45353. [Google Scholar] [PubMed]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cardoso-Isidoro, C.; Delgado, F. Symmetries in Teleportation Assisted by N-Channels under Indefinite Causal Order and Post-Measurement. Symmetry 2020, 12, 1904. https://doi.org/10.3390/sym12111904
Cardoso-Isidoro C, Delgado F. Symmetries in Teleportation Assisted by N-Channels under Indefinite Causal Order and Post-Measurement. Symmetry. 2020; 12(11):1904. https://doi.org/10.3390/sym12111904
Chicago/Turabian StyleCardoso-Isidoro, Carlos, and Francisco Delgado. 2020. "Symmetries in Teleportation Assisted by N-Channels under Indefinite Causal Order and Post-Measurement" Symmetry 12, no. 11: 1904. https://doi.org/10.3390/sym12111904
APA StyleCardoso-Isidoro, C., & Delgado, F. (2020). Symmetries in Teleportation Assisted by N-Channels under Indefinite Causal Order and Post-Measurement. Symmetry, 12(11), 1904. https://doi.org/10.3390/sym12111904





