1. Introduction
In three notes presented to the Accademia dei Lincei in 1935 [
1,
2], Francesco G. Tricomi introduced a method for the computation of the Laplace transform of functions that can be developed in a series of Laguerre polynomials. Precisely, he proved the following proposition:
Proposition 1. If the analytic function is regular at infinity and we can find a real number h such that it can be represented with a series of the formthen it is the Laplace transform of the sum of the series of Laguerre polynomialswhich is absolutely and uniformly convergent for . In particular, for
, that is, avoiding the shift that follows from a basic rule of the Laplace transform, Tricomi found the functions pair
:
We recall that the Laguerre polynomials are obtained by letting
in the general formula
where
denotes the rising factorial and, in particular, it results
.
Tricomi says that the correspondence of the above functions was suggested to him by the series expansion of the Bessel function, in terms of Laguerre polynomials:
where
More recently it was observed [
3] that the same Bessel function admits another expansion, making use of a power series, the so-called Laguerre type exponential, namely:
In fact, it turns out that the expansions (
4) and (
6) are equivalent, since:
It is worth noting that the Laguerre-type exponentials were previously considered by Le Roi [
4] and used in [
5,
6] in the framework of generalised Wright functions. Actually they are a particular case of more general special functions previously considered by V. Kiryakova [
7].
As it is possible to transform the expansions in Laguerre polynomials, by using the inversion or the connection coefficients, into different expansions in terms of other polynomial sets, as it is shown in many articles [
8,
9,
10,
11], the above considerations suggest that we extend the Tricomi method in order to find different Laplace function pairs. This is done even on the basis of preceding results in [
12] and the connection of the Laplace transform with orthogonal polynomials, reported in [
13].
In what follows, we first apply the inversion coefficients in order to find the Laplace transform corresponding to a given power series, then we apply the same methodology to the series of orthogonal polynomials.
2. Tricomi LT of Laguerre Series
We use the classical notations. We denote the Laplace transform by
by using for it the symbol
.
and for the Laguerre polynomials of degree
n the symbol
,
Then the Proposition 1, asserts that under the conditions recalled in [
1], assuming:
it results:
3. LT of Power Series
The inversion coefficients of Laguerre polynomials are reported in [
8,
9], in the form:
where
Then we can proclaim the result
Theorem 1. If the analytic function is regular at infinity, decreasing at least as 1/s and such that it can be represented with the power series:then it is the Laplace transform of the power serieswhere the coefficients and are linked by Equation (
14)
, with the in Equation (
12).
Therefore, under the condition (
14), we have found the pair:
4. LT of Orthogonal Polynomial Expansions
Suppose now that a function satisfies the conditions:
The polynomials
can be represented in terms of a different orthogonal polynomials system
by means of the equation
where the connection coefficients
are reported in [
8].
In particular we can choose the basis of Laguerre polynomials
, so that
where
After this transformation, the function is expressed in terms of Laguerre polynomials and the Tricomi method gives the result:
Theorem 2. If the analytic function is regular at infinity, decreasing at least as and such that, for a suitable choice of the parameter h, it can be represented with the power series:then it is the Laplace transform of the power serieswhere the coefficients and are linked by Equation (
21)
, with the , reported in [
8]
, depending on the considered polynomial set . Therefore, under the condition (
21), we have found the pair:
Remark 1. In [8] the inversion and connection coefficients are reported for many other polynomial sets, including the generalized Laguerre polynomials ; however, we could avoid the use of these polynomials since the result stated in this section can be applied even to them. Example 1. As an example, assuming , that is the Hermite polynomials, according to [9], p. 687, it results:that is:and consequently, by Theorem 2, it results:where the connection coefficients are explicitly given above. 5. Numerical Results
In what follows we show some numerical examples of the considered procedure, assuming , since a different choice does not affect the results.
The experiments have highlighted the benefit, in terms of numerical accuracy, arising from a direct numerical implementation rather than the use of automatic routines embedded in the Mathematica© computing system.
5.1. Example: LT of
Let us consider the function:
whose Laplace transform can be trivially evaluated as:
The Laguerre polynomial series approximant of
is given by:
with:
Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
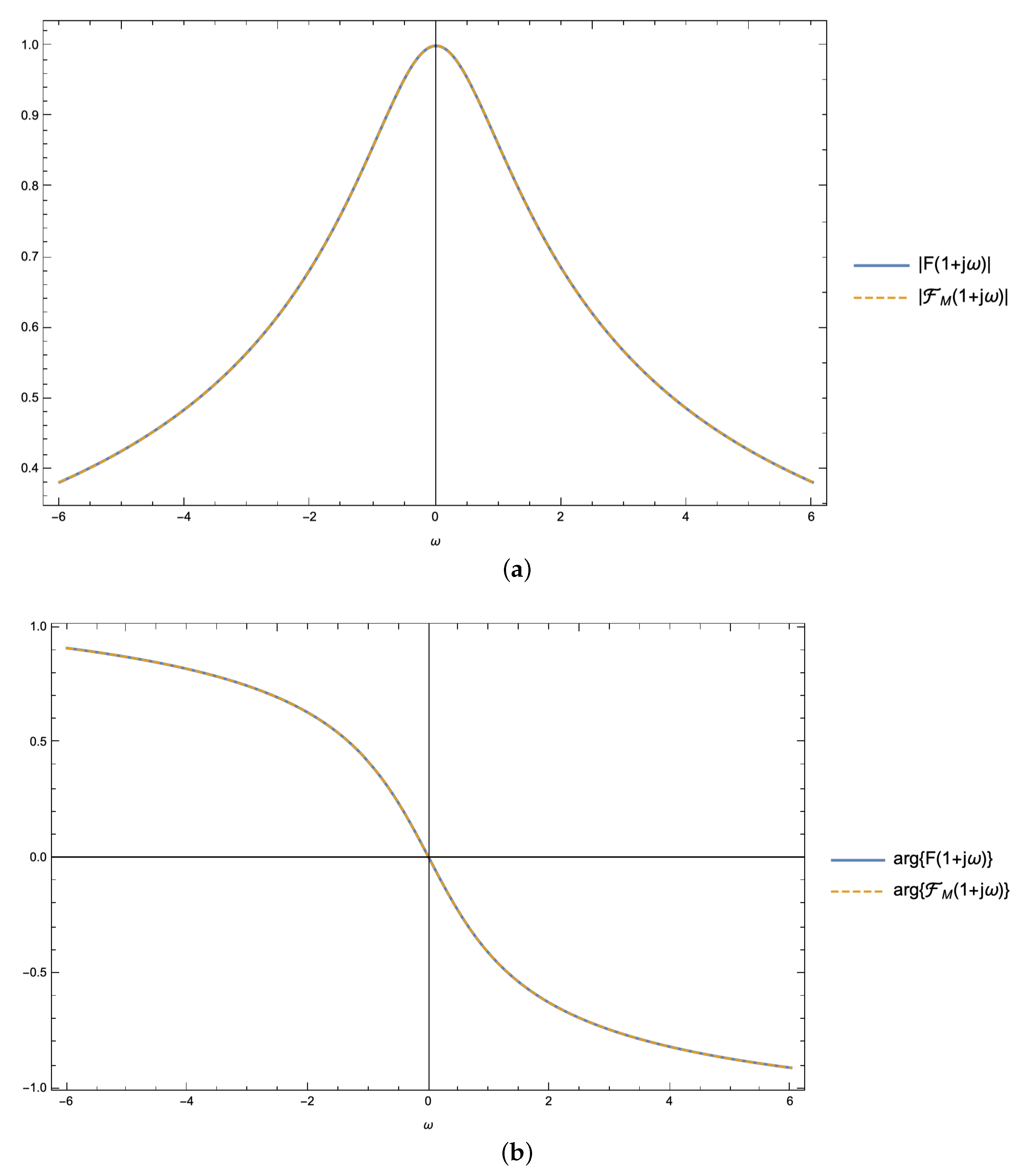
Upon selecting the expansion order
, one can readily verify that the approximant
in (
30) and (
31) is characterized by the distribution shown in
Figure 1, whereas the relevant LT in (
32) shows, along the cut plane
, the behavior plotted in
Figure 2. It can be noticed that the agreement between the exact expression of
in (
29) and its Tricomi’s approximation
is excellent.
5.2. Example: LT of
Let us consider the function:
whose Laplace transform can be evaluated as:
The Laguerre polynomial series approximant of
is given by:
with:
Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
Upon selecting the expansion order
, one can readily verify that the approximant
in (
35) and (
36) is characterized by the distribution shown in
Figure 3, whereas the relevant LT in (
37) shows, along the cut plane
, the behavior plotted in
Figure 4. It can be noticed that the agreement between the exact expression of
in (
34) and its Tricomi’s approximation
is excellent.
5.3. Example: LT of
Let us consider the function:
whose Laplace transform can be evaluated as:
The Laguerre polynomial series approximant of
is given by:
with:
Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
Upon selecting the expansion order
, one can readily verify that the approximant
in (
40) and (
41) is characterized by the distribution shown in
Figure 5, whereas the relevant LT in (
42) shows, along the cut plane
, the behavior plotted in
Figure 6. It can be noticed that the agreement between the exact expression of
in (
39) and its Tricomi’s approximation
is excellent.
5.4. Example: LT of
Let us consider the function:
whose Laplace transform can be trivially evaluated as:
The Maclaurin series approximant of
is given by:
where:
with
denoting the
m-th derivative of
in the origin. In the light of (
11) and (
12), the expansion (
45) can be recast in terms of Laguerre polynomials as follows:
where:
Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
Upon selecting the expansion order
, one can readily verify that the approximant
in (
47) and (
48) is characterized by the distribution shown in
Figure 7, whereas the relevant LT in (
49) shows, along the cut plane
, the behavior plotted in
Figure 8. It can be noticed that the agreement between the exact expression of
in (
44) and its Tricomi’s approximation
is excellent.
5.5. Example: LT of
Let us consider the function:
whose Laplace transform can be easily evaluated as:
The Hermite series approximant of
is given by:
where:
The general polynomial
can be represented in terms of
(
) by means of the equation:
where the connection coefficients
are computed using (
25) or (
26). In this way, the expansion (
52) can be recast as follows:
where:
Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
Upon selecting the expansion order
, one can readily verify that the approximant
in (
55) and (
56) is characterized by the distribution shown in
Figure 9, whereas the relevant LT in (
57) shows, along the cut plane
, the behavior plotted in
Figure 10. It can be noticed that the agreement between the exact expression of
in (
51) and its Tricomi’s approximation
is rather good.
5.6. Example: LT of
Let us consider the function:
whose Laplace transform can be easily evaluated as:
The Hermite series approximant of
is given by:
where:
The expansion (
60) can be recast in terms of Laguerre polynomials as follows:
with:
where the connection coefficients
are computed using (
25) or (
26). Hence, using Tricomi’s method, the Laplace transform
can be approximated as:
Upon selecting the expansion order
, one can readily verify that the approximant
in (
62)–(
63) is characterized by the distribution shown in
Figure 11, whereas the relevant LT in (
64) shows, along the cut plane
, the behavior plotted in
Figure 12. It can be noticed that the agreement between the exact expression of
in (
59) and its Tricomi’s approximation
is rather good.
From the visual inspection of
Figure 12 and
Figure 13, one can readily notice that the derivation of Tricomi’s series approximant directly from the truncated Laguerre expansion of
with a fixed order
M, as per the expression (
3), enables a more accurate representation of the Laplace transform
when compared to the relevant Tricomi’s approximant derived from a truncated Hermite expansion of
with the same order
M.
6. Conclusions
Starting from a classical result by F.G. Tricomi, showing the possibility to use expansions in terms of Laguerre polynomials for obtaining the corresponding Laplace transforms, we have exploited the link between different polynomial sets, via inversion or connection coefficients, in order to apply the Tricomi method to more general expansions.
It has been shown that the described procedure works and that from the numerical point of view, the direct expansion in Laguerre polynomials guarantees a tremendously greater numerical stability of the Tricomi method. This confirms the special role played by Laguerre polynomials in the framework of the Laplace transform, as had been highlighted by the pioneering work of Tricomi.
Author Contributions
P.E.R., D.C., F.M. have contributed equally to this work. All authors have read and agreed to the published version of the manuscript.
Funding
This research received no external funding.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Acknowledgments
The research work of F.M. has been carried out in the framework of the activities of the National Group of Mathematical Physics (GNFM, INdAM), Italy.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Tricomi, F.G. Trasformazione di Laplace e polinomi di Laguerre, I. Inversione della trasformazione; II. Alcune nuove formule sui polinomi di Laguerre. Rend. Lincei 1935, 21, 232–239, 332–335. [Google Scholar]
- Tricomi, F.G. Ancora sull’inversione della trasformazione di Laplace. Rend. Lincei 1935, 21, 420–426. [Google Scholar]
- Dattoli, G.; Ricci, P.E. Laguerre-type exponentials, and the relevant L-circular and L-hyperbolic functions. Georgian Math. J. 2003, 10, 481–494. [Google Scholar] [CrossRef]
- Le Roy, È. Valeurs asymptotiques de certaines séries procédant suivant les puissances entières et positives d’une variable réelle. (French). Darboux Bull. 1899, 4, 245–268. [Google Scholar]
- Garra, R.; Polito, F. On Some Operators Involving Hadamard Derivatives. Integral Transforms Spec. Funct. 2013, 24, 773–782. [Google Scholar] [CrossRef]
- Garrappa, R.; Rogosin, S.; Mainardi, F. On a generalized three-parameter Wright function of Le Roy type. Fract. Calc. Appl. Anal. 2017, 20, 1196–1215. [Google Scholar] [CrossRef]
- Kiryakova, V. Generalized Fractional Calculus and Applications; Pitman Research Notes in Mathematics Series 301; Longman: Harlow, UK, 1994. [Google Scholar]
- Ben Cheikh, Y.; Chaggara, H. Connection coefficients via lowering operators. J. Comput. Appl. Math. 2005, 178, 45–61. [Google Scholar] [CrossRef]
- Ben Cheikh, Y.; Chaggara, H. Connection coefficients between Boas-Buck polynomial sets. J. Math. Anal. Appl. 2006, 319, 665–689. [Google Scholar] [CrossRef]
- Maroni, P.; da Rocha, Z. Connection coefficients between orthogonal polynomials and the canonical sequence: An approach based on symbolic computation. Numer. Algor. 2008, 47, 291–314. [Google Scholar] [CrossRef]
- Ronveaux, A. Orthogonal polynomials: Connection and linearization coefficients. In Proceedings of the International Workshop on Orthogonal Polynomials in Mathematical Physics, Leganés, Spain, 24–26 June 1996; Alfaro, M., Alvarez-Nodarse, R., López Lagomasino, G., Marcellán, F., Eds.; Universidad Carlos III de Madrid: Leganes, Spain, 1997. [Google Scholar]
- Ward, E.E. The calculation of transients in dynamical systems. Camb. Philos. Soc. 1954, 50, 49–54. [Google Scholar] [CrossRef]
- Lee, S.L. Fourier-Laplace transforms and orthogonal polynomials. J. Approx. Theory 2020, 256, 105436. [Google Scholar] [CrossRef]
Figure 1.
Magnitude (a) and argument (b) of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 1.
Magnitude (a) and argument (b) of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 2.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 2.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 3.
Distribution of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 3.
Distribution of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 4.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 4.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 5.
Distribution of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 5.
Distribution of the function as compared to the relevant Laguerre polynomial series approximant of order .
Figure 6.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 6.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the corresponding Tricomi’s series approximant of order .
Figure 7.
Distribution of the function as compared to the relevant Maclaurin series approximant of order .
Figure 7.
Distribution of the function as compared to the relevant Maclaurin series approximant of order .
Figure 8.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Maclaurin expansion of with order .
Figure 8.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Maclaurin expansion of with order .
Figure 9.
Distribution of the function as compared to the relevant Hermite series approximant of order .
Figure 9.
Distribution of the function as compared to the relevant Hermite series approximant of order .
Figure 10.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Hermite expansion of with order .
Figure 10.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Hermite expansion of with order .
Figure 11.
Distribution of the function as compared to the relevant Hermite series approximant of order .
Figure 11.
Distribution of the function as compared to the relevant Hermite series approximant of order .
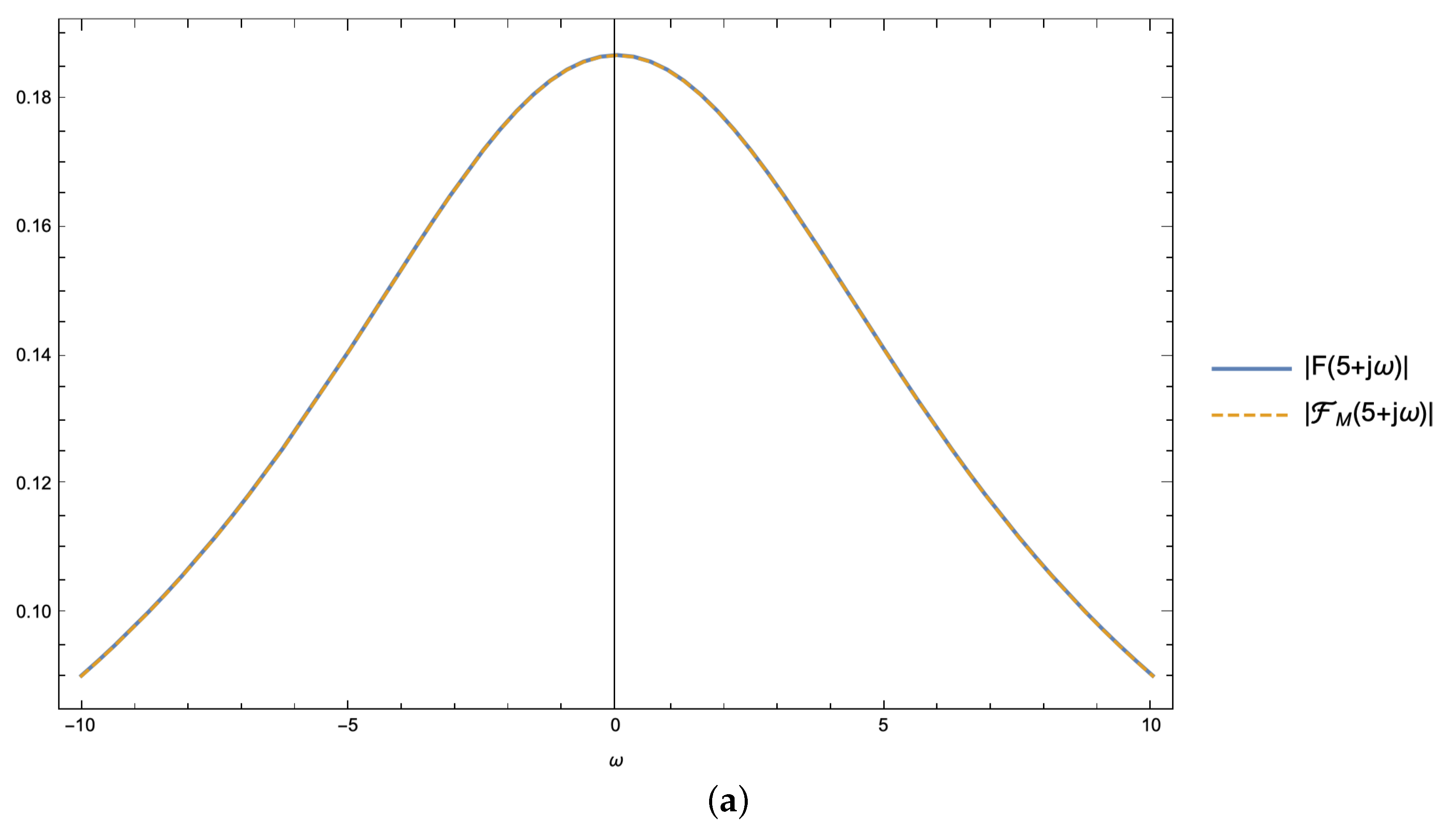
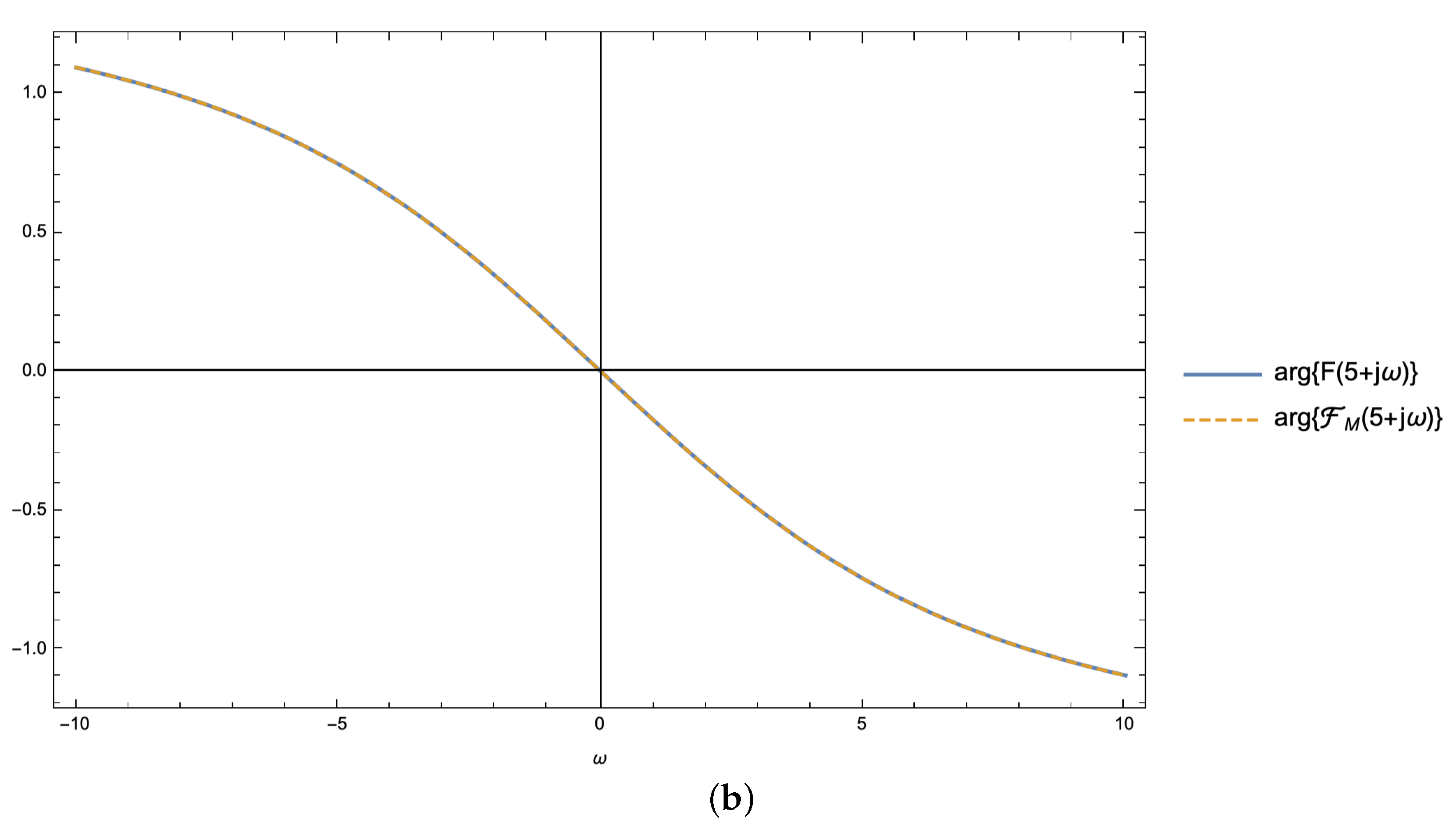
Figure 12.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Hermite expansion of with order .
Figure 12.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived from the truncated Hermite expansion of with order .
Figure 13.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived directly from the truncated Laguerre expansion of with order .
Figure 13.
Magnitude (a) and argument (b) of the Laplace transform relevant to as a function of the complex variable for when computed using the exact integral expression and the Tricomi’s series approximant as derived directly from the truncated Laguerre expansion of with order .
| Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).