First and Second Sound in Two-Dimensional Bosonic and Fermionic Superfluids
Abstract
:1. Introduction
2. Weakly-Interacting 2D Bose Gas
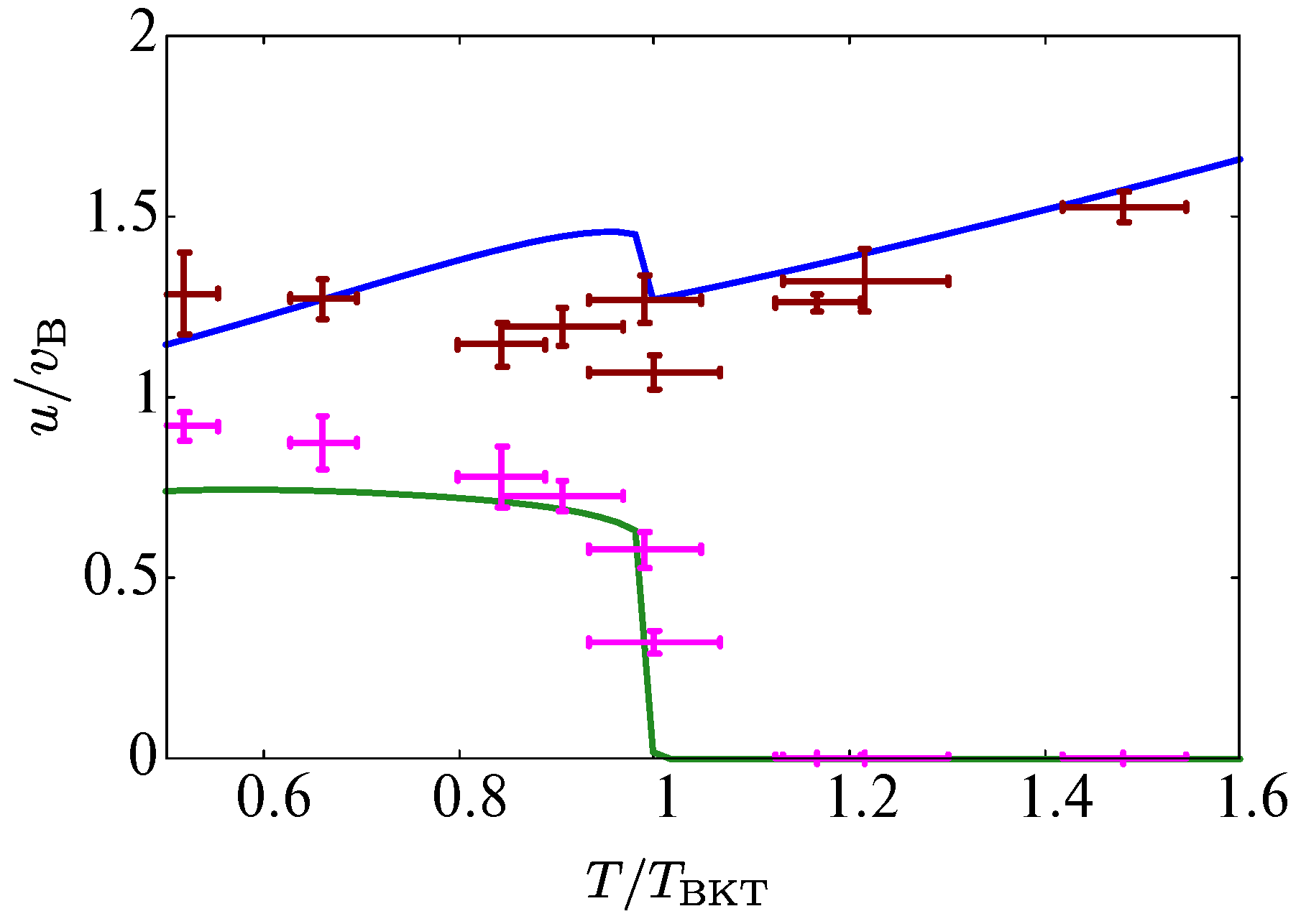
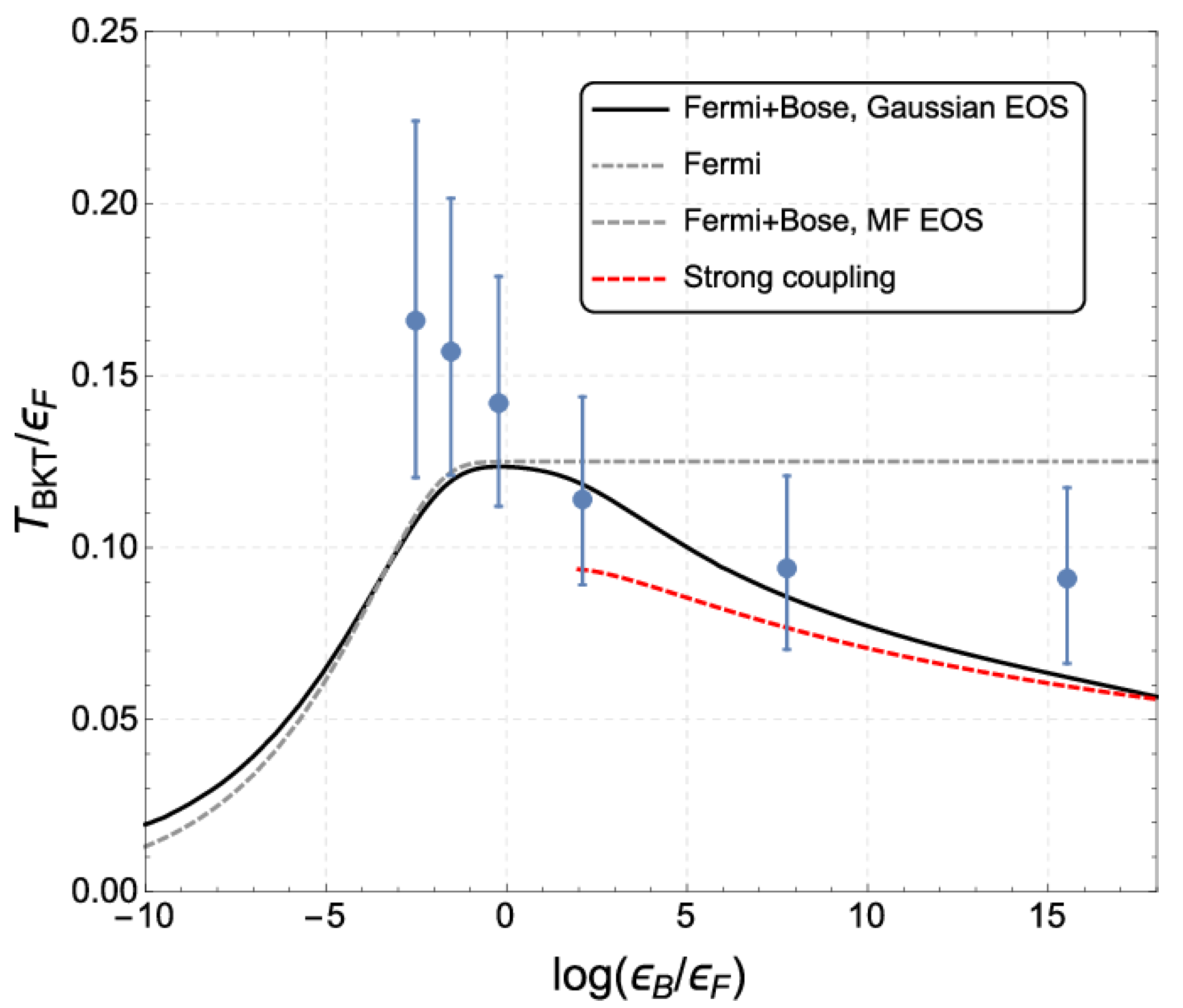
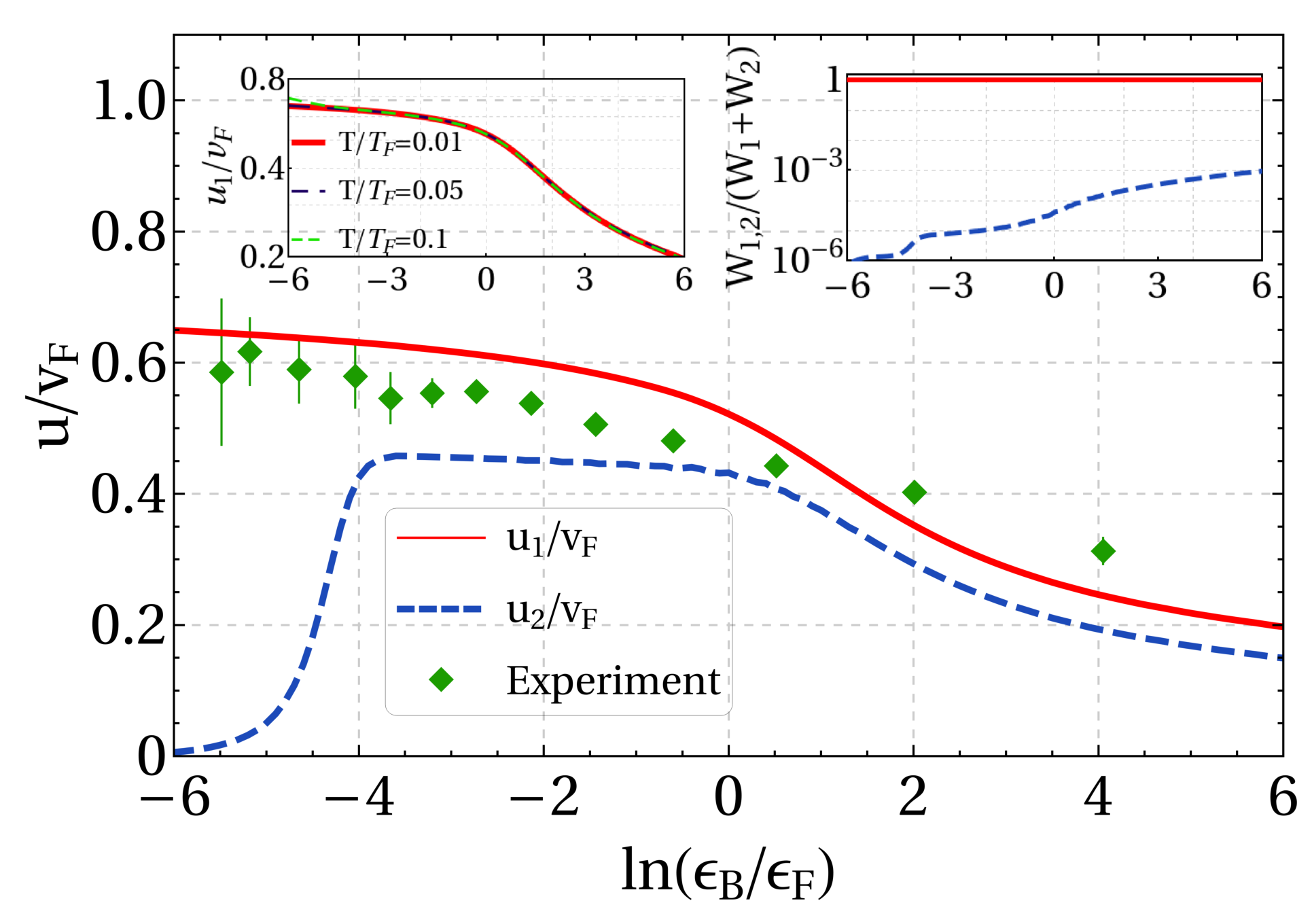
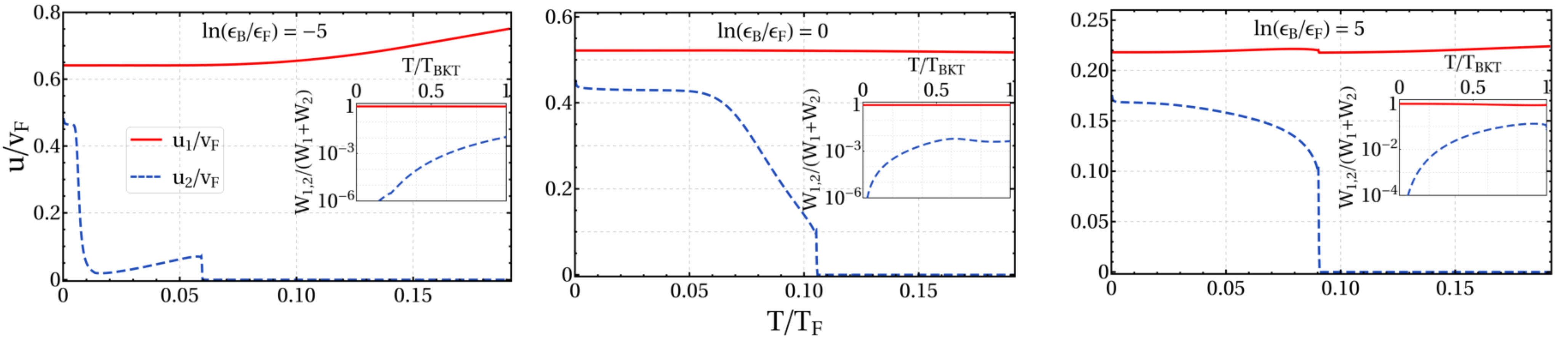
3. 2D Fermi Gas in the BCS-BEC Crossover
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Christodoulou, P.; Galka, M.; Dogra, N.; Lopes, R.; Schmitt, J.; Hadzibabic, Z. Observation of first and second sound in a BKT superfluid. Nature 2021, 594, 191. [Google Scholar] [CrossRef]
- Furutani, K.; Tononi, A.; Salasnich, L. Sound modes in collisional superfluid Bose gases. New J. Phys. 2021, 23, 043043. [Google Scholar] [CrossRef]
- Bohlen, M.; Sobirey, L.; Luick, N.; Biss, H.; Enss, T.; Lompe, T.; Moritz, H. Sound Propagation and Quantum-Limited Damping in a Two-Dimensional Fermi Gas. Phys. Rev. Lett. 2020, 24, 240403. [Google Scholar] [CrossRef] [PubMed]
- Tononi, A.; Cappellaro, A.; Bighin, G.; Salasnich, L. Propagation of first and second sound in a two-dimensional Fermi superfluid. Phys. Rev. A 2021, 103, L061303. [Google Scholar] [CrossRef]
- Landau, L.D. The theory of superfuidity of helium II. J. Phys. (USSR) 1941, 5, 71. [Google Scholar]
- Salasnich, L. Power spectrum and diffusion of the Amari neural field. Symmetry 2019, 11, 134. [Google Scholar] [CrossRef] [Green Version]
- Gross, E.P. Structure of a quantized vortex in boson systems. Nuovo C. 1961, 20, 454. [Google Scholar] [CrossRef] [Green Version]
- Pitaevskii, L.P. Vortex lines in an imperfect Bose gas. Sov. Phys. JETP 1961, 13, 451. [Google Scholar]
- Amari, S. Dynamics of pattern formation in lateral-inhibition type neural fields. Biol. Cyber. 1977, 27, 77–87. [Google Scholar] [CrossRef]
- Salasnich, L.; Toigo, F. Zero-point energy of ultracold atoms. Phys. Rep. 2016, 640, 1. [Google Scholar] [CrossRef] [Green Version]
- Berezinskii, V.L. Destruction of Long-range Order in One-dimensional and Two-dimensional Systems Possessing a Continuous Symmetry Group. II. Quantum Systems. Sov. Phys. JETP 1972, 34, 610. [Google Scholar]
- Kosterlitz, J.M.; Thouless, D.J. Long range order and metastability in two dimensional solids and superfluids. (Application of dislocation theory). J. Phys. C 1972, 5, L124. [Google Scholar] [CrossRef]
- Nelson, D.R.; Kosterlitz, J.M. Universal Jump in the Superfluid Density of Two-Dimensional Superfluids. Phys. Rev. Lett. 1977, 39, 1201. [Google Scholar] [CrossRef]
- Regal, C.A.; Greiner, M.; Jin, D.S. Observation of Resonance Condensation of Fermionic Atom Pairs. Phys. Rev. Lett. 2004, 92, 040403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zwierlein, M.W.; Stan, C.A.; Schunck, C.H.; Raupach, S.M.F.; Kerman, A.J.; Ketterle, W. Condensation of Pairs of Fermionic Atoms near a Feshbach Resonance. Phys. Rev. Lett. 2004, 92, 120403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kinast, J.; Kinast, J.; Hemmer, S.L.; Gehm, M.E.; Turlapov, A.; Thomas, J.E. Evidence for Superfluidity in a Resonantly Interacting Fermi Gas. Phys. Rev. Lett. 2004, 92, 150402. [Google Scholar] [CrossRef] [Green Version]
- Makhalov, V.; Martiyanov, K.; Turlapov, A. Ground-State Pressure of Quasi-2D Fermi and Bose Gases. Phys. Rev. Lett. 2014, 112, 045301. [Google Scholar] [CrossRef]
- Ries, M.G.; Wenz, A.N.; Zurn, G.; Bayha, L.; Boettcher, I.; Kedar, D.; Murthy, P.A.; Neidig, M.; Lompe, T.; Jochim, S. Observation of Pair Condensation in the Quasi-2D BEC-BCS Crossover. Phys. Rev. Lett. 2015, 114, 230401. [Google Scholar] [CrossRef] [Green Version]
- Mora, C.; Castin, Y. Extension of Bogoliubov theory to quasicondensates. Phys. Rev. A 2003, 67, 053615. [Google Scholar] [CrossRef] [Green Version]
- Randeria, M.; Duan, J.-M.; Shieh, L.-Y. Bound states, Cooper pairing, and Bose condensation in two dimensions. Phys. Rev. Lett. 1989, 62, 981. [Google Scholar] [CrossRef]
- Nagaosa, N. Quantum Field Theory in Condensed Matter; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Altland, A.; Simons, B. Condensed Matter Field Theory; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Diener, R.B.; Sensarma, R. Quantum fluctuations in the superfluid state of the BCS-BEC crossover, M. Randeria. Phys. Rev. A 2008, 77, 023626. [Google Scholar] [CrossRef] [Green Version]
- Taylor, E.; Griffin, A.; Fukushima, N.Y. Ohashi, Pairing fluctuations and the superfluid density through the BCS-BEC crossover. Phys. Rev. A 2006, 74, 063626. [Google Scholar] [CrossRef] [Green Version]
- Bighin, G.; Salasnich, L. Finite-temperature quantum fluctuations in two-dimensional Fermi superfluids. Phys. Rev. B 2016, 93, 014519. [Google Scholar] [CrossRef]
- Salasnich, L.; Toigo, F. Composite bosons in the two-dimensional BCS-BEC crossover from Gaussian fluctuations. Phys. Rev. A 2015, 91, 011604(R). [Google Scholar] [CrossRef] [Green Version]
- He, L.; Lu, H.; Cao, G.; Hu, H.; Liu, X.-J. Quantum fluctuations in the BCS-BEC crossover of two-dimensional Fermi gases. Phys. Rev. A 2015, 92, 023620. [Google Scholar] [CrossRef] [Green Version]
- Shi, H.; Chiesa, S.; Zhang, S. Ground-state properties of strongly interacting Fermi gases in two dimensions. Phys. Rev. A 2015, 92, 033603. [Google Scholar] [CrossRef] [Green Version]
- Galea, A.; Dawkins, H.; Gandolfi, S.; Gezerlis, A. Diffusion Monte Carlo study of strongly interacting two-dimensional Fermi gases. Phys. Rev. A 2016, 93, 023602. [Google Scholar] [CrossRef] [Green Version]
- Bighin, G.; Salasnich, L. Renormalization of the superfluid density in the two-dimensional BCS-BEC crossover. Int. J. Mod. Phys. B 2018, 32, 1840022. [Google Scholar] [CrossRef] [Green Version]
- Babaev, E.; Kleinert, H.K. Nonperturbative XY-model approach to strong coupling superconductivity in two and three dimensions. Phys. Rev. B 1999, 59, 12083. [Google Scholar] [CrossRef] [Green Version]
- Benfatto, L.; Toschi, A.; Caprara, S. Low-energy phase-only action in a superconductor: A comparison with the XY model. Phys. Rev. B 2004, 69, 184510. [Google Scholar] [CrossRef] [Green Version]
- Murthy, P.A.; Boettcher, I.; Bayha, L.; Holzmann, M.; Kedar, D.; Neidig, M.; Ries, M.G.; Wenz, A.N.; Zurn, G.; Jochim, S. Observation of the Berezinskii-Kosterlitz-Thouless Phase Transition in an Ultracold Fermi Gas. Phys. Rev. Lett. 2015, 115, 010401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bighin, G.; Salasnich, L. Vortices and antivortices in two-dimensional ultracold Fermi gases. Sci. Rep. 2017, 7, 45702. [Google Scholar] [CrossRef] [PubMed]
- Ozawa, T.; Stringari, S. Discontinuities in the First and Second Sound Velocities at the Berezinskii-Kosterlitz-Thouless Transition. Phys. Rev. Lett. 2014, 112, 025302. [Google Scholar] [CrossRef] [PubMed]
- Bighin, G.; Cappellaro, A.; Salasnich, L. Unitary Fermi superfluid near the critical temperature: Thermodynamics and sound modes from elementary excitations. Phys. Rev. A 2022, 105, 063329. [Google Scholar] [CrossRef]
- Sattin, F.; Salasnich, L. Collisionless sound of bosonic superfluids in lower dimensions. Phys. Rev. A 2021, 103, 043324. [Google Scholar]
- Ville, J.L.; Saint-Jalm, R.; Cerf, E.L.; Aidelsburger, M.; Nascimbene, S.; Dalibard, J.; Beugnon, J. Sound Propagation in a Uniform Superfluid Two-Dimensional Bose Gas. Phys. Rev. Lett. 2018, 121, 145301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ota, M.; Larcher, F.; Dalfovo, F.; Pitaevskii, L.; Proukakis, N.P.; Stringari, S. Collisionless Sound in a Uniform Two-Dimensional Bose Gas. Phys. Rev. Lett. 2018, 121, 145302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cappellaro, A.; Toigo, F.; Salasnich, L. Collisionless dynamics in two-dimensional bosonic gases. Phys. Rev. A 2018, 98, 043605. [Google Scholar] [CrossRef]
- Hu, H.; Yao, X.-C.; Liu, X.-J. Second sound with ultracold atoms: A brief historical account. arXiv 2022, arXiv:2206.05914. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salasnich, L.; Cappellaro, A.; Furutani, K.; Tononi, A.; Bighin, G. First and Second Sound in Two-Dimensional Bosonic and Fermionic Superfluids. Symmetry 2022, 14, 2182. https://doi.org/10.3390/sym14102182
Salasnich L, Cappellaro A, Furutani K, Tononi A, Bighin G. First and Second Sound in Two-Dimensional Bosonic and Fermionic Superfluids. Symmetry. 2022; 14(10):2182. https://doi.org/10.3390/sym14102182
Chicago/Turabian StyleSalasnich, Luca, Alberto Cappellaro, Koichiro Furutani, Andrea Tononi, and Giacomo Bighin. 2022. "First and Second Sound in Two-Dimensional Bosonic and Fermionic Superfluids" Symmetry 14, no. 10: 2182. https://doi.org/10.3390/sym14102182
APA StyleSalasnich, L., Cappellaro, A., Furutani, K., Tononi, A., & Bighin, G. (2022). First and Second Sound in Two-Dimensional Bosonic and Fermionic Superfluids. Symmetry, 14(10), 2182. https://doi.org/10.3390/sym14102182






