Design and Implementation of Simplified Symmetry Chaotic Circuit
Abstract
:1. Introduction
2. Function Analysis of Circuit Module
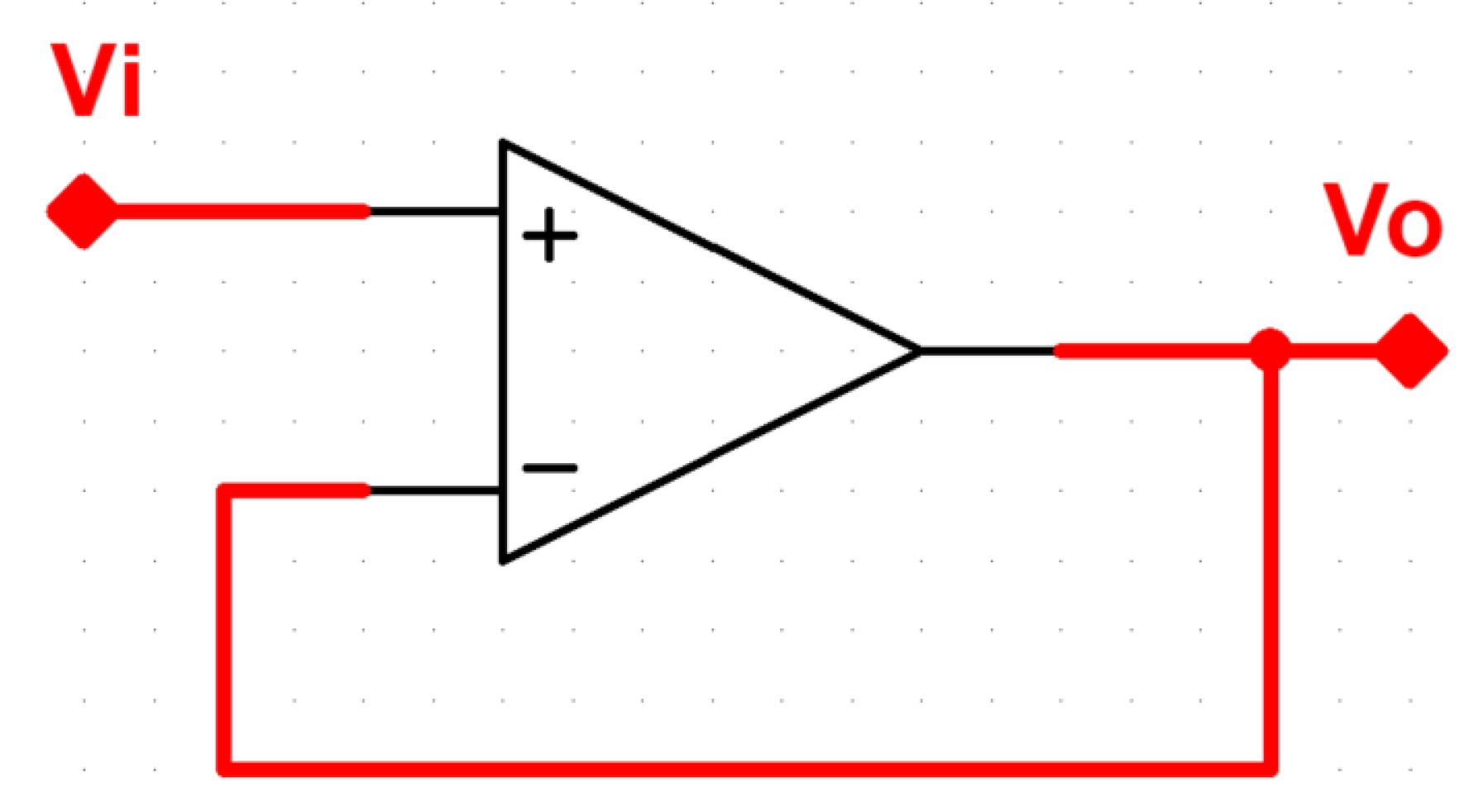
2.1. Function Analysis of the Voltage Follower and Multiplier
2.2. Function Analysis of the Voltage Follower Circuit as Isolators
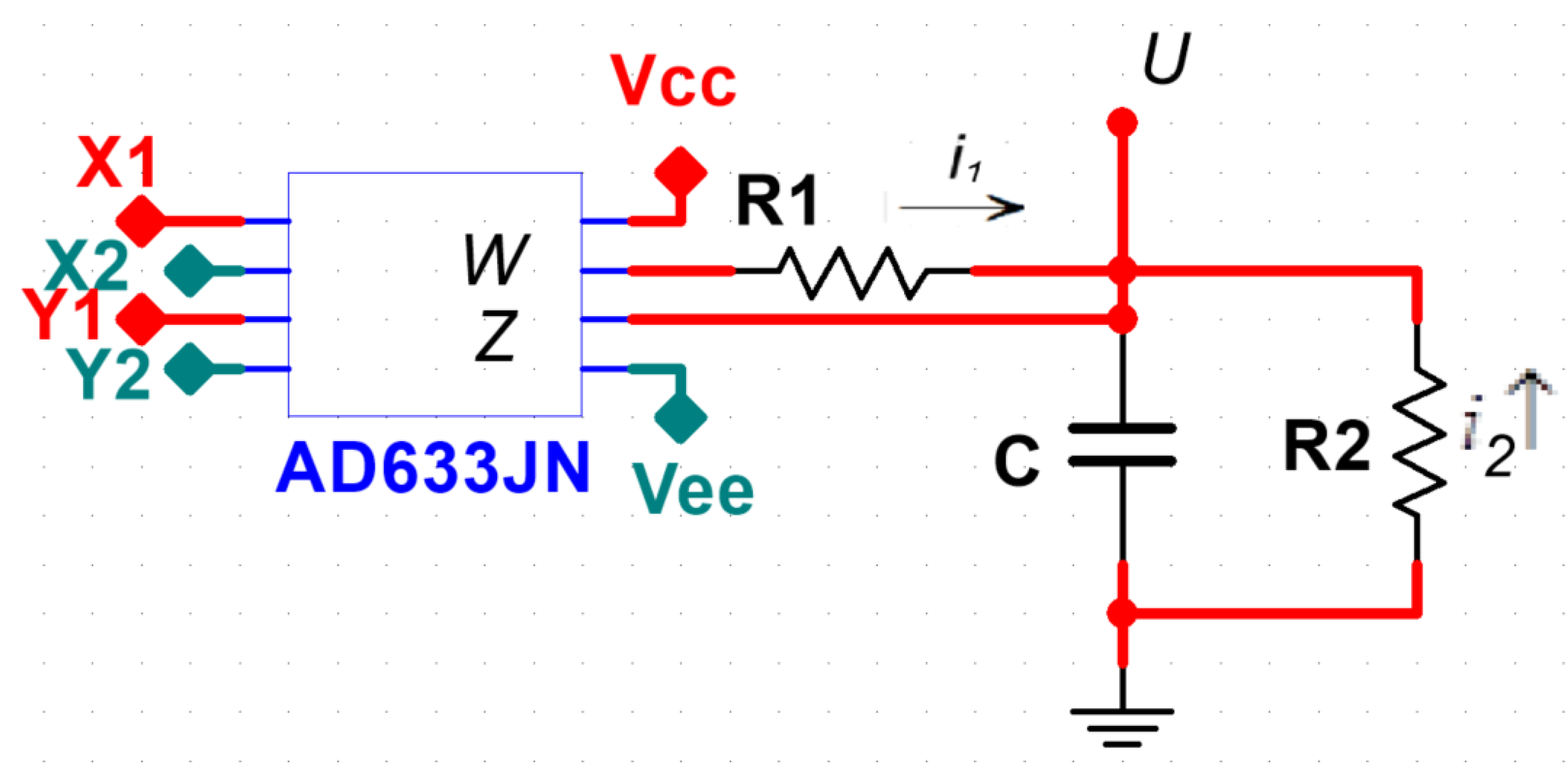
2.3. Function Analysis of Multiplier and Resistance-Capacity Parallel Connection in Series
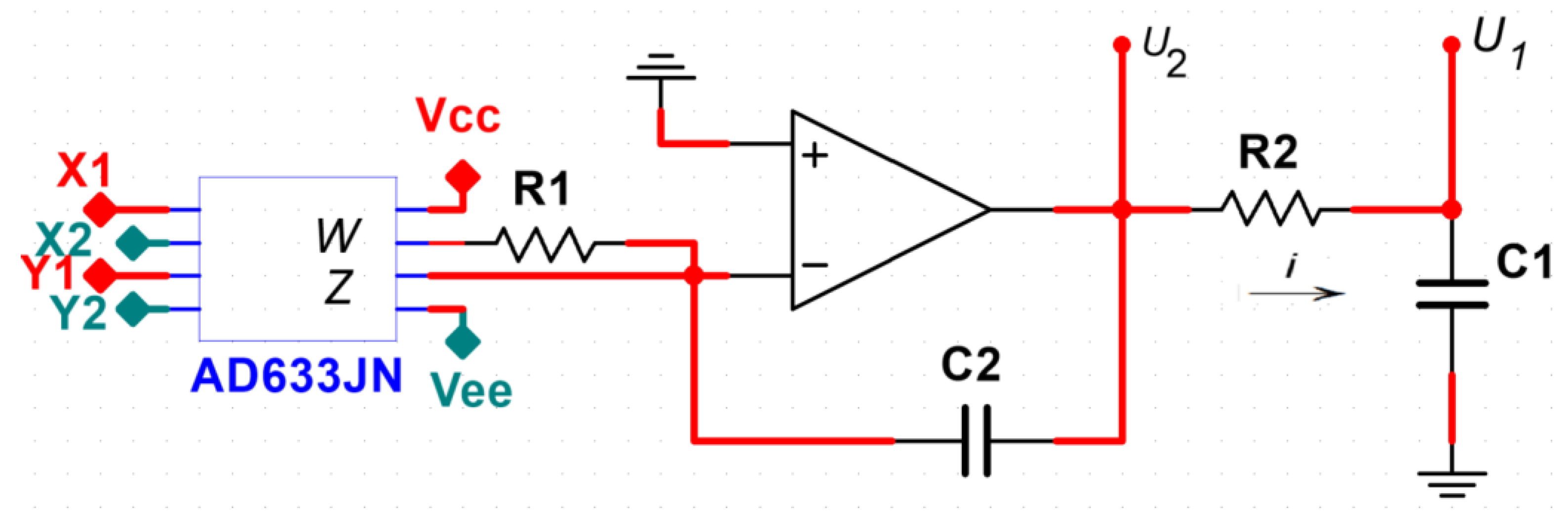
2.4. Function Analysis of the Inverse Integration Circuit as Isolators
3. Bifurcation Analysis of Lyapunov Exponents Spectrum and Bifurcation Diagram
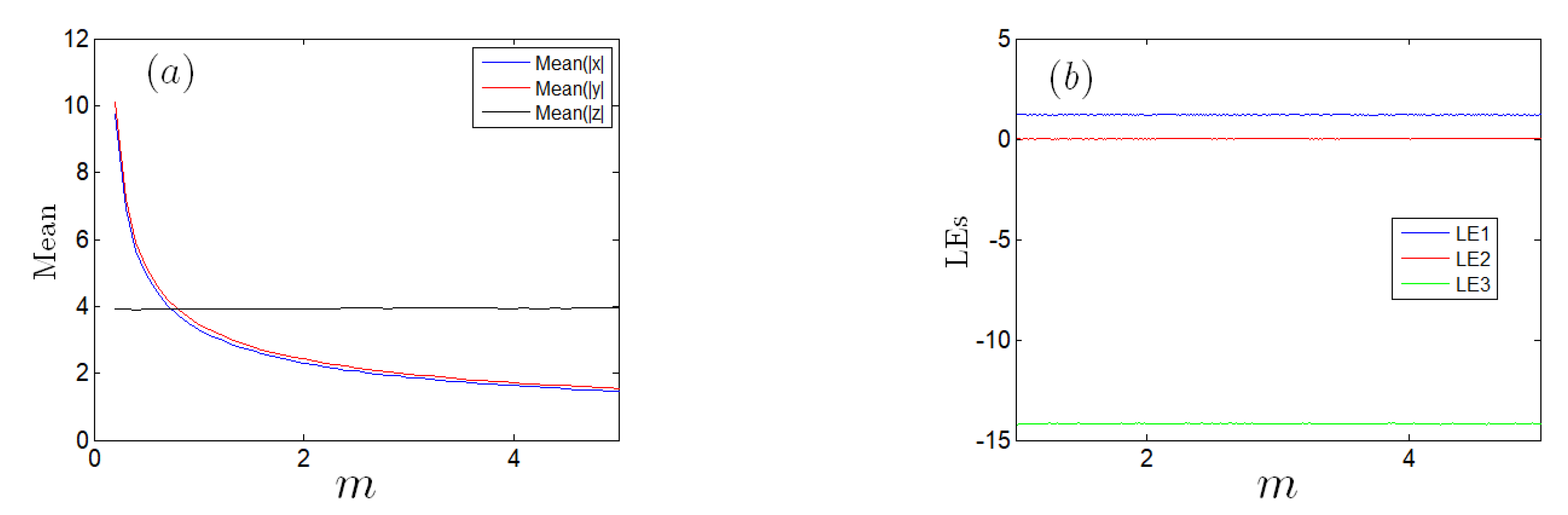
3.1. Fixing and Varying
3.2. Fixing and Varying
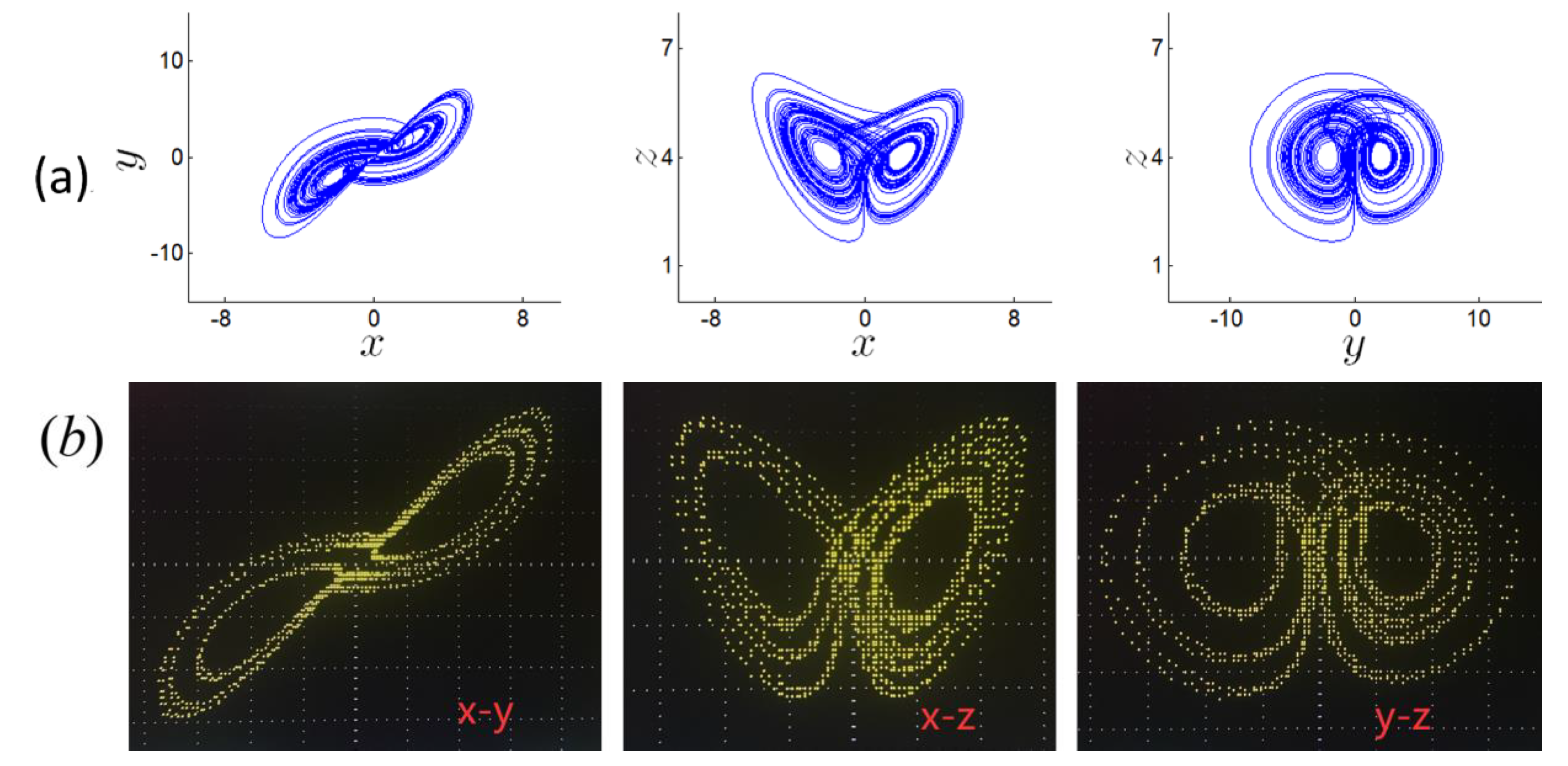
4. Simplified Circuit Realization and Experimental Results
4.1. Simplified Circuit Realization Based on Voltage Follower
4.2. Simplified Circuit Realization Based on Inverse Integration
4.3. The Frequency Behavior of the System (4)
5. Amplitude Control by a Single Parameter
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lorenz, E.N. Deterministic non-perodic flows. Atoms. Sci. 1963, 20, 130. [Google Scholar] [CrossRef]
- Chen, G.; Ueta, T. Yet another chaotic attractor. Int. J. Bifurc. Chaos 1999, 9, 1465–1466. [Google Scholar] [CrossRef]
- Lü, J.; Chen, G. A new chaotic attractor coined. Int. J. Bifurc. Chaos 2002, 12, 659–661. [Google Scholar] [CrossRef] [Green Version]
- Yang, Q.; Chen, G.; Zhou, T. A Unified Lorenz-Type System and its Canonical Form. Int. J. Bifurc. Chaos 2006, 16, 2855–2871. [Google Scholar] [CrossRef]
- Yang, Q.; Chen, G. A Chaotic System with One saddle and Two Stable Node-Foci. Int. J. Bifurc. Chaos 2008, 18, 1393–1414. [Google Scholar] [CrossRef]
- Liu, C.; Liu, T.; Liu, L.; Liu, K. A new chaotic attracator. Chaos Solitons Fractals 2004, 22, 1031–1038. [Google Scholar] [CrossRef]
- Wang, G.-Y.; Qiu, S.-S.; Li, H.-W.; Li, C.-F.; Zheng, Y. A new chaotic system and its circuit realization. Chin. Phys. B 2006, 15, 2872–2877. [Google Scholar]
- Li, C.; Sprott, J.C.; Joo-Chen Thio, W.; Gu, Z. A simple memristive jerk system. IET Circuits Devices Syst. 2020, 15, 383–392. [Google Scholar] [CrossRef]
- Song, Y.; Yuan, F.; Li, Y. Coexisting Attractors and Multistability in a Simple Memristive Wien-Bridge Chaotic Circuit. Entropy 2019, 21, 678. [Google Scholar] [CrossRef] [Green Version]
- Mello, L.F.; Messias, M.; Braga, D.C. Bifurcation analysis of a new Lorenz-like chaotic system. Chaos Solitons Fractals 2008, 37, 1244–1255. [Google Scholar] [CrossRef]
- Valencia-Ponce, M.A.; Castañeda-Aviña, P.R.; Tlelo-Cuautle, E.; Carbajal-Gómez, V.H.; González-Díaz, V.R.; Sandoval-Ibarra, Y.; Nuñez-Perez, J.C. CMOS OTA-based filters for designing fractional-order chaotic oscillators. Fractal Fract. 2021, 5, 122. [Google Scholar] [CrossRef]
- Sambas, A.; Vaidyanathan, S.; Zhang, X.; Koyuncu, I.; Bonny, T.; Tuna, M.; Alçin, M.; Zhang, S.; Sulaiman, I.M.; Awwal, A.M.; et al. A Novel 3D Chaotic System with Line Equilibrium: Multistability, Integral Sliding Mode Control, Electronic Circuit, FPGA Implementation and Its Image Encryption. IEEE Access 2022, 10, 68057–68074. [Google Scholar] [CrossRef]
- Mohamed, S.M.; Sayed, W.S.; Radwan, A.G.; Said, L.A. FPGA Implementation of Reconfigurable CORDIC Algorithm and a Memristive Chaotic System with Transcendental Nonlinearities. IEEE Trans. Circuits Syst. I Regul. Pap. 2022, 69, 2885–2892. [Google Scholar] [CrossRef]
- Yu, W.; Wang, J.; Wang, J.; Zhu, H.; Li, M.; Li, Y.; Jiang, D. Design of a new seven-dimensional hyperchaotic circuit and its application in secure communication. IEEE Access 2019, 7, 125586–125608. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, X.; Gao, M. A Novel Voltage-Controlled Tri-Valued Memristor and Its Application in Chaotic System. Complexity 2020, 2020, 6949703. [Google Scholar] [CrossRef]
- Chang, H.; Li, Y.; Chen, G.; Yuan, F. Extreme Multistability and Complex Dynamics of a Memristor-Based Chaotic System. Int. J. Bifurc. Chaos 2020, 30, 2030019. [Google Scholar] [CrossRef]
- Wang, X.; Jin, C.; Min, X.; Yu, D.; Iu, H.H.C. An exponential chaotic oscillator design and its dynamic analysis. IEEE/CAA J. Autom. Sin. 2020, 7, 1081–1086. [Google Scholar] [CrossRef]
- Xiu, C.; Zhou, R.; Liu, Y. New chaotic memristive cellular neural network and its application in secure communication system. Chaos Solitons Fractals 2020, 141, 110316. [Google Scholar] [CrossRef]
- Li, Y.; Li, C.; Zhang, S.; Chen, G.; Zeng, Z. A Self-Reproduction Hyperchaotic Map with Compound Lattice Dynamics. IEEE Trans. Ind. Electron. 2022, 69, 10564–10572. [Google Scholar] [CrossRef]
- Jia, H.Y.; Chen, Z.Q.; Qi, G.Y. Chaotic Characteristics Analysis and Circuit Implementation for a Fractional-Order System. Circuits Syst. I Regul. Pap. IEEE Trans. 2014, 61, 845–853. [Google Scholar] [CrossRef]
- Li, Y.; Zhao, M.; Geng, F. Dynamical Analysis and Simulation of a New Lorenz-Like Chaotic System. Math. Probl. Eng. 2021, 2021, 6669956. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C. Variable-boostable chaotic flows. Optik 2016, 127, 10389–10398. [Google Scholar] [CrossRef]
- Tsafack, N.; Kengne, J.; Abd-El-Atty, B.; Iliyasu, A.M.; Hirota, K.; Abd EL-Latif, A.A. Design and implementation of a simple dynamical 4-D chaotic circuit with applications in image encryption. Inf. Sci. 2020, 515, 191–217. [Google Scholar] [CrossRef]
- Blakely, J.N.; Eskridge, M.B.; Corron, N.J. A simple Lorenz circuit and its radio frequency implementation. Chaos 2007, 17, 023112. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Li, C.; Ma, X.; Lei, T.; Chen, G. Simplification of Chaotic Circuits with Quadratic Nonlinearity. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 1837–1841. [Google Scholar] [CrossRef]
- Jiang, Y.; Li, C.; Liu, Z.; Lei, T.; Chen, G. Simplified Memristive Lorenz Oscillator. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 3344–3348. [Google Scholar] [CrossRef]





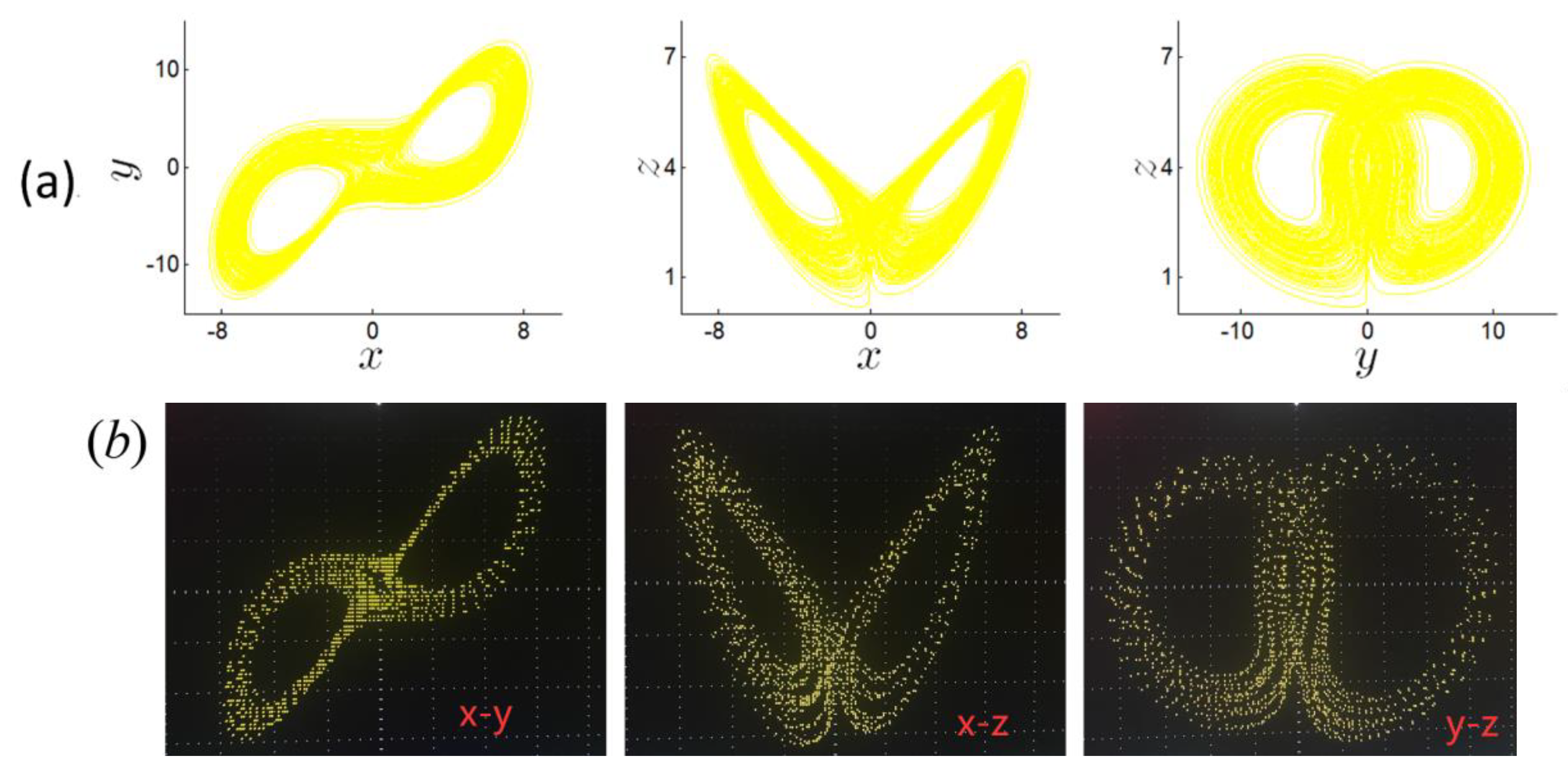
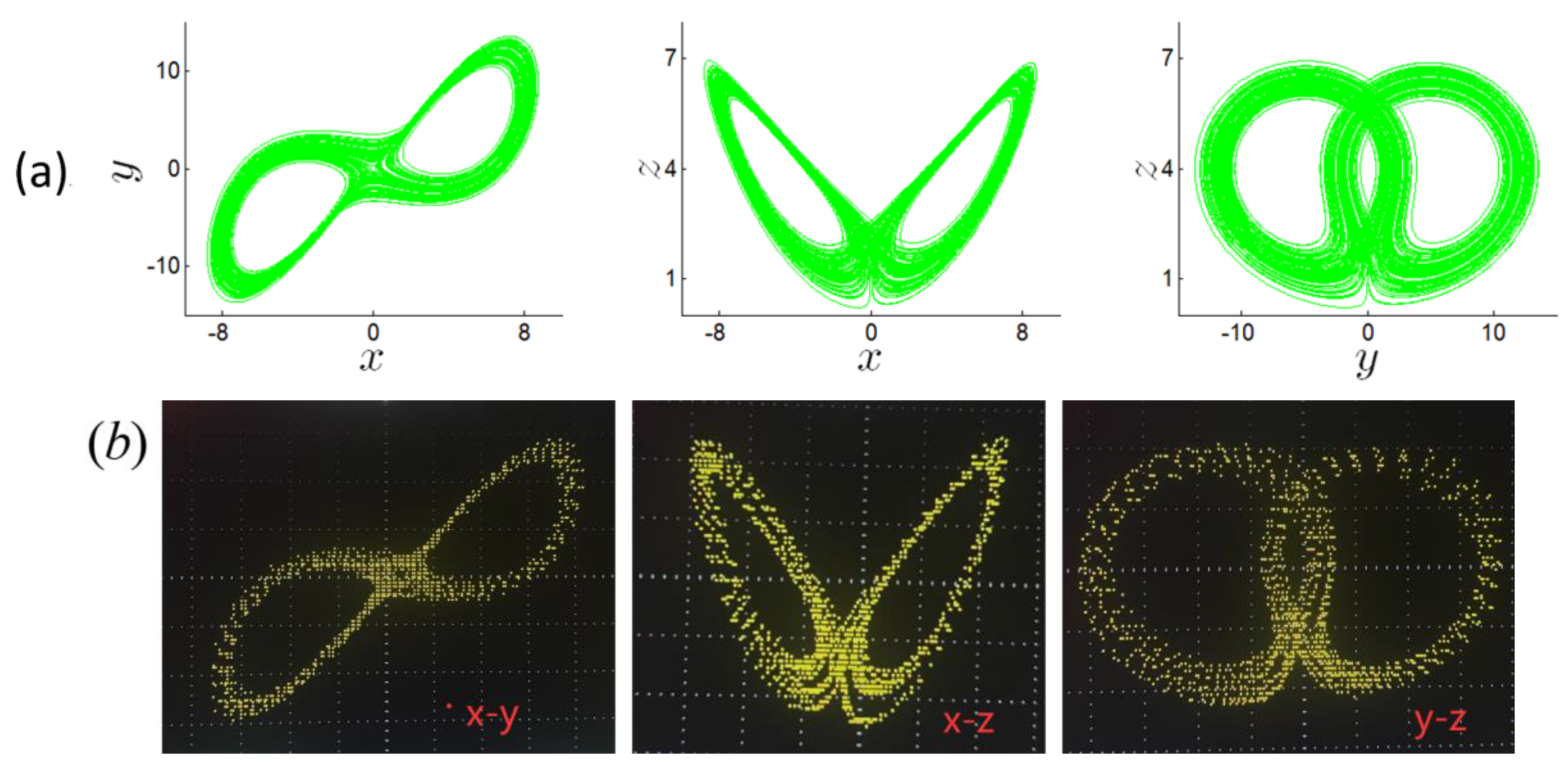



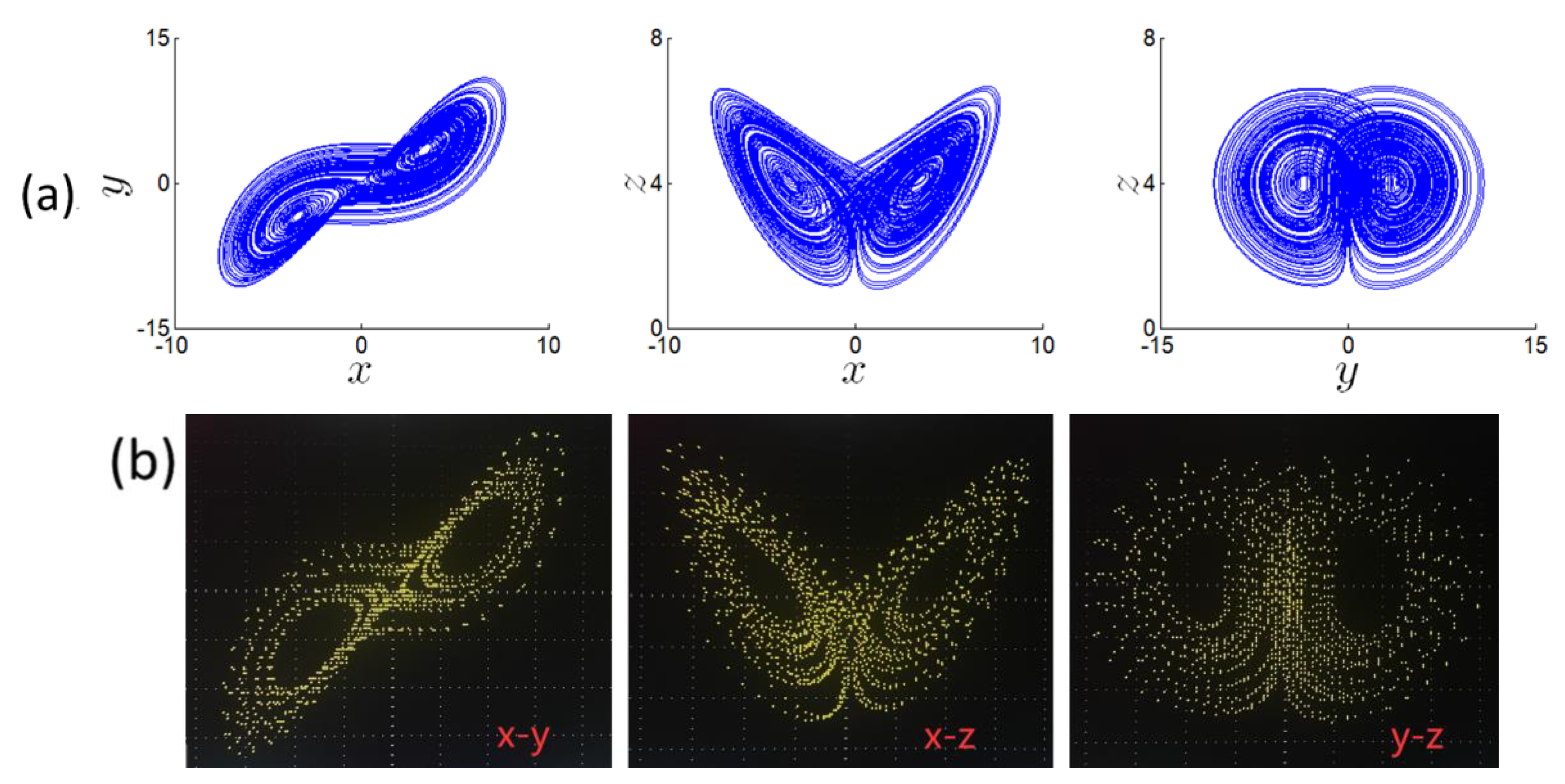




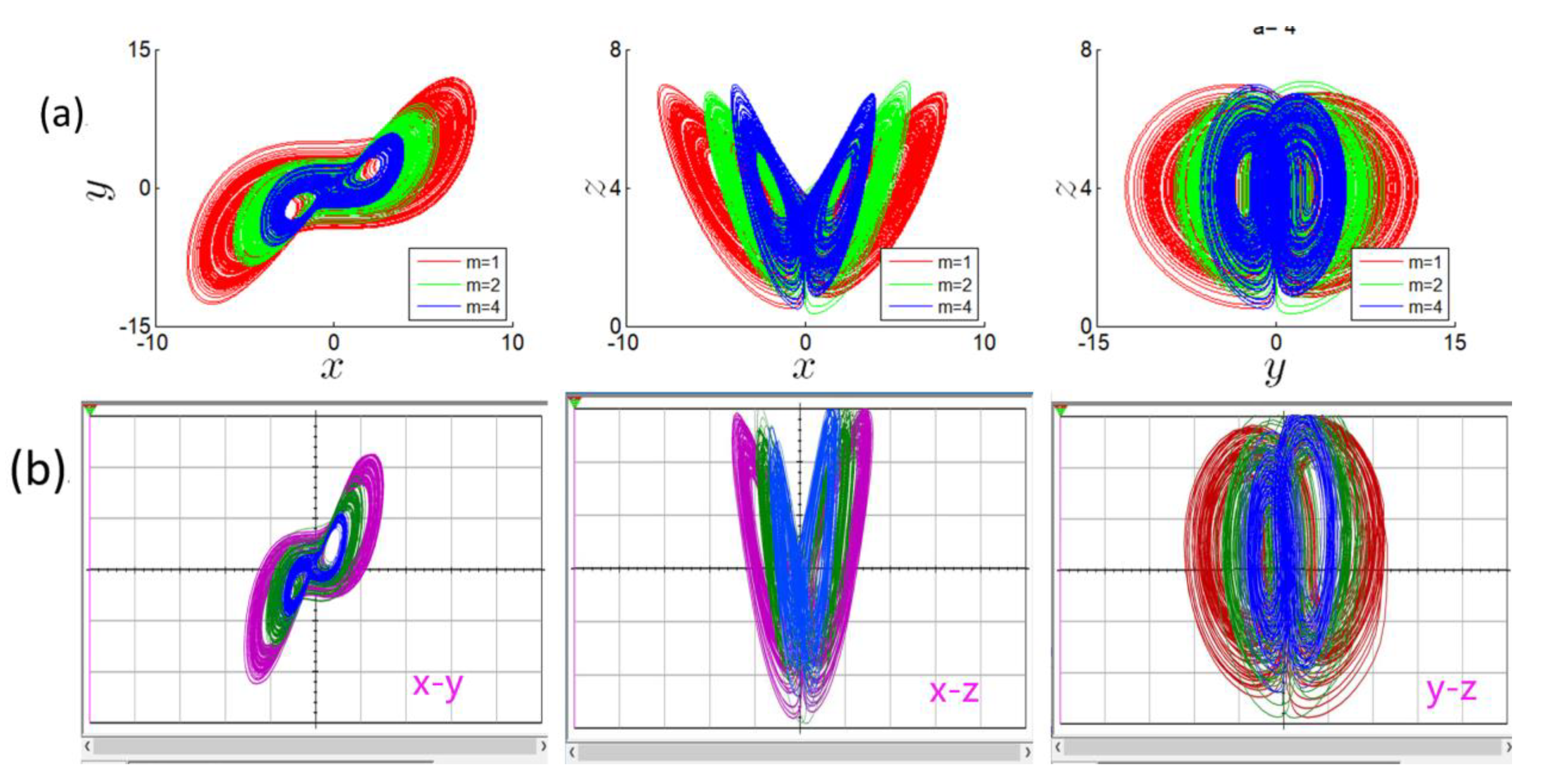
| Attractor | Lyapunov Exponents | |
|---|---|---|
| 1 | chaotic | (0.853348,0,−11.781354) |
| 2 | chaotic | (1.173480,0,−13.136391) |
| 3.5 | chaotic | (1.233097,0,−14.707076) |
| 4 | chaotic | (1.24612,0,−15.218009) |
| 5 | chaotic | (1.252662,0,−16.225784) |
| 6 | chaotic | (1.282429,0,−17.261460) |
| Attractor | Lyapunov Exponents | |
|---|---|---|
| 3.75 | chaotic | (0.383651,0,−7.128153) |
| 7.5 | chaotic | (0.935087,0,−11.4205181) |
| 12 | chaotic | (1.294339,0,−16.245770) |
| 15 | chaotic | (1.297606,0,−19.201739) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Liu, S. Design and Implementation of Simplified Symmetry Chaotic Circuit. Symmetry 2022, 14, 2299. https://doi.org/10.3390/sym14112299
Wang Z, Liu S. Design and Implementation of Simplified Symmetry Chaotic Circuit. Symmetry. 2022; 14(11):2299. https://doi.org/10.3390/sym14112299
Chicago/Turabian StyleWang, Zhonglin, and Shutang Liu. 2022. "Design and Implementation of Simplified Symmetry Chaotic Circuit" Symmetry 14, no. 11: 2299. https://doi.org/10.3390/sym14112299






