Stabilization of Axisymmetric Airy Beams by Means of Diffraction and Nonlinearity Management in Two-Dimensional Fractional Nonlinear Schrödinger Equations
Abstract
:1. Introduction
2. The Model
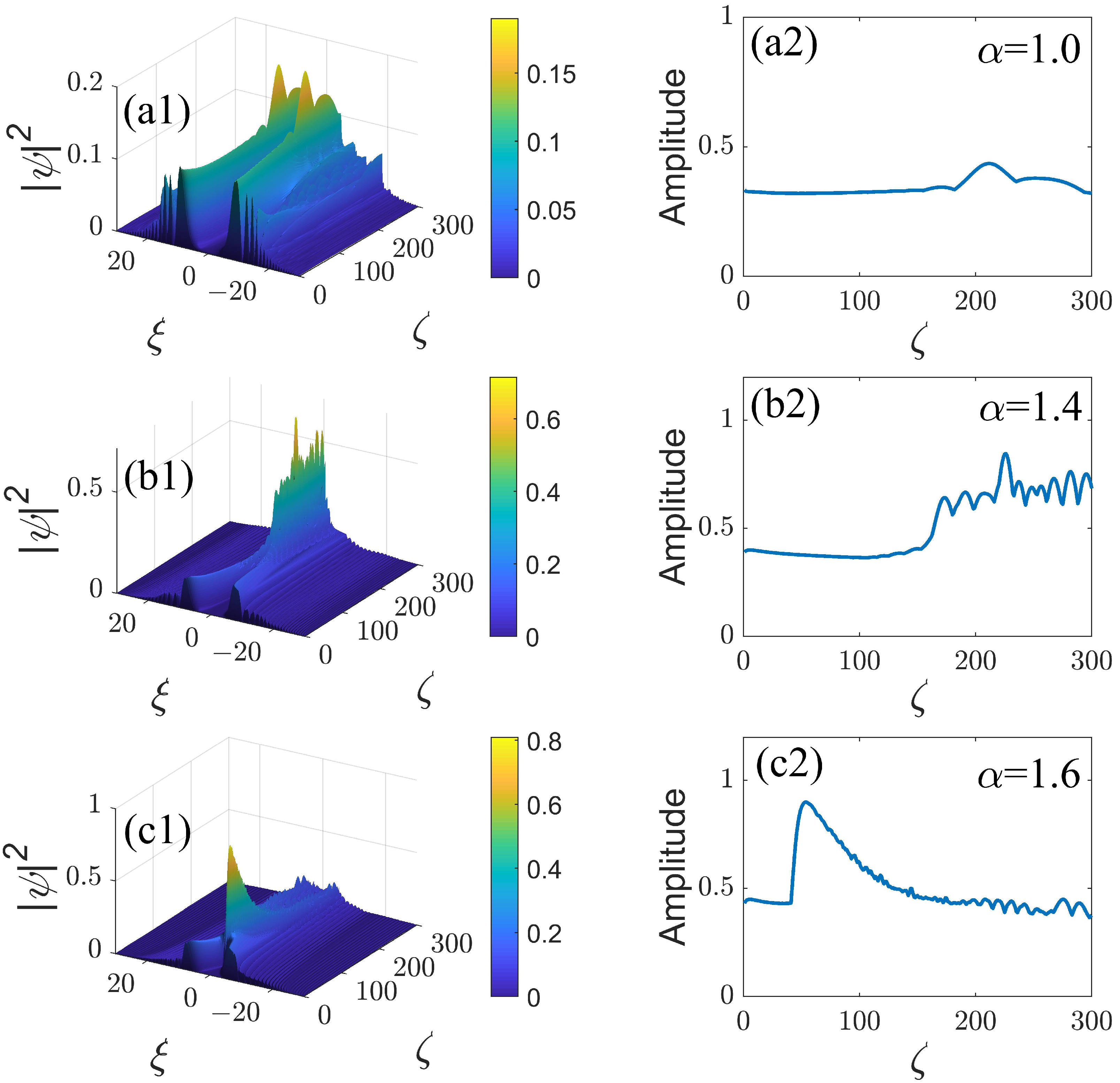
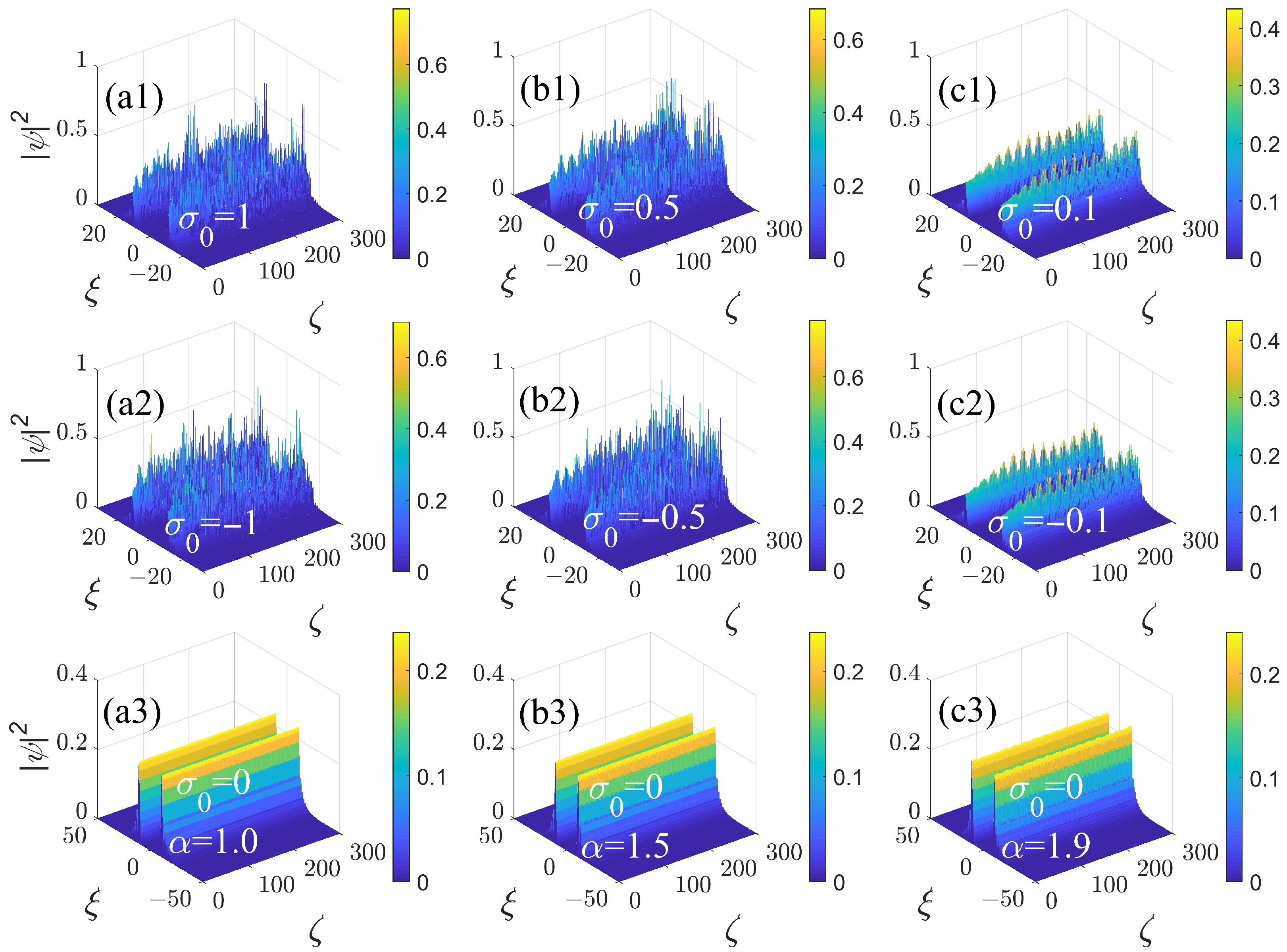
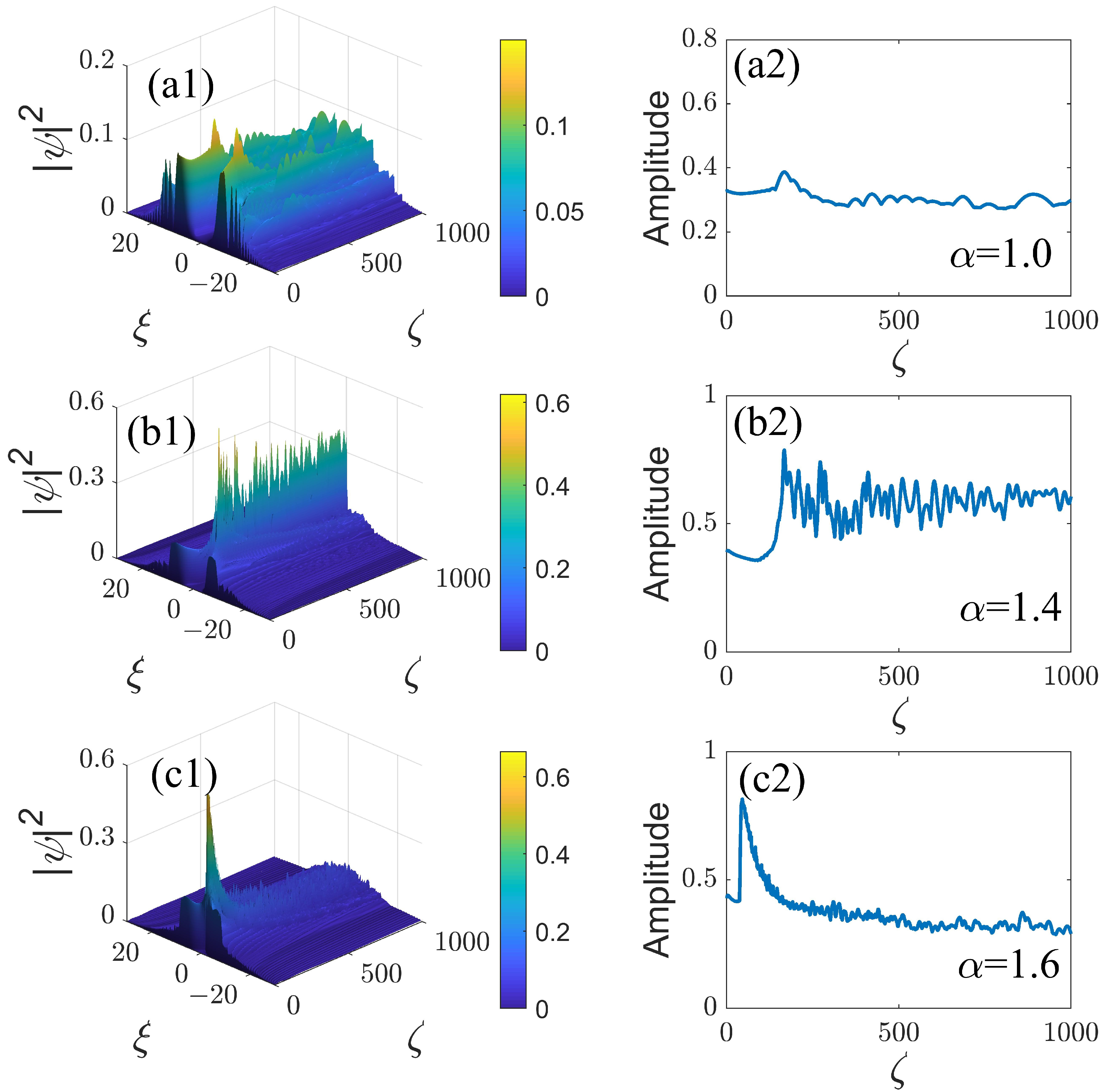
3. Numerical Results
3.1. The Model with Diffraction Management
3.2. The Model with Nonlinearity Management
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| 2D | Two-dimensional |
| LI | Lévy index |
| FSE | Fractional Schrödinger equation |
| NLSE | Nonlinear Schrödinger equation |
References
- Berry, M.V.; Balazs, N.L. Diffraction-free beams. Am. J. Phys. 1979, 47, 264–267. [Google Scholar] [CrossRef]
- Voloch-Bloch, N.; Lereah, Y.; Lilach, Y.; Gover, A.; Arie, A. Generation of electron Airy beams. Nature 2013, 494, 331–335. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Efremidis, N.K.; Chen, Z.G.; Segev, M.; Christodoulides, D.N. Airy beams and accelerating waves: An overview of recent advances. Optica 2019, 6, 686–701. [Google Scholar] [CrossRef] [Green Version]
- Minovich, A.E.; Klein, A.E.; Neshev, D.N.; Pertsch, T.; Kivshar, Y.S.; Christodoulides, D.N. Airy plasmons: Non-diffracting optical surface waves. Laser. Phot. Res. 2014, 8, 221–232. [Google Scholar] [CrossRef]
- Durnin, J. Exact solutions for nondiffracting beams. I. The scalar theory. J. Opt. Soc. Am. A 1987, 4, 651–654. [Google Scholar] [CrossRef]
- Durnin, J.; Miceli, J.J.; Eberly, J.H. Diffraction-free beams. Phys. Rev. Lett. 1987, 58, 1499–1501. [Google Scholar] [CrossRef]
- Gutiérrez-Vega, J.C.; Iturbe-Castillo, M.D.; Chávez-Cerda, S. Alternative formulation for invariant optical fields: Mathieu beams. Opt. Lett. 2000, 25, 1493–1495. [Google Scholar] [CrossRef]
- Bandres, M.A.; Gutiérrez-Vega, J.C.; Chávez-Cerda, S. Parabolic nondiffracting optical wave fields. Opt. Lett. 2004, 29, 44–46. [Google Scholar] [CrossRef]
- Recami, E.; Zamboni-Rached, M. Localized waves: A review. Adv. Imaging Electron. Phys. 2009, 56, 235–353. [Google Scholar]
- Siviloglou, G.A.; Broky, J.; Dogariu, A.; Christodoulides, D.N. Observation of accelerating Airy beams. Phys. Rev. Lett. 2007, 99, 213901. [Google Scholar] [CrossRef]
- Siviloglou, G.A.; Christodoulides, D.N. Accelerating finite energy Airy beams. Opt. Lett. 2007, 32, 979–981. [Google Scholar] [CrossRef]
- Efremidis, N.K.; Christodoulides, D.N. Abruptly autofocusing waves. Opt. Lett. 2010, 35, 4045–4047. [Google Scholar] [CrossRef] [Green Version]
- Papazoglou, D.G.; Efremidis, N.K.; Christodoulides, D.N.; Tzortzakis, S. Observation of abruptly autofocusing waves. Opt. Lett. 2011, 36, 1842–1844. [Google Scholar] [CrossRef] [Green Version]
- Zhang, P.; Prakash, J.; Zhang, Z.; Mills, M.S.; Efremidis, N.K.; Christodoulides, D.N.; Chen, Z.G. Trapping and guiding microparticles with morphing autofocusing Airy beams. Opt. Lett. 2011, 36, 2883–2885. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chremmos, I.; Zhang, P.; Prakash, J.; Efremidis, N.K.; Christodoulides, D.N.; Chen, Z.G. Fourier-space generation of abruptly autofocusing beams and optical bottle beams. Opt. Lett. 2011, 36, 3675–3677. [Google Scholar] [CrossRef] [Green Version]
- Liu, S.; Wang, M.R.; Li, P.; Zhang, P.; Zhao, J.L. Abrupt polarization transition of vector autofocusing Airy beams. Opt. Lett. 2013, 38, 2416–2418. [Google Scholar] [CrossRef]
- Jiang, Y.F.; Huang, K.K.; Lu, X.H. Propagation dynamics of abruptly autofocusing Airy beams with optical vortices. Opt. Express 2012, 20, 18579–18584. [Google Scholar] [CrossRef] [PubMed]
- Chen, B.; Chen, C.D.; Peng, X.; Peng, Y.L.; Zhou, M.L.; Deng, D.M. Propagation of sharply autofocused ring Airy Gaussian vortex beams. Opt. Express 2015, 23, 19288–19298. [Google Scholar] [CrossRef] [PubMed]
- Li, P.; Liu, S.; Peng, T.; Xie, G.F.; Gan, X.T.; Zhao, J.L. Spiral autofocusing Airy beams carrying power-exponent-phase vortices. Opt. Express 2014, 22, 7598–7606. [Google Scholar] [CrossRef]
- Polynkin, P.; Kolesik, M.; Moloney, J.V.; Siviloglou, G.A.; Christodoulides, D.N. Curved plasma channel generation using ultraintense Airy beams. Science 2009, 324, 229–232. [Google Scholar] [CrossRef]
- Polynkin, P.; Kolesik, M.; Moloney, J.V. Filamentation of femtosecond laser Airy beams in water. Phys. Rev. Lett. 2009, 103, 123902. [Google Scholar] [CrossRef] [PubMed]
- Clerici, M.; Hu, Y.; Lassonde, P.; Milián, C.; Couairon, A.; Christodoulides, D.N.; Chen, Z.G.; Razzari, L.; Vidal, F.; Légaré, F.; et al. Laser-assisted guiding of electric discharges around objects. Sci. Adv. 2015, 1, e1400111. [Google Scholar] [CrossRef] [Green Version]
- Vettenburg, T.; Dalgarno, H.I.C.; Nylk, J.; Coll-Lladó, C.; Ferrier, D.E.K.; Cizmar, T.; Gunn-Moore, F.J.; Dholakia, K. Light-sheet microscopy using an Airy beam. Nat. Methods 2014, 11, 541–544. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nylk, J.; McCluskey, K.; Preciado, M.A.; Mazilu, M.; Yang, Z.; Gunn-Moore, F.J.; Aggarwal, S.; Tello, J.A.; Ferrier, D.E.; Dholakia, K. Light sheet microscopy with attenuation-compensated propagation-invariant beams. Sci. Adv. 2018, 4, eaar4817. [Google Scholar] [CrossRef] [Green Version]
- Preciado, M.A.; Dholakia, K.; Mazilu, M. Generation of attenuation compensating Airy beams. Opt. Lett. 2014, 39, 4950–4953. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baumgartl, J.; Mazilu, M.; Dholakia, K. Optically mediated particle clearing using Airy wavepackets. Nat. Photonics 2008, 2, 675–678. [Google Scholar] [CrossRef]
- Cheng, H.; Zang, W.P.; Zhou, W.Y.; Tian, J.G. Analysis of optical trapping and propulsion of Rayleigh particles using Airy beam. Opt. Express 2010, 18, 20384–20394. [Google Scholar] [CrossRef]
- Zheng, Z.; Zhang, B.F.; Chen, H.; Ding, J.P.; Wang, H.T. Optical trapping with focused Airy beams. Appl. Opt. 2011, 50, 43–49. [Google Scholar] [CrossRef]
- Salandrino, A.; Christodoulides, D.N. Airy plasmon: A nondiffracting surface wave. Opt. Lett. 2010, 35, 2082–2084. [Google Scholar] [CrossRef]
- Minovich, A.; Klein, A.E.; Janunts, N.; Pertsch, T.; Neshev, D.N.; Kivshar, Y.S. Generation and near-field imaging of Airy surface plasmons. Phys. Rev. Lett. 2011, 107, 116802. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Li, T.; Wang, S.M.; Zhang, C.; Zhu, S.N. Plasmonic Airy beam generated by in-plane diffraction. Phys. Rev. Lett. 2011, 107, 126804. [Google Scholar] [CrossRef] [PubMed]
- Kaminer, I.; Segev, M.; Christodoulides, D.N. Self-accelerating self-trapped optical beams. Phys. Rev. Lett. 2011, 106, 213903. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bekenstein, R.; Segev, M. Self-accelerating optical beams in highly nonlocal nonlinear media. Opt. Express 2011, 19, 23706–23715. [Google Scholar] [CrossRef] [PubMed]
- Fattal, Y.; Rudnick, A.; Marom, D.M. Soliton shedding from Airy pulses in Kerr media. Opt. Express 2011, 19, 17298–17307. [Google Scholar] [CrossRef] [PubMed]
- Shen, M.; Gao, J.S.; Ge, L.J. Solitons shedding from Airy beams and bound states of breathing Airy solitons in nonlocal nonlinear media. Sci. Rep. 2015, 5, 9814. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Sun, Z.; Bongiovanni, D.; Song, D.H.; Lou, C.B.; Xu, J.J.; Chen, Z.G.; Morandotti, R. Reshaping the trajectory and spectrum of nonlinear Airy beams. Opt. Lett. 2012, 37, 3201–3203. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Li, M.; Bongiovanni, D.; Clerici, M.; Yao, J.P.; Chen, Z.G.; Azaña, J.; Morandotti, R. Spectrum to distance mapping via nonlinear Airy pulses. Opt. Lett. 2013, 38, 380–382. [Google Scholar] [CrossRef] [Green Version]
- Malomed, B.A. Self-accelerating solitons. EPL 2022, 140, 22001. [Google Scholar] [CrossRef]
- Dolev, I.; Kaminer, I.; Shapira, A.; Segev, M.; Arie, A. Experimental observation of self-accelerating beams in quadratic nonlinear media. Phys. Rev. Lett. 2012, 108, 113903. [Google Scholar] [CrossRef]
- Mayteevarunyoo, T.; Malomed, B.A. Generation of χ2 solitons from the Airy wave through the parametric instability. Opt. Lett. 2015, 40, 4947–4950. [Google Scholar] [CrossRef]
- Mayteevarunyoo, T.; Malomed, B.A. Two-dimensional χ2 solitons generated by the downconversion of Airy waves. Opt. Lett. 2016, 41, 2919–2922. [Google Scholar] [CrossRef]
- Mayteevarunyoo, T.; Malomed, B.A. The interaction of Airy waves and solitons in the three-wave system. J. Optics. 2017, 19, 085501. [Google Scholar] [CrossRef] [Green Version]
- Prasatsap, U.; Mayteevarunyoo, T.; Malomed, B.A. Two-dimensional Airy waves and three-wave solitons in quadratic media. J. Optics. 2022, 24, 055501. [Google Scholar] [CrossRef]
- Liu, J.F.; Zhang, Y.Q.; Zhong, H.; Zhang, J.W.; Wang, R.; Belić, M.R.; Zhang, Y.P. Optical Bloch oscillations of a dual Airy beam. Ann. Phys. 2017, 530, 1700307. [Google Scholar] [CrossRef]
- Wang, X.N.; Fu, X.Q.; Huang, X.W.; Yang, Y.J.; Bai, Y.F. The robustness of truncated Airy beam in PT Gaussian potentials media. Opt. Commun 2018, 410, 717–722. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2020, 339, 1–77. [Google Scholar] [CrossRef]
- Zaslavsky, G.M. Chaos, fractional kinetics, and anomalous transport. Phys. Rep. 2002, 371, 461–580. [Google Scholar] [CrossRef]
- Chechkin, A.C.; Gorenflo, R.; Sokolov, I.M. Fractional diffusion in inhomogeneous media. J. Phys. A Math. Gen. 2005, 38, L679–L684. [Google Scholar] [CrossRef]
- Laughlin, R.B. Anomalous quantum hall efect: An incompressible quantum fuid with fractionally charged excitations. Phys. Rev. Lett. 1983, 50, 1395–1398. [Google Scholar] [CrossRef] [Green Version]
- Rokhinson, L.P.; Liu, X.Y.; Furdyna, J.K. The fractional A.C. Josephson efect in a semiconductor-superconductor nanowire as a signature of Majorana particles. Nat. Phys. 2012, 8, 795–799. [Google Scholar] [CrossRef] [Green Version]
- Momani, S.; Arqub, O.A.; Maayah, B.; Yousef, F.; Alsaedi, A. A reliable algorithm for solving linear and nonlinear Schrödinger equations. Appl. Comput. Math. 2018, 17, 151–160. [Google Scholar]
- Sulem, C.; Sulem, P. The Nonlinear Schrödinger Equation: Self-Focusing and Wave Collapse; Springer Series in Mathematical Sciences; Springer: Berlin, Germany, 1999. [Google Scholar]
- Bao, W.; Cai, Y. Mathematical theory and numerical methods for Bose-Einstein condensation. Kinet. Relat. Models 2013, 6, 1–135. [Google Scholar] [CrossRef]
- Laskin, N. Fractional quantum mechanics. Phys. Rev. E 2000, 62, 3135–3145. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Laskin, N. Fractional quantum mechanics and Lévy path integrals. Phys. Lett. A 2000, 268, 298–305. [Google Scholar] [CrossRef] [Green Version]
- Laskin, N. Fractional Schrödinger equation. Phys. Rev. E 2002, 66, 056108. [Google Scholar] [CrossRef] [Green Version]
- Longhi, S. Fractional Schrödinger equation in optics. Opt. Lett. 2015, 40, 1117–1120. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Zhang, Y.; Malomed, B.A.; Karimi, E. Experimental realisations of the fractional Schrödinger equation in the temporal domain. arXiv 2022, arXiv:2208.01128. [Google Scholar]
- Huang, X.W.; Deng, Z.X.; Fu, X.Q. Dynamics of finite energy Airy beams modeled by the fractional Schrödinger equation with a linear potential. J. Opt. Soc. Am. B 2017, 34, 976–982. [Google Scholar] [CrossRef]
- Huang, X.W.; Deng, Z.X.; Shi, X.H.; Fu, X.Q. Propagation characteristics of ring Airy beams modeled by fractional Schrödinger equation. J. Opt. Soc. Am. B 2017, 34, 2190–2197. [Google Scholar] [CrossRef]
- Huang, X.W.; Shi, X.H.; Deng, Z.X.; Bai, Y.F.; Fu, X.Q. Potential barrier-induced dynamics of finite energy Airy beams in fractional Schrödinger equation. Opt. Express 2017, 25, 32560–32569. [Google Scholar] [CrossRef]
- He, S.L.; Malomed, B.A.; Mihalache, D.; Peng, X.; Yu, X.; He, Y.J.; Deng, D.M. Propagation dynamics of abruptly autofocusing circular Airy Gaussianvortex beams in the fractional Schrödinger equation. Chaos Solitons Fractals 2021, 142, 110470. [Google Scholar] [CrossRef]
- He, S.L.; Malomed, B.A.; Mihalache, D.; Peng, X.; He, Y.J. Propagation dynamics of radially polarized symmetric Airy beams in the fractional Schrödinger equation. Phys. Lett. A 2021, 404, 127403. [Google Scholar] [CrossRef]
- Zhang, L.F.; Zhang, X.; Wu, H.Z.; Li, C.X.; Pierangeli, D.; Gao, Y.X.; Fan, D.Y. Anomalous interaction of Airy beams in the fractional nonlinear Schrödinger equation. Opt. Express 2019, 27, 27936–27945. [Google Scholar] [CrossRef] [PubMed]
- He, S.L.; Zhou, K.Z.; Malomed, B.A.; Mihalache, D.; Zhang, L.P.; Tu, J.L.; Wu, Y.; Zhao, J.J.; Peng, X.; He, Y.J.; et al. Airy-Gaussian vortex beams in the fractional nonlinear Schrödinger medium. J. Opt. Soc. Am. B 2021, 38, 3230–3236. [Google Scholar] [CrossRef]
- Malomed, B.A. Optical solitons and vortices in fractional media: A mini-review of recent results. Photonics 2021, 8, 353. [Google Scholar] [CrossRef]
- Malomed, B.A. Soliton Management in Periodic Systems; Springer: New York, NY, USA, 2006. [Google Scholar]
- Eisenberg, H.S.; Silberberg, Y.; Morandotti, R.; Aitchinson, J.S. Diffraction management. Phys. Rev. Lett. 2000, 85, 1863–1866. [Google Scholar] [CrossRef]
- Firth, W.J.; Skryabin, D.V. Optical solitons carrying orbital angular momentum. Phys. Rev. Lett. 1997, 79, 2450–2453. [Google Scholar] [CrossRef]
- Malomed, B.A. Multidimensional Solitons; AIP Publishing: Melville, NY, USA, 2022. [Google Scholar]
- Wang, Q.; Zhang, L.; Malomed, B.A.; Mihalache, D.; Zeng, L. Transformation of multipole and vortex solitons in the nonlocal nonlinear fractional Schrödinger equation by means of Lévy-index management. Chaos Solitons Fractals 2022, 157, 111995. [Google Scholar] [CrossRef]
- Samko, G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach: New York, NY, USA, 1993. [Google Scholar]
- Duo, S.W.; Zhang, Y.Z. Mass-conservative Fourier spectral methods for solving the fractional nonlinear Schrödinger equation. Comput. Math. Appl. 2016, 71, 2257–2271. [Google Scholar] [CrossRef] [Green Version]
- Coutaz, J.L.; Kull, M. Saturation of the nonlinear index of refraction in semiconductor-doped glass. J. Opt. Soc. Am. B 1991, 8, 95–98. [Google Scholar] [CrossRef]
- Tikhonenko, V.; Christou, J.; Luther-Davies, B. Three dimensional bright spatial soliton collision and fusion in a saturable nonlinear medium. Phys. Rev. Lett. 1996, 76, 2698–2701. [Google Scholar] [CrossRef] [PubMed]
- Malomed, B.A.; Matera, F.; Settembre, M. Reduction of the jitter for return-to-zero signals. Opt. Commun. 1997, 143, 193–198. [Google Scholar] [CrossRef]
- Towers, I.; Malomed, B.A. Stable (2+1)-dimensional solitons in a layered medium with sign-alternating Kerr nonlinearity. J. Opt. Soc. Am. B 2002, 19, 537–543. [Google Scholar] [CrossRef]
- Abdullaev, F.K.; Caputo, J.G.; Kraenkel, R.A.; Malomed, B.A. Controlling collapse in Bose-Einstein condensation by temporal modulation of the scattering length. Phys. Rev. A 2003, 67, 013605. [Google Scholar] [CrossRef] [Green Version]
- Saito, H.; Ueda, M. Dynamically stabilized bright solitons in a two-dimensional Bose-Einstein condensate. Phys. Rev. Lett. 2003, 90, 040403. [Google Scholar] [CrossRef] [Green Version]
- Itin, A.; Morishita, T.; Watanabe, S. Reexamination of dynamical stabilization of matter-wave solitons. Phys. Rev. A 2006, 74, 033613. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Wei, Y.; Malomed, B.A.; Mihalache, D. Stabilization of Axisymmetric Airy Beams by Means of Diffraction and Nonlinearity Management in Two-Dimensional Fractional Nonlinear Schrödinger Equations. Symmetry 2022, 14, 2664. https://doi.org/10.3390/sym14122664
Li P, Wei Y, Malomed BA, Mihalache D. Stabilization of Axisymmetric Airy Beams by Means of Diffraction and Nonlinearity Management in Two-Dimensional Fractional Nonlinear Schrödinger Equations. Symmetry. 2022; 14(12):2664. https://doi.org/10.3390/sym14122664
Chicago/Turabian StyleLi, Pengfei, Yanzhu Wei, Boris A. Malomed, and Dumitru Mihalache. 2022. "Stabilization of Axisymmetric Airy Beams by Means of Diffraction and Nonlinearity Management in Two-Dimensional Fractional Nonlinear Schrödinger Equations" Symmetry 14, no. 12: 2664. https://doi.org/10.3390/sym14122664
APA StyleLi, P., Wei, Y., Malomed, B. A., & Mihalache, D. (2022). Stabilization of Axisymmetric Airy Beams by Means of Diffraction and Nonlinearity Management in Two-Dimensional Fractional Nonlinear Schrödinger Equations. Symmetry, 14(12), 2664. https://doi.org/10.3390/sym14122664







