The Black Hole Universe, Part I

Abstract

The original Friedmann (1922) and Lemaitre (1927) cosmological model corresponds to a classical solution of General Relativity (GR), with the same uniform (FLRW) metric as the standard cosmology, but bounded to a sphere of radius R and empty space outside. We study the junction conditions for R to show that a co-moving observer, like us, located anywhere inside R, measures the same background and has the same past light-cone as an observer in an infinite FLRW with the same density. We also estimate the mass M inside R and show that in the observed universe $R<r_S\equiv 2$ GM, which corresponds to a Black Hole Universe (BHU). We argue that this original Friedmann–Lemaitre model can explain the observed cosmic acceleration without the need of Dark Energy, because $r_S$ acts like a cosmological constant $\mathrm{\Lambda }=3/r_S^2$. The same solution can describe the interior of a stellar or galactic BHs. In co-moving coordinates the BHU is expanding while in physical or proper coordinates it is asymptotically static. Such frame duality corresponds to a simple Lorentz transformation. The BHU therefore provides a physical BH solution with an asymptotically deSitter metric interior that merges into a Schwarzschild metric exterior without discontinuities.

1. Introduction

A Schwarzschild black hole (BH) metric (SBH, in Equation (A12)) corresponds to a singular object of mass M, whose event horizon, $r_S\equiv 2$ GM, prevents getting information from the interior $r<r_S$ to any exterior observer. Physically, a singular point does not make much sense. The concept of physical infinity is not a scientific one if science involves testability by either observation or experiment [1]. So if the SBH is not a proper physical solution all the way to $r=0$, are there other physical solutions for the metric inside observed BHs? The internal metric and BH content are in fact important to understand gravitational wave emission, BH interactions, BH formation or the origin of BHs as dark matter candidates (see Refs. [2,3] and references for some discussion). We look here for an alternative solution to the SBH interior, defined as an object of energy-mass M and size $0<R<r_S$ that reproduces the SBH metric, i.e., it is approximately empty, on the outside $r>r_S$. The corresponding mean energy density at $R=r_S=2$ GM, as measured by an observer outside (in flat empty space) is:

$${\rho }_{BH}=\frac{M}{V}=\frac{3r_S^{-2}}{8\pi G}.$$

(1)

regardless of its contents. Even when the BH is static outside, a static solution for the BH interior cannot exist for regular matter or radiation because it requires a minimal Buchdahl radius [4]: $R>\frac{9}{8}{r}_S$, which is larger than $r_S$ and it is therefore not a BH (the mass inside $r_S$ is smaller than $r_S/2G$, so there is no event horizon). However, objects with external mass M and sizes $R=r_S$ have been observed. What is then inside a BH? For a perfect fluid, to achieve such a density, the radial pressure inside a BH needs to be negative (see Ref. [5] and references therein). Cosmologist are used to this type of fluids, which are called Quintessence, Inflation, or Dark Energy (DE). Could the inside of a BH be DE [6]? Could it also be an expanding universe, like ours? If so, what can this tell us about our universe?

Here, we explore this idea more generally: a non static but uniform GR solution inside a finite R that we call the FLRW cloud (or FLRW*) based on the Lemaitre [7] cosmological model. As it is well known (see, e.g., Ref. [8]), it was Lemaitre who first published and realised the meaning and implications of the distance-redshift relation ($\dot{r}=Hr$) that made Hubble famous. Lemaitre noticed that this was a consequence of Einstein’s new theory of gravity described by the (later known as) Friedmann–Lemaitre–Robertson–Walker (FLRW) flat metric in co-moving coordinates ${\xi }^{\alpha }=(\tau ,\chi ,\theta ,\delta )$, which corresponds to an homogeneous and isotropic (usually assumed infinite) space-time:

$$d{s}^2=f_{\alpha \beta }d{\xi }^{\alpha }d{\xi }^{\beta }=-d{\tau }^2+a{\left(\tau \right)}^2\left[d{\chi }^2+{\chi }^{2}d\mathrm{\Omega }\right],$$

(2)

For a perfect fluid with density $\rho$ and pressure p, the solution to GR field equations is well-known. The different energy conservation and acceleration equations reduced to (see Equation (10).73 in Ref. [9]):

$$H^2\equiv {\left(\frac{\dot{a}}{a}\right)}^2=\frac{8\pi G}{3}\rho =H_0^2\left[{\mathrm{\Omega }}_m{a}^{-3}+{\mathrm{\Omega }}_R{a}^{-4}+{\mathrm{\Omega }}_{\mathrm{\Lambda }}\right],$$

(3)

where ${\rho }_c\equiv \frac{3H_0^2}{8\pi G}$ and ${\mathrm{\Omega }}_X\equiv \frac{{\rho }_X}{{\rho }_c}$, where ${\mathrm{\Omega }}_m$ and ${\mathrm{\Omega }}_R$ represents the current ($a=1$) matter and radiation density, respectively, and ${\mathrm{\Omega }}_m+{\mathrm{\Omega }}_R+{\mathrm{\Omega }}_{\mathrm{\Lambda }}=1$. The cosmological constant term ${\mathrm{\Omega }}_{\mathrm{\Lambda }}$ results from: ${\rho }_{\mathrm{\Lambda }}\equiv \frac{\mathrm{\Lambda }}{8\pi G}$. This is the standard cosmological ($\mathrm{\Lambda }$CDM ) model. Given ${\mathrm{\Omega }}_X$, we can solve Equation (3) to find $a\left(\tau \right)$. In this paper, we will use these same equations to make the two points below, which will be discussed in more detail in the corresponding section as shown:

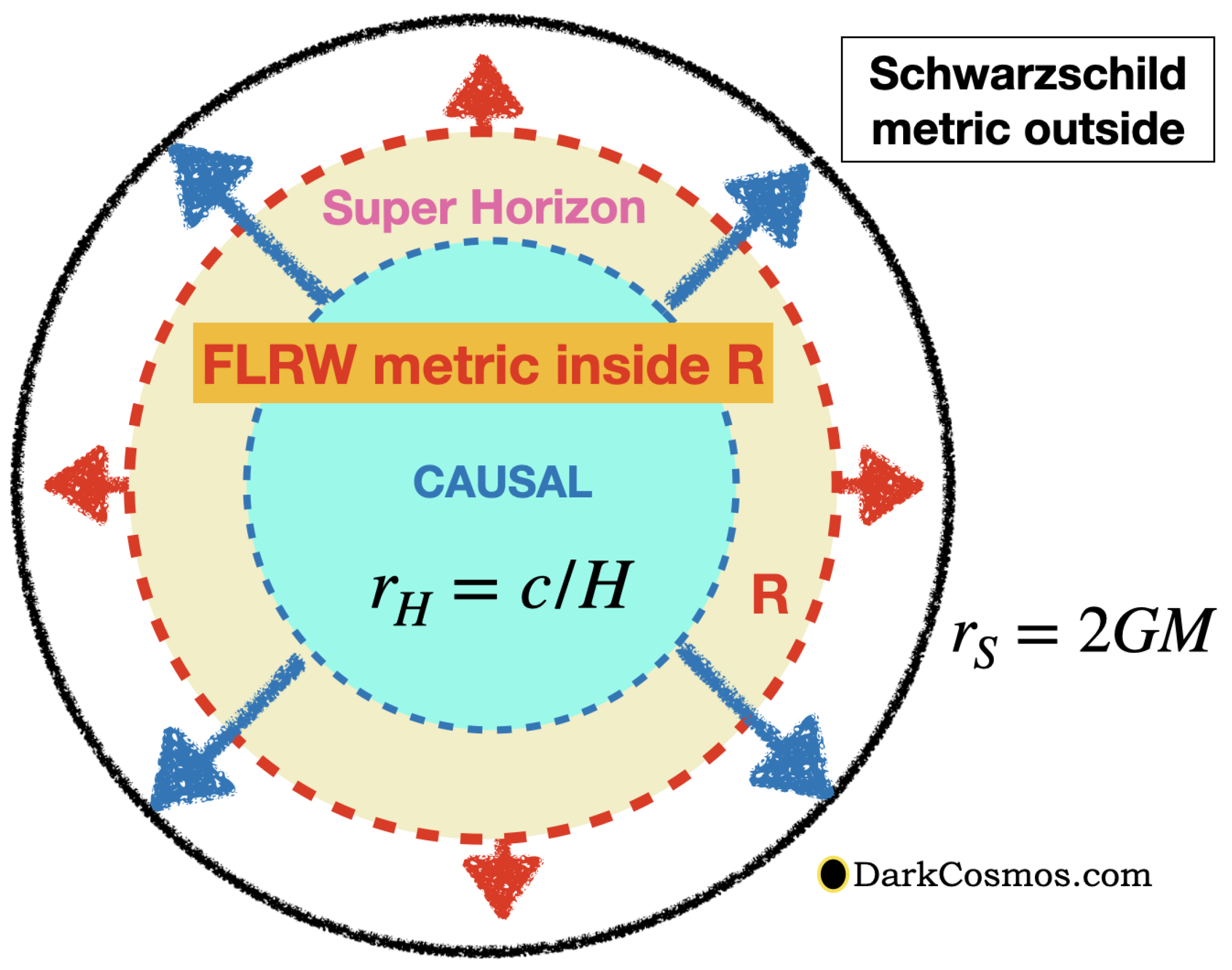
• Section 2: A FLRW cloud (FLRW*) is also a GR solution. The FLRW metric with a finite spherical volume of proper radius $r\equiv a\chi =R$ is also a GR solution. When FLRW* is inside its Schwarzschild radius $R\le r_S$ and the space outside can be approximated as empty, this is a BHU. This solution for a BH interior is different from the SBH solution.
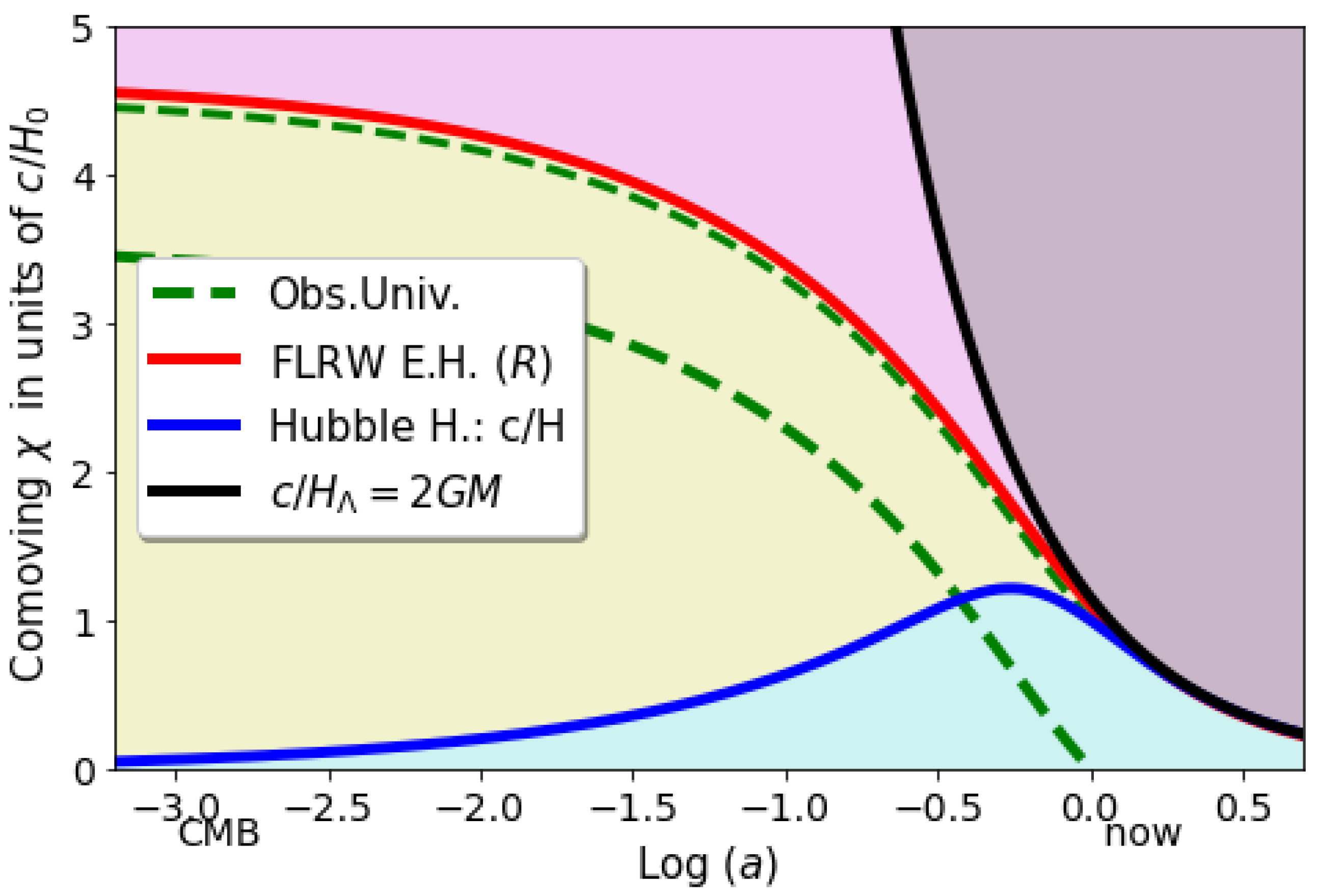
• Section 3: A BHU without DE has the same observable background as ΛCDM. The observed cosmic acceleration can be understood as resulting from a BHU without DE, where $r_S=2$ GM acts like as an effective $\mathrm{\Lambda }$ term, with $\mathrm{\Lambda }=3/r_S^2$. A co-moving observer, anywhere within R in a BHU, sees the same background as an observer in the $\mathrm{\Lambda }$CDM with the same density.

Section 2 and Section 3 are dedicated to each of the points above. As we will show, the existence of the FLRW* solution is not a new result but its interpretation in the light of the observed cosmic acceleration and the BHU is new. In Section 2.2 we present a new calculation of junction conditions for the FLRW* space that will allow us to address the second point above, which is the main result presented in this paper. We end with a discussion and comparison with literature in Section 4 and leave the notation and known solutions for the Appendix A, Appendix B, Appendix C, Appendix D.

2. The FLRW Cloud (FLRW*)

The FLRW* metric is given by the same flat FLRW metric in Equation (2) with the constraint $r=a\chi <R$. We will approximate $r>R$ to be empty, like we do for local solutions of regular stars or BHs in our universe. The FLRW* solution is then globally inhomogeneous and localized. There are several ways to show that FLRW* is an exact solution to GR equations. Here we present two alternative derivations. The first one is based on known classical literature of GR solutions. The second one is an explicit derivation using junction conditions for the join manifold, where we will find what are the constraints on $R\left(\tau \right)$ for the solution to work as a function of proper co-moving time. Misner and Sharp [10] already studied these junction conditions in $T_{\mu \nu }$ back in 1964 and concluded that we can smoothly join the FLRW* metric with the SBH metric as long as the pressure is $p=0$ at $r=R$, which is the condition for a constant mass M inside R. As we will argue, this $p=0$ condition is fulfilled for both the timelike and the null hypersurface of radius $r=R\left(\tau \right)$ presented here in Section 2.2. This result will then be used in Section 3 to show that both the FLRW and the FLRW* models have the same observable universe, despite the fact that FLRW* is a local solution and the observer could be anywhere, not necessarily at the centre. In Appendix B we present another way to look at these results based on the frame duality.

2.1. Classical Solutions

The first evidence for the FLRW* solution in GR comes from the original expanding universe discovery presented by Lemaitre ([7], English translation [11]). The same FLRW* solution was published before by Friedmann in 1922 ([12], English translation [13]), who even estimated $M\simeq 5\times {10}^{21}{M}_{\odot }$, close to its observed value today (see Equation (33)). The special relevance of Lemaitre’s work over Friedmann’s was to connected the FLRW* solution to the observed expansion law. The infinite FLRW metric can of course be recovered in the limit $R\to \infty$ and this has been the model adopted as the standard model of modern Cosmology. However, the finite cloud was further explored by Tolman [14], Oppenheimer and Snyder [15], and Misner and Sharp [10]. For a more extended review, see Ref. [16]. As detailed in Tolman [14] in his Application e), a combination of different FLRW distributions (with different densities at different radius) is also a solution to GR field equations. This is a consequence of the corollary to Birkhoff’s theorem [17] for spherically symmetric GR solutions. This corollary is equivalent to Gauss theorem in classical mechanics or electromagnetism. For an isotropic energy-mass distribution, the space-time geometry inside a region of radius R depends only on the content within R (and it is not influenced by the content outside R). Each sphere $r<R$ evolves with independence of what is outside $r>R$. This solution has also been generalized by Vaidya [18] for the interior of the Schwarzschild metric $r<r_S$ and found the particular cases of the FLRW* and the Schwarzschild (SBH) solutions.

Because R is finite, there is a finite energy-mass M inside R. This can be estimated using the Hawking quasi-local mass, which reduces to the Misner–Sharp mass in spherical symmetry [19,20]. For the FLRW* metric the Misner–Sharp mass is (see Appendix D):

$$M={\int }_0^{{\chi }_{\ast }}4\pi R^2\rho \left(\tau \right)\left(\frac{\partial R}{\partial \chi }\right)d\chi =\frac{1}{2G}{R}^3{H}^2\left(\tau \right)=\frac{4\pi }{3}{R}^3\rho \left(\tau \right)$$

(4)

where $R\equiv a{\chi }_{\ast }$ and $H\equiv \dot{a}/a$. We can now define the corresponding Schwarzschild radius $r_S\equiv 2$ GM and ask the following question: is $R\le r_S$? If the answer is yes, then the FLRW* is inside its own gravitational radius $r_S$ and the FLRW* metric corresponds to the interior of a BH, which we call the Black Hole Universe (BHU). We will later see in Section 3 that this indeed is the situation that corresponds to our observed Universe.

Can the BHU solution be used to described an observed BH? The answer seems affirmative as the BHU has all the desired BH properties: the SBH metric outside $r_S$ and some energy content inside which is not a singular point. The FLRW* solution has a past singularity. In part II of this series (paper II from now on) we explore how the BHU could be formed to avoid such singularity.

2.2. Junction Conditions for FLRW*

We can arrive at the finite FLRW* solution using Israel’s junction conditions [21,22]. We will combine two solutions to Einstein’s field equations with different contents:

$$\begin{array}{ccc}\hfill \rho (t,r)& =& \left\{\begin{array}{cc}\rho \hfill & \mathrm{inside}\mathrm{for}{V_4}^{-},\mathrm{i}.\mathrm{e}.,:r<R\hfill \\ 0\hfill & \mathrm{outside}\mathrm{for}{V_4}^{+},\mathrm{i}.\mathrm{e}.,:r>R\hfill \end{array}\right..\hfill \end{array}$$

(5)

on two sides (${V_4}^{-}$ and ${V_4}^{+}$) of a hypersurface junction $\mathrm{\Sigma }$ which is just given by proper coordinate junction $r=R$. The inside metric, $g^{-}$, is the FLRW metric and the outside one, $g^{+}$, is the SBH metric. The junction conditions require that the metric and its derivative (the extrinsic curvature K) match at $\mathrm{\Sigma }$. This means that the joint space provides a new solution to Einstein’s field equations in $V_4={V_4}^{-}\cup {V_4}^{+}$ (FLRW+SBH). In many situations, as in the Bubble Universes (see Section 4), this does not work and the junction requires a surface term (the bubble) to glue both solutions together (see also Ref. [23] for a more general consideration). We will show that for both timelike and null hypersurface the junction conditions are satisfied for FLRW+SBH and there are no surface terms. In this section, we follow closely the notation in Section 12.5 of Ref. [9] with $d{s}^2=g_{ab}d{x}^{a}d{x}^b$ where $a=0,1,2,3$ for the 4D metric and $d{s}_{\mathrm{\Sigma }}^2=h_{\alpha \beta }d{y}^{\alpha }d{y}^{\beta }$ with $\alpha =0,1,2$ for the 3D induced metric: i.e., $g_{ab}$ restricted to the $\mathrm{\Sigma }$ hypersurface.

2.2.1. Timelike Junction

We start by choosing a timelike hypersurface for $\mathrm{\Sigma }$, fixed in co-moving coordinates at some value ${\chi }_{\ast }$. This can be identified with a causal boundary, like the freefall collapse/expansion of a star of fixed mass M or the particle horizon of Cosmic Inflation ${\chi }_{\S }={\chi }_{\ast }={\left(a_i{H}_i\right)}^{-1}$, where $a_i$ and $H_i$ are the scale factor and Hubble rate when inflation begins [24]. The spherical shell radius R follows a radial geodesic trajectory in the FLRW metric. This corresponds to a FLRW cloud of fixed energy-mass M that is expanding or contracting. The induced 3D metric $h_{\alpha \beta }^{-}$ for $d{y}^{\alpha }=(d\tau ,d\delta ,d\theta )$ and fixed $\chi ={\chi }_{\ast }$, is:

$$d{s}_{{\mathrm{\Sigma }}^{-}}^2=h_{\alpha \beta }^{-}d{y}^{\alpha }d{y}^{\beta }=-d{\tau }^2+a^2\left(\tau \right){\chi }_{\ast }^2{d\mathrm{\Omega }}^2$$

(6)

The only free variable remaining is $\tau$, the FLRW co-moving time (the angles are free but they are the same in both metrics, as we have spherical symmetry). For the outside Schwarzschild frame, the same junction ${\mathrm{\Sigma }}^{+}$ is described by some unknown functions $r=R\left(\tau \right)$ and $t=T\left(\tau \right)$, where t and r are the time and radial coordinates in the physical frame of Equation (A5) of the SBH. We then have:

$$dr=\dot{R}d\tau ;dt=\dot{T}d\tau ,$$

(7)

where the dot refers to derivatives with respect to $\tau$. The induced metric $h^{+}$ estimated from the outside SBH metric (in Equation (A12)) becomes:

$$\begin{array}{ccc}\hfill d{s}_{{\mathrm{\Sigma }}^{+}}^2& =& h_{\alpha \beta }^{+}d{y}^{\alpha }d{y}^{\beta }=-Fd{t}^2+\frac{d{r}^2}{F}+r^2{d\mathrm{\Omega }}^2\hfill \\ & =& -(F{\dot{T}}^2-{\dot{R}}^2/F)d{\tau }^2+R^2{d\mathrm{\Omega }}^2\hfill \end{array}$$

(8)

where $F\equiv 1-r_S/R$. Comparing Equation (6) with Equation (8), the first matching condition $h^{-}=h^{+}$ results in:

$$R\left(\tau \right)=a\left(\tau \right){\chi }_{\ast };F\dot{T}=\sqrt{{\dot{R}}^2+F}\equiv \beta \left(R,\dot{R}\right)$$

(9)

For any given $a\left(\tau \right)$ and ${\chi }_{\ast }$ we can find both $R\left(\tau \right)$ and $\beta \left(\tau \right)$. We also want the derivative of the metric to be continuous at $\mathrm{\Sigma }$. For this, we estimate the extrinsic curvature $K^{\pm }$ normal to $\mathrm{\Sigma }$ from each side of the hypersurface (${\mathrm{\Sigma }}^{\pm }$) as:

$$K_{\alpha \beta }=-\left[{\partial }_a{n}_b-n_c{\mathrm{\Gamma }}_{ab}^c\right]e_{\alpha }^a{e}_{\beta }^b$$

(10)

where $e_{\alpha }^a=\partial x^a/\partial y^{\alpha }$ and $n_a$ is the 4D vector normal to $\mathrm{\Sigma }$. The outward 4D velocity is $u^a=e_{\tau }^a=(1,0,0,0)$ and the normal to ${\mathrm{\Sigma }}^{-}$ on the inside is then $n^{-}=(0,a,0,0)$. On the outside $u^a=(\dot{T},\dot{R},0,0)$ and $n^{+}=(-\dot{R},\dot{T},0,0)$. It is straightforward to verify that: $n_a{u}^a=0$ and $n_a{n}^a=+1$ (for a timelike surface) for both $n^{-}$ and $n^{+}$. The extrinsic curvature estimated with the inside FLRW metric, i.e., $K^{-}$ is:

$$\begin{array}{ccc}\hfill K_{\tau \tau }^{-}& =& -({\partial }_{\tau }{n}_{\tau }^{-}-a{\mathrm{\Gamma }}_{\tau \tau }^{\chi })e_{\tau }^{\tau }{e}_{\tau }^{\tau }=0\hfill \\ \hfill K_{\theta \theta }^{-}& =& a{\mathrm{\Gamma }}_{\theta \theta }^{\chi }{e}_{\theta }^{\theta }{e}_{\theta }^{\theta }=-a{\chi }_{\ast }=-R\hfill \end{array}$$

(11)

where we have used Equation (9) and the following Christoffel symbols for the FLRW:

$$\begin{array}{ccc}\hfill {\mathrm{\Gamma }}_{\tau \tau }^{\tau }={\mathrm{\Gamma }}_{\tau \chi }^{\tau }={\mathrm{\Gamma }}_{\tau \tau }^{\chi }={\mathrm{\Gamma }}_{\chi \chi }^{\chi }=0& ;& {\mathrm{\Gamma }}_{\theta \theta }^{\tau }=a^2{\chi }_{\ast }^{2}H\hfill \\ \hfill {\mathrm{\Gamma }}_{\tau \chi }^{\chi }={\mathrm{\Gamma }}_{\chi \chi }^{\tau }{a}^{-2}=H& ;& {\mathrm{\Gamma }}_{\theta \theta }^{\chi }=-{\chi }_{\ast }\hfill \end{array}$$

(12)

For the SBH metric we have:

$$\begin{array}{ccc}\hfill {\mathrm{\Gamma }}_{tt}^t={\mathrm{\Gamma }}_{tr}^r=0& ;& {\mathrm{\Gamma }}_{\theta \theta }^r=-FR;\hfill \\ \hfill {\mathrm{\Gamma }}_{tr}^t=-{\mathrm{\Gamma }}_{rr}^r& =& {\mathrm{\Gamma }}_{tt}^r{F}^{-2}=\frac{r_S}{2F{R}^2}\hfill \end{array}$$

(13)

which results in $K^{+}$:

$$\begin{array}{ccc}\hfill K_{\tau \tau }^{+}& =& \ddot{R}\dot{T}-\dot{R}\ddot{T}+\frac{\dot{T}{r}_S}{2R^{2}F}({\dot{T}}^2{F}^2-3{\dot{R}}^2)=\frac{\dot{\beta }}{\dot{R}}\hfill \\ \hfill K_{\theta \theta }^{+}& =& \dot{T}{\mathrm{\Gamma }}_{\theta \theta }^r=-\dot{T}FR=-\beta R\hfill \end{array}$$

(14)

where we have used the definition of $\beta$ in Equation (9). In both cases $K_{\delta \delta }={sin}^2\theta K_{\theta \theta }$, so that $K_{\delta \delta }^{-}=K_{\delta \delta }^{+}$ follows from $K_{\theta \theta }^{-}=K_{\theta \theta }^{+}$. Comparing Equation (11) with Equation (14), the matching conditions $K_{\alpha \beta }^{-}=K_{\alpha \beta }^{+}$ require $\beta =1$, which, using Equation (9) gives:

$$R={\left[r_H^2{r}_S\right]}^{1/3}$$

(15)

This reproduces the FLRW energy-mass M inside R in Equation (4). The time equation is:

$$\dot{T}=\frac{1}{1-R^2{H}^2}$$

(16)

which is the generalization of Equation (A20) for $\dot{H}\ne 0$ and agrees with ${\partial }_{\tau }t={(1+2{\mathrm{\Phi }}_W)}^{-1}$ in Equation (A17) for $2{\mathrm{\Phi }}_W=-H^2{R}^2$, so it corresponds to a time dilation in the co-moving frame ($\dot{r}=Hr$).

For constant $r_S$ the timelike $\mathrm{\Sigma }$, only works for a dust ($p=0$) matter dominated FLRW metric $r_H^2\propto a^3$, as only in this case Equation (15) agrees with $R=a{\chi }_{\ast }$ with a constant ${\chi }_{\ast }$. This corresponds to a FLRW dust cloud of fix energy-mass M expanding or collapsing. This case illustrates well the point we want to make. For $p\ne 0$ we need to consider a null junction, which allows for a general $H\left(\tau \right)$, see Section 2.2.3.

2.2.2. The GHY Boundary Term

The action inside an isolated BH is bounded by the event horizon $r<r_S$ and we need to add the GHY boundary term $S_{GHY}$ to the action in Equation (A4), where:

$$S_{GHY}=\frac{1}{8\pi G}{\oint }_{\partial V_4}{d}^{3}y\sqrt{-h}K$$

(17)

As explained in Appendix A, we need to add this term to the action for Einstein’s field Equation (A2) to be valid. We will next show that this term acts exactly like a $\mathrm{\Lambda }$ term in the action. So even if we start with a global $\mathrm{\Lambda }=0$ term, the action inside a BH generates a $\mathrm{\Lambda }$ term that is equal to $\mathrm{\Lambda }=3/r_S^2$.

The integral in Equation (17) is over the induced metric at $\partial V_4$, which corresponds to Equation (6), i.e., $\partial V_4=\mathrm{\Sigma }$ at $R=r_S$:

$$d{s}_{\partial V_4}^2=h_{\alpha \beta }d{y}^{\alpha }d{y}^{\beta }=-d{\tau }^2+r_S^2{d\mathrm{\Omega }}^2$$

(18)

So the only remaining degrees of freedom in the action are time $\tau$ and the angular coordinates. We can use this metric and Equation (11) to estimate K:

$$K=K_{\alpha }^{\alpha }=\frac{K_{\theta \theta }}{R^2}+\frac{K_{\delta \delta }}{R^2{sin}^2\theta }=-\frac{2}{R}=-\frac{2}{r_S}$$

(19)

We then have

$$S_{GHY}=\frac{1}{8\pi G}\int d\tau 4\pi r_S^{2}K=-\frac{r_S}{G}\tau$$

(20)

The $\mathrm{\Lambda }$ contribution to the action in Equation (A4) is:

$$S_{\mathrm{\Lambda }}=-\frac{\mathrm{\Lambda }}{8\pi G}{V}_4=-\frac{r_S^3\mathrm{\Lambda }}{3G}\tau$$

(21)

where we have estimated the total 4D volume $V_4$ as that bounded by $\partial V_4$ inside $r<r_S$: $V_4=2V_3\tau$, where the factor 2 accounts for the fact that $V_3=4\pi r_S^3/3$ can be covered both during collapse and during expansion, and both paths are available to the action inside $r_S$. Comparing the two terms we can see that we need $\mathrm{\Lambda }=3r_S^{-2}$ or equivalently $r_{\mathrm{\Lambda }}=r_S$ to cancel the boundary term. In other words: evolution inside a BH event horizon induces a $\mathrm{\Lambda }$ term in Einstein’s field equations even when there is no $\mathrm{\Lambda }$ term to start with. Such an event horizon is only a boundary for outgoing geodesics, i.e., expanding solutions. This provides a fundamental interpretation to the observed $\mathrm{\Lambda }$ as a causal boundary [25,26].

2.2.3. Null Junction

A null junction has degeneracies that require more elaborate consideration. Here we give a brief account of such a calculation. For more details, see Ref. [22]. We choose $\mathrm{\Sigma }$ to be a radial null surface in the FLRW metric, i.e.,: $d\tau =ad\chi$. This results in a radial coordinate ${\chi }_{\ast }\left(\tau \right)$, which is not always constant and that we want to identify with the FLRW event horizon of Equation (32). At any given time the corresponding physical distance is $r_{\ast }\left(\tau \right)=a\left(\tau \right){\chi }_{\ast }\left(\tau \right)$ with ${\dot{\chi }}_{\ast }=1/a$. For the outside Schwarzschild coordinate system, ${\mathrm{\Sigma }}^{+}$ is described as previously by Equation (7). The induced inside metric $h^{-}$ is then:

$$h_{\alpha \beta }^{-}d{y}^{\alpha }d{y}^{\beta }=a^2{\chi }_{\ast }^2{d\mathrm{\Omega }}^2=r_{\ast }^2\left(\tau \right)\left[d{\theta }^2+{sin}^2\left(\theta \right)d{\delta }^2\right]$$

(22)

This has to agree with $h^{+}$ in Equation (8). The first matching conditions $h^{-}=h^{+}$ are in this case:

$$\begin{array}{ccc}\hfill R=r_{\ast }\left(\tau \right)=a{\chi }_{\ast }& \Rightarrow & \dot{R}=HR+1\hfill \end{array}$$

(23)

$$\begin{array}{ccc}\hfill F^2{\dot{T}}^2={\dot{R}}^2& \Rightarrow & \dot{T}=\pm \frac{\dot{R}}{1-r_S/R}\hfill \end{array}$$

(24)

The outward 4D velocity is $u^a=e_t^a=(1,1/a,0,0)$, so it has a radial component in the co-moving frame. For a null surface we define a transverse extrinsic curvature [22]. We use the same notation as in Equation (10) with the difference that n is now a transverse null vector: $n_a{u}^a=0$ and $n_a{n}^a=0$. We then have: $n^{-}=A(1,-a,0,0)$ where $A=A\left(\tau \right)$ is an arbitrary function of $\tau$. On the outside $u^a=(\dot{T},\dot{R},0,0)$ and $n^{+}=(-\dot{R},\dot{T},0,0)$ as before, but where we have now used the new matching condition above $\dot{R}=F\dot{T}$ in Equation (24). Using the Christoffel symbols in Equations (12) and (13), we find:

$$\begin{array}{ccc}\hfill K_{\tau \tau }^{-}& =& -{\partial }_{\tau }{n}_{\tau }^{-}-2A{\mathrm{\Gamma }}_{\tau \chi }^{\chi }-\frac{1}{a}{\partial }_{\tau }{n}_{\chi }^{-}-\frac{A}{a^2}{\mathrm{\Gamma }}_{\chi \chi }^{\tau }=0\hfill \\ \hfill K_{\tau \tau }^{+}& =& \ddot{R}\dot{T}-\dot{R}\ddot{T}+\frac{\dot{T}{r}_S}{2R^{2}F}({\dot{T}}^2{F}^2-3{\dot{R}}^2)=0\hfill \\ \hfill K_{\theta \theta }^{-}& =& A{\mathrm{\Gamma }}_{\theta \theta }^{\tau }-aA{\mathrm{\Gamma }}_{\theta \theta }^{\chi }=AR(HR-1)\hfill \\ \hfill K_{\theta \theta }^{+}& =& \dot{T}{\mathrm{\Gamma }}_{\theta \theta }^r=-\dot{T}FR=\pm \dot{R}R\hfill \\ \hfill K_{\delta \delta }^{\pm }& =& {sin}^2\theta K_{\theta \theta }^{\pm }\hfill \end{array}$$

(25)

Thus, the second matching conditions $K_{\alpha \beta }^{-}=K_{\alpha \beta }^{+}$ together with Equation (23) results in:

$$\dot{R}=HR+1;A=\pm \frac{HR+1}{HR-1}$$

(26)

The left hand side is fulfilled for any $H\left(\tau \right)$ as long as $R=a{\chi }_{\ast }$ is a null geodesic (i.e., ${\dot{\chi }}_{\ast }=1/a$), which is our starting point in Equation (23) and agrees with Equation (A25) for $V_0=1$. The right hand side fixes the normalization $A=A\left(\tau \right)$ of $n^{-}$ in ${\mathrm{\Sigma }}^{-}$.

When a is small, the null geodesics $R=R_{\ast }$ in the integral of Equation (32) is dominated by the late time value of $H_{\mathrm{\Lambda }}=1/r_S$ and this means that the FLRW event horizon ${\chi }_{\ast }$ is approximately fixed in co-moving coordinates. This reproduces the junction in Equation (15). Note how $R>r_H$ implies that $R<r_S$, this is illustrated in Figure 1.

On the opposite limit, when H is constant: $H=H_{\mathrm{\Lambda }}=r_{\mathrm{\Lambda }}^{-1}$ we have $\dot{R}=0$ and $R=r_{\mathrm{\Lambda }}=r_H=r_S$. This results in $2\mathrm{\Psi }=2\mathrm{\Phi }=-H^2{R}^2=-r_S/R=-1$ (see Equation (A19)) in the junction $\mathrm{\Sigma }$. This makes sense because dS metric null events are fixed in physical coordinates. Note that we have $p=0$ at $r=R$ because $R_{\ast }>r_H$ (see also Appendix D and Section 4) as required by the Misner and Sharp [10] boundary condition.

2.2.4. The GHY Null Boundary Term

We estimate the GHY boundary term to the action $S_{GHY}$ following the steps in Section 2.2.2. We use the formalism in Ref. [27] for boundaries of null surfaces. This is similar to what we did before with the difference that the induced metric is now 2D instead of 3D:

$$\begin{array}{ccc}\hfill d{s}_{\partial V_4}^2& =& q_{AB}d{z}^{A}d{z}^B=r_S^2{d\mathrm{\Omega }}^2\hfill \end{array}$$

(27)

$$\begin{array}{ccc}\hfill S_{GHY}& =& \frac{1}{8\pi G}{\oint }_{\partial V_4}d\lambda d^2{z}_{\perp }\sqrt{q}(\mathrm{\Theta }+\kappa )\hfill \end{array}$$

(28)

where $\kappa$ is the non-affinity coefficient: $l^a{\nabla }_a{l}_b=\kappa l_b$ and $\mathrm{\Theta }\equiv q^{AB}{\mathrm{\Theta }}_{AB}=\frac{K_{\theta \theta }}{R^2}+\frac{K_{\delta \delta }}{R^2{sin}^2\theta }.$ We can use Equation (25) to find $\mathrm{\Theta }=-2\dot{R}/R=-2\kappa$. So the corresponding trace of the extrinsic curvature is:

$$\mathrm{\Theta }+\kappa =-\frac{\dot{R}}{R}$$

(29)

For $\partial V_4$ we have $R=r_S$ and $\dot{R}=2$. Thus, we recover the same result as Equation (19). From this we can arrive at the same conclusion that the boundary GHY term fixes $r_{\mathrm{\Lambda }}=r_S$.

3. The Observable Universe

One could wonder if an observer that is off-centered and close to the boundary $r=R$ of the FLRW* space could see anisotropies due to the change in the background at $r>R$. We will study this point in this section. Let us start by assuming the infinite FLRW space, as most cosmologist seem to believe, for the $\mathrm{\Lambda }$CDM model (flat FLRW with $\mathrm{\Lambda }\ne 0$). The physical observable Universe is given by the past null cone integral of the FLRW metric in Equation (2):

$$R_O\left(\tau \right)=a\left(\tau \right){\int }_{\tau }^{{\tau }_0}\frac{cd\tau }{a\left(\tau \right)}=a{\int }_a^1\frac{da}{H{a}^2}$$

(30)

This is also what cosmologists call the proper angular diameter distance. It gives the proper distance radius ($r=a\chi$) at time $\tau$ when the light signal was emitted so that it reaches us today at time ${\tau }_0$ or $a=1$ (see Section VI in [28]). This is shown in Figure 2 for the $\mathrm{\Lambda }$CDM model as a dashed green line. We can only see photons emitted along this green line radial trajectory (in all directions). Therefore, this is the observable universe.

Figure 2. Proper radial distances $R=a\left(\tau \right)\chi$ in units of $c/H_0$ as a function of cosmic time (given by the scale factor a) for a flat ${\mathrm{\Omega }}_{\mathrm{\Lambda }}=0.75$ FLRW metric. The Hubble horizon $c/H$ (blue line), is compared to the observable universe $R_o$ in Equation (30) (dashed green line) and the FLRW Event Horizon $R_{\ast }$ in Equation (32) (red line). This figure is the same for an infinite FLRW and for a finite one. The only difference is that in the former we assume that $r>R$ (magenta and grey shaded regions) have the same uniform density as the rest, while in the FLRW cloud we assume them to be (approximately) empty. Direct observations can not tell the difference because those regions are outside our past light-cone.

Figure 2. Proper radial distances $R=a\left(\tau \right)\chi$ in units of $c/H_0$ as a function of cosmic time (given by the scale factor a) for a flat ${\mathrm{\Omega }}_{\mathrm{\Lambda }}=0.75$ FLRW metric. The Hubble horizon $c/H$ (blue line), is compared to the observable universe $R_o$ in Equation (30) (dashed green line) and the FLRW Event Horizon $R_{\ast }$ in Equation (32) (red line). This figure is the same for an infinite FLRW and for a finite one. The only difference is that in the former we assume that $r>R$ (magenta and grey shaded regions) have the same uniform density as the rest, while in the FLRW cloud we assume them to be (approximately) empty. Direct observations can not tell the difference because those regions are outside our past light-cone.

What is striking about the $R_O$ curve is that it is not a monotonic function of $\tau$ (or a): photons reach a maximum radius and then return. This is easy to understand: as we look back around us, the distance travelled by photons increases with increasing redshift $z=1/a-1$. However, because the universe is expanding, the scale factor (and proper distance) decreases with increasing redshift. So there is a point where these two effects cancel each other out and we reach a maximum proper distance. From there on, the expansion (or backwards contraction) dominates until all points merge in the past. Ellis and Rothman [28] use the analogy with gravitational lensing: as we follow our lightcone back into the past, the gravitation of the matter it encloses causes refocusing so it reaches a maximum and then contracts. However, note that there is no light bending here: paths are just stretched by the expansion. In co-moving coordinates $R_0/a$ does monotonically increase with redshift (see Figure 3). A null past geodesic in the FLRW metric: $ds=0$ corresponds to $\dot{\chi }=-1/a$ (the minus sign reflects incoming radial direction) so that:

$$\dot{r}=\dot{a}\chi +a\dot{\chi }=Hr-1$$

(31)

So the maximum radius ($\dot{r}=0$) corresponds to the point where $R_O$ intercepts the Hubble horizon $r=r_H\equiv 1/H$ in our past, shown as a blue line in Figure 2. The maximum occurs at $z\simeq 1.5$ or $R_O\simeq 0.4$. So we have never seem photons from distances larger than about 40% of our Hubble horizon ($c/H_0)$ today, even for an infinite FLRW space.

The red line in Figure 2 shows a null geodesic $R=R_{\ast }$, where $R_{\ast }$ is the FLRW Event Horizon:

$$R_{\ast }\left(a\right)=a{\chi }_{\ast }=a{\int }_a^{\infty }\frac{da}{H{a}^2}<\frac{1}{H_{\mathrm{\Lambda }}}\equiv r_{\mathrm{\Lambda }}$$

(32)

where ${\chi }_{\ast }$ is the corresponding co-moving scale. This is the maximum distance ever travel by a photon (outgoing radial null geodesic or Event Horizon [28] at cosmic time $\tau$ or a). For small a the value of ${\chi }_{\ast }$ is fixed to a constant ${\chi }_{\ast }\simeq 3r_{\mathrm{\Lambda }}$ (this corresponds to a time-like geodesic for a matter dominated universe). Thus, the physical trapped surface radius $R_{\ast }$ increases with time. As we approach $a\simeq 1$ the Hubble rate becomes constant and $R_{\ast }$ freezes to a constant value $R_{\ast }\to r_{\mathrm{\Lambda }}$. No signal from inside $R_{\ast }$ can ever reach outside, just like in the interior of a BH. We will identify $R_{\ast }$ with the size of the FLRW* as indicated by Equation (26), and $r_{\mathrm{\Lambda }}$ with $r_S$ (as explained in Section 2.2.4). For ${\mathrm{\Omega }}_{\mathrm{\Lambda }}\simeq 0.7$ and $H_0\simeq 70$ km/s/Mpc, we have that $R<r_S$, where:

$$r_S=H_0^{-1}{\mathrm{\Omega }}_{\mathrm{\Lambda }}^{-1/2}\simeq 1.6\times {10}^{23}\mathrm{km};M=\frac{r_S}{2G}\simeq 5.5\times {10}^{22}{M}_{\odot }$$

(33)

This bound on $R<r_S$ might seem puzzling at first because $R=a\left(\tau \right)\chi$, so that for a fixed co-moving coordinate $\chi$, the R coordinate can grow unbounded as $a\left(\tau \right)$ grows to infinity. However, note that as $a\left(\tau \right)$ grows to infinite the integral in Equation (32) (which represents the co-moving distance, ${\chi }_{\ast }$, travel by a photon) goes to zero, because there is increasingly less time available for the photon to travel. Another way to understand this is to look at Figure A1 or Equation (A20) where we can see that a given fixed coordinate distance $\chi$ in dS space acts like an Horizon: proper coordinates r and proper time $\tau$, asymptotically freeze for $t\to \infty$.

Given that $R_O<R$, as indicated by Figure 2, can we distinguish with observations an infinite FLRW universe from a finite FLRW* one? Let us try to answer this question next.

3.1. The Black Hole Universe

Even when we have assumed the standard infinite FLRW, we still have something that resembles very much the inside of a BHU within FLRW*. The first thing to notice is that $R=r_{\mathrm{\Lambda }}\equiv 1/H_{\mathrm{\Lambda }}$ corresponds to $R=r_S=2GM$ in Equation (4). The observed H tends to a constant $H=H_{\mathrm{\Lambda }}$ so that $\rho$ becomes static. So we can identify $r_{\mathrm{\Lambda }}$ with $r_S$. Following Birkoff’s theorem, if we approximate the outside of $r_{\mathrm{\Lambda }}$ to be empty space, we will then have a SBH space outside. This is the definition of a BH. However, note that it does not have the same interior as the static SBH. The interior is the FLRW metric and is only static in the limit $r_H\to r_{\mathrm{\Lambda }}$.

More generally, $r_H$ behaves like an apparent BH event horizon (see also Ref. [29]) as the energy-mass inside $r<r_H$ is always $2GM$ in Equation (4) and the density is the same as the density of a BH in Equation (1). It is only apparent because $r_H$ is moving as the universe expands. However, observations tell us that H approaches a constant value and we have that asymptotically $r_H\to r_{\mathrm{\Lambda }}=R_{\ast }$ (see Figure 2) so that the FLRW event horizon in Equation (32) becomes a static BH. This is what we call the BHU.

We can also find the same result if we look at this from the prespective of deSitter space. The infinite $\mathrm{\Lambda }$CDM universe asymptotically tends to a deSitter (dS) space. There is a frame duality for a dS space (i.e., Equation (A20)) that allows us to equivalently describe the dS Universe as either static (in proper coordinates) or exponentially expanding (in co-moving coordinates). In proper coordinates the Universe is static with a radial metric element: $g_{rr}={(1-H_{\mathrm{\Lambda }}^2{r}^2)}^{-1}$ and uniform density $\rho$. The region $r<1/H_{\mathrm{\Lambda }}$ is causally disconnected from $r>1/H_{\mathrm{\Lambda }}$ (see Equation (A14)). The energy-mass inside this region corresponds to that of a BH inside $r_S=2$ GM, where M in Equation (4) is the energy-mass inside $1/H_{\mathrm{\Lambda }}$. However, for this to be a BH we need the outside $r>1/H_{\mathrm{\Lambda }}$ to be empty. In the infinite FLRW universe the outside should have the same density as the inside. As shown in previous subsection we can not observationally tell (at least from the center) if there is or there is no matter outside $r_{\mathrm{\Lambda }}$ because that region of space-time is causally disconnected from the rest. Moreover, as we have already seen, Birkhoff’s corollary tell us that it does not matter what is outside if we have spherical symmetry.

This means that if we are at the centre of such a BH, we can not distinguish between the finite and the infinite FLRW spaces. So you may argue that this is not a scientific question [1]. However there are other considerations. A constant density everywhere, independent of time, corresponds to the Steady State Universe solution (similar to that originally proposed by Einstein) and is not asymptotically flat at infinity. In fact dS metric becomes singular at infinity. In that respect, the BHU solution is more easy to understand and implement given that empty space is asymptotically flat. In the FLRW*, the region of the Universe with content is finite, which avoids the need to explain how to place matter at infinite distances in a finite amount of time [25,26]. Having an asymptotically flat space also helps defining the mass and avoids some inconsistencies of the dS interpretation (see also Appendix D).

We thus conclude that if we are at the centre of such BH, we can not tell if we have a finite or an infinite FLRW space, just because $R_O<R$. However, what happens when the observer is not at the centre of the BH?

3.2. Off-Centred Co-Moving Observer

The metric in Equation (2) is the same for the infinite or the finite FLRW model, as long as $a\left(\tau \right)$ is the same, which only requires that the energy density inside R is the same. The only difference is given by the coordinate restriction $r=a\chi <R\left[a\right]$ for the FLRW cloud. Both spaces are therefore equivalent for an observer whose past light-cone does not intersect $R\left(a\right)$. In the discussion and in part II of this paper we will mention possible observational differences related to the local nature of the FLRW cloud and the origin of fluctuations. Here we will only discuss if an observer sees the same background.

Let us now assume a co-moving observer at our time ${\tau }_0$ ($a=1$) but located at some arbitrary position inside the FLRW cloud. Without lost of generality, we can still choose its angular coordinates to be at the origin and only study the radial constraints. Let us call $r_0=a{\chi }_0$ the radial proper coordinate where the off-centred observer is located. We then have by construction that ${\chi }_0<R[a=1]$, as we want the observer to be co-moving with the matter inside. If we ignore the fact that there is a boundary $r<R\left[a\right]$ (that moves in time), the observable universe $R_O$ around $r_0$ is obviously the same as the one in Equation (30), because the FLRW cloud is uniform and it has the same dynamics as the $\mathrm{\Lambda }$CDM universe everywhere inside R. This can also be understood as a simple shift of the radial coordinates. The condition that the null past light-cone of $r_0$ does not cross $R\left[a\right]$ anytime in the past is then:

$$R\left[a\right]>R_O\left[a\right]+r_0$$

(34)

As argued before (e.g., in Equation (26)), R corresponds to the FLRW event horizon $R\left[a\right]=R_{\ast }\left[a\right]$ in Equation (32). Using Equation (30) for $R_O\left[a\right]$ and Equation (32) for $R\left[a\right]$ we then have that Equation (34) becomes:

$${\chi }_0<{\int }_1^{\infty }\frac{da}{H{a}^2}=R[a=1]$$

(35)

This condition is clearly fulfilled because, by construction, $r_0$ needs to be inside R today: ${\chi }_0<R(a=1)$.

As the off-centred observer looks back, the observable past light-cone $R_O$ grows, but R grows by the same amount, so no photons from the boundary $r>R$ can reach ${\chi }_0$. Figure 3 illustrates this result. We thus conclude that no matter where the off-centred observer is located within the FLRW cloud today, the observer will see the same background universe as the one seen by an observer located in the centre.

4. Discussion and Conclusions

The solution in Equation (A22) corresponds to what we here call a FLRW cloud (FLRW*). That such FLRW* solutions exist is well known since Friedmann and Lemaitre, see Section 2.1. When $R<r_S$ we call it a Black Hole Universe (BHU). Such a BH is not static inside, which explains why it avoids the constraint $R>9r_S/8$ in Ref. [4]. We have studied the junction conditions (see Section 2.2) to show that the joint manifold (FLRW+SBH) is also a solution to EFE and there are no surface terms in the junction.

Contrary to the standard Cosmological model of the infinite FLRW space or $\mathrm{\Lambda }$CDM universe, here we have assumed that the background outside R is flat with $k=0$ and $\mathrm{\Lambda }=0$, as in empty space. The field equations of GR are local and can not change k or $\mathrm{\Lambda }$, which are global geometrical values, regardless of the matter or $T_{\mu \nu }$ content. We therefore adopt the most simple topology, that of empty space, unless we find some evidence or good reason to the contrary. So we do not expect a global curvature or $\mathrm{\Lambda }$ in the BHU (this will be discussed further in paper II of the BHU series). As it happens with the exterior of the SBH space, the exterior metric of the BHU does not need to be exactly empty and could also be a perturbation inside a lower density FLRW or a dS metric (e.g., see Ref. [30] and Equation (A7) or Equation (A15)). So in the BHU we could have two nested FLRW manifolds (as in "Application e)" of Ref. [14]). This is illustrated in the bottom right of Figure A2. We can have smaller BHs inside larger BHs or smaller FLRW submanifolds inside larger FLRW universes. Mathematically this looks like a Matryoshka (or nesting) doll [31] or a fractal structure [32]. However, physically, each BH has a different energy-mass and therefore different physical properties, expansion time, and internal structure.

The fact that the universe might be generated from the inside of a BH has been studied extensively in the literature [33,34,35,36,37,38]. Most of these previous approaches involve modifications to Classical GR and will not be discussed here. There are also some simple scalar field $\phi \left(x\right)$ examples (e.g., Ref. [35]) which presented models within the scope of a classical GR and classical field theory with a false vacuum interior. A particular case are Bubble or Baby Universe solutions where the BH interior is de-Sitter metric [3,6,39,40,41,42,43]. The BHU solution is similar, but has some important differences. In the BHU, no surface term (or Bubble) is needed and the matter and radiation inside are regular–not just false vacuum solutions. There is no need for a false vacuum in the BHU because $r_S$ plays the role of $\mathrm{\Lambda }$. In this respect, the BHU is not quite a Bubble Universe.

A deSitter BH interior without bubble has been proposed before by several authors (e.g., Refs. [44,45]). However, the idea has been criticised by Poisson and Israel [46], who argued that such a combination is not possible because of the O’Brien–Synge junction condition, which requires $p=0$ in the junction, as already pointed out by Misner and Sharp [10]. Another source of concern comes from the fact that the resulting deSitter+SW solution represents a non singular BH, which seems to contradict Penrose’s singularity theorems [47] (however, see also Ref. [48] who argues that this matter is not settled yet). Unlike the deSitter metric, the BHU (or the FLRW*) metric is not static and has a past singularity. The BHU becomes deSitter only asymptotically when $R\to r_S$. In that limit we have argued here that $r_S$ becomes a boundary to the Einstein–Hilbert action (see Equations (A4) and (20)), because nothing can escape $r_S$. So there is no action or pressure in the junction. More generally, because of causality, perturbations freeze out for separations $r>r_H$.

In the 1970s Pathria [31] and Good [49] proposed that the FLRW space could be the interior of a BH. However, these were not proper GR solutions, but just incomplete analogies (see Ref. [50]). The model by Zhang [32] has the same name and similar features to our BHU proposal, but it is built as a new postulate to GR and not as a solution to a classical GR problem. Our BHU solution is quite different from that of Ref. [33], who just speculated that all final (e.g., BH) singularities ’bounce’ or tunnel to initial singularities of new universes. The solution by Stuckey [51] with $\mathrm{\Lambda }=0$ agrees well with the time-like junction in Section 2.2.1, but does not interpret the observed cosmic acceleration as resulting from $r_S$. Poplawski [52] proposed the torsion in the Einstein–Cartan gravity model with $\mathrm{\Lambda }$ to generate a non singular bounce that results in a BH universe. We instead use Classical GR without torsion and argue that $\mathrm{\Lambda }$ is not needed once you realise that you are inside a BH that produces the same effect.

Criticising some of these previous results, Knutsen [50] argued that p and $\rho$ in the homogeneous (infinite) FLRW solution are only a function of co-moving time and can not change at $r=r_S$ to become zero in the exterior. However, this is no longer the case for the FLRW* solution discussed here, where we have an inhomogeneous local universe.

As shown in Section 3, a co-moving observer located anywhere inside a local FLRW* sees the same background as a co-moving observer of an infinite FLRW universe with equal density content. We can also understand this curious behaviour in the dual frame by considering radial null events in deSitter metric, which follow Equation (A14). If the FLRW cloud is moving or rotating within a larger background, this could show as a dipole in the CMB. Such a dipole has already been observed, but it is usually interpreted as a local flow. This interpretation has recently been challenged by new observations of our local neighbourhood (see [53]).

The BHU solution does not require a $\mathrm{\Lambda }$ term or Dark Energy to explain cosmic acceleration. As shown in Section 2.2.2, the BH event horizon $r_S$ corresponds to a boundary in the action, which has the same effect as $\mathrm{\Lambda }$. Note how this is quite different from the LBT model [54], which tries to explain cosmic acceleration with a local spherical void centred around us. You could in principle picture our FLRW* as an LBT model with a central over-density (instead of a void) where the outside background ($r>R$) is empty. This is quite different in scale and signature from the LBT. Moreover, the LBT provides a smooth transition between the two backgrounds while in the BHU the two backgrounds are causally separated by an event horizon ($R=R_{\ast }$ in Equation (32)). Due to all these differences, the observation constraints that defeat the LBT model (see Ref. [54]) do not affect the BHU, which has the same observed background as the $\mathrm{\Lambda }$CDM universe.

While we can not differentiate with background observations in our past light-cone between an infinite and finite FLRW, an observer could in principle detect differences between spaces by measuring tidal forces. However, note that both spaces are identical in the internal submanifold and we find no defects or discontinuities in the junction with the external SBH space (see Section 2.2). The yellow region in Figure 1 and Figure 2 contains primordial frozen perturbations, which we can observe today, and could be different in each model, depending on the formation mechanism. In paper II we will address the issue of how the BHU forms. This will provide an observational window to distinguish the BHU from the standard Big Bang model that emerged out of Cosmic Inflation. We can observe transverse perturbations in the CMB that are larger than R. At the time of CMB last scattering, R corresponds to an angle $\theta ={\chi }_{\ast }/{\chi }_o\simeq 60$ deg. Such super-horizon scales could be related to the so-called CMB anomalies, deviations with respect to inflationary predictions from $\mathrm{\Lambda }$CDM (see Refs. [26,55,56,57,58,59,60] and references therein), or the apparent tensions in measurements from different cosmic scales or times [61]. The BHU can also be challenged by a measurement a the DE equation of state $\omega \ne -1$. This would indicate that cosmic acceleration is not solely caused by the BHU event horizon $r_S$.

The BHU model allows for a Perfect Cosmological Principle, the one advocated by Einstein (when he introduced $\mathrm{\Lambda }$) and the Steady State Cosmology [62,63,64]. However, there is no need for ad hoc matter creation (the C-field) to explain the observed cosmic expansion. The frame duality in Equation (A19) explains how we can have at the same time an expanding universe in co-moving coordinates (as observed by the Hubble–Lemaitre law) and an asymptotically static BHU in the outside Schwarzschild frame.