Liouville Theory for Fully Analytic Studies of Transverse Beam Dynamics in Laser-Plasma Ion Accelerators
Abstract
:1. Introduction
2. Methods
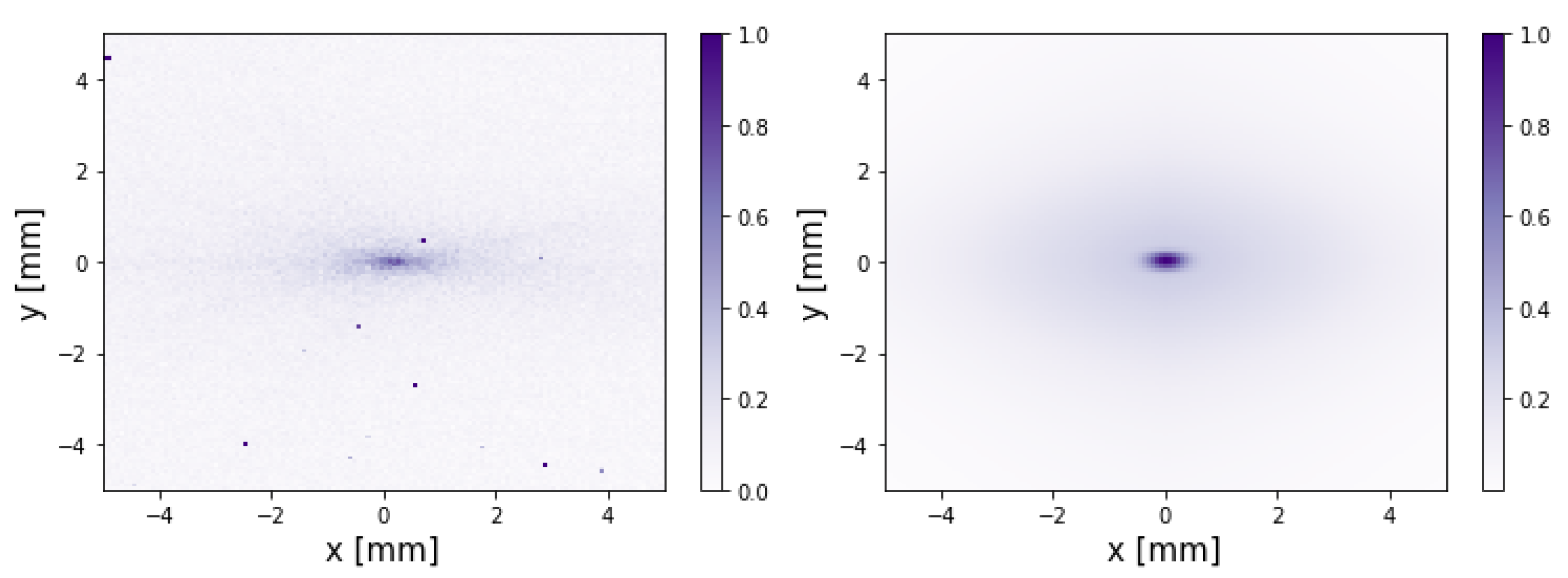
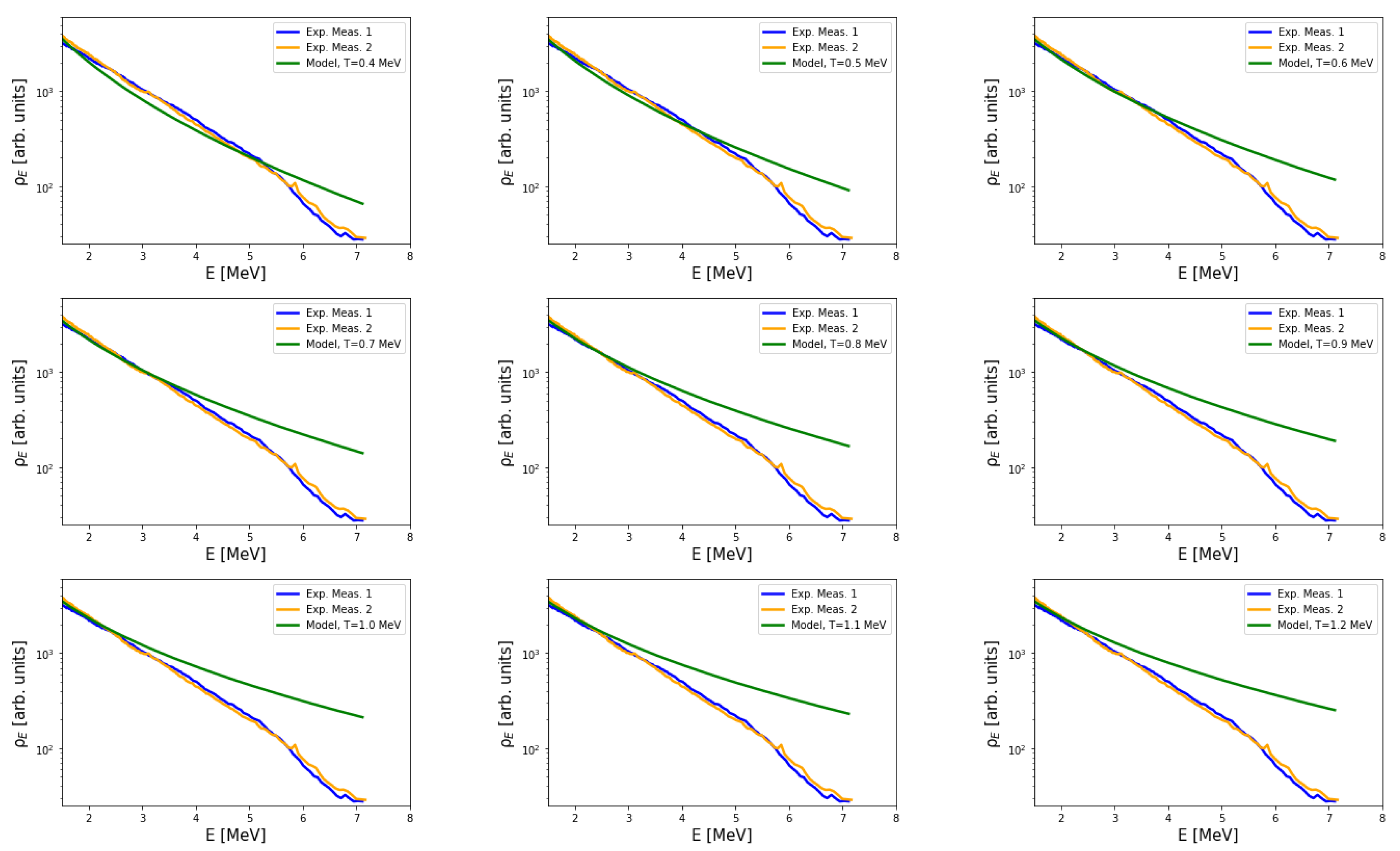
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Liouville Equation with Aperture Losses
Appendix B. Single-Particle Dynamics for the Case Relevant to This Paper
References
- Brüning, O.; Rossi, L. (Eds.) The High Luminosity Large Hadron Collider: The New Machine for Illuminating the Mysteries of Universe; World Scientific Publishing Company: Singapore, 2015; Volume 24. [Google Scholar]
- Muramatsu, M.; Kitagawa, A. A review of ion sources for medical accelerators. Rev. Sci. Instrum. 2012, 83, 02B909. [Google Scholar] [CrossRef]
- Hamm, R.W.; Hamm, M.E. (Eds.) Industrial Accelerators and Their Applications; World Scientific Publishing Company: Singapore, 2012. [Google Scholar]
- Amaldi, U. The importance of particle accelerators. Europhys. News 2000, 31, 5–9. [Google Scholar] [CrossRef]
- Morabito, A.; Scisciò, M.; Veltri, S.; Migliorati, M.; Antici, P. Design and optimization of a laser-PIXE beamline for material science applications. Laser Part. Beams 2019, 37, 354–363. [Google Scholar] [CrossRef]
- Macchi, A.; Borghesi, M.; Passoni, M. Ion acceleration by superintense laser-plasma interaction. Rev. Mod. Phys. 2013, 85, 751. [Google Scholar] [CrossRef]
- Passoni, M.; Bertagna, L.; Zani, A. Target normal sheath acceleration: Theory, comparison with experiments and future perspectives. New J. Phys. 2010, 12, 045012. [Google Scholar] [CrossRef]
- Apiñaniz, J.I.; Malko, S.; Fedosejevs, R.; Cayzac, W.; Vaisseau, X.; De Luis, D.; Gatti, G.; McGuffey, C.; Bailly-Grandvaux, M.; Bhutwala, K.; et al. A quasi-monoenergetic short time duration compact proton source for probing high energy density states of matter. Sci. Rep. 2021, 11, 6881. [Google Scholar] [CrossRef]
- Chen, S.N.; Gauthier, M.; Higginson, D.P.; Dorard, S.; Mangia, F.; Riquier, R.; Atzeni, S.; Marquès, J.R.; Fuchs, J. Monochromatic short pulse laser produced ion beam using a compact passive magnetic device. Rev. Sci. Instrum. 2014, 85, 043504. [Google Scholar] [CrossRef]
- Wu, M.; Zhu, J.; Li, D.; Yang, T.; Liao, Q.; Geng, Y.; Xu, X.; Li, C.; Shou, Y.; Zhao, Y.; et al. Collection and focusing of laser accelerated proton beam by an electromagnetic quadrupole triplet lens. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrometers Detect. Assoc. Equip. 2020, 955, 163249. [Google Scholar] [CrossRef]
- Schollmeier, M.; Becker, S.; Geißel, M.; Flippo, K.A.; Blažević, A.; Gaillard, S.A.; Gautier, D.C.; Grüner, F.; Harres, K.; Kimmel, M.; et al. Controlled transport and focusing of laser-accelerated protons with miniature magnetic devices. Phys. Rev. Lett. 2008, 101, 055004. [Google Scholar] [CrossRef]
- Brandi, F.; Labate, L.; Palla, D.; Kumar, S.; Fulgentini, L.; Koester, P.; Baffigi, F.; Chiari, M.; Panetta, D.; Gizzi, L.A. A Few MeV Laser-Plasma Accelerated Proton Beam in Air Collimated Using Compact Permanent Quadrupole Magnets. Appl. Sci. 2021, 11, 6358. [Google Scholar] [CrossRef]
- Burris-Mog, T.; Harres, K.; Nürnberg, F.; Busold, S.; Bussmann, M.; Deppert, O.; Hoffmeister, G.; Joost, M.; Sobiella, M.; Tauschwitz, A.; et al. Laser accelerated protons captured and transported by a pulse power solenoid. Phys. Rev. Spec. Top.-Accel. Beams 2011, 14, 121301. [Google Scholar] [CrossRef]
- Masood, U.; Bussmann, M.; Cowan, T.E.; Enghardt, W.; Karsch, L.; Kroll, F.; Schramm, U.; Pawelke, J. A compact solution for ion beam therapy with laser accelerated protons. Appl. Phys. B 2014, 117, 41–52. [Google Scholar] [CrossRef]
- Busold, S.; Schumacher, D.; Deppert, O.; Brabetz, C.; Frydrych, S.; Kroll, F.; Joost, M.; Al-Omari, H.; Blažević, A.; Zielbauer, B.; et al. Focusing and transport of high-intensity multi-MeV proton bunches from a compact laser-driven source. Phys. Rev. Spec. Top.-Accel. Beams 2013, 16, 101302. [Google Scholar] [CrossRef]
- Ter-Avetisyan, S.; Schnürer, M.; Polster, R.; Nickles, P.V.; Sandner, W. First demonstration of collimation and monochromatisation of a laser accelerated proton burst. Laser Part. Beams 2008, 26, 637–642. [Google Scholar] [CrossRef]
- Kroll, F.; Brack, F.E.; Bernert, C.; Bock, S.; Bodenstein, E.; Brüchner, K.; Cowan, T.E.; Gaus, L.; Gebhardt, R.; Helbig, U.; et al. Tumour irradiation in mice with a laser-accelerated proton beam. Nat. Phys. 2022, 18, 316–322. [Google Scholar] [CrossRef]
- Brown, A.; Suit, H. The centenary of the discovery of the Bragg peak. Radiother. Oncol. 2004, 73, 265–268. [Google Scholar] [CrossRef]
- Flottmann, K.; Lidia, S.; Piot, P. Recent Improvements to the ASTRA Particle Tracking Code (No. LBNL-52933); Lawrence Berkeley National Lab. (LBNL): Berkeley, CA, USA, 2003. [Google Scholar]
- Borland, M. Elegant: A Flexible SDDS-Compliant Code for Accelerator Simulation (No. LS-287); Argonne National Lab.: Argonne, IL, USA, 2000. [Google Scholar]
- Curcio, A.; Panas, R.; Knafel, M.; Wawrzyniak, A.I. Liouville theory for fully analytic studies of longitudinal beam dynamics and bunch profile reconstruction in dispersive lines. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrometers Detect. Assoc. Equip. 2021, 986, 164755. [Google Scholar] [CrossRef]
- Babusci, D.; Dattoli, G.; Quattromini, M.; Sabia, E. Relativistic harmonic oscillator, the associated equations of motion, and algebraic integration methods. Phys. Rev. E 2013, 87, 033202. [Google Scholar] [CrossRef]
- Fuchs, J.; Antici, P.; d’Humières, E.; Lefebvre, E.; Borghesi, M.; Brambrink, E.; Cecchetti, C.A.; Kaluza, M.; Malka, V.; Manclossi, M.; et al. Laser-driven proton scaling laws and new paths towards energy increase. Nat. Phys. 2006, 2, 48–54. [Google Scholar] [CrossRef]
- Volpe, L.; Fedosejevs, R.; Gatti, G.; Pérez-Hernández, J.A.; Méndez, C.; Apiñaniz, J.; Vaisseau, X.; Salgado, C.; Huault, M.; Malko, S.; et al. Generation of high energy laser-driven electron and proton sources with the 200 TW system VEGA 2 at the Centro de Laseres Pulsados. High Power Laser Sci. Eng. 2019, 7, e25. [Google Scholar] [CrossRef] [Green Version]
- Salgado-López, C.; Apiñaniz, J.I.; Henares, J.L.; Pérez-Hernández, J.A.; de Luis, D.; Volpe, L.; Gatti, G. Angular-Resolved Thomson Parabola Spectrometer for Laser-Driven Ion Accelerators. Sensors 2022, 22, 3239. [Google Scholar] [CrossRef] [PubMed]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Curcio, A.; Apiñaniz Aginako, J.I.; Cebriano Ramírez, T.; Ehret, M.; Kebladj, B.; Morabito, A.; Pérez Delgado, A.; Salgado López, C.; Volpe, L.; Gatti, G. Liouville Theory for Fully Analytic Studies of Transverse Beam Dynamics in Laser-Plasma Ion Accelerators. Symmetry 2022, 14, 1875. https://doi.org/10.3390/sym14091875
Curcio A, Apiñaniz Aginako JI, Cebriano Ramírez T, Ehret M, Kebladj B, Morabito A, Pérez Delgado A, Salgado López C, Volpe L, Gatti G. Liouville Theory for Fully Analytic Studies of Transverse Beam Dynamics in Laser-Plasma Ion Accelerators. Symmetry. 2022; 14(9):1875. https://doi.org/10.3390/sym14091875
Chicago/Turabian StyleCurcio, Alessandro, Jon Imanol Apiñaniz Aginako, Teresa Cebriano Ramírez, Michael Ehret, Berkahoum Kebladj, Antonia Morabito, Alberto Pérez Delgado, Carlos Salgado López, Luca Volpe, and Giancarlo Gatti. 2022. "Liouville Theory for Fully Analytic Studies of Transverse Beam Dynamics in Laser-Plasma Ion Accelerators" Symmetry 14, no. 9: 1875. https://doi.org/10.3390/sym14091875




