Symmetric Strange Attractors: A Review of Symmetry and Conditional Symmetry
Abstract
1. Introduction
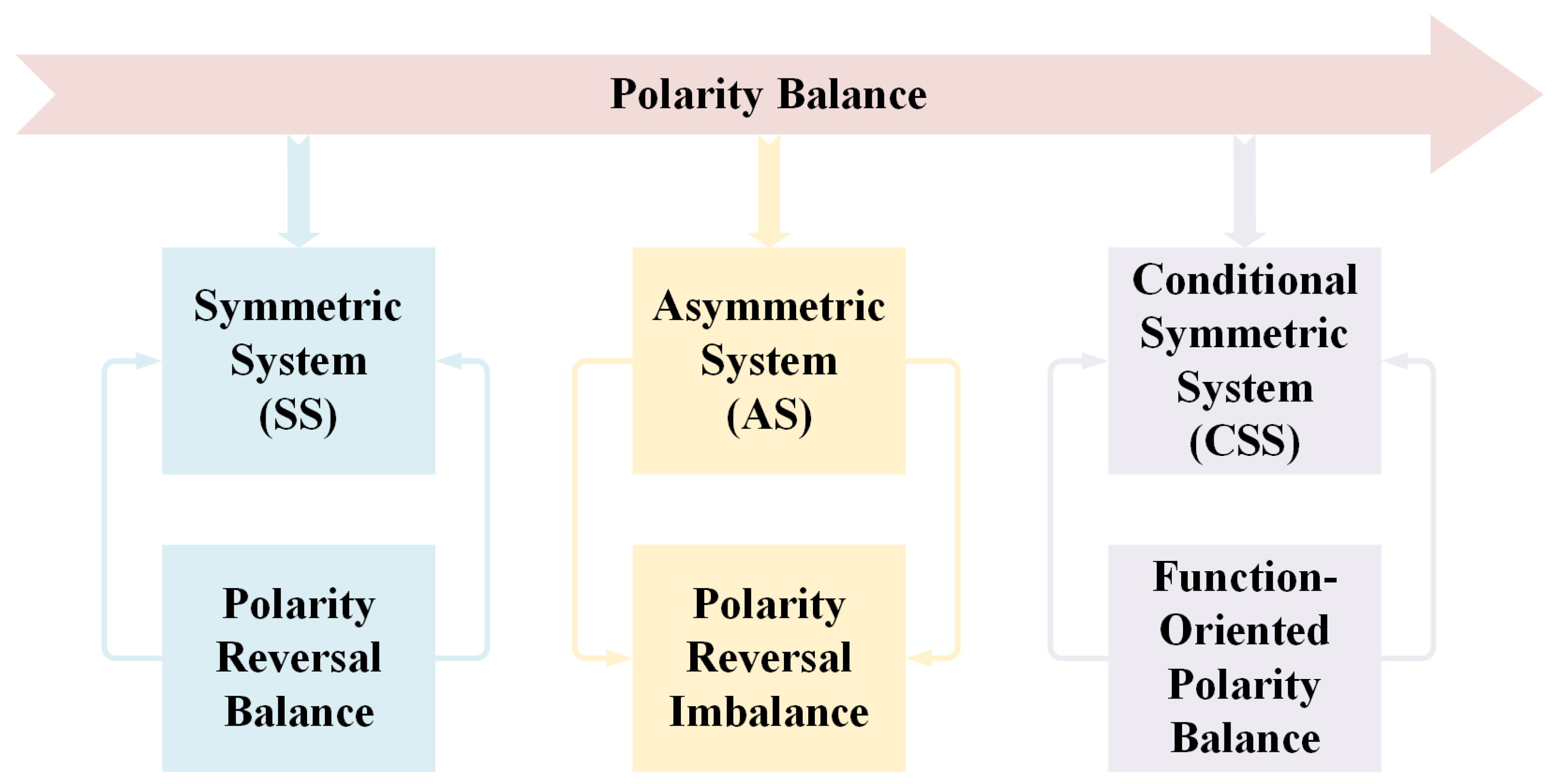
2. Symmetric Strange Attractors and Symmetric Pairs of Coexisting Attractors
2.1. Various Regimes of Symmetry
2.2. Multiple Modes of Coexisting Attractors in a Symmetric System
2.3. Diversities of Stability in Symmetric Systems
3. Offset Boosting for Symmetric Pairs of Strange Attractors
- (I)
- System (6) is one of symmetry according to the dimension of ;
- (II)
- System (6) has 2 m coexisting attractors ;
- (III)
- All the attractors in system (6) share the same structure with the ones in system (5), and all the equilibria in system (6) have the same stabilities with those of system (5).
4. Coexisting Strange Attractors of Conditional Symmetry
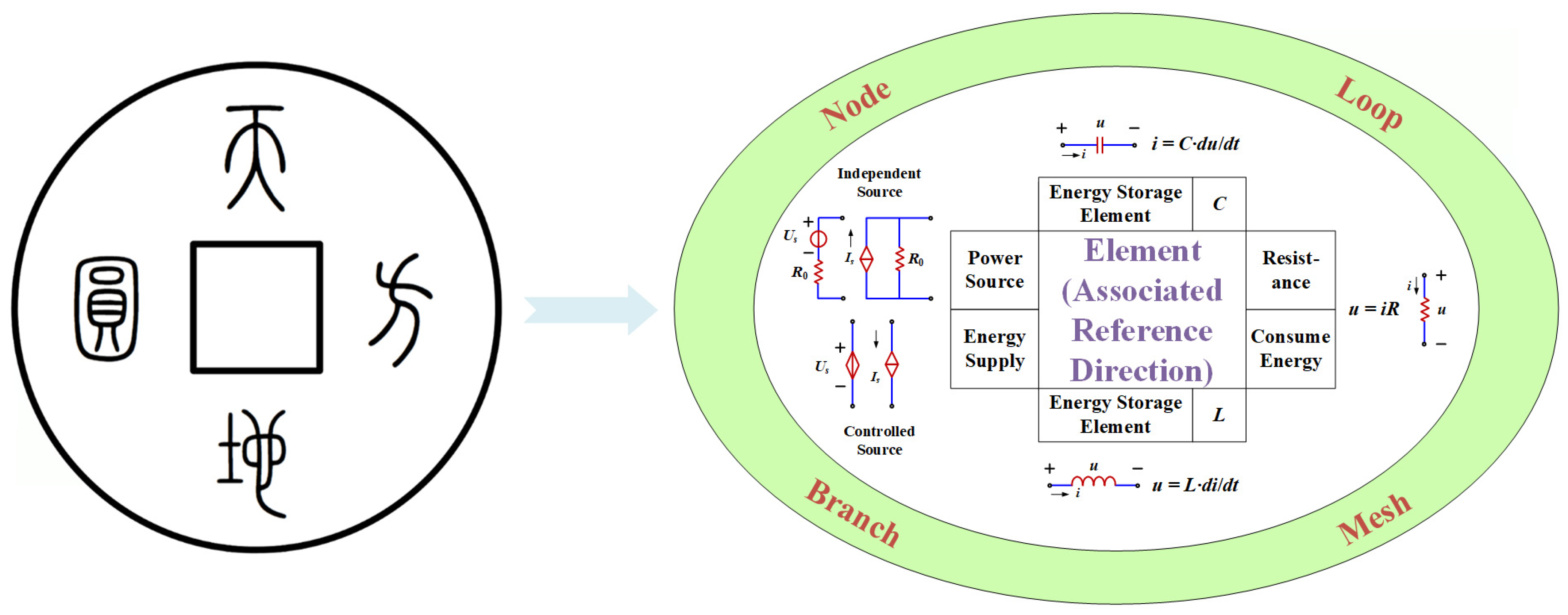
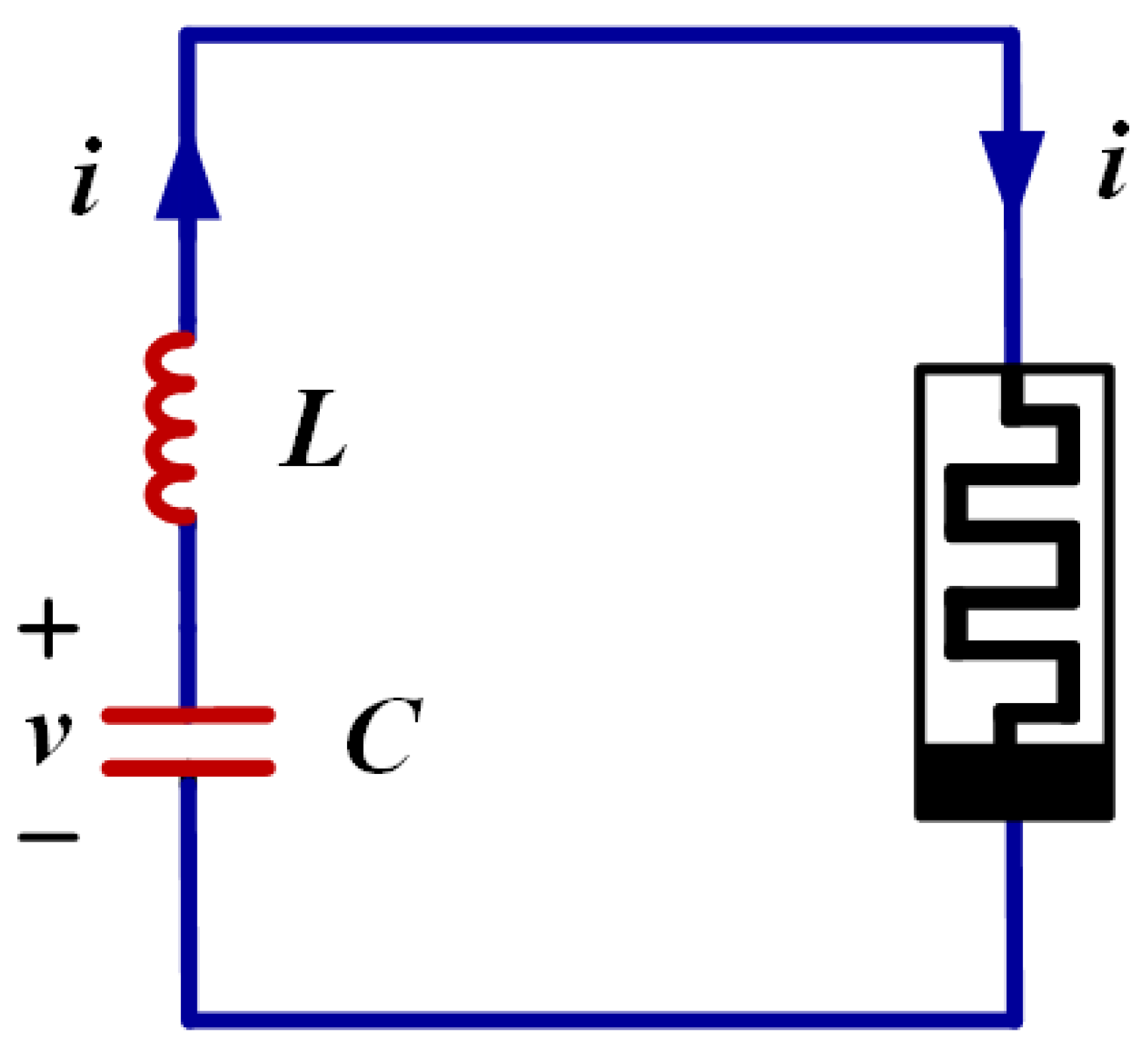
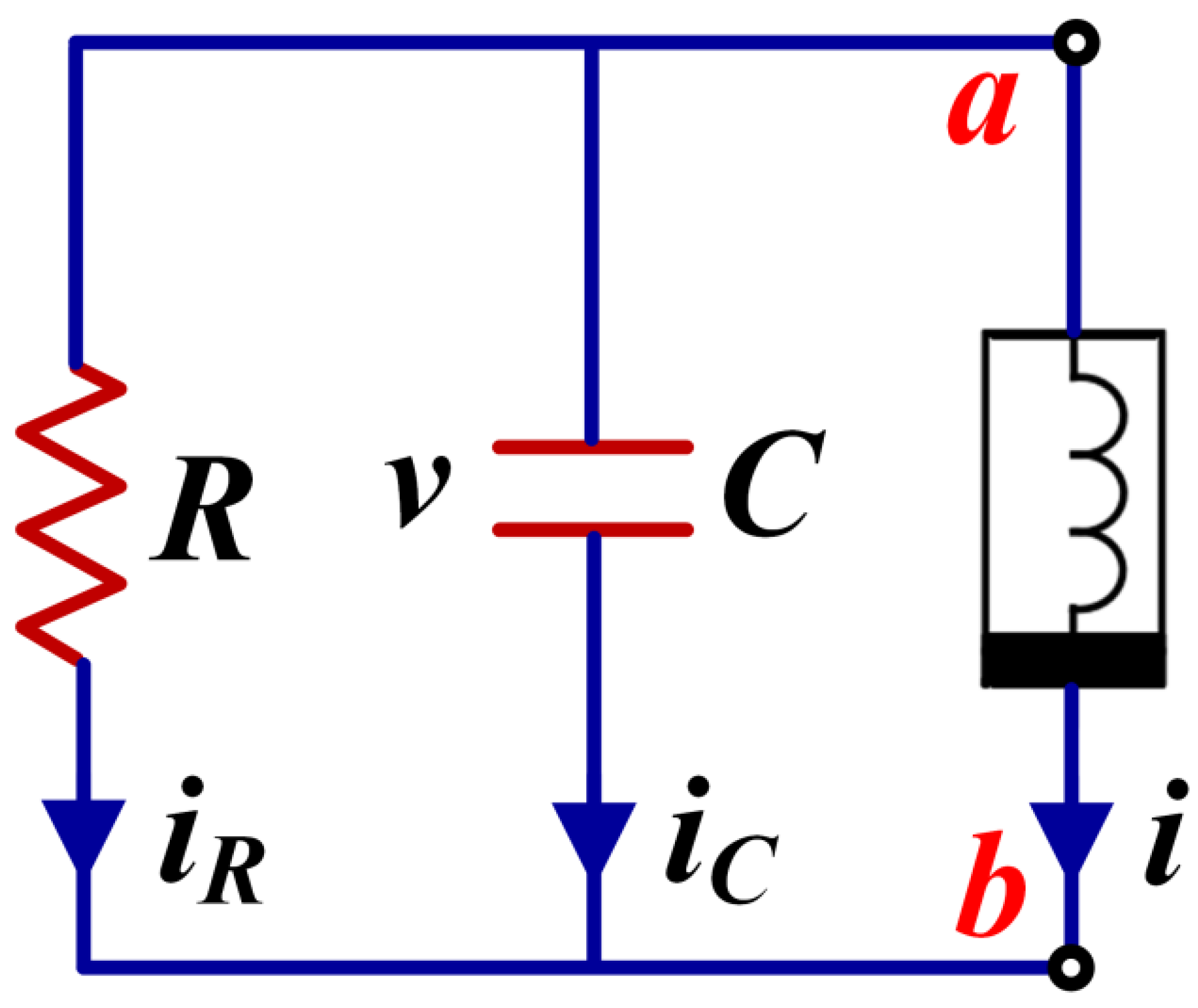
5. Symmetry and Elegance in Simple Chaotic Circuits
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Chen, G.; Ueta, T. Yet another chaotic attractor. Int. J. Bifurcat. Chaos 1999, 9, 1465–1466. [Google Scholar] [CrossRef]
- Chua, L.E.; Komuro, M.; Matsumoto, T. The double scroll family. IEEE Trans. Circuits Syst. 1986, 33, 1072–1118. [Google Scholar] [CrossRef]
- Mobayen, S.; Volos, C.; Çavuşoğlu, Ü.; Kaçar, S. A simple chaotic flow with hyperbolic sinusoidal function and its application to voice encryption. Symmetry 2020, 12, 2047. [Google Scholar] [CrossRef]
- Zhang, Z.; Huang, L.; Liu, J.; Guo, Q.; Du, X. A new method of constructing cyclic symmetric conservative chaotic systems and improved offset boosting control. Chaos Solitons Fractals 2022, 158, 112103. [Google Scholar] [CrossRef]
- Khan, J.; Hussain, T.; Mlaiki, N.; Fatima, N. Symmetries of locally rotationally symmetric Bianchi type V spacetime. Results Phys. 2023, 44, 106143. [Google Scholar] [CrossRef]
- Hruda, L.; Kolingerová, I.; Lávička, M.; Maňák, M. Rotational symmetry detection in 3D using reflectional symmetry candidates and quaternion-based rotation parameterization. Comput. Aided Geom. Des. 2022, 98, 102138. [Google Scholar] [CrossRef]
- Boui, A.; Boya, B.F.; Danao, A.A.; Kengne, L.K.; Kengne, J. Control and symmetry breaking aspects of a geomagnetic field inversion model. Chaos 2023, 33, 013139. [Google Scholar] [CrossRef]
- Leutcho, G.D.; Wang, H.; Kengne, R.; Kengne, L.K.; Njitacke, Z.T.; Fozin, T.F. Symmetry-breaking, amplitude control and constant Lyapunov exponent based on single parameter snap flows. Eur. Phys. J. Spec. Top. 2021, 230, 1887–1903. [Google Scholar] [CrossRef]
- Karthikeyan, R.; Jafari, S.; Karthikeyan, A.; Srinivasan, A.; Ayele, B. Hyperchaotic Memcapacitor Oscillator with Infinite Equilibria and Coexisting Attractors. Circuits Syst. Signal Process. 2018, 37, 3702–3724. [Google Scholar]
- Volos, C.K.; Kyprianidis, I.M.; Stouboulos, I.N. Various synchronization phenomena in bidirectionally coupled double scroll circuits. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 3356–3366. [Google Scholar] [CrossRef]
- Hu, W.; Liu, T.; Han, Z. Dynamical Symmetry Breaking of Infinite-Dimensional Stochastic System. Symmetry 2022, 14, 1627. [Google Scholar] [CrossRef]
- Ramamoorthy, R.; Rajagopal, K.; Leutcho, G.D.; Krejcar, O.; Namazi, H.; Hussain, I. Multistable dynamics and control of a new 4D memristive chaotic Sprott B system. Chaos Solitons Fractals 2022, 156, 111834. [Google Scholar] [CrossRef]
- Bloch, A.M.; Krishnaprasad, P.S.; Marsden, J.E.; Murray, R.M. Nonholonomic mechanical systems with symmetry. Arch. Ration. Mech. Anal. 1996, 136, 21–99. [Google Scholar] [CrossRef]
- Olfati-Saber, R. Normal forms for underactuated mechanical systems with symmetry. IEEE Trans. Autom. Control 2002, 47, 305–308. [Google Scholar] [CrossRef]
- Leonard, N.E.; Marsden, J.E. Stability and drift of underwater vehicle dynamics: Mechanical systems with rigid motion symmetry. Phys. D Nonlinear Phenom. 1997, 105, 130–162. [Google Scholar] [CrossRef]
- Mory, J.F. Oil prices and economic activity: Is the relationship symmetric? Energy J. 1993, 14, 151–161. [Google Scholar] [CrossRef]
- Bischi, G.I.; Gallegati, M.; Naimzada, A. Symmetry-breaking bifurcations and representativefirm in dynamic duopoly games. Ann. Oper. Res. 1999, 89, 252–271. [Google Scholar] [CrossRef]
- Kondepudi, D.K.; Nelson, G.W. Chiral-symmetry-breaking states and their sensitivity in nonequilibrium chemical systems. Phys. A Stat. Mech. Its Appl. 1984, 125, 465–496. [Google Scholar] [CrossRef]
- Piñeros, W.D.; Tlusty, T. Spontaneous chiral symmetry breaking in a random driven chemical system. Nat. Commun. 2022, 13, 2244. [Google Scholar] [CrossRef]
- Kondepudi, D.K.; Nelson, G.W. Chiral symmetry breaking in nonequilibrium chemical systems: Time scales for chiral selection. Phys. Lett. A 1984, 106, 203–206. [Google Scholar] [CrossRef]
- Amit, D.J.; Tsodyks, M.V. Quantitative study of attractor neural networks retrieving at low spike rates: II. Low-rate retrieval in symmetric networks. Netw. Comput. Neural Syst. 1991, 2, 275–294. [Google Scholar] [CrossRef]
- Cho, M.W.; Chun, M.Y. Two symmetry-breaking mechanisms for the development of orientation selectivity in a neural system. J. Korean Phys. Soc. 2015, 67, 1661–1666. [Google Scholar] [CrossRef]
- Meyra, A.G.; Zarragoicoechea, G.J.; Kuz, V.A. Self-organization of plants in a dryland ecosystem: Symmetry breaking and critical cluster size. Phys. Rev. E 2015, 91, 052810. [Google Scholar] [CrossRef]
- Persson, L.; de Roos, A.M. Symmetry breaking in ecological systems through different energy efficiencies of juveniles and adults. Ecology 2013, 94, 1487–1498. [Google Scholar] [CrossRef] [PubMed]
- Dunitz, J.D.; Orgel, L.E. Electronic properties of transition-metal oxides—I: Distortions from cubic symmetry. J. Phys. Chem. Solids 1957, 3, 20–29. [Google Scholar] [CrossRef]
- Berg, R.A.; Wharton, L.; Klemperer, W.; Büchler, A.; Stauffer, J.L. Determination of electronic symmetry by electric deflection: LiO and LaO. J. Chem. Phys. 1965, 43, 2416–2421. [Google Scholar] [CrossRef]
- Renner, R. Symmetry of large physical systems implies independence of subsystems. Nat. Phys. 2007, 3, 645–649. [Google Scholar] [CrossRef]
- Koptsik, V.A. Symmetry principle in physics. J. Phys. C Solid State Phys. 1983, 16, 23. [Google Scholar] [CrossRef]
- Ivanova, I.A.; Leydesdorff, L. Rotational symmetry and the transformation of innovation systems in a Triple Helix of university–industry–government relations. Technol. Forecast. Soc. Chang. 2014, 86, 143–156. [Google Scholar] [CrossRef]
- Daum, S.O.; Hecht, H. Effects of symmetry, texture, and monocular viewing on geographical slant estimation. Conscious. Cogn. 2018, 64, 183–195. [Google Scholar] [CrossRef] [PubMed]
- Hammond, K.R.; Mumpower, J.L.; Smith, T.H. Linking environmental models with models of human judgment: A symmetrical decision aid. IEEE Trans. Syst. Man Cybern. 1977, 7, 358–367. [Google Scholar] [CrossRef]
- Turvey, M.T.; Shaw, R.E. Ecological foundations of cognition. I: Symmetry and specificity of animal-environment systems. J. Conscious. Stud. 1999, 6, 95–110. [Google Scholar]
- Shilnikov, L.P.; Shilnikov, A.L.; Turaev, D.V.; Chua, L.O. Methods of Qualitative Theory in Nonlinear Dynamics, Part 2; World-Scientific: Singapore, 2009. [Google Scholar]
- Shil’nikov, A.L. On bifurcations of the Lorenz attractor in the Shimizu-Morioka model. Phys. D Nonlinear Phenom. 1993, 62, 338–346. [Google Scholar] [CrossRef]
- Grassi, G.; Severance, F.L.; Miller, D.A. Multi-wing hyperchaotic attractors from coupled Lorenz systems. Chaos Solitons Fractals 2009, 41, 284–291. [Google Scholar] [CrossRef]
- Yu, S.; Tang, W.K.; Lu, J.; Chen, G. Generation of n × m wing Lorenz-Like attractors from a modified Shimizu–Morioka model. IEEE Trans. Circuits Syst. II Express Briefs 2008, 55, 1168–1172. [Google Scholar] [CrossRef]
- Chang, H.; Li, Y.; Chen, G. A novel memristor-based dynamical system with multi-wing attractors and symmetric periodic bursting. Chaos 2020, 30, 043110. [Google Scholar] [CrossRef]
- Loskutov, A.Y. Dynamical chaos: Systems of classical mechanics. Phys.-Uspekhi 2007, 50, 939. [Google Scholar] [CrossRef]
- Smale, S. Diffeomorphisms with many periodic points. Matematika 1967, 11, 88–106. [Google Scholar]
- Anosov, D.V.; Sinai, Y.G. Some smooth ergodic systems. Russ. Math. Surv. 1967, 22, 103. [Google Scholar] [CrossRef]
- Guan, Z.H.; Huang, F.; Guan, W. Chaos-based image encryption algorithm. Phys. Lett. A 2005, 346, 153–157. [Google Scholar] [CrossRef]
- Zheng, J.; Hu, H. A symmetric image encryption scheme based on hybrid analog-digital chaotic system and parameter selection mechanism. Multimed. Tools Appl. 2021, 80, 20883–20905. [Google Scholar] [CrossRef]
- Chen, G.; Mao, Y.; Chui, C.K. A symmetric image encryption scheme based on 3D chaotic cat maps. Chaos Solitons Fractals 2004, 21, 749–761. [Google Scholar] [CrossRef]
- Radwan, A.G.; AbdElHaleem, S.H.; Abd-El-Hafiz, S.K. Symmetric encryption algorithms using chaotic and non-chaotic generators: A review. J. Adv. Res. 2016, 7, 193–208. [Google Scholar] [CrossRef] [PubMed]
- Mokhnache, S.; Daachi, M.E.H.; Bekkouche, T.; Diffellah, N. A Combined Chaotic System for Speech Encryption. Eng. Technol. Appl. Sci. Res. 2022, 12, 8578–8583. [Google Scholar] [CrossRef]
- Sathiyamurthi, P.; Ramakrishnan, S. Speech encryption using hybrid-hyper chaotic system and binary masking technique. Multimed. Tools Appl. 2022, 81, 6331–6349. [Google Scholar] [CrossRef]
- Ashtiyani, M.; Birgani, P.M.; Madahi, S. Speech signal encryption using chaotic symmetric cryptography. J. Basic. Appl. Sci. Res. 2012, 2, 1678–1684. [Google Scholar]
- Yu, F.; Li, L.; Tang, Q.; Cai, S.; Song, Y.; Xu, Q. A survey on true random number generators based on chaos. Discret. Dyn. Nat. Soc. 2019, 2019, 2545123. [Google Scholar] [CrossRef]
- Koyuncu, I.; Özcerit, A.T. The design and realization of a new high speed FPGA-based chaotic true random number generator. Comput. Electr. Eng. 2017, 58, 203–214. [Google Scholar] [CrossRef]
- Sprott, J.C. Simplest chaotic flows with involutional symmetries. Int. J. Bifurcat. Chaos 2014, 24, 1450009. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C. Coexisting hidden attractors in a 4-D simplified Lorenz system. Int. J. Bifurcat. Chaos 2014, 24, 1450034. [Google Scholar] [CrossRef]
- Wang, X.; Wang, M. A hyperchaos generated from Lorenz system. Phys. A Stat. Mech. Its Appl. 2008, 387, 3751–3758. [Google Scholar] [CrossRef]
- Barboza, R.U.Y. Dynamics of a hyperchaotic Lorenz system. Int. J. Bifurcat. Chaos 2007, 17, 4285–4294. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C. Variable-boostable chaotic flows. Optik 2016, 127, 10389–10398. [Google Scholar] [CrossRef]
- Ma, C.; Mou, J.; Xiong, L.; Banerjee, S.; Liu, T.; Han, X. Dynamical analysis of a new chaotic system: Asymmetric multistability, offset boosting control and circuit realization. Nonlinear Dyn. 2021, 103, 2867–2880. [Google Scholar] [CrossRef]
- Leutcho, G.D.; Kengne, J. A unique chaotic snap system with a smoothly adjustable symmetry and nonlinearity: Chaos, offset-boosting, antimonotonicity, and coexisting multiple attractors. Chaos Solitons Fractals 2018, 113, 275–293. [Google Scholar] [CrossRef]
- Xu, C.; Ur Rahman, M.; Baleanu, D. On fractional-order symmetric oscillator with offset-boosting control. Nonlinear Anal. Model. Control 2022, 27, 994–1008. [Google Scholar] [CrossRef]
- Gu, S.; He, S.; Wang, H.; Du, B. Analysis of three types of initial offset-boosting behavior for a new fractional-order dynamical system. Chaos Solitons Fractals 2021, 143, 110613. [Google Scholar] [CrossRef]
- Sayed, W.S.; Roshdy, M.; Said, L.A.; Radwan, A.G. Design and FPGA verification of custom-shaped chaotic attractors using rotation, offset boosting and amplitude control. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 3466–3470. [Google Scholar] [CrossRef]
- Bayani, A.; Rajagopal, K.; Khalaf, A.J.M.; Jafari, S.; Leutcho, G.D.; Kengne, J. Dynamical analysis of a new multistable chaotic system with hidden attractor: Antimonotonicity, coexisting multiple attractors, and offset boosting. Phys. Lett. A 2019, 383, 1450–1456. [Google Scholar] [CrossRef]
- Leutcho, G.D.; Kengne, J.; Kengne, R. Remerging Feigenbaum trees, and multiple coexisting bifurcations in a novel hybrid diode-based hyperjerk circuit with offset boosting. Int. J. Dyn. Control 2019, 7, 61–82. [Google Scholar] [CrossRef]
- Li, C.; Wang, R.; Ma, X.; Jiang, Y.; Liu, Z. Embedding any desired number of coexisting attractors in memristive system. Chin. Phys. B 2021, 30, 120511. [Google Scholar] [CrossRef]
- Li, C.; Lu, T.; Chen, G.; Xing, H. Doubling the coexisting attractors. Chaos 2019, 29, 051102. [Google Scholar] [CrossRef] [PubMed]
- Wang, R.; Li, C.; Kong, S.; Jiang, Y.; Lei, T. A 3D memristive chaotic system with conditional symmetry. Chaos Solitons Fractals 2022, 158, 111992. [Google Scholar] [CrossRef]
- Rössler, O.E. An equation for continuous chaos. Phys. Lett. A 1976, 57, 397–398. [Google Scholar] [CrossRef]
- Letellier, C.; Dutertre, P.; Maheu, B. Unstable periodic orbits and templates of the Rössler system: Toward a systematic topological characterization. Chaos 1995, 5, 271–282. [Google Scholar] [CrossRef]
- Rafikov, M.; Balthazar, J.M. On an optimal control design for Rössler system. Phys. Lett. A 2004, 333, 241–245. [Google Scholar] [CrossRef]
- Li, C.; Sun, J.; Lu, T.; Lei, T. Symmetry evolution in chaotic system. Symmetry 2020, 12, 574. [Google Scholar] [CrossRef]
- Li, C.; Sun, J.; Sprott, J.C.; Lei, T. Hidden attractors with conditional symmetry. Int. J. Bifurcat. Chaos 2020, 30, 2030042. [Google Scholar] [CrossRef]
- Gu, Z.; Li, C.; Iu, H.H.; Min, F.; Zhao, Y. Constructing hyperchaotic attractors of conditional symmetry. Eur. Phys. J. B 2019, 92, 221. [Google Scholar] [CrossRef]
- Lu, T.; Li, C.; Jafari, S.; Min, F. Controlling coexisting attractors of conditional symmetry. Int. J. Bifurcat. Chaos 2019, 29, 1950207. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C.; Xing, H. Constructing chaotic systems with conditional symmetry. Nonlinear Dyn. 2017, 87, 1351–1358. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C.; Liu, Y.; Gu, Z.; Zhang, J. Offset boosting for breeding conditional symmetry. Int. J. Bifurcat. Chaos 2018, 28, 1850163. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C.; Zhang, X.; Chai, L.; Liu, Z. Constructing conditional symmetry in symmetric chaotic systems. Chaos Solitons Fractals 2022, 155, 111723. [Google Scholar] [CrossRef]
- Li, C.; Xu, Y.; Chen, G.; Liu, Y.; Zheng, J. Conditional symmetry: Bond for attractor growing. Nonlinear Dyn. 2019, 95, 1245–1256. [Google Scholar] [CrossRef]
- Jia, L.L.; Lai, B.C. A new continuous memristive chaotic system with multistability and amplitude control. Eur. Phys. J. Plus 2022, 137, 604. [Google Scholar] [CrossRef]
- Wu, Q.; Hong, Q.; Liu, X.; Wang, X.; Zeng, Z. A novel amplitude control method for constructing nested hidden multi-butterfly and multiscroll chaotic attractors. Chaos Solitons Fractals 2020, 134, 109727. [Google Scholar] [CrossRef]
- Wang, X.; Deng, L.; Zhang, W. Hopf bifurcation analysis and amplitude control of the modified Lorenz system. Appl. Math. Comput. 2013, 225, 333–344. [Google Scholar] [CrossRef]
- Liu, Z.; Lai, Q. A novel memristor-based chaotic system with infinite coexisting attractors and controllable amplitude. Indian J. Phys. 2023, 97, 1159–1167. [Google Scholar] [CrossRef]
- Moon, S.; Baik, J.J.; Hong, S.H. Coexisting attractors in a physically extended Lorenz system. Int. J. Bifurcat. Chaos 2021, 31, 2130016. [Google Scholar] [CrossRef]
- Boya, B.F.B.A.; Ramakrishnan, B.; Effa, J.Y.; Kengne, J.; Rajagopal, K. The effects of symmetry breaking on the dynamics of an inertial neural system with a non-monotonic activation function: Theoretical study, asymmetric multistability and experimental investigation. Phys. A Stat. Mech. Its Appl. 2022, 602, 127458. [Google Scholar] [CrossRef]
- Yan, S.; Sun, X.; Wang, Q.; Ren, Y.; Shi, W.; Wang, E. A novel double-wing chaotic system with infinite equilibria and coexisting rotating attractors: Application to weak signal detection. Phys. Scr. 2021, 96, 125216. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C.; Liu, Y. Time-reversible chaotic system with conditional symmetry. Int. J. Bifurcat. Chaos 2020, 30, 2050067. [Google Scholar] [CrossRef]
- Lai, Q.; Kuate, P.D.K.; Liu, F.; Iu, H.H.C. An extremely simple chaotic system with infinitely many coexisting attractors. IEEE Trans. Circuits Syst. II Express Briefs 2019, 67, 1129–1133. [Google Scholar] [CrossRef]
- Wu, H.; Bao, H.; Xu, Q.; Chen, M. Abundant coexisting multiple attractors’ behaviors in three-dimensional sine chaotic system. Complexity 2019, 2019, 3687635. [Google Scholar] [CrossRef]
- Volos, C.; Akgul, A.; Pham, V.T.; Stouboulos, I.; Kyprianidis, I. A simple chaotic circuit with a hyperbolic sine function and its use in a sound encryption scheme. Nonlinear Dyn. 2017, 89, 1047–1061. [Google Scholar] [CrossRef]
- Hu, X.; Sang, B.; Wang, N. The chaotic mechanisms in some jerk systems. AIMS Math. 2022, 7, 15714–15740. [Google Scholar] [CrossRef]
- Karawanich, K.; Kumngern, M.; Chimnoy, J.; Prommee, P. A four-scroll chaotic generator based on two nonlinear functions and its telecommunications cryptography application. AEU Int. J. Electron. Commun. 2022, 157, 154439. [Google Scholar] [CrossRef]
- Qiu, H.; Xu, X.; Jiang, Z.; Sun, K.; Cao, C. Dynamical behaviors, circuit design, and synchronization of a novel symmetric chaotic system with coexisting attractors. Sci. Rep. 2023, 13, 1893. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, S. Design and implementation of simplified symmetry chaotic circuit. Symmetry 2022, 14, 2299. [Google Scholar] [CrossRef]
- Kengne, J.; Mogue, R.L.T.; Fozin, T.F.; Telem, A.N.K. Effects of symmetric and asymmetric nonlinearity on the dynamics of a novel chaotic jerk circuit: Coexisting multiple attractors, period doubling reversals, crisis, and offset boosting. Chaos Solitons Fractals 2019, 121, 63–84. [Google Scholar] [CrossRef]
- Kahlert, C. The effects of symmetry breaking in Chua’s circuit and related piecewise-linear dynamical systems. Int. J. Bifurcat. Chaos 1993, 3, 963–979. [Google Scholar] [CrossRef]
- Kamdem Tchiedjo, S.; Kamdjeu Kengne, L.; Kengne, J.; Djuidje Kenmoe, G. Dynamical behaviors of a chaotic jerk circuit based on a novel memristive diode emulator with a smooth symmetry control. Eur. Phys. J. Plus 2022, 137, 940. [Google Scholar] [CrossRef]
- Kengne, L.K.; Kengne, J.; Fotsin, H.B. The effects of symmetry breaking on the dynamics of a simple autonomous jerk circuit. Analog. Integr. Circuits Signal Process. 2019, 101, 489–512. [Google Scholar] [CrossRef]
- Nishio, Y.O.; Inaba, N.A.; Mori, S.H.; Saito, T.O. Rigorous analyses of windows in a symmetric circuit. IEEE Trans. Circuits Syst. 1990, 37, 473–487. [Google Scholar] [CrossRef]
- Volos, C. Symmetry in Chaotic Systems and Circuits. Symmetry 2022, 14, 1612. [Google Scholar] [CrossRef]
- Itoh, M.; Chua, L.O. Memristor hamiltonian circuits. Int. J. Bifurcat. Chaos 2011, 21, 2395–2425. [Google Scholar] [CrossRef]
- Jiang, Y.; Li, C.; Zhang, C.; Lei, T.; Jafari, S. Constructing meminductive chaotic oscillator. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 2675–2679. [Google Scholar] [CrossRef]
- Wu, J.; Li, C.; Ma, X.; Lei, T.; Chen, G. Simplification of chaotic circuits with quadratic nonlinearity. IEEE Trans. Circuits Syst. II Express Briefs 2021, 69, 1837–1841. [Google Scholar] [CrossRef]
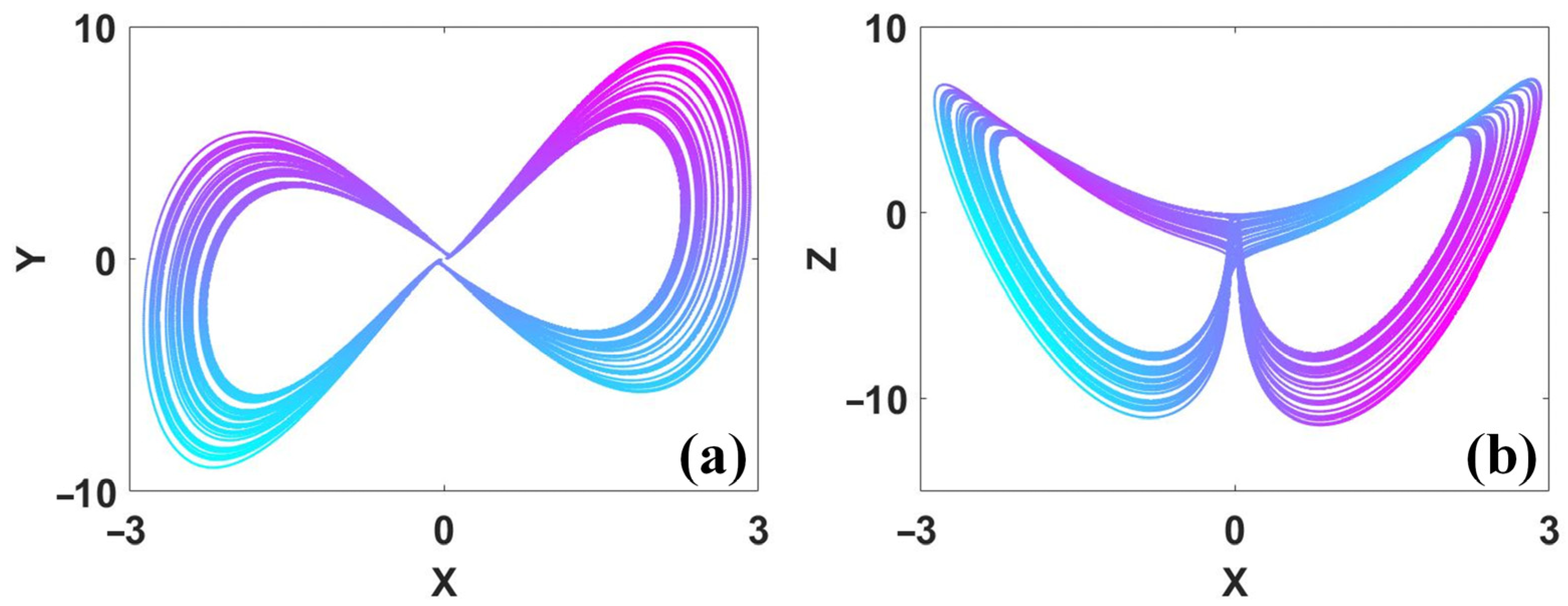

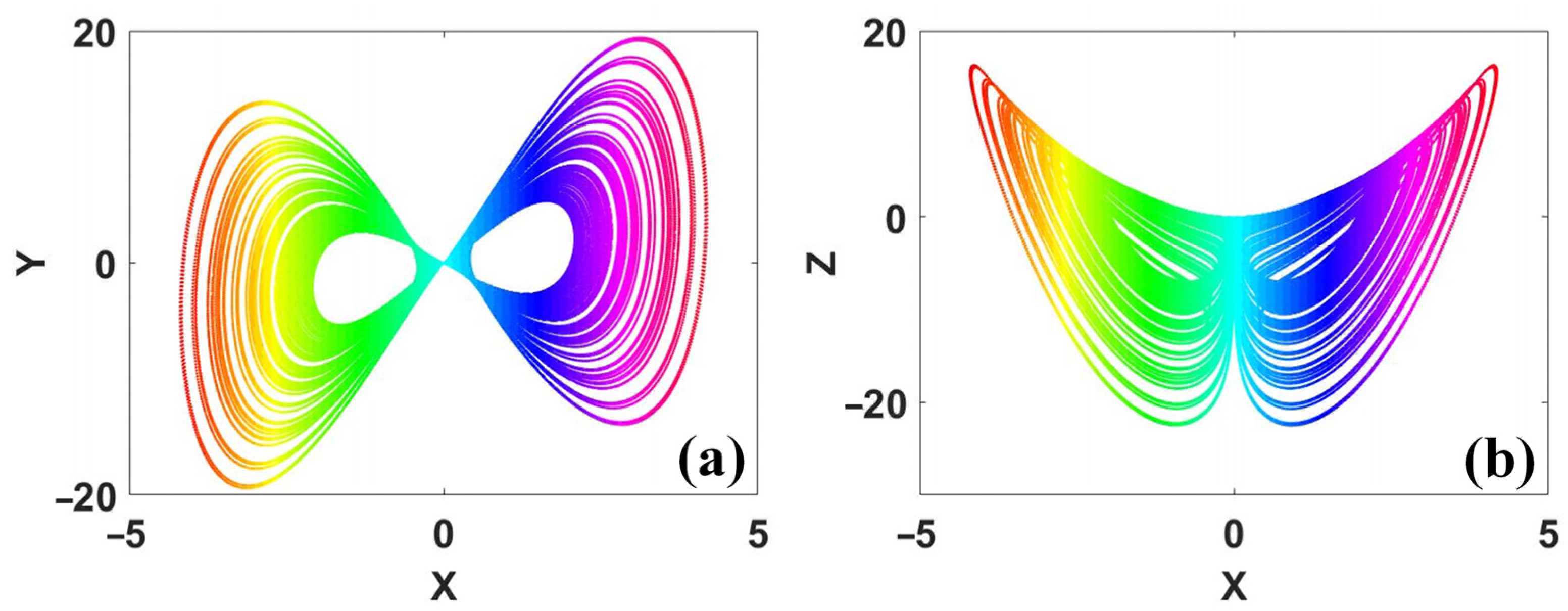


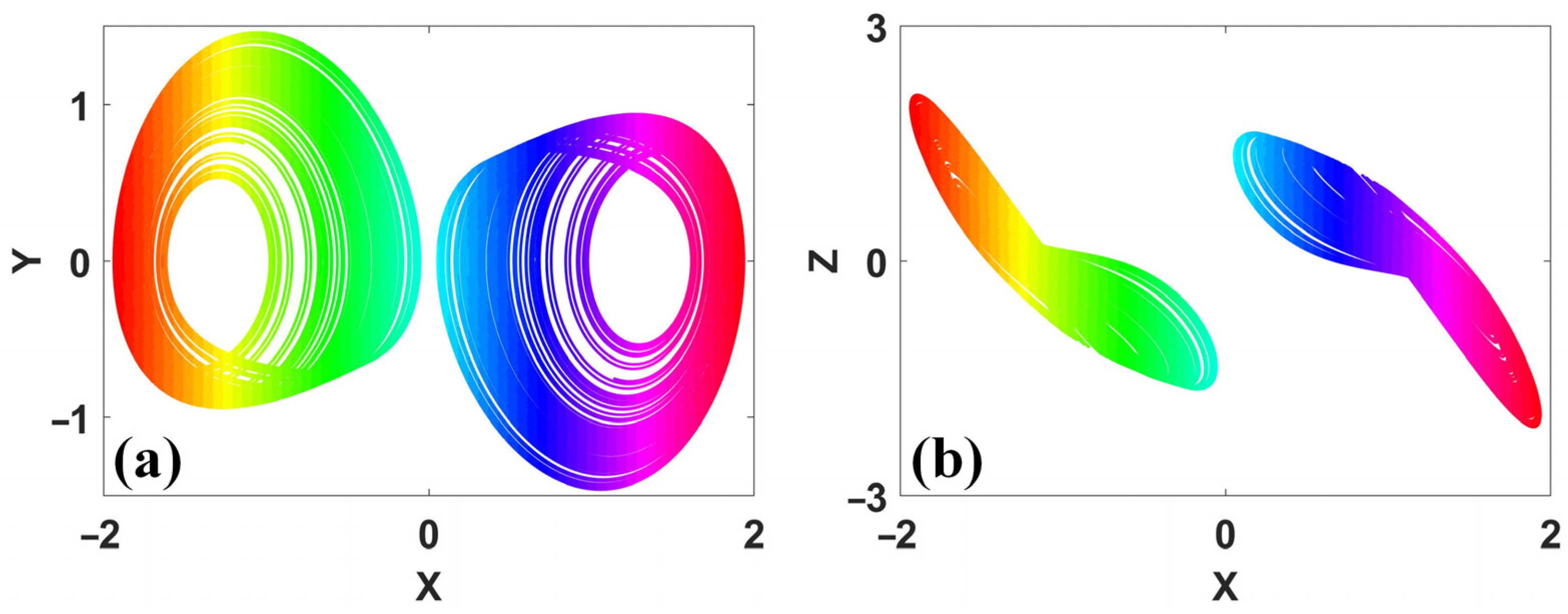
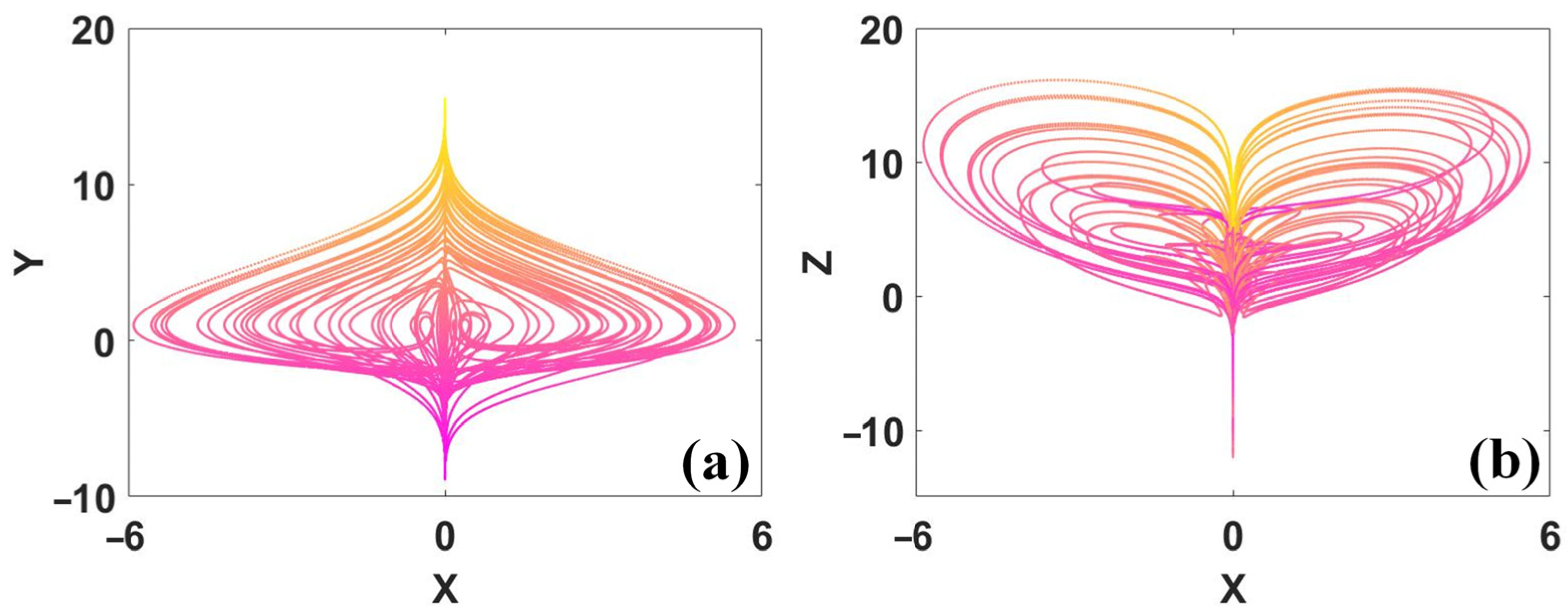
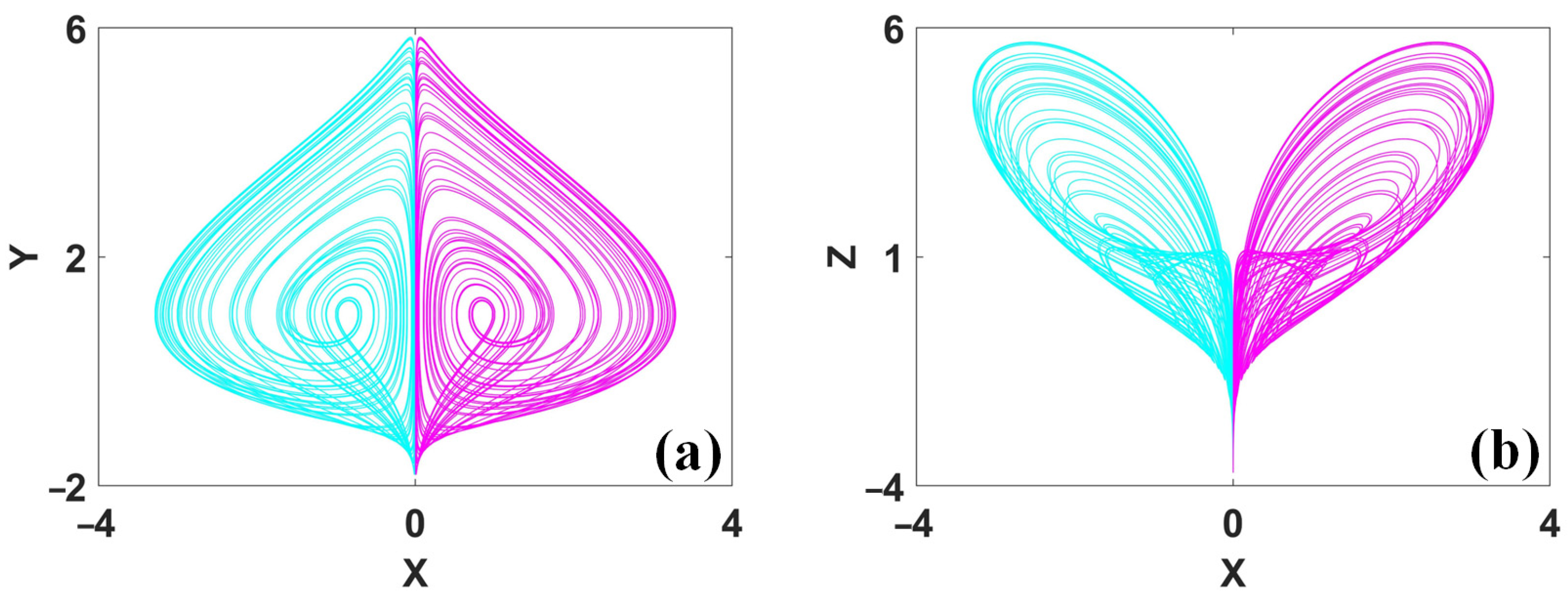


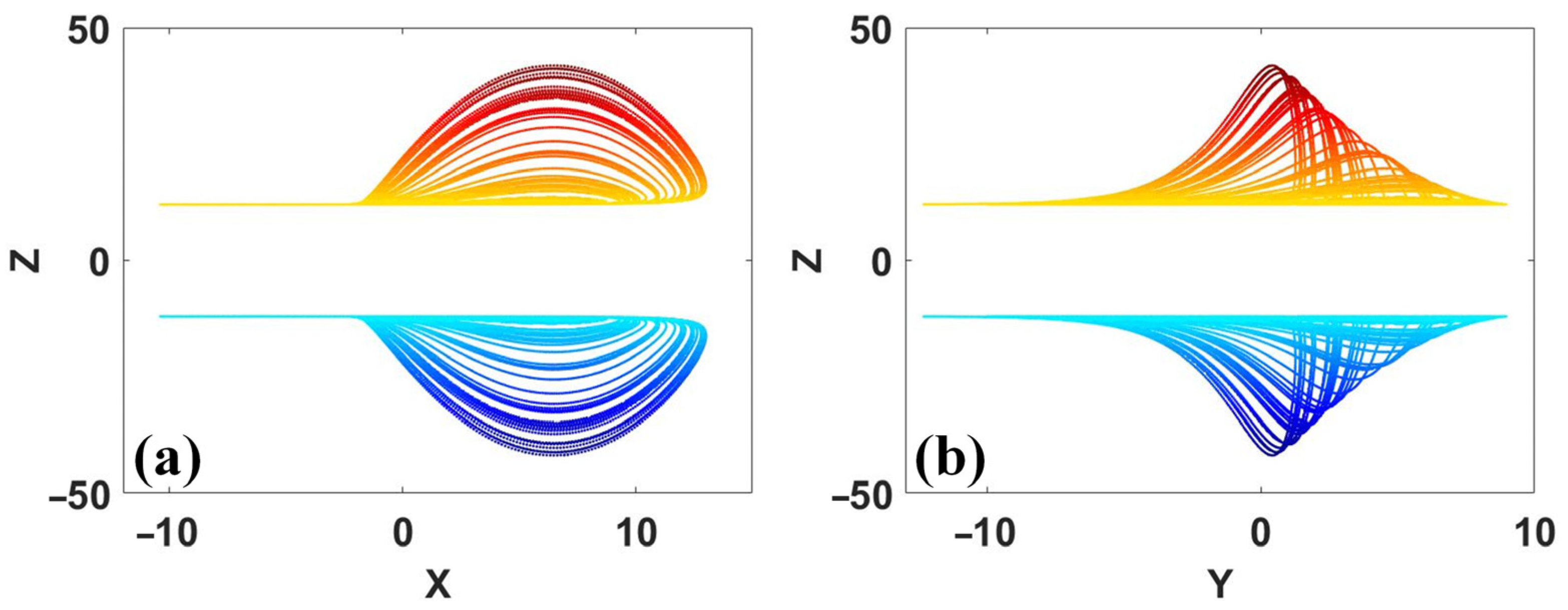
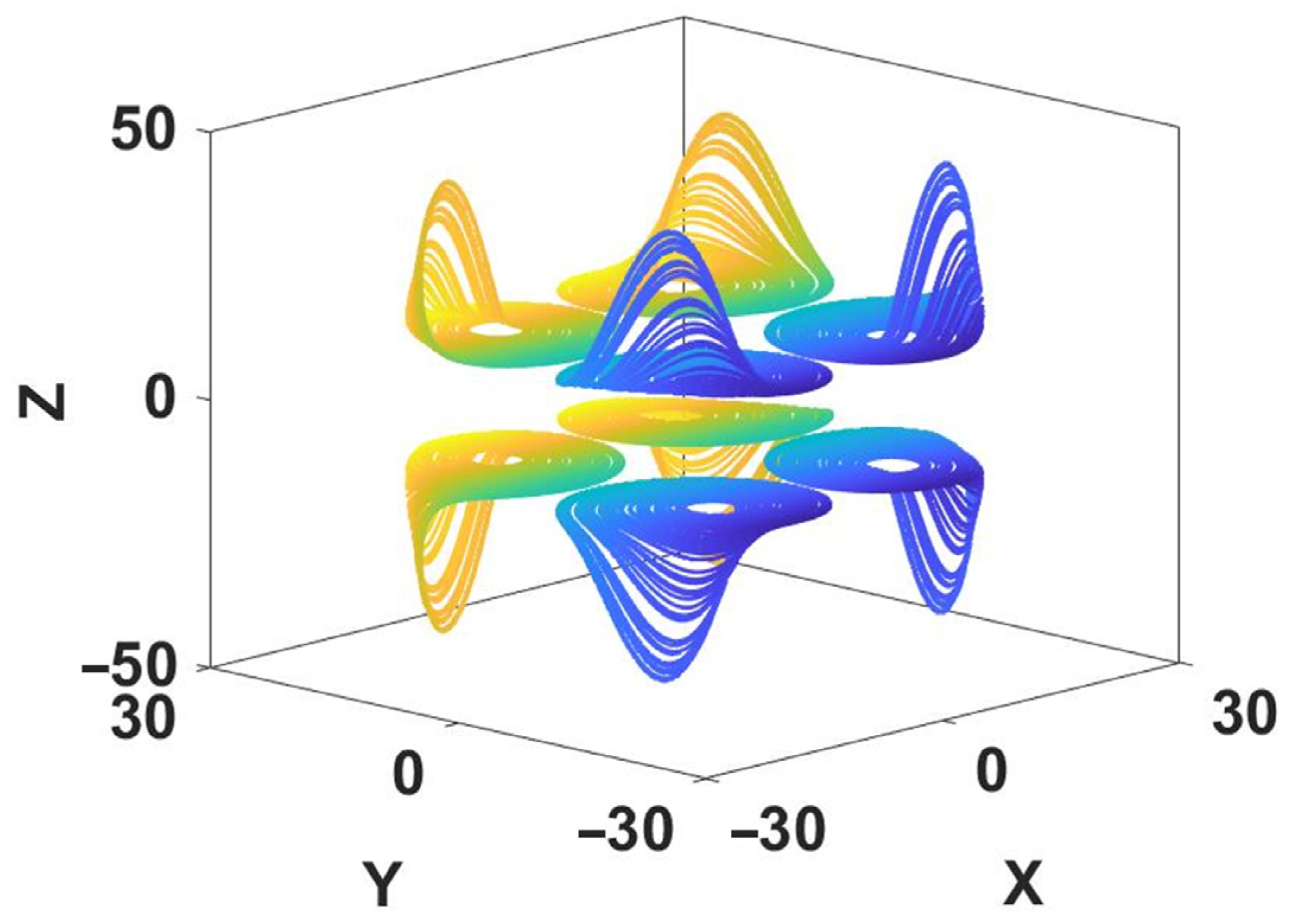


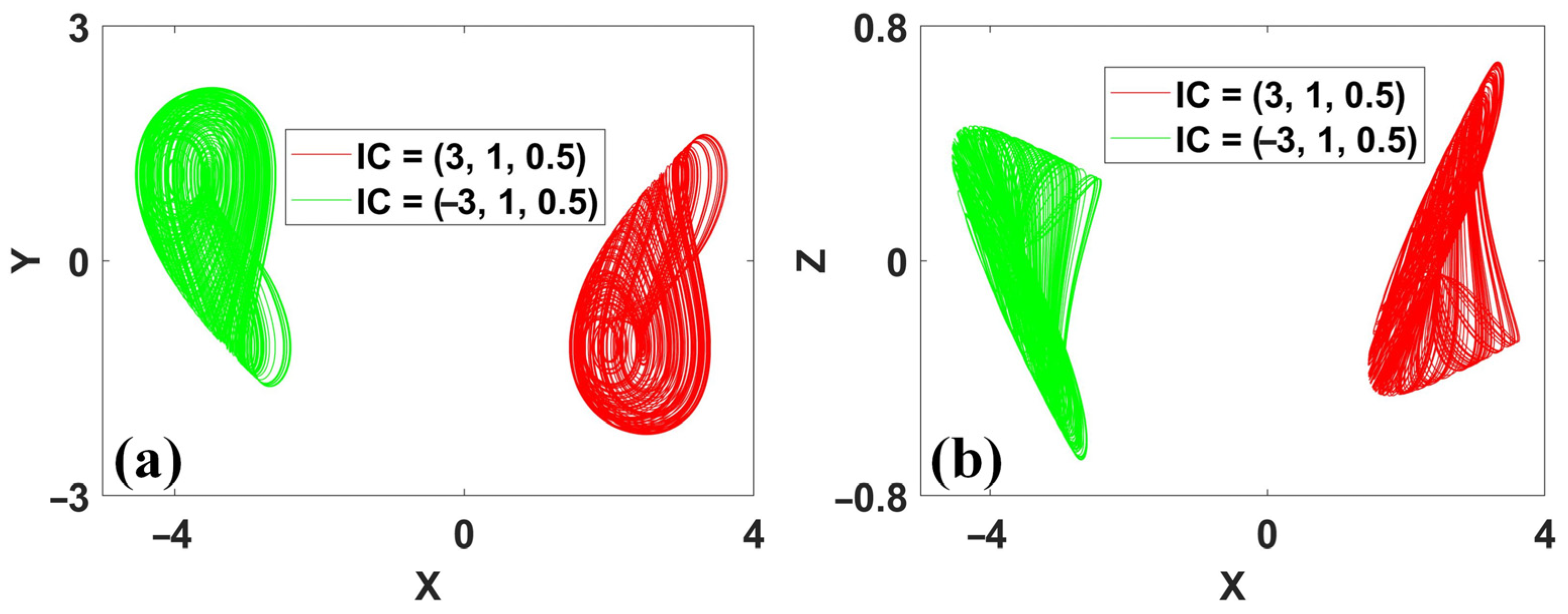
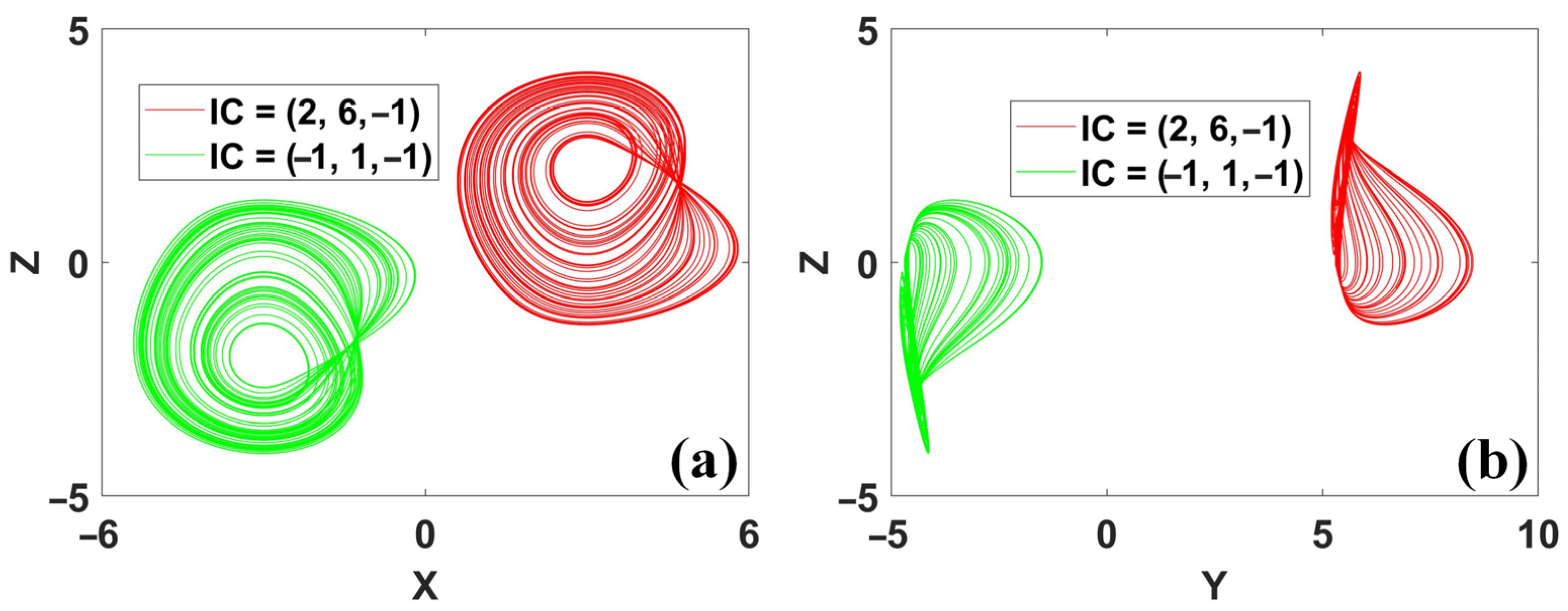
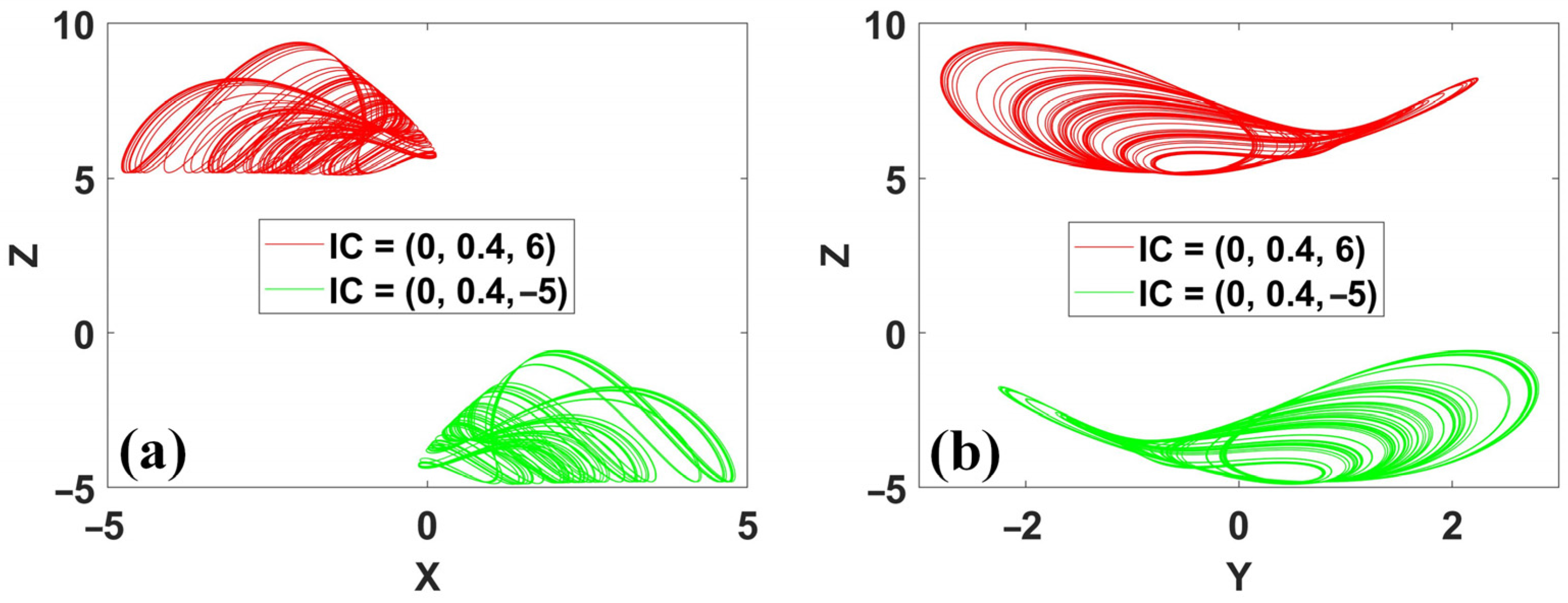
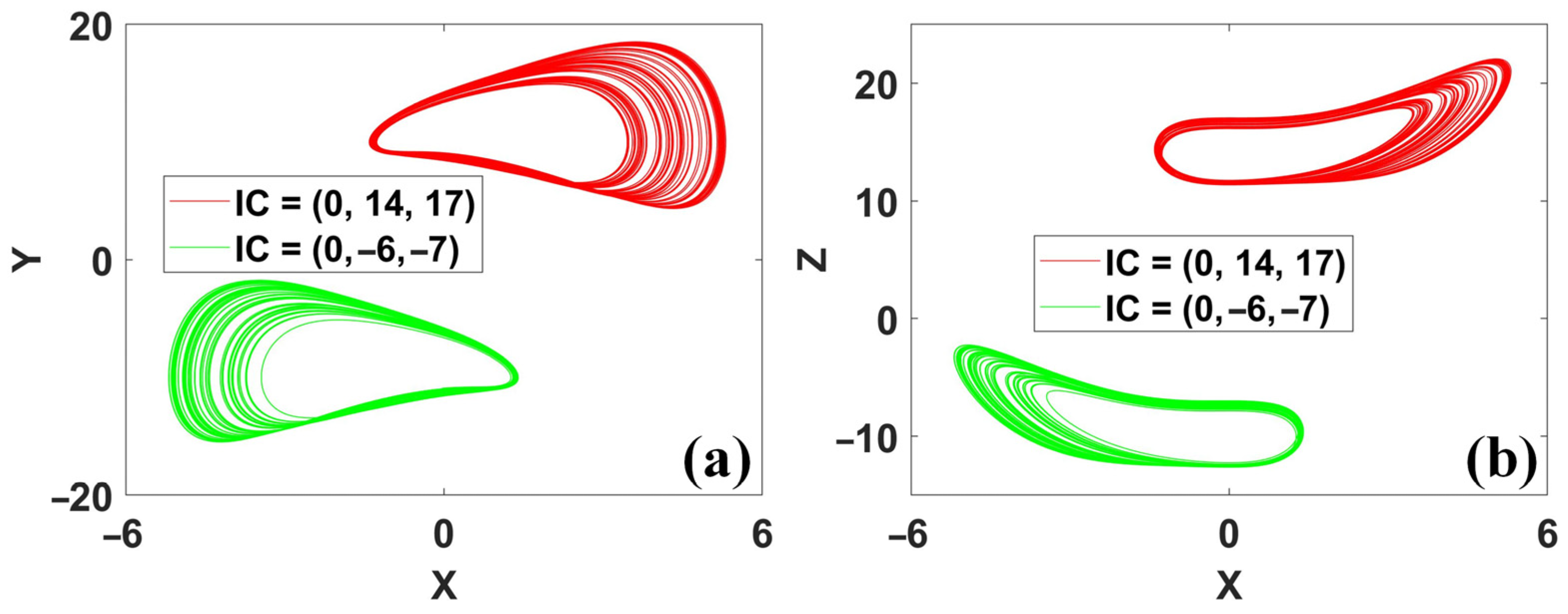
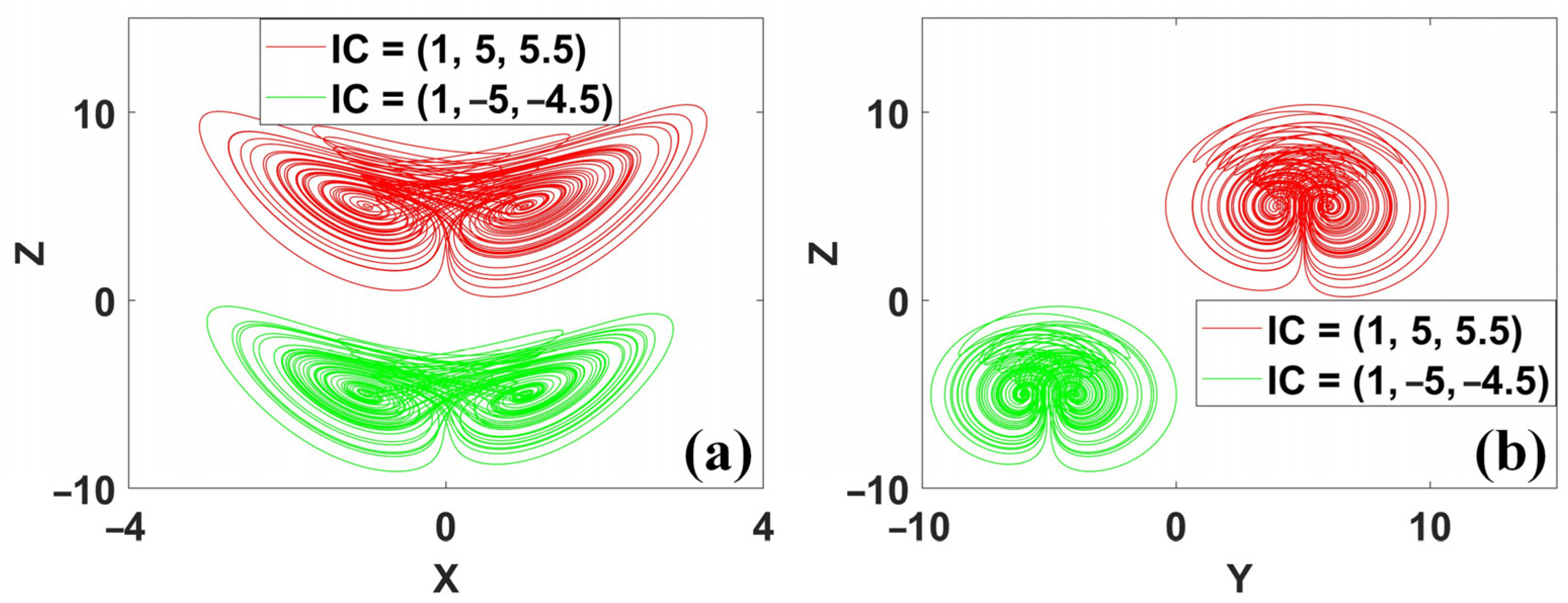

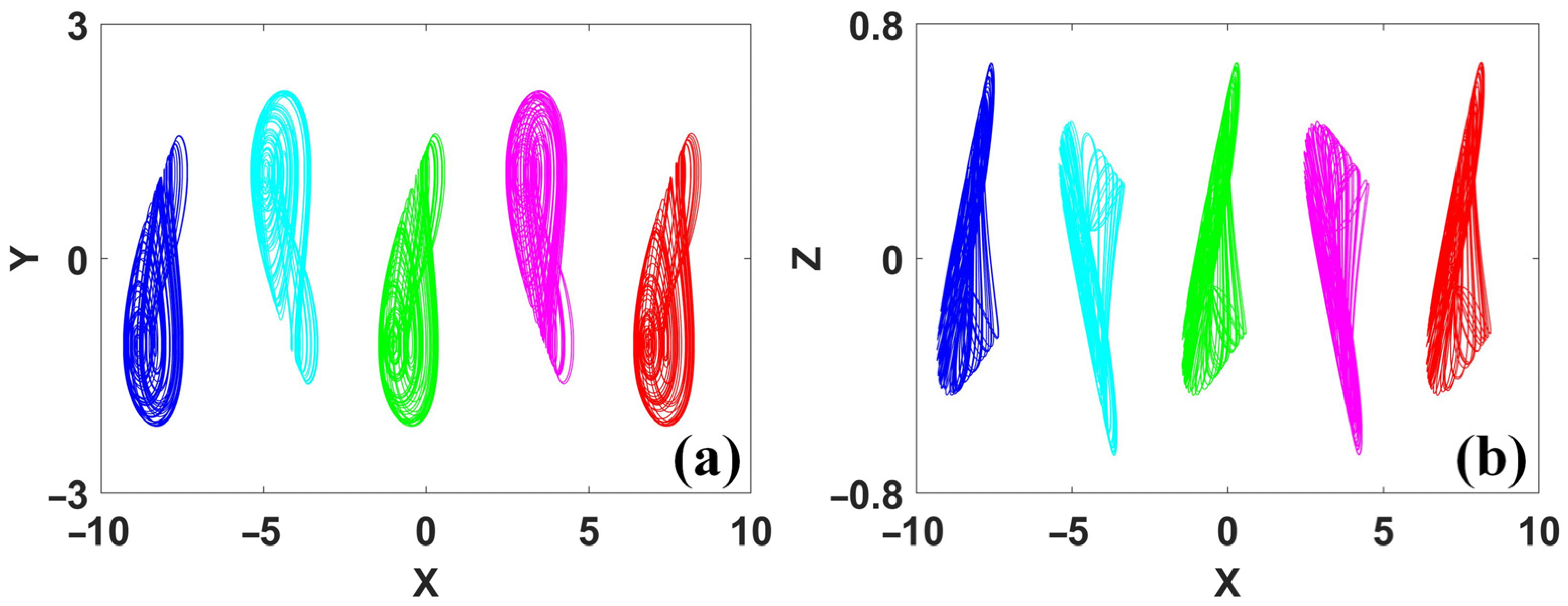

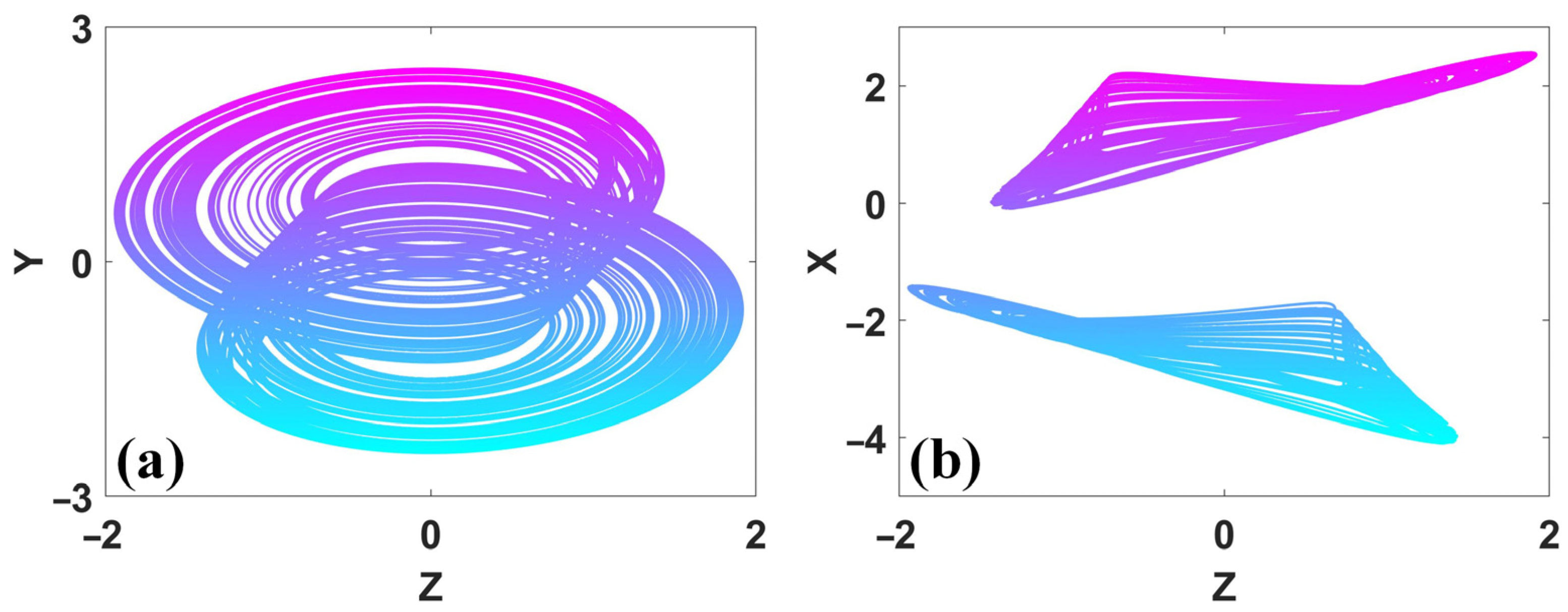
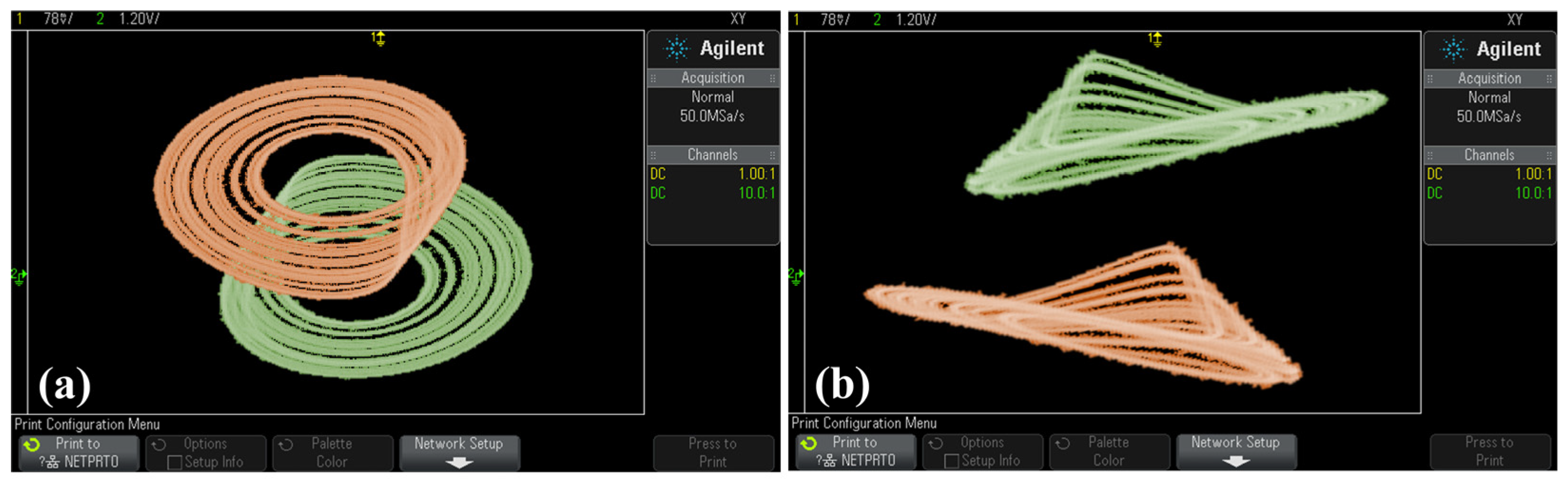
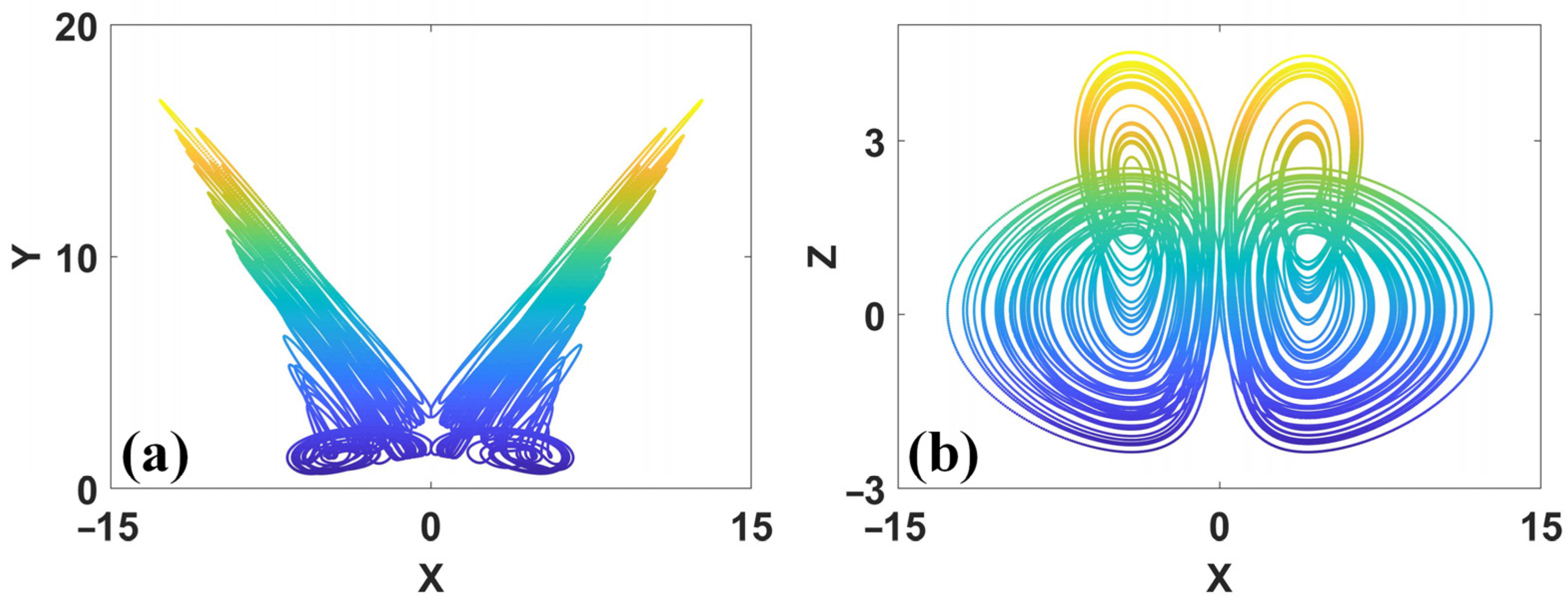

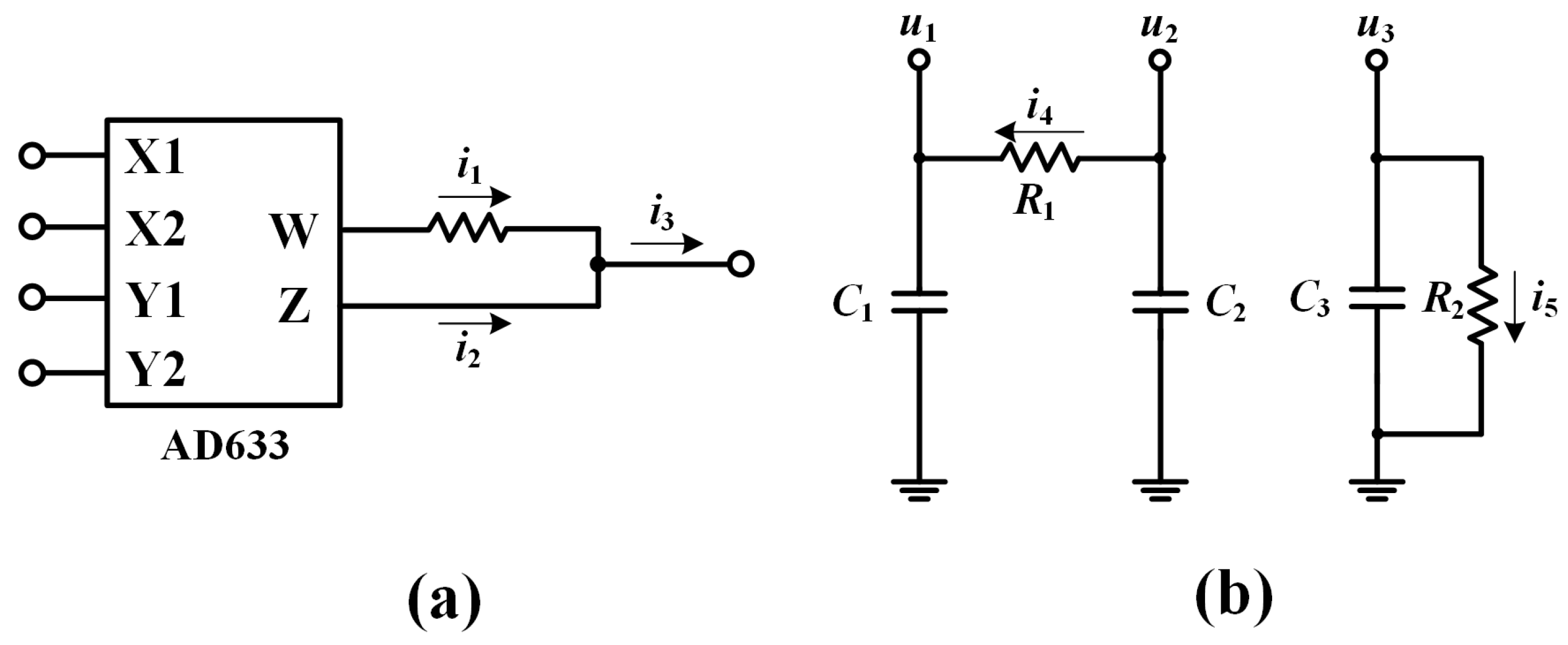
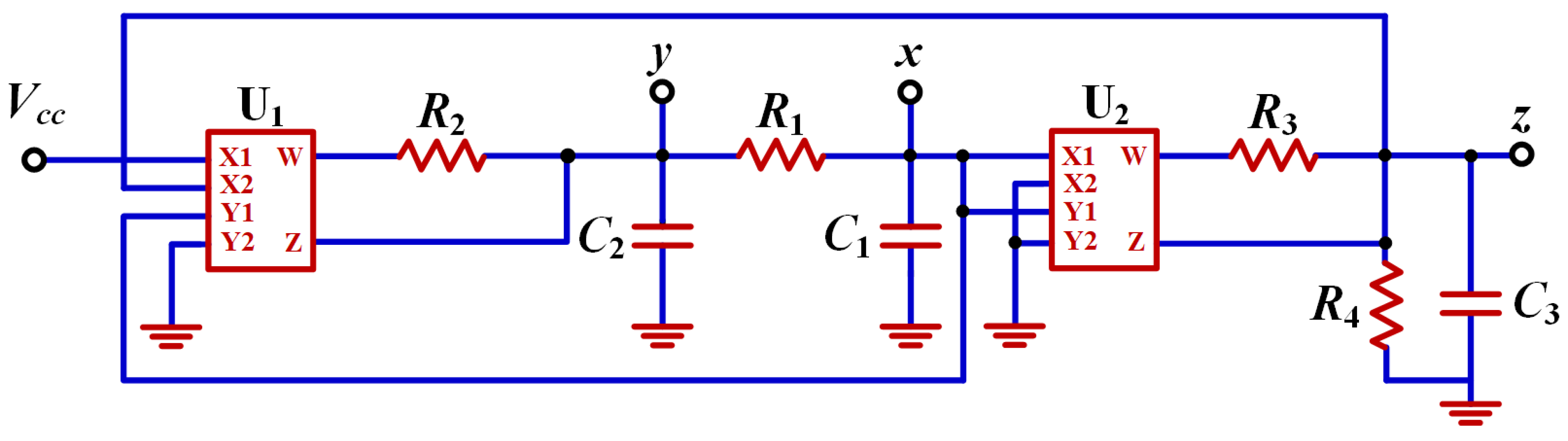
| Equations | Parameters | Equilibria | Eigenvalues |
|---|---|---|---|
| [51] | a = 2.1 b = 2 | (0, 0, 0) (, 0, 0) (, 0, 0) | (0.6366, 0.8183 1.5723i) (1.4516, 0.2258 1.6446i) |
| [51] | a = 0.279 b = 0.3 c = 0 | (0, 0, 0) (, , 1) (, , 1) | (1, 0.279, 0.3) (1.1722, 0.0966 0.3653i) |
| [51] | a = 4.7 | (, , 0) (, , 0) | (1.6369, 0.3185 2.3751i) |
| [51] | a = 1 b = 1.06 | (50/53, 1, 50/53) (50/53, 1, 50/53) | (0.8218, 0.3526 1.5669i) |
| [51] | a = 0.7 | (0, 0, 0) (1, 1, 0) (1, 1, 0) | (1, 0.35 0.9368i) (1.2216, 0.2608 1.2527i) |
| [52] | a = 6 b = 0.1 | None | None |
| Equations | Parameters | (x0, y0, z0) | LEs | DKY |
|---|---|---|---|---|
| [69] F(x) = |x| 3 | a = 0.4 b = 1.75 c = 3 | (3, 1.5, 2) (3, 1.5, 1) | 0.1191, 0, 1.2500 | 2.0953 |
| [69] F(x) = |x| 3 | a = 1.22 b = 8.48 | (3, 1, 0.5) (3, 1, 0.5) | 0.2335, 0, 1.2335 | 2.1893 |
| [69] F(y) = |y| 4 | a = 2.6 b = 2 | (0.5, 4, 1) (0.5, 4, 1) | 0.0463, 0, 2.6463 | 2.0175 |
| [69] F(z) = |z| 8 | a = 1.24 b = 1 | (4, 0.8, 2) (4, 0.8, 14) | 0.0645, 0, 1.2582 | 2.0513 |
| [69] F(x) = |x| 3 G(y) = |y| 5 | a = 0.22 | (1, 1, 1) (2, 6, 1) | 0.0729, 0, 1.6732 | 2.0436 |
| [69] F(y) = |y| 5 G(z) = |z| 5 | a = 3 b = 1.2 | (0, 6, 6) (0, 6, 6) | 0.0506, 0, 0.2904 | 2.1735 |
| [70] F(z) = |z| 5 | a = 0.35 | (0, 0.4, 6) (0, 0.4, 5) | 0.0776, 0, 1.5008 | 2.0517 |
| [70] F(z) = |z| 12 | a = 2.0 | (0, 2.3, 12) (0, 2.3, 12) | 0.0252, 0, 6.8521 | 2.0037 |
| [70] F(z) = |z| 12 | a = 0.1 | (0.5, 0, 11) (0.5, 0, 13) | 0.0665, 0, 2.0410 | 2.0326 |
| [70] F(z) = |z| 15 | a = 0.4 | (2.5, 0, 15) (2.5, 0, 15) | 0.1026, 0, 2.1275 | 2.0482 |
| [70] F(z) = |z| 15 | a = 2.0 | (1, 0, 11) (1, 0, 19) | 0.0538, 0, 11.8591 | 2.0045 |
| [70] F(z) = |z| 30 | a = 1.0 | (0, 1, 26.1) (0, 1, 32.9) | 0.1105, 0, 1.3882 | 2.0796 |
| [70] F(x) = |x| 9 | a = 1.62 b = 0.2 | (9, 1, 0.8) (9, 1, 0.8) | 0.0645, 0, 0.6845 | 2.0943 |
| [70] G(y) = |y| 10 F(z) = |z| 12 | a = 0.4 b = 1 | (0, 14, 17) (0, 6, 7) | 0.0749, 0, 0.7390 | 2.1013 |
| [70] F(z) = |z| 4 | a = 0.8 b = 0.5 c = 0.5 | (1, 0.5, 5) (1, 0.5, 4) | 0.0177, 0, 0.4092 | 2.0433 |
| [71] F(z) = |z| 50 | a = 10 b = 8/3 c = 28 k = 4 e = 2 | (0, 0.3, 2, 0) (0, 0.3, 50, 0) | 0.2563, 0.1674, 0, 14.0917 | 3.0301 |
| [72] F(x) = |x| 3 | a = 3.55 b = 0.6 | (3, 0, 1) (3, 0, 1) | 0.1455, 0, 0.7455 | 2.1952 |
| [73] F(x) = |x| 3 | a = 0.4 b = 1.75 c = 3 | (3, 1.5, 2) (3, 1.5, 1) | 0.1191, 0, 1.2500 | 2.0953 |
| [73] F(x) = |x| 3 | a = 1.22 b = 8.48 | (3, 1, 0.5) (3, 1, 0.5) | 0.2335, 0, 1.2335 | 2.1893 |
| [74] F(y) = |y| 4 | a = 2.6 b = 2 | (0.5, 4, 1) (0.5, 4, 1) | 0.0463, 0, 2.6463 | 2.0175 |
| [74] F(z) = |z| 8 | a = 1.24 b = 1 | (4, 0.8, 2) (4, 0.8, 2) | 0.0645, 0, 1.2582 | 2.0513 |
| [74] F(x) = |x| 3 G(y) = |y| 5 | a = 0.22 | (1, 1, 1) (2, 6, 1) | 0.0729, 0, 1.6731 | 2.0436 |
| [74] F(y) = |y| 5 G(z) = |z| 5 | a = 3 b = 1.2 | (0, 6, 6) (0, 6, 6) | 0.0506, 0, 0.2904 | 2.1742 |
| [75] F(y) = |y| 5 G(z) = |z| 5 | a = 1 | (1, 5, 6) (1, 5, 4) | 0.2102, 0, 1.2102 | 2.1737 |
| [75] F(x) = |x| 7 G(z) = |z| 7 | a = 1 | (8, 1, 6) (6, 1, 8) | 0.2100, 0, 1.210 | 2.1736 |
| [75] F(x) = |x| 4 G(y) = |y| 5 | a = 2 | (5, 6, 1) (3, 4, 1) | 0.0568, 0, 2.0568 | 2.0276 |
| [75] F(y) = |y| 22 | a = 18 b = 1.93 | (1, 23, 1) (1, 21, 1) | 0.120, 0, 1.12 | 2.1071 |
| [75] F(y) = |y| 5 G(z) = |z| 12 | a = 0.33 b = 0.75 c = 0.35 d = 0.9 | (2, 1, 1) (2, 1, 1) | 0.0301, 0, 2.0419 | 2.0148 |
| [75] F(y) = |y| 3 | a = 16.8 b = 2.8 c = 8.25 | (1, 1, 1) (1, 1, 1) | 0.2985, 0, 1.1319 | 2.2638 |
| [75] F(y) = |y| 3 | a = 7.3 b = 6.4 c = 118 d = 0.1 | (1, 4, 1) (1, 2, 1) | 0.0708, 0, 25.6071 | 2.0028 |
| [75] F(x) = |x| 4 | a = 1 b = 20 | (5, 1, 3) (3, 1, 3) | 0.4543, 0, 20.4543 | 2.0222 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Li, Z.; Jiang, Y.; Lei, T.; Wang, X. Symmetric Strange Attractors: A Review of Symmetry and Conditional Symmetry. Symmetry 2023, 15, 1564. https://doi.org/10.3390/sym15081564
Li C, Li Z, Jiang Y, Lei T, Wang X. Symmetric Strange Attractors: A Review of Symmetry and Conditional Symmetry. Symmetry. 2023; 15(8):1564. https://doi.org/10.3390/sym15081564
Chicago/Turabian StyleLi, Chunbiao, Zhinan Li, Yicheng Jiang, Tengfei Lei, and Xiong Wang. 2023. "Symmetric Strange Attractors: A Review of Symmetry and Conditional Symmetry" Symmetry 15, no. 8: 1564. https://doi.org/10.3390/sym15081564
APA StyleLi, C., Li, Z., Jiang, Y., Lei, T., & Wang, X. (2023). Symmetric Strange Attractors: A Review of Symmetry and Conditional Symmetry. Symmetry, 15(8), 1564. https://doi.org/10.3390/sym15081564






