General Relativistic Stability and Gravitational Wave Content of Rotating Triaxial Neutron Stars
Abstract
:1. Introduction
2. Numerical Methods and Model Parameters
2.1. Initial Data
2.2. Evolutions
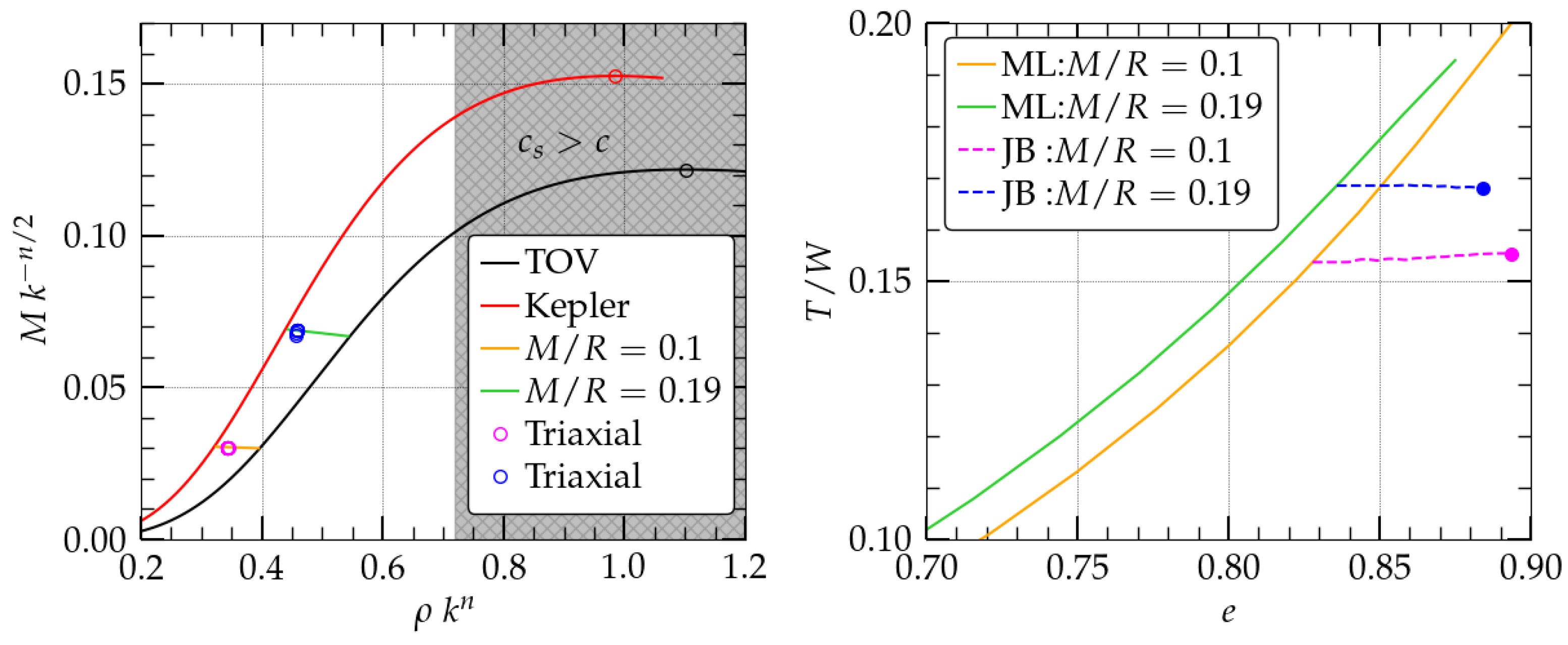
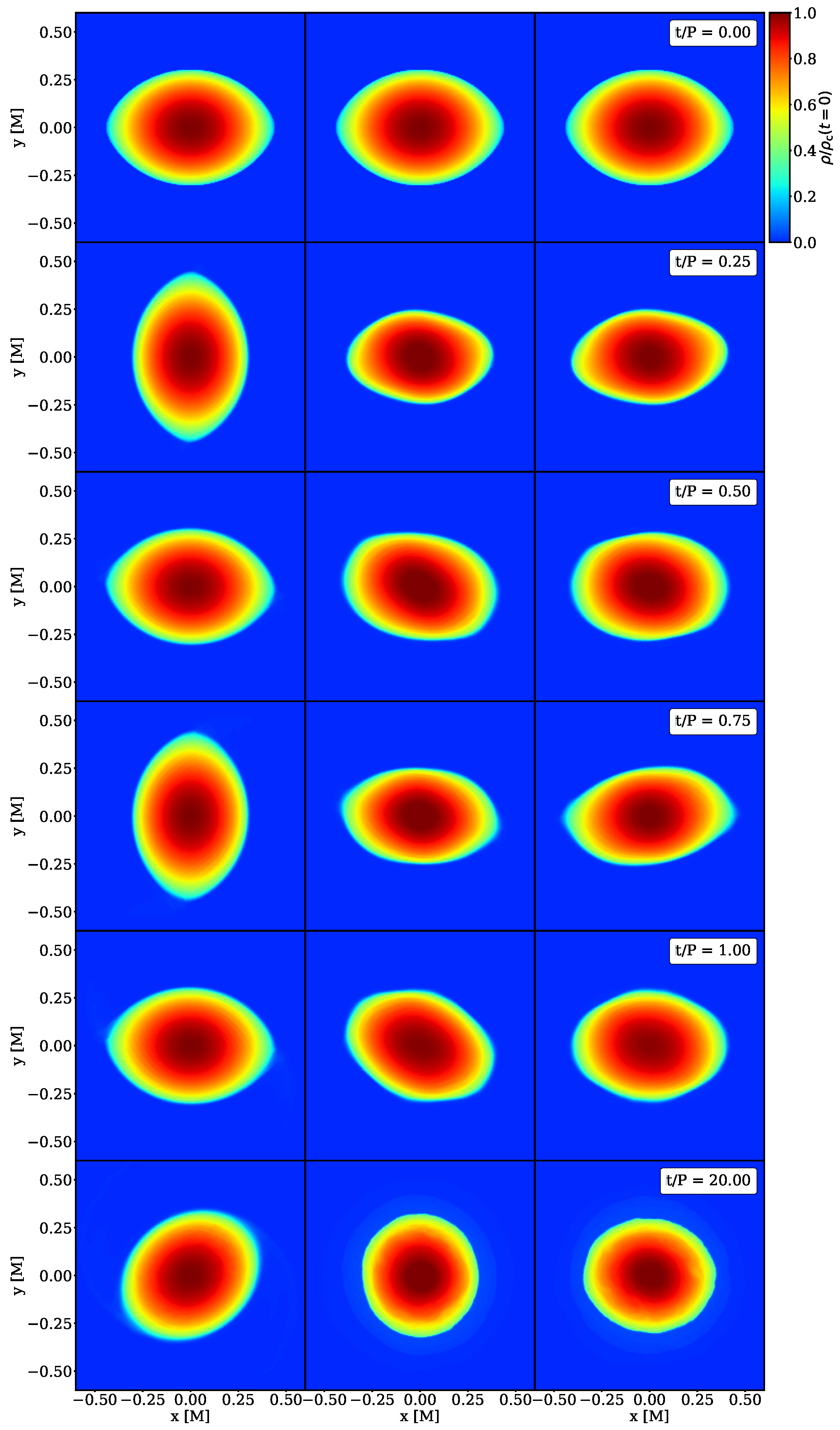
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- The LIGO Scientific Collaboration; The Virgo Collaboration. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Properties of the Binary Black Hole Merger GW150914. Phys. Rev. Lett. 2016, 116, 241102. [Google Scholar] [CrossRef] [PubMed]
- Aasi, J.; Abbott, B.P.; Abbott, R.; Abbott, T.; Abernathy, M.R.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Advanced LIGO. Class. Quant. Grav. 2015, 32, 074001. [Google Scholar] [CrossRef]
- Acernese, F.A.; Agathos, M.; Agatsuma, K.; Aisa, D.; Allem, O.N.; Allocca, A.; Amarni, J.; Astone, P.; Balestri, G.; Ballardin, G.; et al. Advanced Virgo: A second-generation interferometric gravitational wave detector. Class. Quant. Grav. 2015, 32, 024001. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GWTC-1: A Gravitational-Wave Transient Catalog of Compact Binary Mergers Observed by LIGO and Virgo during the First and Second Observing Runs. Phys. Rev. X 2019, 9, 031040. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GWTC-2: Compact Binary Coalescences Observed by LIGO and Virgo During the First Half of the Third Observing Run. Phys. Rev. X 2021, 11, 021053. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, N.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agarwal, D.; et al. GWTC-3: Compact Binary Coalescences Observed by LIGO and Virgo During the Second Part of the Third Observing Run. arXiv 2021, arXiv:2111.03606. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Multi-messenger Observations of a Binary Neutron Star Merger. Astrophys. J. Lett. 2017, 848, L12. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GW190425: Observation of a Compact Binary Coalescence with Total Mass ∼3.4M⊙. Astrophys. J. Lett. 2020, 892, L3. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. Observation of Gravitational Waves from Two Neutron Star—Black Hole Coalescences. Astrophys. J. Lett. 2021, 915, L5. [Google Scholar] [CrossRef]
- Haskell, B.; Andersson, N.; D‘Angelo, C.; Degenaar, N.; Glampedakis, K.; Ho, W.C.; Lasky, P.D.; Melatos, A.; Oppenoorth, M.; Patruno, A.; et al. Gravitational waves from rapidly rotating neutron stars. Astrophys. Space Sci. Proc. 2015, 40, 85–102. [Google Scholar] [CrossRef]
- Riles, K. Searches for continuous-wave gravitational radiation. Living Rev. Rel. 2023, 26, 3. [Google Scholar] [CrossRef]
- Pagliaro, G.; Papa, M.A.; Ming, J.; Lian, J.; Tsuna, D.; Maraston, C.; Thomas, D. Continuous Gravitational Waves from Galactic Neutron Stars: Demography, Detectability, and Prospects. Astrophys. J. 2023, 952, 123. [Google Scholar] [CrossRef]
- Wette, K. Searches for continuous gravitational waves from neutron stars: A twenty-year retrospective. Astropart. Phys. 2023, 153, 102880. [Google Scholar] [CrossRef]
- Friedman, J.L.; Stergioulas, N. Rotating Relativistic Stars; Cambridge University Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Baumgarte, T.W.; Shapiro, S.L. Numerical Relativity: Solving Einstein’s Equations on the Computer; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Ellipsoidal Figures of Equilibrium; The Silliman Foundation Lectures; Yale University Press: New Haven, CT, USA, 1969. [Google Scholar]
- Shibata, M.; Karino, S.; Eriguchi, Y. Dynamical instability of differentially rotating stars. Mon. Not. R. Astron. Soc. 2002, 334, L27. [Google Scholar] [CrossRef]
- Shibata, M.; Karino, S.; Eriguchi, Y. Dynamical bar-mode instability of differentially rotating stars: Effects of equations of state and velocity profiles. Mon. Not. R. Astron. Soc. 2003, 343, 619. [Google Scholar] [CrossRef]
- Watts, A.L.; Andersson, N.; Jones, D.I. The Nature of Low T/|W| Dynamical Instabilities in Differentially Rotating Stars. Astrophys. J. Lett. 2005, 618, L37–L40. [Google Scholar] [CrossRef]
- Roberts, P.H.; Stewartson, K. On the Stability of a Maclaurin Spheroid of Small Viscosity. Astrophys. J. 1963, 137, 777. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Solutions of Two Problems in the Theory of Gravitational Radiation. Phys. Rev. Lett. 1970, 24, 611–615. [Google Scholar] [CrossRef]
- Friedman, J.L.; Schutz, B.F. Secular instability of rotating Newtonian stars. Astrophys. J. 1978, 222, 281–296. [Google Scholar] [CrossRef]
- Friedman, J.L. Generic instability of rotating relativistic stars. Commun. Math. Phys. 1978, 62, 247–278. [Google Scholar] [CrossRef]
- Lai, D.; Rasio, F.A.; Shapiro, S.L. Ellipsoidal figures of equilibrium—Compressible models. Astrophys. J. Suppl. Ser. 1993, 88, 205–252. [Google Scholar] [CrossRef]
- Lai, D.; Shapiro, S.L. Gravitational radiation from rapidly rotating nascent neutron stars. Astrophys. J. 1995, 442, 259–272. [Google Scholar] [CrossRef]
- Christodoulou, D.M.; Kazanas, D.; Shlosman, I.; Tohline, J.E. Phase-Transition Theory of Instabilities. I. Second-Harmonic Instability and Bifurcation Points. Astrophys. J. 1995, 446, 472. [Google Scholar] [CrossRef]
- Lindblom, L.; Detweiler, S.L. On the secular instabilities of the Maclaurin spheroids. Astrophys. J. 1977, 211, 565–567. [Google Scholar] [CrossRef]
- James, R.A. The Structure and Stability of Rotating Gas Masses. Astrophys. J. 1964, 140, 552. [Google Scholar] [CrossRef]
- Ipser, J.R.; Managan, R.A. On the existence and structure of inhomogeneous analogs of the Dedekind and Jacobi ellipsoids. Astrophys. J. 1981, 250, 362–372. [Google Scholar] [CrossRef]
- Bonazzola, S.; Frieben, J.; Gourgoulhon, E. Spontaneous Symmetry Breaking of Rapidly Rotating Stars in General Relativity. Astrophys. J. 1996, 460, 379. [Google Scholar] [CrossRef]
- Skinner, D.; Lindblom, L. On the Viscosity-driven Secular Instability in Rotating Neutron Stars. Astrophys. J. 1996, 461, 920. [Google Scholar] [CrossRef]
- Bonazzola, S.; Frieben, J.; Gourgoulhon, E. Spontaneous symmetry breaking of rapidly rotating stars in general relativity: Influence of the 3D-shift vector. Astron. Astrophys. 1998, 331, 280–290. [Google Scholar]
- Gondek-Rosińska, D.; Gourgoulhon, E. Jacobi-like bar mode instability of relativistic rotating bodies. Phys. Rev. D 2002, 66, 044021. [Google Scholar] [CrossRef]
- Stergioulas, N.; Friedman, J.L. Nonaxisymmetric Neutral Modes in Rotating Relativistic Stars. Astrophys. J. 1998, 492, 301. [Google Scholar] [CrossRef]
- Morsink, S.M.; Stergioulas, N.; Blattnig, S.R. Quasi-normal Modes of Rotating Relativistic Stars – Neutral Modes for Realistic Equations of State. Astrophys. J. 1999, 510, 854. [Google Scholar] [CrossRef]
- Huang, X.; Markakis, C.; Sugiyama, N.; Uryū, K. Quasi-equilibrium models for triaxially deformed rotating compact stars. Phys. Rev. D 2008, D78, 124023. [Google Scholar] [CrossRef]
- Uryū, K.; Tsokaros, A.; Galeazzi, F.; Hotta, H.; Sugimura, M.; Taniguchi, K.; Yoshida, S. New code for equilibriums and quasiequilibrium initial data of compact objects. III. Axisymmetric and triaxial rotating stars. Phys. Rev. D 2016, D93, 044056. [Google Scholar] [CrossRef]
- Uryū, K.; Tsokaros, A.; Baiotti, L.; Galeazzi, F.; Sugiyama, N.; Taniguchi, K.; Yoshida, S. Do triaxial supramassive compact stars exist? Phys. Rev. D 2016, 94, 101302. [Google Scholar] [CrossRef]
- Cook, G.B.; Shapiro, S.L.; Teukolsky, S.A. Spin-up of a rapidly rotating star by angular momentum loss—Effects of general relativity. Astrophys. J. 1992, 398, 203–223. [Google Scholar] [CrossRef]
- Tsokaros, A.; Ruiz, M.; Paschalidis, V.; Shapiro, S.L.; Baiotti, L.; Uryū, K. Gravitational wave content and stability of uniformly, rotating, triaxial neutron stars in general relativity. Phys. Rev. D 2017, 95, 124057. [Google Scholar] [CrossRef]
- Zhou, E.; Tsokaros, A.; Rezzolla, L.; Xu, R.; Uryū, K. Uniformly rotating, axisymmetric and triaxial quark stars in general relativity. Phys. Rev. 2018, D97, 023013. [Google Scholar] [CrossRef]
- Brandt, S.R.; Brendal, B.; Gabella, W.E.; Haas, R.; Karakaş, B.; Kedia, A.; Rosofsky, S.G.; Schaffarczyk, A.P.; Alcubierre, M.; Alic, D.; et al. The Einstein Toolkit, Turing release, ET_2020_05 2020; Zenodo: Geneva, Switzerland, 2020. [CrossRef]
- Etienne, Z.B.; Paschalidis, V.; Haas, R.; Mösta, P.; Shapiro, S.L. IllinoisGRMHD: An open-source, user-friendly GRMHD code for dynamical spacetimes. Class. Quantum Grav. 2015, 32, 175009. [Google Scholar] [CrossRef]
- Noble, S.C.; Gammie, C.F.; McKinney, J.C.; Del Zanna, L. Primitive Variable Solvers for Conservative General Relativistic Magnetohydrodynamics. Astrophys. J. 2006, 641, 626–637. [Google Scholar] [CrossRef]
- Schnetter, E.; Hawley, S.H.; Hawke, I. Evolutions in 3D numerical relativity using fixed mesh refinement 2003. Class. Quantum Gravity 2004, 21, 1465. [Google Scholar] [CrossRef]
- Dreyer, O.; Krishnan, B.; Shoemaker, D.; Schnetter, E. Introduction to isolated horizons in numerical relativity. Phys. Rev. D 2003, 67, 024018. [Google Scholar] [CrossRef]
- Tsokaros, A.; Uryū, K. Methods for relativistic self-gravitating fluids: From binary neutron stars to black hole-disks and magnetized rotating neutron stars. Gen. Relativ. Gravit. 2022, 54, 52. [Google Scholar] [CrossRef]
- Isenberg, J.A. Waveless Approximation Theories of Gravity. Int. J. Mod. Phys. 2008, 17, 265–273. [Google Scholar] [CrossRef]
- Wilson, J.R.; Mathews, G.J. Relativistic hydrodynamics. In Frontiers in Numerical Relativity; Evans, C.R., Finn, L.S., Hobill, D.W., Eds.; Cambridge University Press: Cambridge, MA, USA, 1989; pp. 306–314. [Google Scholar]
- Wilson, J.R.; Mathews, G.J. Instabilities in Close Neutron Star Binaries. Phys. Rev. Lett. 1995, 75, 4161. [Google Scholar] [CrossRef]
- Wilson, J.R.; Mathews, G.J.; Marronetti, P. Relativistic numerical model for close neutron-star binaries. Phys. Rev. D 1996, 54, 1317–1331. [Google Scholar] [CrossRef]
- Friedman, J.L.; Ipser, J.R.; Parker, L. Rapidly Rotating Neutron Star Models. Astrophys. J. 1986, 304, 115. [Google Scholar] [CrossRef]
- Beig, R. Arnowitt-Deser-Misner energy and g00. Phys. Lett. A 1978, 69, 153–155. [Google Scholar] [CrossRef]
- Gourgoulhon, E.; Bonazzola, S. A formulation of the virial theorem in general relativity. Class. Quantum Gravity 1994, 11, 443–452. [Google Scholar] [CrossRef]
- Komatsu, H.; Eriguchi, Y.; Hachisu, I. Rapidly rotating general relativistic stars. I - Numerical method and its application to uniformly rotating polytropes. Mon. Not. R. Astron. Soc. 1989, 237, 355–379. [Google Scholar] [CrossRef]
- Komatsu, H.; Eriguchi, Y.; Hachisu, I. Rapidly rotating general relativistic stars. II – Differentially rotating polytropes. Mon. Not. R. Astron. Soc. 1989, 239, 153–171. [Google Scholar] [CrossRef]
- Ruchlin, I.; Etienne, Z.B.; Baumgarte, T.W. SENR/NRPy+: Numerical Relativity in Singular Curvilinear Coordinate Systems. Phys. Rev. 2018, D97, 064036. [Google Scholar] [CrossRef]
- Baker, J.G.; Centrella, J.; Choi, D.I.; Koppitz, M.; van Meter, J. Gravitational wave extraction from an inspiraling configuration of merging black holes. Phys. Rev. Lett. 2006, 96, 111102. [Google Scholar] [CrossRef]
- Campanelli, M.; Lousto, C.O.; Marronetti, P.; Zlochower, Y. Accurate Evolutions of Orbiting Black-Hole Binaries without Excision. Phys. Rev. Lett. 2006, 96, 111101. [Google Scholar] [CrossRef]
- Alcubierre, M.; Brügmann, B.; Diener, P.; Koppitz, M.; Pollney, D.; Seidel, E.; Takahashi, R. Gauge conditions for long-term numerical black hole evolutions without excision. Phys. Rev. D 2003, 67, 084023. [Google Scholar] [CrossRef]
- Del Zanna, L.; Bucciantini, N. An efficient shock-capturing central-type scheme for multidimensional relativistic flows. I. Hydrodynamics. Astron. Astrophys. 2002, 390, 1177–1186. [Google Scholar] [CrossRef]
- Reisswig, C.; Pollney, D. Notes on the integration of numerical relativity waveforms. Class. Quantum Grav. 2011, 28, 195015. [Google Scholar] [CrossRef]
- Updated Advanced LIGO Sensitivity Design Curve, LIGO Document T1800044-v5. 2023. Available online: https://dcc.ligo.org/LIGO-T1800044-v5/public (accessed on 4 March 2024).
- ET-B Sensitivity Curve, 2023. ET Document ET-0002A-18. Available online: https://apps.et-gw.eu/tds/ql/?c=13222 (accessed on 4 March 2024).
- Towns, J.; Cockerill, T.; Dahan, M.; Foster, I.; Gaither, K.; Grimshaw, A.; Hazlewood, V.; Lathrop, S.; Lifka, D.; Peterson, G.D.; et al. XSEDE: Accelerating Scientific Discovery. Comput. Sci. Eng. 2014, 16, 62–74. [Google Scholar] [CrossRef]
- Boerner, T.J.; Deems, S.; Furlani, T.R.; Knuth, S.L.; Towns, J. ACCESS: Advancing Innovation: NSF’s Advanced Cyberinfrastructure Coordination Ecosystem: Services & Support. In Proceedings of the In Practice and Experience in Advanced Research Computing (PEARC ’23), ACM, Portland, OR, USA, 23–27 July 2023; p. 4. [Google Scholar] [CrossRef]
- Hunter, J.D. Matplotlib: A 2D graphics environment. Comput. Sci. Eng. 2007, 9, 90–95. [Google Scholar] [CrossRef]
- Caswell, T.A.; Droettboom, M.; Lee, A.; de Andrade, E.S.; Hoffmann, T.; Hunter, J.; Klymak, J.; Firing, E.; Stansby, D.; Varoquaux, N.; et al. matplotlib, v3.4.3; Zenodo: Geneva, Switzerland, 2021. [CrossRef]
- Bozzola, G. kuibit: Analyzing Einstein Toolkit simulations with Python. J. Open Source Softw. 2021, 6, 3099. [Google Scholar] [CrossRef]



| Model | e | |||||
|---|---|---|---|---|---|---|
| C010s17 | ||||||
| C019s08 | ||||||
| Model | ||||||
| C010s17 | ||||||
| C019s08 |
| : Radial coordinate where the radial grids start. | |
| : Radial coordinate where the radial grids end. | |
| : Radial coordinate between and where the radial grid spacing changes. | |
| : Number of intervals in . | |
| : Number of intervals in . | |
| : Number of intervals in . | |
| : Number of intervals in . | |
| : Number of intervals in . | |
| : Order of included multipoles. |
| Model | Grid Hierarchy | N | |
|---|---|---|---|
| C010s17 | 80 | ||
| C019s08 | 80 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, Y.; Tsokaros, A.; Haas, R.; Uryū, K. General Relativistic Stability and Gravitational Wave Content of Rotating Triaxial Neutron Stars. Symmetry 2024, 16, 343. https://doi.org/10.3390/sym16030343
Luo Y, Tsokaros A, Haas R, Uryū K. General Relativistic Stability and Gravitational Wave Content of Rotating Triaxial Neutron Stars. Symmetry. 2024; 16(3):343. https://doi.org/10.3390/sym16030343
Chicago/Turabian StyleLuo, Yufeng, Antonios Tsokaros, Roland Haas, and Kōji Uryū. 2024. "General Relativistic Stability and Gravitational Wave Content of Rotating Triaxial Neutron Stars" Symmetry 16, no. 3: 343. https://doi.org/10.3390/sym16030343
APA StyleLuo, Y., Tsokaros, A., Haas, R., & Uryū, K. (2024). General Relativistic Stability and Gravitational Wave Content of Rotating Triaxial Neutron Stars. Symmetry, 16(3), 343. https://doi.org/10.3390/sym16030343






