PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process
Abstract
:1. Introduction
2. Seven Classes of Fractional Vibrators
2.1. Motion Equations
2.2. Frequency Transfer Functions
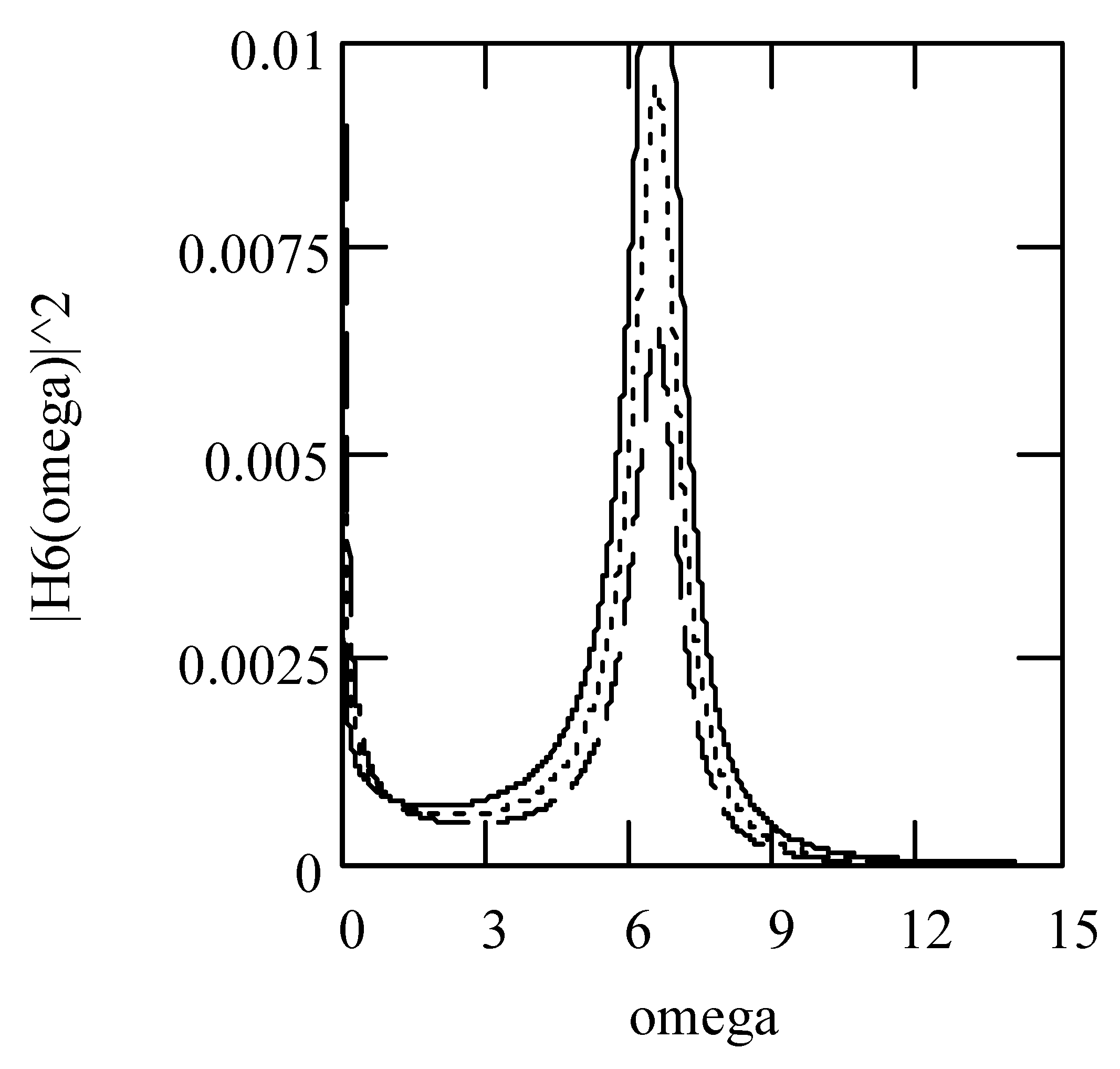
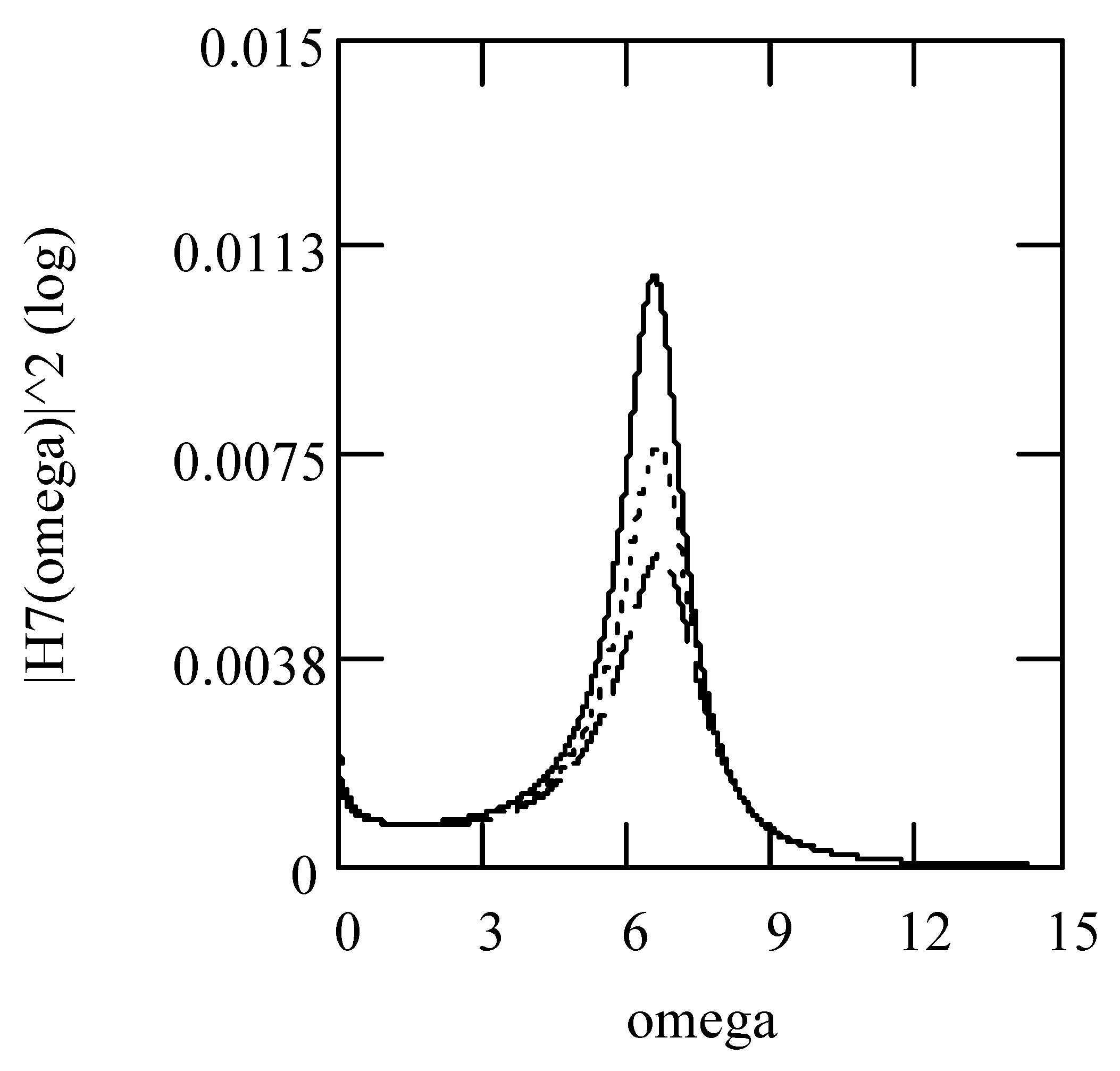
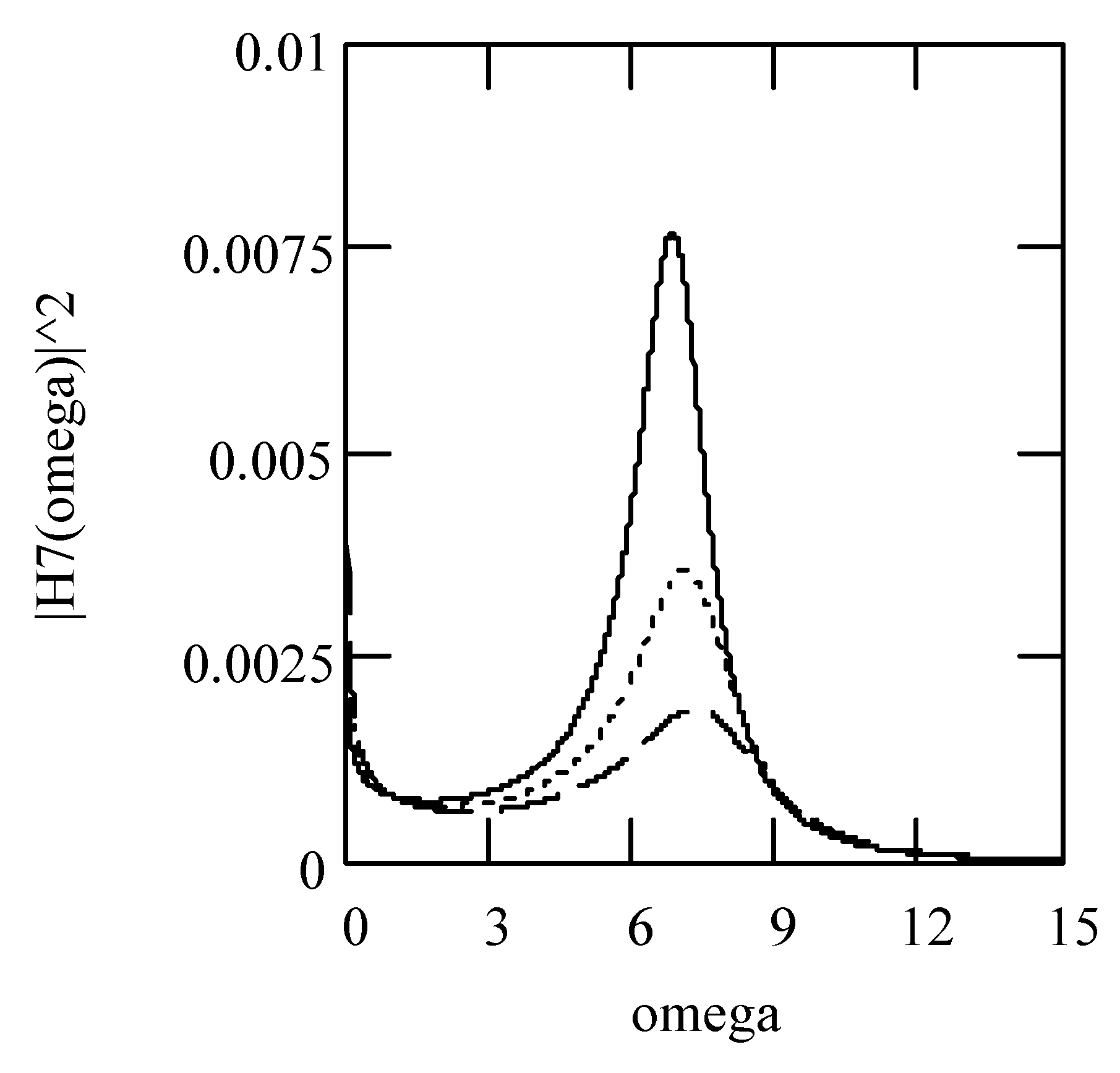
2.3. Impulse Response Functions
3. PSD and Cross-PSD Responses to Seven Classes of Fractional Vibrations Driven by fGn
3.1. Background
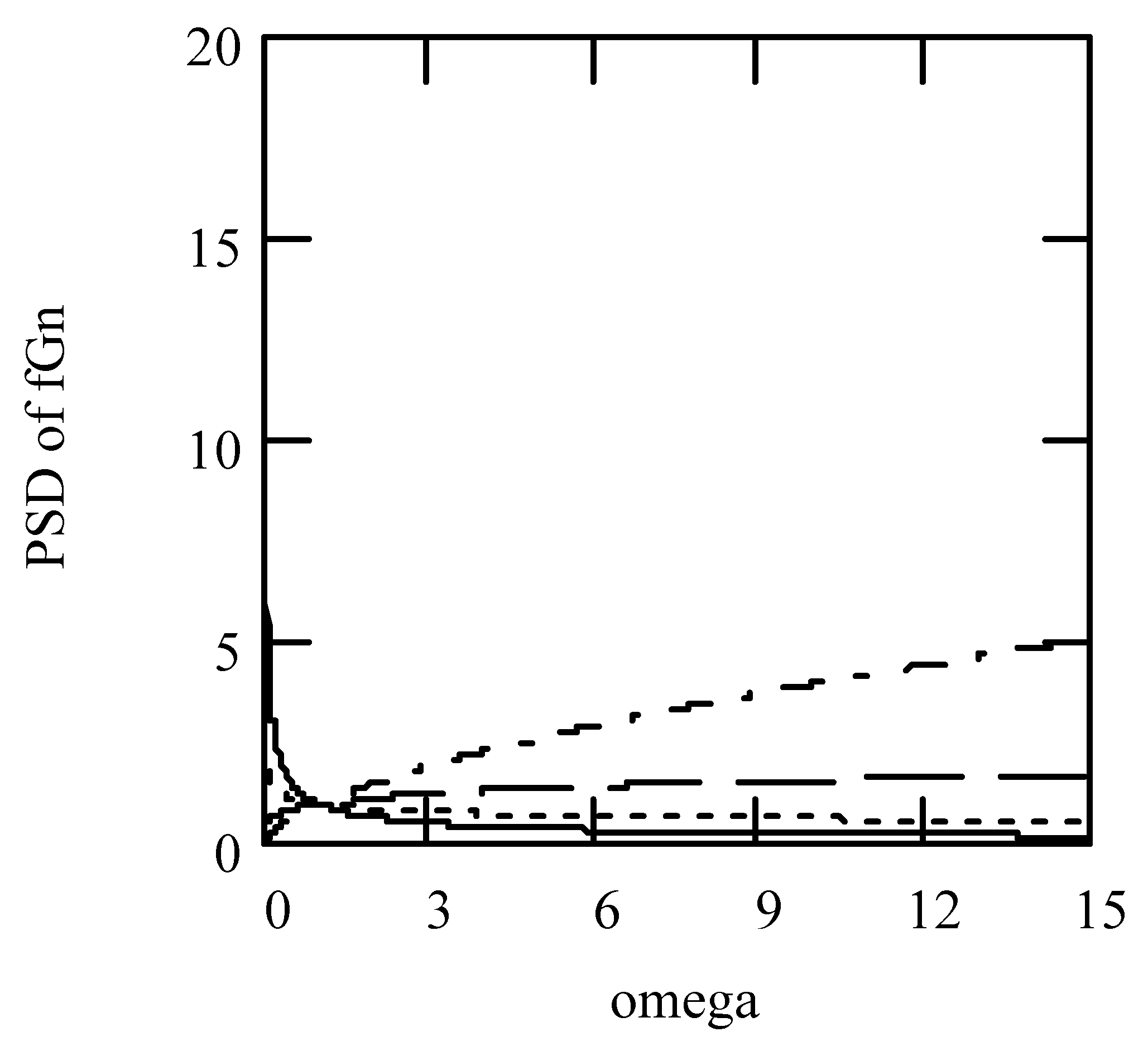
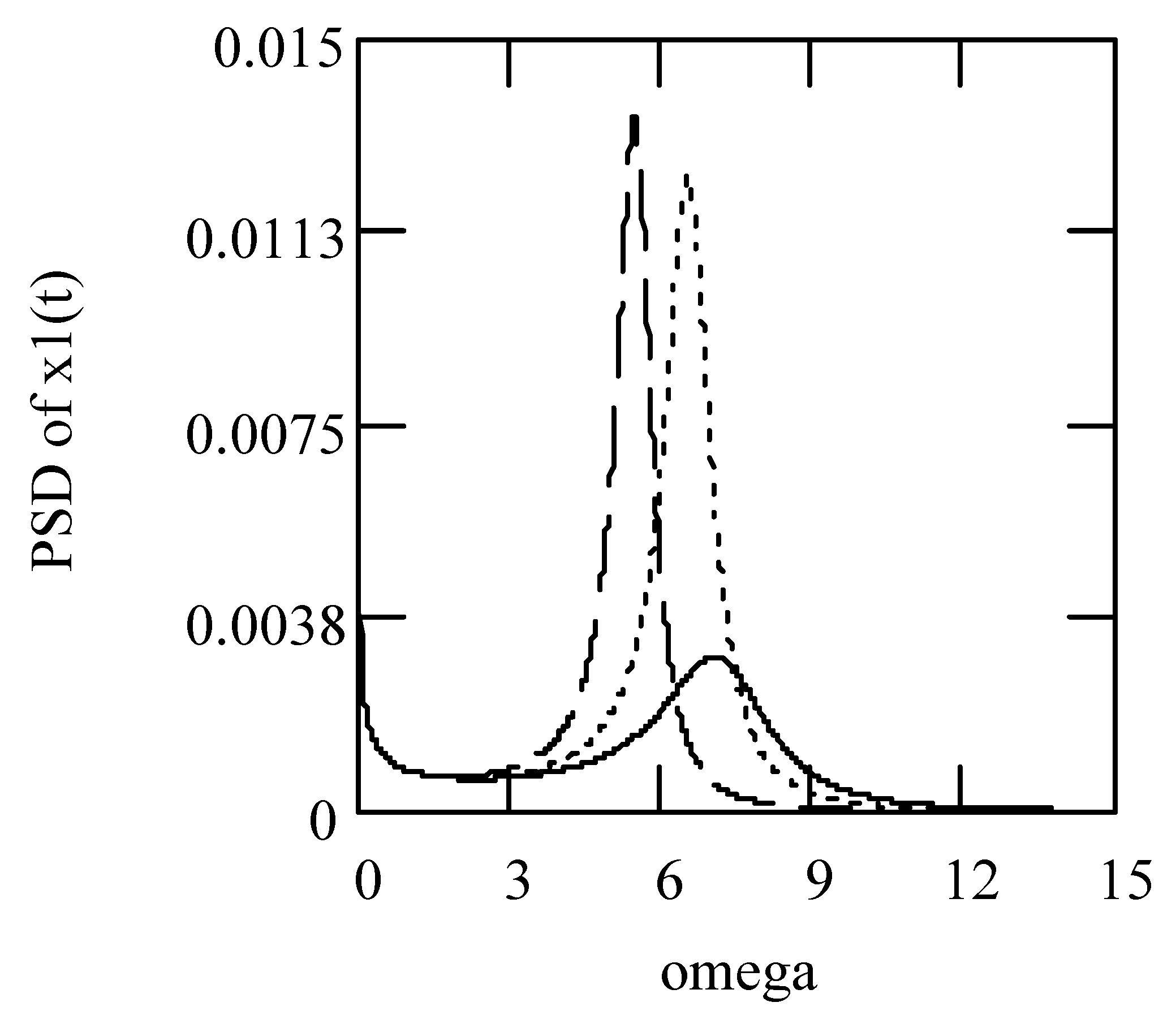
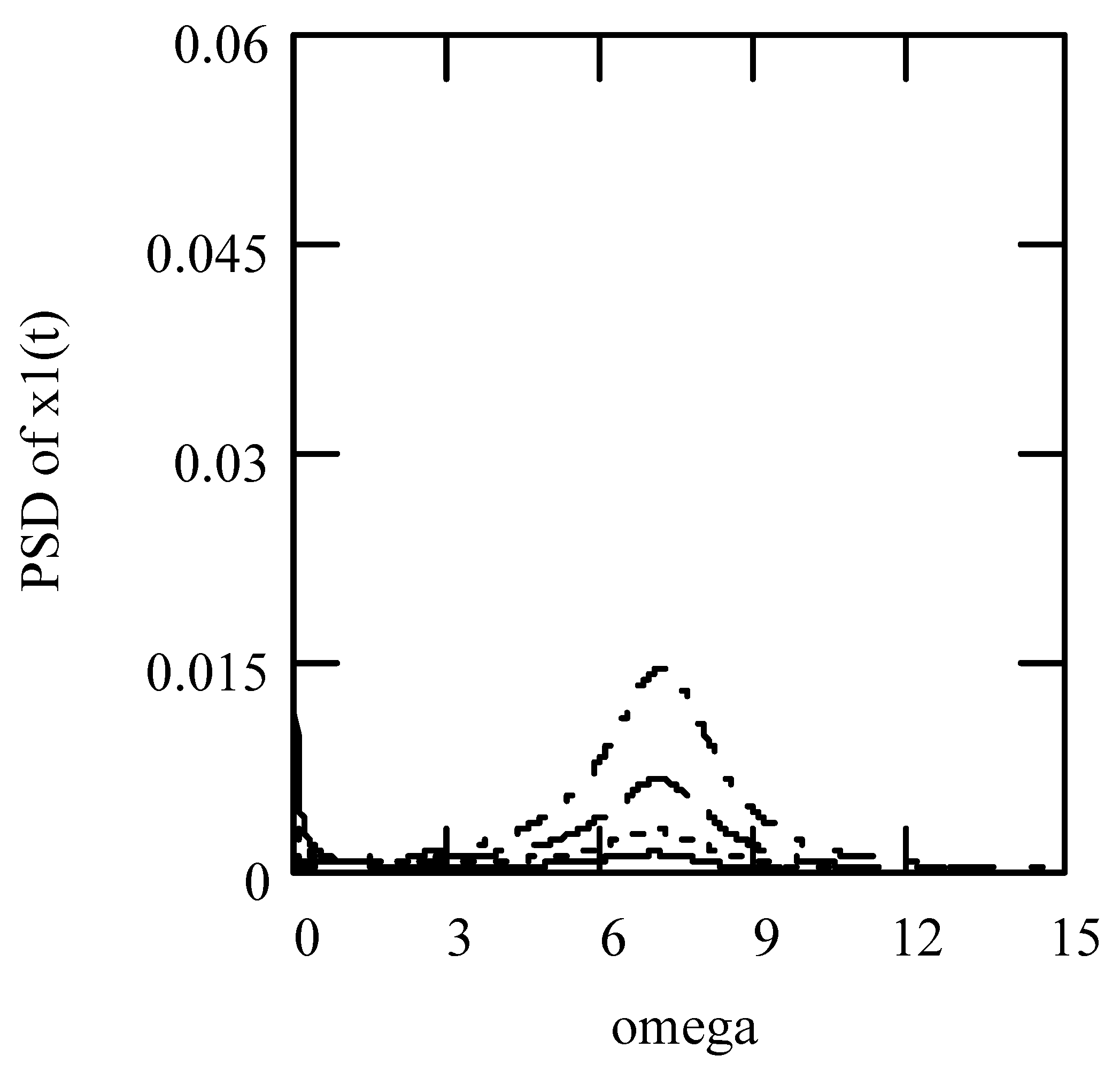
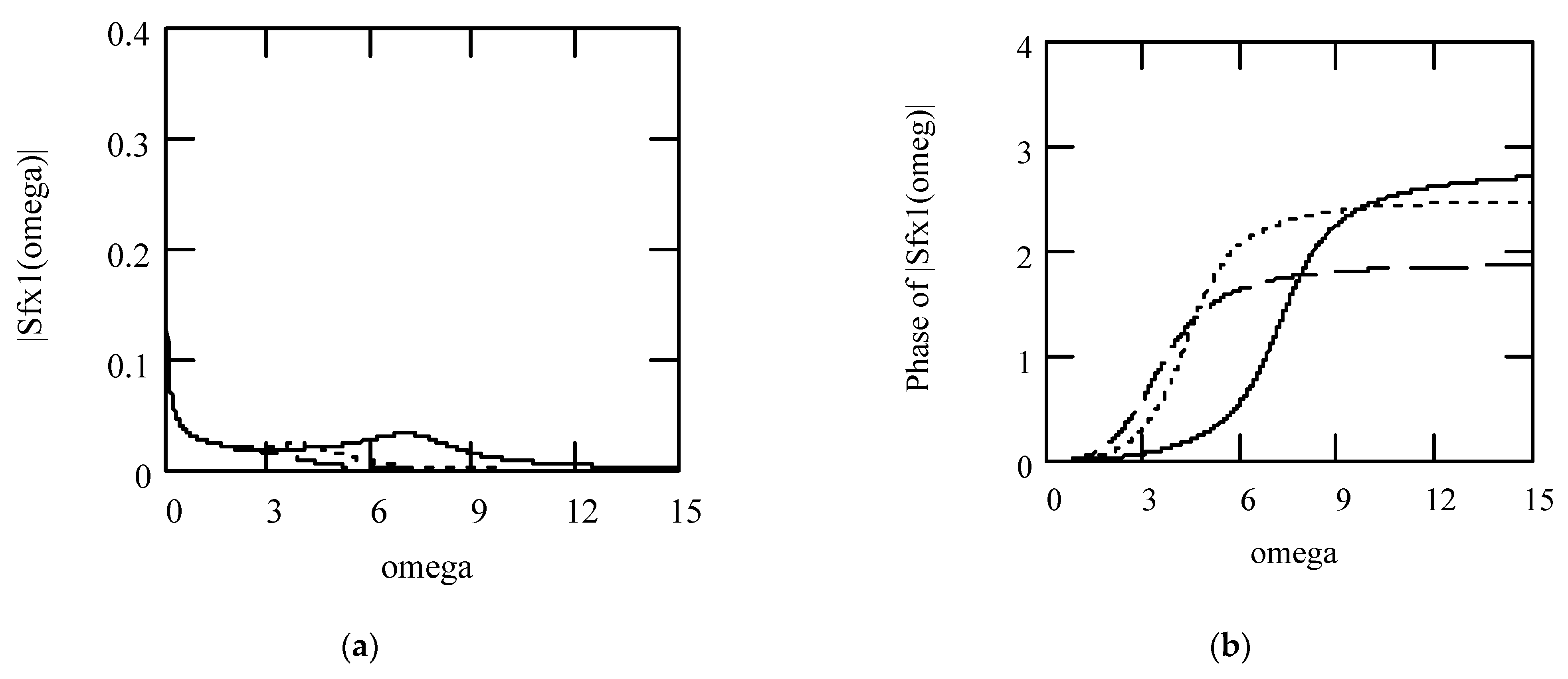
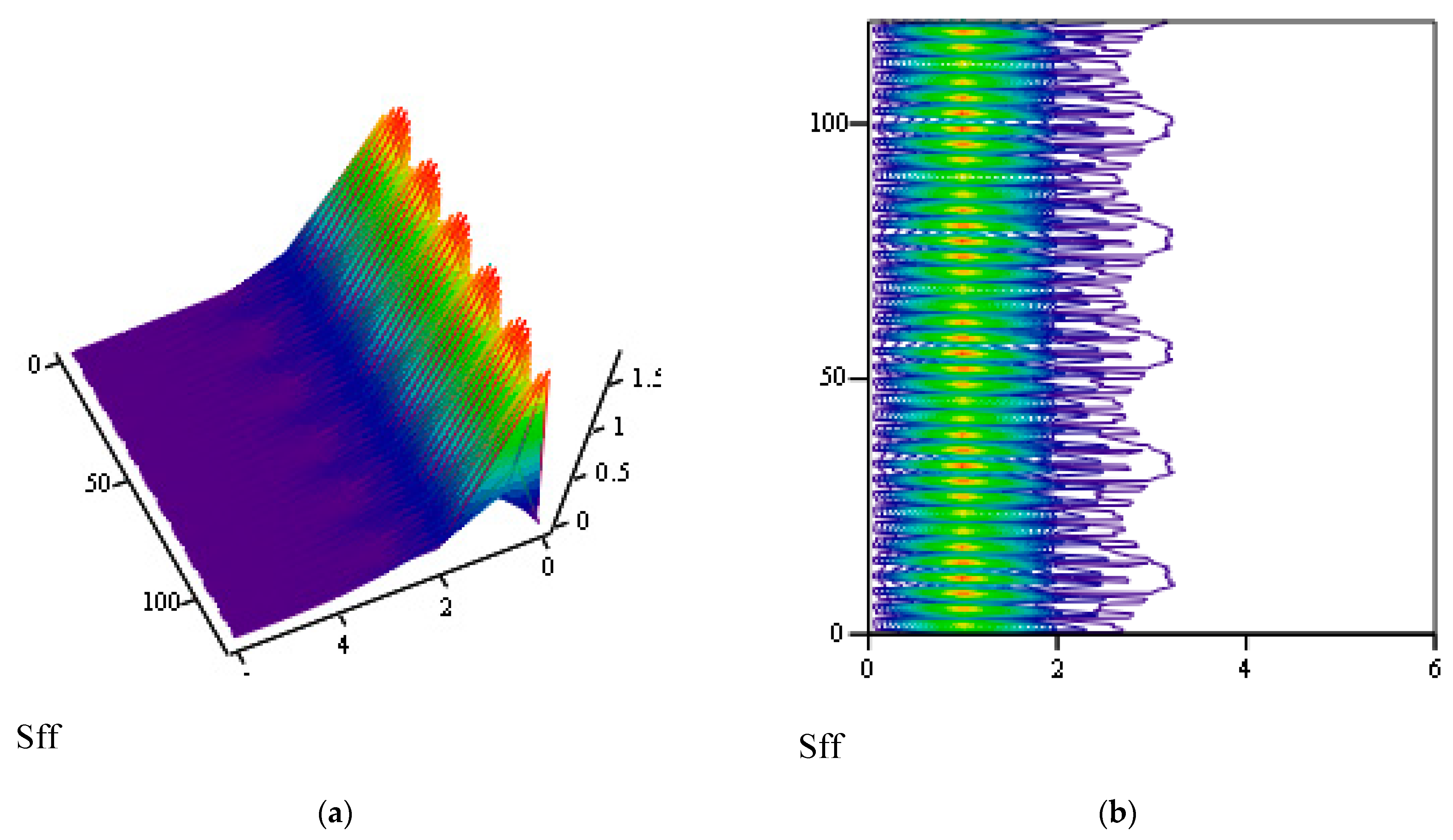
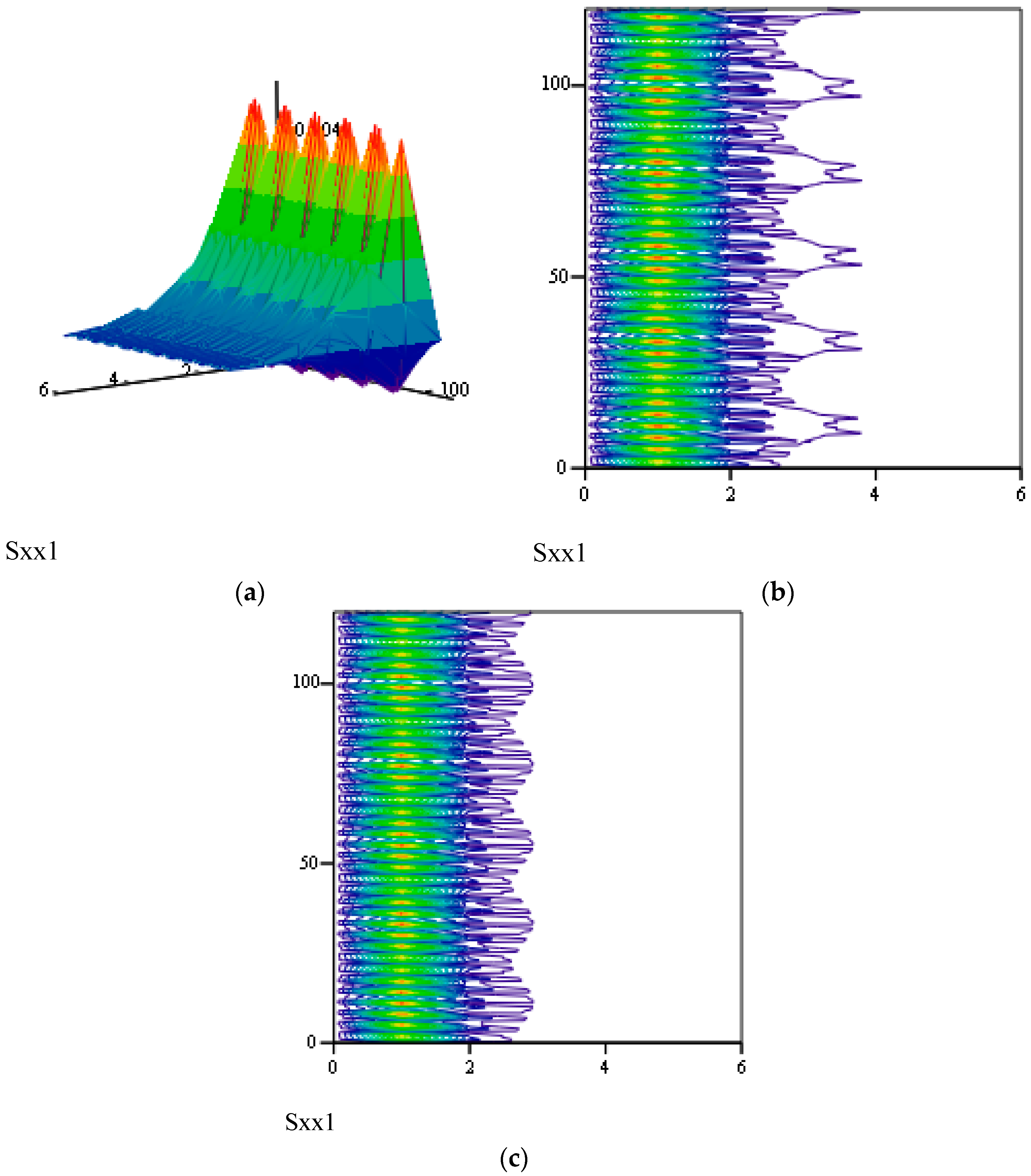
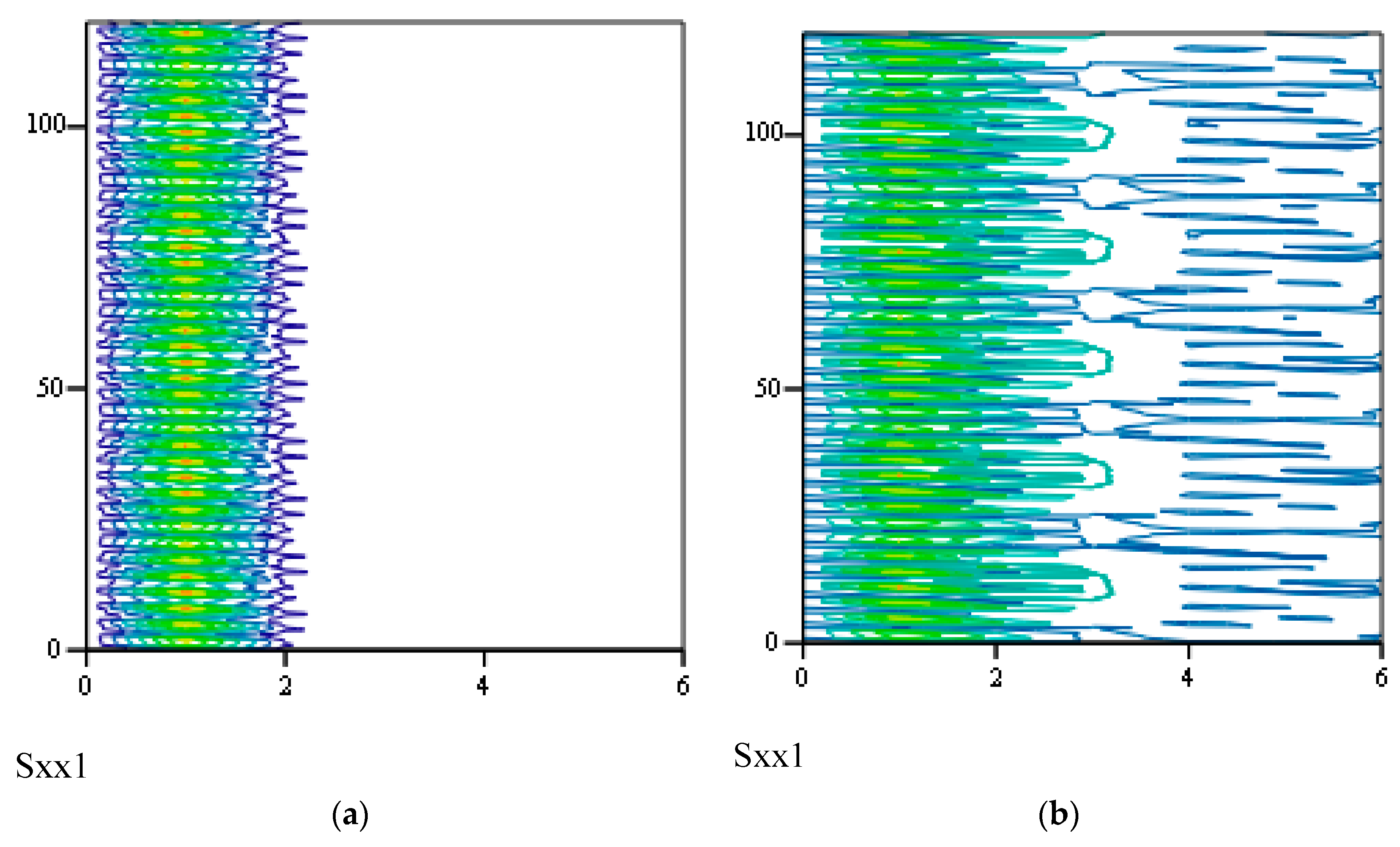
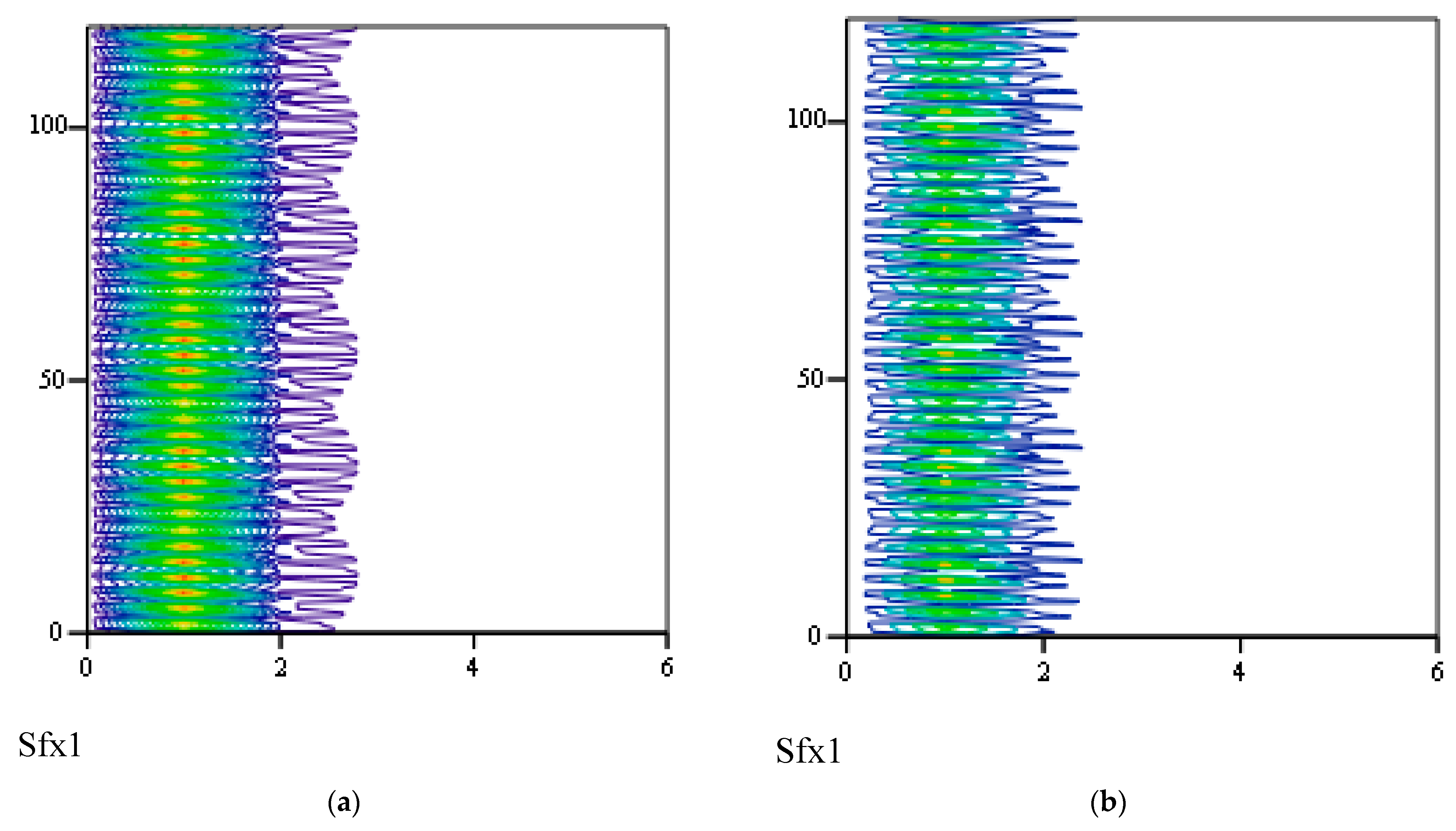
3.2. Responses of Class I Fractional Vibrators Driven by fGn
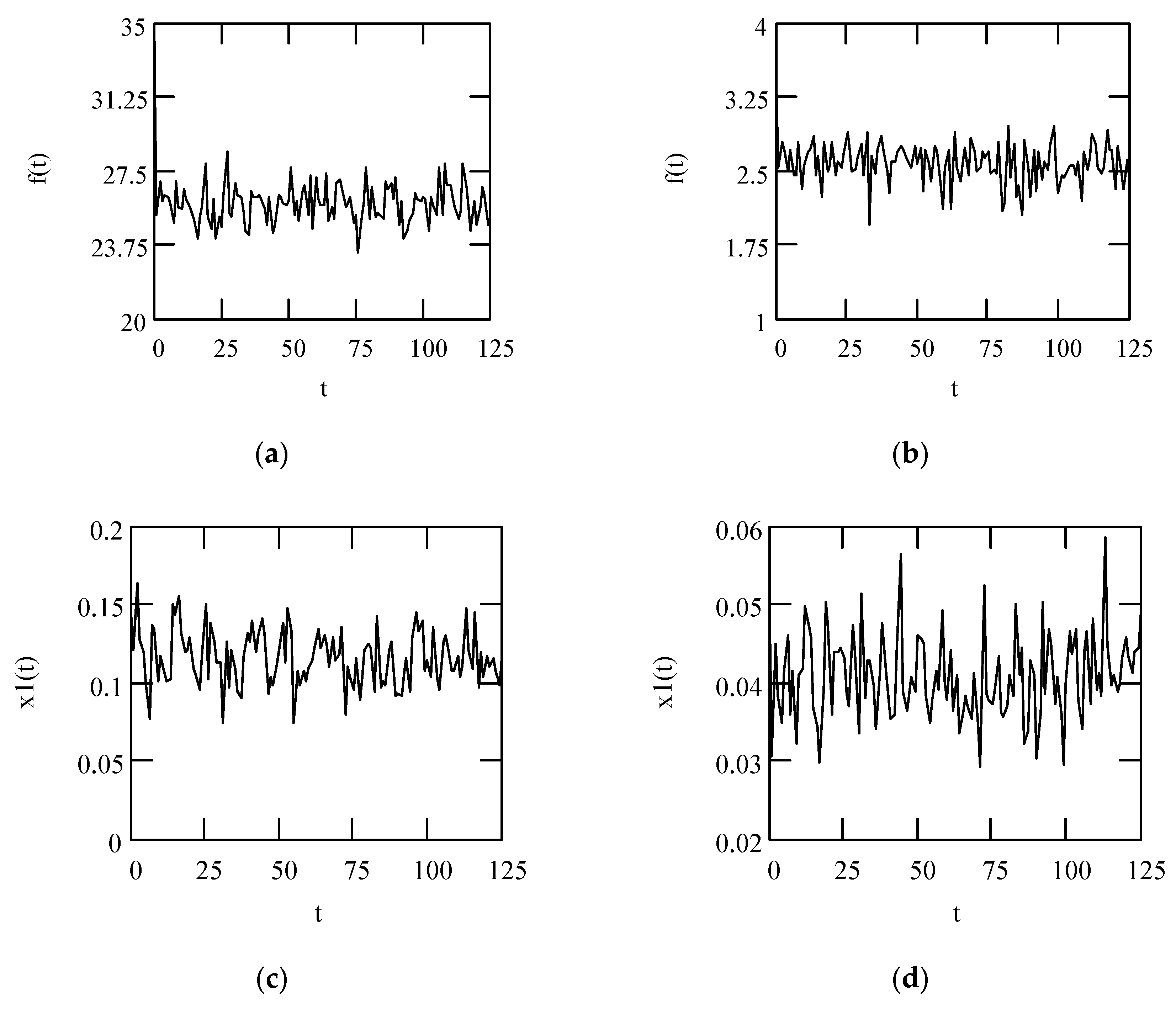
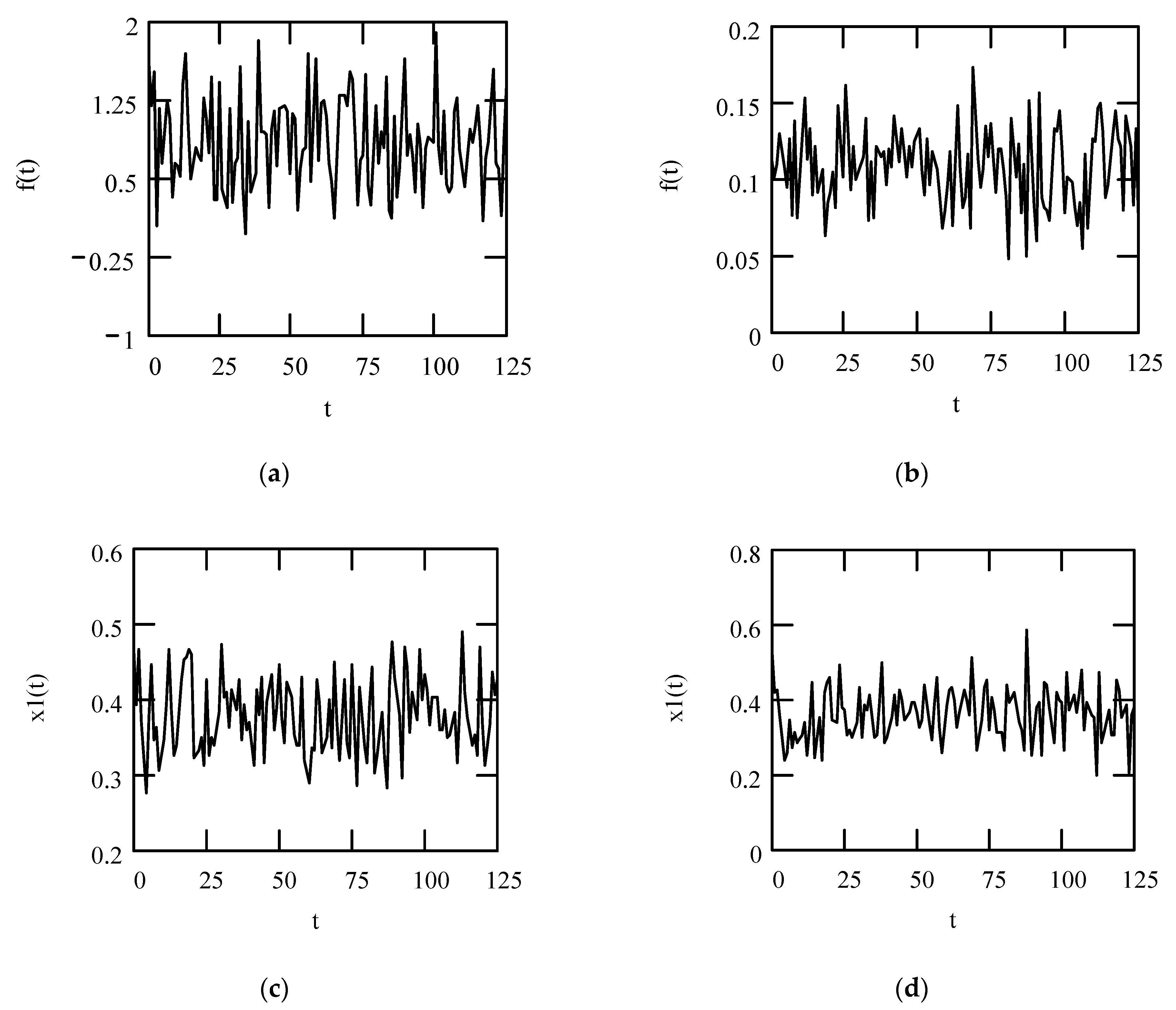
3.2.1. Computations
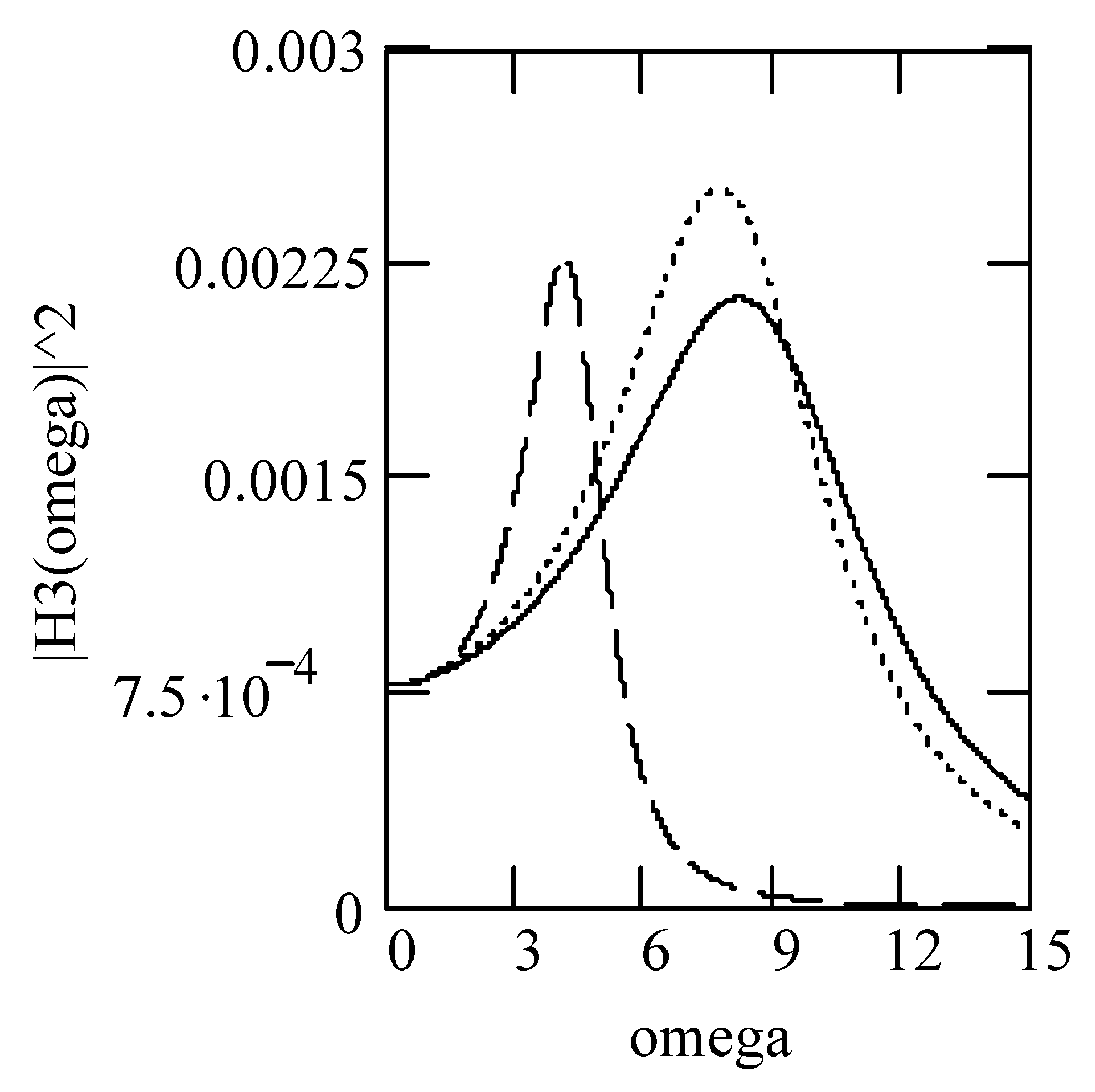
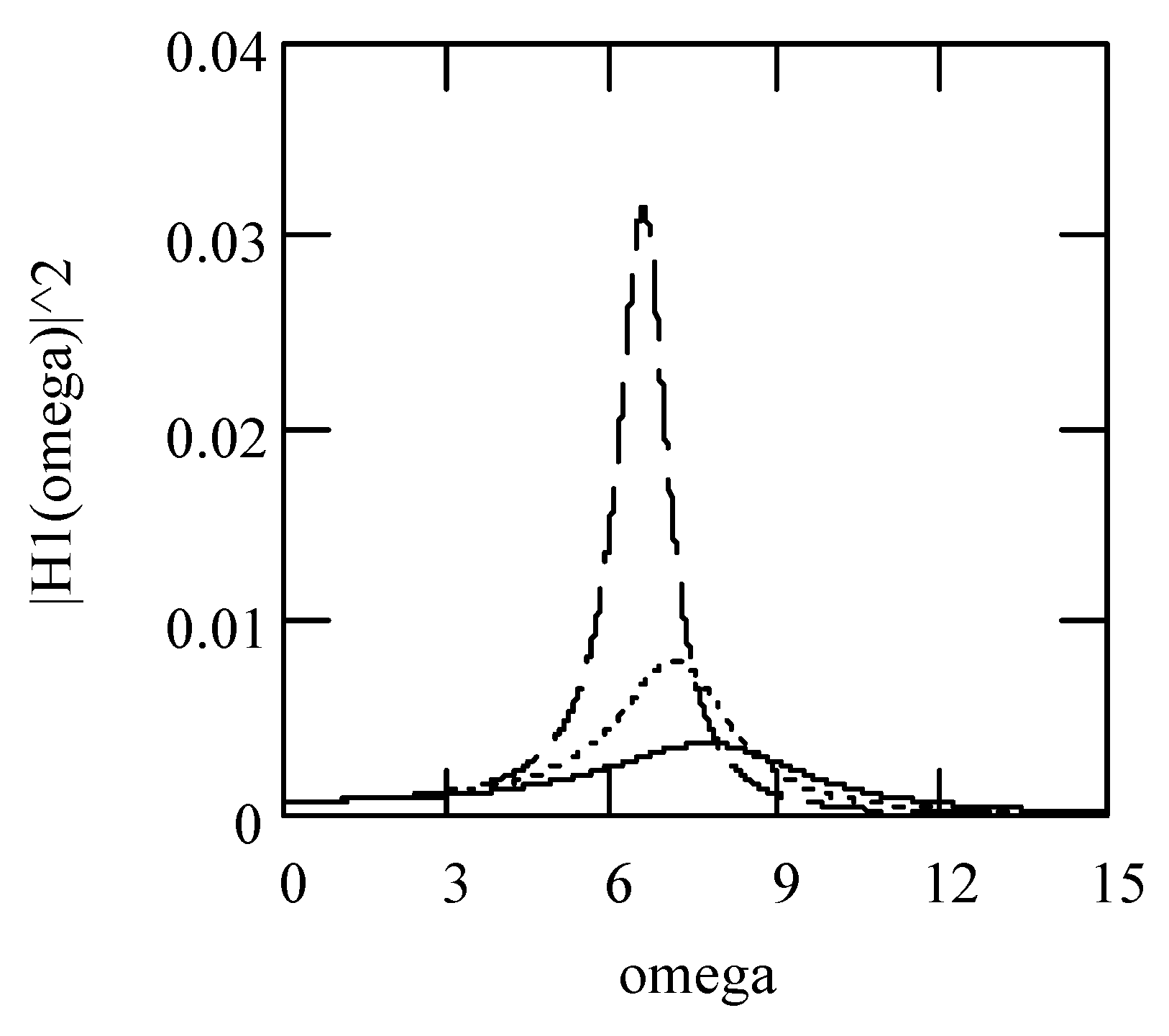
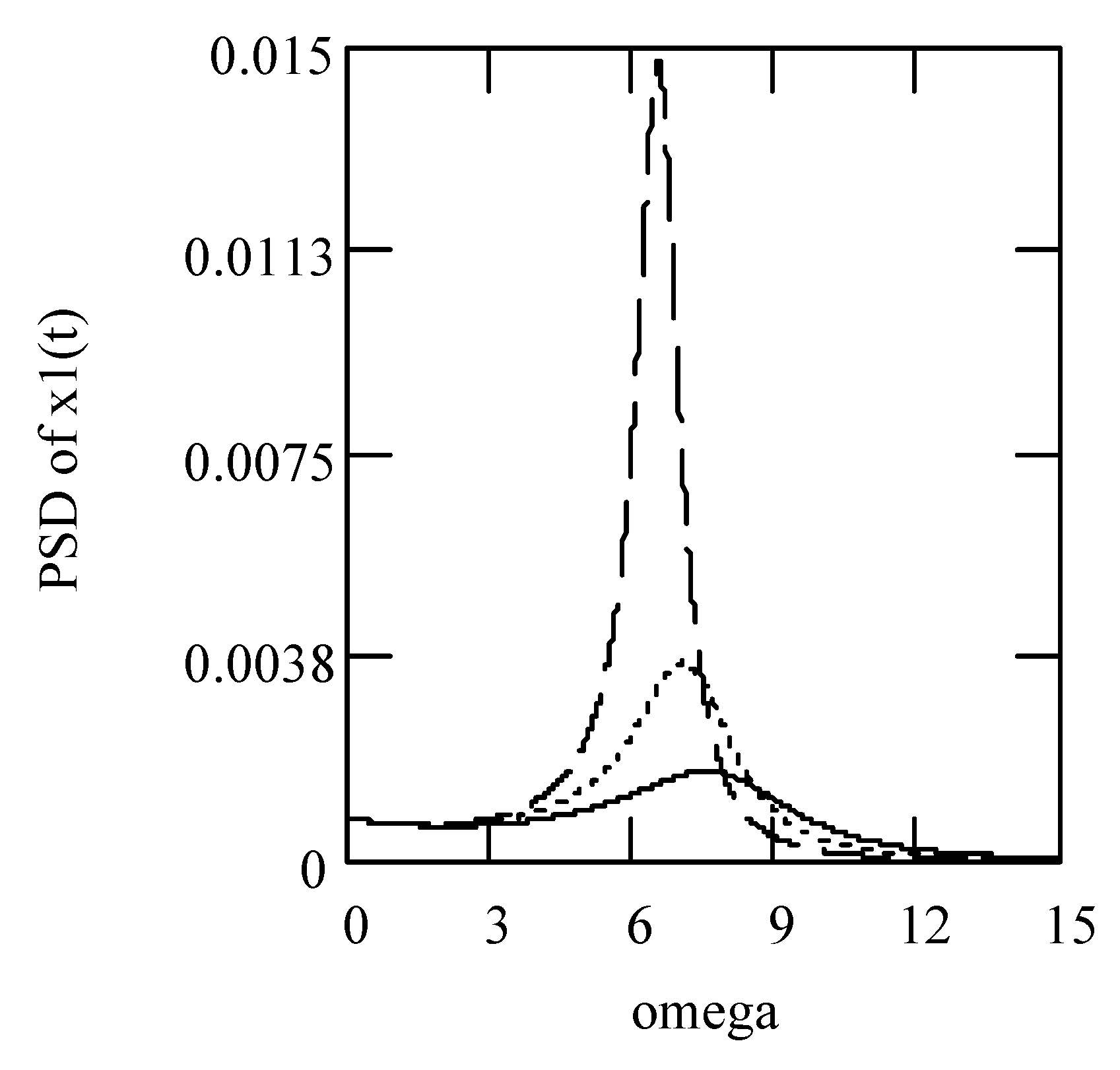
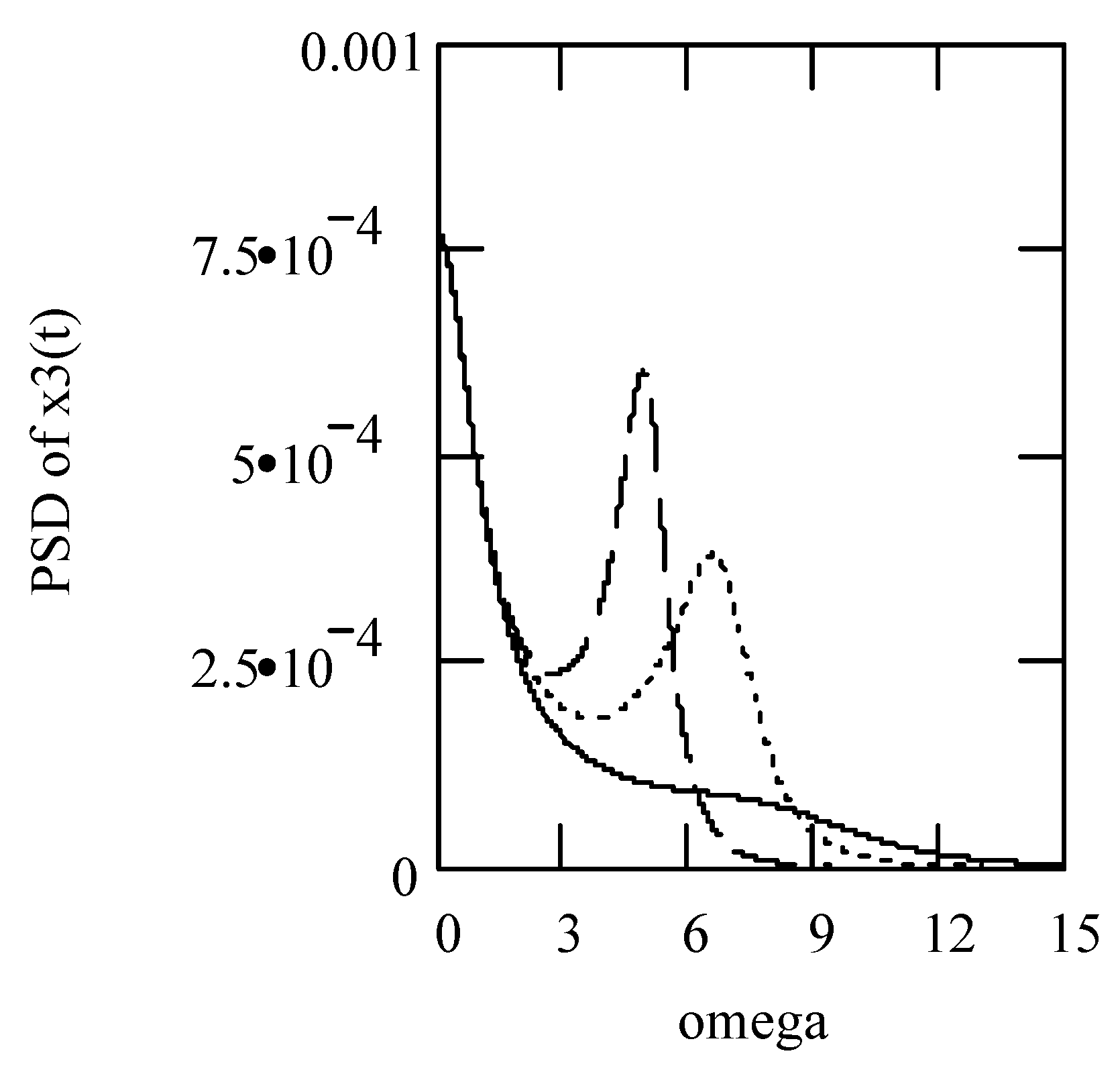
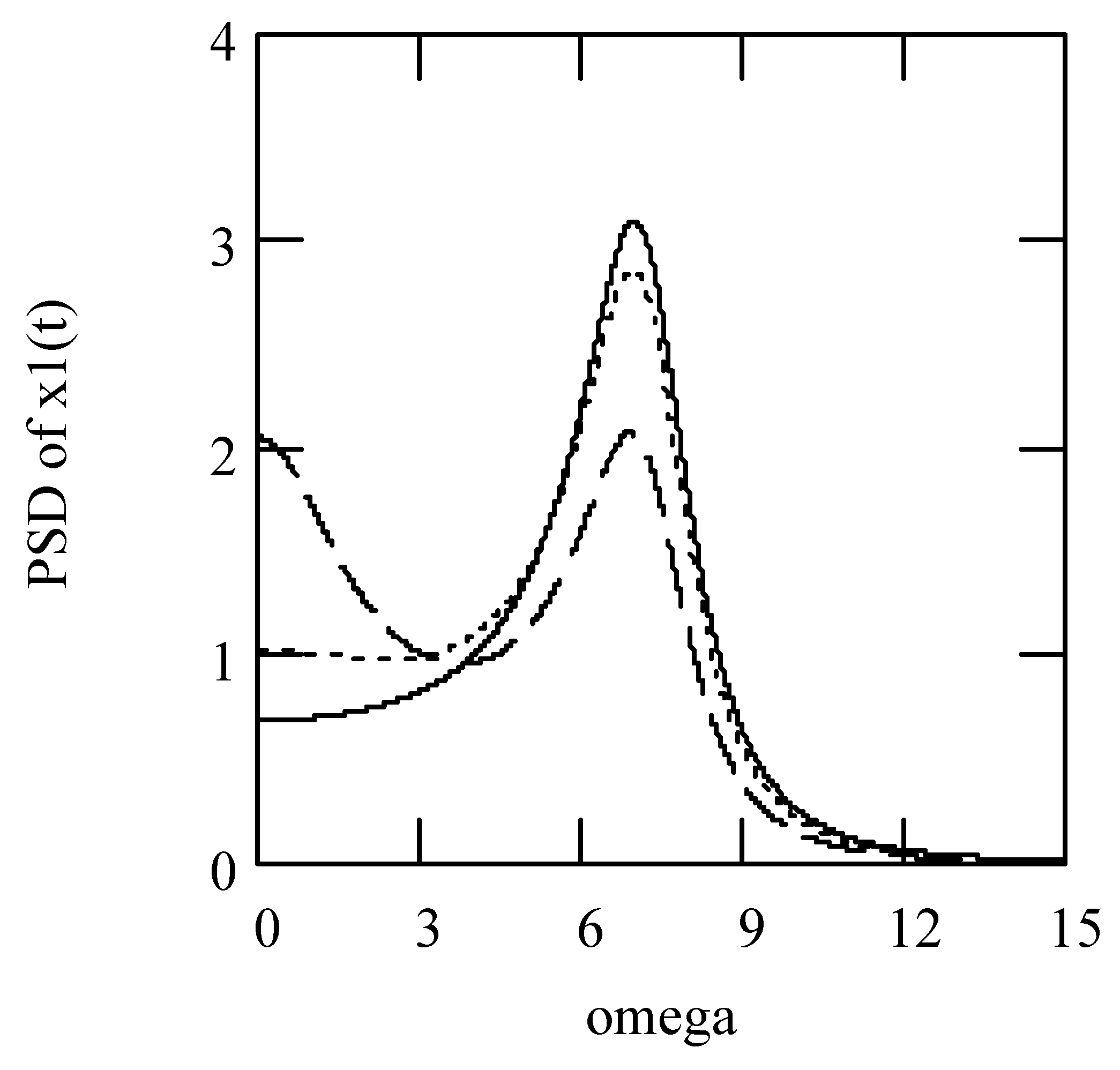
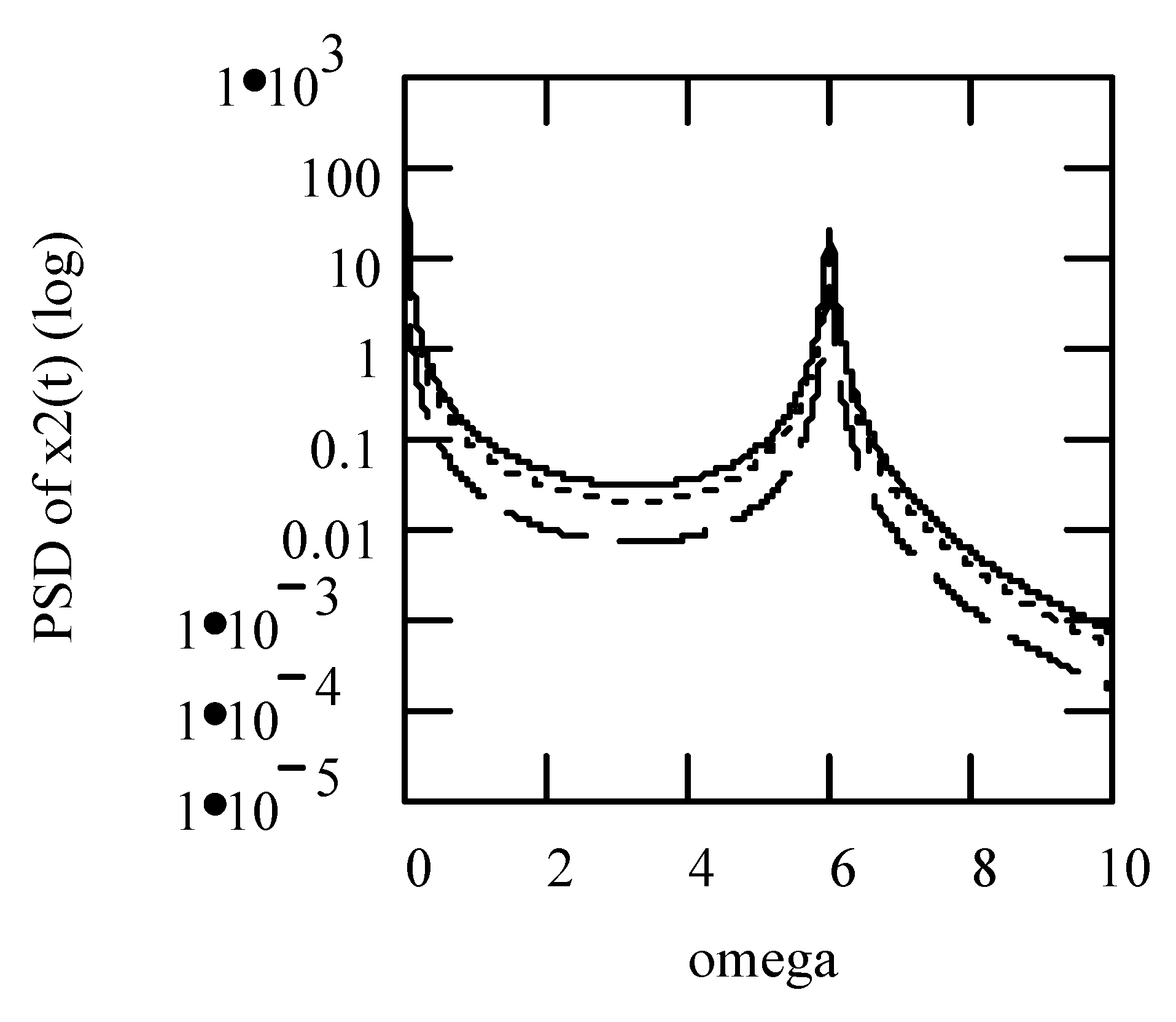
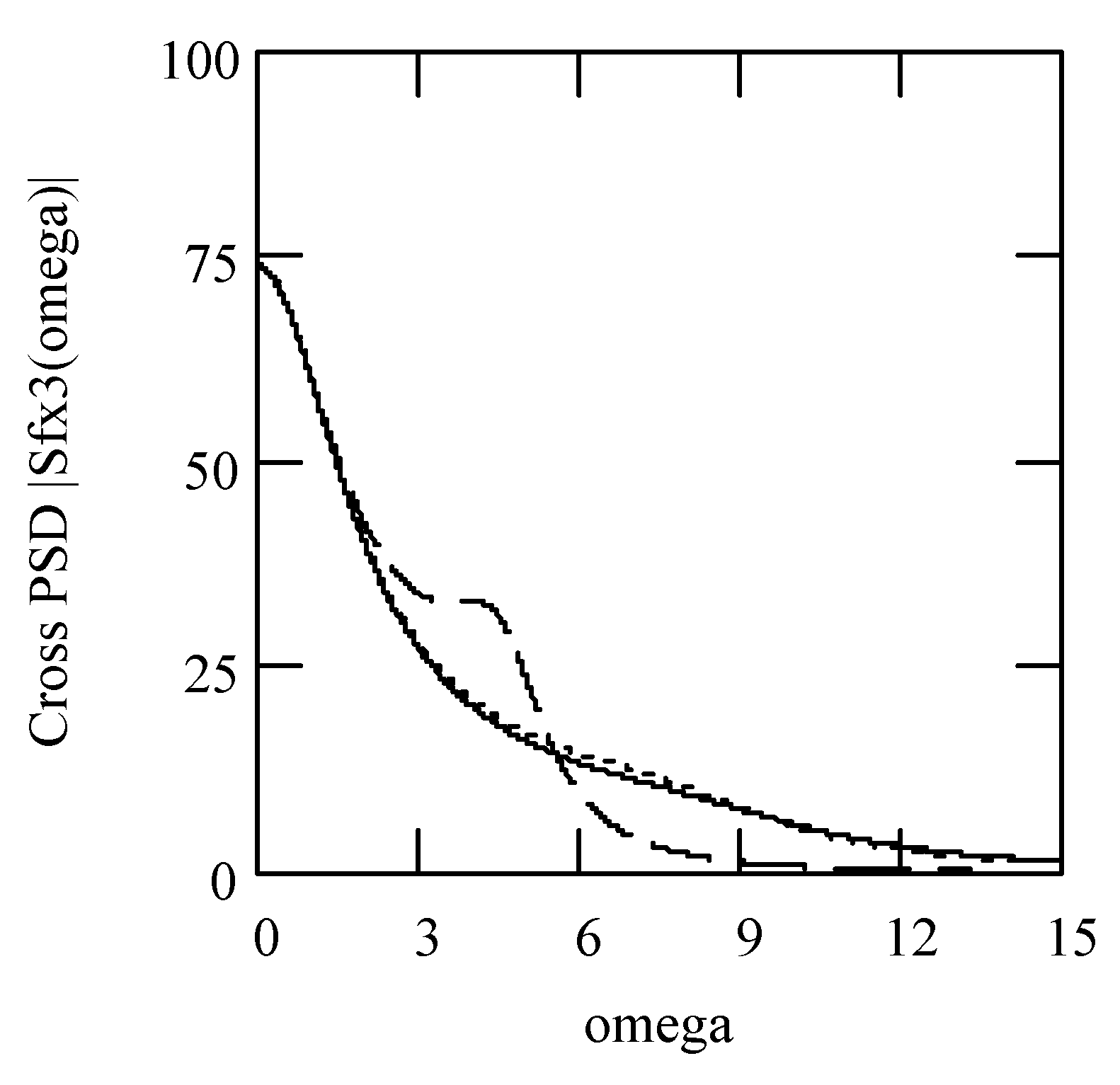
3.2.2. Effects of α on Responses
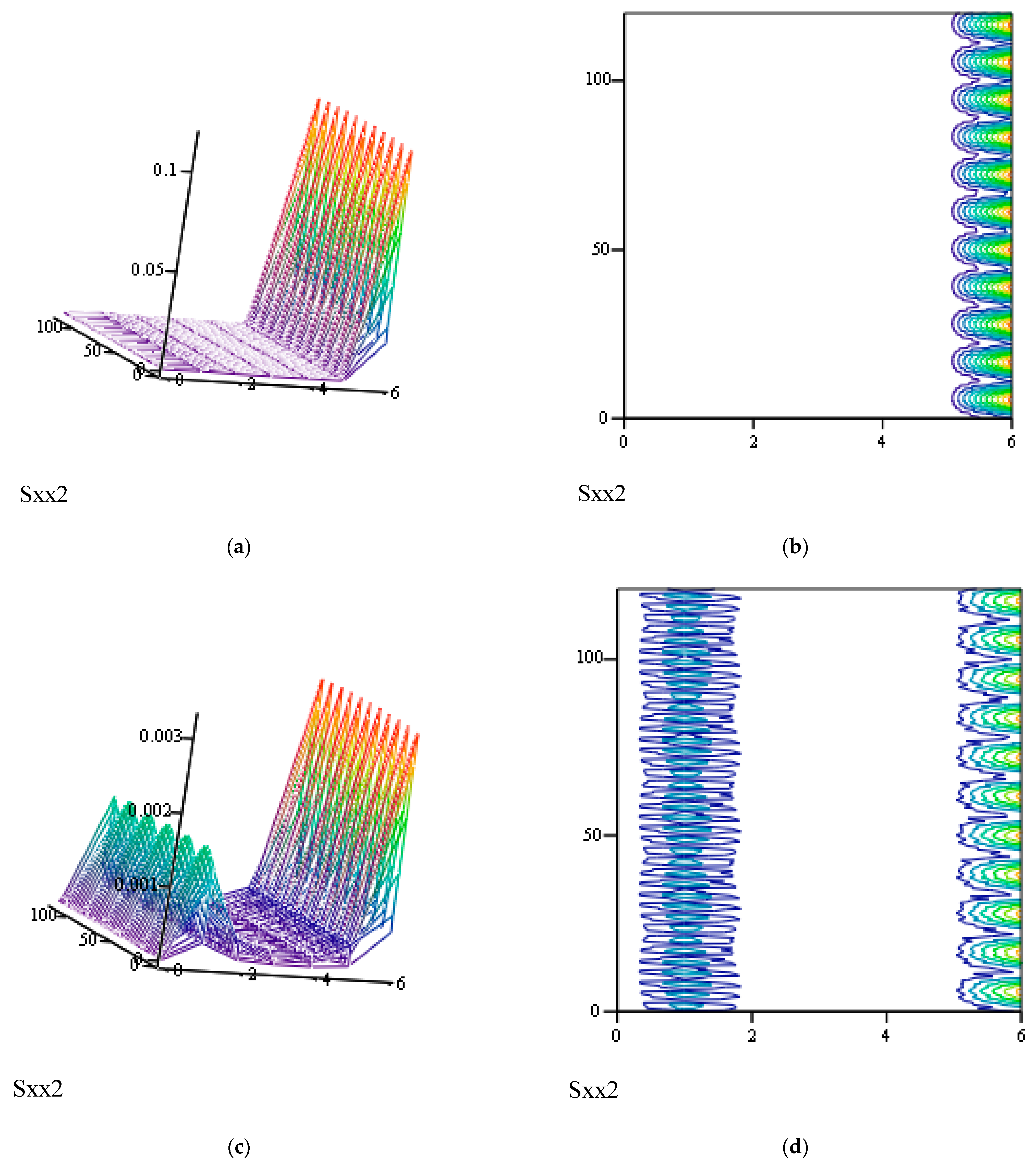
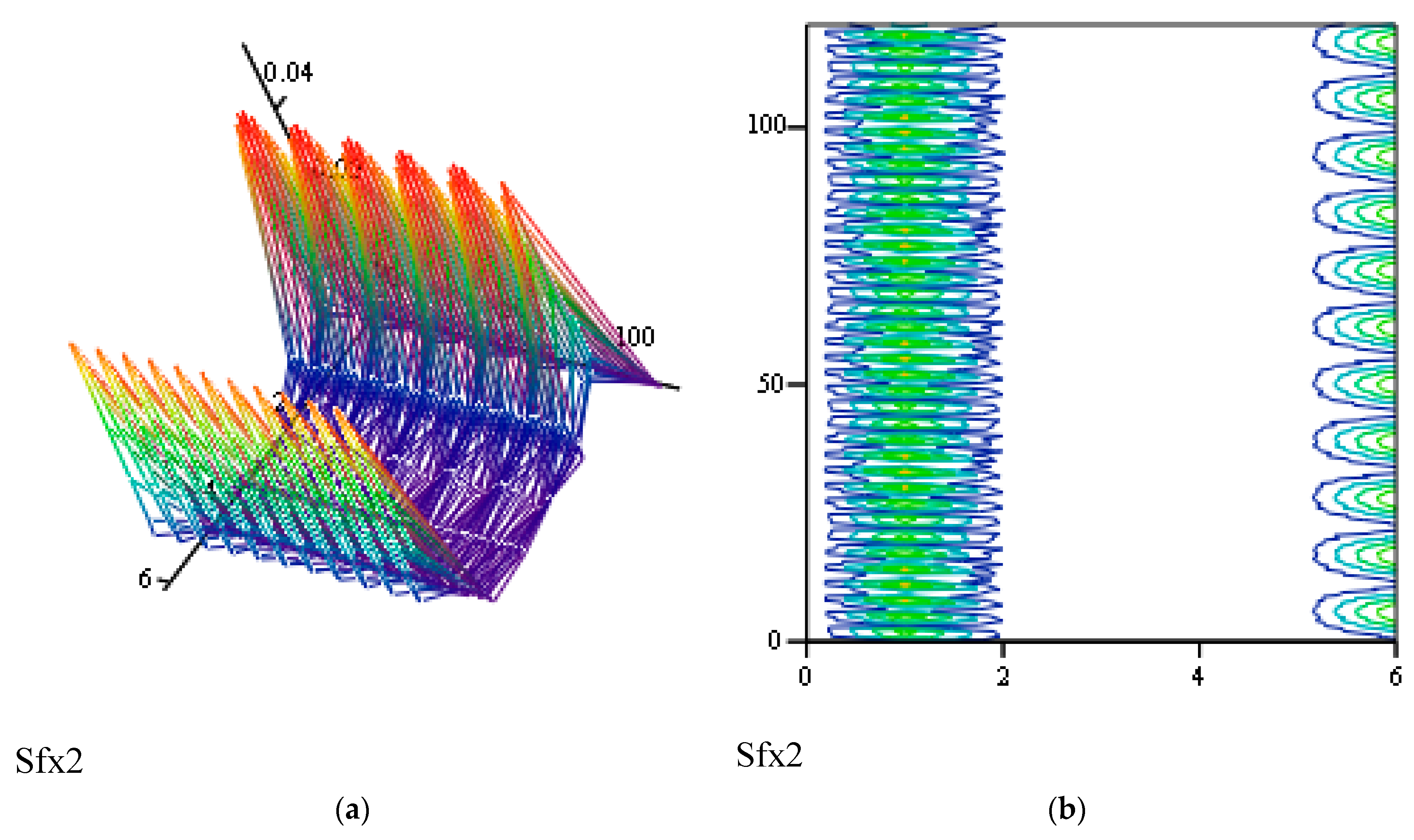
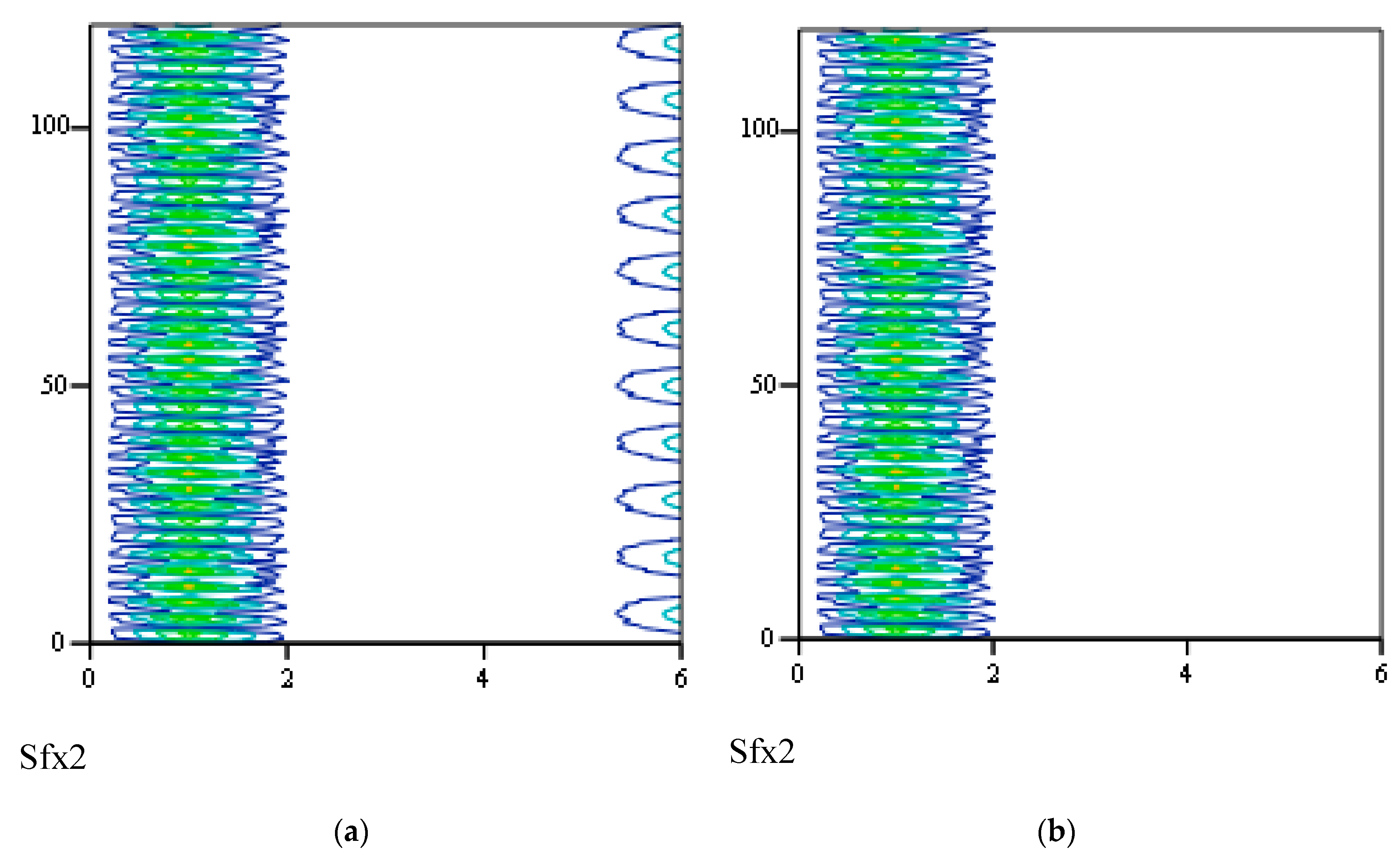
3.3. Responses of Class II Fractional Vibrators Driven by fGn
3.3.1. Computation Methods
3.3.2. Effects of β on Responses
3.4. Responses of Class III Fractional Vibrators Driven by fGn
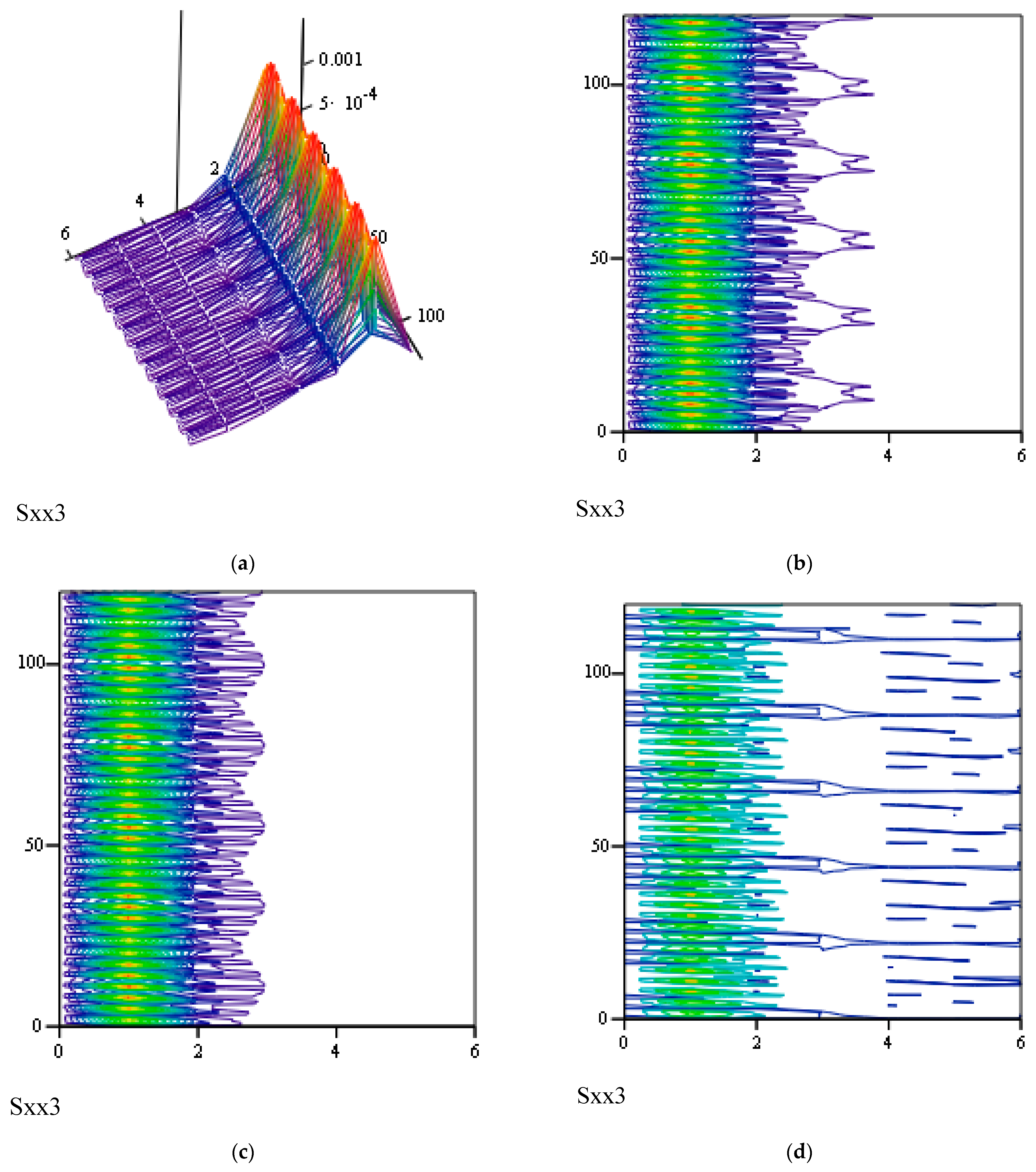
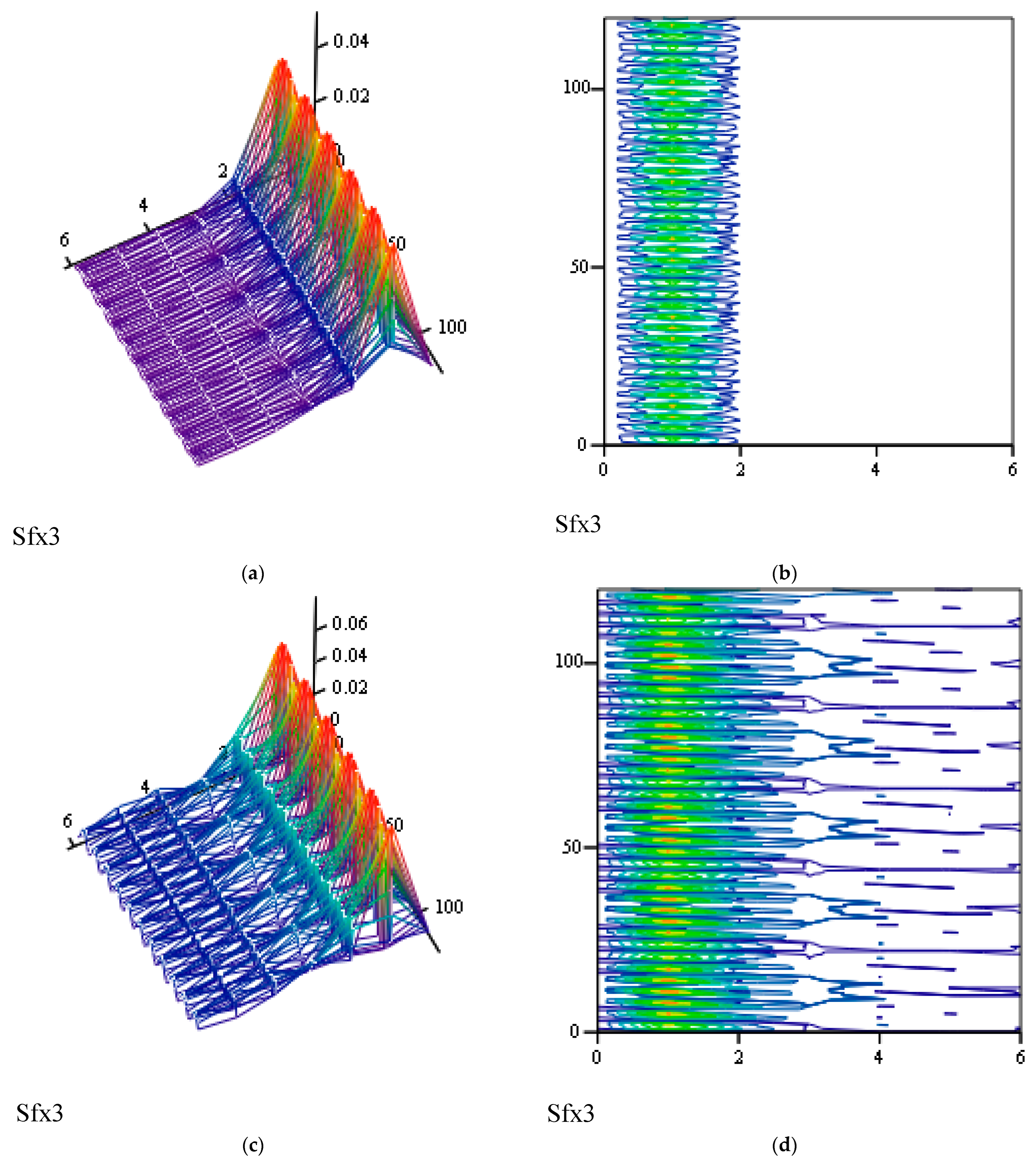
3.4.1. Computations
3.4.2. Effects of α and β on Responses
3.5. Responses of Class IV Fractional Vibrators Driven by fGn
3.5.1. Computations
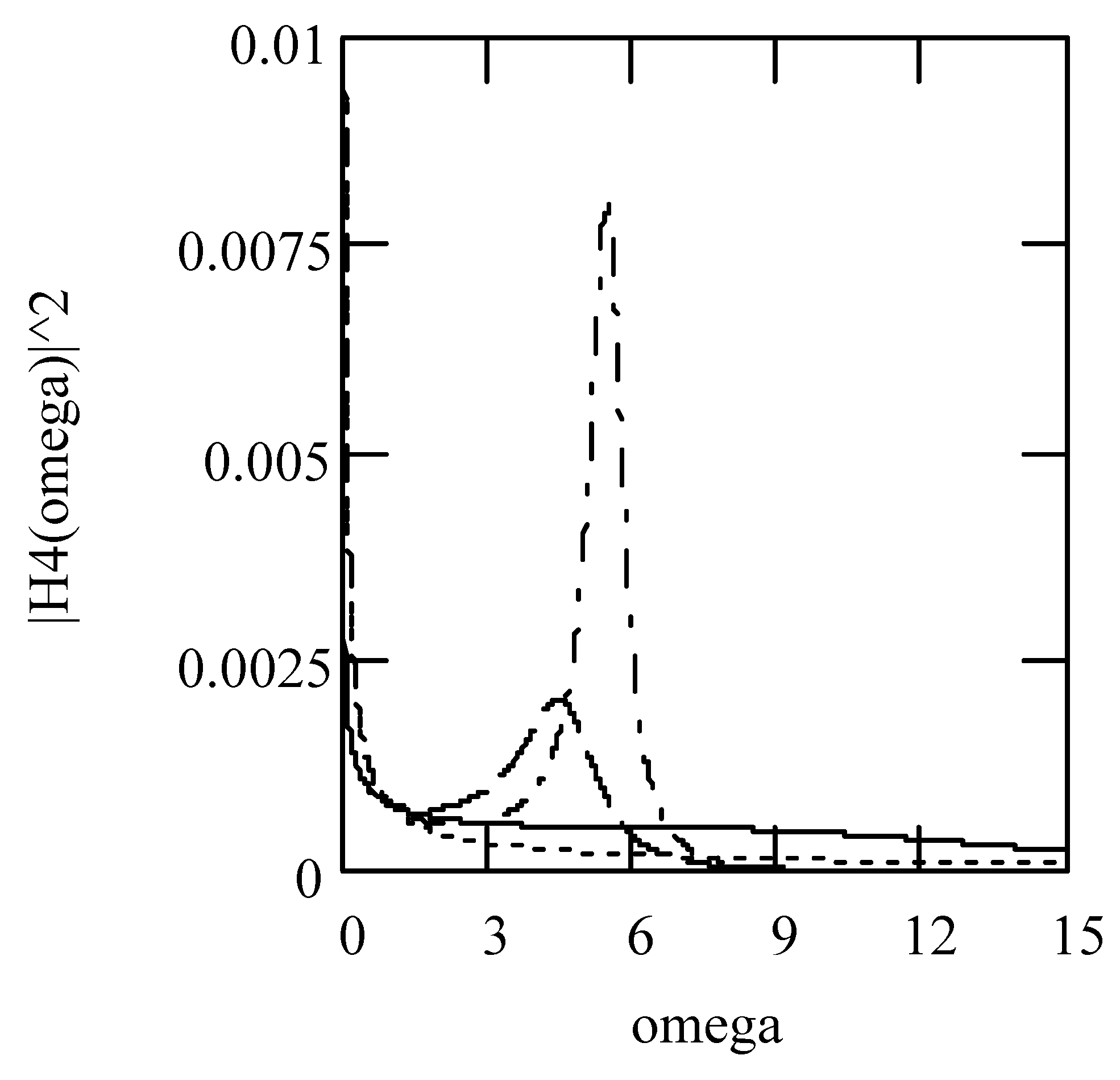
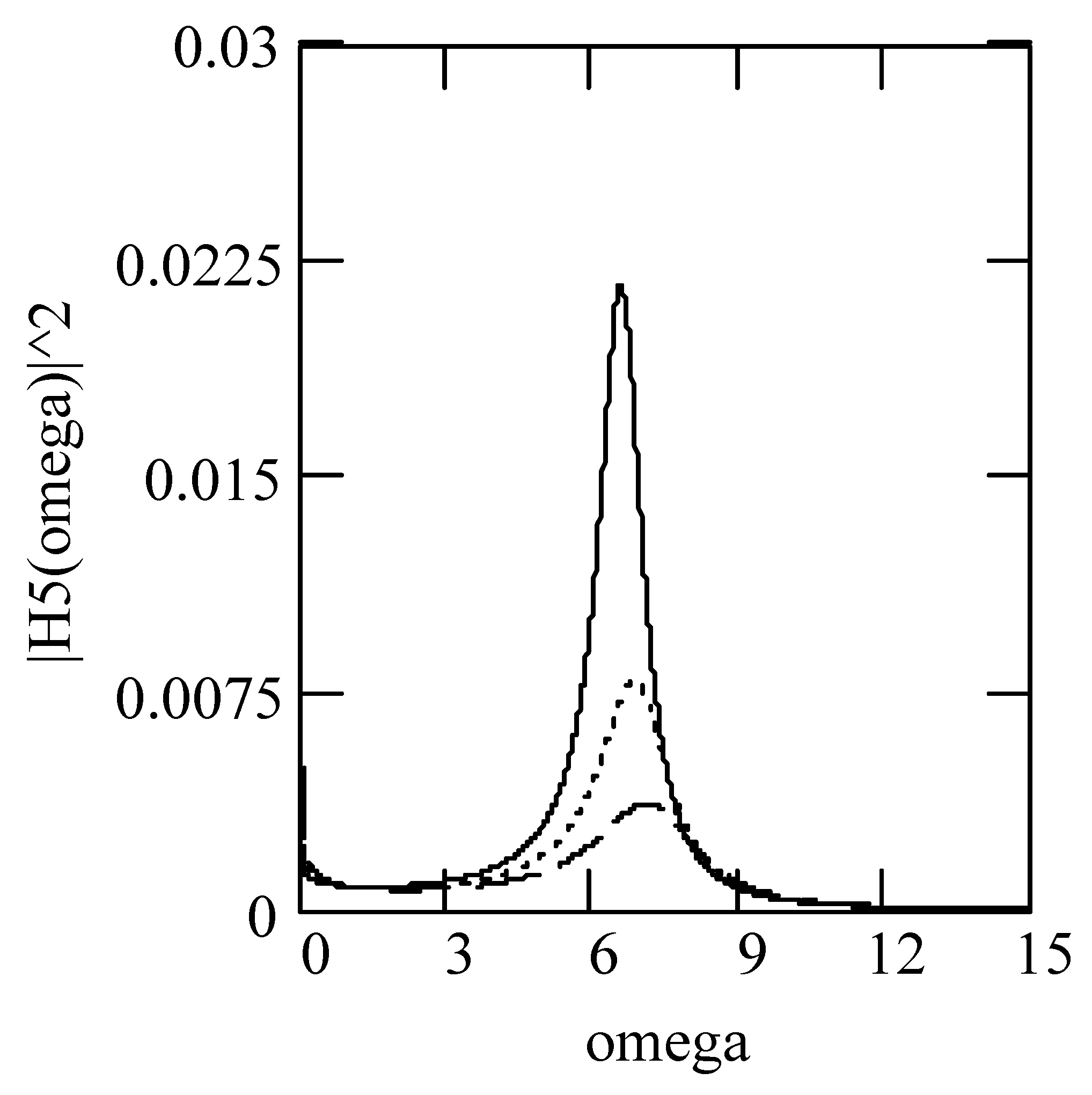
3.5.2. Effects of α and λ on Responses
3.6. Responses of Class V Fractional Vibrators Driven by fGn
3.6.1. Computation Methods
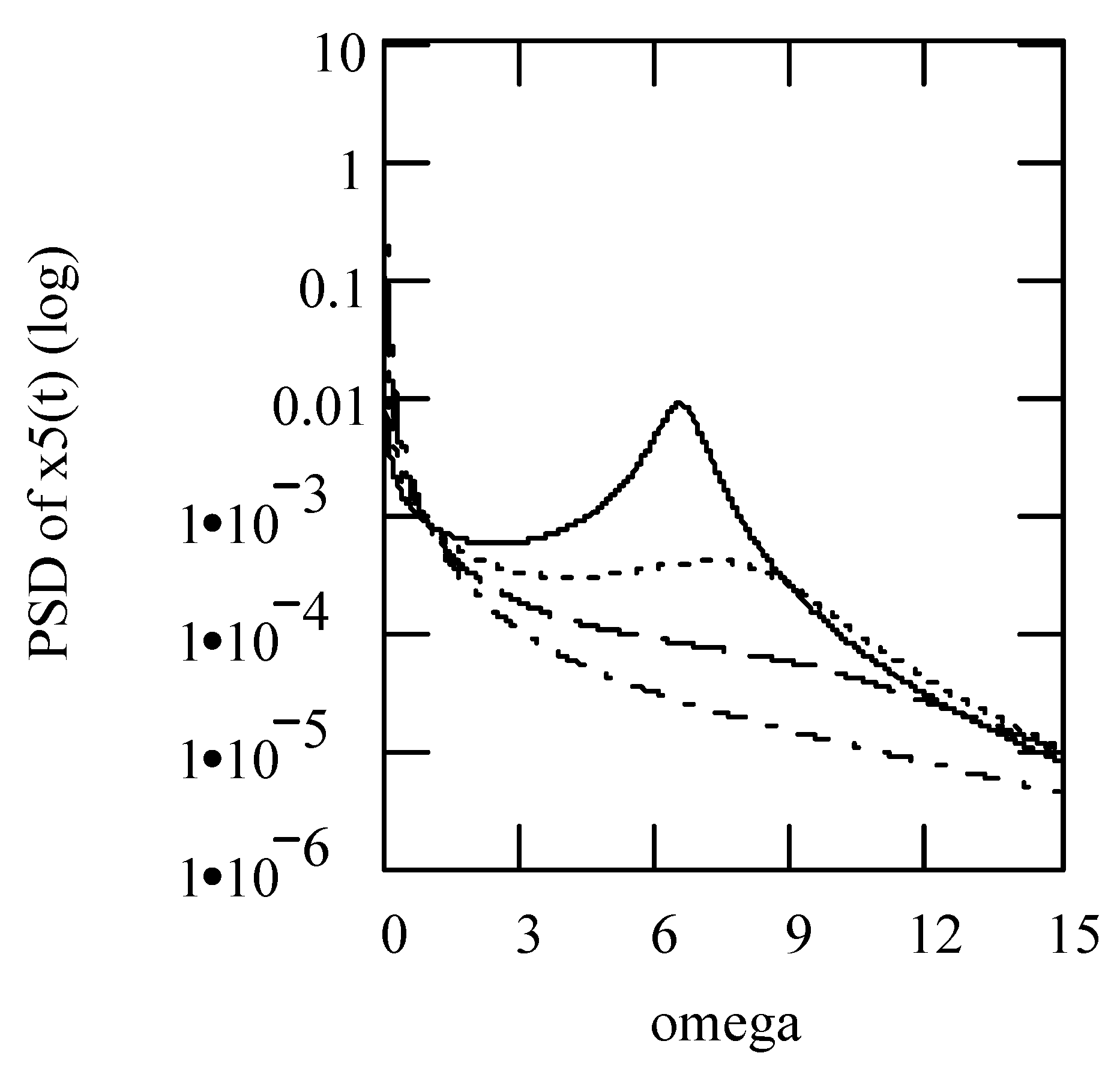
3.6.2. Effects of λ on Responses
3.7. Responses of Class VI Fractional Vibrators Driven by fGn
3.7.1. Computations
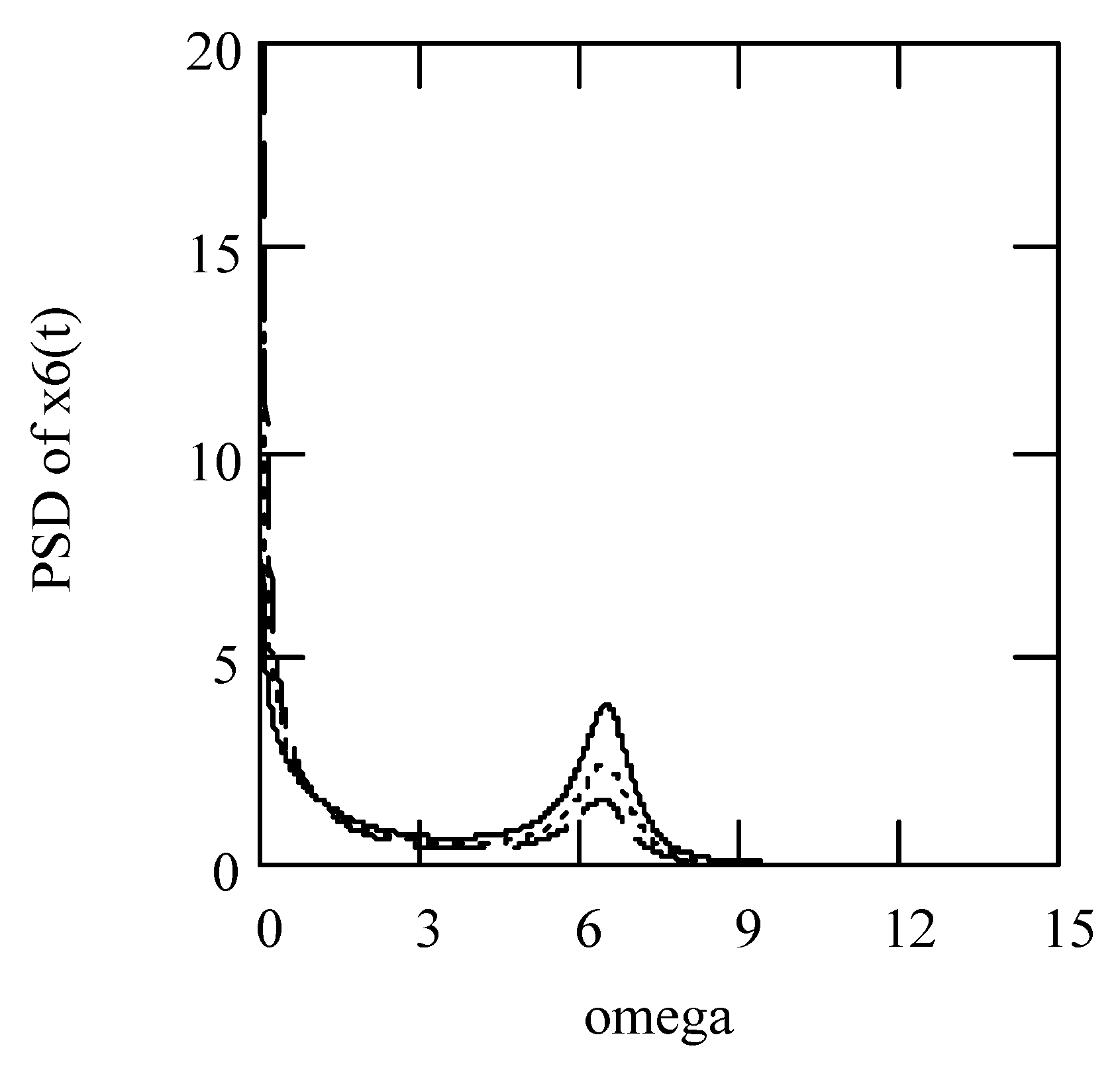
3.7.2. Effects of α, β, λ on Responses
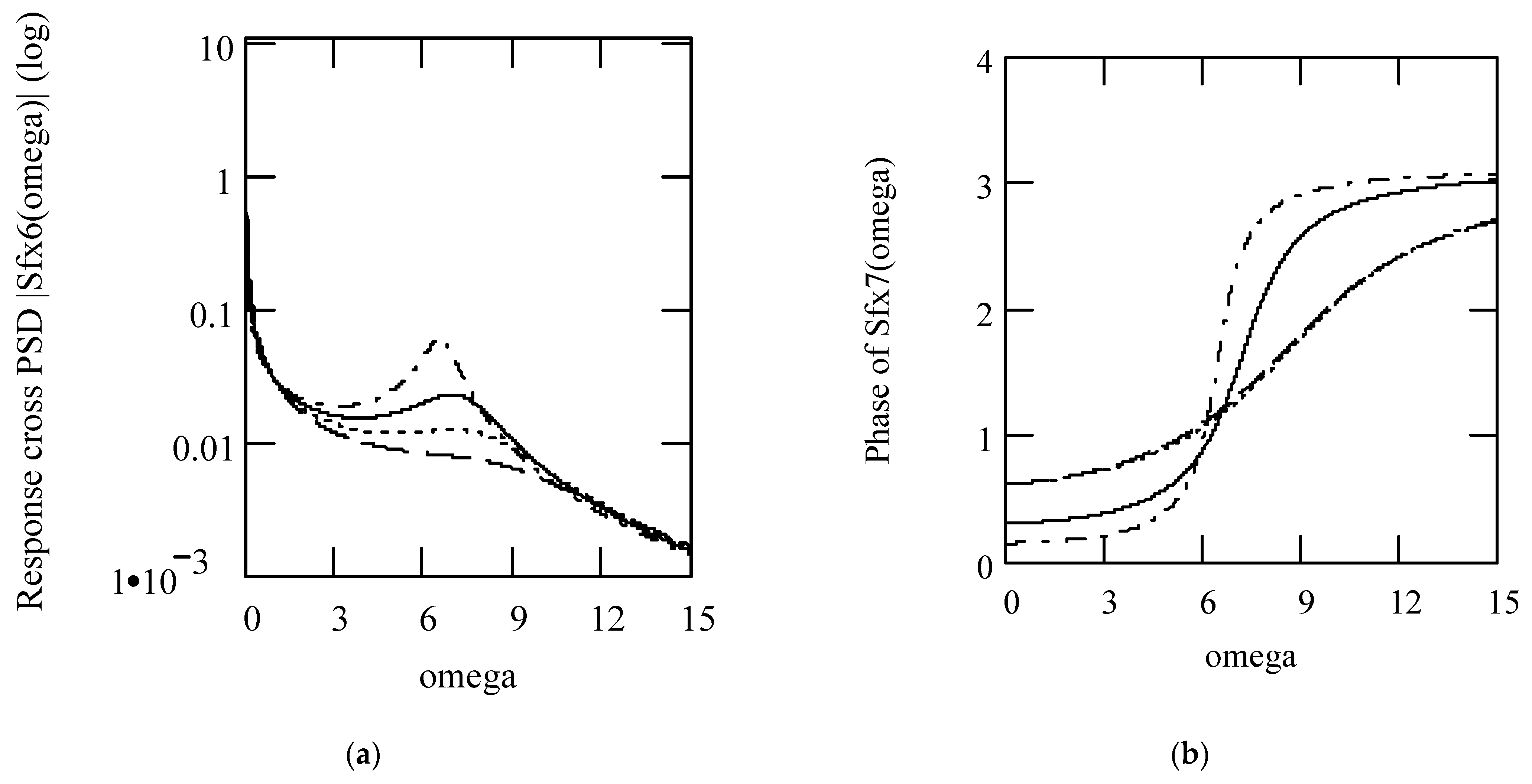
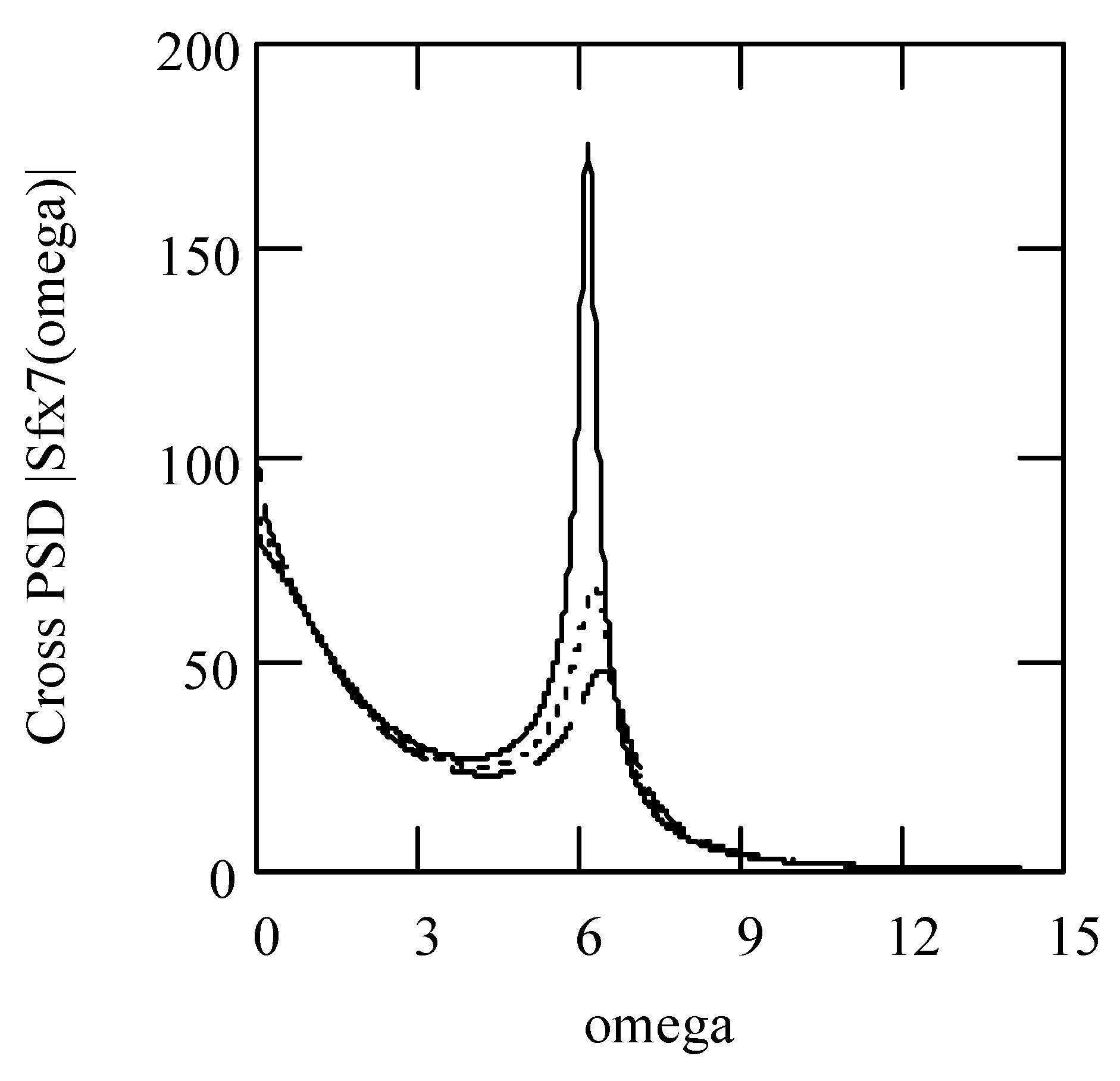
3.8. Responses of Class VII Fractional Vibrators Driven by fGn
3.8.1. Computations
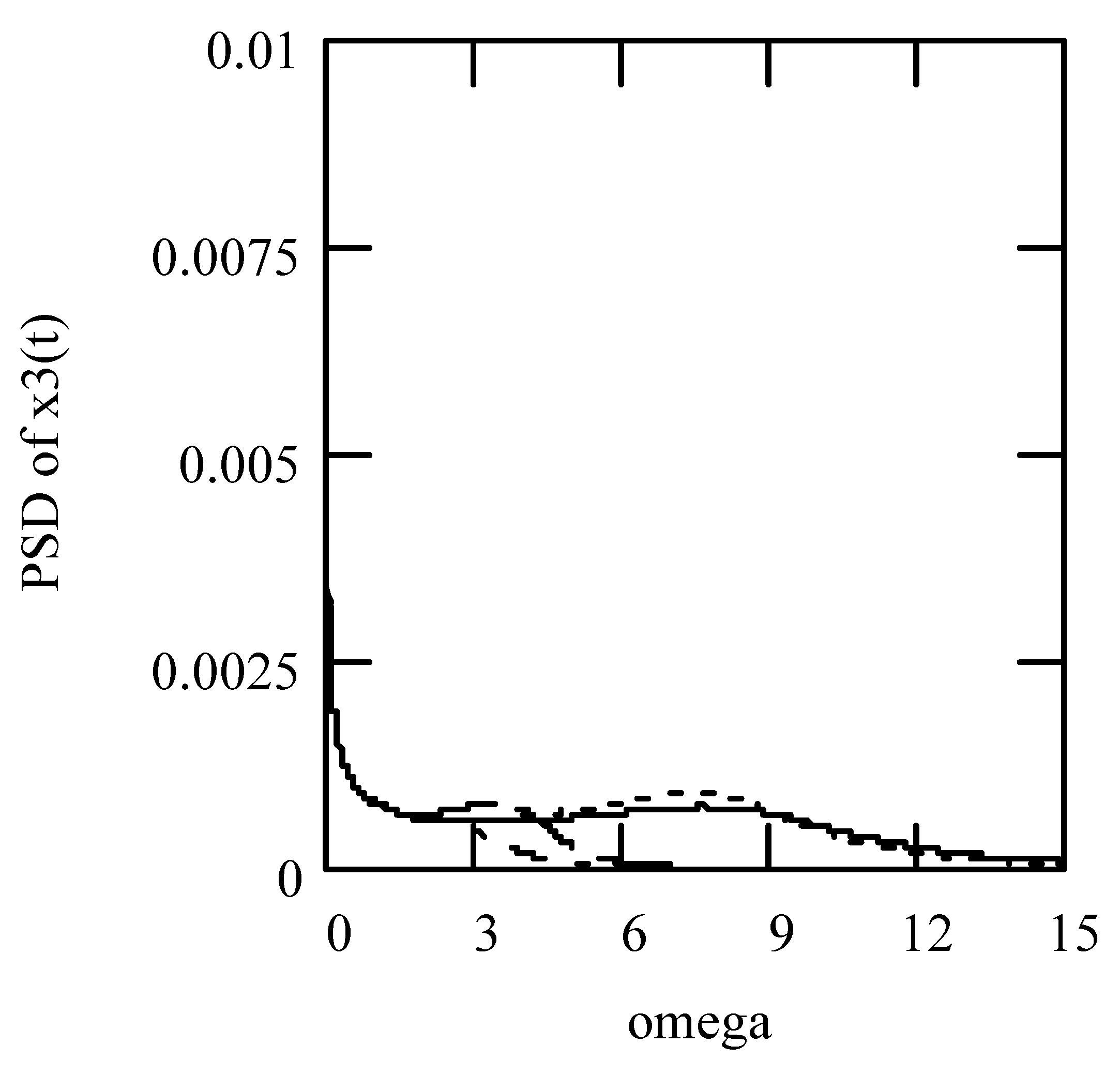
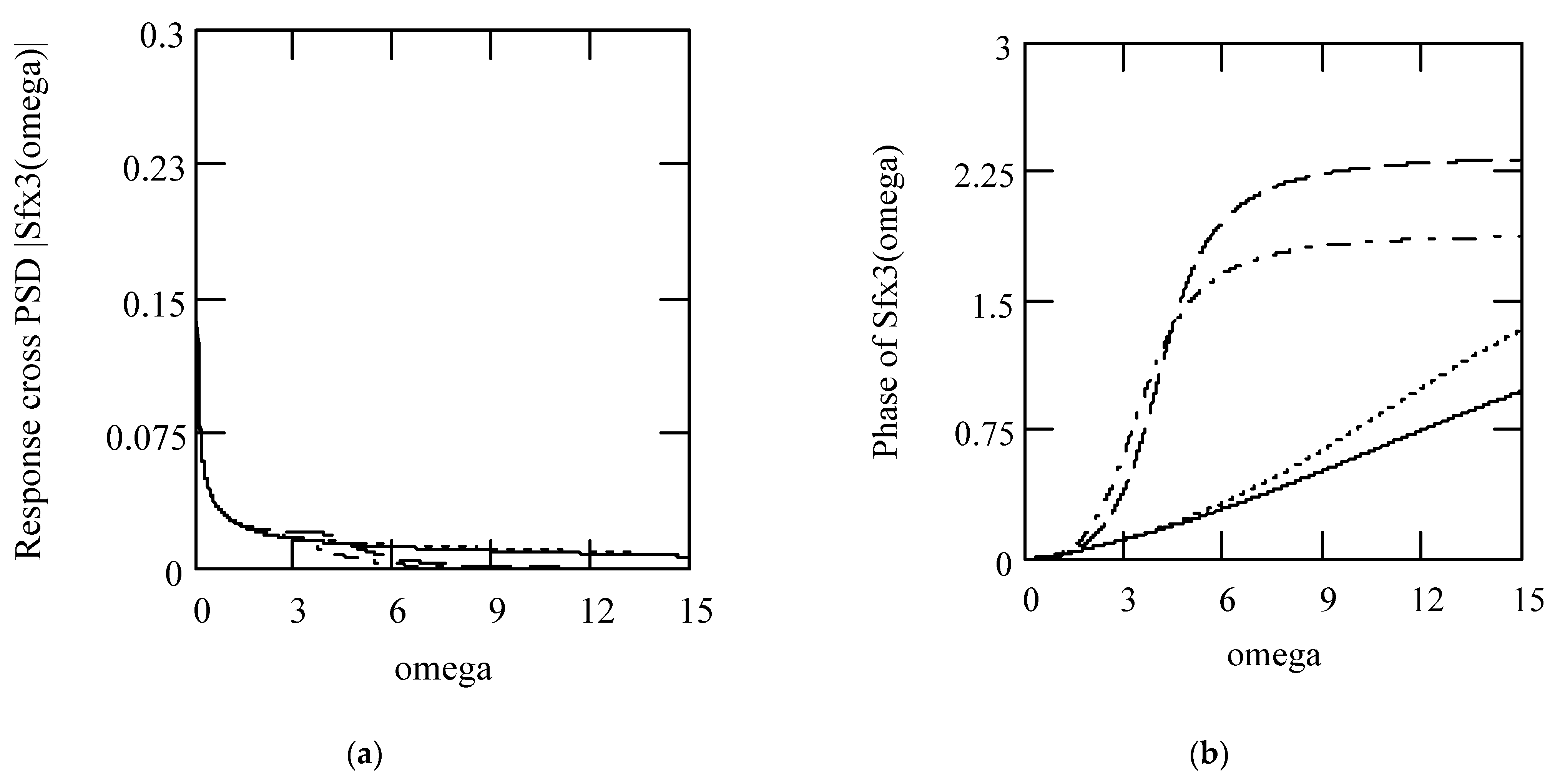
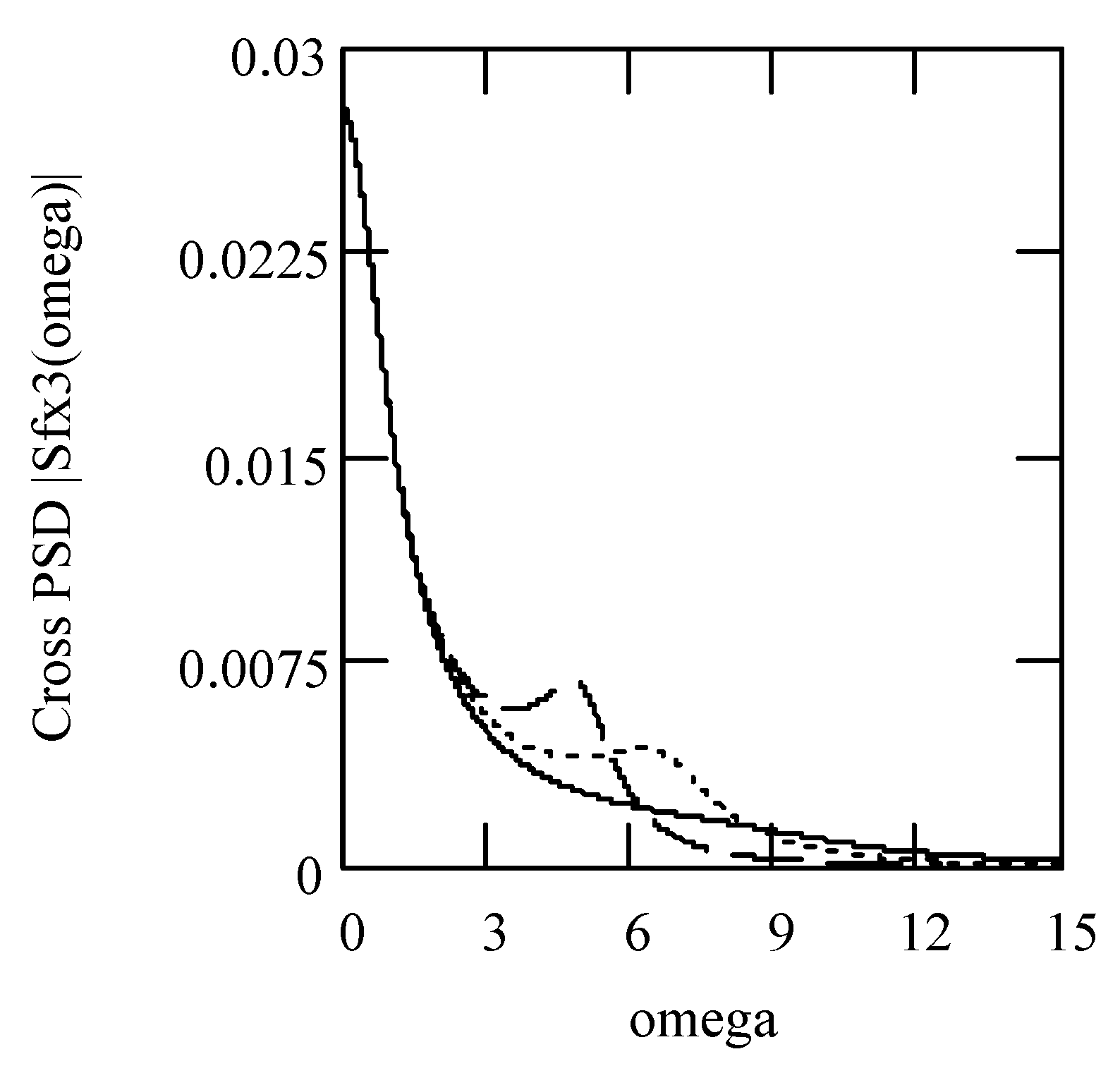
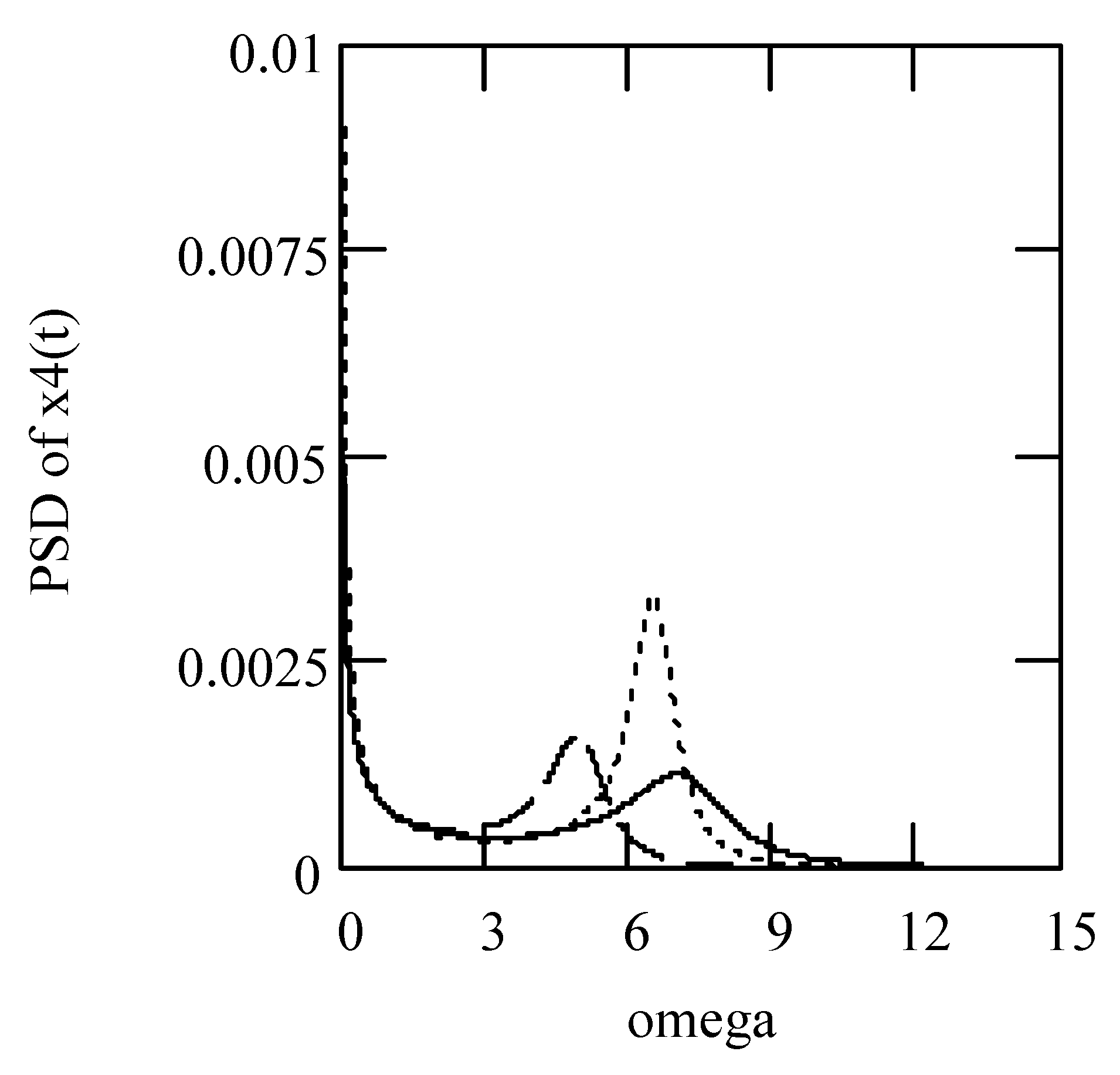
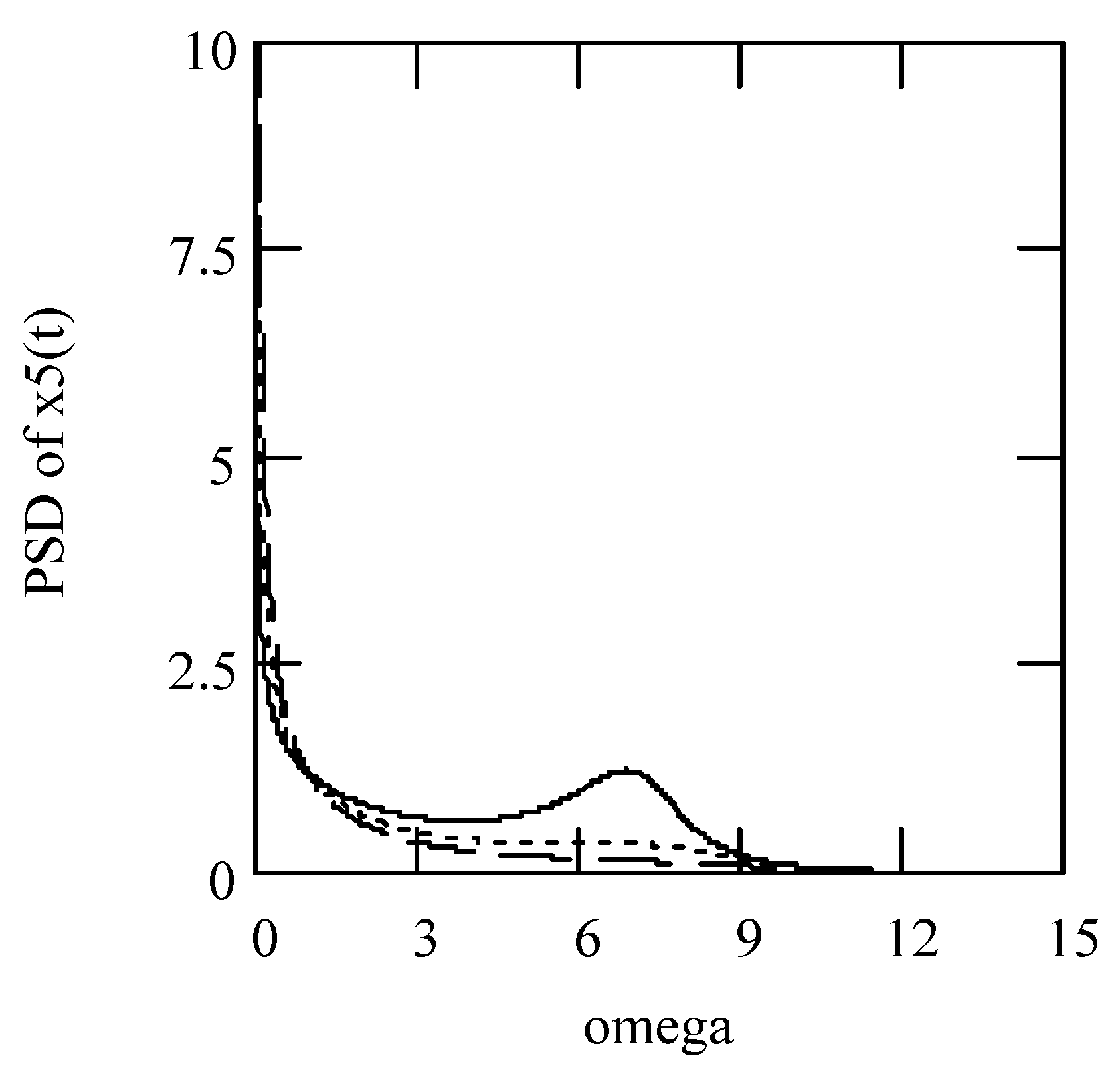
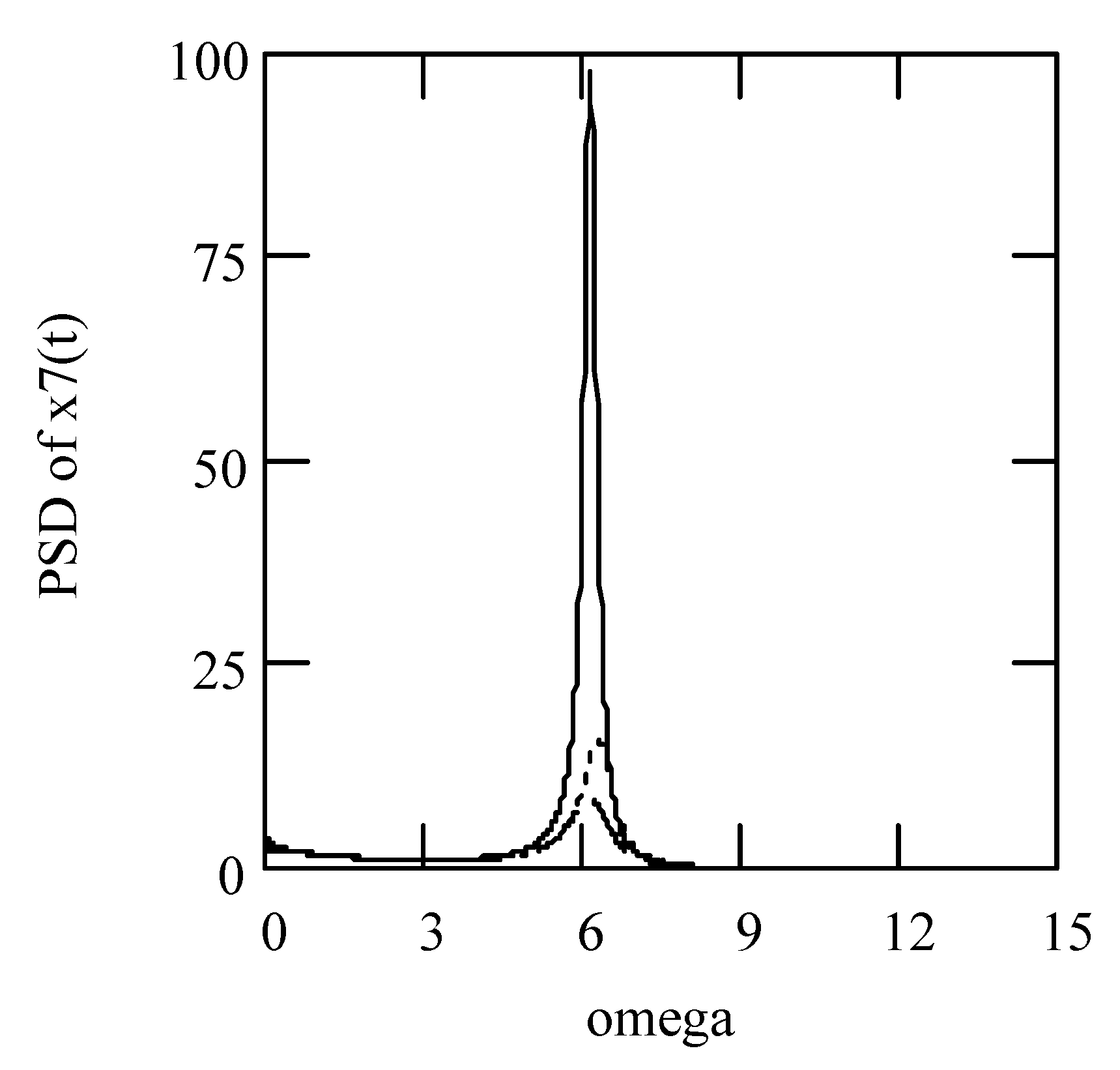
3.8.2. Effects of β, λ on Responses
3.9. Summary
4. PSD and Cross-PSD Responses to Seven Classes of Fractional Vibrations Driven by fBm
4.1. Background
4.2. Responses of Class I Fractional Vibrators Driven by fBm
4.2.1. Computations
4.2.2. Effects of α on Responses
4.3. Responses of Class II Fractional Vibrators Driven by fBm
4.3.1. Computations
4.3.2. Effects of β on Responses
4.4. Responses of Class III Fractional Vibrators Driven by fBm
4.4.1. Computations
4.4.2. Effects of α and β on Responses
4.5. Responses of Class IV Fractional Vibrators Driven by fBm
4.5.1. Computations
4.5.2. Effects of α and λ on Responses
4.6. Responses of Class V Fractional Vibrators Driven by fBm
4.6.1. Computation Methods
4.6.2. Effects of λ on Responses
4.7. Responses of Class VI Fractional Vibrators Driven by fBm
4.7.1. Computations
4.7.2. Effects of α, β, λ on Responses
4.8. Responses of Class VII Fractional Vibrators Driven by fBm
4.8.1. Computations
4.8.2. Effects of β, λ on Responses
4.9. Summary
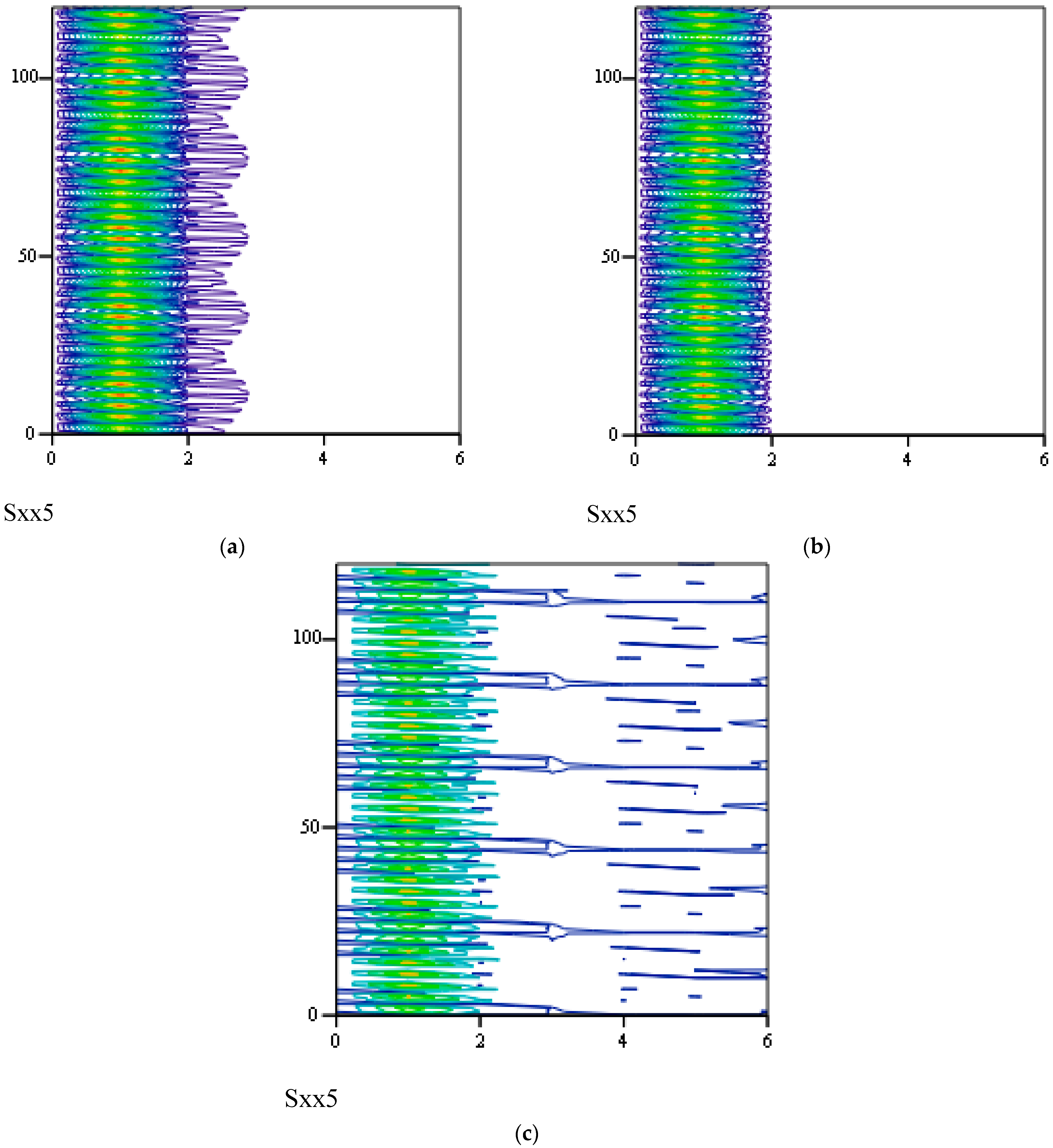
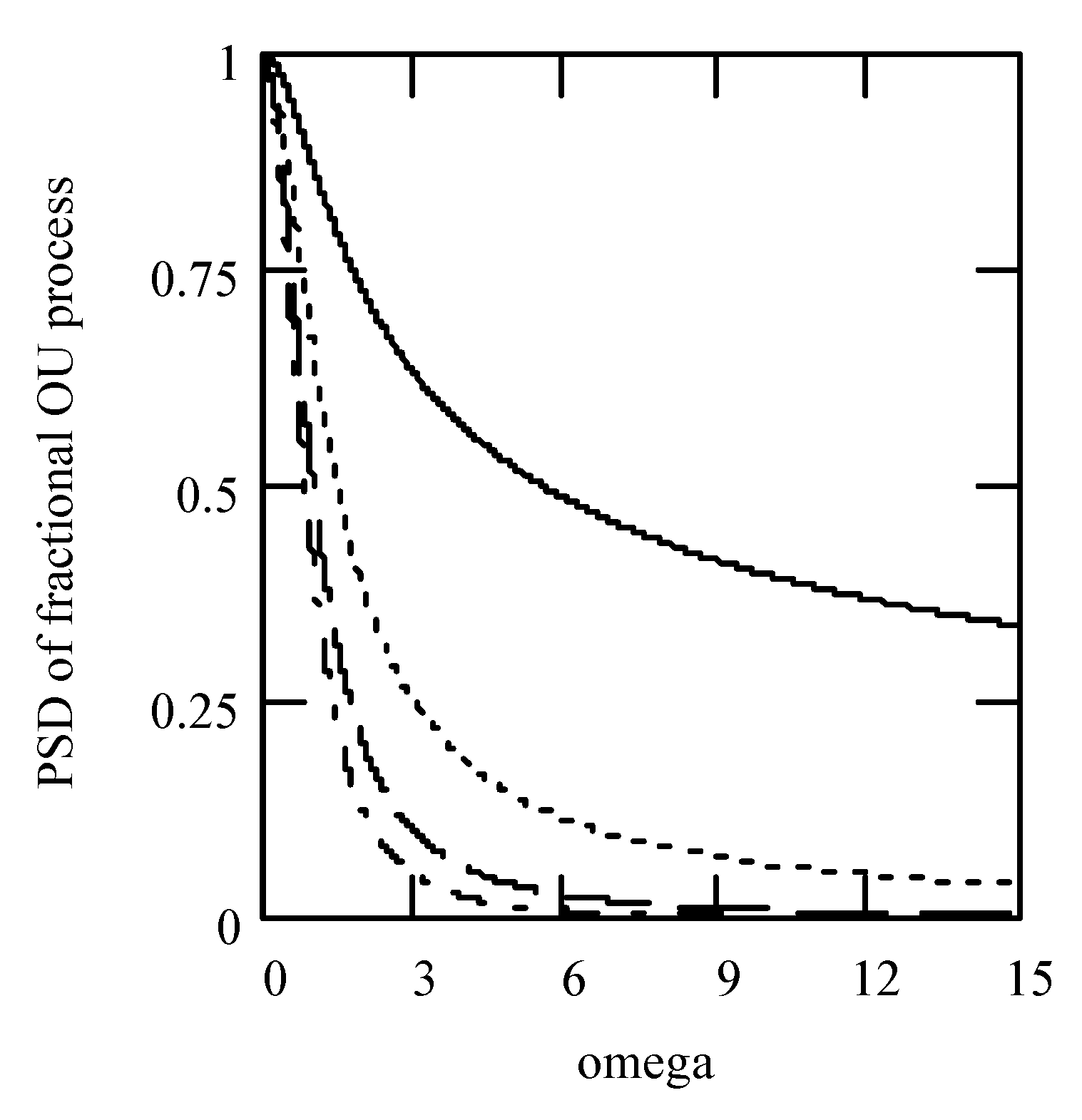
5. PSD and Cross-PSD Responses to Seven Classes of Fractional Vibrations Driven by Fractional OU Processes
5.1. Background
5.2. Responses of Class I Fractional Vibrators Driven by Fractional OU Processes
5.2.1. Computations
5.2.2. Effects of α on Responses
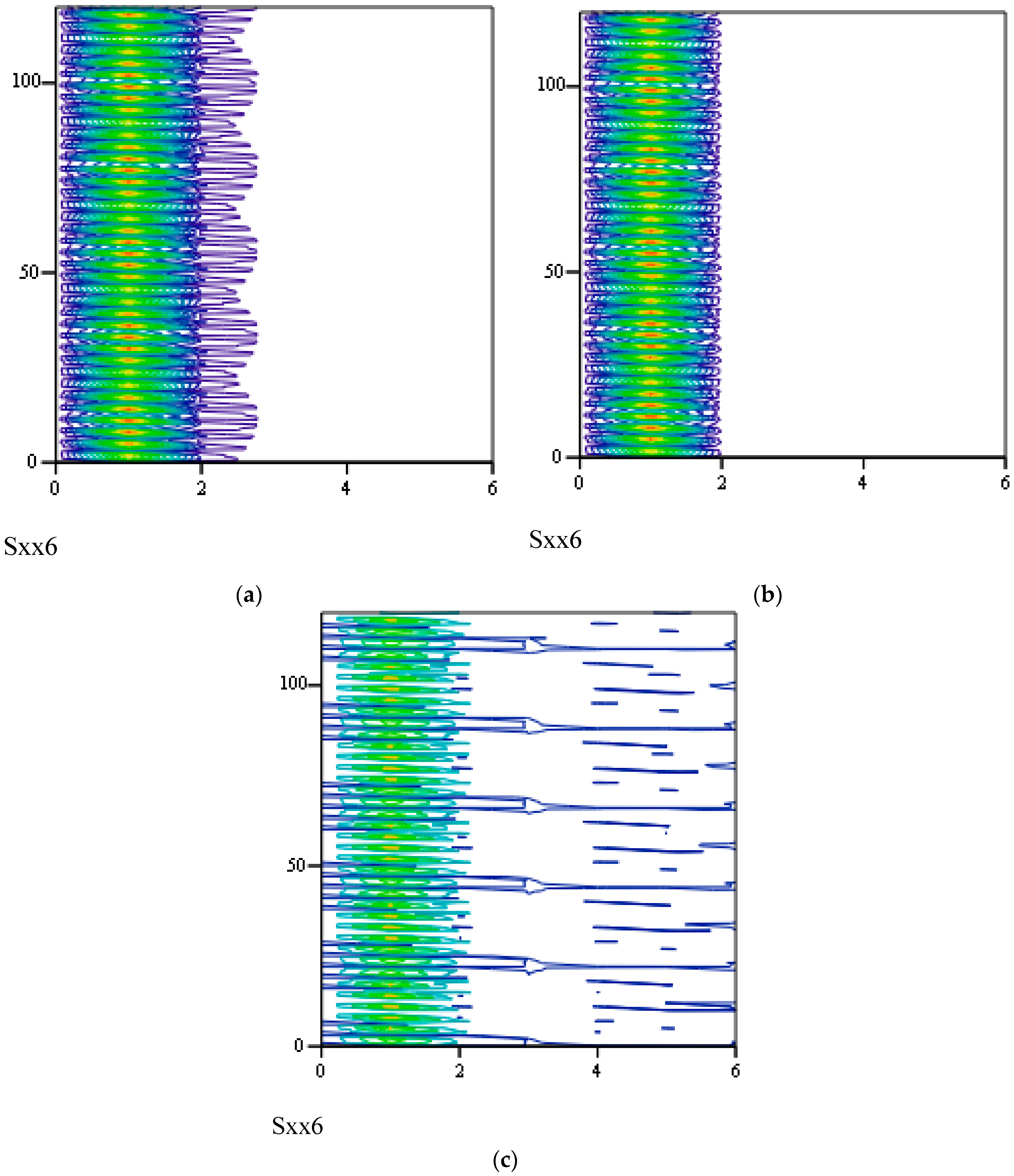
5.3. Responses of Class II Fractional Vibration Systems Driven by Fractional OU Processes
5.3.1. Computation Methods
5.3.2. Effects of β on Responses
5.4. Responses of Class III Fractional Vibrators Driven by Fractional OU Processes
5.4.1. Computations
5.4.2. Effects of α and β on Responses
5.5. Responses of Class IV Fractional Vibration Systems Driven by Fractional OU Processes
5.5.1. Computations
5.5.2. Effects of α and λ on Responses
5.6. Responses of Class V Fractional Vibrators Driven by Fractional OU Processes
5.6.1. Computation Methods
5.6.2. Effects of λ on Responses
5.7. Responses of Class VI Fractional Vibrators Driven by Fractional OU Processes
5.7.1. Computations
5.7.2. Effects of α, β, λ on Responses
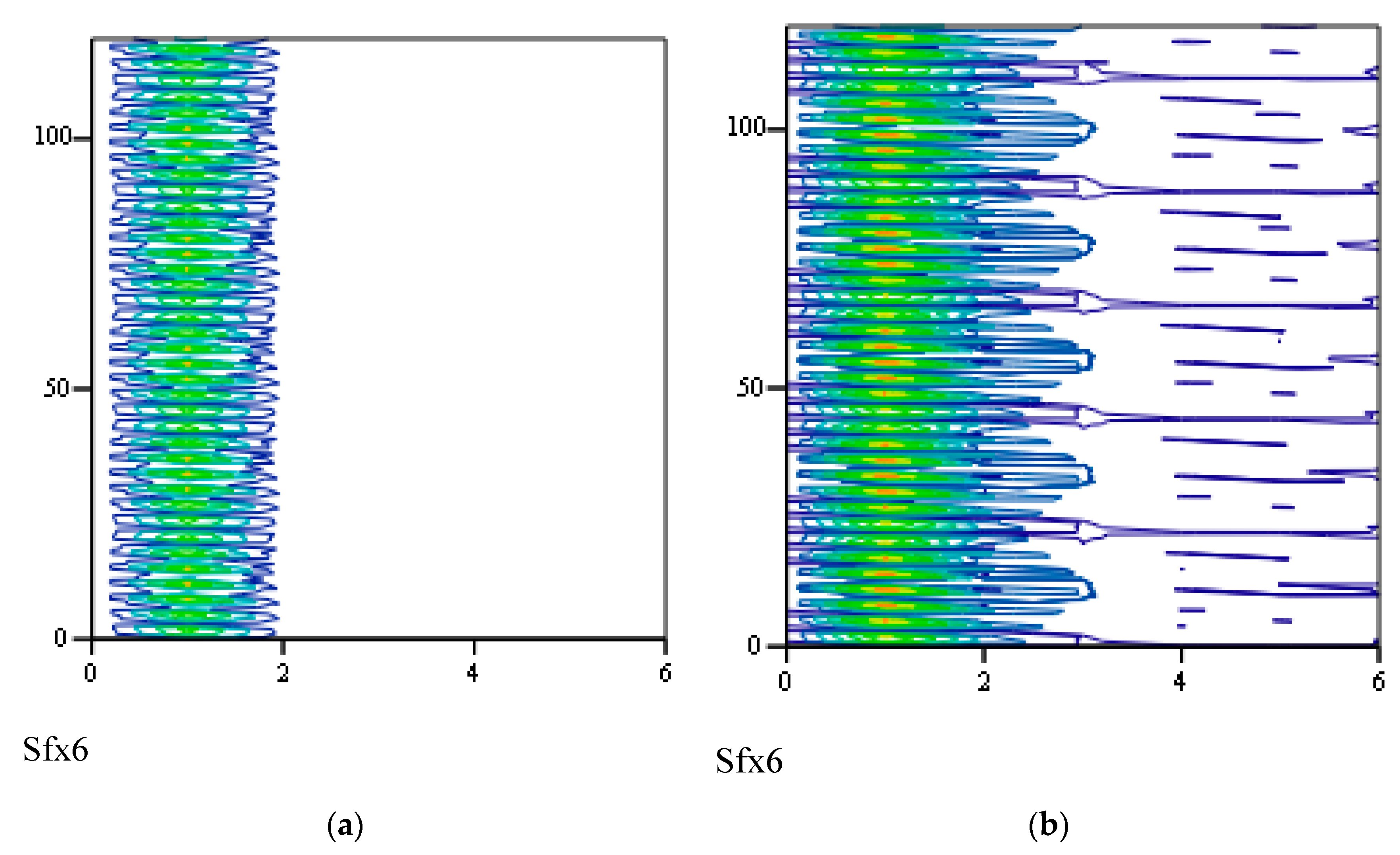
5.8. Responses of Class VII Fractional Vibrators Driven by Fractional OU Processes
5.8.1. Computations
5.8.2. Effects of β, λ on Responses
5.9. Summary
6. PSD and Cross-PSD Responses to Seven Classes of Fractional Vibrations Driven by the von Kármán Process
6.1. Background
6.2. Responses of Class I Fractional Vibrators Driven by von Kármán Process
6.2.1. Computations
6.2.2. Effects of α on Responses
6.3. Responses of Class II Fractional Vibration Systems Driven by von Kármán Process
6.3.1. Computation Methods
6.3.2. Effects of β on Responses
6.4. Responses of Class III Fractional Vibrators Driven by von Kármán Process
6.4.1. Computations
6.4.2. Effects of α and β on Responses
6.5. Responses of Class IV Fractional Vibration Systems Driven by the von Kármán Process
6.5.1. Computations
6.5.2. Effects of α and λ on Responses
6.6. Responses of Class V Fractional Vibrators Driven by von Kármán Process
6.6.1. Computation Methods
6.6.2. Effects of λ on Responses
6.7. Responses of Class VI Fractional Vibrators Driven by von Kármán Process
6.7.1. Computations
6.7.2. Effects of α, β, λ on Responses
6.8. Responses of Class VII Fractional Vibrators Driven by the von Kármán Process
6.8.1. Computations
6.8.2. Effects of β, λ on Responses
6.9. Summary
7. Discussions and Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Springer: Berlin, Germany, 2013; Volume II. [Google Scholar]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Damping characteristics of a fractional oscillator. Physical A 2004, 339, 311–319. [Google Scholar] [CrossRef]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Response characteristics of a fractional oscillator. Physical A 2002, 309, 275–288. [Google Scholar] [CrossRef]
- Achar, B.N.N.; Hanneken, J.W.; Enck, T.; Clarke, T. Dynamics of the fractional oscillator. Physical A 2001, 297, 361–367. [Google Scholar] [CrossRef]
- Duan, J.-S. The periodic solution of fractional oscillation equation with periodic input. Adv. Math. Phys. 2013, 2013, 869484. [Google Scholar] [CrossRef]
- Coccolo, M.; Seoane, J.M.; Sanjuán, M.A.F. Fractional damping induces resonant behavior in the Duffing oscillator. Commun. Nonlinear Sci. Numer. Simul. 2024, 133, 107965. [Google Scholar] [CrossRef]
- Sidorov, V.; Shitikova, M.V.; Badina, E.; Detina, E.A. Review of nonlocal-in-time damping models in the dynamics of structures. Axioms 2023, 12, 676. [Google Scholar] [CrossRef]
- Di Paola, M.; Heuer, R.; Pirrotta, A. Fractional visco-elastic Euler–Bernoulli beam. Int. J. Solids Struct. 2013, 50, 3505–3510. [Google Scholar] [CrossRef]
- Pirrotta, A.; Cutrona, S.; Di Lorenzo, S.; Di Matteo, A. Fractional visco-elastic Timoshenko beam deflection via single equation. Int. J. Numer. Methods Eng. 2015, 104, 869–886. [Google Scholar] [CrossRef]
- Lewandowski, R.; Wielentejczyk, P. Nonlinear vibration of viscoelastic beams described using fractional order derivatives. J. Sound Vib. 2017, 399, 228–243. [Google Scholar] [CrossRef]
- Spanos, P.D.; Malara, G. Nonlinear vibrations of beams and plates with fractional derivative elements subject to combined harmonic and random excitations. Probab. Eng. Mech. 2020, 59, 103043. [Google Scholar] [CrossRef]
- Blaszczyk, T. A numerical solution of a fractional oscillator equation in a non-resisting medium with natural boundary conditions. Rom. Rep. Phys. 2015, 67, 350–358. [Google Scholar]
- Blaszczyk, T.; Ciesielski, M. Fractional oscillator equation—Transformation into integral equation and numerical solution. Appl. Math. Comput. 2015, 257, 428–435. [Google Scholar] [CrossRef]
- Blaszczyk, T.; Ciesielski, M.; Klimek, M.; Leszczynski, J. Numerical solution of fractional oscillator equation. Appl. Math. Comput. 2011, 218, 2480–2488. [Google Scholar] [CrossRef]
- Blaszczyk, T.; Siedlecki, J.; Sun, H.G. An exact solution of fractional Euler-Bernoulli equation for a beam with fixed-supported and fixed-free ends. Appl. Math. Comput. 2021, 396, 125932. [Google Scholar] [CrossRef]
- Al-Rabtah, A.; Ertürk, V.S.; Momani, S. Solutions of a fractional oscillator by using differential transform method. Comput. Math. Appl. 2010, 59, 1356–1362. [Google Scholar] [CrossRef]
- Zurigat, M. Solving fractional oscillators using Laplace homotopy analysis method. Ann. Univ. Craiova Math. Comput. Sci. Ser. 2011, 38, 1–11. [Google Scholar]
- Rossikhin, Y.A.; Shitikova, M.V. Analysis of the viscoelastic rod dynamics via models involving fractional derivatives or operators of two different orders. Shock Vib. Dig. 2004, 36, 3–26. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Application of fractional calculus for dynamic problems of solid mechanics: Novel trends and recent results. Appl. Mech. Rev. 2010, 63, 010801. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Application of fractional operators to the analysis of damped vibrations of viscoelastic single-mass systems. J. Sound Vib. 1997, 199, 567–586. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Classical beams and plates in a fractional derivative medium, Impact response. In Encyclopedia of Continuum Mechanics; Springer: Berlin, Germany, 2020; Volume 1, pp. 294–305. [Google Scholar]
- Rossikhin, Y.A. Reflections on two parallel ways in progress of fractional calculus in mechanics of solids. Appl. Mech. Rev. 2010, 63, 010701. [Google Scholar] [CrossRef]
- Shitikova, M.V.; Kandu, V.V.; Krusser, A.I. On nonlinear vibrations of an elastic plate on a fractional viscoelastic foundation in a viscoelastic medium in the presence of the one-to-one internal resonance. J. Sound Vib. 2023, 549, 117564. [Google Scholar] [CrossRef]
- Shitikova, M.V. Impact response of a thin shallow doubly curved linear viscoelastic shell rectangular in plan. Math. Mech. Solids 2022, 27, 1721–1739. [Google Scholar] [CrossRef]
- Shitikova, M.V. Fractional operator viscoelastic models in dynamic problems of mechanics of solids: A review. Mech. Solids 2022, 57, 1–33. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A.; Anukool, W. Vlasov equation, waves and dispersion relations in fractal dimensions: Landau damping and the toroidal ion temperature gradient instability problem. Waves Random Complex Media 2022, 1–21. [Google Scholar] [CrossRef]
- Banerjee, J.R. An exact method for free vibration of beams and frameworks using frequency-dependent mass, elastic and geometric stiffness matrices. Comput. Struct. 2024, 292, 107235. [Google Scholar] [CrossRef]
- Sofi, A. Nonlinear vibrations of beams with fractional derivative elements crossed by moving loads. Int. J. Non-Linear Mech. vol. 2024, 159, 104567. [Google Scholar] [CrossRef]
- Sofi, A.; Muscolino, G. Improved pseudo-force approach for Monte Carlo Simulation of non-linear fractional oscillators under stochastic excitation. Probab. Eng. Mech. 2023, 71, 103403. [Google Scholar] [CrossRef]
- Molina-Villegas, J.C.; Ortega, J.E.B.; Martínez, G.M. Closed-form solution for non-uniform Euler—Bernoulli beams and frames. Eng. Struct. 2023, 292, 116381. [Google Scholar] [CrossRef]
- Parovik, R.I. Quality factor of forced oscillations of a linear fractional oscillator. Tech. Phys. 2020, 65, 1015–1019. [Google Scholar] [CrossRef]
- Li, M.; Lim, S.C.; Chen, S.Y. Exact solution of impulse response to a class of fractional oscillators and its stability. Math. Probl. Eng. 2011, 2011, 657839. [Google Scholar] [CrossRef]
- Li, M.; Lim, S.C.; Cattani, C.; Scalia, M. Characteristic roots of a class of fractional oscillators. Adv. High Energy Phys. 2013, 2013, 853925. [Google Scholar] [CrossRef]
- Li, M. Three classes of fractional oscillators. Symmetry 2018, 10, 91. [Google Scholar] [CrossRef]
- Li, M. Theory of Fractional Engineering Vibrations; De Gruyter: Berlin, Germany; Boston, MA, USA, 2021. [Google Scholar]
- Li, M. Fractional Vibrations with Applications to Euler-Bernoulli Beams; CRC Press: Boca Raton, UK, 2023. [Google Scholar]
- Mandelbrot, B.B. Gaussian Self-Affinity and Fractals; Springer: New York, NY, USA, 2001. [Google Scholar]
- Mandelbrot, B.B. Multifractals and 1/f Noise; Springer: New York, NY, USA, 1998. [Google Scholar]
- Beran, J. Statistics for Long-Memory Processes; Chapman & Hall: New York, NY, USA, 1994. [Google Scholar]
- Levy-Vehel, J.; Lutton, E. Fractals in Engineering; Springer: Berlin, Germany, 2005. [Google Scholar]
- Véhel, J.L.; Rams, M. Large deviation multifractal analysis of a class of additive processes with correlated non-stationary increments. IEEE/ACM Trans. Netw. 2013, 21, 1309–1321. [Google Scholar] [CrossRef]
- Bender, C.; Lebovits, J.; Véhel, J.L. General transfer formula for stochastic integral with respect to multifractional Brownian motion. J. Theor. Prob. 2024, 37, 905–932. [Google Scholar] [CrossRef]
- Guével, R.L.; Lévy-Véhel, J. Hausdorff, large deviation and Legendre multifractal spectra of Lévy multistable processes. Stoch. Process. Their Appl. 2020, 130, 2032–2057. [Google Scholar] [CrossRef]
- Lebovits, J.; Lévy-Véhel, J.; Herbin, E. Stochastic integration with respect to multifractional Brownian motion via tangent fractional Brownian motions. Stoch. Process. Their Appl. 2014, 124, 678–708. [Google Scholar] [CrossRef]
- Corlay, S.; Lebovits, J.; Lévy-Véhel, J. multifractional stochastic volatility models. Math. Financ. 2014, 24, 364–402. [Google Scholar] [CrossRef]
- Ayache, A.; Roueff, F.; Xiao, Y. Linear fractional stable sheets: Wavelet expansion and sample path properties. Stoch. Process. Their Appl. 2009, 119, 1168–1197. [Google Scholar] [CrossRef]
- Park, H.; Xiao, Y.; Yang, X. Uniform dimension results for the inverse images of symmetric Lévy processes. J. Theor. Probab. 2020, 33, 2213–2232. [Google Scholar] [CrossRef]
- Luks, T.; Xiao, Y. Multiple points of operator semistable Lévy processes. J. Theor. Probab. 2020, 33, 153–179. [Google Scholar] [CrossRef]
- Li, Y.; Xiao, Y. A class of fractional Brownian fields from branching systems and their regularity properties. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2013, 16, 1350023. [Google Scholar] [CrossRef]
- Flandrin, P. On the spectrum of fractional Brownian motions. IEEE Trans. Inf. Theory 1989, 35, 197–199. [Google Scholar] [CrossRef]
- Flandrin, P. Wavelet analysis and synthesis of fractional Brownian motion. IEEE Trans. Inf. Theory 1992, 38, 910–917. [Google Scholar] [CrossRef]
- Zao, L.; Coelho, R.; Flandrin, P. Speech Enhancement with EMD and Hurst-based mode selection. IEEE/ACM Trans. Audio Speech Lang. Process. 2014, 22, 897–909. [Google Scholar] [CrossRef]
- Borgnat, P.; Amblard, P.-O.; Flandrin, P. Scale Invariances and Lamperti Transformations for Stochastic Processes. J. Phys. A Math. Theor. 2005, 38, 2081–2101. [Google Scholar] [CrossRef]
- Borgnat, P.; Flandrin, P.; Amblard, P.-O. Stochastic discrete scale invariance. IEEE Signal Process. Lett. 2002, 9, 181–184. [Google Scholar] [CrossRef]
- Salcedo-Sanz, S.; Casillas-Pérez, D.; Del Ser, J.; Casanova-Mateo, C.; Cuadra, L.; Piles, M.; Camps-Valls, G. Persistence in complex systems. Phys. Rep. 2022, 957, 1–73. [Google Scholar] [CrossRef]
- Levin, Y.; Pakter, R.; Rizzato, F.B.; Teles, T.N.; Benetti, F.P.C. Nonequilibrium statistical mechanics of systems with long-range interactions. Phys. Rep. 2014, 535, 1–60. [Google Scholar] [CrossRef]
- Marković, D.; Gros, C. Power laws and Self-Organized Criticality in Theory and Nature. Phys. Rep. 2014, 536, 41–74. [Google Scholar] [CrossRef]
- Eliazar, I.I.; Shlesinger, M.F. Fractional motions. Phys. Rep. 2013, 527, 101–129. [Google Scholar] [CrossRef]
- Campa, A.; Dauxois, T.; Ruffo, S. Statistical mechanics and dynamics of solvable models with long-range interactions. Phys. Rep. 2009, 480, 57–159. [Google Scholar] [CrossRef]
- Pinchas, M.; Avraham, Y. Performance of the modified clock skew estimator and its upper bound for the IEEE 1588v2 (PTP) case under packet loss and fractional Gaussian noise environment. Front. Phys. 2023, 11, 222735. [Google Scholar]
- Pinchas, M.; Avraham, Y. A novel clock skew estimator and its performance for the IEEE 1588v2 (PTP) case in fractional Gaussian noise/generalized fractional Gaussian noise environment. Front. Phys. 2021, 9, 796811. [Google Scholar]
- Mirás-Avalos, J.M.; Trigo-Córdoba, E.; da Silva-Dias, R.; Varela-Vila, I.; García-Tomillo, A. Multifractal behaviour of the soil water content of a vineyard in northwest Spain during two growing seasons. Nonlinear Process. Geophys. 2016, 23, 205–213. [Google Scholar] [CrossRef]
- Kaulakys, B.; Alaburda, M.; Ruseckas, J. 1/f noise from the nonlinear transformations of the variables. Mod. Phys. Lett. B 2015, 29, 1550223. [Google Scholar] [CrossRef]
- Lubashevsky, I.A. Truncated Lévy flights and generalized Cauchy processes. Eur. Phys. J. B 2011, 82, 189–195. [Google Scholar] [CrossRef]
- Starchenko, V. Images fractal compression algorithms. Comput.-Integr. Technol. Educ. Sci. Prod. 2023, 53, 215–221. (In Ukrainian) [Google Scholar]
- Gorev, V.N.; Gusev, A.Y.; Korniienko, V.I.; Shedlovska, Y.I. Generalized fractional Gaussian noise prediction based on the Walsh functions. Radio Electron. Comput. Sci. Control 2023, 3, 48. [Google Scholar] [CrossRef]
- Sheluhin, O.; Rybakov, S.; Vanyushina, A. Modified algorithm for detecting network attacks using the fractal dimension jump estimation method in online mode. Proc. Telecommun. Univ. 2022, 8, 117–126. (In Russian) [Google Scholar] [CrossRef]
- Sousa-Vieira, M.E.; Fernández-Veiga, M. Efficient generators of the generalized Fractional Gaussian noise and Cauchy processes. Fract. Fract. 2023, 7, 455. [Google Scholar] [CrossRef]
- Beskardes, G.D.; Weiss, C.J.; Everett, M.E. Estimating the power-law distribution of Earth electrical conductivity from low-frequency, controlled-source electromagnetic responses. Geophys. J. Int. 2017, 208, 639–651. [Google Scholar] [CrossRef]
- Ercan, A.; Kavvas, M.L. Time-space fractional governing equations of one-dimensional unsteady open channel flow process: Numerical solution and exploration. Hydrol. Process. 2017, 31, 2961–2971. [Google Scholar] [CrossRef]
- Lee, M.; Genton, M.G.; Jun, M. Testing self-similarity through Lamperti transformations. J. Agric. Biol. Environ. Stat. 2016, 21, 426–447. [Google Scholar] [CrossRef]
- Li, M. Fractal time series—A tutorial review. Math. Probl. Eng. 2010, 2010, 157264. [Google Scholar] [CrossRef]
- Li, M. Multi-Fractal Traffic and Anomaly Detection in Computer Communications; CRC Press: Boca Raton, UK, 2022. [Google Scholar]
- Li, M. Fractal Teletraffic Modeling and Delay Bounds in Computer Communications; CRC Press: Boca Raton, UK, 2022. [Google Scholar]
- Li, Z.; Yan, L. Harnack inequalities for SDEs driven by subordinator fractional Brownian motion. Stat. Probab. Lett. 2018, 134, 45–53. [Google Scholar] [CrossRef]
- Gao, H.; Guo, R.; Jin, Y.; Yan, L. Large time behavior on the linear self-interacting diffusion driven by sub-fractional Brownian motion I: Self-repelling case. Front. Phys. 2021, 9, 795210. [Google Scholar] [CrossRef]
- Guo, H.; Gao, R.; Jin, Y.; Yan, L. Large time behavior on the linear self-interacting diffusion driven by sub-fractional Brownian motion II: Self-attracting case. Front. Phys. 2021, 9, 791858. [Google Scholar] [CrossRef]
- Gao, L.; Sun, X. Almost periodic solutions to impulsive stochastic delay differential equations driven by fractional Brownian motion with 0.5 < H < 1. Front. Phys. 2021, 9, 783125. [Google Scholar]
- Pei, W.; Zhang, Z. Stability of hybrid SDEs driven by fBm. Front. Phys. 2021, 9, 783434. [Google Scholar] [CrossRef]
- Barth, A.; Stüwe, T. Weak convergence of Galerkin approximations of stochastic partial differential equations driven by additive Lévy noise. Math. Comput. Simul. 2018, 143, 215–225. [Google Scholar] [CrossRef]
- Kim, K.-H.; Park, D. A Sobolev space theory for time-fractional stochastic partial differential equations driven by Lévy processes. J. Theor. Probab. 2024, 37, 671–720. [Google Scholar] [CrossRef]
- Noupelah, A.J.; Tambue, A.; Woukeng, J.L. Strong convergence of a fractional exponential integrator scheme for finite element discretization of time-fractional SPDE driven by fractional and standard Brownian motions. Commun. Nonlinear Sci. Numer. Simul. 2023, 125, 107371. [Google Scholar] [CrossRef]
- Massing, T. Approximation and error analysis of forward–backward SDEs driven by general Lévy processes using shot noise series representations. ESAIM Probab. Stat. 2023, 27, 694–722. [Google Scholar] [CrossRef]
- Lee, C.Y. Local nondeterminism and local times of the stochastic wave equation driven by fractional-colored noise. J. Fourier Anal. Appl. 2022, 28, 26. [Google Scholar] [CrossRef]
- Fa, K.S.; Pianegonda, S.; da Luz, M.G.E. Broad class of nonlinear Langevin equations driven by multiplicative Ornstein–Uhlenbeck noise: Generalized n-moment and generalized second Einstein relation. Physical A 2023, 622, 128807. [Google Scholar] [CrossRef]
- Freundlich, J.; Sado, D. Dynamics of a mechanical system with a spherical pendulum subjected to fractional damping: Analytical analysis. Nonlinear Dyn. 2023, 111, 7961–7973. [Google Scholar] [CrossRef]
- Burlon, A. On the numerical solution of fractional differential equations under white noise processes. Probab. Eng. Mech. 2023, 73, 103465. [Google Scholar] [CrossRef]
- Wang, W.; Yan, Z.; Liu, X. The escape problem and stochastic resonance in a bistable system driven by fractional Gaussian noise. Phys. Lett. A 2017, 381, 2324–2336. [Google Scholar] [CrossRef]
- Hu, Y.; Zhou, X.Y. Stochastic control for linear systems driven by fractional noises. SIAM J. Control Optim. 2005, 43, 2245–2277. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, Y.; Caraballo, T. The continuity, regularity and polynomial stability of mild solutions for stochastic 2D-Stokes equations with unbounded delay driven by tempered fractional Gaussian noise. Stoch. Dyn. 2022, 22, 2250022. [Google Scholar] [CrossRef]
- Li, M.; Lim, S.C. A rigorous derivation of power spectrum of fractional Gaussian noise. Fluct. Noise Lett. 2006, 6, C33–C36. [Google Scholar] [CrossRef]
- Li, M.; Chi, C.-H. A correlation-based computational method for simulating long-range dependent data. J. Frankl. Inst. 2003, 340, 503–514. [Google Scholar] [CrossRef]
- He, J.; Xing, Z.; Guo, Q. Spectral collocation method for stochastic differential equations driven by fractional Brownian motion. Fluct. Noise Lett. 2023, 22, 2350019. [Google Scholar] [CrossRef]
- He, J.; Guo, Q. An explicit method for the self-interacting diffusion driven by fractional Brownian motion under global Lipschitz conditions. Appl. Math. Lett. 2022, 134, 108379. [Google Scholar] [CrossRef]
- Liu, X. Strong approximation for fractional wave equation forced by fractional Brownian motion with Hurst parameter H ∈ (0, 0.5). J. Comput. Appl. Math. 2023, 432, 115285. [Google Scholar] [CrossRef]
- Tuan, N.H.; Caraballo, T.; Thach, T.N. New results for stochastic fractional pseudo-parabolic equations with delays driven by fractional Brownian motion. Stoch. Process. Their Appl. 2023, 161, 24–67. [Google Scholar] [CrossRef]
- Tuan, N.H.; Foondun, M.; Thach, T.N.; Wang, R. On backward problems for stochastic fractional reaction equations with standard and fractional Brownian motion. Bul. Sci. Math. 2022, 179, 103158. [Google Scholar] [CrossRef]
- Sharma, N.; Selvamuthu, D.; Natarajan, S. Variable annuities valuation under a mixed fractional Brownian motion environment with jumps considering mortality risk. Appl. Stoch. Models Bus. Ind. 2022, 38, 1019–1038. [Google Scholar] [CrossRef]
- Fan, X.; Huang, X.; Suo, Y.; Yuan, C. Distribution dependent SDEs driven by fractional Brownian motions. Stoch. Process. Their Appl. 2022, 151, 23–67. [Google Scholar] [CrossRef]
- Zhang, S.-Q.; Yuan, C. Stochastic differential equations driven by fractional Brownian motion with locally Lipschitz drift and their implicit Euler approximation. Proc. R. Soc. Edinb. Sect. A Math. 2021, 151, 1278–1304. [Google Scholar] [CrossRef]
- Shahnazi-Pour, A.; Moghaddam, B.P.; Babaei, A. Numerical simulation of the Hurst index of solutions of fractional stochastic dynamical systems driven by fractional Brownian motion. J. Comput. Appl. Math. 2021, 386, 113210. [Google Scholar] [CrossRef]
- Araya, H.; León, J.A.; Torres, S. Numerical scheme for stochastic differential equations driven by fractional Brownian motion with ¼ < H < 1/2. J. Theor. Probab. 2020, 33, 1211–1237. [Google Scholar]
- Gairing, J.; Imkeller, P.; Shevchenko, R.; Tudor, C. Hurst Index estimation in stochastic differential equations driven by fractional Brownian motion. J. Theor. Probab. 2020, 33, 1691–1714. [Google Scholar] [CrossRef]
- Xu, P.; Huang, J.; Zeng, C. Ergodicity of stochastic Rabinovich systems driven by fractional Brownian motion. Physical A 2020, 546, 122955. [Google Scholar] [CrossRef]
- Heydari, M.H.; Avazzadeh, Z.; Mahmoudi, M.R. Chebyshev cardinal wavelets for nonlinear stochastic differential equations driven with variable-order fractional Brownian motion. Chaos Solitons Fractals 2019, 124, 105–124. [Google Scholar] [CrossRef]
- Heydari, M.H.; Mahmoudi, M.R.; Shakiba, A.; Avazzadeh, Z. Chebyshev cardinal wavelets and their application in solving nonlinear stochastic differential equations with fractional Brownian motion. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 98–121. [Google Scholar] [CrossRef]
- Pavliotis, G.A. Stochastic Processes and Applications, Diffusion Processes, the Fokker-Planck and Langevin Equations; Texts in Applied Mathematics; Springer: New York, NY, USA, 2014; Volume 60. [Google Scholar]
- Coffey, W.T.; Kalmykov, Y.P.; Waldron, J.T. The Langevin Equation with Applications to Stochastic Problems in Physics, Chemistry and Electrical Engineering, 2nd ed.; World Scientific: Singapore, 2004. [Google Scholar]
- West, B.J.; Geneston, E.L.; Grigolini, P. Maximizing information exchange between complex networks. Phys. Rep. 2008, 468, 1–99. [Google Scholar] [CrossRef]
- Lim, S.C.; Li, M.; Teo, L.P. Langevin equation with two fractional orders. Phys. Lett. A 2008, 372, 6309–6320. [Google Scholar] [CrossRef]
- Lim, S.C.; Li, M.; Teo, L.P. Locally self-similar fractional oscillator processes. Fluct. Noise Lett. 2007, 7, L169–L179. [Google Scholar] [CrossRef]
- Shao, Y. The fractional Ornstein-Uhlenbeck process as a representation of homogeneous Eulerian velocity turbulence. Physical D 1995, 83, 461–477. [Google Scholar] [CrossRef]
- Cheridito, P.; Kawaguchi, H.; Maejima, M. Fractional Ornstein-Uhlenbeck processes. Electron. J. Probab. 2003, 8, 1–14. [Google Scholar] [CrossRef]
- Magdziarz, M. Fractional Ornstein–Uhlenbeck processes, Joseph effect in models with infinite variance. Physical A 2008, 387, 123–133. [Google Scholar] [CrossRef]
- Gehringer, J.; Li, X.-M. Functional Limit Theorems for the fractional Ornstein–Uhlenbeck process. J. Theor. Probab. 2022, 35, 426–456. [Google Scholar] [CrossRef]
- Patel, H.G.; Sharma, S.N. Some evolution equations for an Ornstein–Uhlenbeck process-driven dynamical system. Fluct. Noise Lett. 2012, 11, 1250020. [Google Scholar] [CrossRef]
- Faltinsen, O.M. Sea Loads on Ships and Offshore Structures, 2nd ed.; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Holmes, J.D. Wind Loading of Structure, 2nd ed.; Taylor & Francis: London, UK; New York, NY, USA, 2007. [Google Scholar]
- Crandall, S.H.; Mark, W.D. Random Vibration in Mechanical Systems; Elsevier Inc.: New York, NY, USA; Academic Press: New York, NY, USA, 1963. [Google Scholar]
- Elishakoff, I.; Lyon, R.H. (Eds.) Random Vibration Status and Recent Developments; Elsevier: New York, NY, USA, 1986. [Google Scholar]
- Rothbart, H.A.; Brown, T.H., Jr. Mechanical Design Handbook, 2nd ed.; Measurement, Analysis and Control of Dynamic Systems; McGraw–Hill: New York, NY, USA, 2006. [Google Scholar]
- Jensen, J.J. (Ed.) Load and Global Response of Ships; Elsevier: Oxford, UK; Academic Press: Oxford, UK, 2001; Volume 4. [Google Scholar]
- Harris, C.M. Shock and Vibration Handbook, 5th ed.; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock, 2nd ed.; Random Vibration; John Wiley & Sons: Hoboken, NJ, USA, 2013; Volume 3. [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock, 2nd ed.; Fatigue Damage; John Wiley & Sons: Hoboken, NJ, USA, 2013; Volume 4. [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock, 2nd ed.; Specification Development; John Wiley & Sons: Hoboken, NJ, USA, 2013; Volume 5. [Google Scholar]
- Li, M. An iteration method to adjusting random loading for a laboratory fatigue test. Int. J. Fatigue 2005, 27, 783–789. [Google Scholar] [CrossRef]
- Li, M. Experimental stability analysis of test system for doing fatigue test under random loading. J. Test. Eval. 2006, 34, 364–367. [Google Scholar]







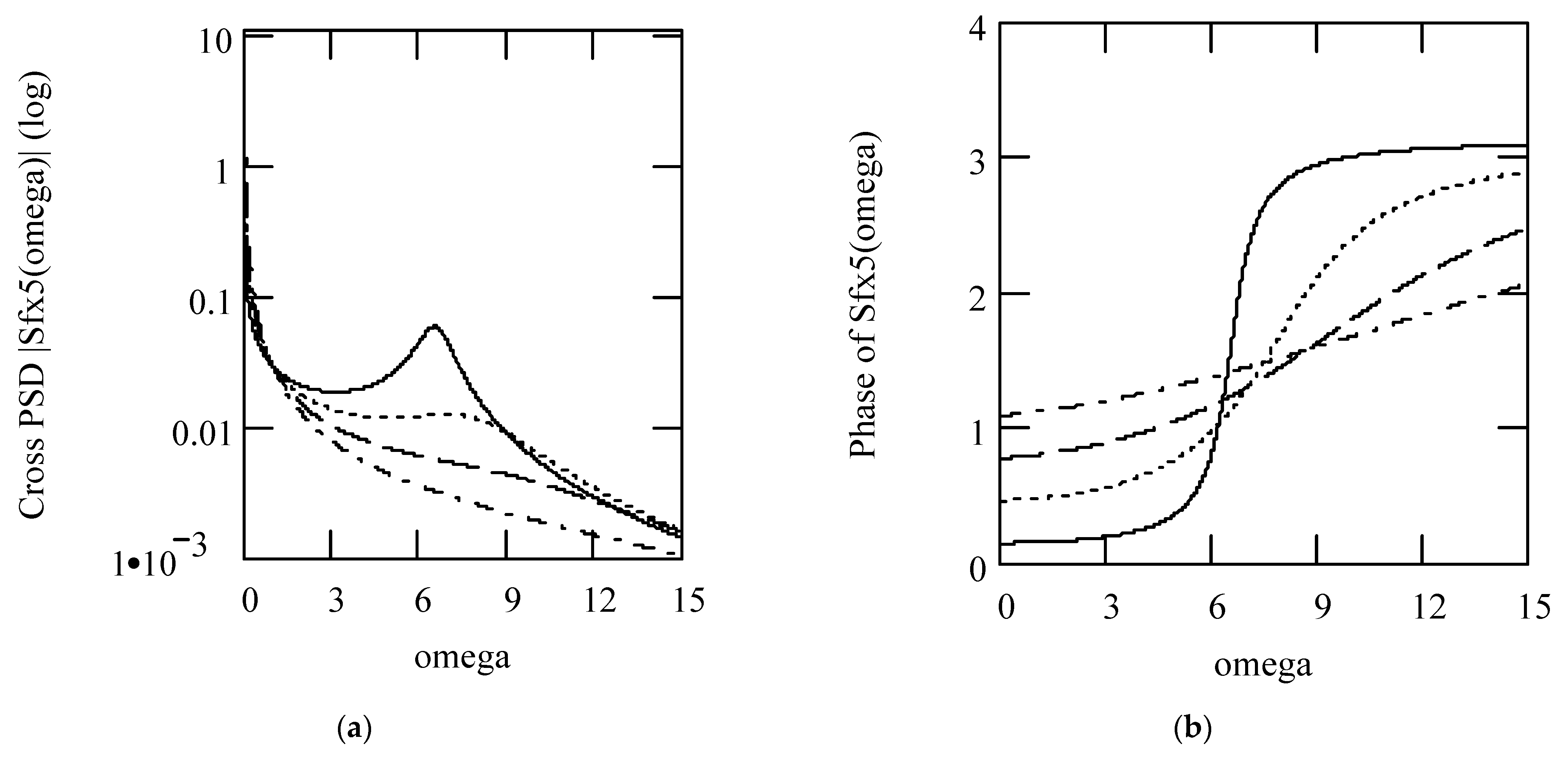

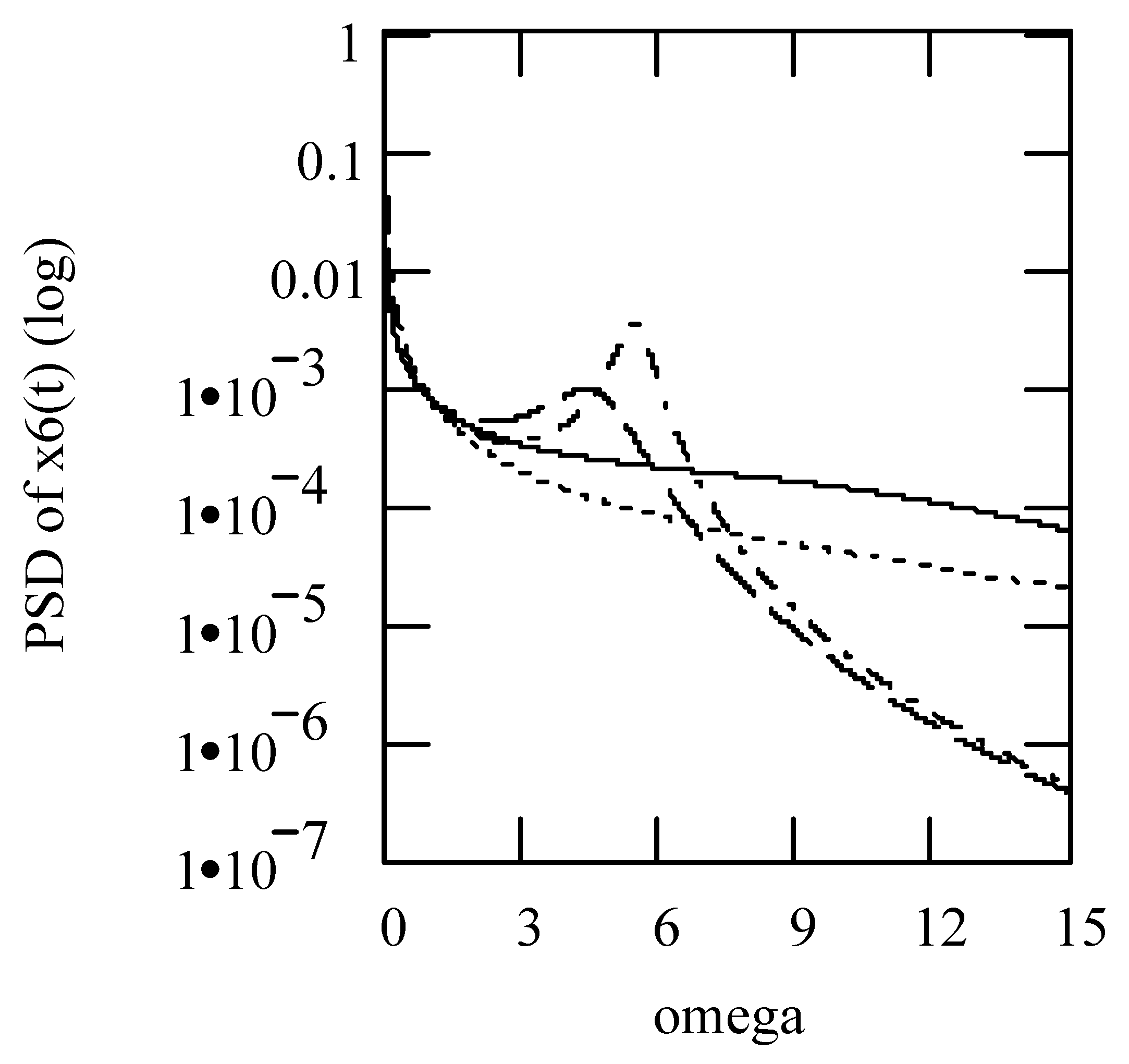
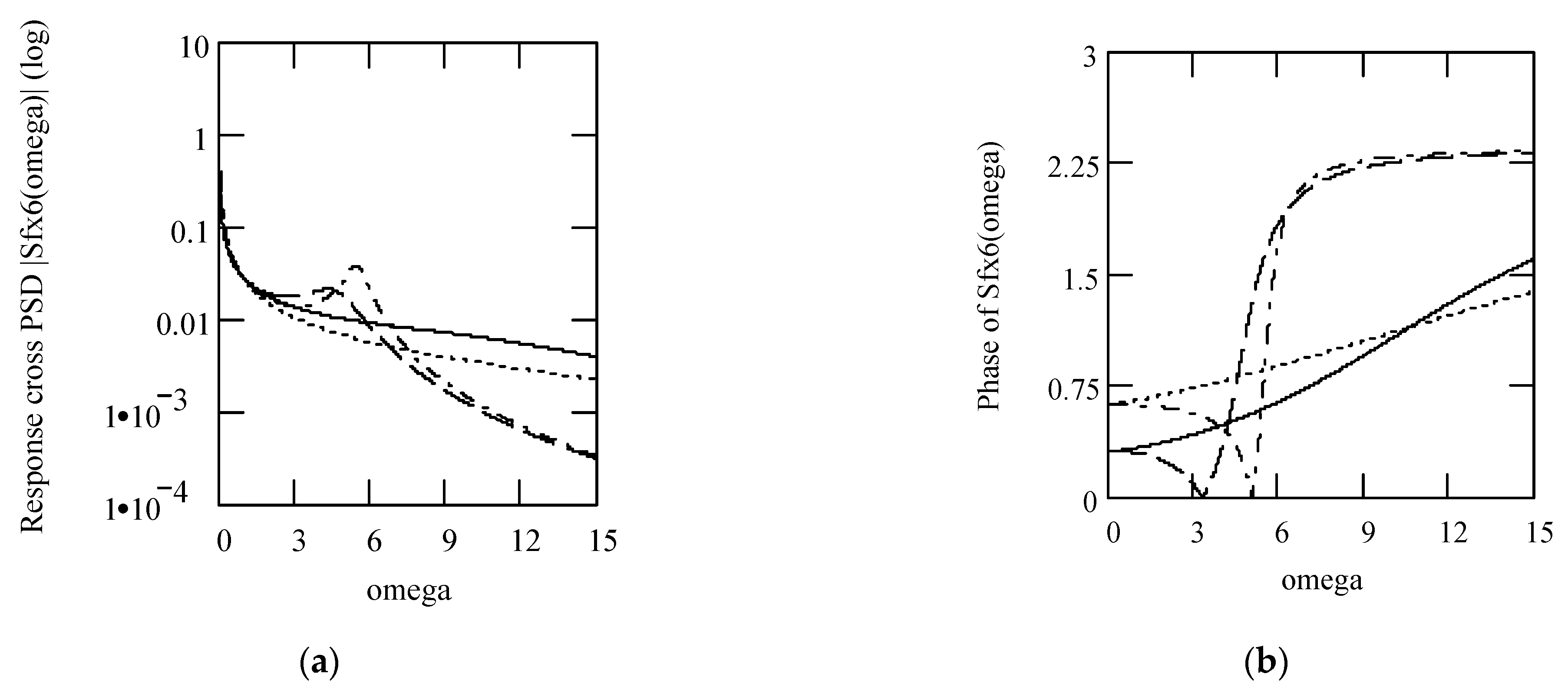

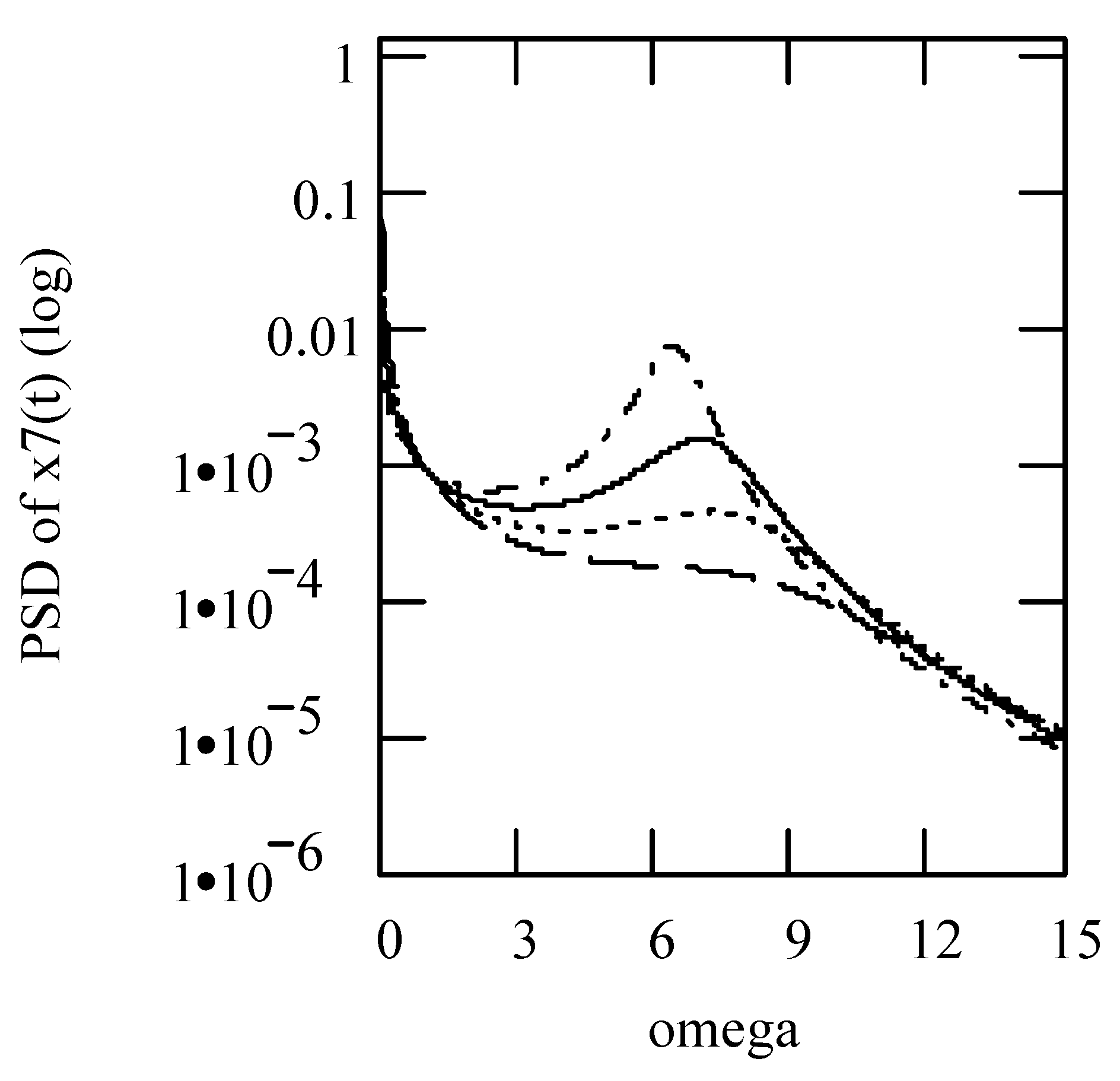




























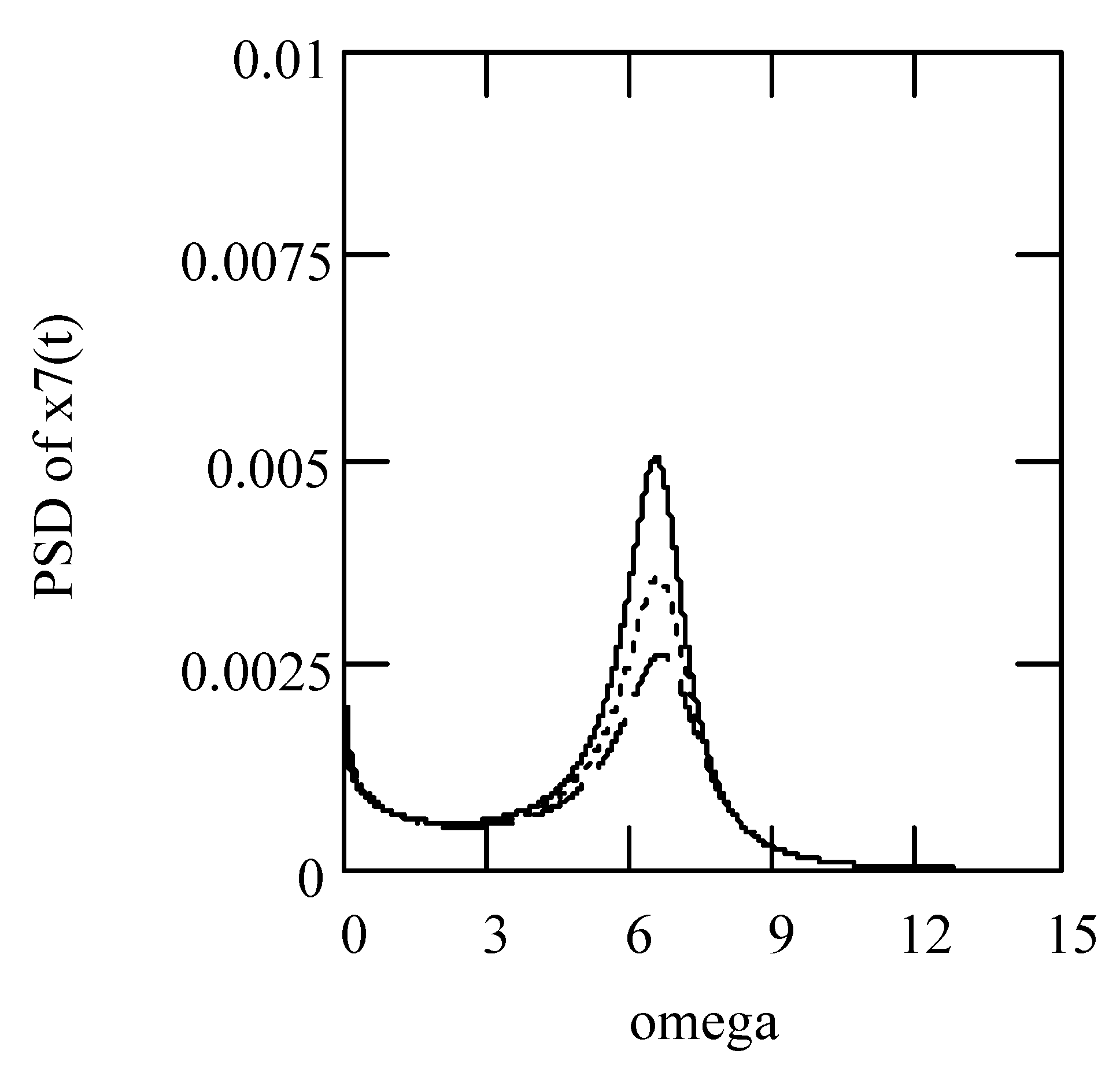
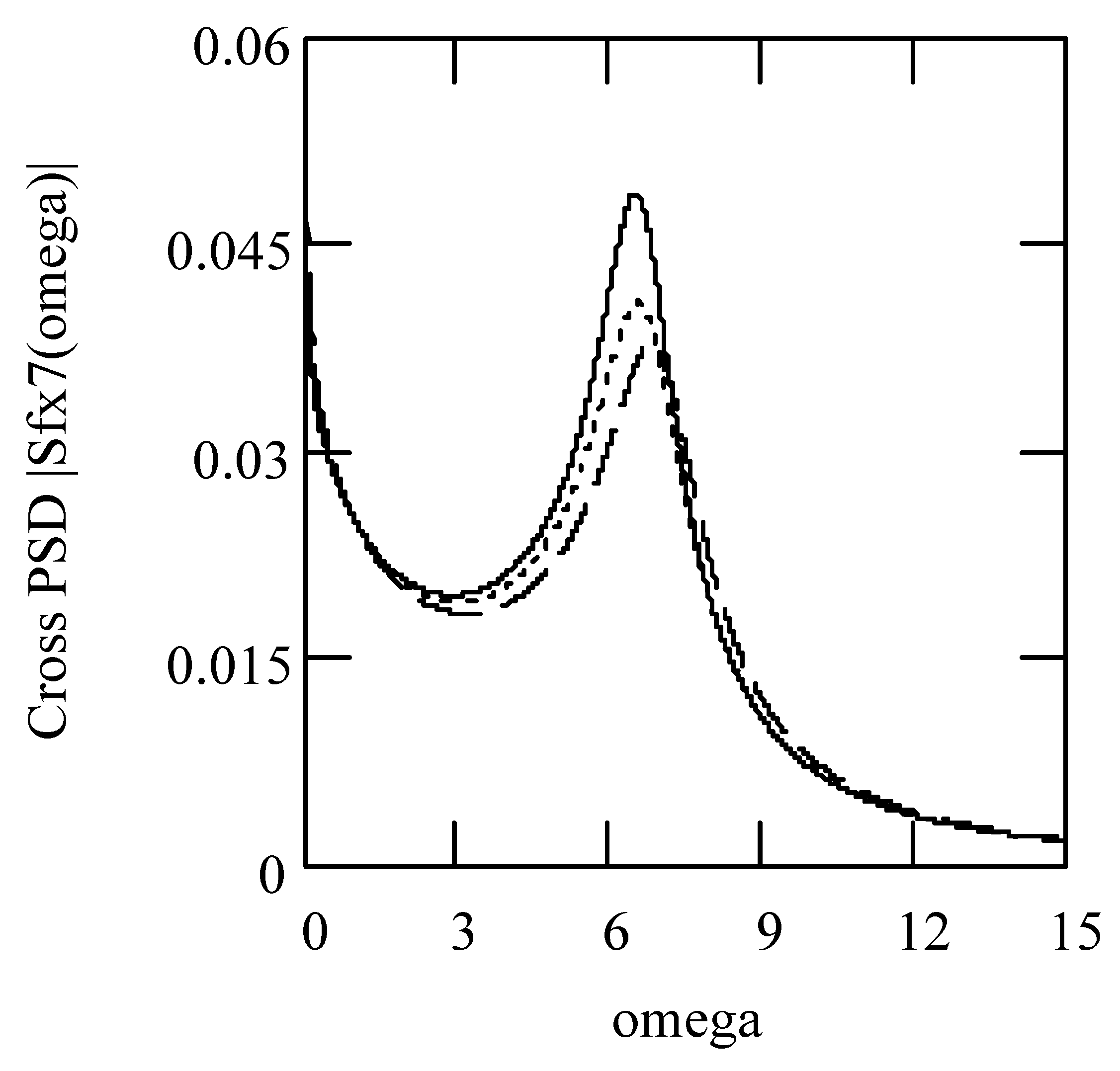
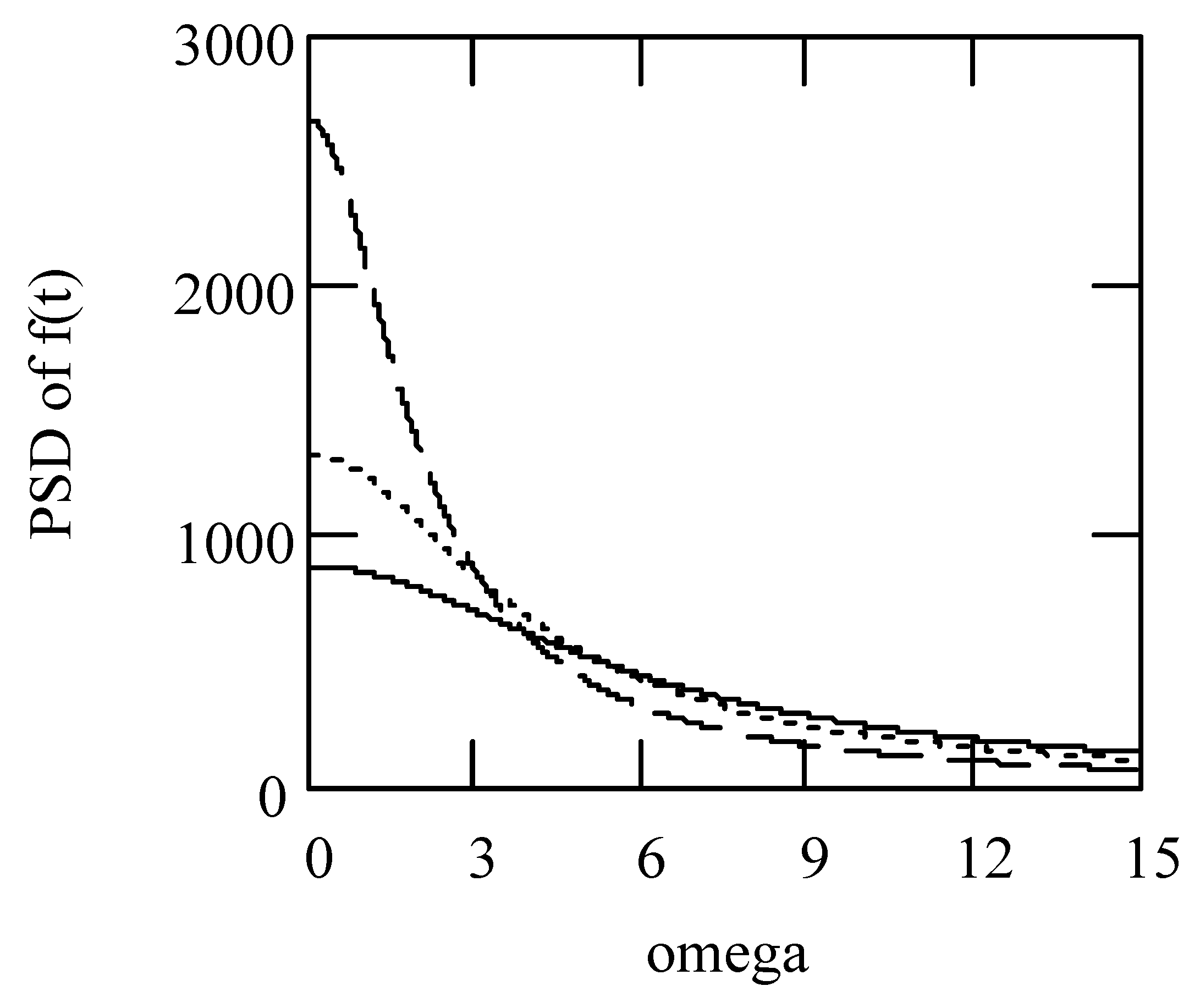
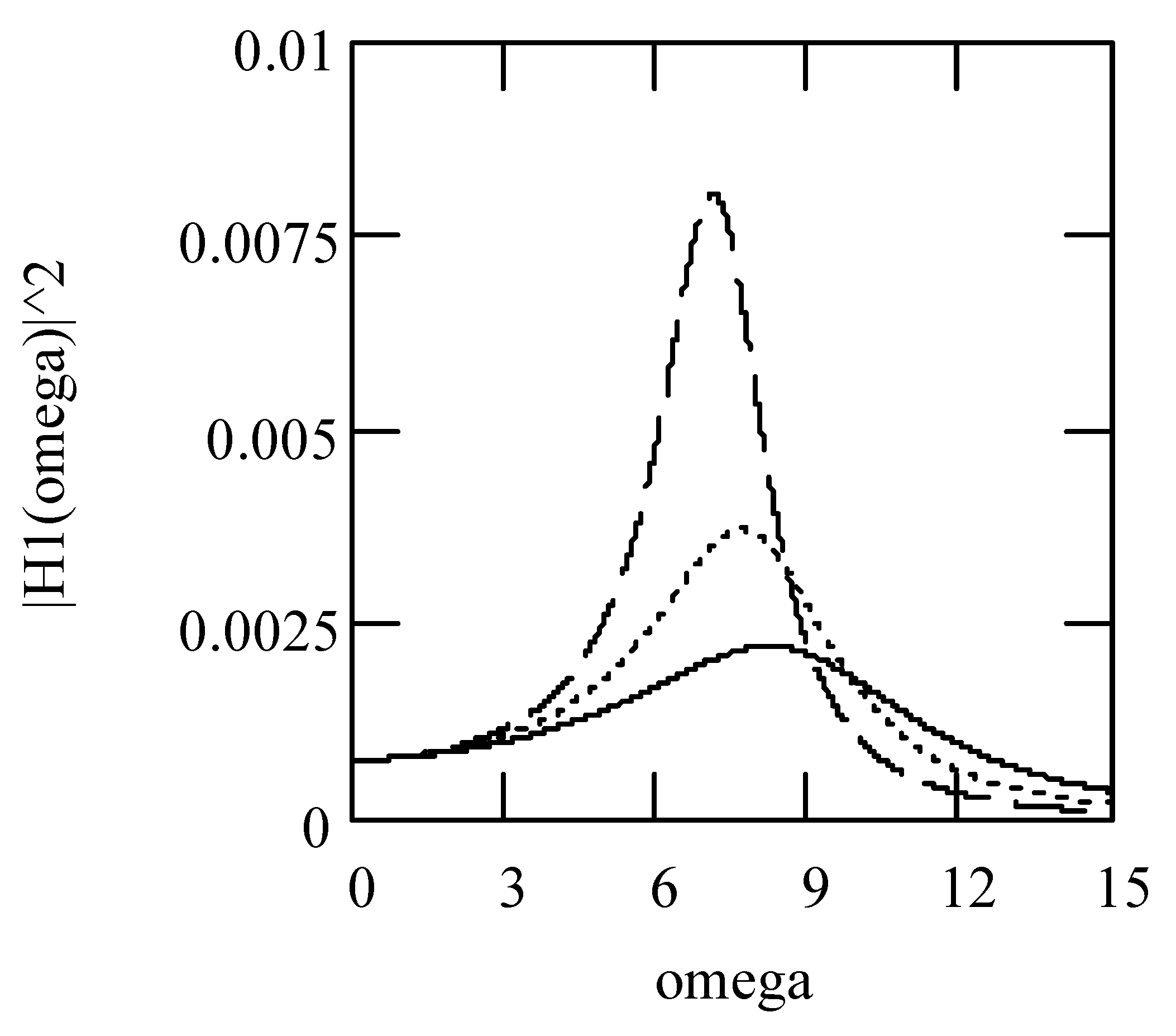















Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M. PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process. Symmetry 2024, 16, 635. https://doi.org/10.3390/sym16050635
Li M. PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process. Symmetry. 2024; 16(5):635. https://doi.org/10.3390/sym16050635
Chicago/Turabian StyleLi, Ming. 2024. "PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process" Symmetry 16, no. 5: 635. https://doi.org/10.3390/sym16050635
APA StyleLi, M. (2024). PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process. Symmetry, 16(5), 635. https://doi.org/10.3390/sym16050635





