Using Voronoi Tessellation Diagrams to Visualize the Mechanical Response of Interacting Axisymmetric Simultaneous Propagating Waves
Abstract
1. Introduction
Transient Stress Fields in Rock Blasting Applications
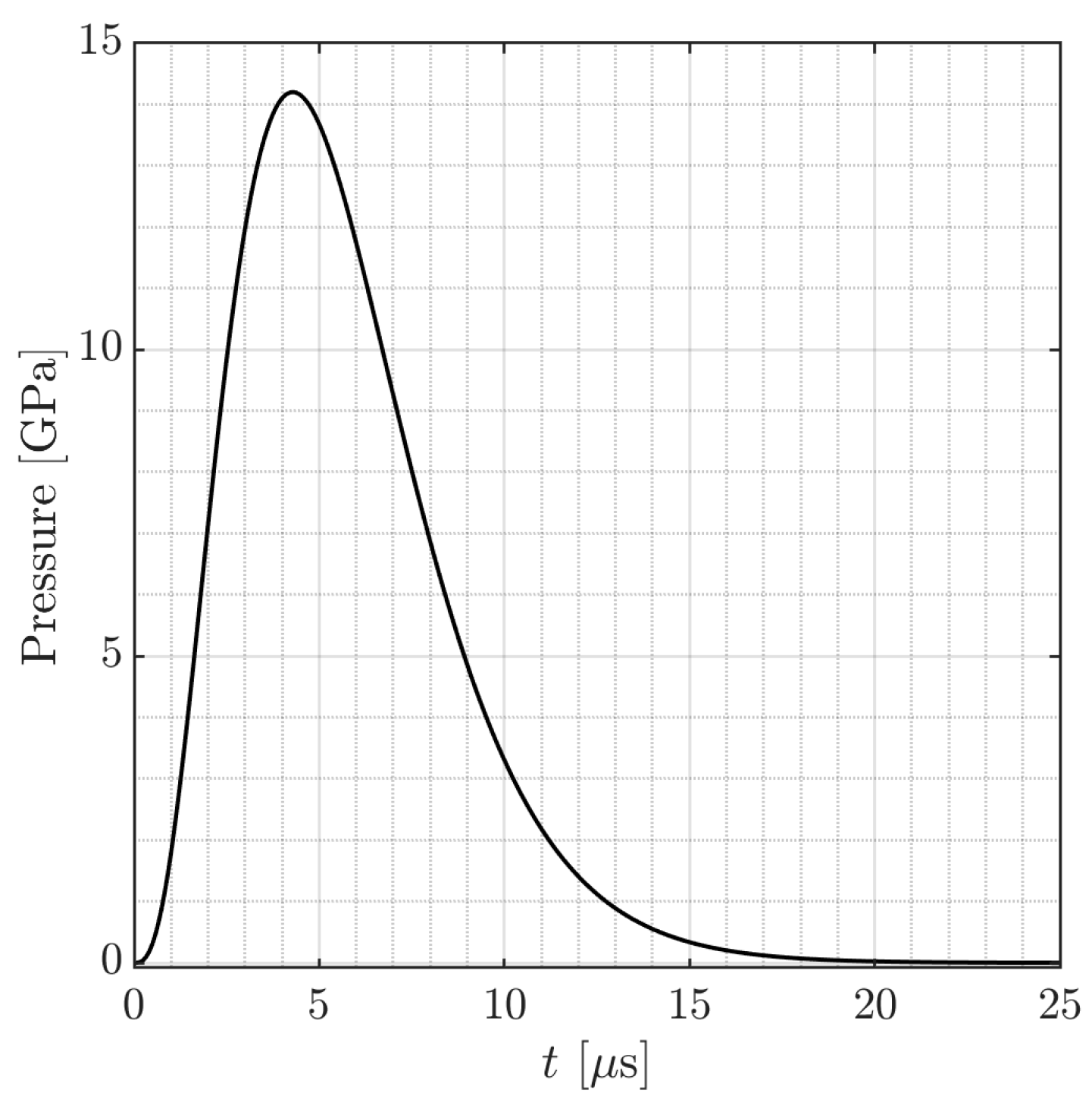
2. Solution of Axisymmetric Stress Wave Propagation from Single Source
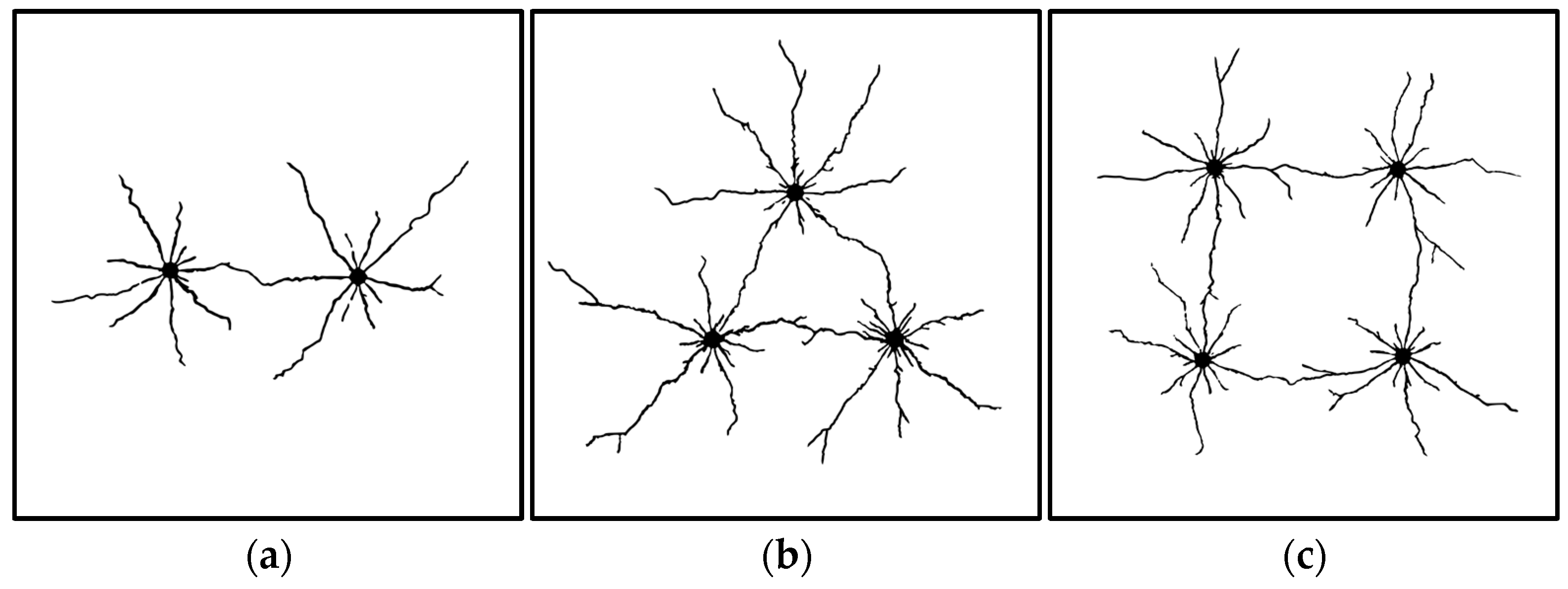
3. Stress Wave Propagation and Interaction Between Multiple Regularly Spaced Blast Sources
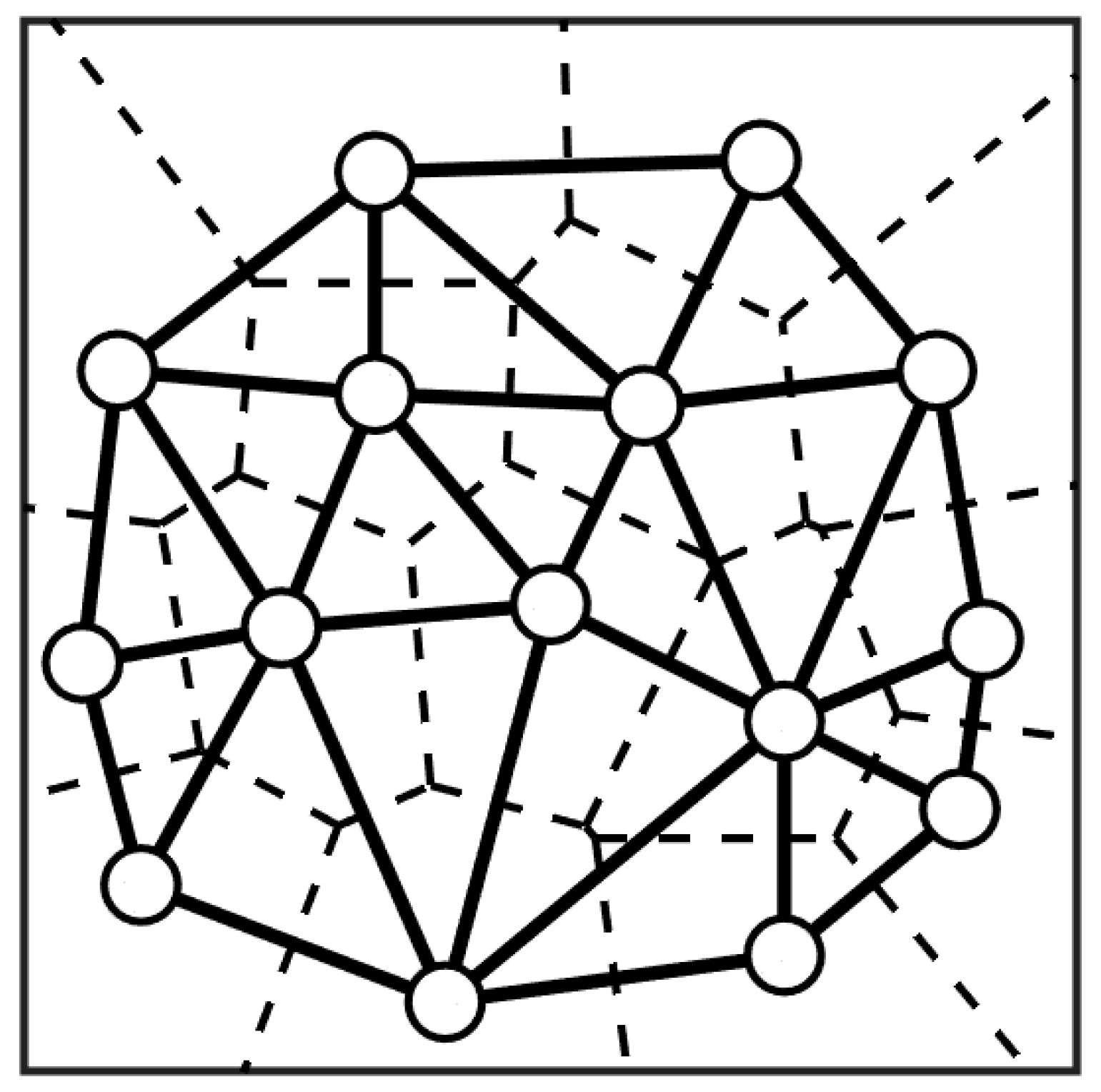
4. Voronoi Tessellation and Delaunay Triangulation Diagrams
Prediction of Principal Stress and Crack Behavior for Multiple Sources
5. Stress Wave Interaction and Fracture Pattern Prediction Using Abaqus/Explicit
5.1. Visualization of Stress Interactions in Simplified Rock Model
5.2. Visualization of Stress Wave Interactions and Fracture Patterns in Granite
5.3. Visualization of Stress Wave Interactions and Fracture Patterns in Silicon Carbide
6. Further Discussion
7. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Parameter | Unit | Value | |
|---|---|---|---|
| Density | kg/m3 | 2660 | |
| Bulk modulus | K1 | GPa | 20.45 |
| Polynomial EOS constant | K2 | GPa | 4500 |
| Polynomial EOS constant | K3 | GPa | 0.0003 |
| Shear modulus | G | GPa | 15.33 |
| Hugoniot Elastic Limit | HEL | GPa | 4.19 |
| Intact strength constant | A | - | 0.97 |
| Intact strength exponent | N | - | 0.64 |
| Strain rate constant | C | - | 0.005 |
| Reference strain rate | s−1 | 1.0 | |
| Fractured strength constant | B | - | 0.32 |
| Fractured strength exponent | M | - | 0.64 |
| Normalized tensile strength | T * | - | 0.014 |
| Pressure at Hugoniot Elastic Limit | PHEL | GPa | 3.63 |
| Damage constant | D1 | - | 0.005 |
| Damage constant | D2 | - | 0.70 |
| Bulking constant | β | - | 0.50 |
| Parameter | Unit | Value | |
|---|---|---|---|
| Density | kg/m3 | 3215 | |
| Bulk modulus | K1 | GPa | 220 |
| Polynomial EOS constant | K2 | GPa | 361 |
| Polynomial EOS constant | K3 | GPa | 0 |
| Shear modulus | G | GPa | 193 |
| Hugoniot Elastic Limit | HEL | GPa | 11.7 |
| Intact strength constant | A | - | 0.96 |
| Intact strength exponent | N | - | 0.65 |
| Strain rate constant | C | - | 0.009 |
| Reference strain rate | s−1 | 1.0 | |
| Fractured strength constant | B | - | 0.35 |
| Fractured strength exponent | M | - | 1.0 |
| Tensile strength | T | - | 0.75 |
| Pressure at Hugoniot Elastic Limit | PHEL | GPa | 5.13 |
| Damage constant | D1 | - | 0.48 |
| Damage constant | D2 | - | 0.48 |
| Bulking constant | β | - | 1.0 |
| Max. equivalent plastic fracture strain | - | 1.2 | |
| Min. equivalent plastic fracture strain | - | 0.0 | |
| Element deletion parameter | FS | - | 0.2 |
References
- Qiu, S.; Eliasson, V. Interaction and coalescence of multiple simultaneous and non-simultaneous blast waves. Shock Waves 2016, 26, 287–297. [Google Scholar] [CrossRef]
- Almustafa, M.K.; Nehdi, M.L. Fundamental review on collision of blast waves. Phys. Fluids 2023, 35, 031302. [Google Scholar] [CrossRef]
- Rossmanith, H.P.; Uenishi, K.; Kouzniak, N. Blast wave propagation in rock mass—Part I: Monolithic medium. Fragblast 1997, 1, 317–359. [Google Scholar] [CrossRef]
- He, C.; Gao, J.; Chen, D.; Xiao, J. Investigation of Stress Wave Interaction and Fragmentation in Granite During Multihole Blastings. IEEE Access 2020, 8, 185187–185197. [Google Scholar] [CrossRef]
- Yi, C.; Johansson, D.; Nyberg, U.; Beyglou, A. Stress Wave Interaction Between Two Adjacent Blast Holes. Rock Mech. Rock Eng. 2016, 49, 1803–1812. [Google Scholar] [CrossRef]
- Chew, L.P.; Dyrsdale, R.L. Voronoi diagrams based on convex distance functions. In Proceedings of the First Annual Symposium on Computational Geometry—SCG’85, Baltimore, MD, USA, 5–7 June 1985; ACM Press: Baltimore, MD, USA, 1985; pp. 235–244. [Google Scholar] [CrossRef]
- Zhang, Z.-X. Rock Fracture and Blasting: Theory and Applications; Elsevier: Oxford, UK, 2016. [Google Scholar]
- Yuan, W.; Liu, S.; Wang, W.; Su, X.; Li, Z.; Li, J.; Wen, L.; Chang, J.; Sun, X. Numerical study on the fracturing mechanism of shock wave interactions between two adjacent blast holes in deep rock blasting. Earthq. Eng. Eng. Vib. 2019, 18, 735–746. [Google Scholar] [CrossRef]
- Yang, R.; Fang, S.; Yang, A.; Xie, H.; Yang, L. In Situ Stress Effects on Smooth Blasting: Model Test and Analysis. Shock Vib. 2020, 2020, 2124694. [Google Scholar] [CrossRef]
- Ding, C.; Yang, R.; Feng, C. Stress wave superposition effect and crack initiation mechanism between two adjacent boreholes. Int. J. Rock Mech. Min. Sci. 2021, 138, 104622. [Google Scholar] [CrossRef]
- Rossmanith, H.P. The Use of Lagrange Diagrams in Precise Initiation Blasting. Part I: Two Interacting Blastholes. Fragblast 2002, 6, 104–136. [Google Scholar] [CrossRef]
- Tang, H.-L.; Yang, J.; Yu, Q. Experimental Investigation of the Effect of Delay Time on Rock Fragmentation in Multi-Hole Bench Blasting. Appl. Sci. 2023, 13, 7329. [Google Scholar] [CrossRef]
- Rossmanith, H.P.; Knasmillner, R.E.; Daehnke, A.; Mishnaevsky, L. Wave propagation, damage evolution, and dynamic fracture extension. Part II. Blasting. Mater. Sci. 1996, 32, 403–410. [Google Scholar] [CrossRef]
- Needham, C.E. Blast Waves; Springer International Publishing: Cham, Switzerland, 2018. [Google Scholar] [CrossRef]
- Scott, R.A.; Miklowitz, J. Transient Compressional Waves in an Infinite Elastic Plate with a Circular Cylindrical Cavity. J. Appl. Mech. 1964, 31, 627–634. [Google Scholar] [CrossRef]
- Miklowitz, J. The Theory of Elastic Waves and Waveguides; North Holland Publishing Company: Amsterdam, NY, USA, 1984. [Google Scholar]
- Blair, D.P. A comparison of Heelan and exact solutions for seismic radiation from a short cylindrical charge. Geophysics 2007, 72, E33–E41. [Google Scholar] [CrossRef]
- Grady, D.E.; Kipp, M.E.; Smith, C.S. Explosive Fracture Studies on Oil Shale. Soc. Pet. Eng. J. 1980, 20, 349–356. [Google Scholar] [CrossRef]
- Larson, D.B. Explosive energy coupling in geologic materials. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1982, 19, 157–166. [Google Scholar] [CrossRef]
- Bezanson, J.; Edelman, A.; Karpinski, S.; Shah, V.B. Julia: A Fresh Approach to Numerical Computing. SIAM Rev. 2017, 59, 65–98. [Google Scholar] [CrossRef]
- MethodOfLines.jl: Automated Finite Difference for Physics-Informed Learning. Available online: https://docs.sciml.ai/MethodOfLines/stable/ (accessed on 26 March 2025).
- Kutter, H.H.K.; Fairhurst, C. On the fracture process in blasting. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1971, 8, 181–202. [Google Scholar] [CrossRef]
- Whittaker, B.N.; Singh, R.N.; Sun, G. Rock Fracture Mechanics: Principles, Design, and Applications; Elsevier: Amsterdam, NY, USA, 1992. [Google Scholar]
- MATLAB Software, Version R2023b; The MathWorks, Inc.: Natick, MA, USA, 2023.
- Voronoi Diagrams—MATLAB & Simulink. Available online: https://www.mathworks.com/help/matlab/math/voronoi-diagrams.html (accessed on 2 November 2024).
- ABAQUS Software/Standard User’s Manual, Version 2014; Dassault Systèmes: Vélizy-Villacoublay, France, 2014.





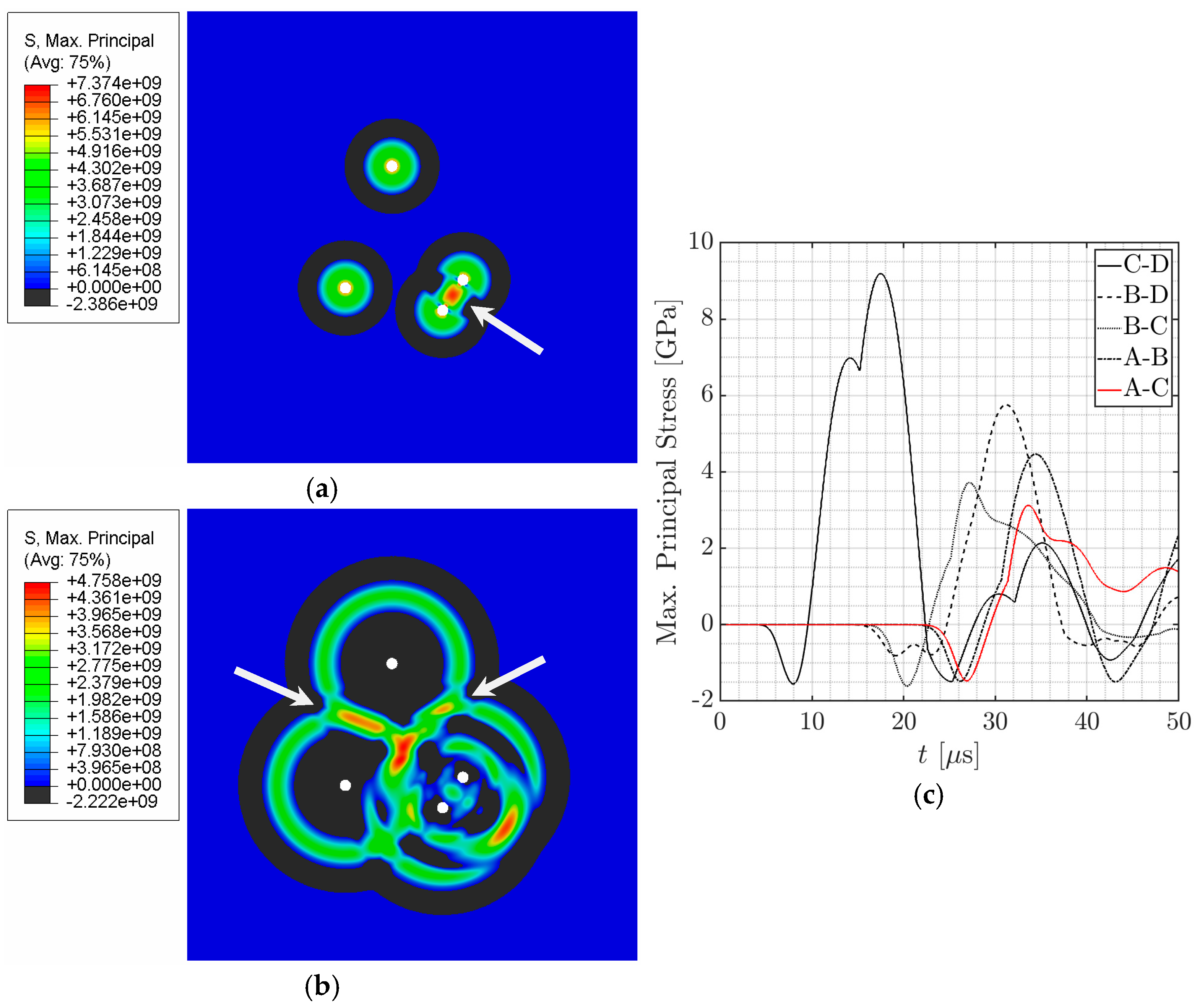
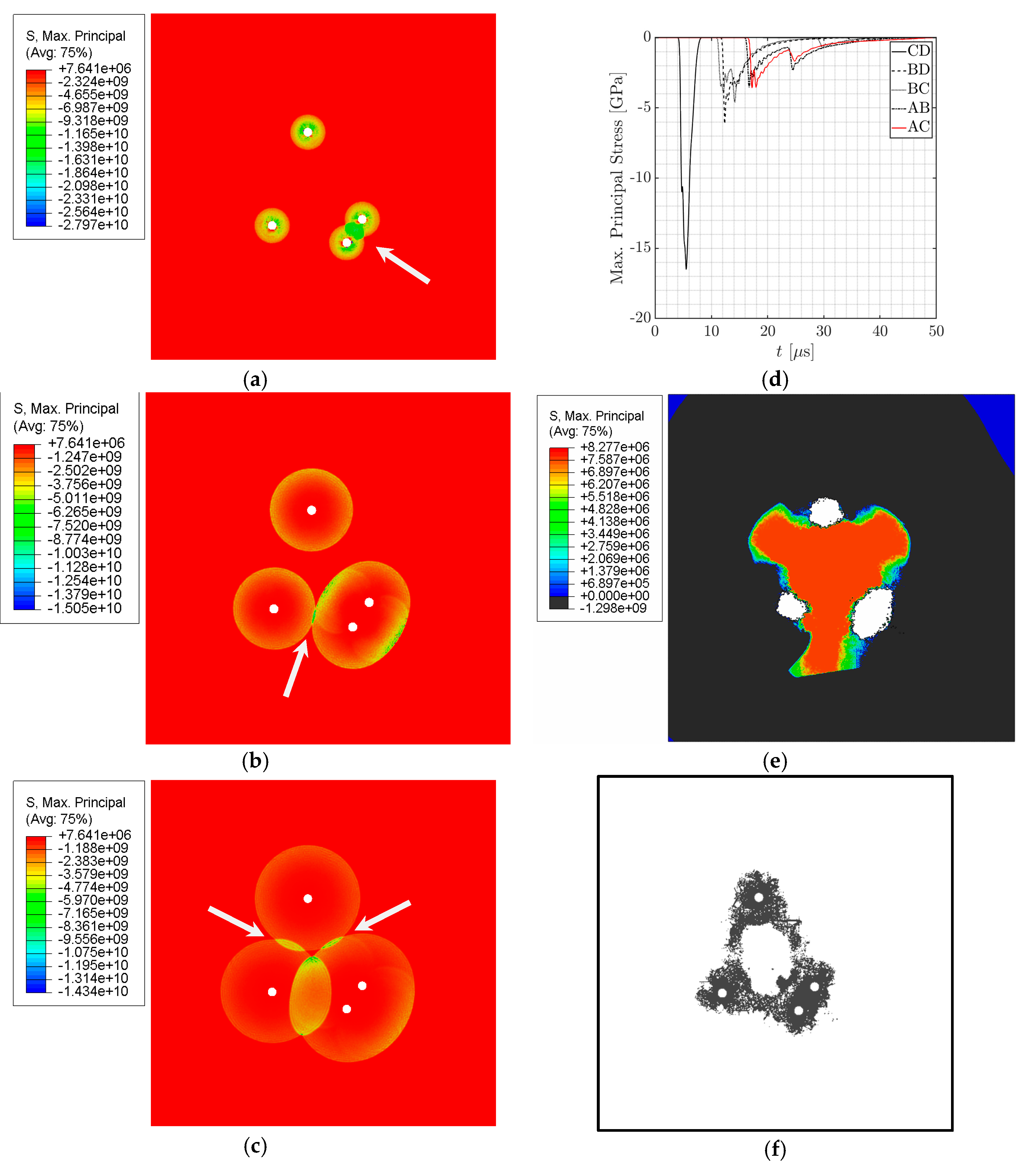
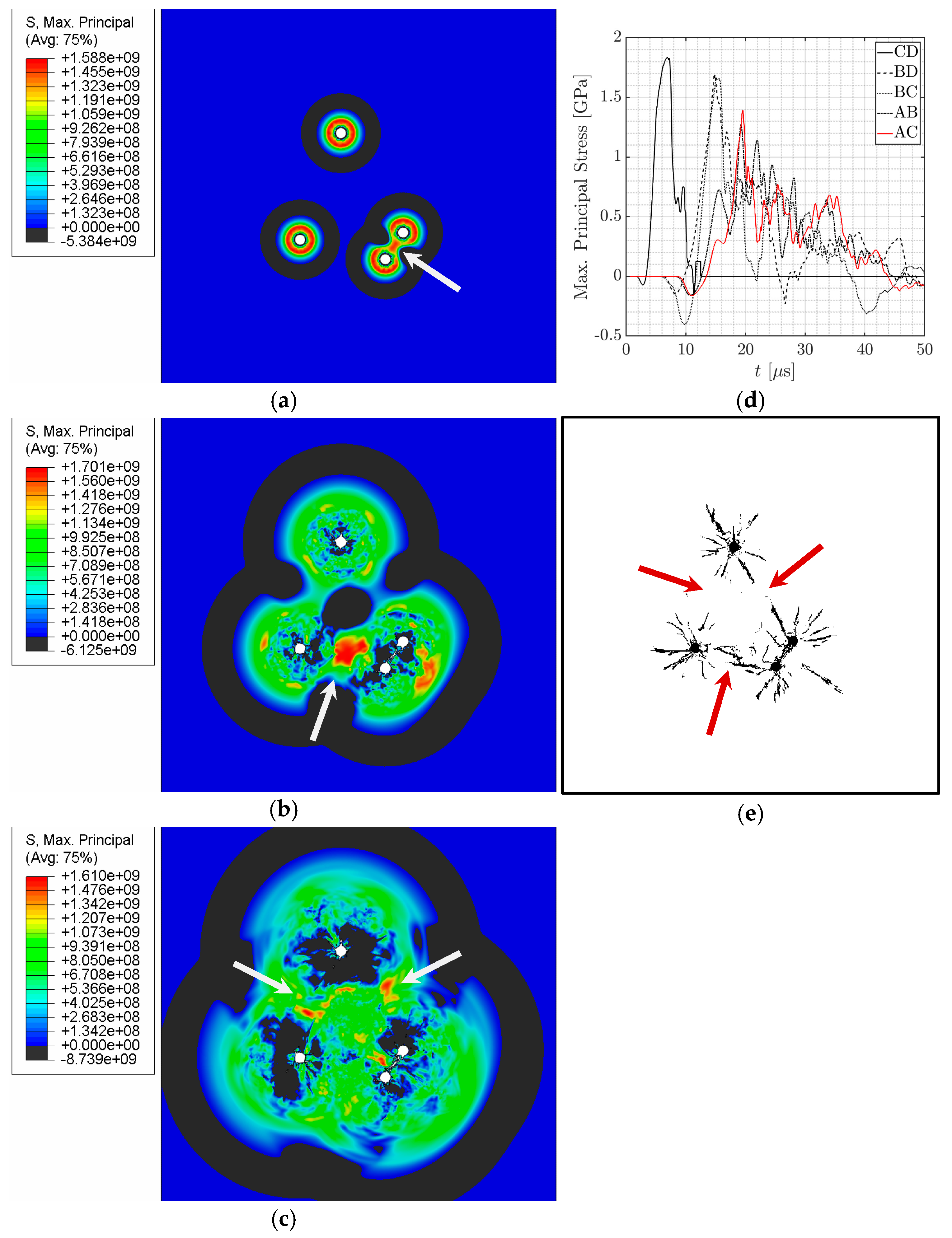
| Analytical | Abaqus Simulations | ||||
|---|---|---|---|---|---|
| Edge | Length [mm] | [GPa] | [µs] | Max. Principal Tensile Stress [GPa] | [µs] |
| A-B | 231.3 | 3.23 | 22.8 | 4.47 | 22.2 |
| A-C | 237.7 | 3.44 | 23.5 | 3.12 | 22.7 |
| B-C | 209.3 | 2.23 | 20.4 | 3.72 | 16.4 |
| B-D | 177.3 | 2.43 | 17.0 | 5.76 | 15.7 |
| C-D | 64.9 | 7.73 | 4.8 | 9.19 | 5.0 |
| Edge | Length [mm] | Prediction [µs] | Simulation [µs] |
|---|---|---|---|
| A-B | 231.3 | 19.1 | 16.40 |
| A-C | 237.7 | 19.7 | 15.90 |
| B-C | 209.3 | 17.1 | 10.90 |
| B-D | 177.3 | 14.2 | 11.70 |
| C-D | 64.9 | 4.1 | 4.10 |
| Edge | Length [mm] | Prediction [µs] | Simulation [µs] |
|---|---|---|---|
| A-B | 231.3 | 8.7 | 9.05 |
| A-C | 237.7 | 8.5 | 8.75 |
| B-C | 209.3 | 7.6 | 6.30 |
| B-D | 177.3 | 6.3 | 6.60 |
| C-D | 64.9 | 1.8 | 2.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinez-Guo, Z. Using Voronoi Tessellation Diagrams to Visualize the Mechanical Response of Interacting Axisymmetric Simultaneous Propagating Waves. Symmetry 2025, 17, 555. https://doi.org/10.3390/sym17040555
Martinez-Guo Z. Using Voronoi Tessellation Diagrams to Visualize the Mechanical Response of Interacting Axisymmetric Simultaneous Propagating Waves. Symmetry. 2025; 17(4):555. https://doi.org/10.3390/sym17040555
Chicago/Turabian StyleMartinez-Guo, Zherui. 2025. "Using Voronoi Tessellation Diagrams to Visualize the Mechanical Response of Interacting Axisymmetric Simultaneous Propagating Waves" Symmetry 17, no. 4: 555. https://doi.org/10.3390/sym17040555
APA StyleMartinez-Guo, Z. (2025). Using Voronoi Tessellation Diagrams to Visualize the Mechanical Response of Interacting Axisymmetric Simultaneous Propagating Waves. Symmetry, 17(4), 555. https://doi.org/10.3390/sym17040555






