Analysis of Near-Polar and Near-Circular Periodic Orbits Around the Moon with J2, C22 and Third-Body Perturbations
Abstract
:1. Introduction
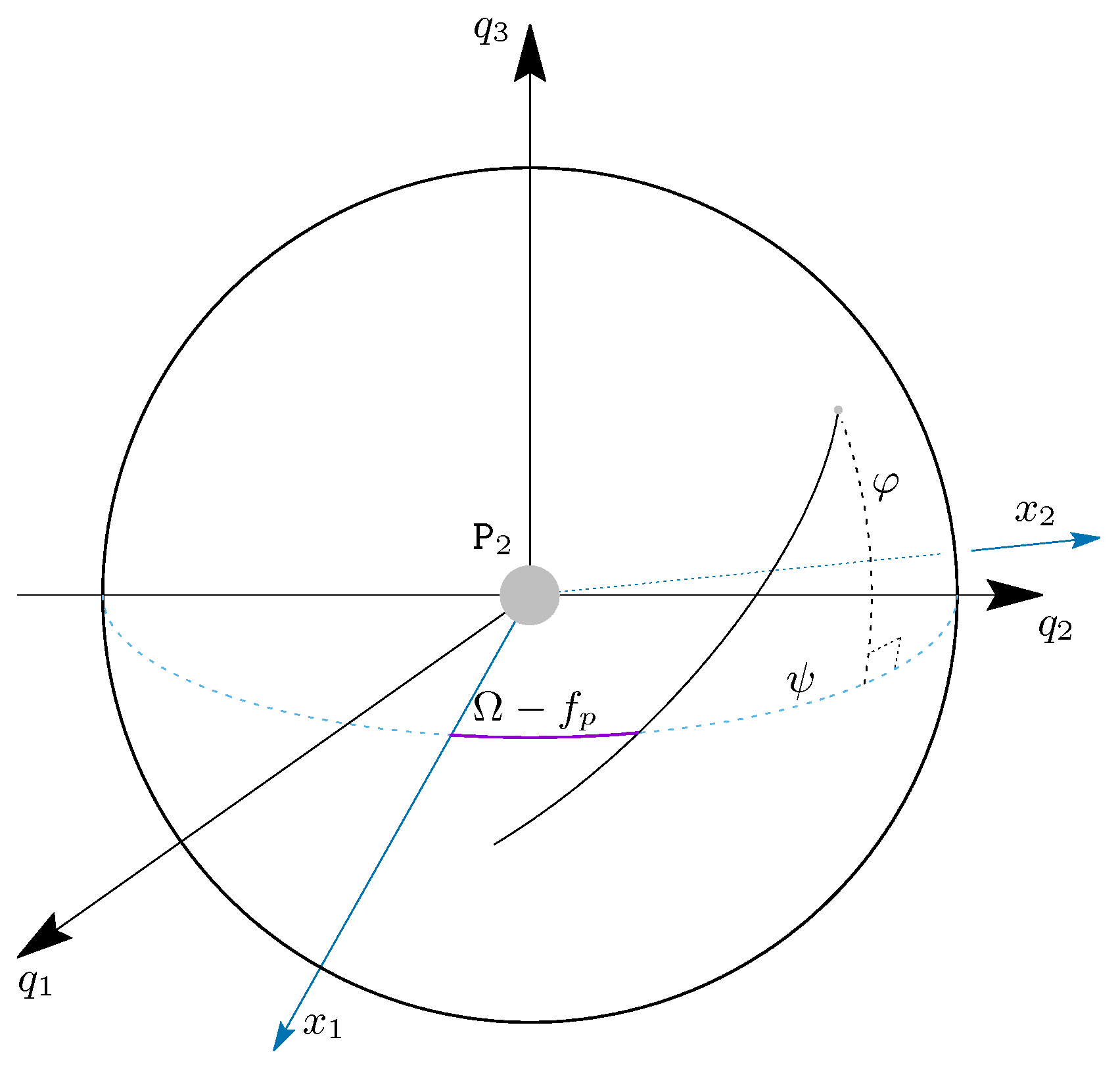
2. Elliptic RTBP
2.1. Scaled Hamiltonian System
2.2. Symmetry and Periodicity
2.3. Some Periodic Orbits
3. Elliptic RTBP with J2, C22 Perturbations
3.1. The Model and Numerical Experiment
3.2. First-Order Averaged System
4. Linear Stability
5. Discussion and Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| RTBP | restricted three-body problem |
References
- Zhang, R.; Wang, Y.; Zhang, H.; Zhang, C. Transfers from distant retrograde orbits to low lunar orbits. Celest. Mech. Dyn. Astr. 2020, 132, 41. [Google Scholar] [CrossRef]
- Guzzetti, D.; Bosanac, N.; Haapala, A.; Howell, K.C.; Folta, D.C. Rapid trajectory design in the Earth–Moon ephemeris system via an interactive catalog of periodic and quasi-periodic orbits. Acta Astronaut. 2016, 126, 439–455. [Google Scholar] [CrossRef]
- Xu, X. Determination of the doubly symmetric periodic orbits in the restricted three-body problem and Hill’s lunar problem. Celest. Mech. Dyn. Astr. 2023, 135, 8. [Google Scholar] [CrossRef]
- Aydin, C.; Batkhin, A. Studying network ofsymmetric periodic orbit families of the Hill problem via symplectic invariants. Celest. Mech. Dyn. Astr. 2025, 137, 12. [Google Scholar] [CrossRef]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes in Fortran 77, the Art of Scientific Computing; Cambridge University Press: New York, NY, USA, 1992. [Google Scholar]
- Xu, X.; Song, Y. Continuation of some nearly circular symmetric periodic orbits in the elliptic restricted three-body problem. Astrophys. Space Sci. 2023, 368, 13. [Google Scholar] [CrossRef]
- Lei, H. A systematic study about orbit flips of test particles caused by eccentric Von Zeipel-Lidov-Kozai effects. Astron. J. 2022, 163, 214. [Google Scholar] [CrossRef]
- Tselousova, A.; Trofimov, S.; Shirobokov, M. Station-keeping in high near-circular polar orbits around the Moon. Acta Astronaut. 2021, 188, 185–192. [Google Scholar] [CrossRef]
- Park, B.; Howell, K.C. Assessment of dynamical models for transitioning from the Circular Restricted Three-Body Problem to an ephemeris model with applications. Celest. Mech. Dyn. Astr. 2024, 136, 6. [Google Scholar] [CrossRef]
- Lara, M.; Ferrer, S.; De Saedeleer, B. Lunar analytical theory for polar orbits in a 50-degree zonal model plus third-body effect. J. Astronaut. Sci. 2009, 57, 561–577. [Google Scholar] [CrossRef]
- Saedeleer, B.D. Analytical theory of a lunar artificial satellite with third body perturbations. Celes. Mech. Dyn. Astron. 2006, 95, 407–423. [Google Scholar] [CrossRef]
- Nie, T.; Gurfil, P. Lunar frozen orbits revisited. Celest. Mech. Dyn. Astr. 2018, 130, 61. [Google Scholar] [CrossRef]
- Carvalho, J.P.S.; de Moraes, R.V.; Prado, A.F.B.A. Some orbital characteristics of lunar artificial satellites. Celest. Mech. Dyn. Astr. 2010, 108, 371–388. [Google Scholar] [CrossRef]
- Tzirti, S.; Tsiganis, K.; Varvoglis, H. Effect of 3rd-degree gravity harmonics and Earth perturbations on lunar artificial satellite orbits. Celest. Mech. Dyn. Astr. 2010, 108, 389–404. [Google Scholar] [CrossRef]
- Tzirti, S.; Noullez, A.; Tsiganis, K. Secular dynamics of a lunar orbiter: A global exploration using Prony’s frequency analysis. Celest. Mech. Dyn. Astr. 2014, 118, 379–397. [Google Scholar] [CrossRef]
- El-Salam, F.A.A.; El-Bar, S.E.A. Families of frozen orbits of lunar artificial satellites. Appl. Math. Model. 2016, 40, 9739–9753. [Google Scholar] [CrossRef]
- Sirwah, M.A.; Tarek, D.; Radwan, M.; Ibrahim, A.H. A study of the moderate altitude frozen orbits around the Moon. Results Phys. 2020, 17, 103148. [Google Scholar] [CrossRef]
- Dena, Á.; Abad, A.; Barrio, R. Efficient computational approaches to obtain periodic orbits in Hamiltonian systems: Application to the motion of a lunar orbiter. Celest. Mech. Dyn. Astr. 2016, 124, 51–71. [Google Scholar] [CrossRef]
- Franz, C.J.; Russell, R.P. Database of Planar and Three-Dimensional Periodic Orbits and Families Near the Moon. J. Astronaut. Sci. 2022, 69, 1573–1612. [Google Scholar] [CrossRef]
- Legnaro, E.; Efthymiopoulos, C. Secular dynamics and the lifetimes of lunar artificial satellites under natural force-driven orbital evolution. Acta Astronaut. 2024, 225, 768–787. [Google Scholar] [CrossRef]
- Cors, J.M.; Pinyol, C.; Soler, J. Analytic continuation in the case of non-regular dependency on a small parameter with an application to celestial mechanics. J. Differ. Equ. 2005, 219, 1–19. [Google Scholar] [CrossRef]
- Villegas-Pinto, D.; Baresi, N.; Locoche, S.; Hestroffer, D. Resonant quasi-periodic near-rectilinear Halo orbits in the Elliptic-Circular Earth-Moon-Sun Problem. Adv. Space Res. 2023, 71, 336–354. [Google Scholar] [CrossRef]
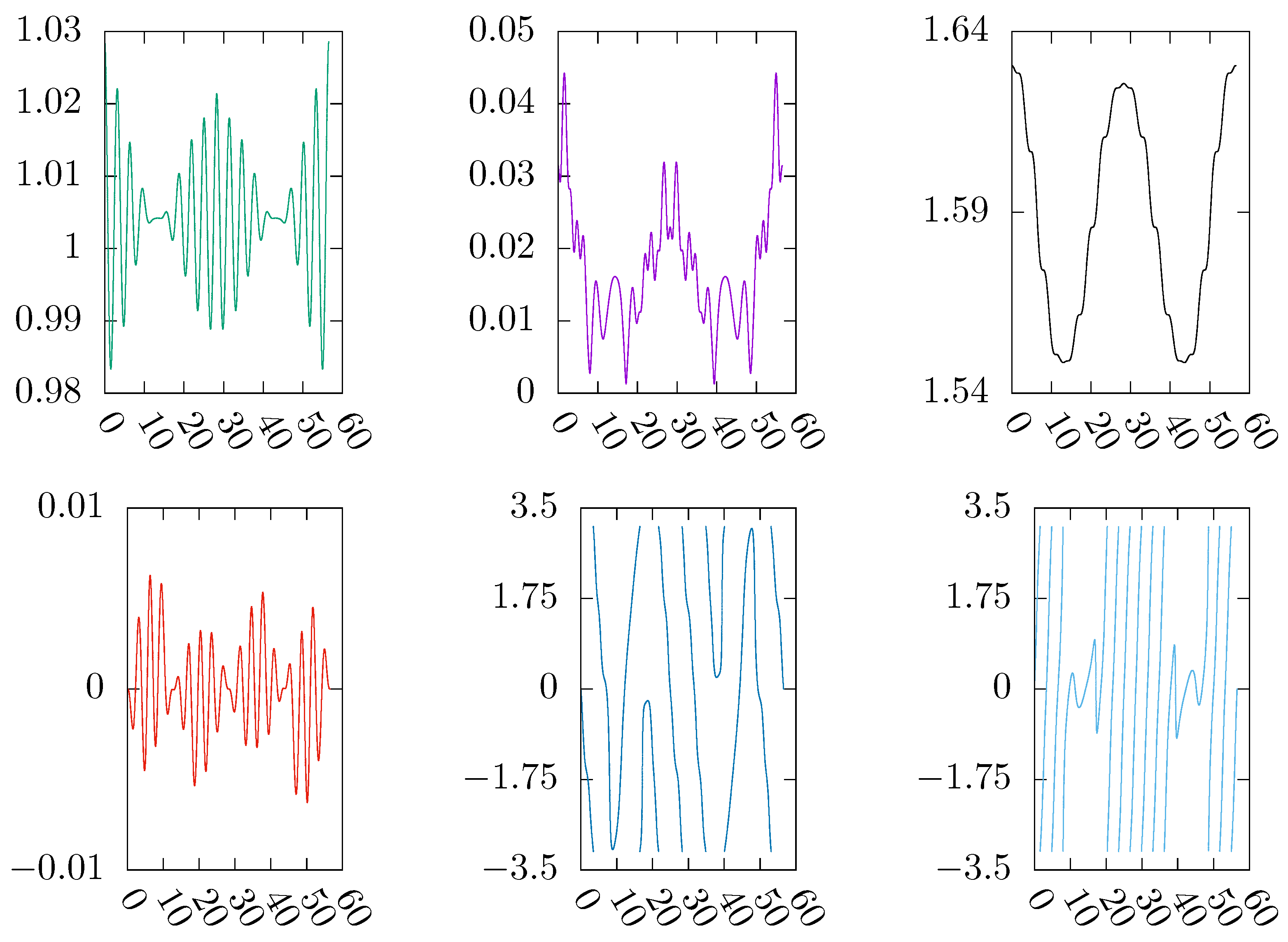


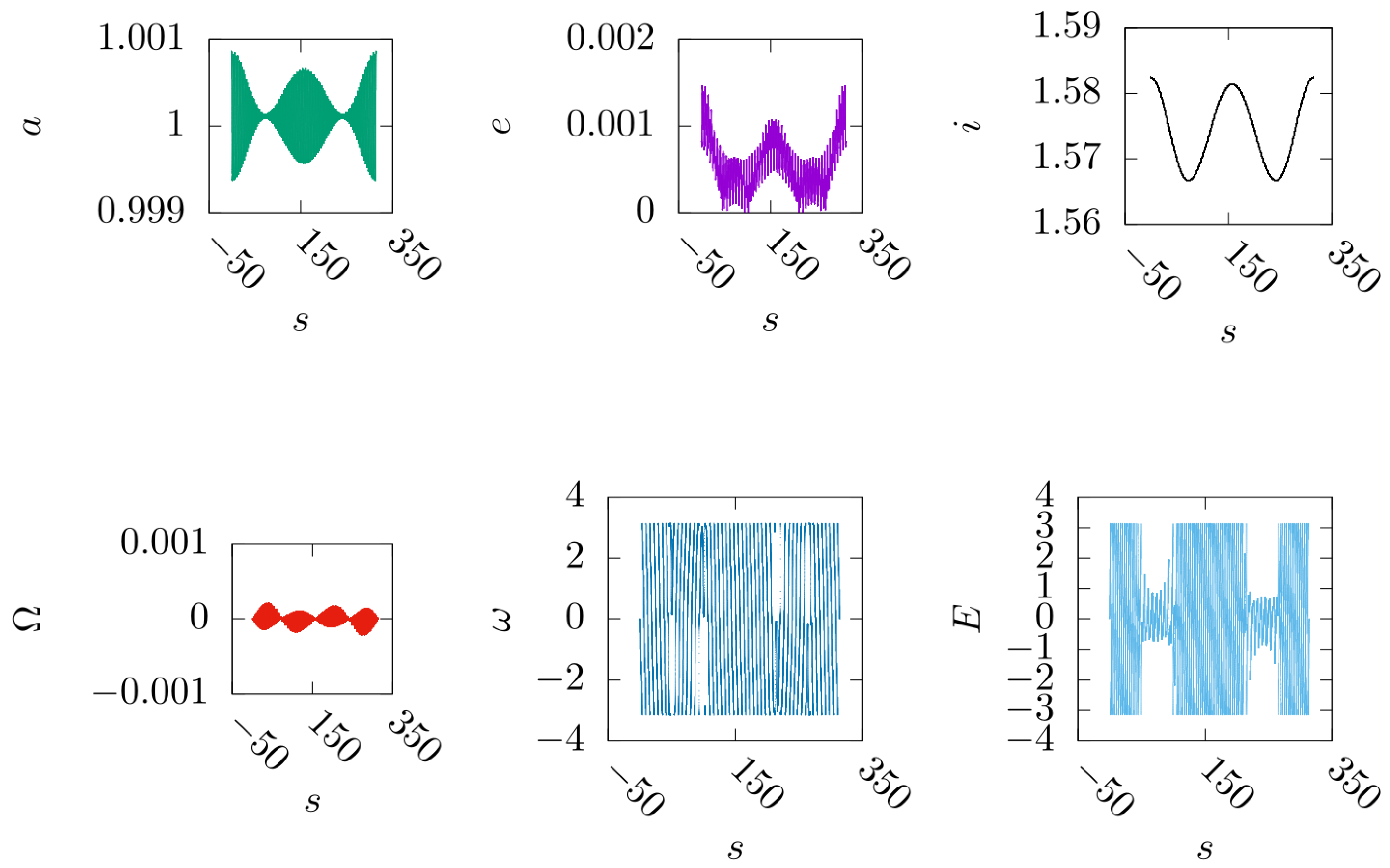
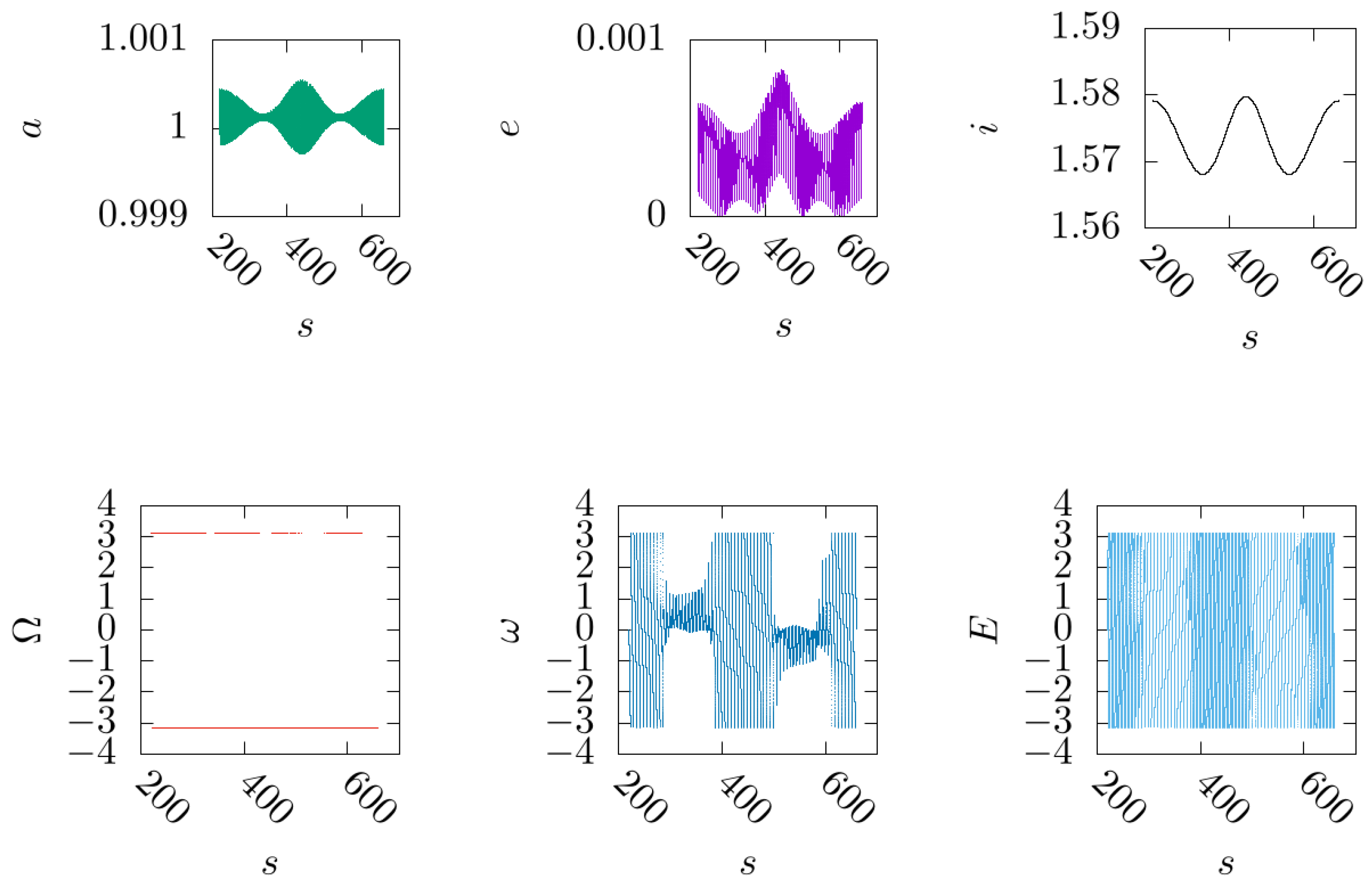
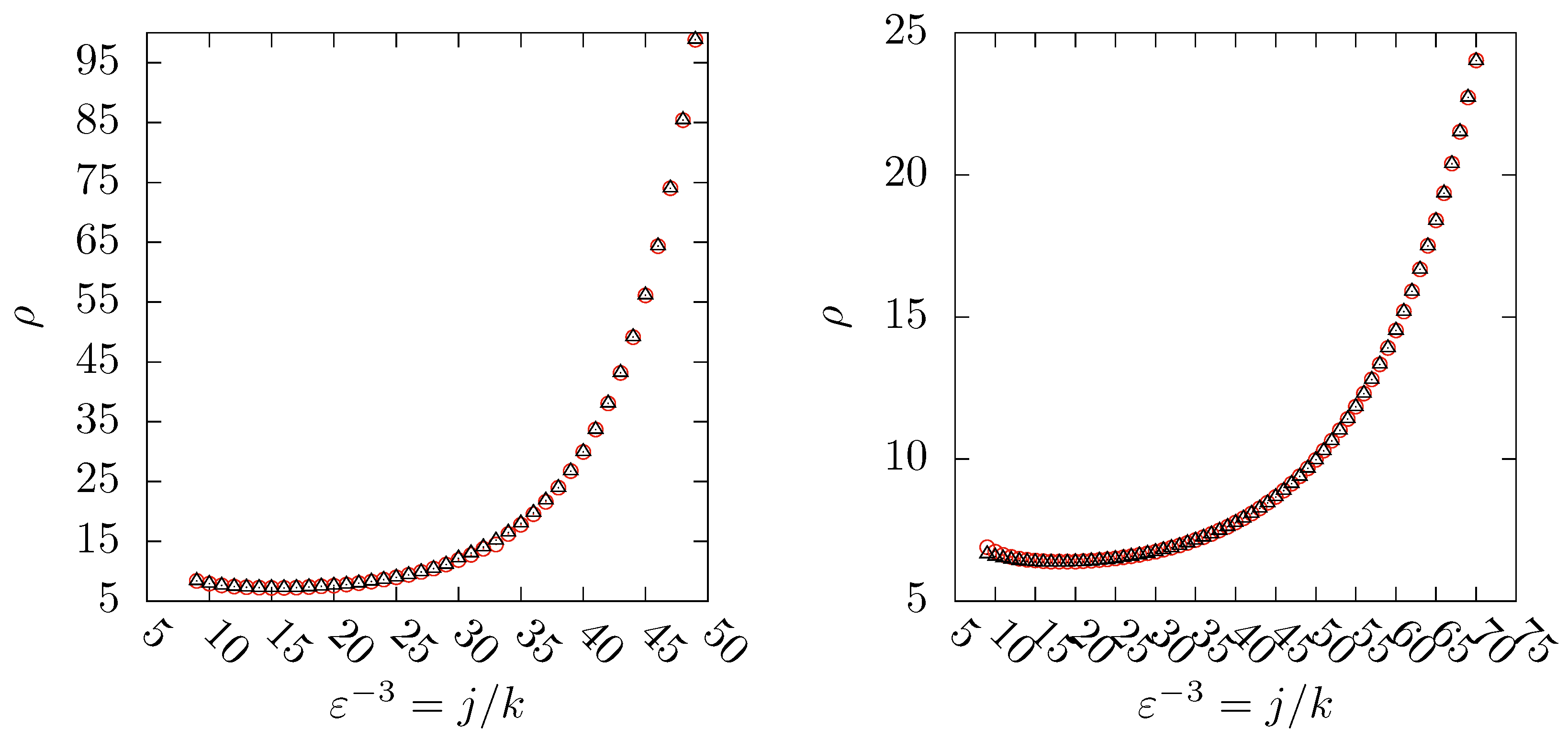
| , Type | |||
|---|---|---|---|
| 9/1, | 0.99620440178 | −0.06082772318 | ±1.0157184687 |
| 9/1, | −0.99470649817 | 0.06185840160 | ±1.0154002218 |
| 10/1, | 0.99910153226 | −0.050852737 | ±1.0072154827 |
| 10/1, | −0.99837950690 | 0.0506041258 | ±1.0087412525 |
| 16/1, | 0.99925242695 | −0.035922494 | ±1.0043641526 |
| 16/1, | −0.99851083910 | 0.0360488668 | ±1.0047376373 |
| 36/1, | 1.00010727626 | −0.0146998968 | ±1.0003869346 |
| 36/1, | −0.99974967250 | 0.0147026686 | ±1.0007698831 |
| 37/1, | 0.99999430950 | −0.0157684742 | ±1.0006628074 |
| 37/1, | −0.99962561652 | 0.0157786232 | ±1.0009929915 |
| 50/1, | 1.0000454998 | −1.16852281 | ±1.0003121582 |
| 50/1, | −0.999748470093 | 1.169015346 | ±1.000591975 |
| 50/1, | 1.00010809345 | −1.05990235 | ±1.00014917507 |
| 50/1, | −0.99981879968 | 1.060132171 | ±1.00044900515 |
| 120/1, | 1.000063875 | −4.87551 | ±0.999997510 |
| 120/1, | −0.9999007299785 | 4.8763318183 | −1.000158994073 |
| 150/1, | 1.00005889302967 | −3.900799586228 | 0.99998031895716 |
| 150/1, | −0.99991304641419 | 3.225120713516 | −1.0001276778590 |
| , Type 1 | |||
|---|---|---|---|
| 38, | 0.999996415501457 | −1.53265760125584 | 1.00063234441343 |
| 38, | 0.999996323959347 | −1.53265221246967 | −1.00063243652928 |
| 38, | −0.999631537537576 | 1.53360715507054 | 1.00096137315743 |
| 38, | −0.999631572957102 | 1.53361607836104 | −1.00096133644985 |
| 38, | 1.00010457611908 | −1.39262375517334 | 1.00034505669525 |
| 38, | 1.00010457613606 | −1.39262375145222 | −1.00034505667884 |
| 38, | −0.999755336066306 | 1.39291300677261 | 1.00071623453464 |
| 38, | −0.999755336071655 | 1.39291298616095 | −1.00071623453208 |
| 50, | 1.00004036237187 | −1.16399095055137 | 1.00032604091855 |
| 50, | 1.00004036442821 | −1.16399055608615 | −1.00032603891154 |
| 50, | −0.999736185844210 | 1.16448719424375 | 1.00061301131761 |
| 50, | −0.999736185751775 | 1.16448718613465 | −1.00061301141084 |
| 50, | 1.00010324877502 | −1.06014270499141 | 1.00016221064751 |
| 50, | 1.00010324742691 | −1.06015693670615 | −1.00016221050752 |
| 50, | −0.999806261225514 | 1.06039245231929 | 1.00046973982848 |
| 50, | −0.999806260045887 | 1.06039241923682 | −1.00046974101071 |
| 50, | 1.00004772495397 | −1.16437280686876 | 1.00031865811095 |
| 50, | −0.999727871097841 | 1.16482584577867 | 1.00062129340388 |
| 50, | −0.999727871210650 | 1.16482585774023 | −1.0006212932898344 |
| 50, | 1.000047724953971 | −1.16437280686877 | 1.00031865811095 |
| 50, | 1.000047723820043 | −1.16437303795379 | −1.00031865921609 |
| 60, | 1.00005445614547 | −9.69443727684011 | 1.00020411562384 |
| 60, | 1.00005445666738 | −9.69443733030811 | −1.00020411510206 |
| 60, | −0.999780858404711 | 9.69780610332384 | 1.00046714423176 |
| 60, | −0.999780830682264 | 9.69781696395454 | −1.00046717183446 |
| 60, | 1.00009836808750 | −8.86151811506304 | 1.00009091857796 |
| 60, | 1.00009836626972 | −8.86152667714532 | −1.00009092031881 |
| 60, | −0.999829057478298 | 8.86344960938257 | 1.00036671317776 |
| 60, | −0.999829058314773 | 8.86344660447464 | −1.00036671236851 |
| 70, | 1.00006122089056 | −8.32258582949308 | 1.00013342805500 |
| 70, | 1.00006122070046 | −8.32258595263936 | −1.00013342824388 |
| 70, | −0.999807148471916 | 8.32518964348363 | 1.00038049962717 |
| 70, | −0.999807149512763 | 8.32518602724036 | −1.00038049861647 |
| 70, | 1.00009370579491 | −7.65010043854636 | 1.00005034663127 |
| 70, | 1.00009370571585 | −7.65010142502368 | −1.00005034670266 |
| 70, | −0.999842137241648 | 7.65183065190509 | 1.00030621538970 |
| 70, | −0.999842137612930 | 7.65182240120627 | −1.00030621508136 |
| 70, | −0.99980224406757 | 8.326726523174 | 1.000385394761 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, X. Analysis of Near-Polar and Near-Circular Periodic Orbits Around the Moon with J2, C22 and Third-Body Perturbations. Symmetry 2025, 17, 630. https://doi.org/10.3390/sym17050630
Xu X. Analysis of Near-Polar and Near-Circular Periodic Orbits Around the Moon with J2, C22 and Third-Body Perturbations. Symmetry. 2025; 17(5):630. https://doi.org/10.3390/sym17050630
Chicago/Turabian StyleXu, Xingbo. 2025. "Analysis of Near-Polar and Near-Circular Periodic Orbits Around the Moon with J2, C22 and Third-Body Perturbations" Symmetry 17, no. 5: 630. https://doi.org/10.3390/sym17050630
APA StyleXu, X. (2025). Analysis of Near-Polar and Near-Circular Periodic Orbits Around the Moon with J2, C22 and Third-Body Perturbations. Symmetry, 17(5), 630. https://doi.org/10.3390/sym17050630





