Effect of Mineral Composition on Transverse Relaxation Time Distributions and MR Imaging of Tight Rocks from Offshore Ireland
Abstract
:1. Introduction
2. Materials and Methods
2.1. Lithological Overview
2.2. Core Plug Characterization
2.3. Core Plug Preparation
2.4. NMR/MRI Characterization
3. Results and Discussion
3.1. Mineralogical Composition
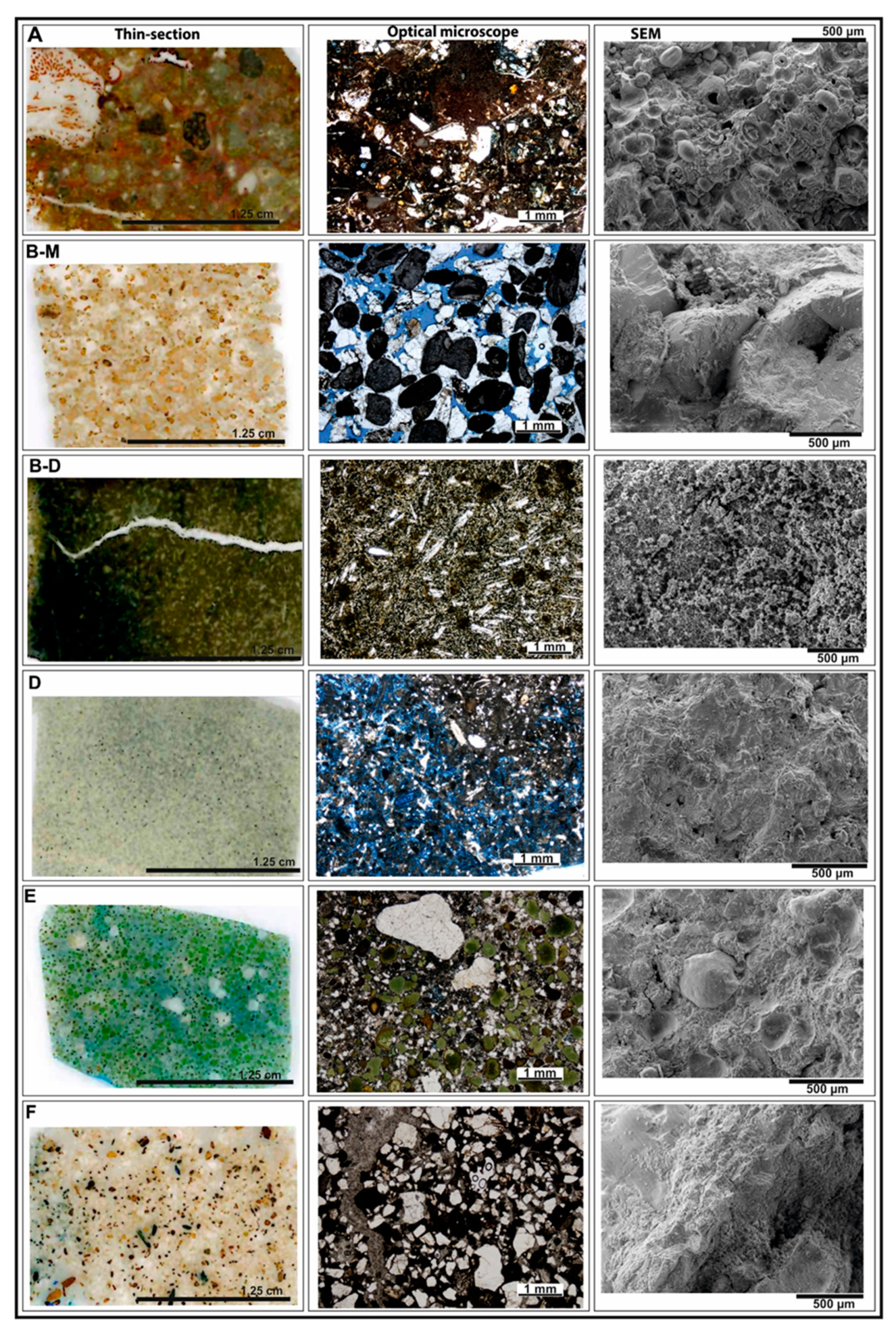
3.1.1. Core Plug A—Volcanic Tuff
3.1.2. Core Plug B-M—Lithic-Rich Sandstone
3.1.3. Core Plug B-D—Basalt
3.1.4. Core Plug D—Siltstone
3.1.5. Core Plug E—Upper Cretaceous Sandstone
3.1.6. Core Plug F—Mid-Cretaceous Sandstone
3.2. NMR/MRI Characterization
3.3. Effect of Magnetic Field Strength
3.3.1. Core Plug A
3.3.2. Core Plug BM
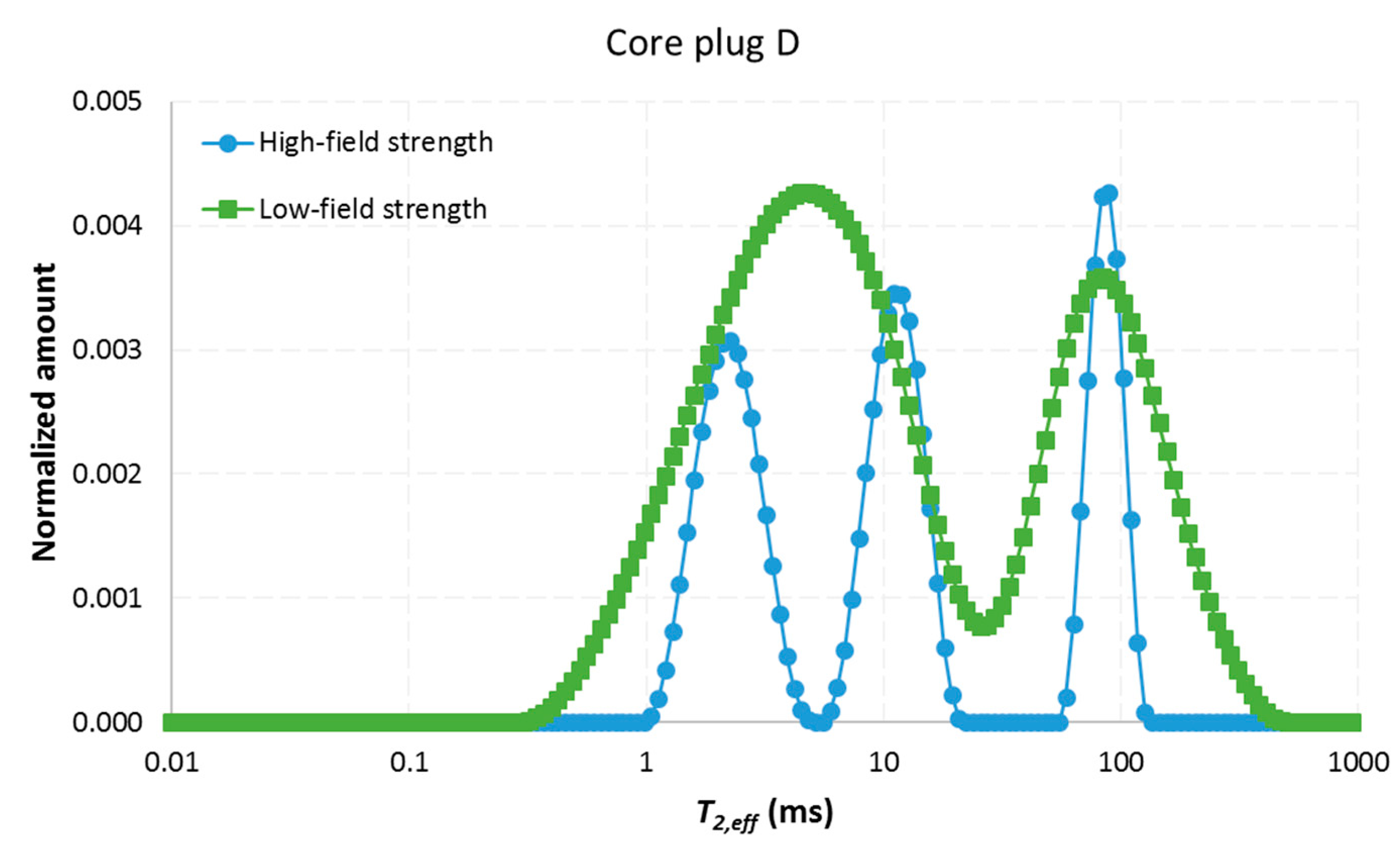
3.3.3. Core Plug D
3.3.4. Core Plug E
3.3.5. Core Plug F
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fheed, A.; Krzyzak, A.; Swierczewska, A. Exploring a carbonate reef reservoir—Nuclear magnetic resonance and computed microtomography confronted with narrow channel and fracture porosity. J. Appl. Geophys. 2018, 151, 343–358. [Google Scholar] [CrossRef]
- Berg, S.; Armstrong, R.; Ott, H.; Georgiadis, A.; Klapp, S.A.; Schwing, A.; Neiteler, R.; Brussee, N.; Makurat, A.; Leu, L.; et al. Multiphase Flow in Porous Rock Imaged Under Dynamic Flow Conditions with Fast X-Ray Computed Microtomography. Petrophysics 2014, 55, 304–312. [Google Scholar]
- Cnudde, V.; Boone, M. High-resolution X-ray computed tomography in geosciences: A review of the current technology and applications. Earth-Sci. Rev. 2013, 123, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Mansfield, P.; Issa, B. Fluid Transport in Porous Rocks. I. EPI Studies and a Stochastic Model of Flow. J. Magn. Reson. Ser. A 1996, 122, 137–148. [Google Scholar] [CrossRef]
- Oswald, S.E.; Spiegel, M.A.; Kinzelbach, W. Three-dimensional saltwater–freshwater fingering in porous media: Contrast agent MRI as basis for numerical simulations. Magn. Reson. Imaging 2007, 25, 537–540. [Google Scholar] [CrossRef]
- Baldwin, B.A.; Stevens, J.; Howard, J.J.; Graue, A.; Kvamme, B.; Aspenes, E.; Ersland, G.; Husebø, J.; Zornes, D.R. Using magnetic resonance imaging to monitor CH4 hydrate formation and spontaneous conversion of CH4 hydrate to CO2 hydrate in porous media. Magn. Reson. Imaging 2009, 27, 720–726. [Google Scholar] [CrossRef]
- Li, M.; Romero-Zerón, L.; Marica, F.; Balcom, B.J. Polymer Flooding Enhanced Oil Recovery Evaluated with Magnetic Resonance Imaging and Relaxation Time Measurements. Energy Fuels 2017, 31, 4904–4914. [Google Scholar] [CrossRef]
- Kleinhans, M.G.; Jeukens, C.R.L.P.N.; Bakker, C.J.G.; Frings, R.M. Magnetic Resonance Imaging of coarse sediment. Sediment. Geol. 2008, 208, 69–78. [Google Scholar] [CrossRef]
- Gingras, M.K.; Macmillan, B.; Balcom, B.J.; Saunders, T.; Pemberton, S.G. Using Magnetic Resonance Imaging and Petrographic Techniques to Understand the Textural Attributes and Porosity Distribution in Macaronichnus-Burrowed Sandstone. J. Sediment. Res. 2002, 72, 552–558. [Google Scholar] [CrossRef]
- Al-Muthana, A.S.; Hursan, G.G.; Mark Ma, S.; Valori, A.; Nicot, B.; Singer, P.M. Wettability as a function of pore size by Nmr. In Proceedings of the International Symposium of the Society of Core Analysts, Aberdeen, UK, 27–30 August 2012. [Google Scholar]
- Arnold, J.; Clauser, C.; Pechnig, R.; Anferova, C.; Anferov, V.; Blümich, B. Porosity and permeability from mobile NMR core-scanning. Petrophysics 2006, 47. [Google Scholar]
- Lewis, R.T.; Seland, J.G. A multi-dimensional experiment for characterization of pore structure heterogeneity using NMR. J. Magn. Reson. 2016, 263, 19–32. [Google Scholar] [CrossRef] [PubMed]
- Lewis, R.T.; Seland, J.G. Characterization of pore geometry using correlations between magnetic field and internal gradient. Microporous Mesoporous Mater. 2018, 269, 31–34. [Google Scholar] [CrossRef]
- Lyu, C.; Ning, Z.; Wang, Q.; Chen, M. Application of NMR T2 to Pore Size Distribution and Movable Fluid Distribution in Tight Sandstones. Energy Fuels 2018, 32, 1395–1405. [Google Scholar] [CrossRef]
- Yan, J.; He, X.; Zhang, S.; Feng, C.; Wang, J.; Hu, Q.; Cai, J.; Wang, M. Sensitive parameters of NMR T2 spectrum and their application to pore structure characterization and evaluation in logging profile: A case study from Chang 7 in the Yanchang Formation, Heshui area, Ordos Basin, NW China. Mar. Pet. Geol. 2020, 111, 230–239. [Google Scholar] [CrossRef]
- Mitchell, J.; Chandrasekera, T.C.; Holland, D.J.; Gladden, L.F.; Fordham, E.J. Magnetic resonance imaging in laboratory petrophysical core analysis. Phys. Rep. 2013, 526, 165–225. [Google Scholar] [CrossRef]
- Washburn, K.E.; Eccles, C.D.; Callaghan, P.T. The dependence on magnetic field strength of correlated internal gradient relaxation time distributions in heterogeneous materials. J. Magn. Reson. 2008, 194, 33–40. [Google Scholar] [CrossRef]
- Hürlimann, M.D. Effective Gradients in Porous Media Due to Susceptibility Differences. J. Magn. Reson. 1998, 131, 232–240. [Google Scholar] [CrossRef]
- Mitchell, J.; Chandrasekera, T.C.; Johns, M.L.; Gladden, L.F.; Fordham, E.J. Nuclear magnetic resonance relaxation and diffusion in the presence of internal gradients: The effect of magnetic field strength. Phys. Rev. E 2010, 81, 026101. [Google Scholar] [CrossRef]
- Foley, I.; Farooqui, S.A.; Kleinberg, R.L. Effect of Paramagnetic Ions on NMR Relaxation of Fluids at Solid Surfaces. J. Magn. Reson. Ser. A 1996, 123, 95–104. [Google Scholar] [CrossRef]
- Haughton, P.; Praeg, D.; Shannon, P.; Harrington, G.; Higgs, K.; Amy, L.; Tyrrell, S.; Morrissey, T. First results from shallow stratigraphic boreholes on the eastern flank of the Rockall Basin, offshore western Ireland. Geol. Soc. Lond. Pet. Geol. Conf. Ser. 2005, 6, 1077–1094. [Google Scholar] [CrossRef]
- Carr, H.Y.; Purcell, E.M. Effects of Diffusion on Free Precession in Nuclear Magnetic Resonance Experiments. Phys. Rev. 1954, 94, 630–638. [Google Scholar] [CrossRef]
- Meiboom, S.; Gill, D. Modified Spin-Echo Method for Measuring Nuclear Relaxation Times. Rev. Sci. Instrum. 1958, 29, 688–691. [Google Scholar] [CrossRef] [Green Version]
- Song, Y.Q.; Venkataramanan, L.; Hürlimann, M.D.; Flaum, M.; Frulla, P.; Straley, C. T1–T2 Correlation Spectra Obtained Using a Fast Two-Dimensional Laplace Inversion. J. Magn. Reson. 2002, 154, 261–268. [Google Scholar] [CrossRef] [PubMed]
- Mitchell, J. Magnetic resonance core analysis AT 0.3 T. In Proceedings of the International Symposium of the Society of Core Analysts, Avignon, France, 8–11 September 2014. [Google Scholar]
- Mastrogiacomo, S.; Dou, W.; Jansen, J.A.; Walboomers, X.F. Magnetic Resonance Imaging of Hard Tissues and Hard Tissue Engineered Bio-substitutes. Mol. Imaging Biol. 2019, 21, 1003–1019. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Weglarz, W.P.; Krzyzak, A.; Machowski, G.; Stefaniuk, M. ZTE MRI in high magnetic field as a time effective 3D imaging technique for monitoring water ingress in porous rocks at sub-millimetre resolution. Magn. Reson. Imaging 2018, 47, 54–59. [Google Scholar] [CrossRef] [PubMed]










| Sample ID | Borehole Name | Depth of Sample | Age and Description |
|---|---|---|---|
| A | Bucentaur 11/20-sb01 | 8.4 m (bsf) | Paleogene: yellowish-red to brown tuff, lithic and crystal fragments, scattered dark-gray basalt clasts, vesicular with zeolites, glass commonly altered to Palagonite. Foraminifera and bivalve shell fragments are scattered throughout the interval. |
| B-M | Bucentaur 16/28-sb01 | 145 m (bsf) | L. Eocene: interbedded mudstone and siltstone: greenish gray, firm to hard, commonly massive, bioturbated, sporadic fractures, rare shell fragments, glauconite. |
| B-D | Bucentaur 16/28-sb01 | 147.7 m (bsf) | U. Cretaceous: basalt: black, fractures with calcite veins, highly altered. Top is microphyric with flow-banded plagioclase laths, remainder is fine-grained. |
| D | Bucentaur 16/28-sb01 | 145.1 m (bsf) | L. Eocene: mostly siltstone (interbedded mudstone and siltstone): greenish gray, firm to hard, commonly massive, bioturbated, sporadic fractures, rare shell fragments, glauconite. |
| E | Bucentaur 83/20-sb01 | 112.5 m (bsf) | U. Cretaceous: sandstone: dark greenish gray, fine to coarse, poorly sorted, soft to hard, glauconite, carbonate cemented, locally shelly. |
| F | Bucentaur 83/20-sb01 | 138 m (bsf) | M. Cretaceous: sandstone: yellowish brown, fine to very coarse, poorly sorted, brown glauconite, frosted quartz, shell debris, carbonate cement, thin limestone. |
| Core Plug | Length | Diameter | Φ (frac.) | |
|---|---|---|---|---|
| (cm) | (cm) | Weight Calculation | Low-Field Strength | |
| ±0.01 | B0 = 0.28 T | |||
| A | 2.97 | 2.66 | 0.34 | 0.33 |
| BD | 3.27 | 2.68 | 0.09 | - |
| BM | 2.23 | 2.63 | 0.14 | 0.16 |
| D | 3.30 | 2.66 | 0.22 | 0.23 |
| E | 3.19 | 2.66 | 0.13 | 0.17 |
| F | 3.26 | 2.64 | 0.06 | 0.06 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almenningen, S.; Roy, S.; Hussain, A.; Seland, J.G.; Ersland, G. Effect of Mineral Composition on Transverse Relaxation Time Distributions and MR Imaging of Tight Rocks from Offshore Ireland. Minerals 2020, 10, 232. https://doi.org/10.3390/min10030232
Almenningen S, Roy S, Hussain A, Seland JG, Ersland G. Effect of Mineral Composition on Transverse Relaxation Time Distributions and MR Imaging of Tight Rocks from Offshore Ireland. Minerals. 2020; 10(3):232. https://doi.org/10.3390/min10030232
Chicago/Turabian StyleAlmenningen, Stian, Srikumar Roy, Arif Hussain, John Georg Seland, and Geir Ersland. 2020. "Effect of Mineral Composition on Transverse Relaxation Time Distributions and MR Imaging of Tight Rocks from Offshore Ireland" Minerals 10, no. 3: 232. https://doi.org/10.3390/min10030232
APA StyleAlmenningen, S., Roy, S., Hussain, A., Seland, J. G., & Ersland, G. (2020). Effect of Mineral Composition on Transverse Relaxation Time Distributions and MR Imaging of Tight Rocks from Offshore Ireland. Minerals, 10(3), 232. https://doi.org/10.3390/min10030232






