Design of Finite Difference Method and Neural Network Approach for Casson Nanofluid Flow: A Computational Study
Abstract
1. Introduction
- To resolve first-order linear and non-linear ordinary differential equations (ODEs), the third-order numerical technique has been put forward as a potential solution in two stages.
- The construction of a computational numerical scheme is considered to solve the proposed mathematical model of the heat and mass transfer of non-Newtonian Casson nanofluid flow.
- The proposed numerical scheme is highly accurate and attains the predicted order of convergence shown through various examples.
- To verify the scheme’s efficacy, a couple of non-linear examples and a few real-life problems can be solved.
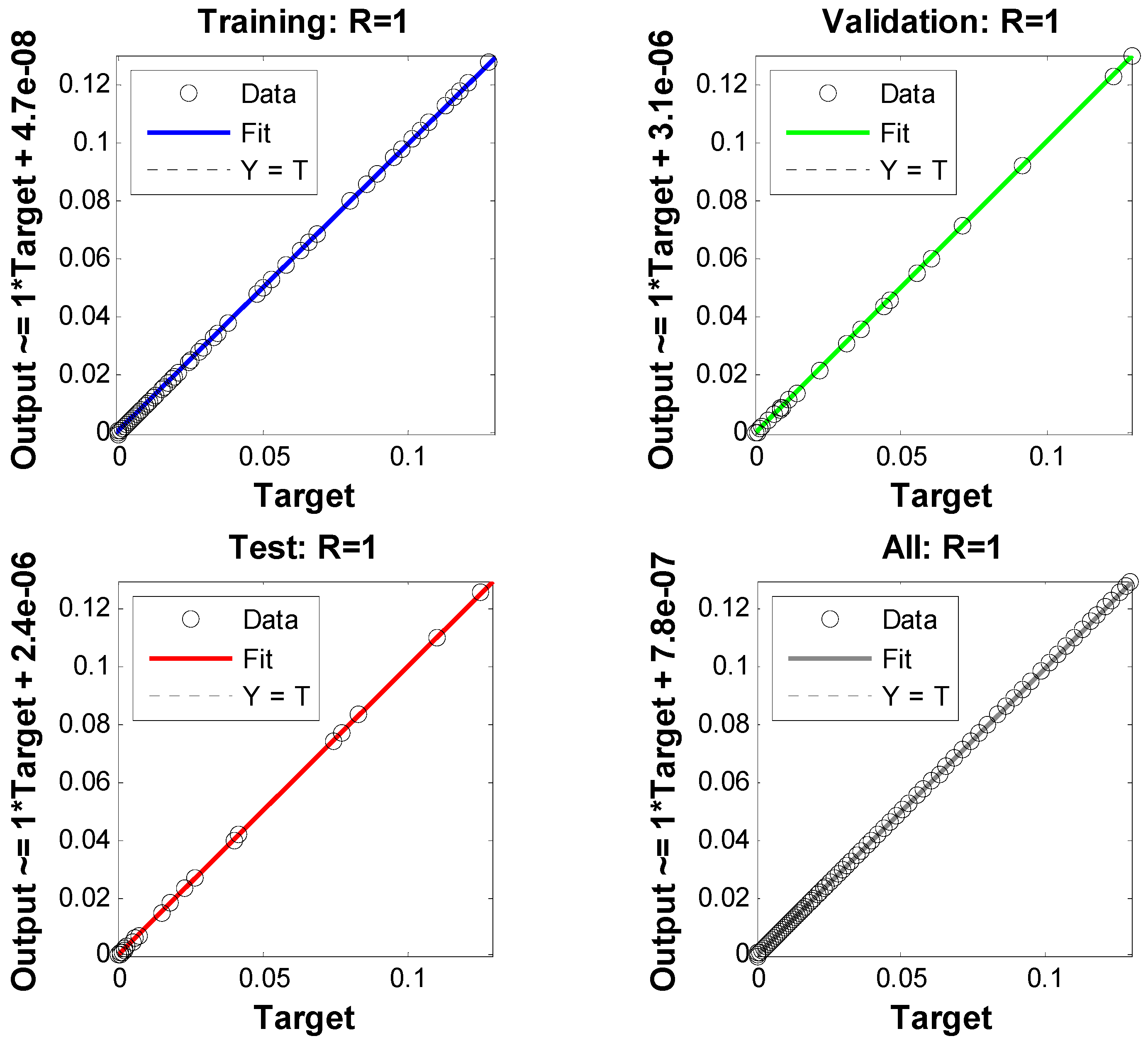
- The mathematical model of heat and mass transfer of non-Newtonian Casson nanofluid flow is given under the induced magnetic field’s effects. Its numerical performance is provided through stochastic processes based on Levenberg–Marquardt backpropagation artificial neural networks.
- Accuracy evaluations, histograms, and regression analysis for the fluid flow model are provided in sufficient graphical and numerical detail to validate and verify the Levenberg–Marquardt backpropagation technique.
2. Numerical Scheme
3. Stability Analysis
4. Consistency of the Scheme
5. Problem Formulation
6. Results and Discussions
7. Conclusions
- The proposed scheme was third-order accurate in two stages.
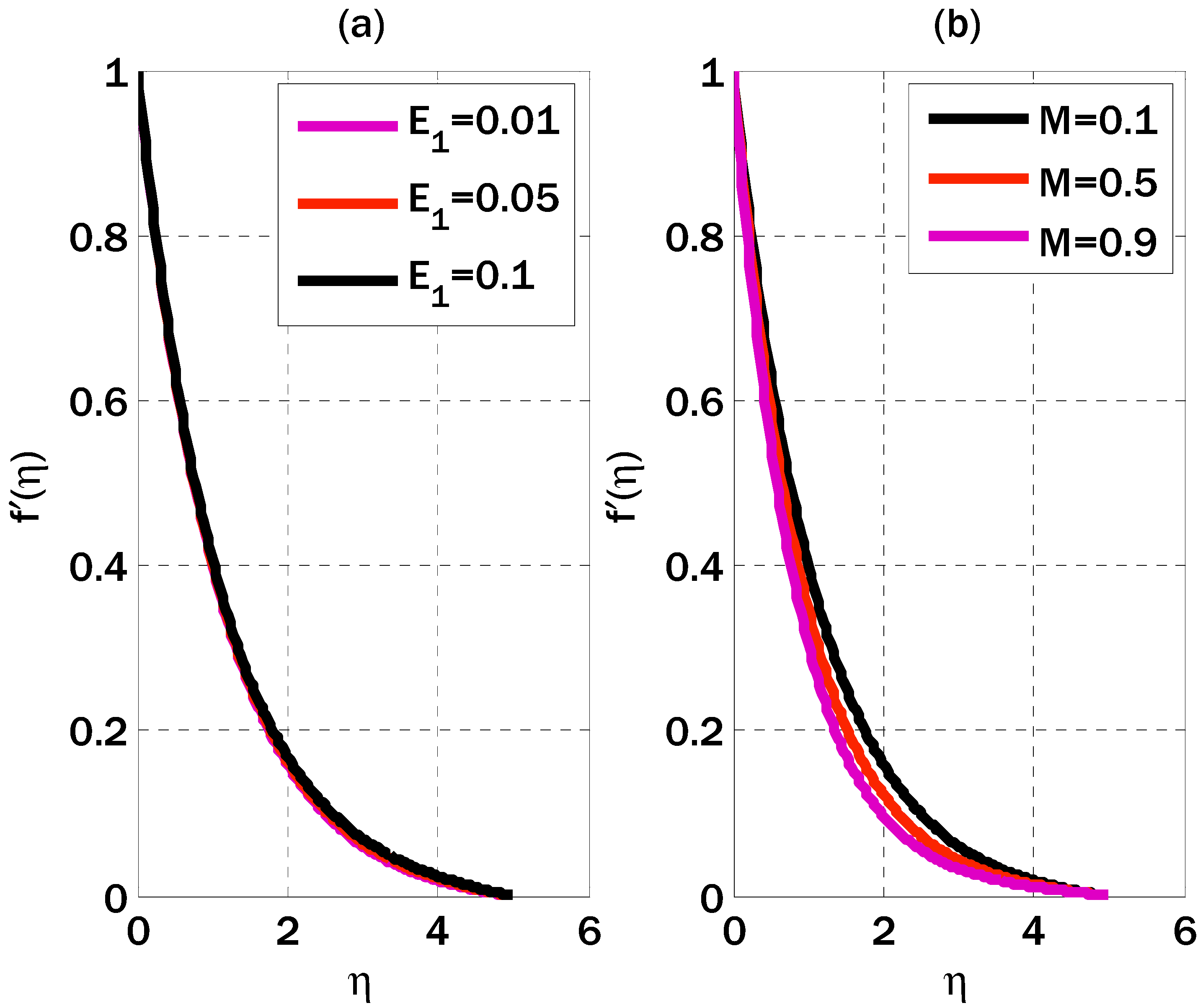
- As the Casson parameter increased, the velocity profile slowed down, and as the magnetic parameter increased, it displayed a dual behavior.
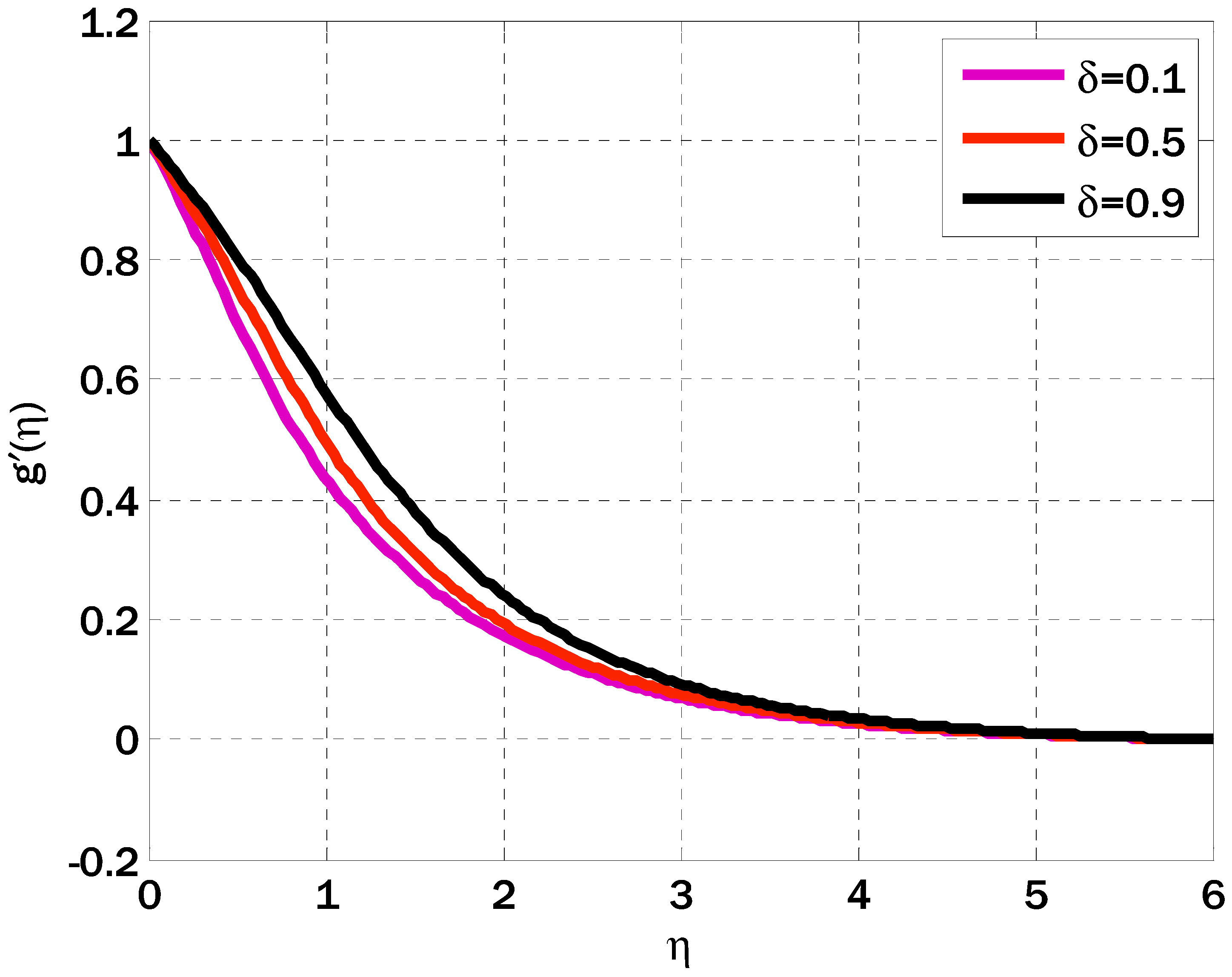
- Growing values of the reciprocal of the magnetic Prandtl number raised the horizontal component of the induced magnetic field.
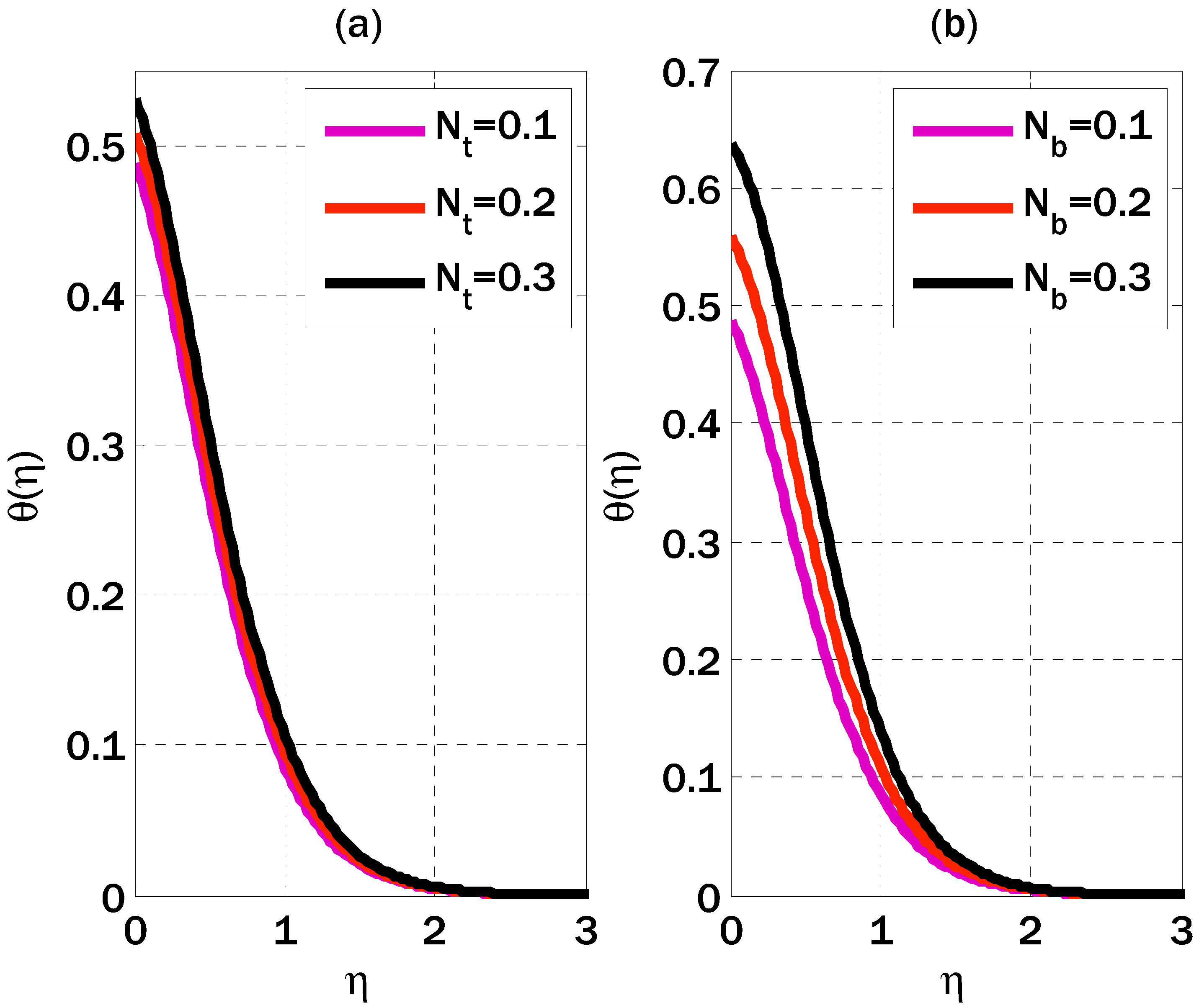
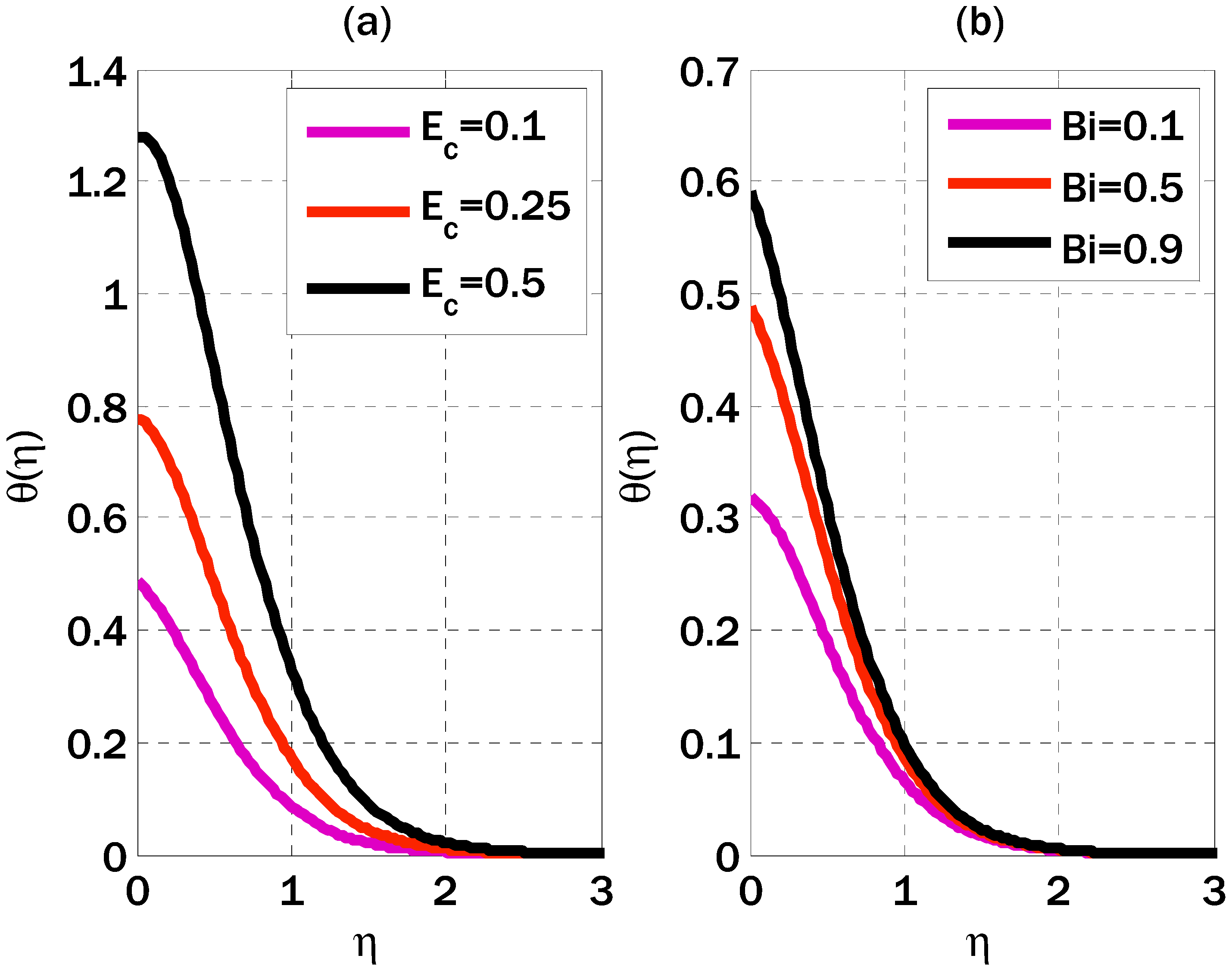
- Three models representing velocity, temperature, and concentration profiles were implemented using a neural network approach.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Horizontal components of velocity | Electrical conductivity of the fluid | ||
| Cartesian co-ordinate | Temperature of fluid | ||
| Kinematic viscosity | Temperature of fluid at the wall | ||
| Density of fluid | Ambient temperature of the fluid | ||
| Concentration of fluid | Concentration on the wall | ||
| Brownian diffusion coefficient | Ambient concentration | ||
| Specific heat capacity | Thermophoresis coefficient | ||
| Horizontal component of induced magnetic field | Vertical component of induced magnetic field | ||
| Reaction rate | Thermal diffusivity | ||
| Reaction rate parameter | Dynamic viscosity | ||
| Time constant (s) | Effective heat capacity of fluid | ||
| Magnetic diffusivity | Eckert number | ||
| Prandtl number | Thermophoresis variable | ||
| Brownian motion variable | Schmidt number | ||
| Magnetic parameter | Weissenberg number | ||
| Reciprocal of magnetic Prandtl number | Magnetic parameter | ||
| local electric parameter | Biot number |
References
- Casson, N. A flow equation for pigment-oil suspensions of the printing ink type. In Rheology of Disperse Systems; Mill, C.C., Ed.; Pergamon Press: Oxford, UK, 1959; pp. 84–104. [Google Scholar]
- Mustafa, M.; Hayat, T.; Ioan, P.; Hendi, A. Stagnation-point flow and heat transfer of a Casson fluid towards a stretching sheet. Z. Für Nat. A 2012, 67, 70–76. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. Casson fluid flow and heat transfer over a nonlinearly stretching surface. Chin. Phys. B 2013, 22, 074701. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Mondal, I.C.; Chamkha, A.J. Casson fluid flow and heat transfer past a symmetric wedge. Heat Transf. Asian Res. 2013, 42, 665–675. [Google Scholar] [CrossRef]
- Pramanik, S. Casson fluid flow and heat transfer past an exponentially porous stretching surface in presence of thermal radiation. Ain Shams Eng. J. 2014, 5, 205–212. [Google Scholar] [CrossRef]
- Mahdy, A. Heat transfer and flow of a Casson fluid due to a stretching cylinder with the Soret and Dufour effects. J. Eng. Phys. Thermophys. 2015, 88, 928–936. [Google Scholar] [CrossRef]
- Abbas, Z.; Sheikh, M.; Motsa, S.S. Numerical solution of binary chemical reaction on stagnation point flow of Casson fluid over a stretching/shrinking sheet with thermal radiation. Energy 2016, 95, 12–20. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Hoque, M.M.; Sivasankar, T. Radiative flow of Casson fluid over a moving wedge filled with gyrotactic microorganisms. Adv. Powder Technol. 2017, 28, 575–583. [Google Scholar] [CrossRef]
- Reddy, G.J.; Kethireddy, B.; Umavathi, J.C.; Sheremet, M.A. Heat flow visualization for unsteady Casson fluid past a vertical slender hollow cylinder. Ther. Sci. Eng. Prog. 2018, 5, 172–181. [Google Scholar] [CrossRef]
- Sulochana, C.; Ashwinkumar, G.P.; Sandeep, N. Effect of frictional heating on mixed convection flow of chemically reacting radiative Casson nanofluid over an inclined porous plate. Alex. Eng. J. 2018, 57, 2573–2584. [Google Scholar] [CrossRef]
- Ali, A.; Umar, M.; Bukhari, Z.; Abbas, Z. Pulsating flow of a micropolar-Casson fluid through a constricted channel influenced by a magnetic field and Darcian porous medium: A numerical study. Results Phys. 2020, 19, 103544. [Google Scholar] [CrossRef]
- Rehman, K.U.; Shatanawi, W.; Çolak, A.B. Artificial Neural Networking Magnification for Heat Transfer Coefficient in Convective Non-Newtonian Fluid with Thermal Radiations and Heat Generation Effects. Mathematics 2023, 11, 342. [Google Scholar] [CrossRef]
- Alizadeh, R.; Gomari, S.R.; Alizadeh, A.; Karimi, N.; Li, L.K. Combined heat and mass transfer and thermodynamic irreversibilities in the stagnation-point flow of Casson rheological fluid over a cylinder with catalytic reactions and inside a porous medium under local thermal nonequilibrium. Comput. Math. Appl. 2021, 81, 786–810. [Google Scholar] [CrossRef]
- Jamshed, W.; Goodarzi, M.; Prakash, M.; Nisar, K.S.; Zakarya, M.; Abdel-Aty, A.H. Evaluating the unsteady Casson nanofluid over a stretching sheet with solar thermal radiation: An optimal case study. Case Stud. Therm. Eng. 2021, 26, 101160. [Google Scholar] [CrossRef]
- Rehman, K.U.; Shatanawi, W.; Yaseen, S. A Comparative Numerical Study of Heat and Mass Transfer Individualities in Casson Stagnation Point Fluid Flow Past a Flat and Cylindrical Surfaces. Mathematics 2023, 11, 470. [Google Scholar] [CrossRef]
- Shatnawi, T.A.; Abbas, N.; Shatanawi, W. Mathematical analysis of unsteady stagnation point flow of radiative Casson hybrid nanofluid flow over a vertical Riga sheet. Mathematics 2022, 10, 3573. [Google Scholar] [CrossRef]
- Awan, A.U.; Ahammad, N.A.; Shatanawi, W.; Allahyani, S.A.; Tag-ElDin, E.M.; Abbas, N.; Ali, B. Significance of magnetic field and Darcy–Forchheimer law on dynamics of Casson-Sutterby nanofluid subject to a stretching circular cylinder. Int. Commun. Heat Mass Transf. 2022, 139, 106399. [Google Scholar] [CrossRef]
- Nawaz, Y.; Arif, M.S.; Abodayeh, K. Predictor–Corrector Scheme for Electrical Magnetohydrodynamic (MHD) Casson Nanofluid Flow: A Computational Study. Appl. Sci. 2023, 13, 1209. [Google Scholar] [CrossRef]
- Ly, H.B.; Nguyen, M.H.; Pham, B.T. Metaheuristic optimization of Levenberg-Marquardt-based artificial neural network using particle swarm optimization for prediction of foamed concrete compressive strength. Neural Comput. Appl. 2021, 33, 17331–17351. [Google Scholar] [CrossRef]
- Zhao, J.; Nguyen, H.; Nguyen-Thoi, T.; Asteris, P.G.; Zhou, J. Improved Levenberg-Marquardt backpropagation neural network by particle swarm and whale optimization algorithms to predict the deflection of RC beams. Eng. Comput. 2022, 38, 3847–3869. [Google Scholar] [CrossRef]
- Nguyen, H.X.; Cao, H.Q.; Nguyen, T.T.; Tran, T.N.C.; Tran, H.N.; Jeon, J.W. Improving robot precision positioning using a neural network based on Levenberg Marquardt–APSO algorithm. IEEE Access 2021, 9, 75415–75425. [Google Scholar] [CrossRef]
- Ali, M.S.; Ayaz, M.; Mansoor, T. Prediction of discharge through a sharp-crested triangular weir using ANN model trained with Levenberg-Marquardt algorithm, model. Earth Syst. Environ. 2022, 8, 1405–1417. [Google Scholar] [CrossRef]
- Ye, Z.; Kim, M.K. Predicting electricity consumption in a building using an optimized backpropagation and Levenberg-Marquardt backpropagation neural network: Case study of a shopping mall in China, Sustain. Cities Soc. 2018, 42, 176–183. [Google Scholar] [CrossRef]
- Bharati, S.; Rahman, M.A.; Podder, P.; Robel, M.R.A.; Gandhi, N. Comparative performance analysis of neural network base training algorithm and neuro-fuzzy system with SOM for the purpose of prediction of the features of superconductors. In International Conference on Intelligent Systems Design and Applications, Advances in Intelligent Systems and Computing; Springer: Cham, Switzerland, 2019; Volume 1181, pp. 69–79. [Google Scholar] [CrossRef]
- Shoaib, M.; Zubair, G.; Nisar, K.S.; Raja, M.A.Z.; Khan, M.I.; Gowda, R.P.; Prasannakumara, B. Ohmic heating effects and entropy generation for nanofluidic system of Ree-Eyring fluid: Intelligent computing paradigm. Int. Commun. Heat Mass Transf. 2021, 129, 105683. [Google Scholar] [CrossRef]
- Ahmad, I.; Ilyas, H.; Raja, M.A.Z.; Khan, Z.; Shoaib, M. Stochastic numerical computing with Levenberg-Marquardt backpropagation for performance analysis of heat Sink of functionally graded material of the porous fin. Surf. Interfaces 2021, 26, 101403. [Google Scholar] [CrossRef]
- Shoaib, M.; Raja, M.A.Z.; Sabir, M.T.; Bukhari, A.H.; Alrabaiah, H.; Shah, Z.; Kumam, P.; Islam, S. A stochastic numerical analysis based on hybrid NAR-RBFs networks non-linear SITR model for novel COVID-19 dynamics. Comput. Methods Programs Biomed. 2021, 202, 105973. [Google Scholar] [CrossRef]
- Shafiq, A.; Çolak, A.B.; Sindhu, T.N.; Al-Mdallal, Q.M.; Abdeljawad, T. Estimation of unsteady hydromagnetic Williamson fluid flow in a radiative surface through numerical and artificial neural network modeling. Sci. Rep. 2021, 11, 14509. [Google Scholar] [CrossRef] [PubMed]
- Shafiq, A.; Çolak, A.B.; Naz Sindhu, T. Designing artificial neural network of nanoparticle diameter and solid–fluid interfacial layer on single walled carbon nanotubes/ethylene glycol nanofluid flow on thin slendering needles. Int. J. Numer. Methods Fluids 2021, 93, 3384–3404. [Google Scholar] [CrossRef]
- Sabir, Z.; Raja, M.A.Z.; Guirao, J.L.; Shoaib, M. Integrated intelligent computing with neuroswarming solver for multi-singular fourth-order non-linear Emden–Fowler equation. Comput. Appl. Math. 2020, 39, 307. [Google Scholar] [CrossRef]
- Sabir, Z.; Raja, M.A.Z.; Umar, M.; Shoaib, M. Neuro-swarm intelligent computing to solve the second-order singular functional differential model. Eur. Phys. J. Plus 2020, 135, 474. [Google Scholar] [CrossRef]
- Shafiq, A.; Çolak, A.B.; Sindhu, T.N.; Muhammad, T. Optimization of Darcy-Forchheimer squeezing flow in non-linear stratified fluid under convective conditions with artificial neural network. Heat Transf. Res. 2022, 53, 67–89. [Google Scholar] [CrossRef]
- Shoaib, M.; Kausar, M.; Nisar, K.S.; Raja, M.A.Z.; Zeb, M.; Morsy, A. The design of intelligent networks for entropy generation in Ree-Eyring dissipative fluid flow system along quartic autocatalysis chemical reactions. Int. Commun. Heat Mass Transf. 2022, 133, 105971. [Google Scholar] [CrossRef]
- Umar, M.; Raja, M.A.Z.; Sabir, Z.; Alwabli, A.S.; Shoaib, M. A stochastic computational intelligent solver for numerical treatment of mosquito dispersal model in a heterogeneous environment. Eur. Phys. J. Plus 2020, 135, 565. [Google Scholar] [CrossRef]
- Abodayeh, K.; Raza, A.; Arif, M.S.; Rafiq, M.; Bibi, M.; Mohsin, M. Stochastic numerical analysis for impact of heavy alcohol consumption on transmission dynamics of gonorrhoea epidemic. CMC-Comput. Mater. Contin. 2020, 62, 1125–1142. [Google Scholar] [CrossRef]
- Shoaib Arif, M.; Raza, A.; Abodayeh, K.; Rafiq, M.; Bibi, M.; Nazeer, A. A numerical efficient technique for the solution of susceptible infected recovered epidemic model. Comput. Model. Eng. Sci. 2020, 124, 477–491. [Google Scholar] [CrossRef]
- Raza, A.; Rafiq, M.; Baleanu, D.; Shoaib Arif, M.; Naveed, M.; Ashraf, K. Competitive numerical analysis for stochastic HIV/AIDS epidemic model in a two-sex population. IET Syst. Biol. 2019, 13, 305–315. [Google Scholar] [CrossRef] [PubMed]
- Umar, M.; Amin, F.; Al-Mdallal, Q.; Ali, M.R. A stochastic computing procedure to solve the dynamics of prevention in HIV system. Biomed. Signal Process. Control 2022, 78, 103888. [Google Scholar] [CrossRef]
- Sajjad, U.; Hussain, I.; Imran, M.; Sultan, M.; Wang, C.-C.; Alsubaie, A.S.; Mahmoud, K.H. Boiling Heat Transfer Evaluation in Nanoporous Surface Coatings. Nanomaterials 2021, 11, 3383. [Google Scholar] [CrossRef]
- Yih, K.A. Free convection effect on MHD coupled heat and mass transfer of a moving permeable vertical surface. Int. Commun. Heat Mass Transf. 1999, 26, 95–104. [Google Scholar] [CrossRef]
- Hayat, T.; Mustafa, M.; Pop, I. Heat and mass transfer for Soret and Dufour’s effect on mixed convection boundary layer flow over a stretching vertical surface in a porous medium filled with a viscoelastic fluid. Commun. Non-Linear Sci. Numer. Simul. 2010, 15, 1183–1196. [Google Scholar] [CrossRef]
- Nawaz, Y.; Arif, M.S.; Abodayeh, K.; Bibi, M. Finite Element Method for Non-Newtonian Radiative Maxwell Nanofluid Flow under the Influence of Heat and Mass Transfer. Energies 2022, 15, 4713. [Google Scholar] [CrossRef]
- Arif, M.S.; Abodayeh, K.; Nawaz, Y. A Reliable Computational Scheme for Stochastic Reaction–Diffusion Non-linear Chemical Model. Axioms 2023, 12, 460. [Google Scholar] [CrossRef]
- Arif, M.S.; Shatanawi, W.; Nawaz, Y. A numerical scheme for Darcy-Forchheimer flow of non-Newtonian nanofluid under the effects of convective and zero mass flux boundary conditions. Ther. Sci. 2023. [Google Scholar] [CrossRef]
- Arif, M.S.; Abodayeh, K.; Nawaz, Y. A Computational Scheme for Stochastic Non-Newtonian Mixed Convection Nanofluid Flow over Oscillatory Sheet. Energies 2023, 16, 2298. [Google Scholar] [CrossRef]


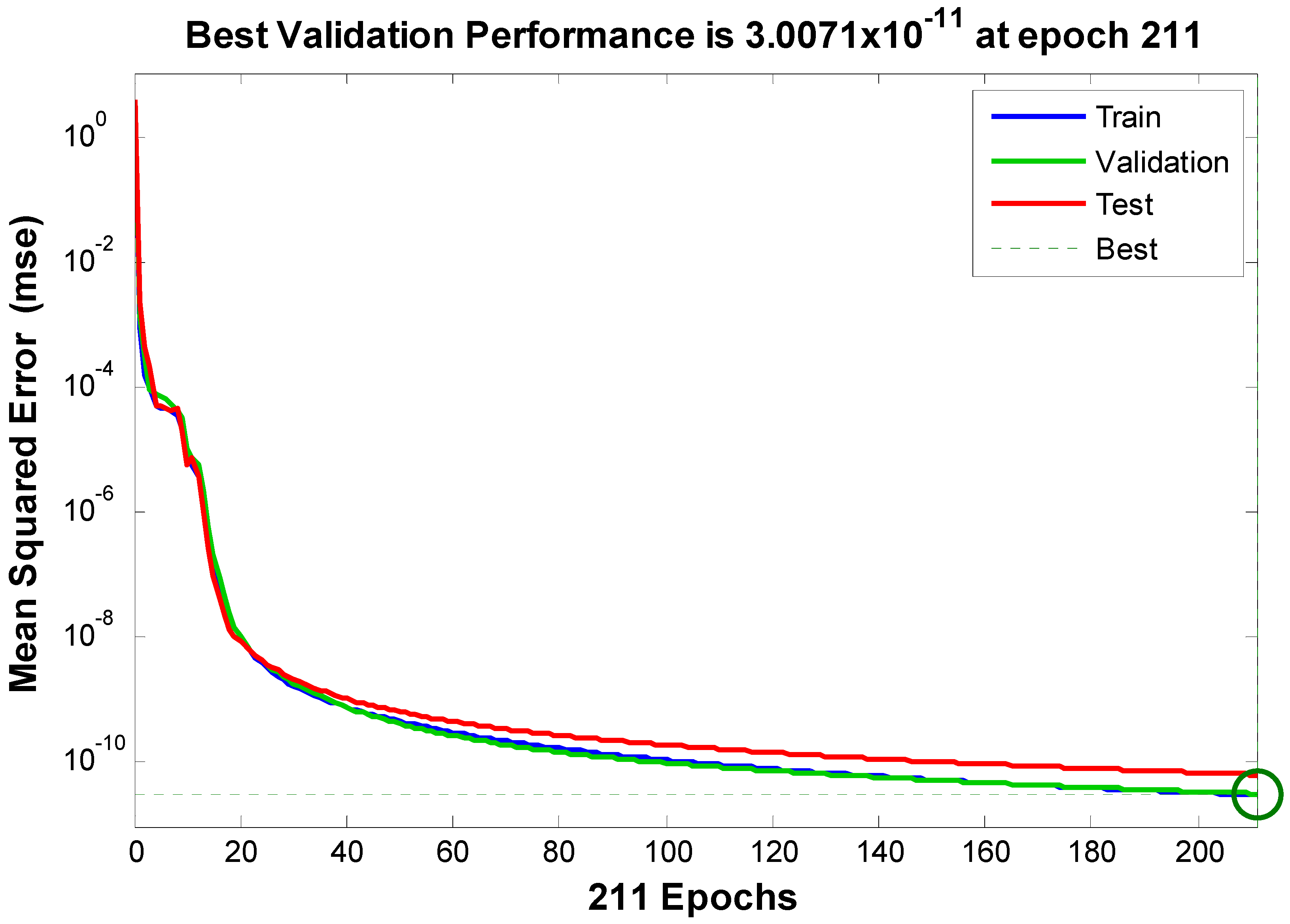

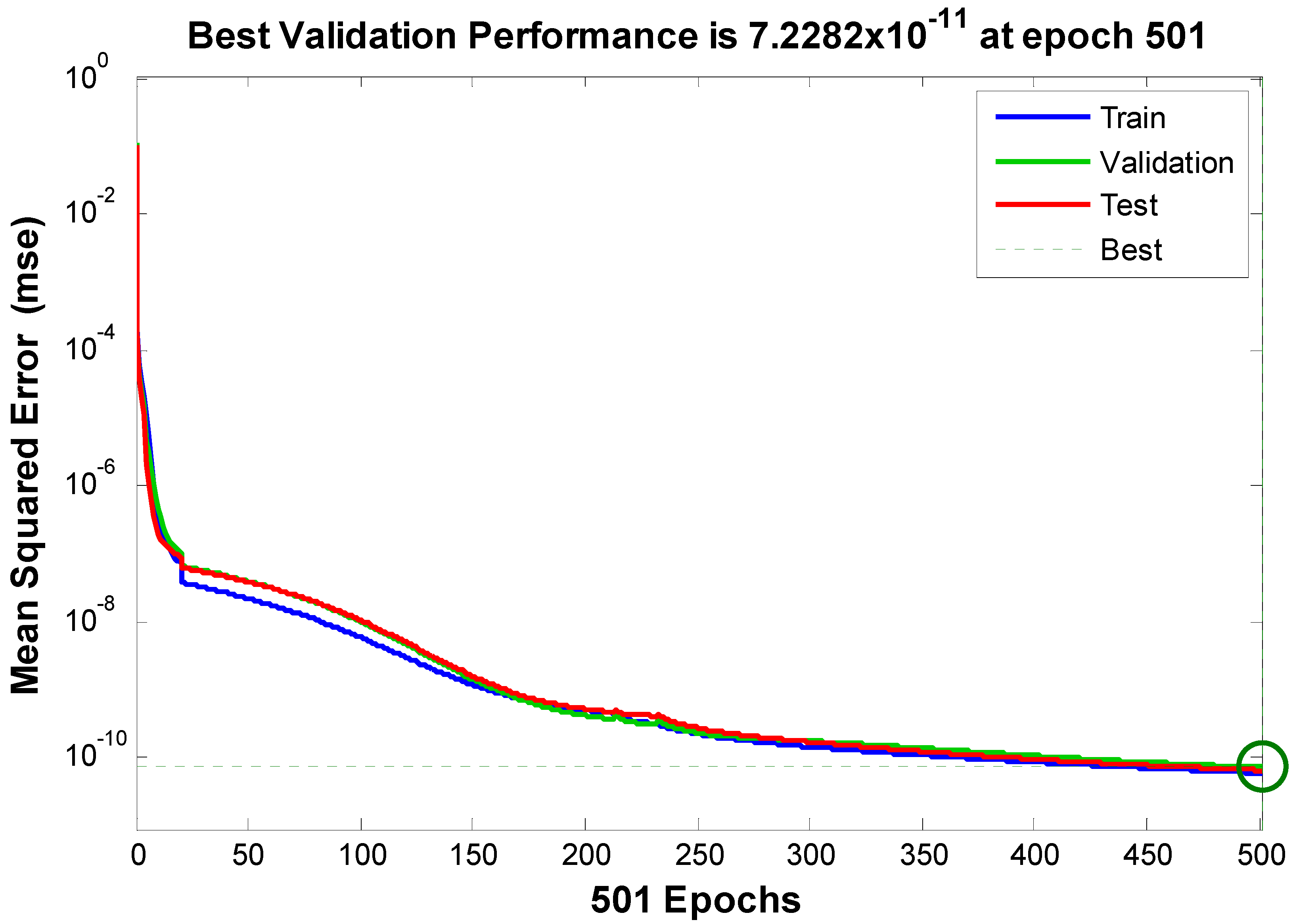
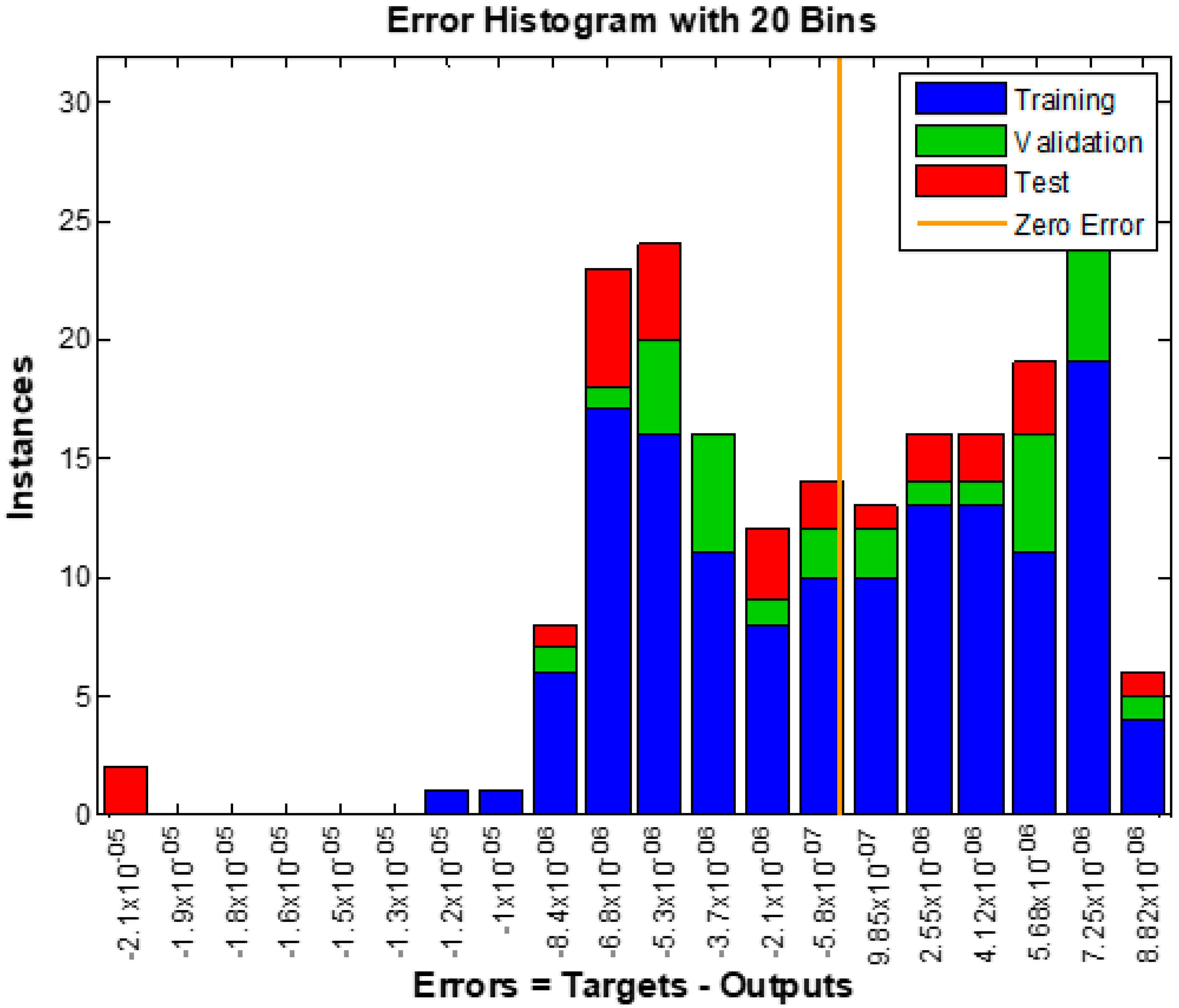

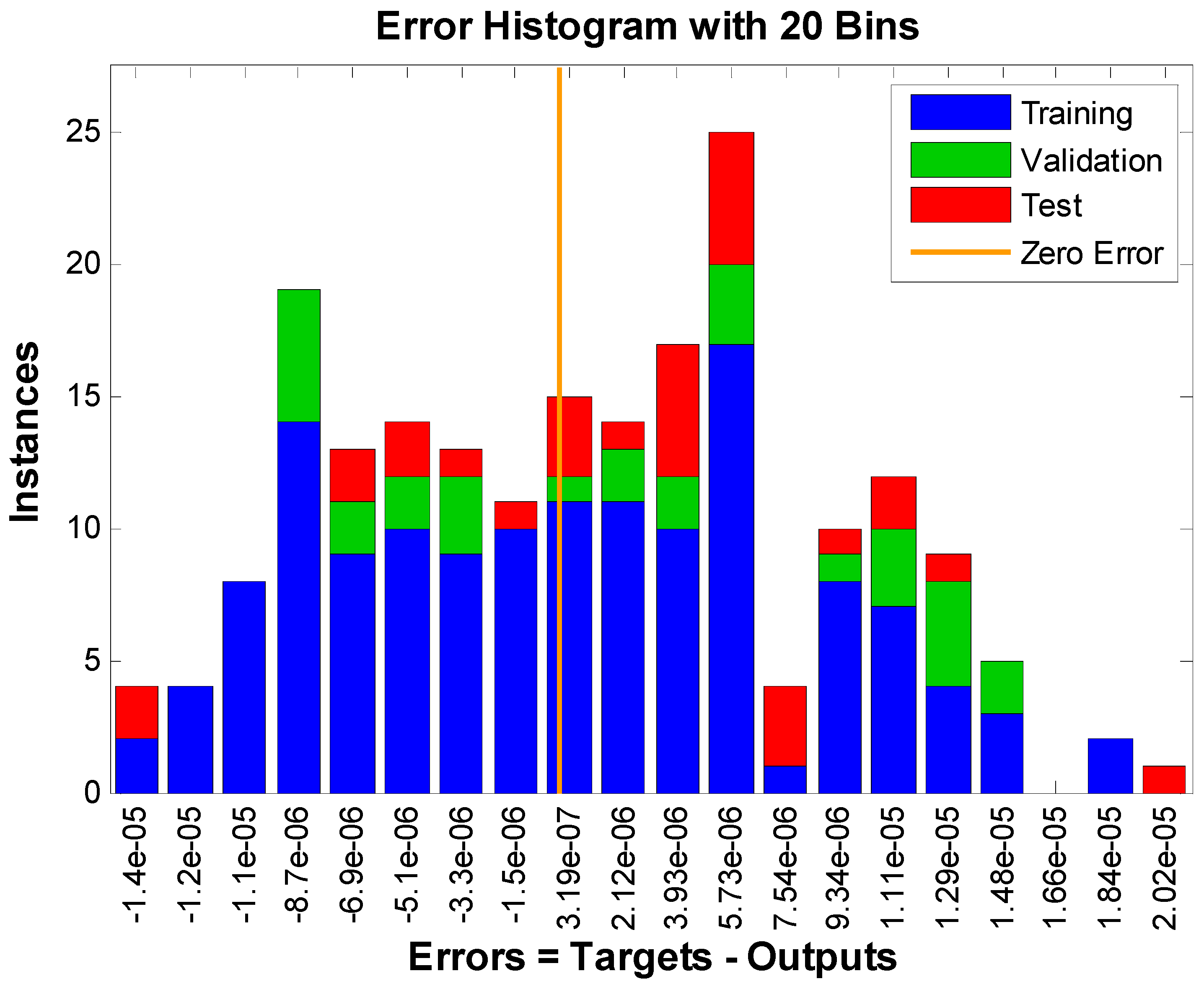



| Yih [40] | Hayat et al. [41] | Proposed | |
|---|---|---|---|
| 1.6578 | ||||
| 1.6521 | ||||
| 1.9152 | ||||
| 1.4984 | ||||
| 1.6744 |
| 0.0870 | |||||
| 0.0589 | |||||
| 0.0871 | |||||
| 0.0865 | |||||
| 0.3523 | |||||
| 0.0873 |
| 1.5 | 0.8604 | |||
| 0.6672 | ||||
| 0.9001 | ||||
| 3 | 1.2769 | |||
| 1.5 | 1.4034 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arif, M.S.; Abodayeh, K.; Nawaz, Y. Design of Finite Difference Method and Neural Network Approach for Casson Nanofluid Flow: A Computational Study. Axioms 2023, 12, 527. https://doi.org/10.3390/axioms12060527
Arif MS, Abodayeh K, Nawaz Y. Design of Finite Difference Method and Neural Network Approach for Casson Nanofluid Flow: A Computational Study. Axioms. 2023; 12(6):527. https://doi.org/10.3390/axioms12060527
Chicago/Turabian StyleArif, Muhammad Shoaib, Kamaleldin Abodayeh, and Yasir Nawaz. 2023. "Design of Finite Difference Method and Neural Network Approach for Casson Nanofluid Flow: A Computational Study" Axioms 12, no. 6: 527. https://doi.org/10.3390/axioms12060527
APA StyleArif, M. S., Abodayeh, K., & Nawaz, Y. (2023). Design of Finite Difference Method and Neural Network Approach for Casson Nanofluid Flow: A Computational Study. Axioms, 12(6), 527. https://doi.org/10.3390/axioms12060527









