On the Internal Counter-Torque between Springs in Serially Connected Statically Balanced Manipulators
Abstract
:1. Introduction
1.1. Technical Background
1.2. Related Literature
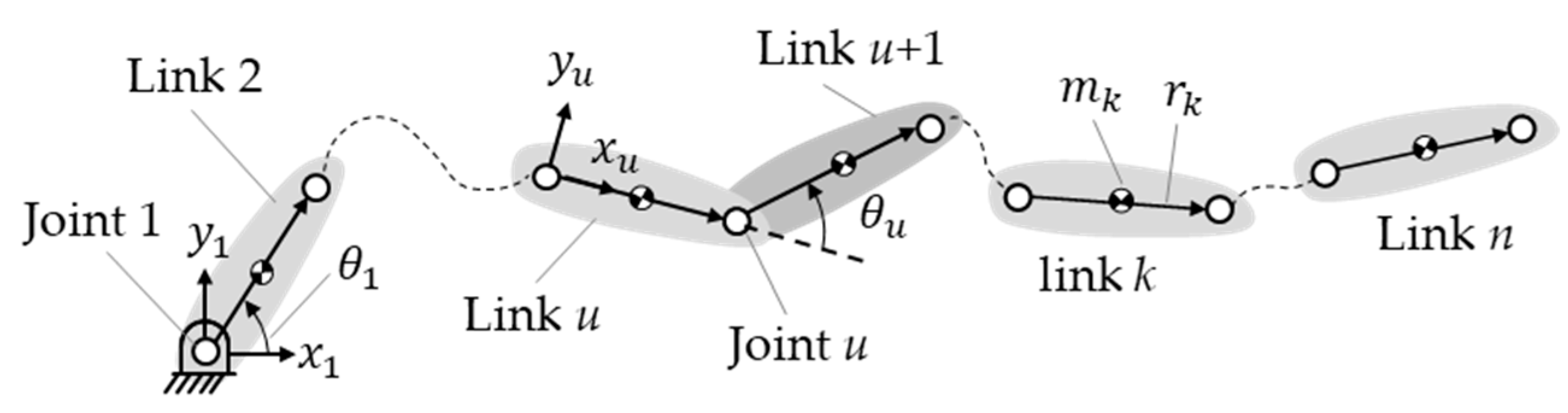
2. Torque Representation at a Typical Joint
2.1. Torque Contribution Caused by Gravity of a Typical Link at Joint u
2.2. Torque Contribution Caused by Spring at Joint u
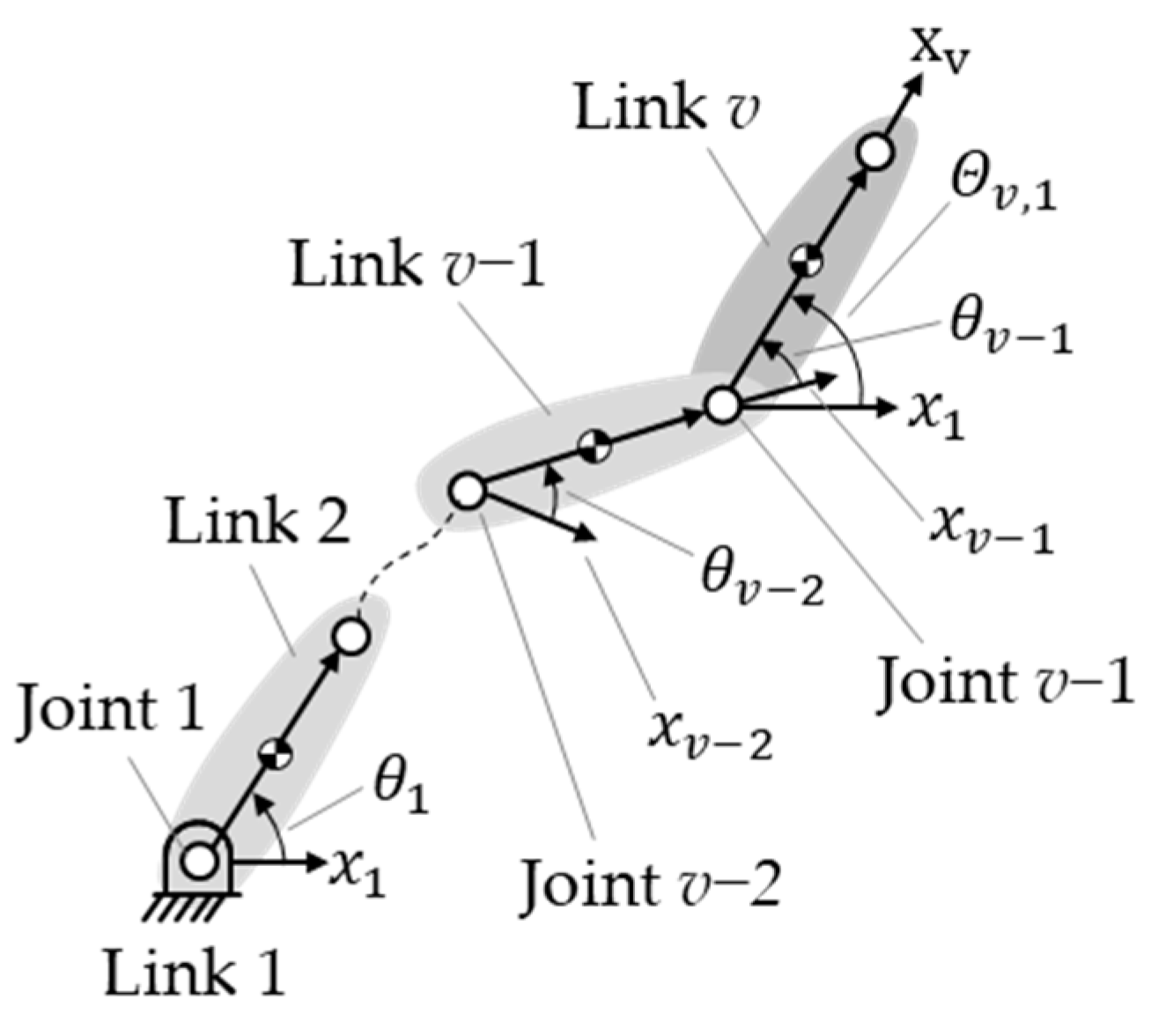
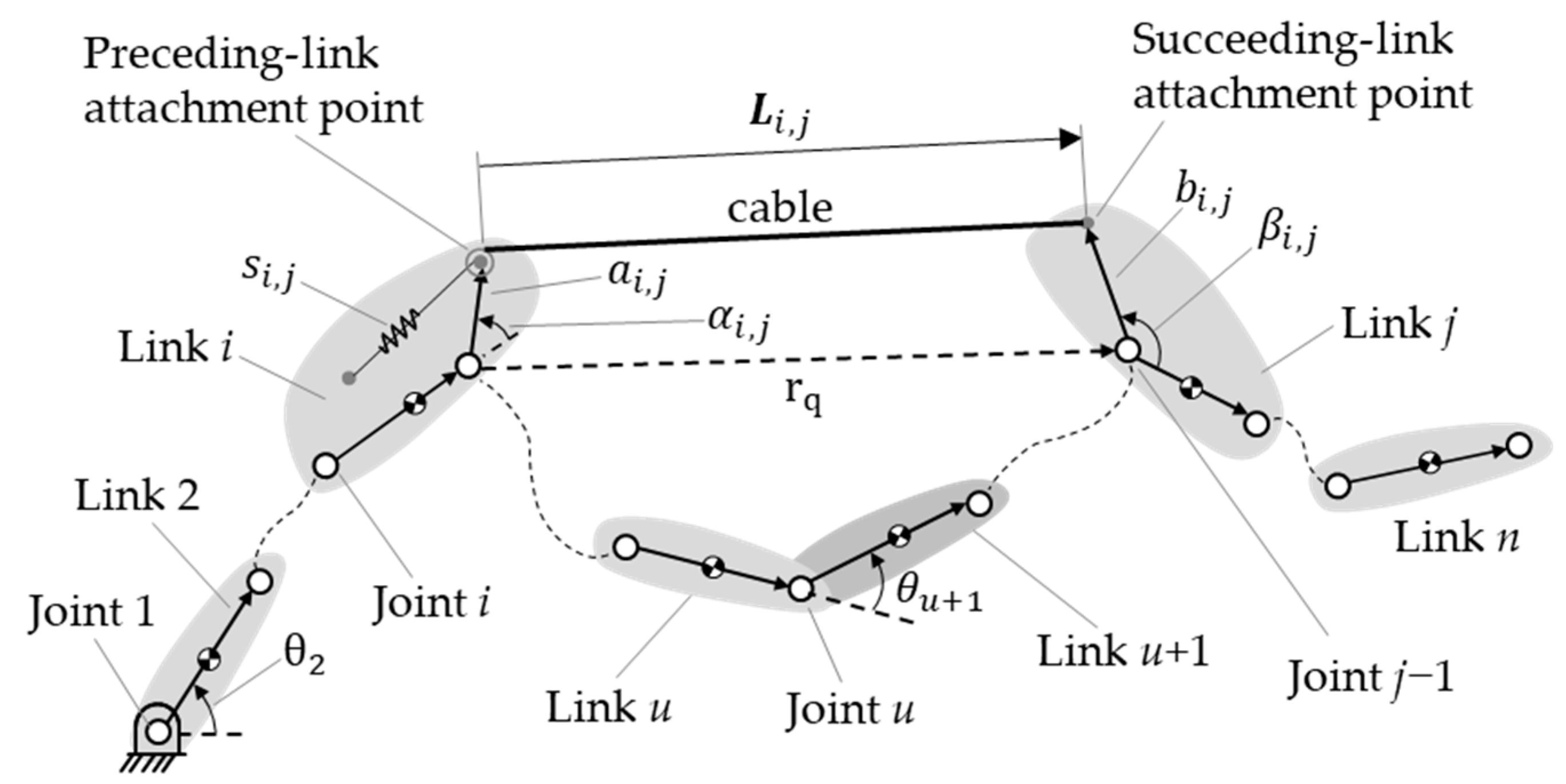
3. Internal Counter-Torque Classified from Torque Contribution Caused by Springs
3.1. Classification of Torque Contribution Caused by Springs
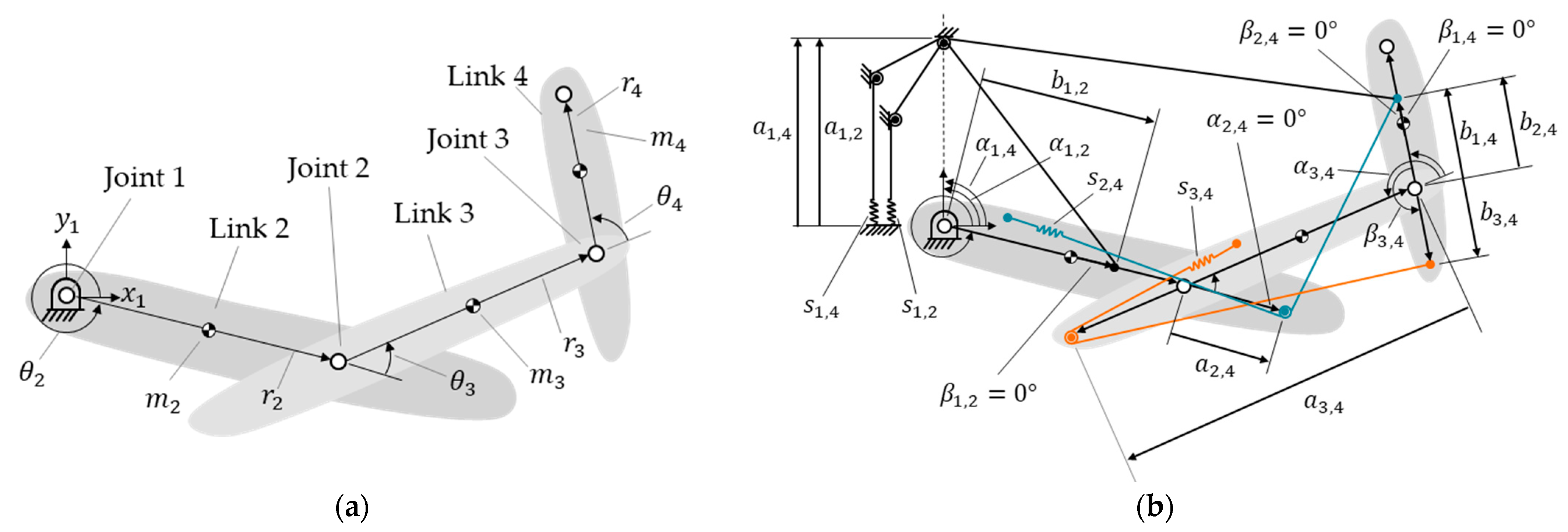
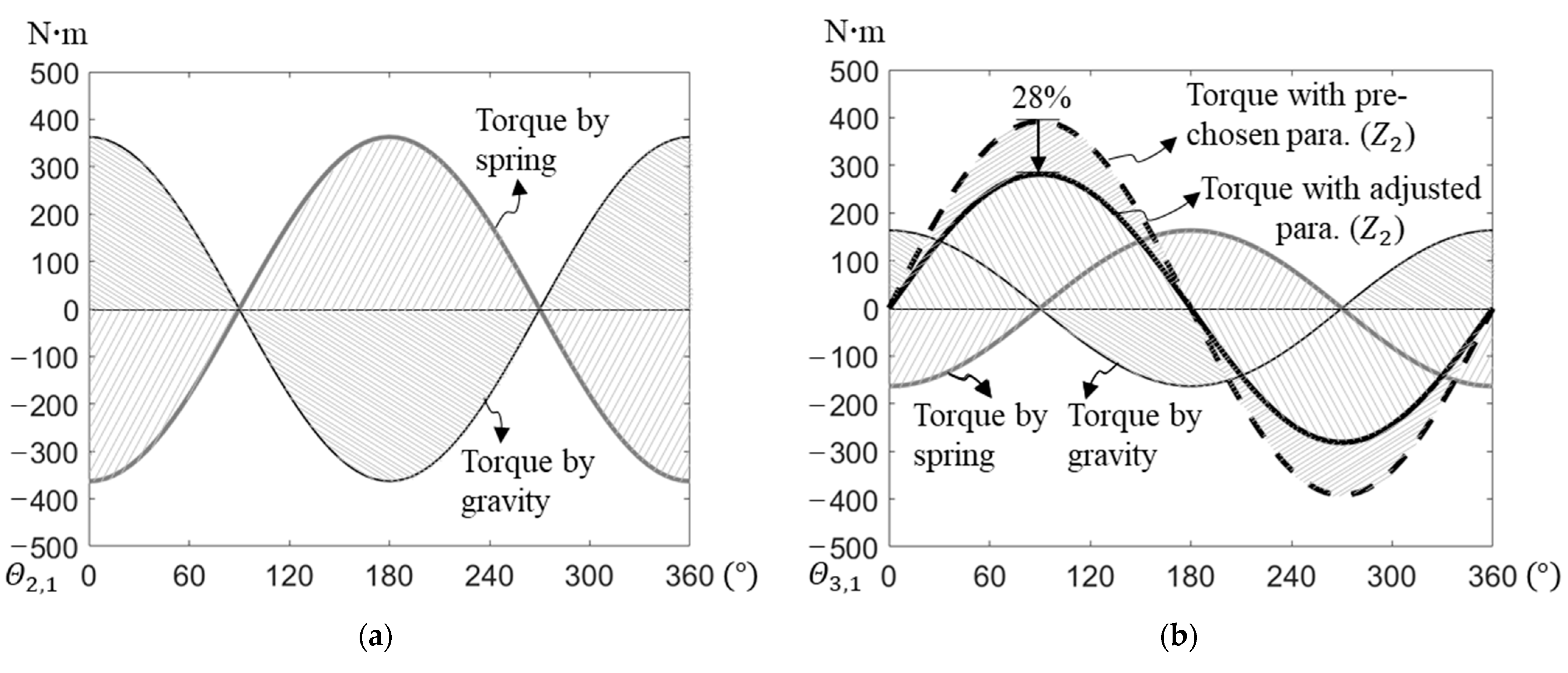
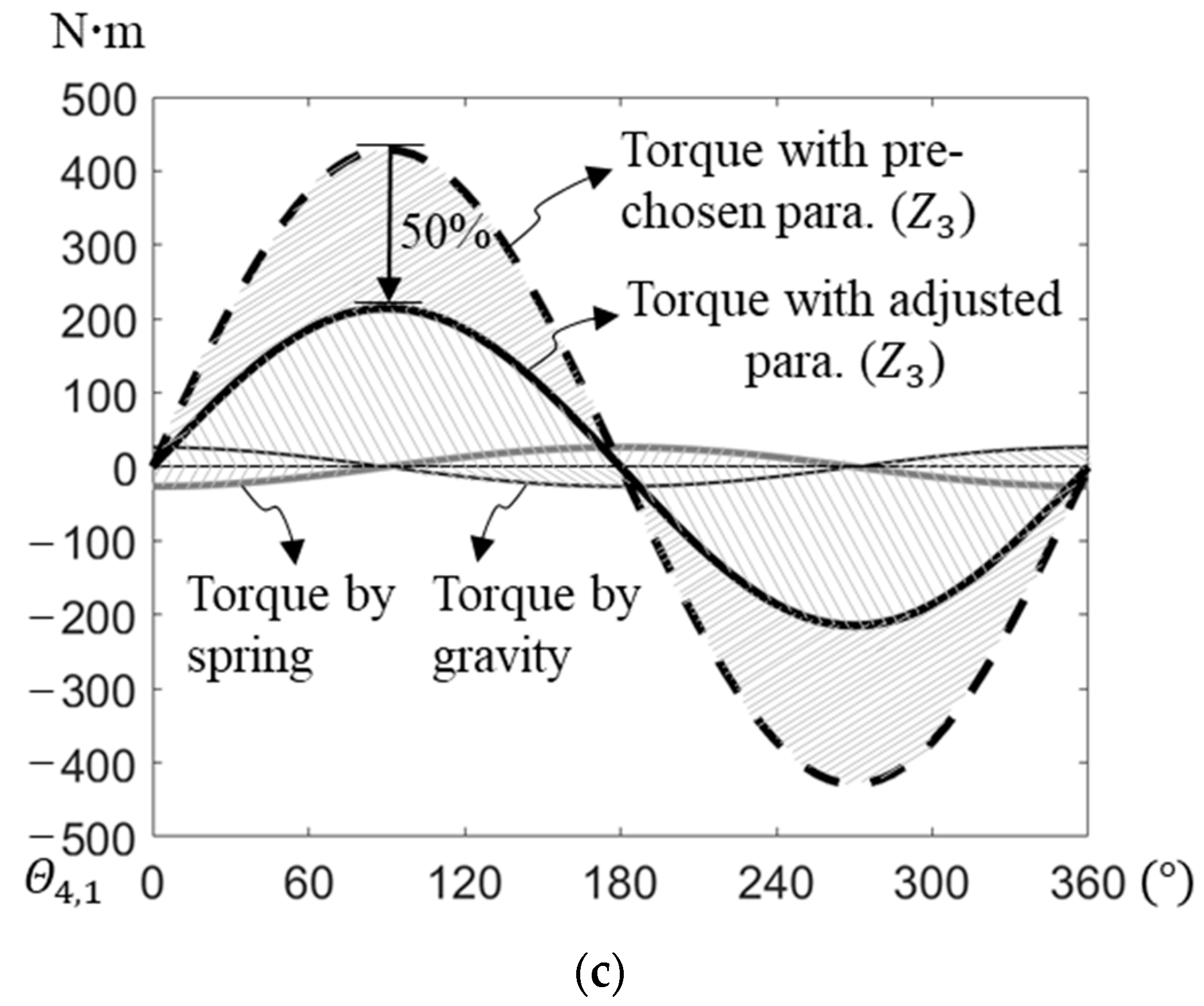
3.2. Internal Counter-Torque of an Illustrative Four-Link Manipulator
4. Minimum of Internal Counter-Torque of a Statically Balanced Manipulator
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hockstein, N.G.; Nolan, J.P.; O’Malley, B.W.; Woo, Y.J. Robotic microlaryngeal surgery: A technical feasibility study using the daVinci surgical robot and an airway mannequin. Laryngoscope 2005, 115, 780–785. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Li, J.; Wang, S.; Su, H.; Jiang, X. System design and animal experiment study of a novel minimally invasive surgical robot. Int. J. Med. Robot. Comput. Assist. Surg. 2016, 12, 73–84. [Google Scholar] [CrossRef] [PubMed]
- Lin, P.Y.; Shieh, W.B.; Chen, D.Z. A theoretical study of weight-balanced mechanisms for design of spring assistive mobile arm support (MAS). Mech. Mach. Theory 2013, 61, 156–167. [Google Scholar] [CrossRef]
- Zhou, L.B.; Chen, W.H.; Chen, W.J.; Bai, S.P.; Zhang, J.B.; Wang, J.H. Design of a passive lower limb exoskeleton for walking assistance with gravity compensation. Mech. Mach. Theory 2020, 150, 103840. [Google Scholar] [CrossRef]
- Zhou, L.L.; Bai, S.P.; Andersen, M.S.; Rasmussen, J. Modeling and Design of a Spring-loaded, Cable-driven, Wearable Exoskeleton for the Upper Extremity. Model. Identif. Control 2015, 36, 167–177. [Google Scholar] [CrossRef]
- Dewi, T.; Nurmaini, S.; Risma, P.; Oktarina, Y.; Roriz, M. Inverse kinematic analysis of 4 DOF pick and place arm robot manipulator using fuzzy logic controller. Int. J. Electr. Comput. Eng. 2020, 10, 1376–1386. [Google Scholar] [CrossRef]
- Stenmark, M.; Malec, J. Knowledge-based instruction of manipulation tasks for industrial robotics. Robot. Comput.-Integr. Manuf. 2015, 33, 56–67. [Google Scholar] [CrossRef]
- Martini, A.; Troncossi, M.; Rivola, A. Algorithm for the static balancing of serial and parallel mechanisms combining counterweights and springs: Generation, assessment and ranking of effective design variants. Mech. Mach. Theory 2019, 137, 336–354. [Google Scholar] [CrossRef]
- Segla, S. Static balancing of robot mechanisms and manipulation devices. Stroj. Časopis—J. Mech. Eng. 2018, 68, 77–90. [Google Scholar] [CrossRef]
- Hoevenaars, A.G.L.; Gosselin, C.; Lambert, P.; Herder, J.L. A Systematic Approach for the Jacobian Analysis of Parallel Manipulators with Two End-Effectors. Mech. Mach. Theory 2017, 109, 171–194. [Google Scholar] [CrossRef]
- Baradat, C.; Arakelian, V.; Briot, S.; Guegan, S. Design and prototyping of a new balancing mechanism for spatial parallel manipulators. J. Mech. Design 2008, 130, 072305. [Google Scholar] [CrossRef]
- Cho, C.H.; Lee, W. Design of a static balancer with equivalent mapping. Mech. Mach. Theory 2016, 101, 36–49. [Google Scholar] [CrossRef]
- Lu, Q.; Ortega, C.; Ma, O. Passive gravity compensation mechanisms: Technologies and applications. Recent Pat. Eng. 2011, 5, 32–44. [Google Scholar] [CrossRef]
- Kazerooni, H. Statically balanced direct drive manipulator. Robotica 1989, 7, 143–149. [Google Scholar] [CrossRef]
- Rahman, T.; Ramanathan, R.; Seliktar, R.; Harwin, W. A Simple Technique to Passively Gravity-Balance Articulated Mechanisms. ASME J. Mech. Des. 1995, 117, 655–658. [Google Scholar] [CrossRef]
- Koser, K. A cam mechanism for gravity-balancing. Mech. Res. Commun. 2009, 36, 523–530. [Google Scholar] [CrossRef]
- Simionescu, I.; Ciupitu, L. The static balancing of the industrial robot arms: Part II: Continuous balancing. Mech. Mach. Theory 2000, 35, 1299–1311. [Google Scholar] [CrossRef]
- Chu, Y.-L.; Kuo, C.-H. A single-degree-of-freedom self-regulated gravity balancer for adjustable payload. J. Mech. Robot. 2017, 9, 021006. [Google Scholar] [CrossRef]
- Ulrich, N.; Kumar, V. Passive mechanical gravity compensation for robot manipulators. In Proceedings of the IEEE International Conference on Robotics and Automation, Sacramento, CA, USA, 9–11 April 1991; Volume 2, pp. 1536–1541. [Google Scholar] [CrossRef]
- Agrawal, S.K.; Fattah, A. Gravity-balancing of spatial robotic manipulators. Mech. Mach. Theory 2004, 39, 1331–1344. [Google Scholar] [CrossRef]
- Deepak, S.R.; Ananthasuresh, G. Perfect static balance of linkages by addition of springs but not auxiliary bodies. J. Mech. Robot. 2012, 4, 021014. [Google Scholar] [CrossRef]
- Simionescu, I.; Ciupitu, L. The static balancing of the industrial robot arms: Part I: Discrete balancing. Mech. Mach. Theory 2000, 35, 1287–1298. [Google Scholar] [CrossRef]
- Deepak, S.R.; Ananthasuresh, G.K. Static balancing of spring-loaded planar revolute-joint linkages without auxiliary links. In Proceedings of the 14th National Conference on Machines and Mechanisms (NaCoMM09), Durgapur, India, 17–18 December 2009; pp. 37–44. [Google Scholar]
- Franchetti, D.; Boschetti, G.; Lenzo, B. Passive Gravity Balancing with a Self-Regulating Mechanism for Variable Payload. Machines 2021, 9, 145. [Google Scholar] [CrossRef]
- Mottola, G.; Cocconcelli, M.; Rubini, R.; Carricato, M. Gravity Balancing of Parallel Robots by Constant-Force Generators. In Gravity Compensation in Robotics; Mechanisms and Machine Science; Arakelian, V., Ed.; Springer: Cham, Switzerland, 2022; pp. 229–273. [Google Scholar] [CrossRef]
- Nguyen, V.L. Realization of a Gear-Spring Balancer With Variable Payloads and Its Application to Serial Robots. ASME J. Mech. Robot. 2023, 15, 041013. [Google Scholar] [CrossRef]
- Lee, Y.Y.; Chen, D.Z. Determination of spring installation configuration on statically balanced planar articulated manipulators. Mech. Mach. Theory 2014, 74, 319–336. [Google Scholar] [CrossRef]
- Tschiersky, M.; Hekman, E.E.; Herder, J.L.; Brouwer, D.M. Gravity Balancing Flexure Spring Mechanisms for Shoulder Support in Assistive Orthoses. IEEE Trans. Med. Robot. Bionics 2022, 4, 448–459. [Google Scholar] [CrossRef]
- Hsiu, W.H.; Syu, F.C.; Kuo, C.H. Design and implementation of a new statically balanced mechanism for slider-type desktop monitor stands. Proc. Inst. Mech. Eng. Part C—J. Mech. Eng. Sci. 2015, 229, 1671–1685. [Google Scholar] [CrossRef]
- Kuo, C.H.; Lai, S.J. Design of a Novel Statically Balanced Mechanism for Laparoscope Holders With Decoupled Positioning and Orientating Manipulation. J. Mech. Robot. 2016, 8, 015001. [Google Scholar] [CrossRef]
- Kamenskii, V. On the question of the balancing of plane linkages. J. Mech. 1968, 3, 303–322. [Google Scholar] [CrossRef]
- Raghu, E.; Balasubramonian, A. Experimental study on the elastodynamic behavior of the unbalanced and the counterweighted four bar mechanisms. J. Mech. Des. 1990, 112, 271–277. [Google Scholar] [CrossRef]
- Liu, G.; Liu, Y.; Goldenberg, A.A. Design, analysis, and control of a spring-assisted modular and reconfigurable robot. IEEE/ASME Trans. Mechatron. 2010, 16, 695–706. [Google Scholar] [CrossRef]
- Liao, K.; Huang, Q.; Fang, Y.; Zhu, J. Study on the Combined Spring Balance Technology Based on Multi-Axis Servo System. In Proceedings of the 4th International Conference on Intelligent Information Processing, Guilin, China, 16–17 November 2019; pp. 132–139. [Google Scholar] [CrossRef]
- Martini, A.; Troncossi, M.; Carricato, M.; Rivola, A. Elastodynamic behavior of balanced closed-loop mechanisms: Numerical analysis of a four-bar linkage. Meccanica 2014, 49, 601–614. [Google Scholar] [CrossRef]
- Xu, L.-X.; Li, Y.-G. Investigation of joint clearance effects on the dynamic performance of a planar 2-DOF pick-and-place parallel manipulator. Robot. Comput.-Integr. Manuf. 2014, 30, 62–73. [Google Scholar] [CrossRef]
- Ludovico, D.; Guardiani, P.; Lasagni, F.; Lee, J.; Cannella, F.; Caldwell, D.G. Design of Non-Circular Pulleys for Torque Generation: A Convex Optimisation Approach. IEEE Robot. Autom. Lett. 2021, 6, 958–965. [Google Scholar] [CrossRef]
- Coelho, T.A.H.; Yong, L.; Alves, V.F.A. Decoupling of dynamic equations by means of adaptive balancing of 2-dof open-loop mechanisms. Mech. Mach. Theory 2004, 39, 871–881. [Google Scholar] [CrossRef]
- Vezvari, M.R.; Nikoobin, A.; Ghoddosian, A. Perfect torque compensation of planar 5R parallel robot in point-to-point motions, optimal control approach. Robotica 2021, 39, 1163–1180. [Google Scholar] [CrossRef]
- Arakelian, V.; Le Baron, J.; Mottu, P. Torque minimisation of the 2-DOF serial manipulators based on minimum energy consideration and optimum mass redistribution. Mechatronics 2011, 21, 310–314. [Google Scholar] [CrossRef]
- de Jong, J.J.; Aarts, R.G.K.M. Static Balance of a Flexure-Based Four-Bar Mechanism: Less Torque with More Preload. In RAAD 2022: Advances in Service and Industrial Robotics, Proceedings of the 31st International Conference on Robotics in Alpe-Adria Danube Region, Klagenfurt, Austria, 8–10 June 2022; Mechanisms and Machine Science; Müller, A., Brandstötter, M., Eds.; Springer: Cham, Switzerland, 2022; pp. 306–313. [Google Scholar] [CrossRef]
- Delissen, A.A.; Radaelli, G.; Herder, J.L. Design and optimization of a general planar zero free length spring. Mech. Mach. Theory 2017, 117, 56–77. [Google Scholar] [CrossRef]
- Ou, Y.H.; Chen, D.Z. Compact Arrangements of Cable-Pulley Type Zero-Free-Length Springs. J. Mech. Robot. 2017, 9, 044502. [Google Scholar] [CrossRef]
- Barents, R.; Schenk, M.; van Dorsser, W.D.; Wisse, B.M.; Herder, J.L. Spring-to-Spring Balancing as Energy-Free Adjustment Method in Gravity Equilibrators. J. Mech. Des. 2011, 133, 061010. [Google Scholar] [CrossRef]
- Juang, C.W.; Jhuang, C.S.; Chen, D.Z. Spring efficiency assessment and efficient use of spring methods of statically balanced planar serial manipulators with revolute joints only. Mech. Sci. 2022, 13, 817–830. [Google Scholar] [CrossRef]
| 25 | 26 | 18 | 0.36 | 0.45 | 0.30 |
| 90 | 90 | 0 | 180 | 0 | 0 | 0 | 180 | 0.30 | 0.09 | 0.09 | 0.25 |
| Preselected | 1001 | 1014 | 3649 | 1828 | 0.30 | 0.40 | 0.10 | 0.40 | 0 | 392 | 429 | 821 |
| Optimized | 443 | 1713 | 0.69 | 0.21 | 0.18 | 282 | 215 | 497 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jhuang, C.-S.; Juang, C.-W.; Shih, C.-H.; Chen, D.-Z. On the Internal Counter-Torque between Springs in Serially Connected Statically Balanced Manipulators. Machines 2023, 11, 200. https://doi.org/10.3390/machines11020200
Jhuang C-S, Juang C-W, Shih C-H, Chen D-Z. On the Internal Counter-Torque between Springs in Serially Connected Statically Balanced Manipulators. Machines. 2023; 11(2):200. https://doi.org/10.3390/machines11020200
Chicago/Turabian StyleJhuang, Chi-Shiun, Chia-Wei Juang, Cheng-Hsin Shih, and Dar-Zen Chen. 2023. "On the Internal Counter-Torque between Springs in Serially Connected Statically Balanced Manipulators" Machines 11, no. 2: 200. https://doi.org/10.3390/machines11020200
APA StyleJhuang, C.-S., Juang, C.-W., Shih, C.-H., & Chen, D.-Z. (2023). On the Internal Counter-Torque between Springs in Serially Connected Statically Balanced Manipulators. Machines, 11(2), 200. https://doi.org/10.3390/machines11020200





