Hemodynamic Changes in the Carotid Artery after Infusion of Normal Saline Using Computational Fluid Dynamics
Abstract
:1. Introduction
2. Materials and Methods
2.1. Subject Selection
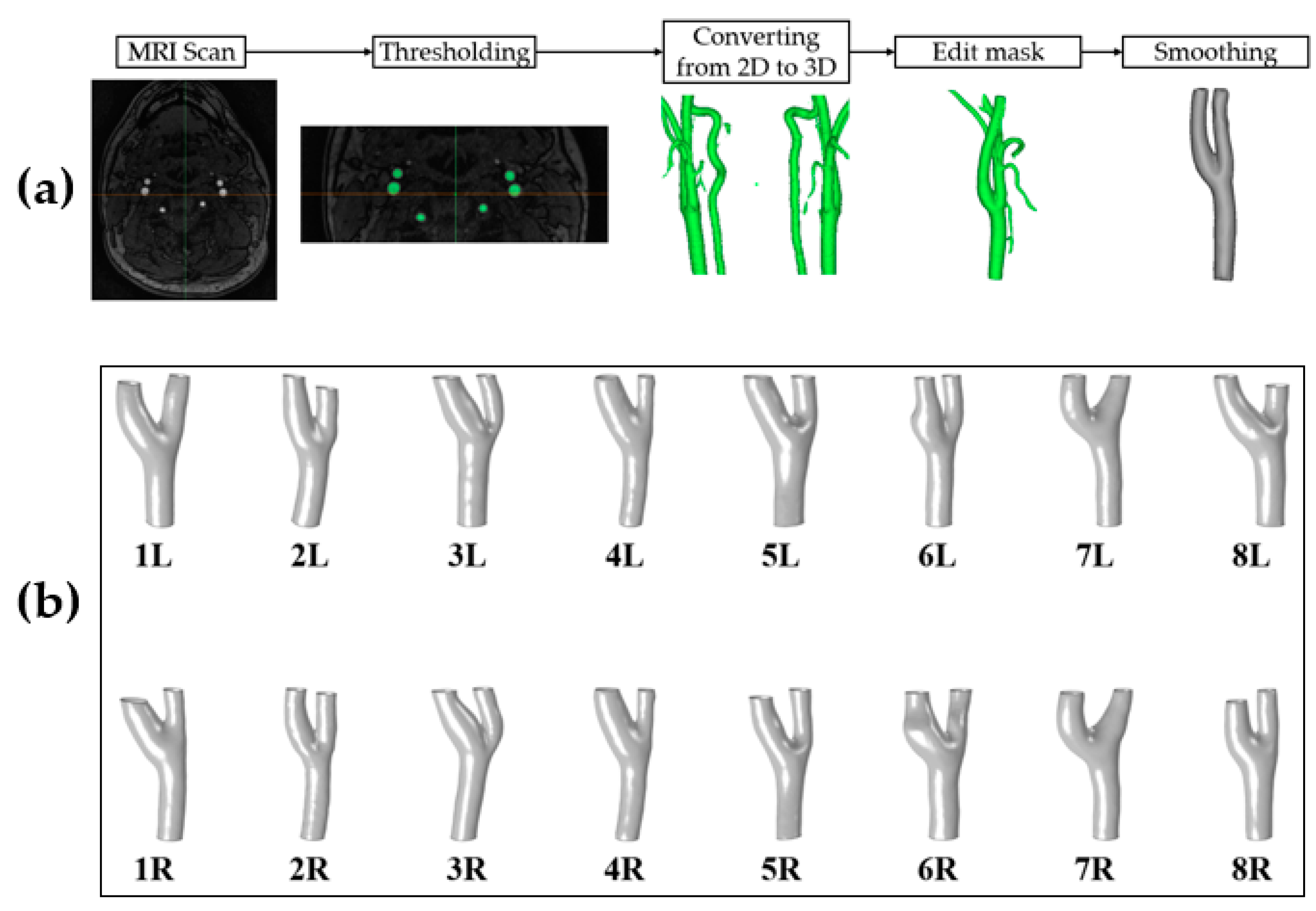
2.2. Image Processing
2.3. Blood Viscosity
2.4. Computational Fluid Dynamics
2.5. Hemodynamic Analysis
3. Results
3.1. Blood Viscosity Change
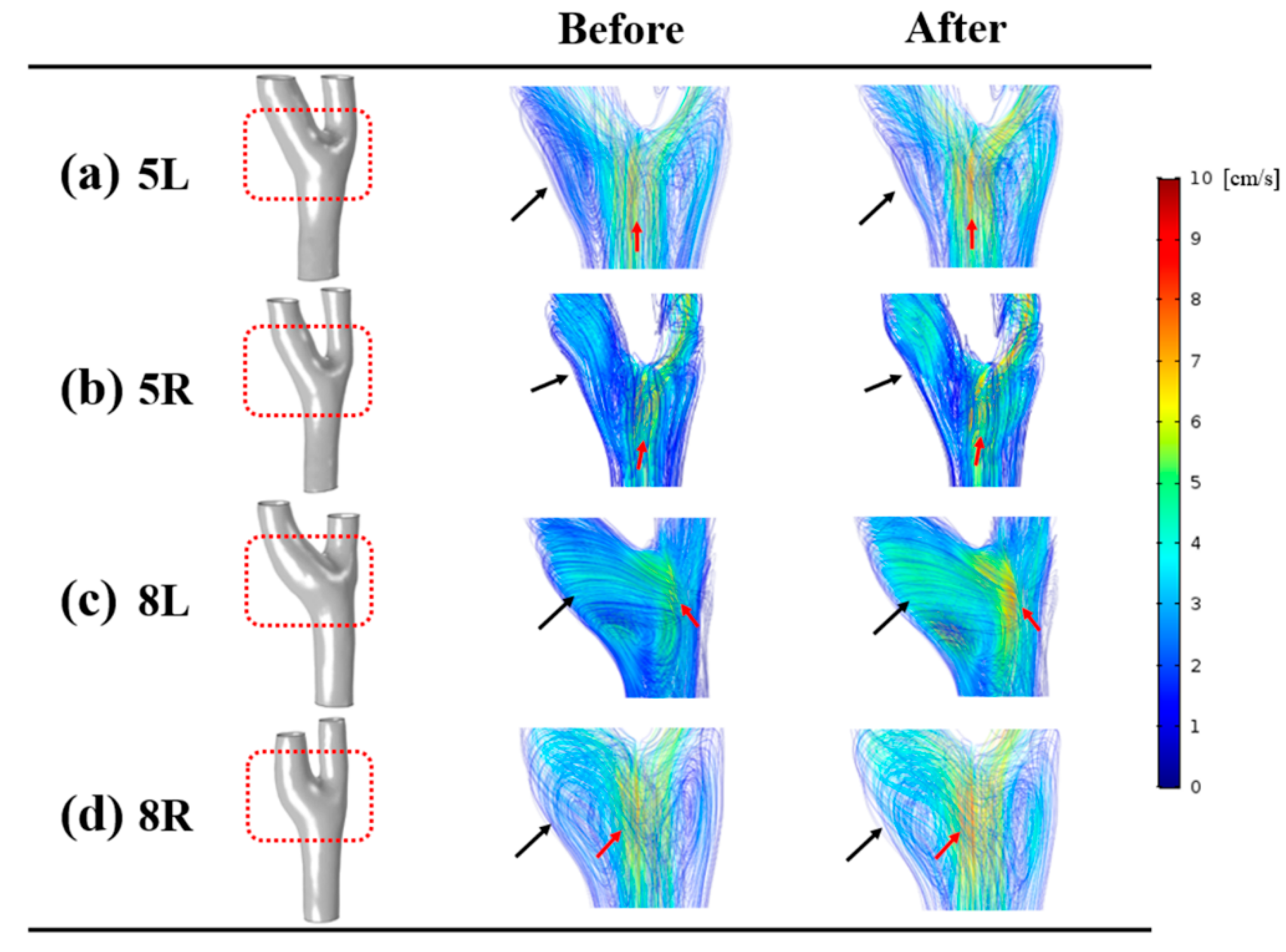
3.2. Velocity
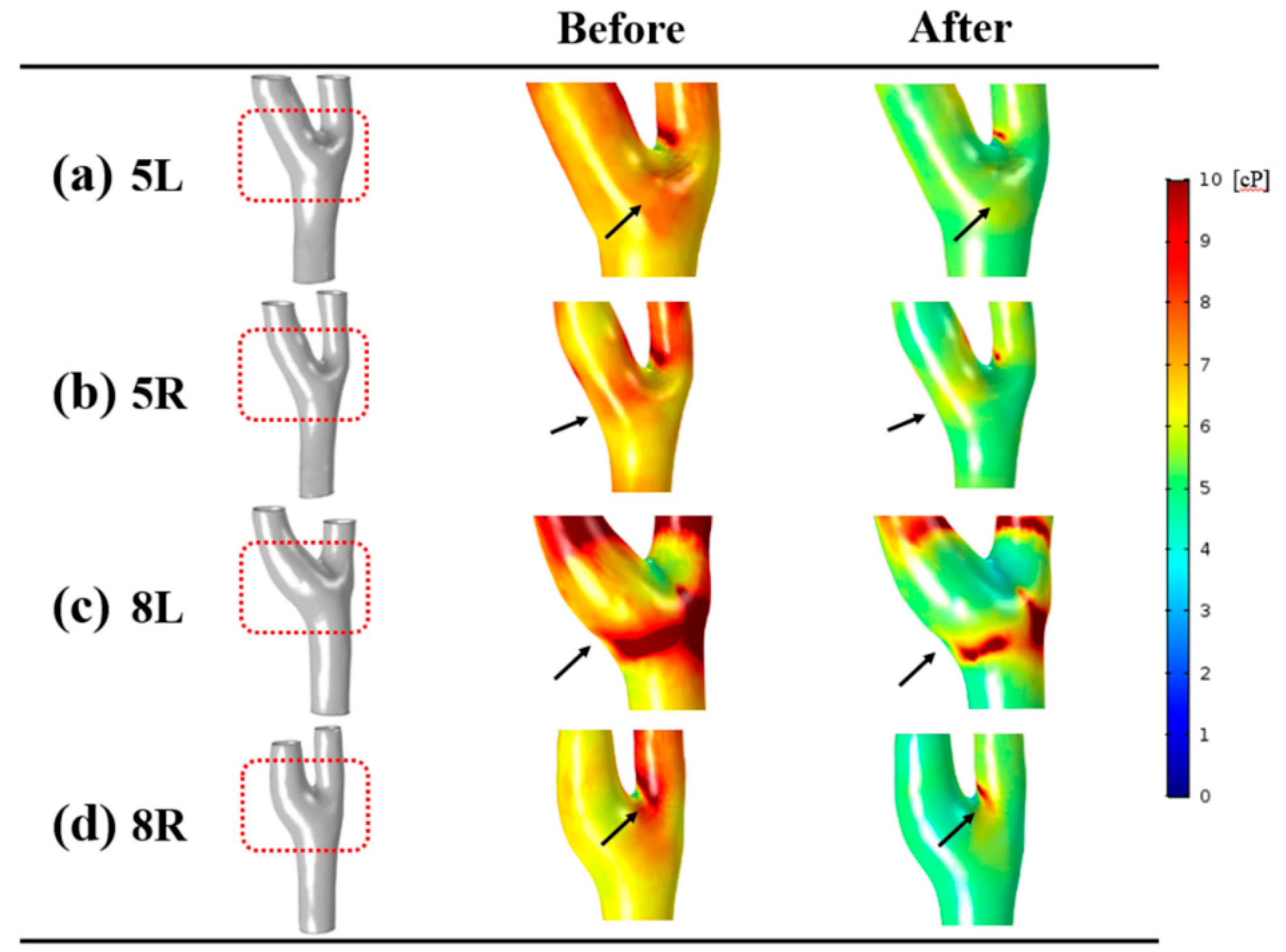
3.3. Shear Rate
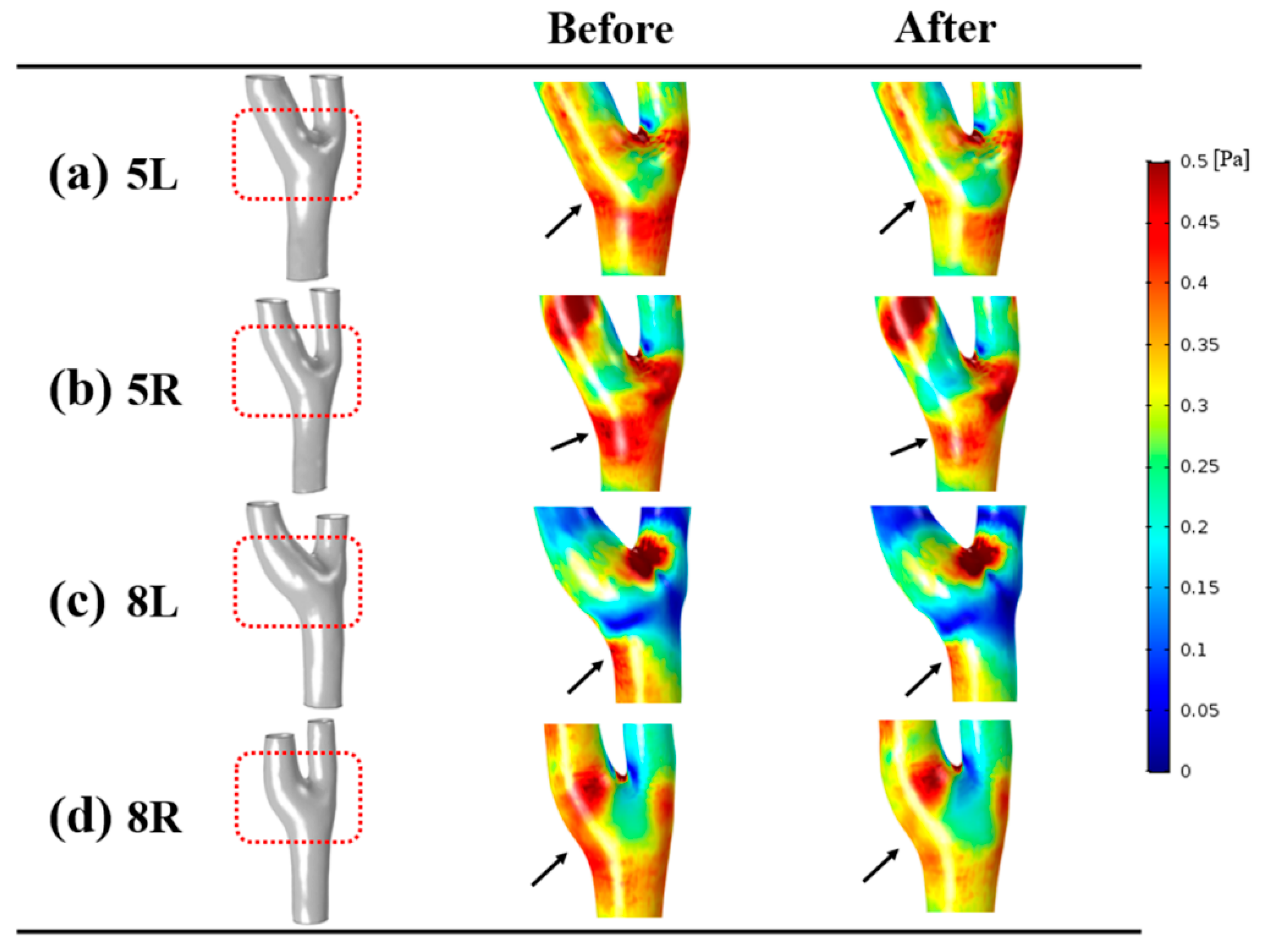
3.4. Wall Shear Stress
4. Discussion
4.1. Normal Saline
4.2. Velocity
4.3. Hemodynamic Characteristics at the Carotid Bulb
4.4. Blood Viscosity
4.5. Relationship between the Hemodynamic Factors
4.6. Limitations
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- ReferencesHa, Y.K.; Hong, H.; Yeom, E.; Song, J.M. Numerical study of the pulsatile flow depending on non-Newtonian viscosity in a stenosed microchannel. J. Vis. 2020, 23, 61–70. [Google Scholar]
- Carty, G.; Chatpun, S.; Espino, D.M. Modeling blood flow through intracranial aneurysms: A comparison of Newtonian and non-Newtonian viscosity. J. Med. Biol. Eng. 2016, 36, 396–409. [Google Scholar] [CrossRef]
- Myburgh, J.A.; Mythen, M.G. Resuscitation fluids. N. Engl. J. Med. 2013, 369, 2462–2463. [Google Scholar] [CrossRef] [PubMed]
- Blumberg, N.; Cholette, J.M.; Pietropaoli, A.P.; Phipps, R.; Spinelli, S.L.; Eaton, M.P.; Noronha, S.A.; Seghatchian, J.; Heal, J.M.; Refaai, M.A. 0.9% NaCl (Normal Saline)-Perhaps not so normal after all? Transfus. Apher. Sci. 2018, 57, 127–131. [Google Scholar] [CrossRef] [PubMed]
- Williams, E.L.; Hildebrand, K.L.; McCormick, S.A.; Bedel, M.J. The effect of intravenous lactated Ringer’s solution versus 0.9% sodium chloride solution on serum osmolality in human volunteers. Anesth. Analg. 1999, 88, 999–1003. [Google Scholar] [CrossRef]
- Chowdhury, A.H.; Cox, E.F.; Francis, S.T.; Lobo, D.N. A randomized, controlled, double-blind crossover study on the effects of 2-L infusions of 0.9% saline and plasma-lyte(R) 148 on renal blood flow velocity and renal cortical tissue perfusion in healthy volunteers. Ann. Surg. 2012, 256, 18–24. [Google Scholar] [CrossRef] [PubMed]
- De Bruyne, B.; Adjedj, J.; Xaplanteris, P.; Ferrara, A.; Mo, Y.; Penicka, M.; Flore, V.; Pellicano, M.; Toth, G.; Barbato, E.; et al. Saline-Induced Coronary Hyperemia: Mechanisms and Effects on Left Ventricular Function. Circ. Cardiovasc. Interv. 2017, 10, e004719. [Google Scholar] [CrossRef]
- Cecchi, E.; Giglioli, C.; Valente, S.; Lazzeri, C.; Gensini, G.F.; Abbate, R.; Mannini, L. Role of hemodynamic shear stress in cardiovascular disease. Atherosclerosis 2011, 214, 249–256. [Google Scholar] [CrossRef] [PubMed]
- Li, C.H.; Gao, B.L.; Wang, J.W.; Liu, J.F.; Li, H.; Yang, S.T. Hemodynamic Factors Affecting Carotid Sinus Atherosclerotic Stenosis. World Neurosurg. 2019, 121, e262–e276. [Google Scholar] [CrossRef]
- Li, X.; Sun, B.; Zhao, H.; Ge, X.; Liang, F.; Li, X.; Xu, J.; Liu, X. Retrospective Study of Hemodynamic Changes Before and After Carotid Stenosis Formation by Vessel Surface Repairing. Sci. Rep. 2018, 8, 5493. [Google Scholar] [CrossRef] [Green Version]
- Birchall, D.; Zaman, A.; Hacker, J.; Davies, G.; Mendelow, D. Analysis of haemodynamic disturbance in the atherosclerotic carotid artery using computational fluid dynamics. Eur. Radiol. 2006, 16, 1074–1083. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.W.; Antiga, L.; Spence, J.D.; Steinman, D.A. Geometry of the carotid bifurcation predicts its exposure to disturbed flow. Stroke 2008, 39, 2341–2347. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Box, F.M.; van der Geest, R.J.; Rutten, M.C.; Reiber, J.H. The influence of flow, vessel diameter, and non-newtonian blood viscosity on the wall shear stress in a carotid bifurcation model for unsteady flow. Invest. Radiol. 2005, 40, 277–294. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benzakoun, J.; Roca, P.; Calvet, D.; Naggara, O.; Lion, S.; Gobin-Metteil, M.-P.; Charron, S.; Cavero, V.; Meder, J.-F.; Edjlali, M. Optimal 4DFlow MR sequence parameters for the assessment of internal carotid artery stenosis: A simulation study. Neuroradiology 2019, 61, 1137–1144. [Google Scholar] [CrossRef]
- Töger, J.; Zahr, M.J.; Aristokleous, N.; Markenroth Bloch, K.; Carlsson, M.; Persson, P.O. Blood flow imaging by optimal matching of computational fluid dynamics to 4D-flow data. Magn. Reson. Med. 2020, 84, 2231–2245. [Google Scholar] [CrossRef] [Green Version]
- Berman, S.E.; Rivera-Rivera, L.A.; Clark, L.R.; Racine, A.M.; Keevil, J.G.; Bratzke, L.C.; Carlsson, C.M.; Bendlin, B.B.; Rowley, H.A.; Blennow, K. Intracranial arterial four-dimensional flow is associated with metrics of brain health and Alzheimer’s disease. Alzheimer’s Dement. Diagn. Assess. Dis. Monit. 2015, 1, 420–428. [Google Scholar] [CrossRef] [Green Version]
- Oshida, S.; Mori, F.; Sasaki, M.; Sato, Y.; Kobayshi, M.; Yoshida, K.; Fujiwara, S.; Ogasawara, K. Wall shear stress and T1 contrast ratio are associated with embolic signals during carotid exposure in endarterectomy. Stroke 2018, 49, 2061–2066. [Google Scholar] [CrossRef]
- Watanabe, T.; Isoda, H.; Takehara, Y.; Terada, M.; Naito, T.; Kosugi, T.; Onishi, Y.; Tanoi, C.; Izumi, T. Hemodynamic vascular biomarkers for initiation of paraclinoid internal carotid artery aneurysms using patient-specific computational fluid dynamic simulation based on magnetic resonance imaging. Neuroradiology 2018, 60, 545–555. [Google Scholar] [CrossRef]
- Sun, L.; Wang, J.; Li, M.; Li, M.; Zhu, Y. The contribution of wall shear stress insult to the growth of small unruptured cerebral aneurysms in longitudinal 3D-TOF-MRA. J. Neurol. Sci. 2020, 413, 116798. [Google Scholar] [CrossRef]
- Khan, M.O.; Arana, V.T.; Rubbert, C.; Cornelius, J.F.; Fischer, I.; Bostelmann, R.; Mijderwijk, H.-J.; Turowski, B.; Steiger, H.-J.; May, R. Association between aneurysm hemodynamics and wall enhancement on 3D vessel wall MRI. J. Neurosurg. 2020, 1, 1–11. [Google Scholar] [CrossRef]
- Zhao, S.Z.; Xu, X.Y.; Hughes, A.D.; Thom, S.A.; Stanton, A.V.; Ariff, B.; Long, Q. Blood flow and vessel mechanics in a physiologically realistic model of a human carotid arterial bifurcation. J. Biomech. 2000, 33, 975–984. [Google Scholar] [CrossRef]
- Gijsen, F.J.; Allanic, E.; van de Vosse, F.N.; Janssen, J.D. The influence of the non-Newtonian properties of blood on the flow in large arteries: Unsteady flow in a 90 degrees curved tube. J. Biomech. 1999, 32, 705–713. [Google Scholar] [CrossRef]
- Perktold, K.; Resch, M.; Florian, H. Pulsatile non-Newtonian flow characteristics in a three-dimensional human carotid bifurcation model. J. Biomech. Eng. 1991, 113, 464–475. [Google Scholar] [CrossRef]
- Chen, Z.; Qin, H.; Liu, J.; Wu, B.; Cheng, Z.; Jiang, Y.; Liu, L.; Jing, L.; Leng, X.; Jing, J. Characteristics of Wall Shear Stress and Pressure of Intracranial Atherosclerosis Analyzed by a Computational Fluid Dynamics Model: A Pilot Study. Front. Neurol. 2020, 10, 1372. [Google Scholar] [CrossRef] [PubMed]
- Dai, Y.; Lv, P.; Javadzadegan, A.; Tang, X.; Qian, Y.; Lin, J. Hemodynamic analysis of carotid artery after endarterectomy: A preliminary and quantitative imaging study based on computational fluid dynamics and magnetic resonance angiography. Quant. Imaging Med. Surg. 2018, 8, 399. [Google Scholar] [CrossRef]
- Domanin, M.; Gallo, D.; Vergara, C.; Biondetti, P.; Forzenigo, L.V.; Morbiducci, U. Prediction of long term restenosis risk after surgery in the carotid bifurcation by hemodynamic and geometric analysis. Ann. Biomed. Eng. 2019, 47, 1129–1140. [Google Scholar] [CrossRef] [PubMed]
- Du, J.; Wu, G.; Wu, B.; Liu, C.; Mai, Z.; Liu, Y.; Wang, Y.; Zhang, P.; Wu, G.; Liu, J. The Hemodynamic Effect of Enhanced External Counterpulsation Treatment on Atherosclerotic Plaque in the Carotid Artery: A Framework of Patient-Specific Computational Fluid Dynamics Analysis. Cardiol. Res. Pract. 2020, 2020, 5903790. [Google Scholar] [CrossRef] [PubMed]
- Cibis, M.; Potters, W.V.; Selwaness, M.; Gijsen, F.J.; Franco, O.H.; Lorza, A.M.A.; de Bruijne, M.; Hofman, A.; van der Lugt, A.; Nederveen, A.J.; et al. Relation between wall shear stress and carotid artery wall thickening MRI versus CFD. J. Biomech. 2016, 49, 735–741. [Google Scholar] [CrossRef]
- Gharahi, H.; Zambrano, B.A.; Zhu, D.C.; DeMarco, J.K.; Baek, S. Computational fluid dynamic simulation of human carotid artery bifurcation based on anatomy and volumetric blood flow rate measured with magnetic resonance imaging. Int. J. Adv. Eng. Sci. Appl. Math. 2016, 8, 40–60. [Google Scholar] [CrossRef] [Green Version]
- Lee, S.H.; Kang, S.; Hur, N.; Jeong, S.K. A fluid-structure interaction analysis on hemodynamics in carotid artery based on patient-specific clinical data. J. Mech. Sci. Technol. 2012, 26, 3821–3831. [Google Scholar] [CrossRef]
- Qin, Y.; Wu, J.H.; Hu, Q.M.; Ghista, D.N.; Wong, K.K.L. Computational evaluation of smoothed particle hydrodynamics for implementing blood flow modelling through CT reconstructed arteries. J. X-Ray Sci. Technol. 2017, 25, 213–232. [Google Scholar] [CrossRef] [PubMed]
- Shojima, M.; Oshima, M.; Takagi, K.; Torii, R.; Hayakawa, M.; Katada, K.; Morita, A.; Kirino, T. Magnitude and role of wall shear stress on cerebral aneurysm: Computational fluid dynamic study of 20 middle cerebral artery aneurysms. Stroke 2004, 35, 2500–2505. [Google Scholar] [CrossRef] [PubMed]
- Kojima, M.; Irie, K.; Fukuda, T.; Arai, F.; Hirose, Y.; Negoro, M. The study of flow diversion effects on aneurysm using multiple enterprise stents and two flow diverters. Asian J. Neurosurg. 2012, 7, 159. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jung, J.M.; Lee, D.H.; Kim, K.T.; Choi, M.S.; Cho, Y.G.; Lee, H.S.; Choi, S.I.; Lee, S.R.; Kim, D.S. Reference intervals for whole blood viscosity using the analytical performance-evaluated scanning capillary tube viscometer. Clin. Biochem. 2014, 47, 489–493. [Google Scholar] [CrossRef] [PubMed]
- Lee, U.Y.; Chung, G.H.; Jung, J.; Kwak, H.S. Size-Dependent Distribution of Patient-Specific Hemodynamic Factors in Unruptured Cerebral Aneurysms Using Computational Fluid Dynamics. Diagnostics 2020, 10, 64. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Holdsworth, D.W.; Norley, C.J.D.; Frayne, R.; Steinman, D.A.; Rutt, B.K. Characterization of common carotid artery blood-flow waveforms in normal human subjects. Physiol. Meas. 1999, 20, 219–240. [Google Scholar] [CrossRef]
- Zakrzewski, A.M.; Anthony, B.W. Noninvasive Blood Pressure Estimation Using Ultrasound and Simple Finite Element Models. IEEE Trans. Biomed. Eng. 2018, 65, 2011–2022. [Google Scholar] [CrossRef]
- Papaioannou, T.G.; Stefanadis, C. Vascular wall shear stress: Basic principles and methods. Hell. J. Cardiol. 2005, 46, 9–15. [Google Scholar]
- Awad, S.; Allison, S.P.; Lobo, D.N. The history of 0.9% saline. Clin. Nutr. 2008, 27, 179–188. [Google Scholar] [CrossRef]
- Kampmeier, T.; Rehberg, S.; Ertmer, C. Evolution of fluid therapy. Best Pract. Res. Clin. Anaesthesiol. 2014, 28, 207–216. [Google Scholar] [CrossRef]
- Saho, T.; Onishi, H. Quantitative analysis of effects of hemodynamic stress on temporal variations of cardiac phases in models of human carotid bulbs. Radiol. Phys. Technol. 2017, 10, 475–482. [Google Scholar] [CrossRef] [PubMed]
- Long, Q.; Xu, X.Y.; Ariff, B.; Thom, S.A.; Hughes, A.D.; Stanton, A.V. Reconstruction of blood flow patterns in a human carotid bifurcation: A combined CFD and MRI study. J. Magn. Reson. Imaging Off. J. Int. Soc. Magn. Reson. Med. 2000, 11, 299–311. [Google Scholar] [CrossRef]
- Webb, A.R.; Nash, G.B.; Dormandy, J.A.; Bennett, E.D. A Comparison of the Effects of Artificial Plasma Substitutes, Albumin and Saline Solutions on Invitro Apparent Blood-Viscosity. Clin. Hemorheol. 1990, 10, 287–296. [Google Scholar]



| Arteries | Location | Shear Rates | Before | After | p |
|---|---|---|---|---|---|
| Whole Carotid Artery | Left | Minimal shear rate [s−1] | 26.65 ± 4.73 | 28.64 ± 5.50 | 0.161 |
| Time-averaged shear rate [s−1] | 186.96 ± 49.43 | 190.30 ± 46.43 | 0.263 | ||
| Maximal shear rate [s−1] | 919.31 ± 236.19 | 935.52 ± 217.66 | 0.263 | ||
| Right | Minimal shear rate [s−1] | 28.48 ± 4.10 | 32.05 ± 4.45 | 0.036 * | |
| Time-averaged shear rate [s−1] | 212.89 ± 57.16 | 219.49 ± 54.55 | 0.050 | ||
| Maximal shear rate [s−1] | 1043.55 ± 298.34 | 1074.73 ± 283.64 | 0.050 | ||
| Internal Carotid Artery | Left | Minimal shear rate [s−1] | 8.29 ± 2.87 | 8.69 ± 2.25 | 0.327 |
| Time-averaged shear rate [s−1] | 91.87 ± 21.29 | 96.54 ± 20.01 | 0.012 * | ||
| Maximal shear rate [s−1] | 433.66 ± 103.99 | 459.23 ± 106.05 | 0.012 * | ||
| Right | Minimal shear rate [s−1] | 9.70 ± 5.48 | 10.17 ± 4.76 | 0.889 | |
| Time-averaged shear rate [s−1] | 89.66 ± 32.36 | 101.51 ± 41.31 | 0.036 * | ||
| Maximal shear rate [s−1] | 461.30 ± 225.85 | 483.00 ± 233.93 | 0.050 |
| Arteries | Location | Wall Shear Stresses | Before | After | p |
|---|---|---|---|---|---|
| Whole Carotid Artery | Left | Minimal WSS [Pa] | 0.19 ± 0.02 | 0.18 ± 0.02 | 0.024 * |
| Time-averaged WSS [Pa] | 0.92 ± 0.18 | 0.84 ± 0.19 | 0.025 * | ||
| Maximal WSS [Pa] | 4.05 ± 0.81 | 3.74 ± 0.88 | 0.043 * | ||
| Right | Minimal WSS [Pa] | 0.20 ± 0.02 | 0.19 ± 0.02 | 0.020 * | |
| Time-averaged WSS [Pa] | 1.04 ± 0.23 | 0.96 ± 0.22 | 0.043 * | ||
| Maximal WSS [Pa] | 4.58 ± 1.14 | 4.24 ± 1.07 | 0.036 * | ||
| Internal Carotid Artery | Left | Minimal WSS [Pa] | 0.09 ± 0.01 | 0.08 ± 0.02 | 0.034 * |
| Time-averaged WSS [Pa] | 0.50 ± 0.07 | 0.46 ± 0.07 | 0.058 | ||
| Maximal WSS [Pa] | 2.02 ± 0.33 | 1.91 ± 0.35 | 0.237 | ||
| Right | Minimal WSS [Pa] | 0.09 ± 0.03 | 0.08 ± 0.03 | 0.034 * | |
| Time-averaged WSS [Pa] | 0.52 ± 0.18 | 0.48 ± 0.17 | 0.025 * | ||
| Maximal WSS [Pa] | 2.14 ± 0.92 | 2.00 ± 0.88 | 0.025 * |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, U.Y.; Kim, C.I.; Chung, G.H.; Jung, J.; Kwak, H.S. Hemodynamic Changes in the Carotid Artery after Infusion of Normal Saline Using Computational Fluid Dynamics. Diagnostics 2020, 10, 473. https://doi.org/10.3390/diagnostics10070473
Lee UY, Kim CI, Chung GH, Jung J, Kwak HS. Hemodynamic Changes in the Carotid Artery after Infusion of Normal Saline Using Computational Fluid Dynamics. Diagnostics. 2020; 10(7):473. https://doi.org/10.3390/diagnostics10070473
Chicago/Turabian StyleLee, Ui Yun, Chul In Kim, Gyung Ho Chung, Jinmu Jung, and Hyo Sung Kwak. 2020. "Hemodynamic Changes in the Carotid Artery after Infusion of Normal Saline Using Computational Fluid Dynamics" Diagnostics 10, no. 7: 473. https://doi.org/10.3390/diagnostics10070473
APA StyleLee, U. Y., Kim, C. I., Chung, G. H., Jung, J., & Kwak, H. S. (2020). Hemodynamic Changes in the Carotid Artery after Infusion of Normal Saline Using Computational Fluid Dynamics. Diagnostics, 10(7), 473. https://doi.org/10.3390/diagnostics10070473





