Development Modeling of Phormia regina (Diptera: Calliphoridae)
Abstract
Simple Summary
Abstract
1. Introduction
2. Methods and Materials
2.1. Flies
2.2. Incubators
2.3. Experimental Design
2.4. Analysis
- A Gaussian equation (a standard normal curve):
- A modified Gaussian equation (a form of Gaussian curve with a plateau at 100%):
- A cumulative Gaussian equation (a form of the Gaussian curve used for adults to model a sigmoidal increase to a plateau):
- A reversed cumulative Gaussian equation (a form of the cumulative Gaussian equation used for eggs to model a sigmoidal decrease from a plateau):
2.5. Degree Days
- Determine the stage transitions by fitting cumulative Gaussian curves to the proportion of insects entering the new stage vs. time for each temperature (curves were calculated for L1, L2, L2f, L3m, P, and A). Only data for the first portion of each curve (0–100%) were included in the regression, which reflects the stage transition;
- Calculate the 50% transition point from the cumulative Gaussian curve for each stage and temperature combination;
- With data from 2, determine the time in stage by subtraction between 50% transition points;
- Express development times in days (rather than in hours as data were initially determined) and calculate 1/days for each time to transition and stage duration;
- Using linear regression, estimate the relationship between development rate (1/days to transition or stage) vs. temperature to determine the slope and x-intercept. Each resulting regression was run test to identify non-linearity, and where non-linearity was indicated, points were excluded from the regression until any non-linearity was eliminated. Primarily, non-linearity was associated with low and high temperatures (as expected) and indicated in development graphs. The regression of 1/days vs. temperature is conventional in degree-day determination, but the use of run testing to identify non-linear points in the regression has not been. To the best of our knowledge, this approach was first used in [14] to ensure that assumptions underlying degree-day analysis were met;
- From the resulting linear regressions, the x-intercept represents the developmental minimum, and 1/slope represents the accumulated degree days required for an event (stage transition or stage duration) [19]. Although this point usually represents the end of most degree-day determinations, we recognized that it is still possible at this point to have included data in the linear regressions that are not properly part of the linear portion of the development curve. Consequently, we performed additional calculations and corrections to determine the validity of our degree-day models;
- Using regression results, we calculated degree-day accumulations for each experimentally determined combination of temperature and time of transition or stage duration. We then performed a linear regression of these data and evaluated the resulting lines for linearity and slope. To meet the core assumption of degree-day models, a regression of degree-day accumulations must be linear and have no slope. Where our results did not meet these requirements, we removed points (again, at high and low temperatures) and recalculated both the 1/days regression and the accumulated degree-day regressions (steps 5–7). We repeated this process until we arrived at linear relationships meeting all degree-day assumptions and noted the range of temperatures for which the resulting equation was valid.
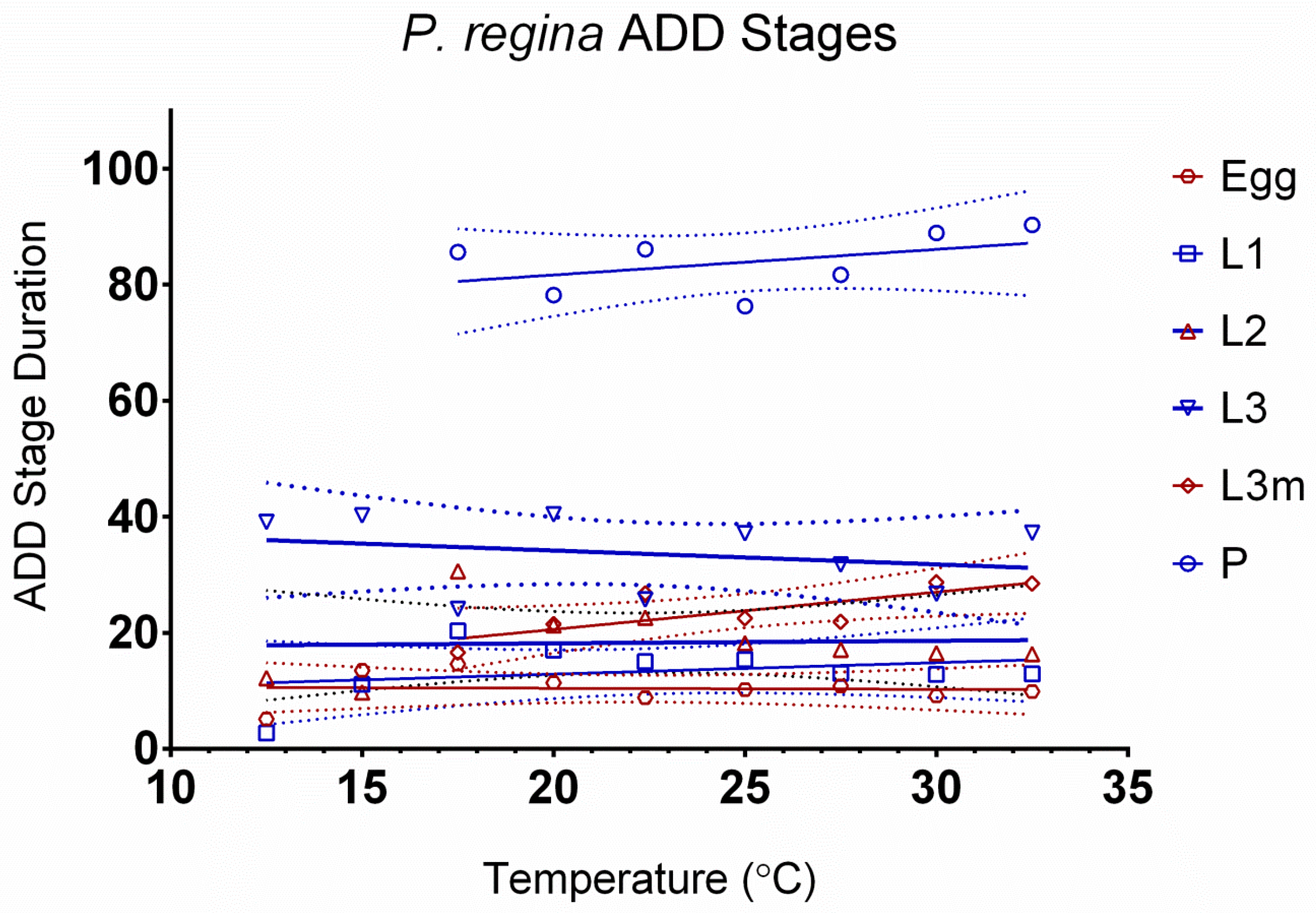
3. Results and Discussion
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Erzinçlioglu, Y.Z. The entomological investigation of a concealed corpse. Med. Sci. Law 1985, 23, 57–63. [Google Scholar] [CrossRef] [PubMed]
- Wells, J.D.; LaMotte, L.R. Estimating maggot age from weight using inverse prediction. J. Forensic Sci. 1995, 40, 585–590. [Google Scholar] [CrossRef]
- Higley, L.G.; Haskell, N.H. Insect development and forensic entomology. In Forensic Entomology: The Utility of Arthropods in Legal Investigations; Byrd, J.H., Castner, J.L., Eds.; CRC Press: Boca Raton, FL, USA, 2010; pp. 389–403. [Google Scholar]
- Roe, A.; Higley, L.G. Development modeling of Lucilia sericata (Diptera: Calliphoridae). PeerJ 2015, 3, e803. [Google Scholar] [CrossRef] [PubMed]
- Roe, A.L.; Higley, L.G. Stage transitions in Lucilia sericata and Phormia regina (Diptera: Calliphoridae) and implications for forensic science. Insects 2023, 14, 315. [Google Scholar] [CrossRef] [PubMed]
- Wells, J.; LaMotte, L. The role of a PMI-Prediction model in evaluating forensic entomology experimental design, the importance of covariates, and the utility of response variables for estimating time since death. Insects 2017, 8, 47. [Google Scholar] [CrossRef]
- LaMotte, L.R.; Roe, A.L.; Wells, J.D.; Higley, L.G. A statistical method to construct confidence sets on carrion insect age from development stage. J. Agric. Biol. Environ. Stat. 2017, 22, 161. [Google Scholar] [CrossRef]
- Hall, D.G. The Blowflies of North America; Thomas Say Foundation: Baltimore, MD, USA, 1948. [Google Scholar]
- Greenberg, B. Flies as forensic indicators. J. Med. Entomol. 1991, 28, 565–577. [Google Scholar] [CrossRef] [PubMed]
- Byrd, J.H.; Castner, J.L. Insects of forensic importance. In Forensic Entomology: The Utility of Arthropods in Legal Investigations, 2nd ed.; Byrd, J.H., Castner, J.L., Eds.; CRC Press: Boca Raton, FL, USA, 2010; pp. 39–126. [Google Scholar]
- Kamal, A.S. Comparative study of thirteen species of sarcosaprophagous Calliphoridae and Sarcophagidae (Diptera) I. Bionomics. Ann. Entomol. Soc. Am. 1958, 51, 261–271. [Google Scholar] [CrossRef]
- Anderson, G.S. Minimum and maximum developmental rates of some forensically important Calliphoridae (Diptera). J. Forensic Sci. 2000, 45, 824–832. [Google Scholar] [CrossRef] [PubMed]
- Byrd, J.H.; Allen, J.C. The development of the black blow fly, Phormia regina (Meigen). Forensic Sci. Int. 2001, 120, 79–88. [Google Scholar] [CrossRef] [PubMed]
- Nabity, P.; Higley, L.; Heng-Moss, T. Effects of temperature on development of Phormia regina (Diptera: Calliphoridae) and use of developmental data in determining time intervals in forensic entomology. J. Med. Entomol. 2006, 43, 1276–1286. [Google Scholar] [CrossRef] [PubMed]
- Carolina Núñez-Vázquez, C.; Tomberlin, J.K.; Cantú-Sifuentes, M.; García-Martínez, O. Laboratory Development and Field Validation of Phormia regina (Diptera: Calliphoridae). J. Med. Entomol. 2013, 50, 252–260. [Google Scholar] [CrossRef] [PubMed]
- Zhang, R.; Hu, G.; Wang, Y.; Li, L.; Guo, Y.; Wang, Y.; Tao, L. Development of Phormia regina at seven constant temperatures for minimum postmortem interval estimation. Int. J. Legal Med. 2024, 138, 1055–1065. [Google Scholar] [CrossRef] [PubMed]
- Richards, C.S.; Villet, M.H. Factors affecting accuracy and precision of thermal summation models of insect development used to estimate post-mortem intervals. Int. J. Legal Med. 2008, 122, 401–408. [Google Scholar] [CrossRef] [PubMed]
- Michaud, J.-P.; Schoenly, K.G.; Moreau, G. Sampling flies or sampling flaws? Experimental design and inference strength in forensic entomology. J. Med. Entomol. 2012, 49, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Lein, M.M. Anoxia Tolerance of Forensically Important Calliphorids. Master’s Thesis, University of Nebraska, Lincoln, NE, USA, 2013. [Google Scholar]
- Nabity, P.; Higley, L.; Heng-Moss, T. Light-induced variability in development of forensically important blow fly Phormia regina (Diptera: Calliphoridae). J. Med. Entomol. 2007, 44, 351–358. [Google Scholar] [CrossRef] [PubMed]
- Arnold, C.Y. The determination and significance of the base temperature in a linear heat unit system. Proc. Am. Soc. Hortic. Sci. 1959, 74, 430–445. [Google Scholar]
- Wagner, T.L.; Wu, H.; Sharpe, P.J.H.; Schoolfield, R.M.; Coulson, R.N. Modeling insect development rates: A literature review and application of a biophysical model. Ann. Entomol. Soc. Am. 1984, 7, 208–225. [Google Scholar] [CrossRef]
- Pornkulwat, S. Molecular Genetic Markers among and within Populations of the European Corn Borer (Lepidoptera: Pyralidae) and Screwworms (Diptera: Calliphoridae). Ph.D. Dissertation, University of Nebraska-Lincoln, Lincoln, NE, USA, 1998. [Google Scholar]
- Kneeland, K.M.; Skoda, S.R.; Foster, J.E. Genetic variability of the stable fly assessed on a global scale using amplified fragment length polymorphism. Insect Sci. 2016, 23, 695–703. [Google Scholar] [CrossRef] [PubMed][Green Version]

| Reference | Locality | Temp. | Analysis | Larval Diet | Stages | L:D Cycle | Replications | Total Maggots/ Sample | Sample Times |
|---|---|---|---|---|---|---|---|---|---|
| [10] | WA U.S. | 27.6 | Mode | Beef liver | E, L1, L2, L3f, L3m, P | Constant | Undef. | Undef. | Undef. |
| [8] | IL, U.S. | 19, 22, 29, 35 | Minimum | Ground beef | E, L1, L2, L3f, L3m, P | Undef. | Undef. | Undef. | Undef. |
| [11] | BC, Canada | 16.1, 23.0 | Minimum and Maximum | Beef liver | E, L1, L2, L3f, L3m, P | Undef. | 3 | 20, returned to jar | Eggs-1 to 2 h L1, L2- 3 to 4 times/day Later stages-2 to 3 times/day |
| [12] | FL, USA | 10, 15, 20, 25, 30, 35, 40 | Mean, mode | Lean pork | E, L1, L2, L3f, L3m, P | Egg-constant Larvae-12:12 Pupae- constant | Egg-3 Larvae-6, with 3 subsamples in each | 6 from each subsample (= 108/sample) | Egg-30 min Larvae-12 h Onset of adult emergence-every 30 min |
| [13] | NE, U.S. | 12, 14, 20, 26, 32 (2001) 12, 15, 20, 25, 30 (2004) | Mean | Ground beef, beef liver | E to P, E to A | 16:8 (2001) 24:0 (2004) | 26, 32-4 8, 10, 14, 20-2 12-1 (2001) All temps-4 (2004) | Undef. | 12 h |
| Temperature (°C) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Life Stage | 7.5 | 10.0 | 12.5 | 15.0 | 17.5 | 20.0 | 22.5 | 25.0 | 27.5 | 30.0 | 32.5 |
| Egg–1st | 16 | 16 | 16 | 8 | 5 | 4 | 3 | 3 | 2 | 2 | 2 |
| 1st–2nd | 44 | 44 | 44 | 22 | 15 | 11 | 9 | 7 | 6 | 6 | 5 |
| 2nd–3f | 63 | 63 | 63 | 31 | 21 | 16 | 13 | 10 | 9 | 8 | 7 |
| 3f–3m | 111 | 111 | 111 | 55 | 37 | 28 | 22 | 18 | 16 | 14 | 12 |
| 3m–Pupal | 281 | 281 | 281 | 141 | 94 | 70 | 56 | 47 | 40 | 35 | 31 |
| Pupal–Adult | 441 | 441 | 441 | 221 | 147 | 110 | 88 | 74 | 63 | 55 | 49 |
| % Time in Stage | ||||||
|---|---|---|---|---|---|---|
| Temp | Egg | L1 | L2 | L3f | L3m | P |
| 10.4 | N/A | N/A | N/A | N/A | N/A | N/A |
| 12.7 | 4.8 | 19.6 | 14.5 | 3.3 | 15.9 | 41.8 |
| 15.1 | 6.4 | 9.6 | 7.9 | 22.3 | 2.3 | 51.5 |
| 17.5 | 6.4 | 14.0 | 14.6 | 9.6 | 6.6 | 48.8 |
| 20.1 | 5.4 | 11.3 | 10.8 | 17.8 | 9.5 | 45.3 |
| 22.5 | 4.4 | 9.6 | 11.7 | 11.9 | 12.4 | 49.9 |
| 25.0 | 5.3 | 9.9 | 9.9 | 18.3 | 11.1 | 45.4 |
| 27.5 | 5.8 | 8.4 | 9.5 | 16.2 | 11.2 | 49.0 |
| 30.0 | 4.7 | 7.8 | 8.9 | 13.3 | 14.3 | 51.0 |
| 32.5 | 4.9 | 7.3 | 8.2 | 17.6 | 13.5 | 48.5 |
| Mean | 5.3 | 10.8 | 10.7 | 14.5 | 10.8 | 47.9 |
| Temp (mean) | Transition ADD by 1/Day | Stage ADD by 1/Days | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| E-L1 | E-L2 | E-L3f | E-L3m | E-P | E-A | Egg | L1 | L2 | L3f | L3m | P | |
| 10.2 | ||||||||||||
| 12.5 | ||||||||||||
| 15.0 | 8.9 | 13.7 | 21.3 | 58.8 | 8.9 | 6.0 | 7.9 | |||||
| 17.5 | 10.0 | 24.6 | 42.8 | 65.6 | 94.0 | 191.2 | 10.0 | 14.7 | 18.9 | 23.3 | 26.2 | 89.2 |
| 20.0 | 8.6 | 22.2 | 37.0 | 69.0 | 97.8 | 184.1 | 8.6 | 13.8 | 15.0 | 39.3 | 31.4 | 80.5 |
| 22.4 | 7.0 | 19.7 | 36.6 | 58.8 | 88.7 | 181.5 | 7.0 | 12.7 | 17.2 | 25.1 | 37.1 | 88.2 |
| 25.0 | 8.5 | 21.9 | 36.2 | 67.5 | 93.9 | 175.3 | 8.5 | 13.5 | 14.5 | 36.4 | 29.9 | 77.8 |
| 27.5 | 9.2 | 20.6 | 34.6 | 62.1 | 86.7 | 172.8 | 9.2 | 11.7 | 14.2 | 31.2 | 28.1 | 83.0 |
| 30.0 | 7.9 | 19.5 | 33.4 | 57.1 | 86.8 | 179.9 | 7.9 | 11.7 | 14.0 | 26.3 | 35.9 | 90.2 |
| 32.5 | 8.8 | 20.5 | 34.5 | 67.3 | 8.8 | 11.9 | 14.1 | 91.5 | ||||
| Linear Regression Results | ||||||||||||
| Dev Min | 11.5 | 12.9 | 12.8 | 11.9 | 10.5 | 10.2 | 11.5 | 13.5 | 12.5 | 8.2 | 2.3 | 10.5 |
| ADD | 8.4 | 20.4 | 34.7 | 62.9 | 91.4 | 181.9 | 8.4 | 12.1 | 14.4 | 29.3 | 30.2 | 84.9 |
| r2 | 0.96 | 0.99 | 0.99 | 0.97 | 0.98 | 0.99 | 0.96 | 0.98 | 0.97 | 0.74 | 0.72 | 0.98 |
| n | 8 | 8 | 8 | 8 | 6 | 6 | 8 | 8 | 8 | 6 | 6 | 7 |
| ADD Range min (°C) | 15.0 | 15.0 | 15.0 | 15.0 | 15.0 | 15.0 | 15.0 | 15.0 | 15.0 | 17.5 | 17.5 | 15.0 |
| ADD Range max (°C) | 32.5 | 32.5 | 32.5 | 32.5 | 32.5 | 32.5 | 32.5 | 32.5 | 32.5 | 30.0 | 27.5 | 32.5 |
| Calculated ADD mean | 8.6 | 20.3 | 34.6 | 63.3 | 91.3 | 180.8 | 8.6 | 12.0 | 14.5 | 30.2 | 31.4 | 85.8 |
| SE | 0.8 | 2.9 | 5.7 | 4.4 | 4.2 | 6.0 | 0.8 | 2.5 | 3.0 | 5.9 | 4.0 | 4.9 |
| Regression ADD | 8 | 20 | 35 | 63 | 91 | 182 | 8 | 12 | 14 | 29 | 30 | 85 |
| % deviation (calculated vs. regression ADD) | 2.1% | −0.5% | −0.4% | 0.6% | −0.1% | −0.6% | 2.1% | −1.0% | 0.7% | 3.1% | 4.2% | 1.0% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roe, A.; Wells, J.D.; Higley, L.G. Development Modeling of Phormia regina (Diptera: Calliphoridae). Insects 2024, 15, 550. https://doi.org/10.3390/insects15070550
Roe A, Wells JD, Higley LG. Development Modeling of Phormia regina (Diptera: Calliphoridae). Insects. 2024; 15(7):550. https://doi.org/10.3390/insects15070550
Chicago/Turabian StyleRoe, Amanda, Jeffrey D. Wells, and Leon G. Higley. 2024. "Development Modeling of Phormia regina (Diptera: Calliphoridae)" Insects 15, no. 7: 550. https://doi.org/10.3390/insects15070550
APA StyleRoe, A., Wells, J. D., & Higley, L. G. (2024). Development Modeling of Phormia regina (Diptera: Calliphoridae). Insects, 15(7), 550. https://doi.org/10.3390/insects15070550







