Preliminary Scale of Reference Values for Evaluating Reactive Strength Index-Modified in Male and Female NCAA Division I Athletes
Abstract
:1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Data Collection
2.3. Data Analysis
2.4. Statistical Analyses
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hoffman, J.R. Norms for Fitness, Performance, and Health; Human Kinetics: Champlain, IL, USA, 2006; ISBN-13: 9780736054836. [Google Scholar]
- McMahon, J.J.; Suchomel, T.J.; Lake, J.P.; Comfort, P. Understanding the key phases of the countermovement jump force-time curve. Strength Cond. J. 2018, 40, 96–106. [Google Scholar] [CrossRef]
- Linthorne, N. Analysis of standing vertical jumps using a force platform. Am. J. Phys. 2001, 69, 1198–1204. [Google Scholar] [CrossRef] [Green Version]
- Lake, J.; Mundy, P.; Comfort, P.; McMahon, J.J.; Suchomel, T.J.; Carden, P. Concurrent validity of a portable force plate using vertical jump force-time characteristics. J. Appl. Biomech. 2018, 34, 410–413. [Google Scholar] [CrossRef] [PubMed]
- Walsh, M.S.; Ford, K.R.; Bangen, K.J.; Myer, G.D.; Hewett, T.E. The validation of a portable force plate for measuring force-time data during jumping and landing tasks. J. Strength Cond. Res. 2006, 20, 730–734. [Google Scholar] [PubMed]
- Loturco, I.; Pereira, L.; Kobal, R.; Cal Abad, C.; Fernandes, V.; Ramirez-Campillo, R.; Suchomel, T.J. Portable force plates: A viable and practical alternative to rapidly and accurately monitor elite sprint performance. Sports 2018, 6, 61. [Google Scholar] [CrossRef] [PubMed]
- Peterson Silveira, R.; Stergiou, P.; Carpes, F.P.; Castro, F.A.d.S.; Katz, L.; Stefanyshyn, D.J. Validity of a portable force platform for assessing biomechanical parameters in three different tasks. Sports Biomech. 2017, 16, 177–186. [Google Scholar] [CrossRef] [PubMed]
- Ebben, W.P.; Petushek, E.J. Using the reactive strength index modified to evaluate plyometric performance. J. Strength Cond. Res. 2010, 24, 1983–1987. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Bailey, C.A.; Sole, C.J.; Grazer, J.L.; Beckham, G.K. Using reactive strength index-modified as an explosive performance measurement tool in division I athletes. J. Strength Cond. Res. 2015, 29, 899–904. [Google Scholar] [CrossRef] [PubMed]
- Kipp, K.; Kiely, M.T.; Geiser, C.F. Reactive strength index modified is a valid measure of explosiveness in collegiate female volleyball players. J. Strength Cond. Res. 2016, 30, 1341–1347. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Jones, P.A.; Suchomel, T.J.; Lake, J.; Comfort, P. Influence of the reactive strength index modified on force-and power-time curves. Int. J. Sports Physiol. Perform. 2017, 13, 220–227. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Lake, J.P.; Comfort, P. Reliability of and relationship between flight time to contraction time ratio and reactive strength index modified. Sports 2018, 6, 81. [Google Scholar] [CrossRef] [PubMed]
- Nikolaidis, P. Age-related differences in countermovement vertical jump in soccer players 8–31 years old: The role of fat-free mass. Am. J. Sports Sci. Med. 2014, 2, 60–64. [Google Scholar]
- Patterson, D.D.; Peterson, D.F. Vertical jump and leg power norms for young adults. Meas. Phys. Educ. Exerc. Sci. 2004, 8, 33–41. [Google Scholar] [CrossRef]
- Castagna, C.; Castellini, E. Vertical jump performance in Italian male and female national team soccer players. J. Strength Cond. Res. 2013, 27, 1156–1161. [Google Scholar] [CrossRef] [PubMed]
- Gabbett, T.J. Physiological characteristics of junior and senior rugby league players. Br. J. Sport Med. 2002, 36, 334–339. [Google Scholar] [CrossRef] [Green Version]
- Sole, C.J.; Kavanaugh, A.A.; Reed, J.P.; Israetel, M.A.; Devine, L.E.; Ramsey, M.W.; Sands, W.A.; Stone, M.H. The sport performance enhancement group: A five-year analysis of interdisciplinary athlete development. In Proceedings of the 8th Annual Center of Excellence for Sport Science and Coach Education Coaches and Sport Science College, Johnson City, TN, USA, 13–14 December 2013. [Google Scholar]
- Kraska, J.M.; Ramsey, M.W.; Haff, G.G.; Fethke, N.; Sands, W.A.; Stone, M.E.; Stone, M.H. Relationship between strength characteristics and unweighted and weighted vertical jump height. Int. J. Sports Physiol. Perform. 2009, 4, 461–473. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Sole, C.J.; Stone, M.H. Comparison of methods that assess lower-body stretch-shortening cycle utilization. J. Strength Cond. Res. 2016, 30, 547–554. [Google Scholar] [CrossRef] [PubMed]
- Moir, G.L. Three different methods of calculating vertical jump height from force platform data in men and women. Meas. Phys. Educ. Exerc. Sci. 2008, 12, 207–218. [Google Scholar] [CrossRef]
- Henry, F.M. “Best” versus “average” individual scores. Res Q. Exerc. Sport 1967, 38, 317–320. [Google Scholar] [CrossRef]
- Kennedy, R.A.; Drake, D. Improving the signal-to-noise ratio when monitoring countermovement jump performance. J. Strength Cond. Res. 2018, in press. [Google Scholar] [CrossRef] [PubMed]
- Hoaglin, D.C.; Iglewicz, B. Fine-tuning some resistant rules for outlier labeling. J. Am. Stat. Assoc. 1987, 82, 1147–1149. [Google Scholar] [CrossRef]
- Hoaglin, D.C.; Iglewicz, B.; Tukey, J.W. Performance of some resistant rules for outlier labeling. J. Am. Stat. Assoc. 1986, 81, 991–999. [Google Scholar] [CrossRef]
- A New View of Statistics. Available online: http://www.sportsci.org/resource/stats/effectmag.html (accessed on 20 September 2018).
- Sole, C.J.; Mizuguchi, S.; Sato, K.; Moir, G.L.; Stone, M.H. Phase characteristics of the countermovement jump force-time curve: A comparison of athletes by jumping ability. J. Strength Cond. Res. 2018, 32, 1155–1165. [Google Scholar] [CrossRef] [PubMed]
- Laffaye, G.; Wagner, P.P.; Tombleson, T.I.L. Countermovement jump height: Gender and sport-specific differences in the force-time variables. J. Strength Cond. Res. 2014, 28, 1096–1105. [Google Scholar] [CrossRef] [PubMed]
- Beckham, G.K.; Suchomel, T.J.; Bailey, C.A.; Sole, C.J.; Grazer, J.L. The relationship of the reactive strength index-modified and measures of force development in the isometric mid-thigh pull. In Proceedings of the XXXIInd International Conference of Biomechanics in Sports, Johnson City,TN, USA, 12–16 July 2014. [Google Scholar]
- Beckham, G.K.; Suchomel, T.J.; Sole, C.J.; Bailey, C.A.; Grazer, J.L.; Kim, S.B.; Talbot, K.B.; Stone, M.H. Influence of sex and maximum strength on reactive strength index-modified. In Proceedings of the National Strength and Conditioning Association’s 41st Annual Meeting, Indianapolis, IN, USA, 11–14 July 2018. [Google Scholar]
- McMahon, J.J.; Rej, S.J.; Comfort, P. Sex differences in countermovement jump phase characteristics. Sports 2017, 5, 8. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Nimphius, S.; Stone, M.H. The importance of muscular strength in athletic performance. Sports Med. 2016, 46, 1419–1449. [Google Scholar] [CrossRef] [PubMed]
- Kipp, K.; Kiely, M.T.; Giordanelli, M.D.; Malloy, P.J.; Geiser, C.F. Biomechanical determinants of the reactive strength index during drop jumps. Int. J. Sports Physiol. Perform. 2018, 13, 44–49. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Suchomel, T.J.; Lake, J.P.; Comfort, P. Relationship between reactive strength index variants in rugby league players. J. Strength Cond Res. 2018, in press. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Sole, C.J.; Bailey, C.A.; Grazer, J.L.; Beckham, G.K. A comparison of reactive strength index-modified between six U.S. Collegiate athletic teams. J. Strength Cond. Res. 2015, 29, 1310–1316. [Google Scholar] [CrossRef] [PubMed]
- Bailey, C.A.; Suchomel, T.J.; Beckham, G.K.; Sole, C.J.; Grazer, J.L. A comparison of baseball positional differences with reactive strength index-modified. In Proceedings of the XXXIInd International Conference of Biomechanics in Sports, Johnson City, TN, 12–16 July 2014. [Google Scholar]
- Street, G.; McMillan, S.; Board, W.; Rasmussen, M.; Heneghan, J.M. Sources of error in determining countermovement jump height with the impulse method. J. Appl. Biomech. 2001, 17, 43–54. [Google Scholar] [CrossRef]
- Owen, N.J.; Watkins, J.; Kilduff, L.P.; Bevan, H.R.; Bennett, M.A. Development of a criterion method to determine peak mechanical power output in a countermovement jump. J. Strength Cond. Res. 2014, 28, 1552–1558. [Google Scholar] [CrossRef] [PubMed]
| Male (n = 76) | Female (n = 75) | |
|---|---|---|
| RSImod (m/s) | 0.424 ± 0.102 | 0.314 ± 0.089 |
| JH (m) | 0.36 ± 0.07 | 0.27 ± 0.06 |
| TTT (s) | 0.868 ± 0.105 | 0.870 ± 0.114 |
| Percentile | RSImod (m/s) |
|---|---|
| 97 | 0.630 |
| 95 | 0.604 |
| 90 | 0.547 |
| 85 | 0.523 |
| 80 | 0.508 |
| 75 | 0.492 |
| 70 | 0.487 |
| 65 | 0.476 |
| 60 | 0.461 |
| 55 | 0.448 |
| 50 | 0.419 |
| 45 | 0.398 |
| 40 | 0.376 |
| 35 | 0.369 |
| 30 | 0.366 |
| 25 | 0.352 |
| 20 | 0.331 |
| 15 | 0.316 |
| 10 | 0.308 |
| 5 | 0.257 |
| 3 | 0.216 |
| Percentile | RSImod (m/s) |
|---|---|
| 97 | 0.497 |
| 95 | 0.461 |
| 90 | 0.434 |
| 85 | 0.413 |
| 80 | 0.391 |
| 75 | 0.379 |
| 70 | 0.366 |
| 65 | 0.351 |
| 60 | 0.333 |
| 55 | 0.315 |
| 50 | 0.308 |
| 45 | 0.293 |
| 40 | 0.279 |
| 35 | 0.273 |
| 30 | 0.266 |
| 25 | 0.248 |
| 20 | 0.241 |
| 15 | 0.214 |
| 10 | 0.202 |
| 5 | 0.173 |
| 3 | 0.139 |
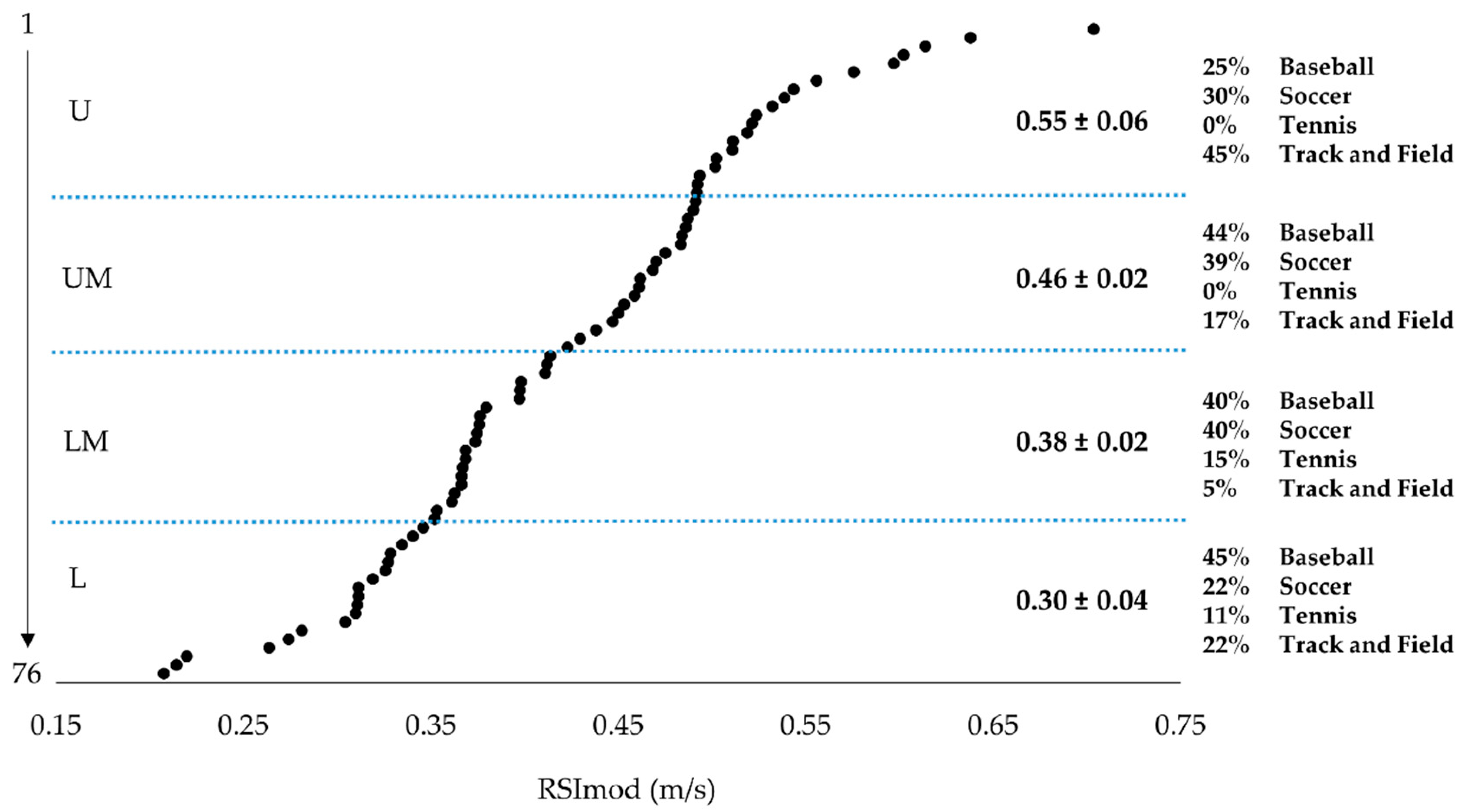
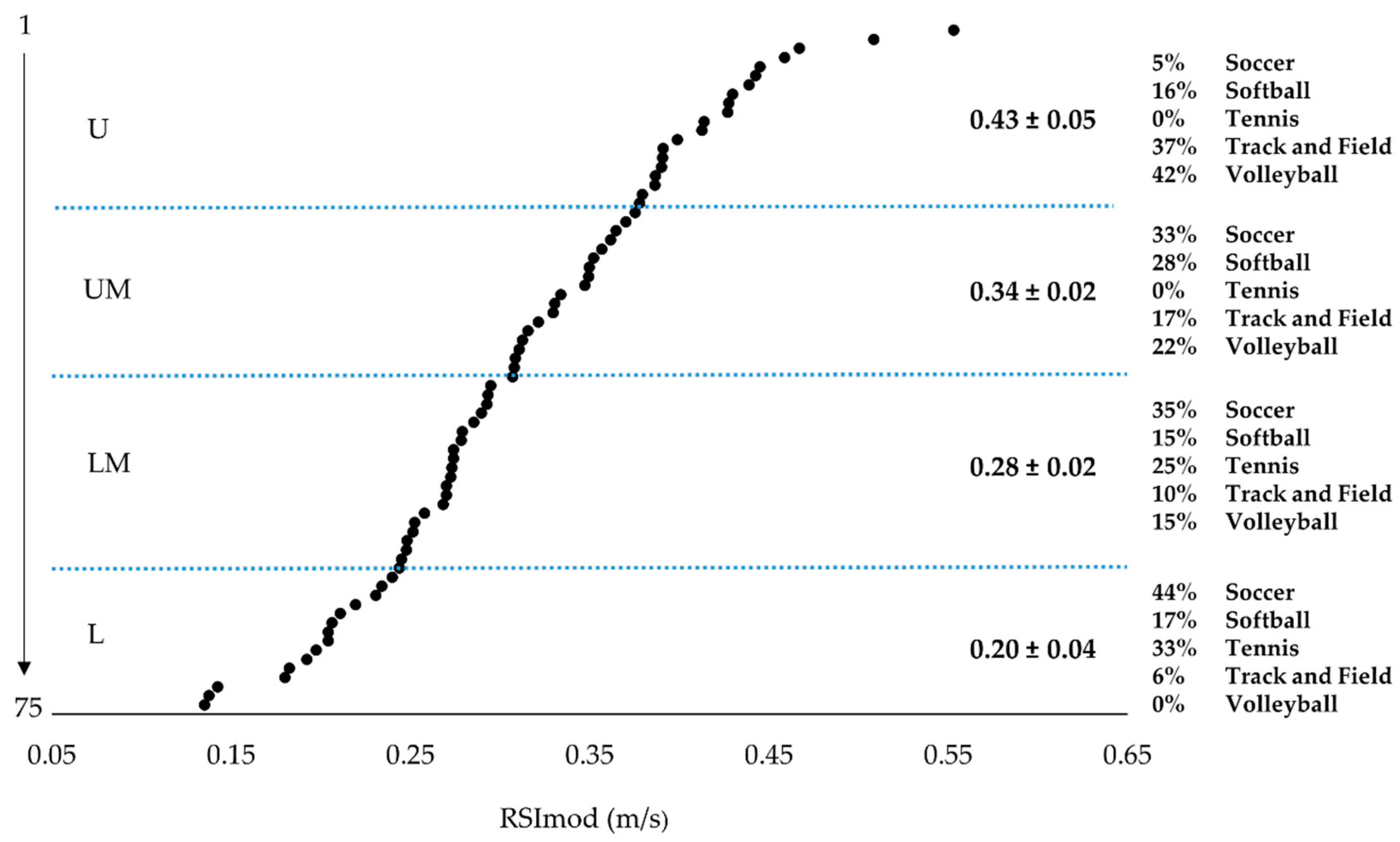
| Male Performance Group | n | RSImod (m/s) | JH (m) | TTT (s) | Female Perfomance Group | n | RSImod (m/s) | JH (m) | TTT (s) |
|---|---|---|---|---|---|---|---|---|---|
| U | 20 | 0.549 ± 0.057 | 0.43 ± 0.05 | 0.795 ± 0.087 | U | 19 | 0.429 ± 0.045 | 0.34 ± 0.03 | 0.792 ± 0.077 |
| UM | 18 | 0.464 ± 0.021 | 0.39 ± 0.04 | 0.850 ± 0.067 | UM | 18 | 0.343 ± 0.023 | 0.29 ± 0.04 | 0.848 ± 0.117 |
| LM | 20 | 0.379 ± 0.019 | 0.33 ± 0.04 | 0.871 ± 0.100 | LM | 20 | 0.277 ± 0.017 | 0.24 ± 0.03 | 0.880 ± 0.092 |
| L | 18 | 0.296 ± 0.044 | 0.28 ± 0.05 | 0.964 ± 0.090 | L | 18 | 0.203 ± 0.036 | 0.19 ± 0.03 | 0.964 ± 0.100 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sole, C.J.; Suchomel, T.J.; Stone, M.H. Preliminary Scale of Reference Values for Evaluating Reactive Strength Index-Modified in Male and Female NCAA Division I Athletes. Sports 2018, 6, 133. https://doi.org/10.3390/sports6040133
Sole CJ, Suchomel TJ, Stone MH. Preliminary Scale of Reference Values for Evaluating Reactive Strength Index-Modified in Male and Female NCAA Division I Athletes. Sports. 2018; 6(4):133. https://doi.org/10.3390/sports6040133
Chicago/Turabian StyleSole, Christopher J., Timothy J. Suchomel, and Michael H. Stone. 2018. "Preliminary Scale of Reference Values for Evaluating Reactive Strength Index-Modified in Male and Female NCAA Division I Athletes" Sports 6, no. 4: 133. https://doi.org/10.3390/sports6040133





