A Full-Field Crystal Plasticity Study on the Bauschinger Effect Caused by Non-Shearable Particles and Voids in Aluminium Single Crystals
Abstract
:1. Introduction
2. Crystal Plasticity Constitutive Model
2.1. The Constitutive Model without a Back stress
2.2. The Constitutive Model with a Back stress
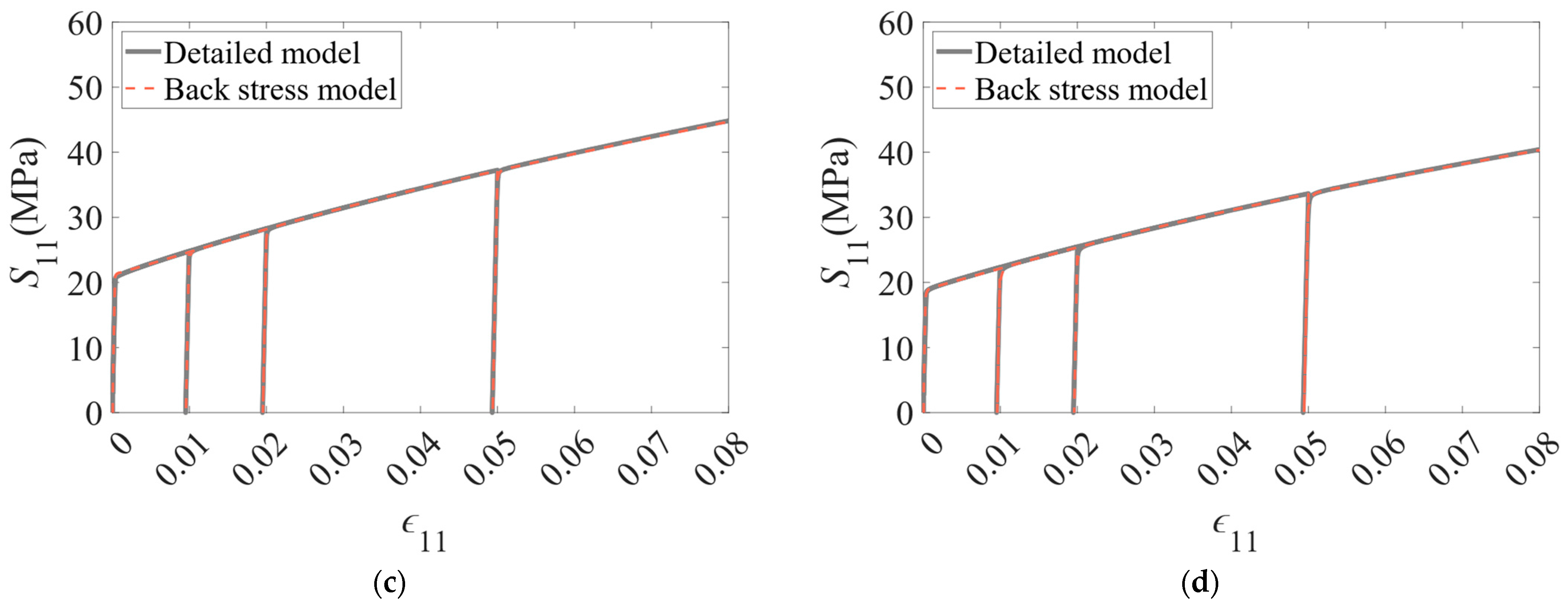
3. Results
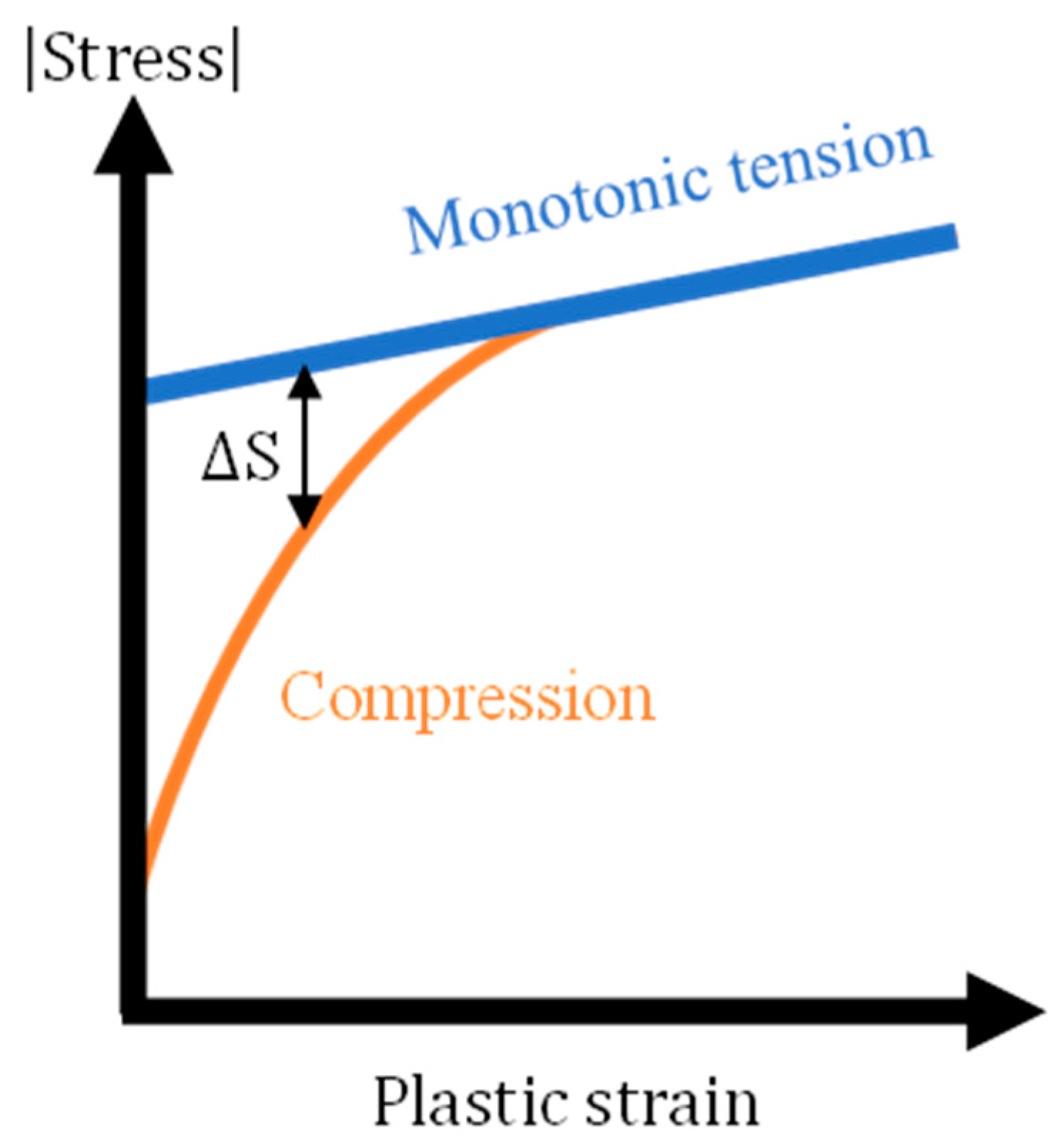
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hatano, T.; Matsui, H. Molecular dynamics investigation of dislocation pinning by a nanovoid in copper. Phys. Rev. B 2005, 72, 094105. [Google Scholar] [CrossRef]
- Santos-Güemes, R.; LLorca, J. Dislocation dynamics analysis of the strengthening induced by shearable and non-shearable precipitates in the presence of dislocation pile-ups. Eur. J. Mech.-A/Solids, 2023; in press. [Google Scholar]
- Ashby, M. The deformation of plastically non-homogeneous materials. Philos. Mag. A J. Theor. Exp. Appl. Phys. 1970, 21, 399–424. [Google Scholar] [CrossRef]
- Atkinson, J.D.; Brown, L.M.; Stobbs, W.M. Work-Hardening of Copper-Silica. 4. Bauschinger Effect and Plastic Relaxation. Philos. Mag. 1974, 30, 1247–1280. [Google Scholar] [CrossRef]
- Brown, L.; Clarke, D. Work hardening due to internal stresses in composite materials. Acta Metall. 1975, 23, 821–830. [Google Scholar] [CrossRef]
- Brown, L.M.; Stobbs, W.M. Work-Hardening of Copper-Silica. 2. Role of Plastic Relaxation. Philos. Mag. 1971, 23, 1201–1233. [Google Scholar] [CrossRef]
- Brown, L.M.; Stobbs, W.M. Work-Hardening of Copper-Silica. Philos. Mag. 1971, 23, 1185–1199. [Google Scholar] [CrossRef]
- Cahoreau, M.; Humphreys, F.J. The Rotation of Particles during the Deformation of a 2-Phase Copper Alloy. Acta Metall. 1984, 32, 1365–1370. [Google Scholar] [CrossRef]
- Hirsch, P.B.; Humphreys, F.J. Deformation of Single Crystals of Copper and Copper-Zinc Alloys Containing Alumina Particles. 1. Macroscopic Properties and Workhardening Theory. Proc. R. Soc. Lond. A 1970, 318, 45–72. [Google Scholar] [CrossRef]
- Humphreys, F.J.; Hirsch, P.B. Deformation of Single Crystals of Copper and Copper-Zinc Alloys Containing Alumina Particles. 2. Microstructure and Dislocation-Particle Interactions. Proc. R. Soc. Lond. A 1970, 318, 73–92. [Google Scholar] [CrossRef]
- Moan, G.; Embury, J. A study of the Bauschinger effect in Al-Cu alloys. Acta Metall. 1979, 27, 903–914. [Google Scholar] [CrossRef]
- Stobbs, W.M. Work-Hardening of Copper-Silica. 3. Diffusional Stress Relaxation. Philos. Mag. 1973, 27, 1073–1092. [Google Scholar] [CrossRef]
- Brown, L.M.; Stobbs, W.M. Work-Hardening of Copper-Silica. 5. Equilibrium Plastic Relaxation by Secondary Dislocations. Philos. Mag. 1976, 34, 351–372. [Google Scholar] [CrossRef]
- Bate, P. Modelling deformation microstructure with the crystal plasticity finite-element method. Philos. Trans. R. Soc. A 1999, 357, 1589–1601. [Google Scholar] [CrossRef]
- Gurtin, M.E. A finite-deformation, gradient theory of single-crystal plasticity with free energy dependent on the accumulation of geometrically necessary dislocations. Int. J. Plast. 2010, 26, 1073–1096. [Google Scholar] [CrossRef]
- Lee, W.M.; Zikry, M.A. Modeling the interfacial plastic strain incompatibilities associated with dispersed particles in high strength aluminum alloys. Acta Mater. 2012, 60, 1669–1679. [Google Scholar] [CrossRef]
- Fribourg, G.; Brechet, Y.; Deschamps, A.; Simar, A. Microstructure-based modelling of isotropic and kinematic strain hardening in a precipitation-hardened aluminium alloy. Acta Mater. 2011, 59, 3621–3635. [Google Scholar] [CrossRef]
- Okuyama, Y.; Tanaka, M.; Ohashi, T.; Morikawa, T. Modelling and Crystal Plasticity Analysis for the Mechanical Response of Alloys with Non-uniformly Distributed Secondary Particles. ISIJ Int. 2020, 60, 1819–1828. [Google Scholar] [CrossRef]
- Zhao, Q.L.; Holmedal, B. Modelling work hardening of aluminium alloys containing dispersoids. Philos. Mag. 2013, 93, 3142–3153. [Google Scholar] [CrossRef]
- Zhao, Q.L.; Holmedal, B. The effect of silicon on the strengthening and work hardening of aluminum at room temperature. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2013, 563, 147–151. [Google Scholar] [CrossRef]
- Bate, P.; Roberts, W.T.; Wilson, D.V. The Plastic Anisotropy of 2-Phase Aluminum-Alloys. 2. Anisotropic Behavior in Load-Reversal Tests. Acta Metall. 1982, 30, 725–737. [Google Scholar] [CrossRef]
- Han, C.S.; Wagoner, R.H.; Barlat, F. On precipitate induced hardening in crystal plasticity: Theory. Int. J. Plast. 2004, 20, 477–494. [Google Scholar] [CrossRef]
- Han, C.S.; Wagoner, R.H.; Barlat, F. On precipitate induced hardening in crystal plasticity: Algorithms and simulations. Int. J. Plast. 2004, 20, 1441–1461. [Google Scholar] [CrossRef]
- Asgharzadeh, A.; Park, T.; Nazari Tiji, S.; Pourboghrat, F. A Computationally Efficient Multiscale, Multi-Phase Modeling Approach Based on CPFEM to Assess the Effect of Second Phase Particles on Mechanical Properties. Crystals 2023, 13, 1199. [Google Scholar] [CrossRef]
- Umar, M.; Qayyum, F.; Farooq, M.U.; Guk, S.; Kirschner, M.; Korpala, G.; Prahl, U. Exploring the Structure-Property Relationship in Spheroidized C45EC Steel Using Full Phase Crystal Plasticity Numerical Simulations. Steel Res. Int. 2022, 93, 2100452. [Google Scholar] [CrossRef]
- Qayyum, F.; Guk, S.; Kawalla, R.; Prahl, U. On Attempting to Create a Virtual Laboratory for Application-Oriented Microstructural Optimization of Multi-Phase Materials. Appl. Sci. 2021, 11, 1506. [Google Scholar] [CrossRef]
- Ali, M.; Qayyum, F.; Tseng, S.; Guk, S.; Overhagen, C.; Chao, C.K.; Prahl, U. Development of Hot Working Process Maps for Incompressible TRIP Steel and Zirconia Composites Using Crystal Plasticity-Based Numerical Simulations. Metals 2022, 12, 2174. [Google Scholar] [CrossRef]
- Sedighiani, K.; Shah, V.; Traka, K.; Diehl, M.; Roters, F.; Sietsma, J.; Raabe, D. Large-deformation crystal plasticity simulation of microstructure and microtexture evolution through adaptive remeshing. Int. J. Plast. 2021, 146, 103078. [Google Scholar] [CrossRef]
- Roters, F.; Diehl, M.; Shanthraj, P.; Eisenlohr, P.; Reuber, C.; Wong, S.L.; Maiti, T.; Ebrahimi, A.; Hochrainer, T.; Fabritius, H.-O. DAMASK–The Düsseldorf Advanced Material Simulation Kit for modeling multi-physics crystal plasticity, thermal, and damage phenomena from the single crystal up to the component scale. Comput. Mater. Sci. 2019, 158, 420–478. [Google Scholar] [CrossRef]
- Groeber, M.A.; Jackson, M.A. DREAM.3D: A Digital Representation Environment for the Analysis of Microstructure in 3D. Integr. Mater. Manuf. Innov. 2014, 3, 56–72. [Google Scholar] [CrossRef]
- Eisenlohr, P.; Diehl, M.; Lebensohn, R.A.; Roters, F. A spectral method solution to crystal elasto-viscoplasticity at finite strains. Int. J. Plast. 2013, 46, 37–53. [Google Scholar] [CrossRef]
- Wollmershauser, J.; Clausen, B.; Agnew, S. A slip system-based kinematic hardening model application to in situ neutron diffraction of cyclic deformation of austenitic stainless steel. Int. J. Fatigue 2012, 36, 181–193. [Google Scholar] [CrossRef]
- Själander, M.; Jahre, M.; Tufte, G.; Reissmann, N. EPIC: An energy-efficient, high-performance GPGPU computing research infrastructure. arXiv 2019, arXiv:1912.05848. [Google Scholar]


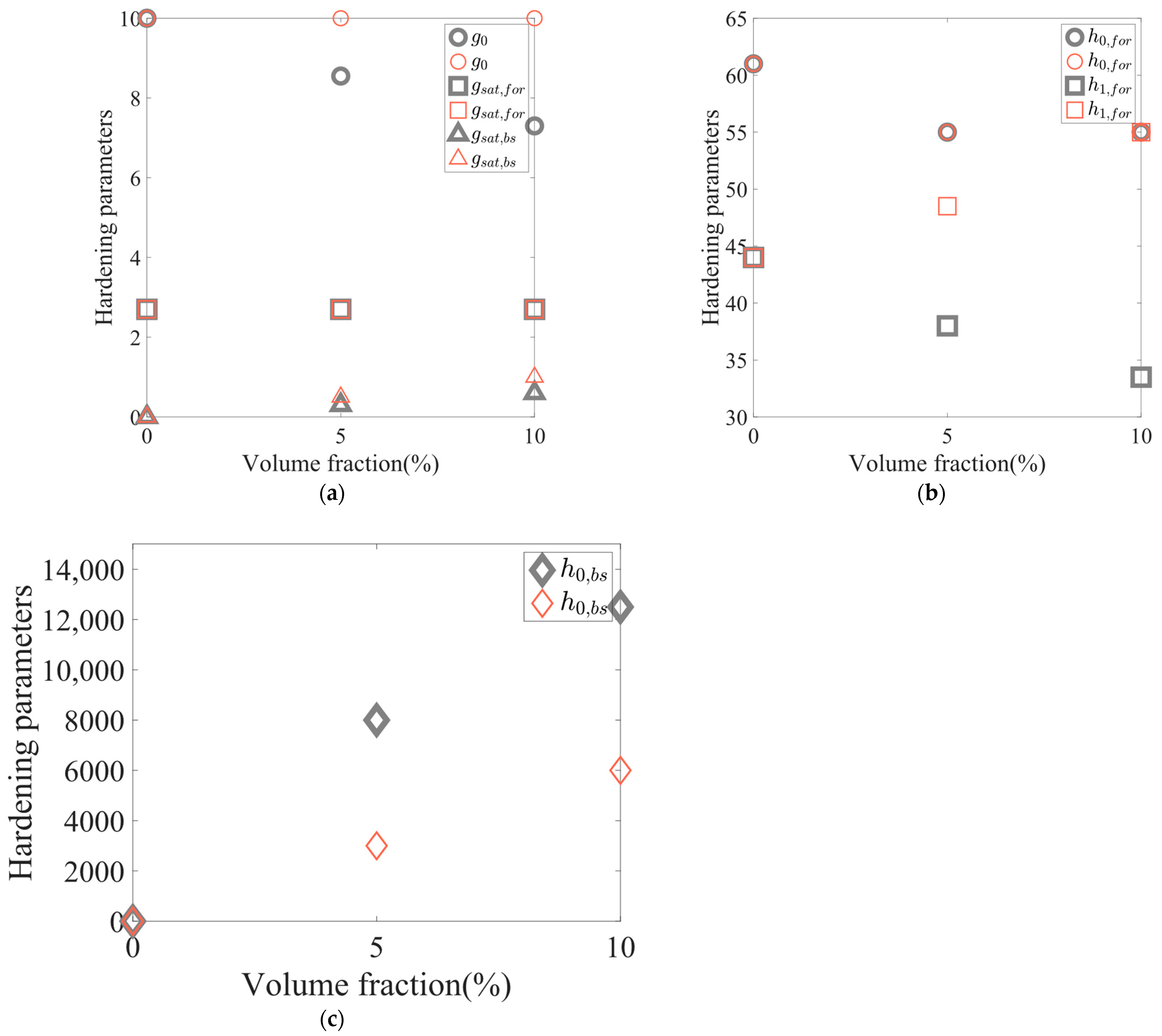


| Aluminium Matrix | Void | Void | Particle | Particle | |
|---|---|---|---|---|---|
| VF = 0% | VF = 5% | VF = 10% | VF = 5% | VF = 10% | |
| 10 | 8.55 | 7.3 | 10 | 10 | |
| 2.7 | 2.7 | 2.7 | 2.7 | 2.7 | |
| 0 | 0.3 | 0.6 | 0.5 | 1 | |
| 61 | 55 | 55 | 55 | 55 | |
| 44 | 38 | 33.5 | 48.5 | 55 | |
| 0 | 8000 | 12,500 | 3000 | 6000 | |
| 0 | 0 | 0 | 0 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aria, A.I.; Holmedal, B.; Mánik, T.; Marthinsen, K. A Full-Field Crystal Plasticity Study on the Bauschinger Effect Caused by Non-Shearable Particles and Voids in Aluminium Single Crystals. Metals 2024, 14, 424. https://doi.org/10.3390/met14040424
Aria AI, Holmedal B, Mánik T, Marthinsen K. A Full-Field Crystal Plasticity Study on the Bauschinger Effect Caused by Non-Shearable Particles and Voids in Aluminium Single Crystals. Metals. 2024; 14(4):424. https://doi.org/10.3390/met14040424
Chicago/Turabian StyleAria, Arash Imani, Bjørn Holmedal, Tomas Mánik, and Knut Marthinsen. 2024. "A Full-Field Crystal Plasticity Study on the Bauschinger Effect Caused by Non-Shearable Particles and Voids in Aluminium Single Crystals" Metals 14, no. 4: 424. https://doi.org/10.3390/met14040424
APA StyleAria, A. I., Holmedal, B., Mánik, T., & Marthinsen, K. (2024). A Full-Field Crystal Plasticity Study on the Bauschinger Effect Caused by Non-Shearable Particles and Voids in Aluminium Single Crystals. Metals, 14(4), 424. https://doi.org/10.3390/met14040424







