Fluid Flow, Solidification and Solute Transport in Slab Continuous Casting with Different S-EMS Installation Positions
Abstract
:1. Introduction
2. Model Description
- The effect of molten steel on the electromagnetic field is ignored due to the low magnetic Reynolds number. Joule heat is ignored due to a low stirring frequency (<10 Hz) [24].
- The molten steel is regarded as incompressible fluid with constant physical parameters.
- The curved section of the continuous casting machine is assumed to be vertical, where the inner and outer arcs have the same drawing speed.
- The slag phase and mold oscillation are ignored.
2.1. Mathematical Model Description
2.1.1. Fluid/Dynamic Model
2.1.2. Heat Transfer Model
2.1.3. Solute Transport Model
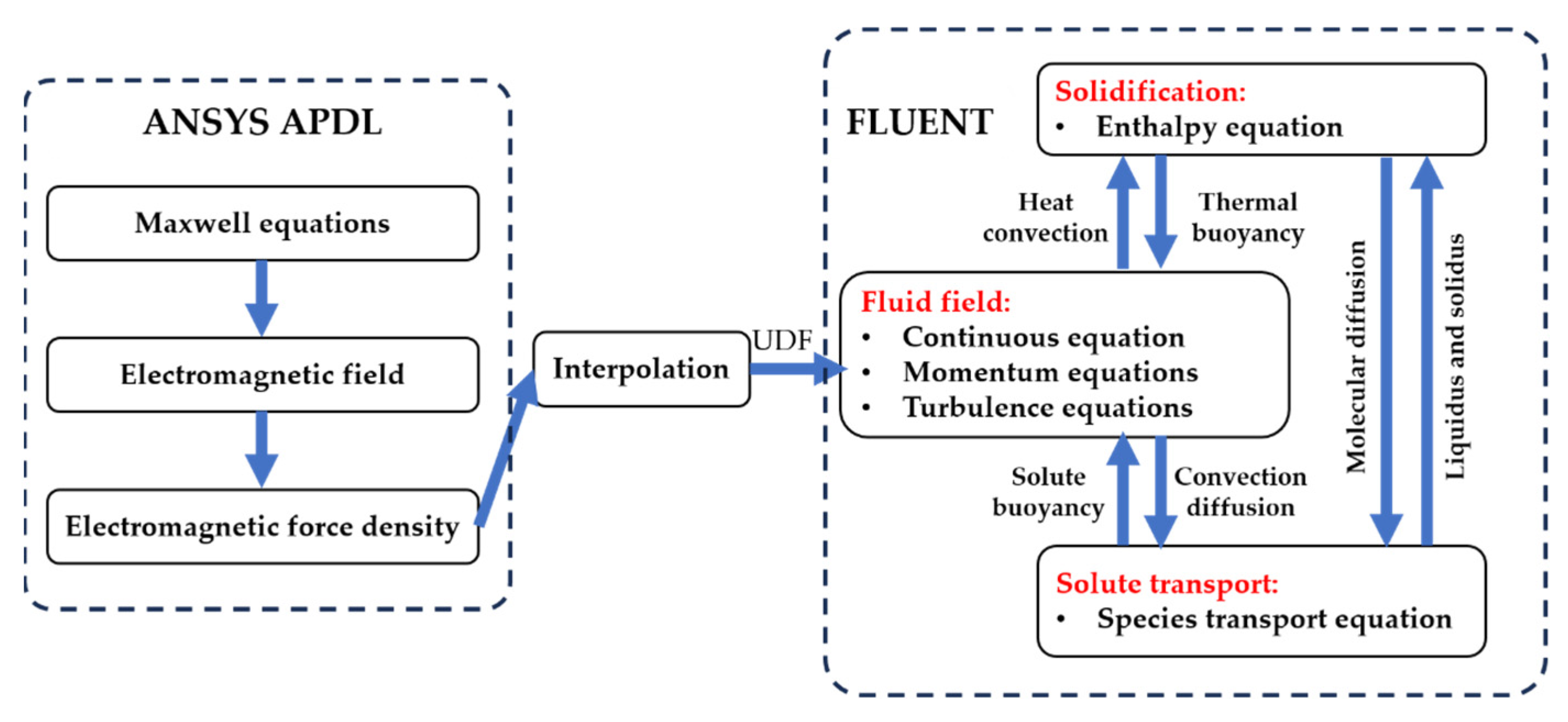
2.1.4. Electromagnetic Model
2.2. Numerical Model and Boundary Conditions
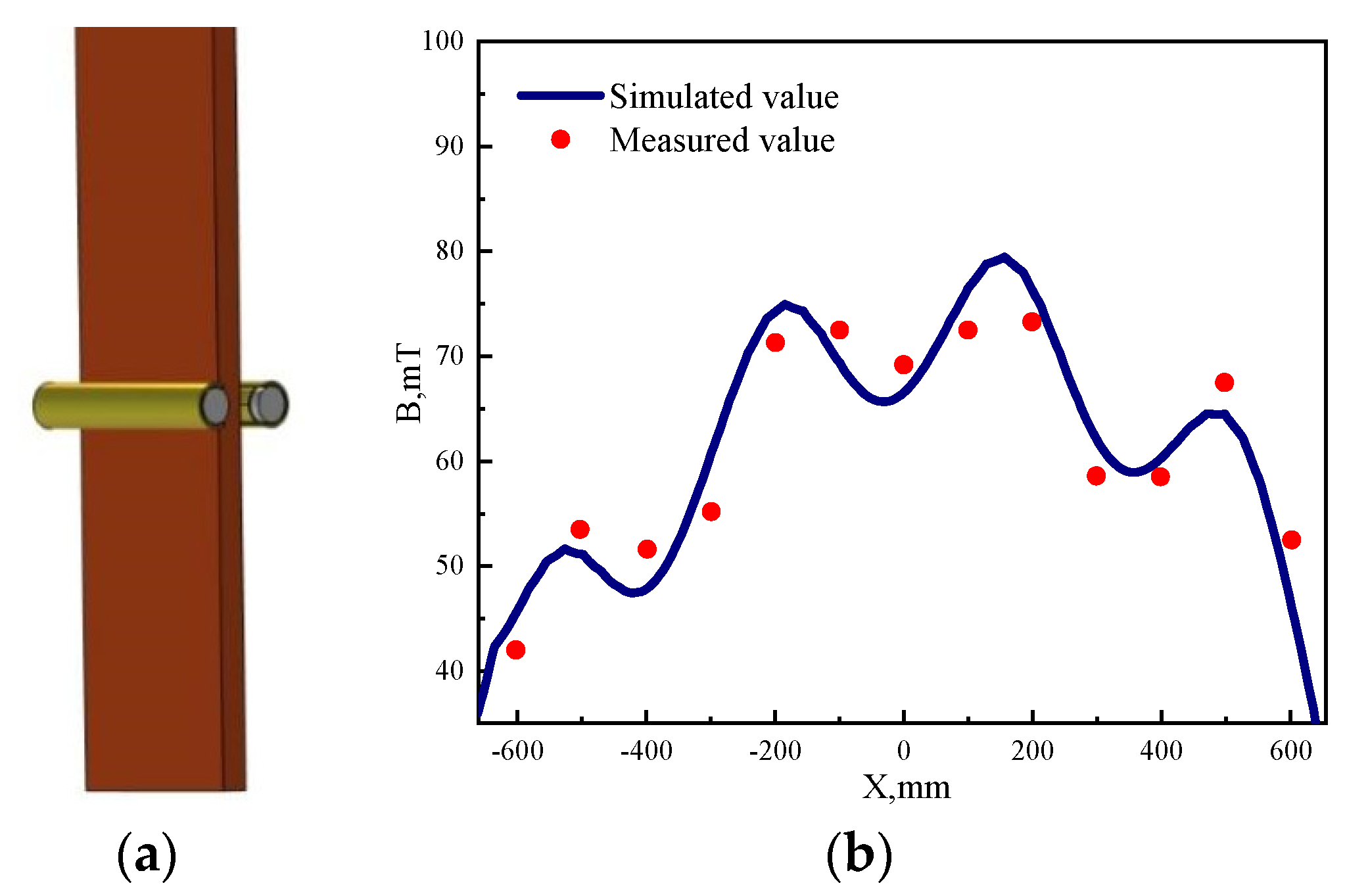
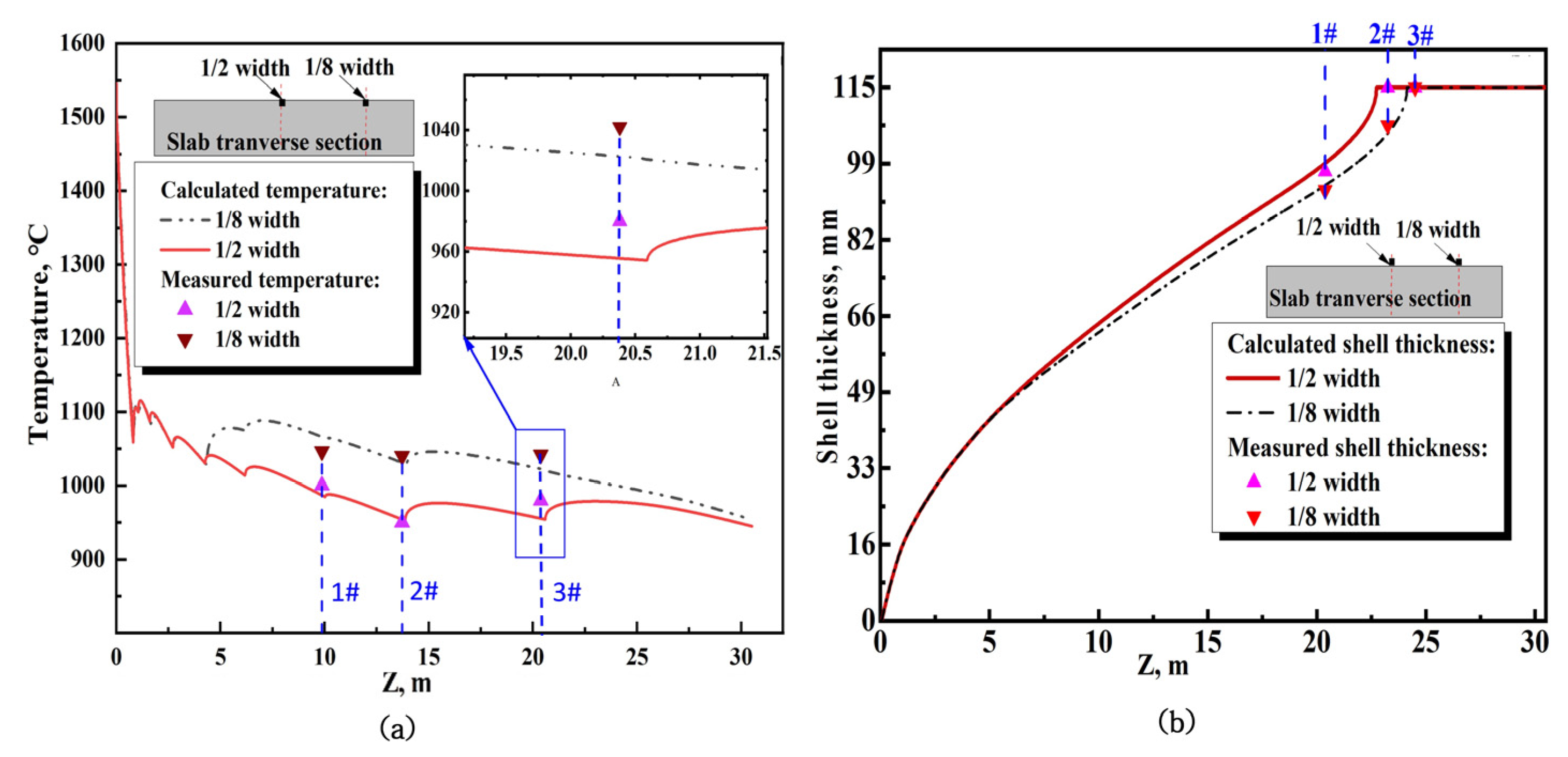
2.3. Model Validation
3. Results and Discussion
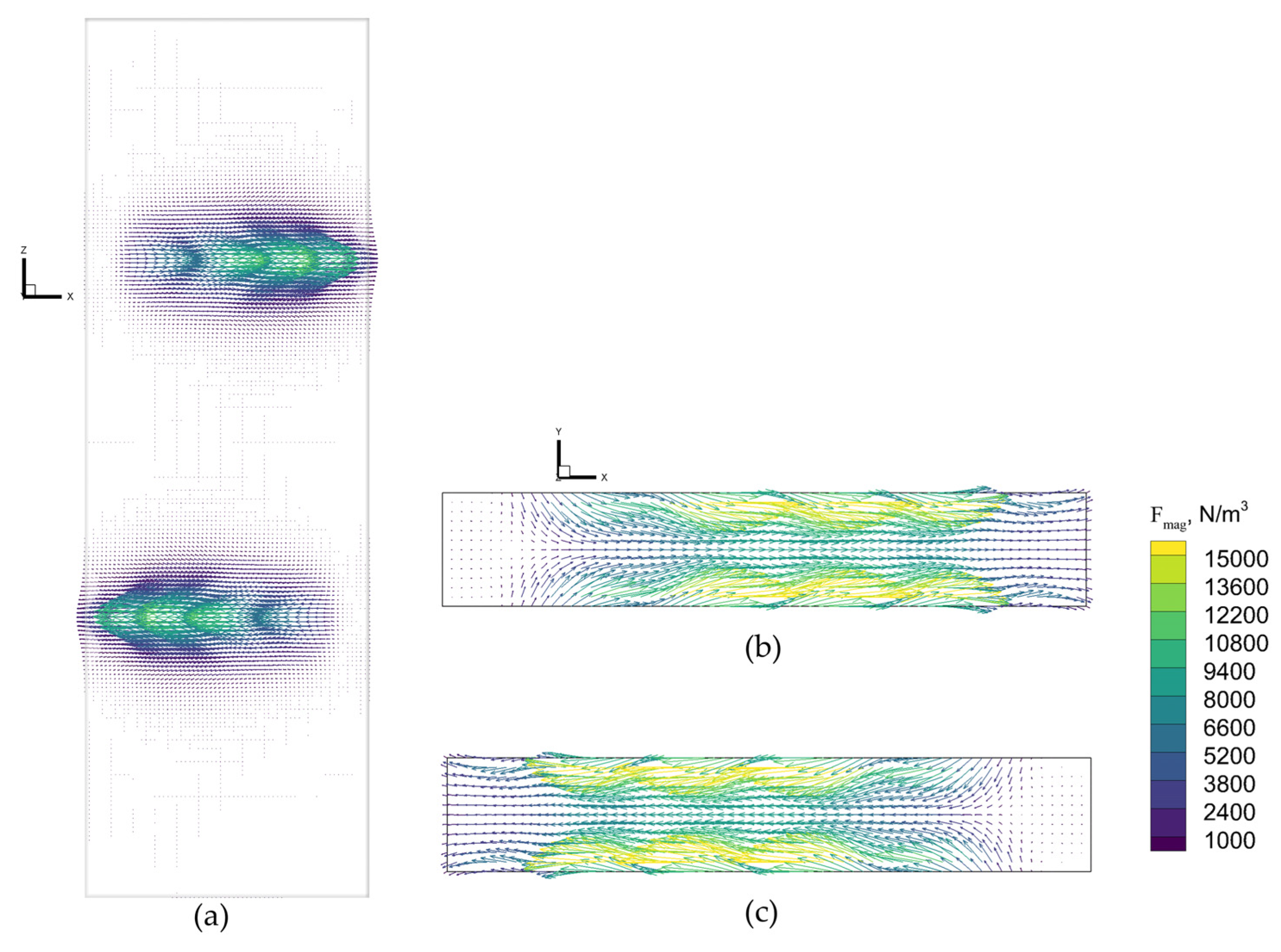
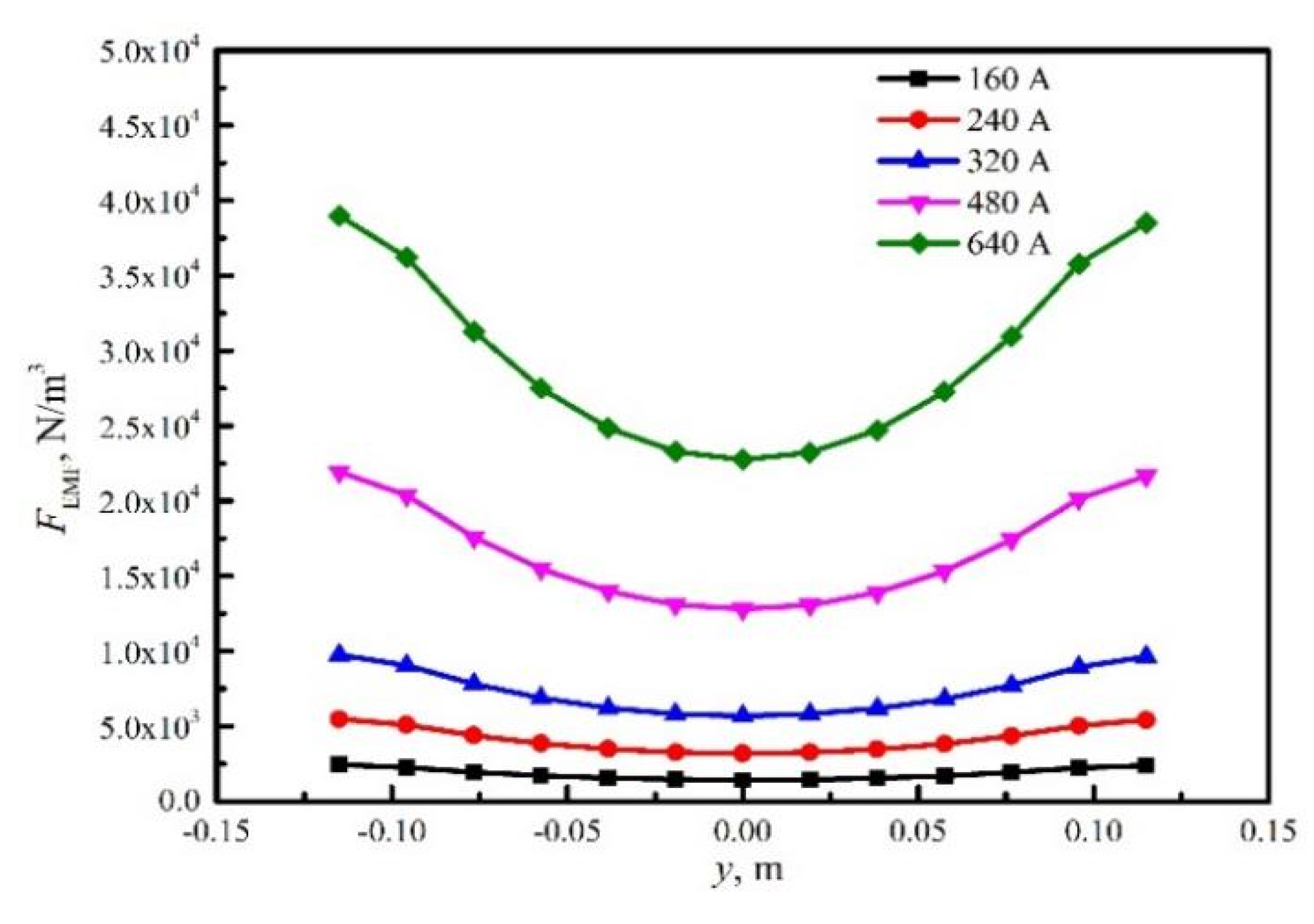
3.1. Electromagnetic Field Analysis
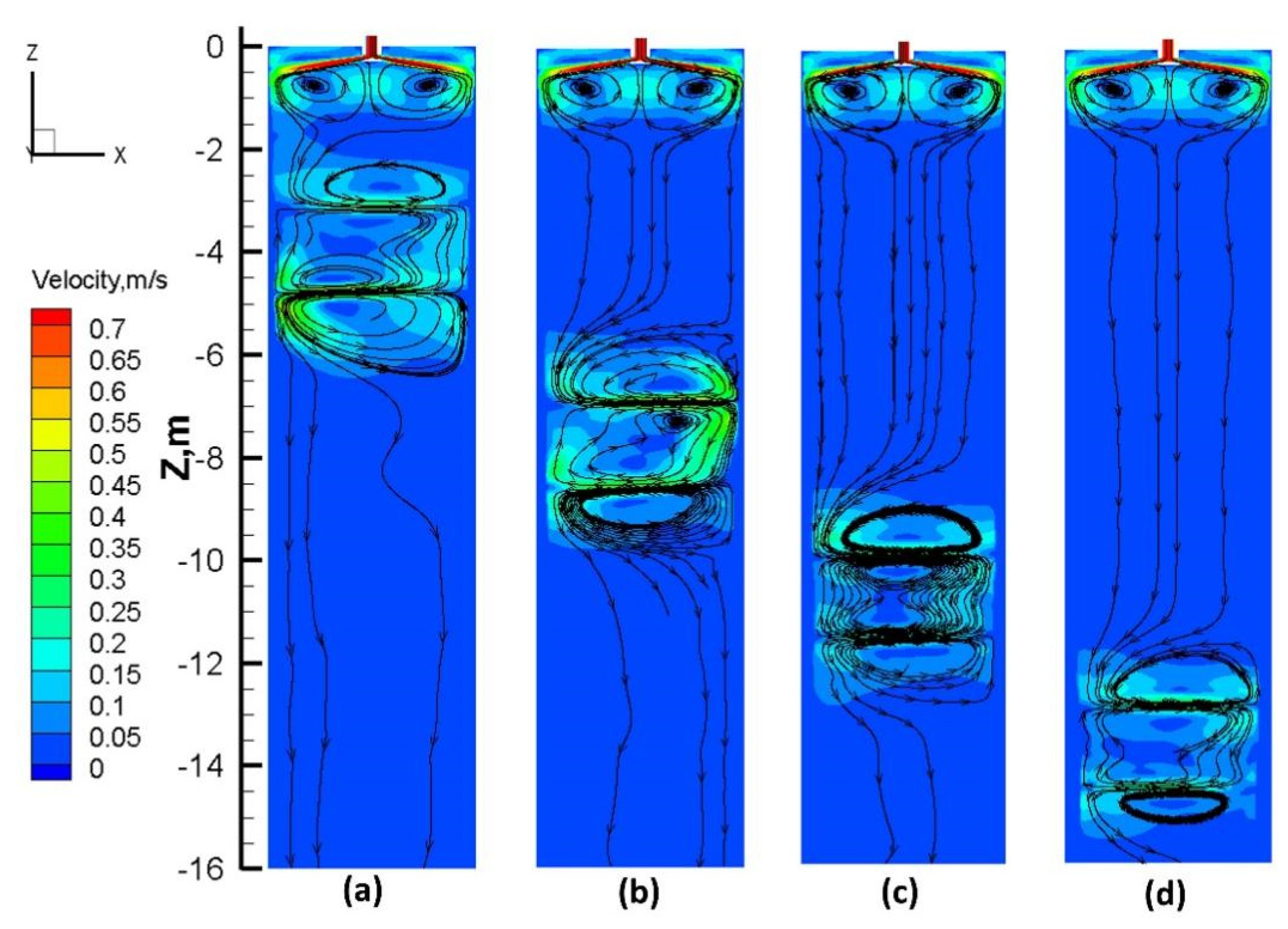
3.2. Influence of S-EMS Position on Metallurgical Behavior
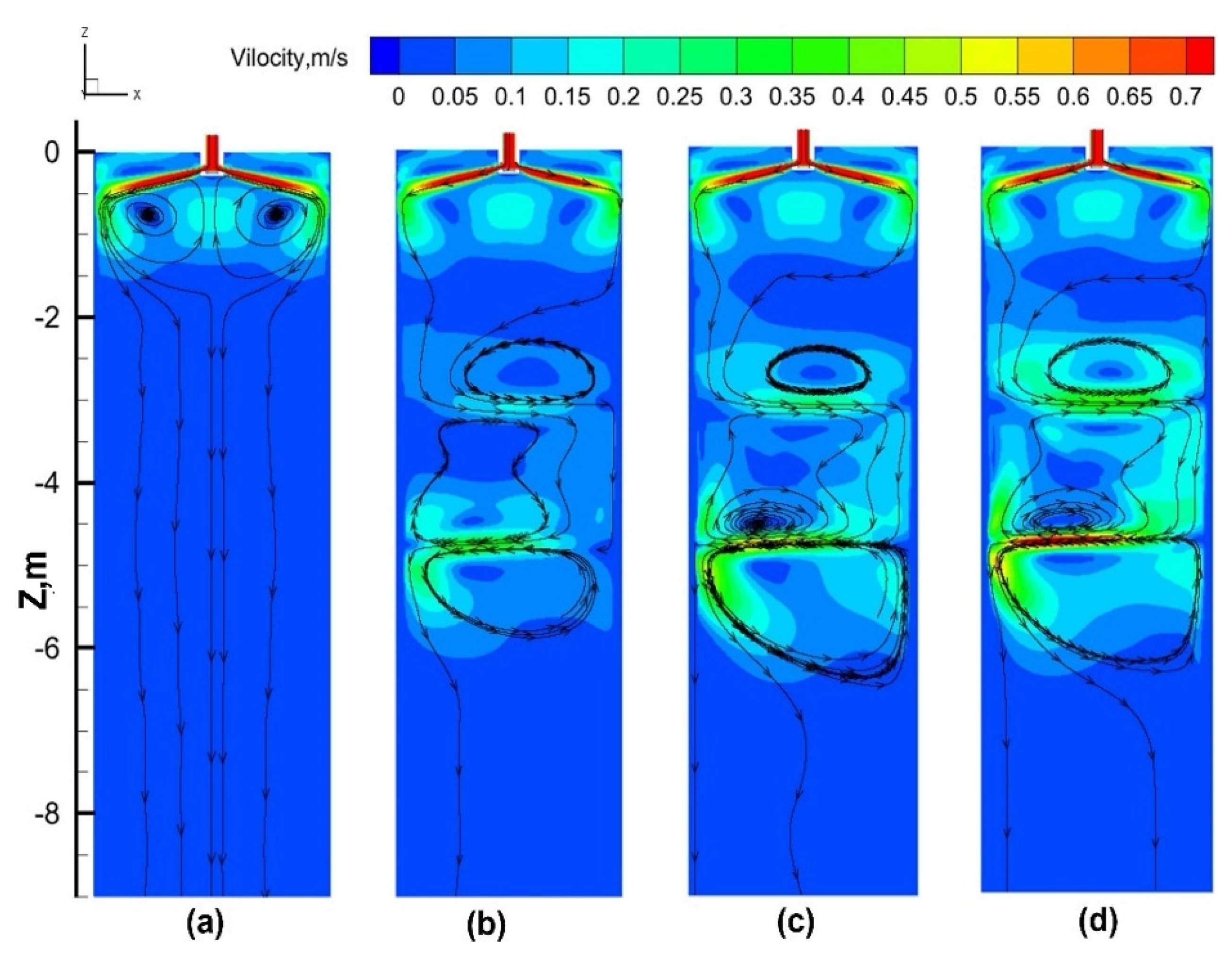
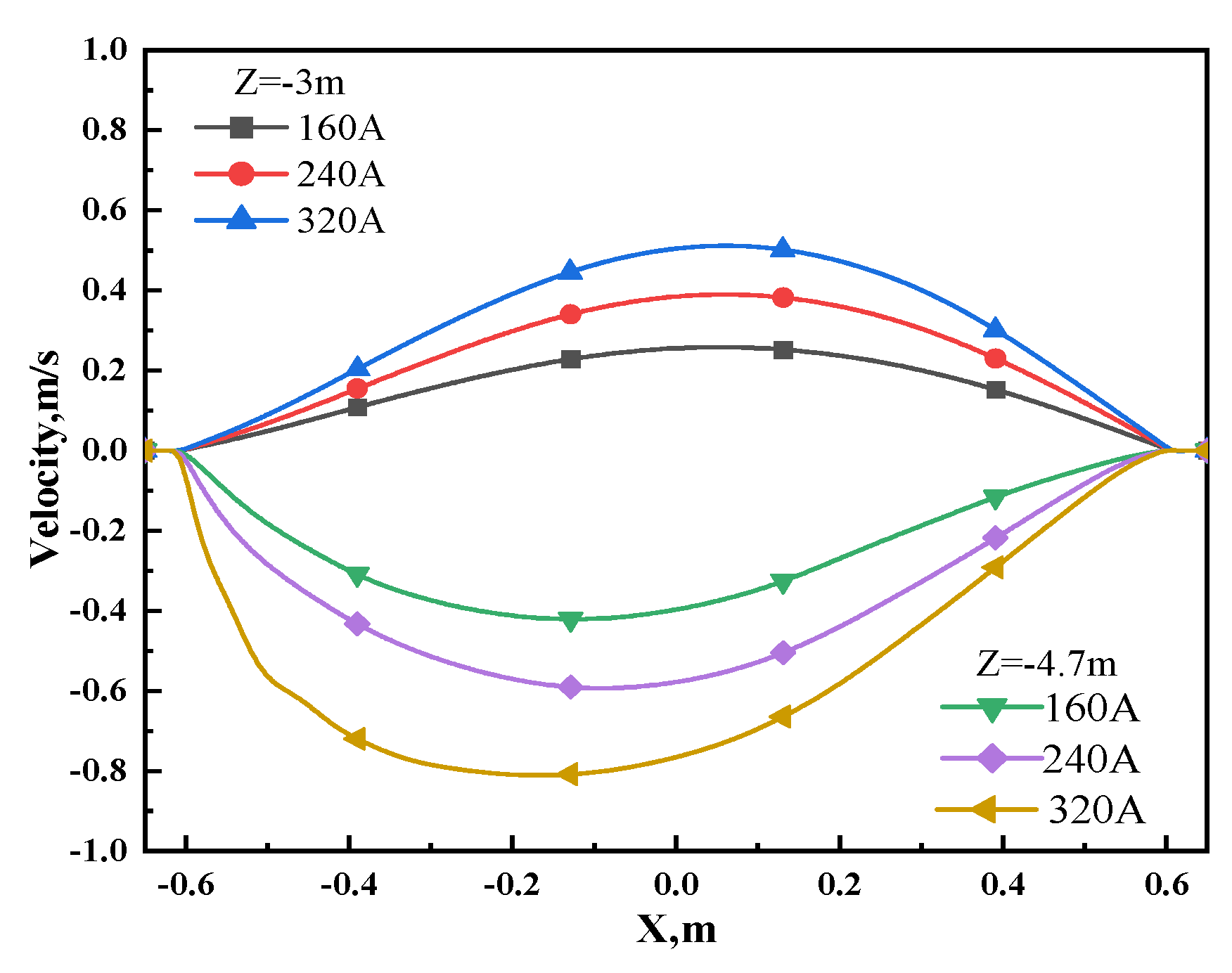
3.2.1. Flow Field and Temperature Field
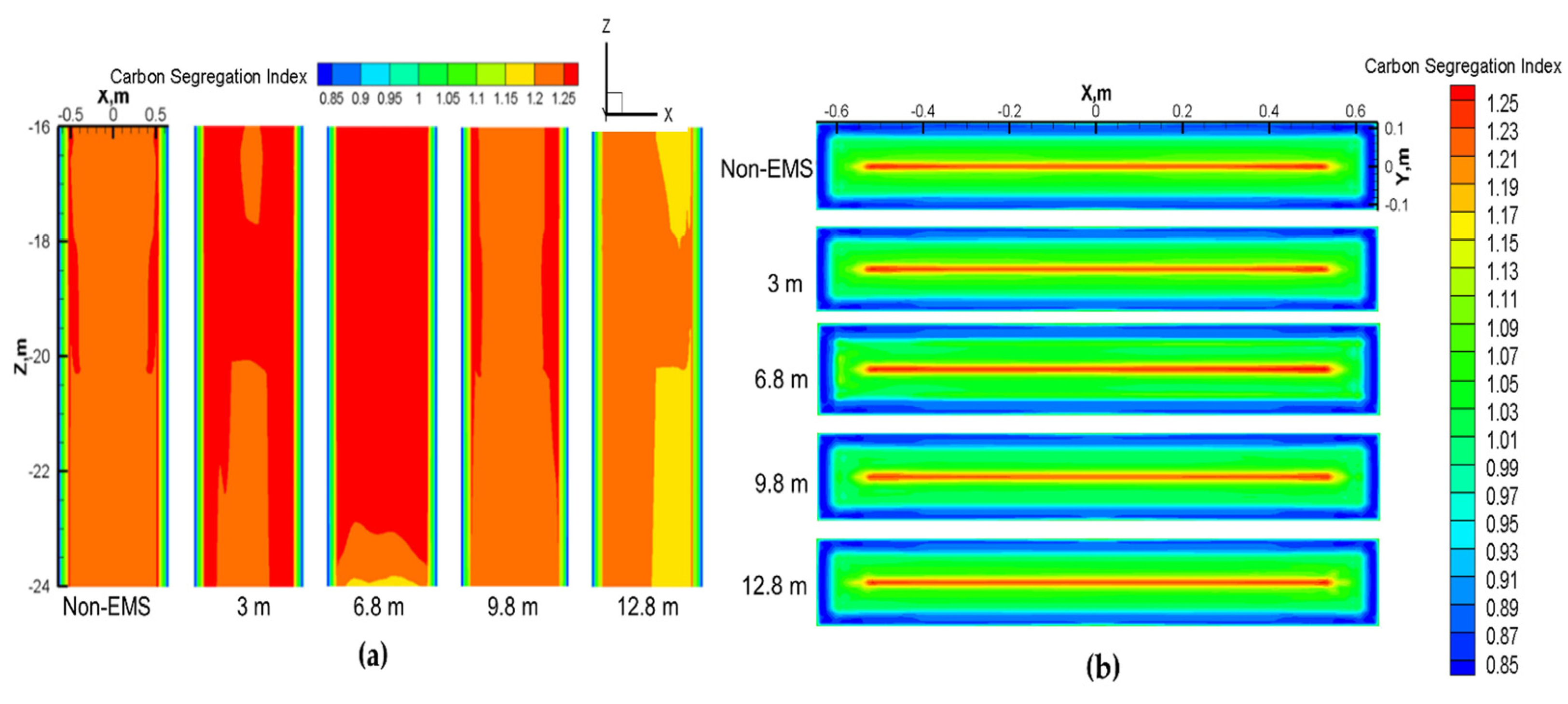
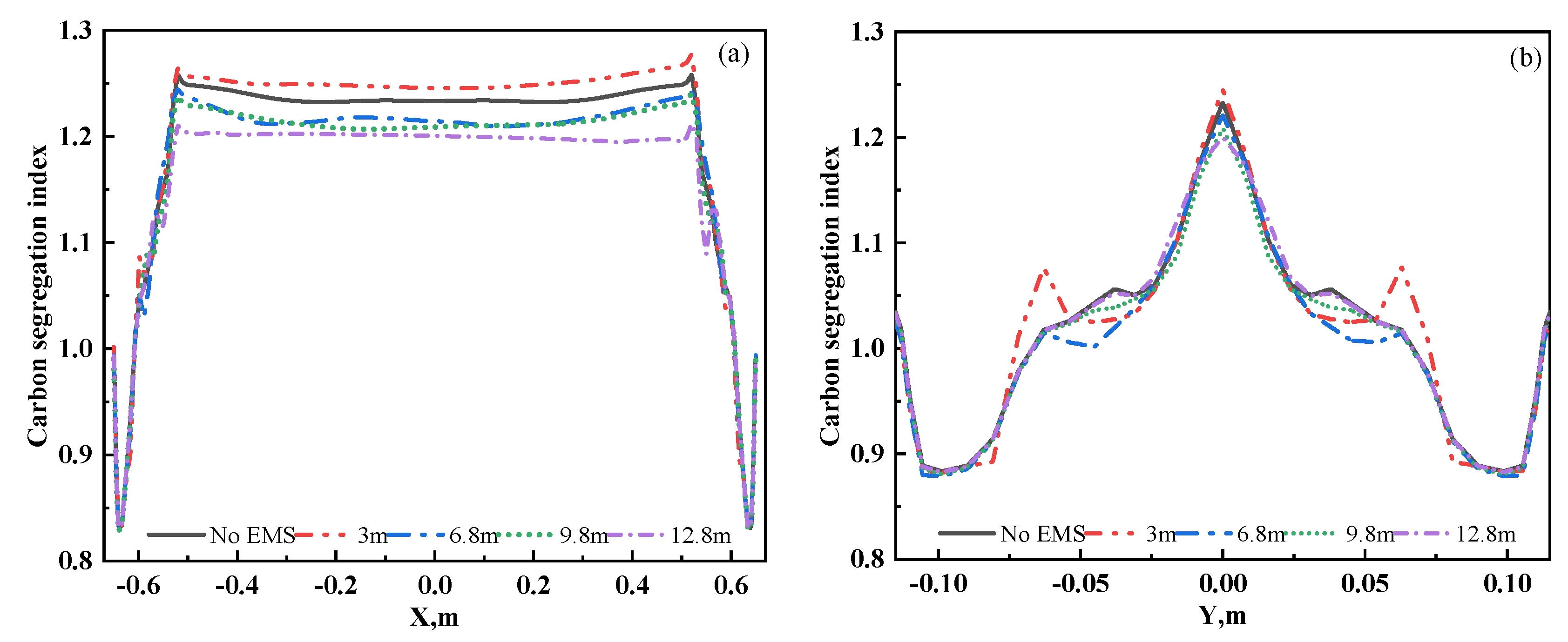
3.2.2. Solute Distribution and Segregation Behavior
3.3. Industrial Trial
| Current Intensity, A | Ratio of Equiaxed Grains, % |
| 0 | 30.9 |
| 200 | 32.7 |
| 250 | 44.7 |
| 300 | 46.3 |
| 350 | 46.5 |

4. Conclusions
- (1)
- By comparing the numerical simulation results, it was found that moving the S-EMS installation position to the solidification end reduces the stirring effect due to the increased shell thickness of the slab.
- (2)
- A higher installation position of S-EMS is beneficial for increasing the equiaxed zone extension, while a lower installation position is advantageous for reducing segregation. In actual production, both factors should be considered when selecting the appropriate stirrer installation position. Based on the comparative analysis of the four installation positions studied in this paper, the 6.8 m position is selected as the optimal installation position.
- (3)
- The installation position has a great effect on central segregation. Simulation results indicate that as the installation position is reduced from 3 m to 12.8 m, the maximum segregation index and segregation range decrease from 1.26 and 0.42 to 1.2 and 0.36, respectively.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jiang, D.; Wang, W.; Luo, S.; Ji, C.; Zhu, M. Mechanism of Macrosegregation Formation in Continuous Casting Slab: A Numerical Simulation Study. Metall. Mater. Trans. B 2017, 48, 3120–3131. [Google Scholar] [CrossRef]
- Cho, S.-M.; Thomas, B.G. Electromagnetic Effects on Solidification Defect Formation in Continuous Steel Casting. JOM 2020, 72, 3610–3627. [Google Scholar] [CrossRef]
- Guan, R.; Ji, C.; Zhu, M.; Deng, S. Numerical Simulation of V-shaped Segregation in Continuous Casting Blooms Based on a Microsegregation Model. Metall. Mater. Trans. B 2018, 49, 2571–2583. [Google Scholar] [CrossRef]
- Kunstreich, S. Electromagnetic stirring for continuous casting (part 2). Rev. Metall. 2003, 100, 1043–1061. [Google Scholar] [CrossRef]
- Poole, G.M.; El-Kaddah, N. An Improved Model for the Flow in an Electromagnetically Stirred Melt during Solidification. Metall. Mater. Trans. B 2013, 44, 1531–1540. [Google Scholar] [CrossRef]
- Zeng, J.; Chen, W.; Wang, Q.; Wang, G. Improving Inner Quality in Continuous Casting Rectangular Billets: Comparison Between Mechanical Soft Reduction and Final Electromagnetic Stirring. Trans. Indian Inst. Met. 2016, 69, 1623–1632. [Google Scholar] [CrossRef]
- Guan, R.; Ji, C.; Wu, C.; Zhu, M. Numerical modelling of fluid flow and macrosegregation in a continuous casting slab with asymmetrical bulging and mechanical reduction. Int. J. Heat Mass Transf. 2019, 141, 503–516. [Google Scholar] [CrossRef]
- Jiang, D.; Wang, W.; Luo, S.; Ji, C.; Zhu, M. Numerical simulation of slab centerline segregation with mechanical reduction during continuous casting process. Int. J. Heat Mass Transf. 2018, 122, 315–323. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, L.; Yang, W.; Ji, S.; Ren, Y. Effect of Mold Electromagnetic Stirring and Final Electromagnetic Stirring on the Solidification Structure and Macrosegregation in Bloom Continuous Casting. Steel Res. Int. 2021, 92, 2000661. [Google Scholar] [CrossRef]
- Wang, F.; Wang, X.; Cui, J. Effect of Low-Frequency Electromagnetic Casting on Micro-Structure and Macro-Segregation of 5A90 Alloy Ingots. Materials 2020, 13, 2720. [Google Scholar] [CrossRef] [PubMed]
- Yao, C.; Wang, M.; Ni, Y.; Gong, J.; Xing, L.; Zhang, H.; Bao, Y. Effects of Secondary Cooling Segment Electromagnetic Stirring on Solidification Behavior and Composition Distribution in High-Strength Steel 22MnB5. JOM 2022, 74, 4823–4830. [Google Scholar] [CrossRef]
- Trindade, L.B.; Nadalon, J.E.A.; Contini, A.C.; Barroso, R.C. Modeling of Solidification in Continuous Casting Round Billet with Mold Electromagnetic Stirring (M-EMS). Steel Res. Int. 2017, 88, 1600319. [Google Scholar] [CrossRef]
- Guan, R.; Ji, C.; Zhu, M. Modeling the Effect of Combined Electromagnetic Stirring Modes on Macrosegregation in Continuous Casting Blooms. Metall. Mater. Trans. B 2020, 51, 1137–1153. [Google Scholar] [CrossRef]
- Gong, J.; Liu, H.; Wang, X.; Bao, Y. Numerical Simulation of Electromagnetic Field and Flow Pattern in a Continuous Slab Caster with In-roll Type Strand Electromagnetic Stirring. J. Iron Steel Res. Int. 2015, 22, 414–422. [Google Scholar] [CrossRef]
- Li, S.; Han, Z.; Zhang, J. Numerical Modeling of the Macrosegregation Improvement in Continuous Casting Blooms by Using F-EMS. JOM 2020, 72, 4117–4126. [Google Scholar] [CrossRef]
- Xiao, H.; Wang, P.; Yi, B.; Chen, X.; Li, A.; Tang, H.; Li, W.; Zhang, J. A Numerical and Experimental Study on the Solidification Structure of Fe-Cr-Ni Steel Slab Casting by Roller Electromagnetic Stirring. Metals 2021, 11, 6. [Google Scholar] [CrossRef]
- Xin, W.; Zheng, S.-G.; Zhu, M.-Y. Fluid flow, solidification and solute transport in billet continuous casting with different stirrer positions. Ironmak. Steelmak. 2022, 49, 343–353. [Google Scholar]
- Dong, Q.; Jiongming, Z.; Yin, Y.; Nagaumi, H. Numerical simulation of macrosegregation in billet continuous casting influenced by electromagnetic stirring. J. Iron Steel Res. Int. 2022, 29, 612–627. [Google Scholar] [CrossRef]
- Song, X.P.; Cheng, S.S.; Cheng, Z.J. Mathematical modelling of billet casting with secondary cooling zone electromagnetic stirrer. Ironmak. Steelmak. 2013, 40, 189–198. [Google Scholar] [CrossRef]
- Liu, H.; Wang, Z.; Qiu, H. Numerical Simulation of Fluid Flow and Solidification in a Vertical Round Bloom Caster Using a Four-port SEN with Mold and Strand Electromagnetic Stirring. ISIJ Int. 2022, 60, 1924–1937. [Google Scholar] [CrossRef]
- Wang, R.; Bao, Y.; Li, Y.; An, H. Optimization of strand and final electromagnetic stirrers of round bloom casters with multiple sections. Int. J. Miner. Metall. Mater. 2016, 23, 1150–1156. [Google Scholar] [CrossRef]
- Jiang, D.; Zhu, M.; Lifeng, Z. Numerical Simulation of Solidification Behavior and Solute Transport in Slab Continuous Casting with S-EMS. Metals 2019, 9, 452. [Google Scholar] [CrossRef]
- Li, X.; Wang, X.; Bao, Y.; Gong, J.; Pang, W.; Wang, M. Effect of Electromagnetic Stirring on the Solidification Behavior of High-Magnetic-Induction Grain-Oriented Silicon Steel Continuous Casting Slab. JOM 2020, 72, 3628–3633. [Google Scholar] [CrossRef]
- Li, Y.; Deng, A.; Yang, B.; Xu, H.; Wang, E. Inhibiting bulging deformation of liquid metal free surface by magnetic pressure. J. Iron Steel Res. Int. 2020, 28, 818–829. [Google Scholar] [CrossRef]
- Li, Y.; Deng, A.Y.; Li, H.; Yang, B.; Wang, E.G. Numerical Study on Flow, Temperature, and Concentration Distribution Features of Combined Gas and Bottom-Electromagnetic Stirring in a Ladle. Metals 2018, 8, 76. [Google Scholar] [CrossRef]
- Luo, S.; Li, K.; Wang, W.; Zhu, M. Numerical simulation of macro-segregation in continuously cast slab with application of S-EMS and MR. J. Mater. Res. Technol. 2023, 24, 6893–6907. [Google Scholar] [CrossRef]

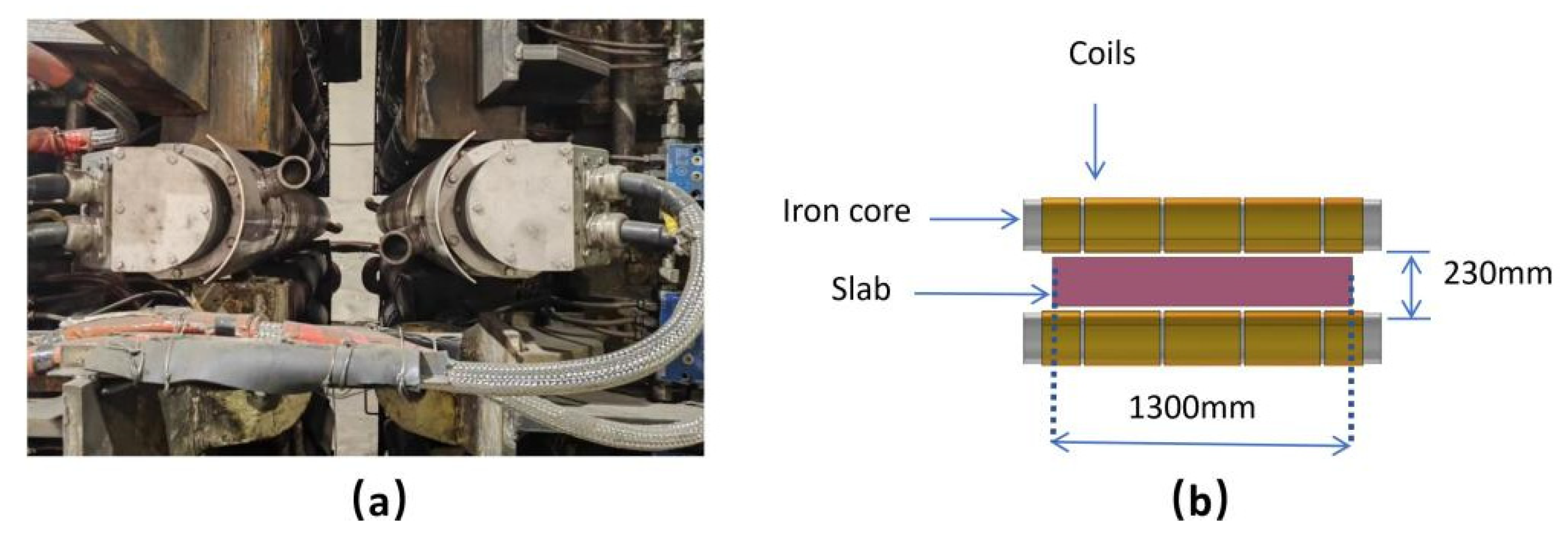







| Item | Value |
|---|---|
| Slab section size, mm × mm | 230 × 1300 |
| Casting speed, m·min−1 | 1.2 |
| Nozzle immersion depth, mm | 210 |
| Nozzle angles, deg | −15 |
| Relative magnetic permeability of molten steel, coils, and air | 1 |
| Relative magnetic permeability of iron core | 1000 |
| Conductivity of molten steel, S/m | 7.14 × 105 |
| Specific heat capacity of molten steel, J·kg−1·K | 680 |
| Thermal conductivity of molten steel, W·m−1·K−1 | 29 |
| Viscosity of molten steel, kg·m−1·s−1 | 0.0055 |
| Density of molten steel, kg·m−3 | 7020 |
| Solidus temperature, K | 1763 |
| Liquidus temperature, K | 1802 |
| Latent heat, kJ/kg | 270 |
| Thermal expansion coefficient, K−1 | 1 × 10−4 |
| Steel grade | E355 |
| Position of stirring rolls, m | 3.0, 6.8, 9.8, 12.8 |
| Turns of EMS coils | 100 |
| Current of EMS coils, A | 160, 240, 320 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, D.; Zhang, G.; Zeng, J.; Li, Y. Fluid Flow, Solidification and Solute Transport in Slab Continuous Casting with Different S-EMS Installation Positions. Metals 2024, 14, 686. https://doi.org/10.3390/met14060686
Liu D, Zhang G, Zeng J, Li Y. Fluid Flow, Solidification and Solute Transport in Slab Continuous Casting with Different S-EMS Installation Positions. Metals. 2024; 14(6):686. https://doi.org/10.3390/met14060686
Chicago/Turabian StyleLiu, Daiwei, Guifang Zhang, Jianhua Zeng, and Yang Li. 2024. "Fluid Flow, Solidification and Solute Transport in Slab Continuous Casting with Different S-EMS Installation Positions" Metals 14, no. 6: 686. https://doi.org/10.3390/met14060686
APA StyleLiu, D., Zhang, G., Zeng, J., & Li, Y. (2024). Fluid Flow, Solidification and Solute Transport in Slab Continuous Casting with Different S-EMS Installation Positions. Metals, 14(6), 686. https://doi.org/10.3390/met14060686





