Study on Formability Improvement of Zr-4 Sheets Based on Texture Optimization
Abstract
:1. Introduction
2. Experiment Material and Method
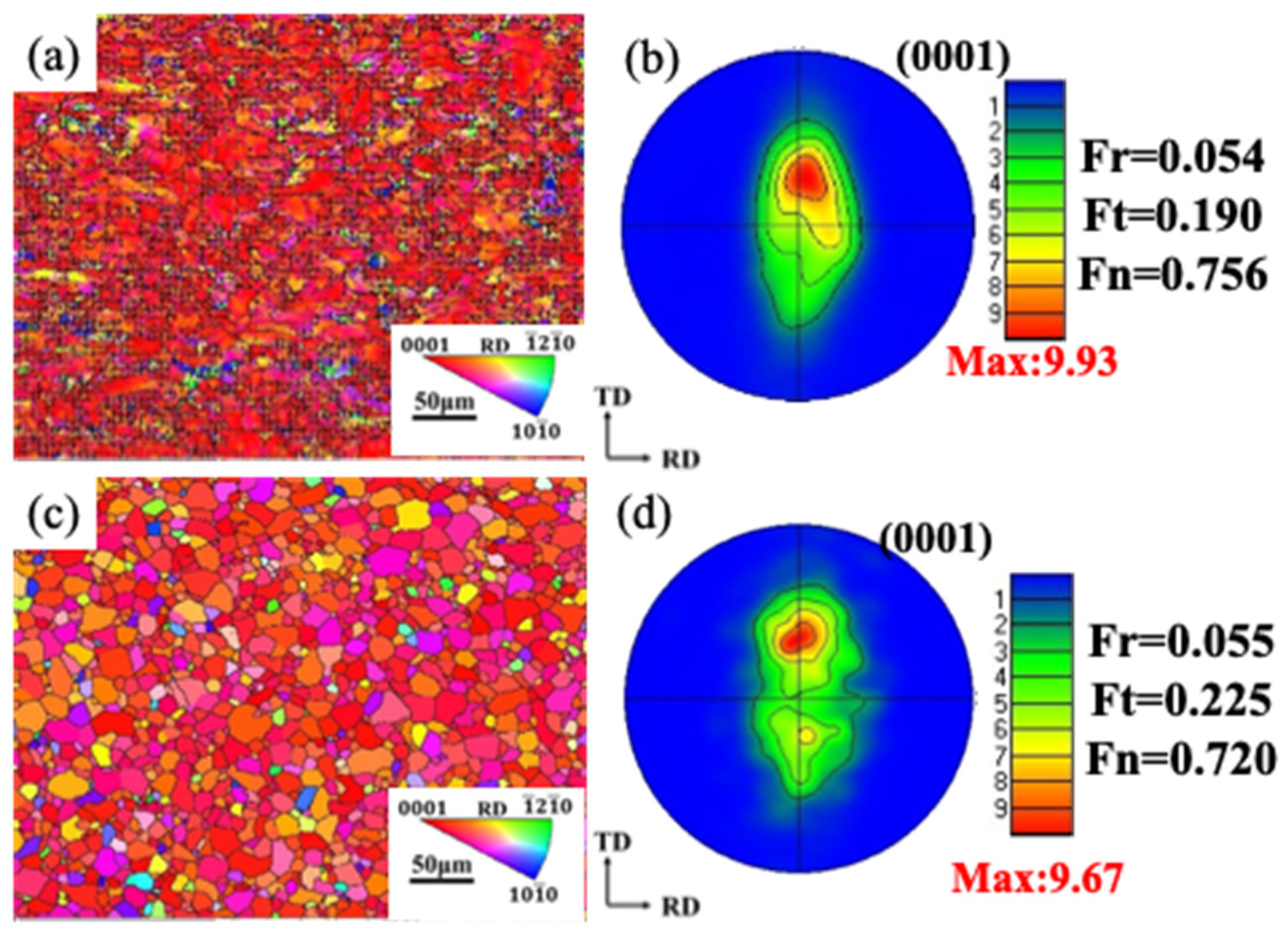
3. Experimental Results
4. Study on Textures Optimization for Formability Improvement
4.1. Prediction of Different Initial Textures on Hardening Index Based on VPSC Model
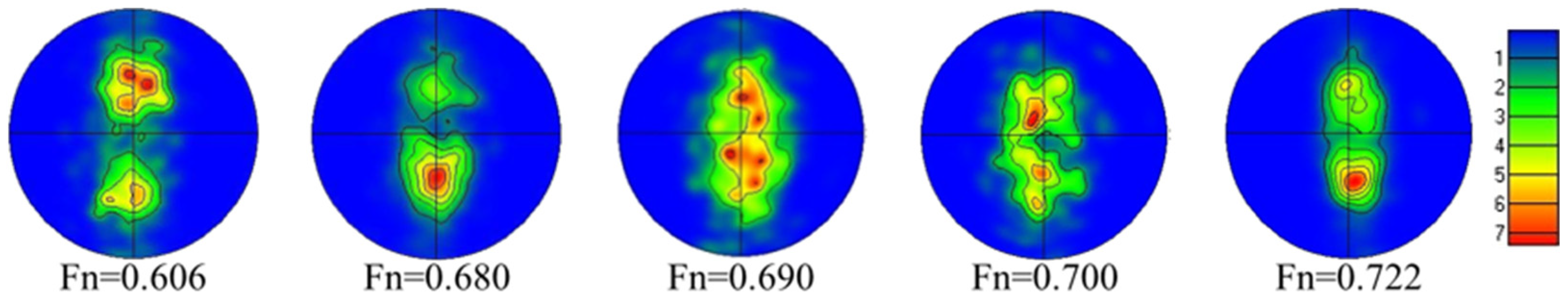
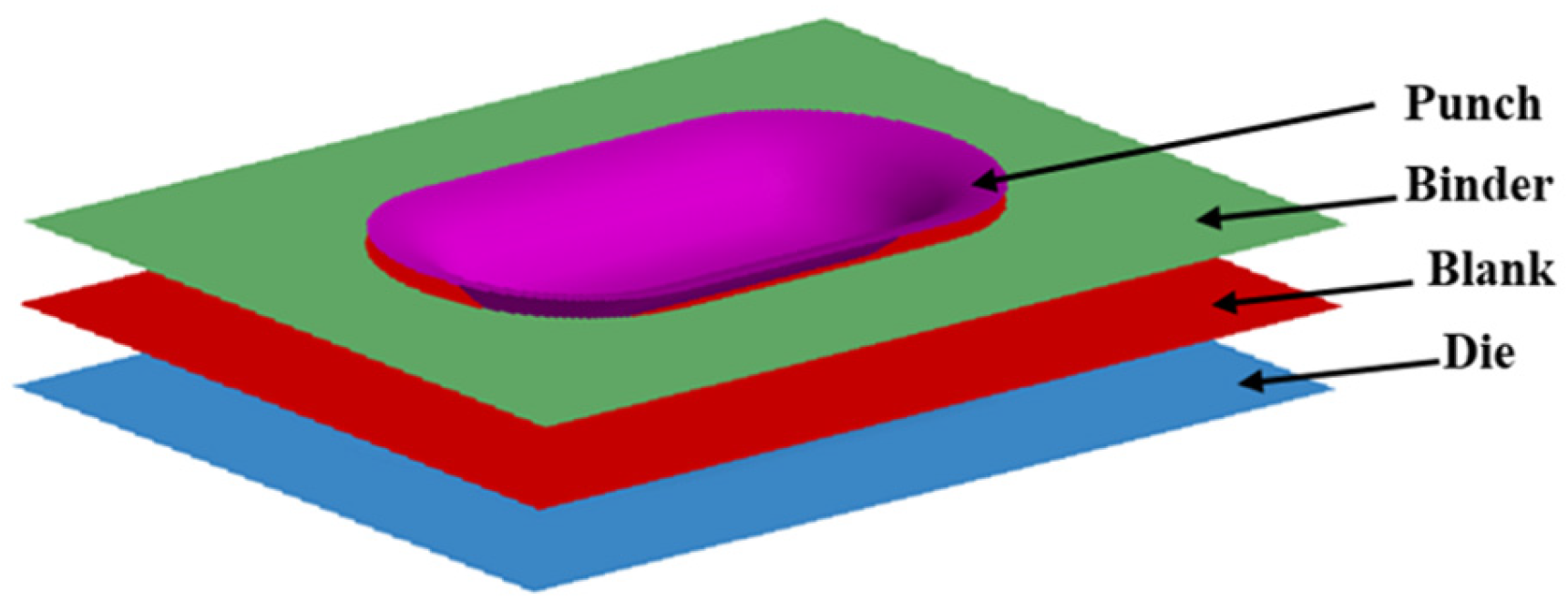
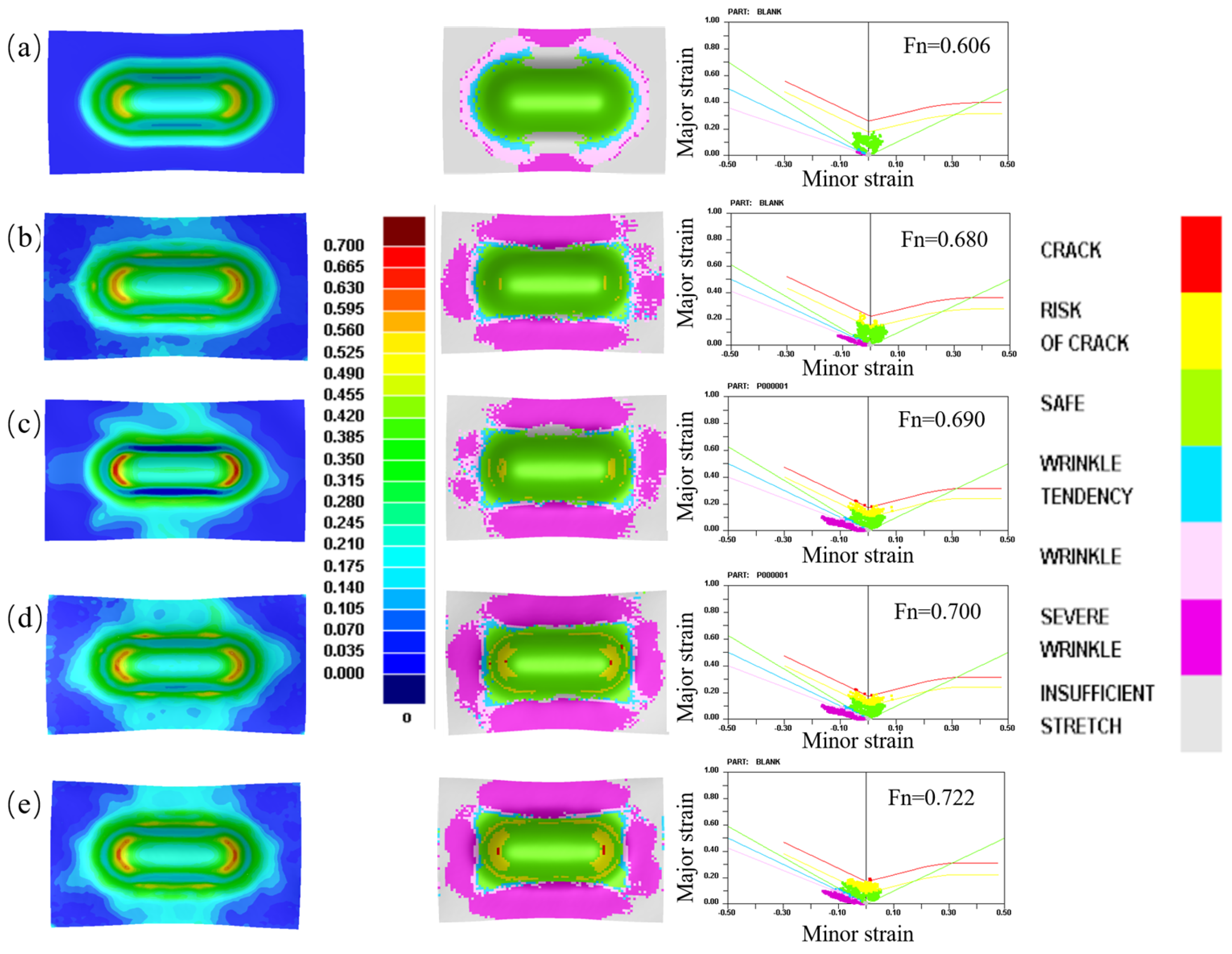
4.2. Effect of Initial Texture with Different Fns on Formability in Stamping Process
5. Discussion
6. Conclusions
- (1)
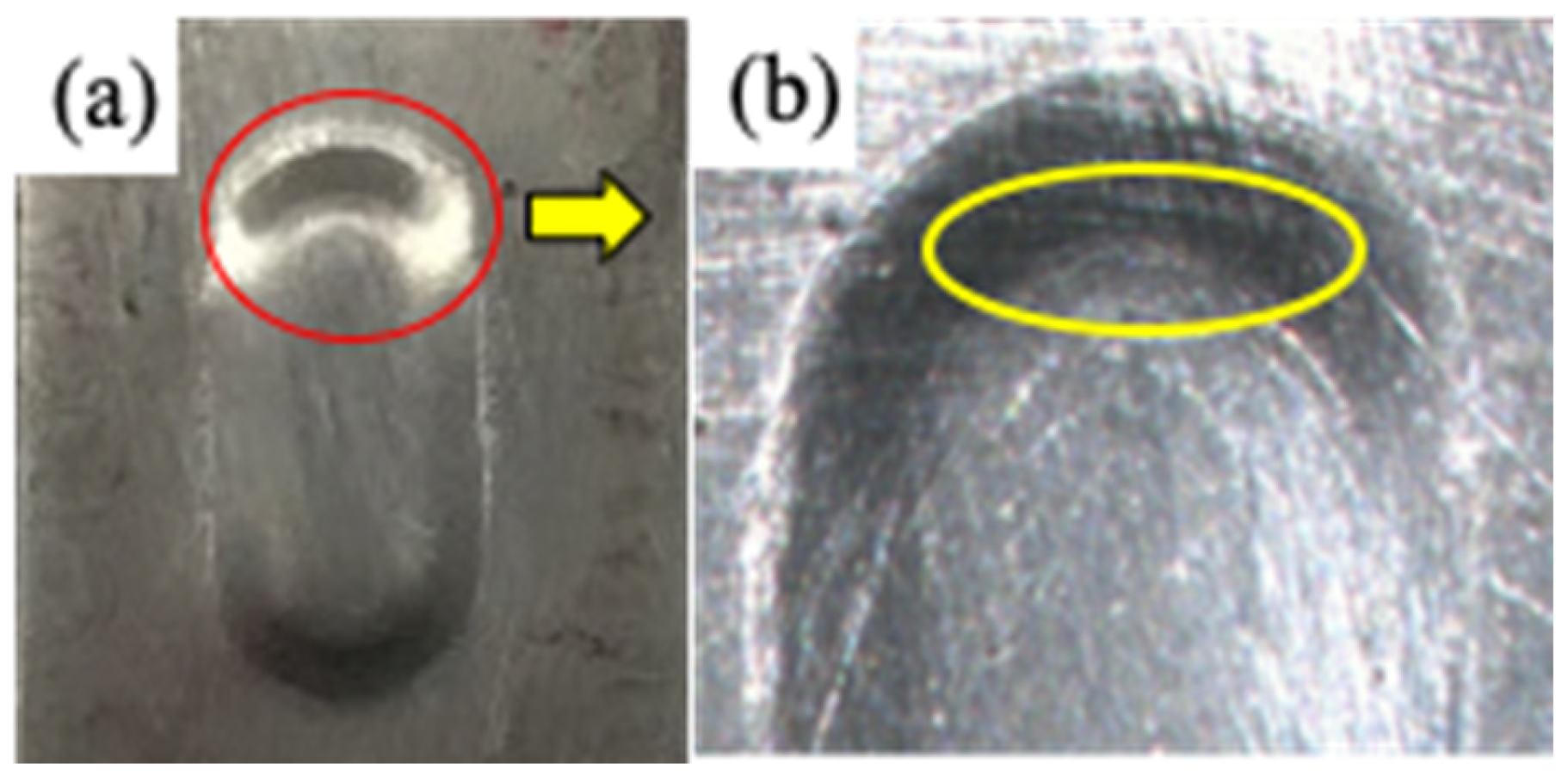
- The sheet prepared by isobaric reduction rolling cracks in the stamping process.
- (2)
- Based on FEM and VPSC simulations, the Fn of the sheet should be controlled to be less than 0.7 in order to prevent the sheet cracking.
- (3)
- A new rolling process named non-isobaric reduction rolling was designed, and the result shows that the Fn of the sheet prepared by isobaric reduction rolling is higher than the sheet prepared by non-isobaric reduction rolling.
- (4)
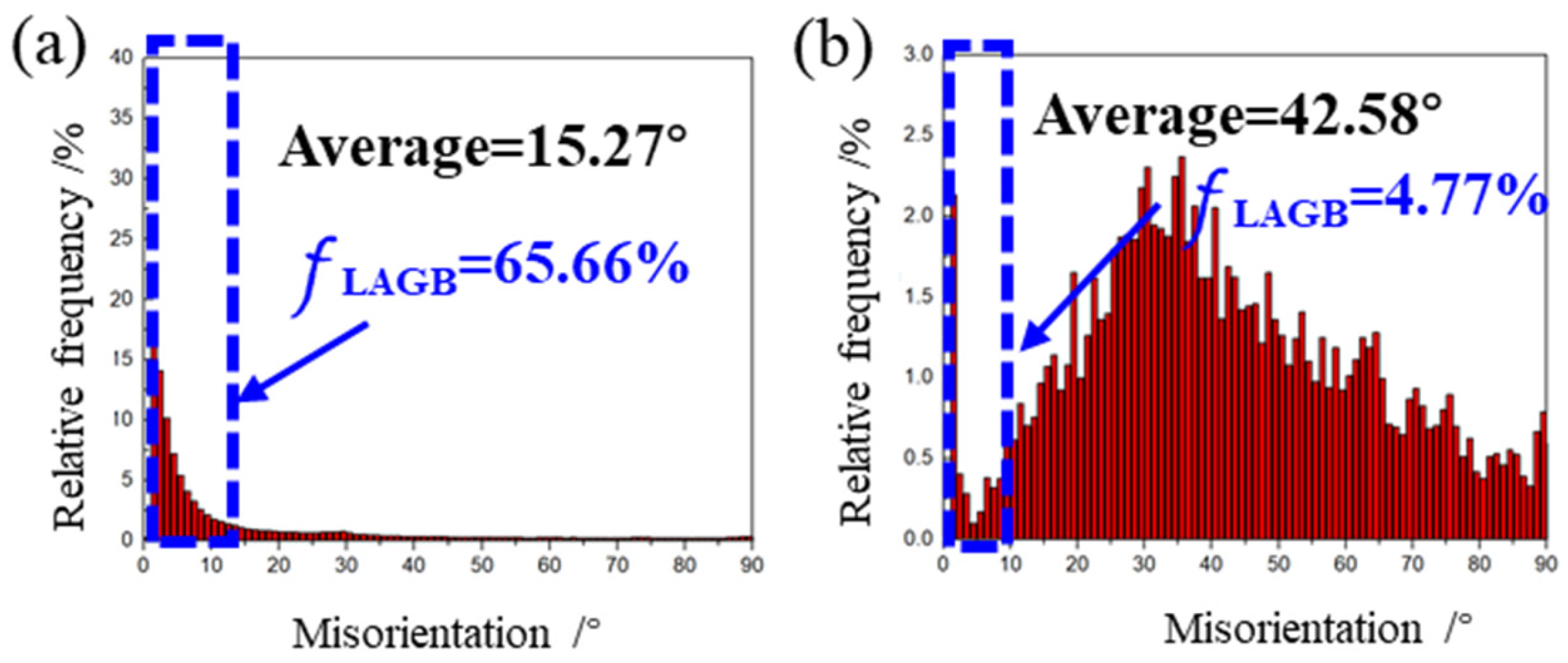
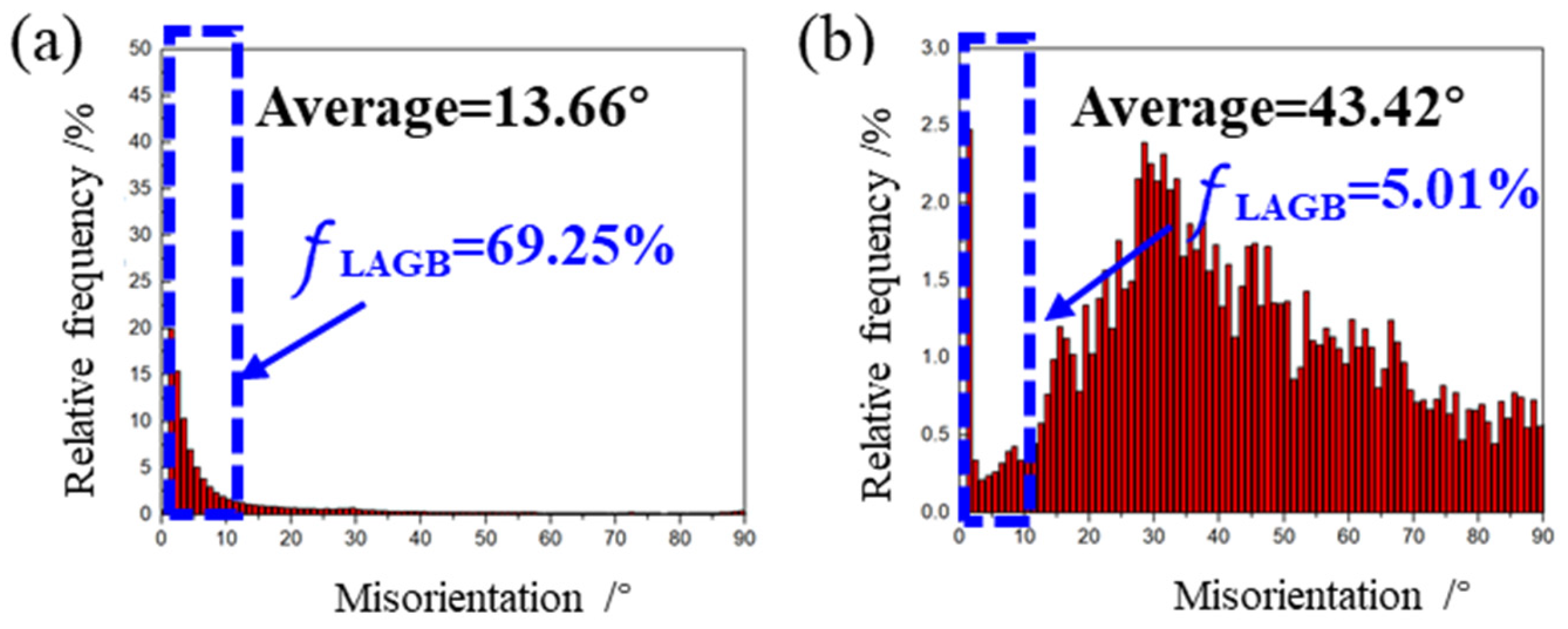
- The fraction of LAGBs and the average angle of grains have no obvious differences between isobaric reduction rolling and non-isobaric reduction rolling.
- (5)
- The Fn of the initial texture prepared by non-isobaric reduction rolling is less than 0.7, and the sheet is free of cracking during stamping.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ronald, B. Adamson. In Zirconium Production and Technology: The Kroll Medal Papers; ASTM International: West Conshohocken, PA, USA, 2010; pp. 321–355. [Google Scholar]
- Holt, R.A.; Limback, M.; Kammenzind, B.; Dean, S.W. In-reactor deformation of zirconium alloy components. J. ASTM Int. 2008, 5, 101354–101369. [Google Scholar] [CrossRef]
- Steinbrück, M.; Böttcher, M. Air oxidation of Zircaloy-4, M5® and ZIRLO™ cladding alloys at high temperatures. J. Nucl. Mater. 2011, 414, 276–285. [Google Scholar] [CrossRef]
- Nikulina, A.V.; Konkov, V.F.; Peregud, M.M.; Vorobev, E.E. Effect of molybdenum on properties of zirconium components of nuclear reactor core. Nucl. Mater. Energy 2018, 14, 8–13. [Google Scholar] [CrossRef]
- Jeong, Y.H.; Park, S.Y.; Lee, M.H.; Choi, B.K.; Baek, J.H.; Park, J.Y.; Kim, J.H.; Kim, H.G. Out-of-pile and in-pile perfomance of advanded zirconium alloys (HANA) for high burn-up fuel. J. Nucl. Sci. Technol. 2006, 43, 977–983. [Google Scholar] [CrossRef]
- Onimus, F.; Gelebart, L.; Brenner, R. Polycrystalline simulations of in-reactor deformation of recrystallized Zircaloy-4 tubes: Fast fourier transform computations and mean-field self-consistent model. Int. J. Plast. 2022, 153, 103272. [Google Scholar] [CrossRef]
- Han, F.Z.; Li, G.P.; Liu, C.Z.; Yuan, F.S.; Zhang, Y.D.; Ali, M.; Guo, W.B.; Gu, H.F. Anisotropic yielding behavior and associated mechanism of cold rolled and annealed Zircaloy-4 alloy thin sheets under tensile condition. Mater. Chem. Phys. 2020, 242, 122539. [Google Scholar] [CrossRef]
- Guo, W.B.; Li, G.P.; Yuan, F.S.; Han, F.Z.; Zhang, Y.D.; Ali, M.; Ren, J.; Yuan, G.H. Texture development and mechanical behavior of Zircaloy-4 alloy plates fabricated by cold rolling and annealing. Mater. Sci. Eng. A 2021, 807, 140846. [Google Scholar] [CrossRef]
- Deng, S.Y.; Song, H.W.; Zheng, C.; Zhao, T.Z.; Zhang, S.H.; Nielsen, K.B. Selection of deformation modes and related texture evolution in Zircaloy-4 during one pass cold pilgering. Mater. Sci. Eng. A 2019, 764, 138280. [Google Scholar] [CrossRef]
- Zhang, Y.D.; Li, G.P.; Liu, C.Z.; Yuan, F.S.; Han, F.Z.; Ali, M.; Guo, W.B.; Gu, H.F. The effect of three-dimensional loading and texture on deformation mechanism of Zircaloy-4 alloy: Using space Schmid factor model. Mater. Sci. Eng. A 2019, 761, 137992. [Google Scholar] [CrossRef]
- Xun, J.; Lin, G.Y.; Liu, H.Q.; Zhao, S.Y.; Chen, J.; Dai, X.; Zhang, R.Q. Texture Evolution and dynamic recrystallization of Zr-1Sn-0.3Nb-0.3Fe-0.1Cr Alloy During Hot Rolling. Acta Metall. Sin. Engl. Lett. 2020, 33, 215–224. [Google Scholar] [CrossRef]
- Li, J.H.; Cui, Y.; Wu, H.; Chen, G. Deformation mechanism of Zr-Sn-Nb-Fe cladding tube under various stress states. Mater. Sci. Eng. A 2020, 771, 138593. [Google Scholar] [CrossRef]
- Hiwarkar, V.D.; Sahoo, S.K.; Samajdar, I.; Narasimhan, K.; Krishna KV, M.; Dey, G.K.; Srivastava, D.; Tewari, R.; Banerjee, S. Annealing of cold worked two-phase Zr-2.5 Nb-Associated microstructural developments. J. Nucl. Mater. 2009, 384, 30–37. [Google Scholar] [CrossRef]
- Zou, L.; Li, H.; Wang, W.X.; Huang, Y.; Li, Y.T. A precision grinding technology for zirconium alloy tubes based on ultrasonic wall thickness automatic measurement system. Int. J. Adv. Manuf. Technol. 2022, 121, 4419–4429. [Google Scholar] [CrossRef]
- Murty, K.L.; Charit, I. Texture development and anisotropic deformation of zircaloys. Prog. Nucl. Energy 2006, 48, 325–359. [Google Scholar] [CrossRef]
- Murty, K.L. Zircaloy life prediction and new generation zircaloys for LWRs. Trans. Indian Inst. Met. 1997, 50, 533–562. [Google Scholar]
- Liu, H.; Deng, S.Y.; Chen, S.F.; Song, H.W.; Zhang, S.H.; Wang, B. Correlation of the anisotropic hardening behavior and texture features of cold rolled Zr-4 sheet under uniaxial tension. J. Mater. Sci. Technol. 2022, 119, 111–122. [Google Scholar] [CrossRef]
- Adamson, R.B.; Coleman, C.E.; Griffiths, M. Irradiation creep and growth of zirconium alloys: A critical review. J. Nucl. Mater. 2019, 521, 167–244. [Google Scholar] [CrossRef]
- Yang, H.L.; Kano, S.; Chai, L.J.; Shen, J.J.; Zhao, Z.S.; McGrady, J.; Duan, Z.G.; Abe, H. Interaction between slip and {10-12} tensile twinning in Zr alloy: Quasi in situ electron back scatter diffraction study under uniaxial tensile test. J. Alloys Compd. 2019, 782, 659–666. [Google Scholar] [CrossRef]
- Shi, H.G.; Li, J.X.; Mao, J.W.; Lu, W.J. The gradual disappearance and re-appearance of yield drop by modulating the pre-strain history in a new zirconium alloy: Dislocation decomposition and recombination. Scr. Mater. 2020, 188, 280–284. [Google Scholar] [CrossRef]
- Kaschner, G.C.; Tome, C.N.; Mccabe, R.J.; Misra, A.; Vogel, S.C.; Brown, D.W. Exploring the dislocation/twin interactions in zirconium. Mater. Sci. Eng. A 2007, 463, 122–127. [Google Scholar] [CrossRef]
- Liu, H.; Deng, S.Y.; Chen, S.F.; Song, H.W.; Zhang, S.H. Virtual design of formability for Zircaloy-4 sheet through texture control. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1157, 012069. [Google Scholar] [CrossRef]
- Lei, C.Y.; Mao, J.Z.; Zhang, X.M.; Liu, J.X.; Wang, L.; Chen, D. A comparison study of the yield surface exponent of the Barlat yield function on the forming limit curve prediction of zirconium alloys with M-K method. Int. J. Mater. Form. 2021, 14, 467–484. [Google Scholar] [CrossRef]
- Lei, C.Y.; Mao, J.Z.; Zhang, X.M.; Wang, L.; Chen, D. Crack prediction in sheet forming of zirconium alloys used in nuclear fuel assembly by support vector machine method. Energy Rep. 2021, 7, 5922–5932. [Google Scholar] [CrossRef]
- Takuda, H.; Hatta, N. Numerical analysis of formability of a commercially pure zirconium sheet in some sheet forming processes. Mater. Sci. Eng. A 1998, 242, 15–21. [Google Scholar] [CrossRef]
- Panchal, M.; Ravi, K.R.; Kaushik, L.; Khatirkar, R.; Choi, S.-H.; Singh, J. Texture control techniques for improving room temperature formability of Mg alloys including pre-twinning: A review. Met. Mater. Int. 2023, 29, 3471–3489. [Google Scholar] [CrossRef]
- Huang, X.S.; Suzuki, K.; Chino, Y.; Mabuchi, M. Influence of initial texture on cold deep drawability of Mg-3Al-1Zn alloy sheets. Mater. Sci. Eng. A 2013, 565, 359–372. [Google Scholar] [CrossRef]
- Barnwal, V.K.; Raghavan, R.; Tewari, A.; Narasimhan, K.; Mishra, S.K. Effect of microstructure and texture on forming behavior of AA6061 aluminium alloy sheet. Mater. Sci. Eng. A 2017, 679, 56–65. [Google Scholar] [CrossRef]
- Miller, V.M.; Berman, T.D.; Beyerlein, I.J.; Pollock, T. Prediction of Magnesium Alloy Formability: The Role of Texture. In Magnesium Technology; Wiley: Hoboken, NJ, USA, 2016; pp. 257–262. [Google Scholar]
- He, W.; Chen, X.; Liu, N.; Luan, B.; Yuan, G.; Liu, Q. Cryo-rolling enhanced inhomogeneous deformation and recrystallization grain growth of a zirconium alloy. J. Alloys Compd. 2017, 699, 160–169. [Google Scholar] [CrossRef]
- He, W.; Chapuis, A.; Chen, X.; Liu, Q. Effect of loading direction on the deformation and annealing behavior of a zirconium alloy. Mater. Sci. Eng. A 2018, 734, 364–373. [Google Scholar] [CrossRef]
- Kearns, J.J. On the relationship among ‘f’ texture factors for the principal planes of zirconium, hafnium and titanium alloys. J. Nucl. Mater. 2001, 299, 171–174. [Google Scholar] [CrossRef]
- Lebensohn, R.A.; Tomé, C.N. A self-consistent anisotropic approach for the simulation of plastic deformation and texture development of polycrystals: Application to zirconium alloys. Acta Metall. Mater. 1993, 41, 2611–2624. [Google Scholar] [CrossRef]
- Tomé, C.N.; Lebensohn, R.A.; Kocks, U.F. A model for texture development dominated by deformation twinning: Application to zirconium alloys. Acta Metall. Mater. 1991, 39, 2667–2680. [Google Scholar] [CrossRef]
- Singh, S.K.; Limbadri, K.; Singh, A.K.; Ram, A.M.; Ravindran, M.; Krishna, M.; Reddy, M.C.; Suresh, K.; Prasad, K.S.; Panda, S. Studies on texture and formability of Zircaloy-4 produced by pilgering route. J. Mater. Res. Technol. 2019, 8, 2120–2129. [Google Scholar] [CrossRef]

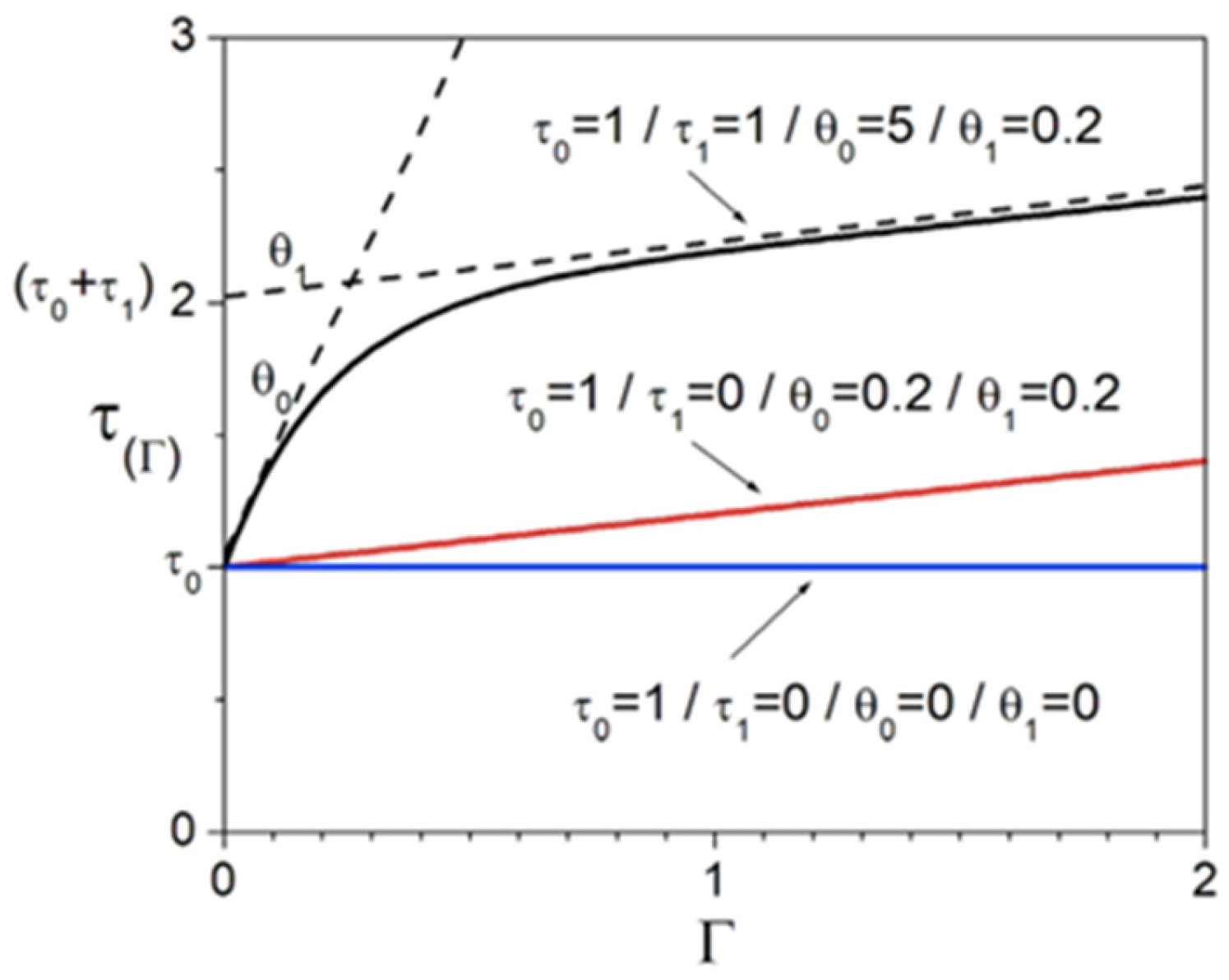

| Modes | τ0 (MPa) | τ1 (MPa) | θ0 | θ1 |
|---|---|---|---|---|
| Prismatic <a> | 60 | 5 | 400 | 200 |
| Basal <a> | 400 | 0 | 0 | 0 |
| Pyramidal <c + a> | 450 | 10 | 2000 | 200 |
| Extension twinning | 460 | 5 | 200 | 50 |
| Fn | n |
|---|---|
| 0.606 | 0.18 |
| 0.680 | 0.16 |
| 0.690 | 0.15 |
| 0.700 | 0.14 |
| 0.722 | 0.128 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Song, H.-W.; Deng, S.-Y.; Chen, S.-F.; Zhang, S.-H. Study on Formability Improvement of Zr-4 Sheets Based on Texture Optimization. Metals 2024, 14, 725. https://doi.org/10.3390/met14060725
Liu H, Song H-W, Deng S-Y, Chen S-F, Zhang S-H. Study on Formability Improvement of Zr-4 Sheets Based on Texture Optimization. Metals. 2024; 14(6):725. https://doi.org/10.3390/met14060725
Chicago/Turabian StyleLiu, Huan, Hong-Wu Song, Si-Ying Deng, Shuai-Feng Chen, and Shi-Hong Zhang. 2024. "Study on Formability Improvement of Zr-4 Sheets Based on Texture Optimization" Metals 14, no. 6: 725. https://doi.org/10.3390/met14060725
APA StyleLiu, H., Song, H.-W., Deng, S.-Y., Chen, S.-F., & Zhang, S.-H. (2024). Study on Formability Improvement of Zr-4 Sheets Based on Texture Optimization. Metals, 14(6), 725. https://doi.org/10.3390/met14060725










