Mechanisms of Low-Temperature Dislocation Motion in High-Entropy Al0.5CoCrCuFeNi Alloy
Abstract
1. Introduction
- Method of resonant mechanical spectroscopy—excitation in samples of cyclic elastic deformation with an amplitude of ~10−7, caused by short segments of dislocation strings (dislocation relaxers), which oscillate with amplitudes on the order of the lattice parameter;
- Method of active deformation—when used, significant plastic deformations of ~3 × 10−1 are achieved, caused by the translational movement of extended dislocations over macroscopic distances.
2. Materials and Methods of Research
3. Experimental Results
3.1. Acoustic Properties
3.2. Mechanical Properties
4. Low-Temperature Dislocation Processes in HEA Al0.5CoCrCuFeNi
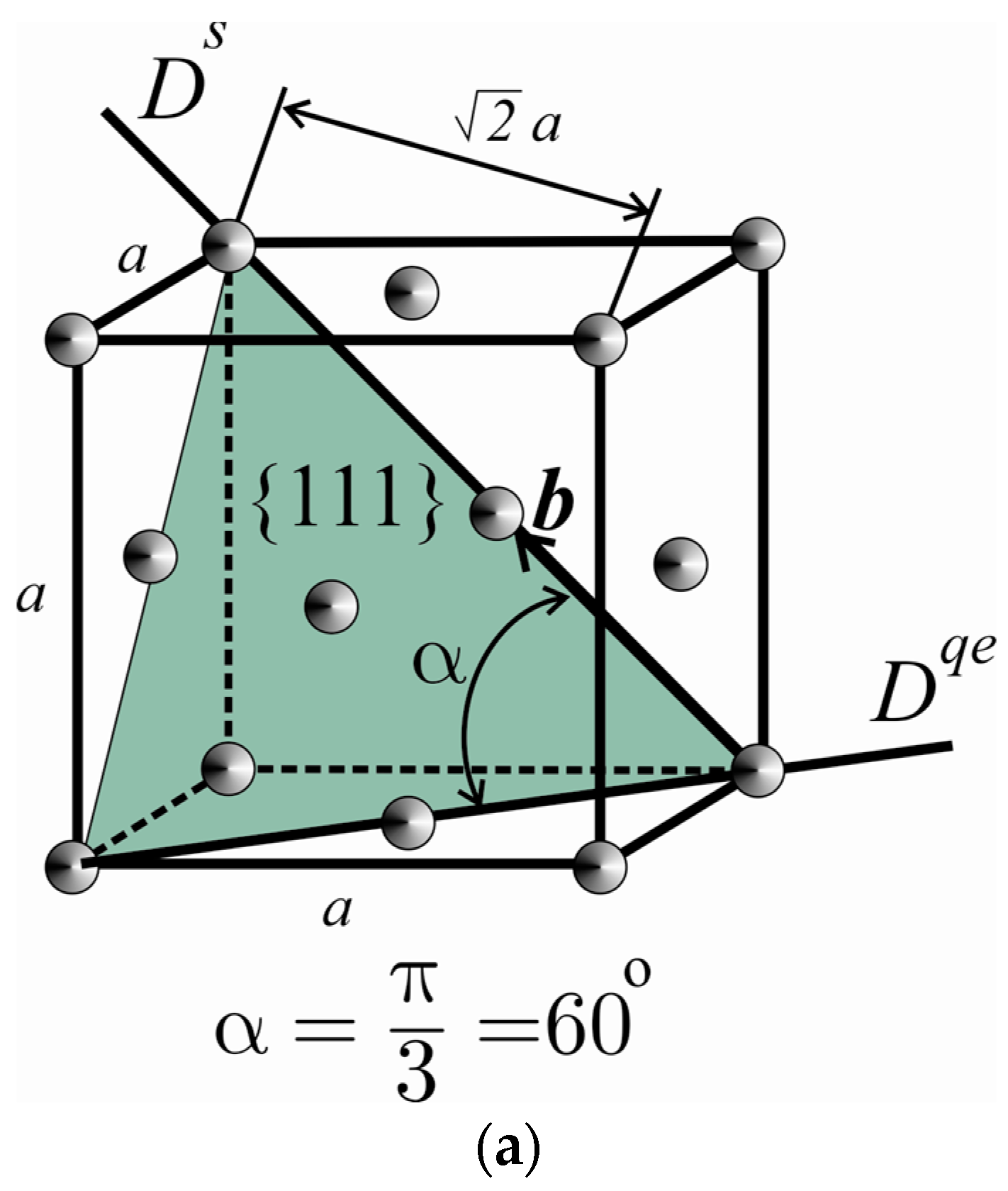
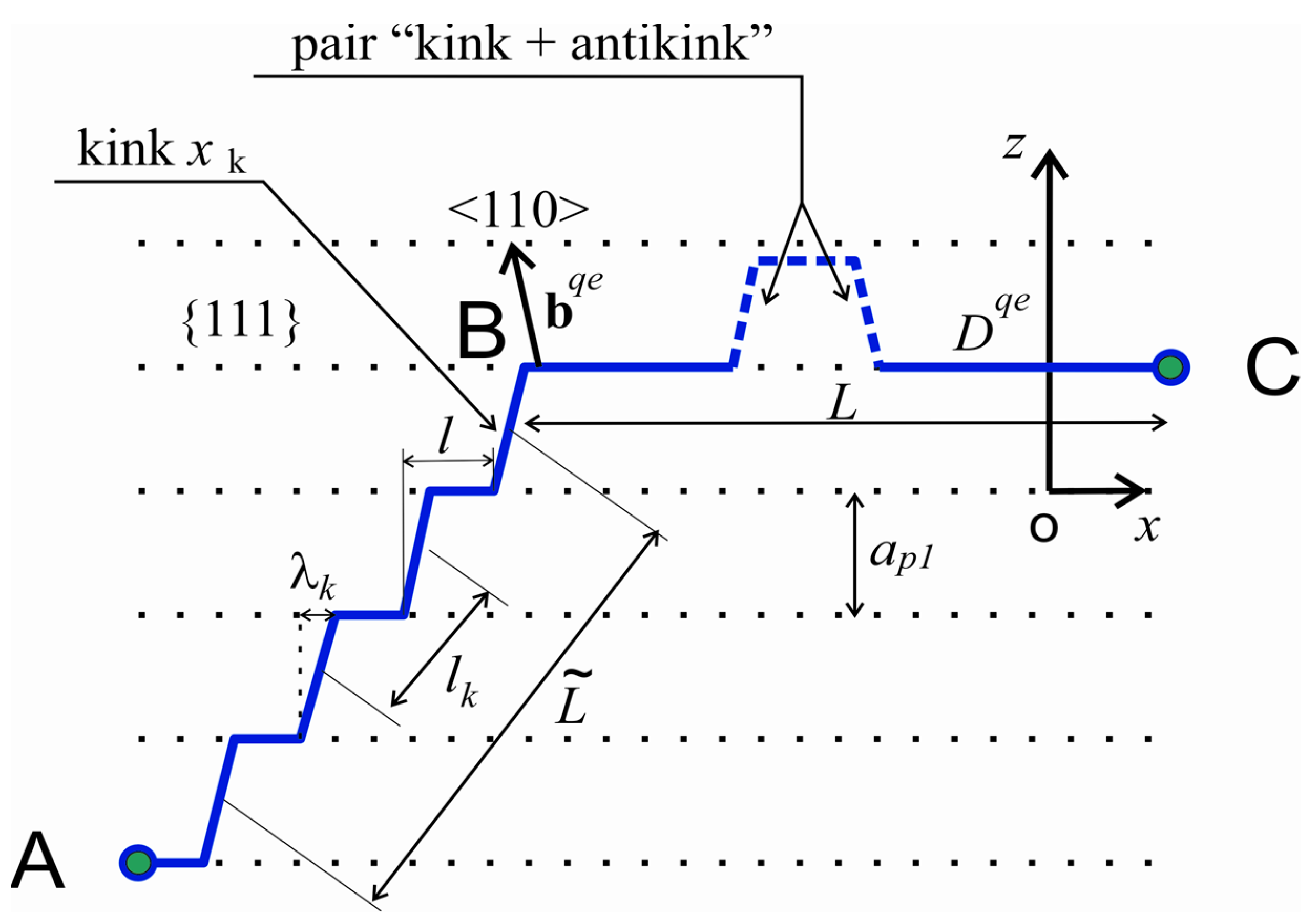
4.1. Models of Dislocation Relaxers
4.2. Thermal Activation and Statistical Analysis of the Dislocation Contribution to Acoustic Relaxation
- for Seeger relaxers,
- for Koiwa–Hasiguti relaxers,
- In [14], it is shown that these processes correspond to different values of the ratio of the peak height to the step height on the temperature–frequency dependences of the contributions of these relaxation processes to the internal friction and dynamic elasticity of materials:
- In [18], it was established that the parameter K determined by relation (7) does not depend on temperature and U0, and its frequency dependence is described by monotonic functions , while
4.3. Dislocation Mechanism of the Internal Friction Peak (Analogue of the HasigutiPeak)
4.4. Dislocation Mechanism of Internal Friction Peak (Analogue of Bordoni Peak)
4.5. Dislocation Processes of Low-Temperature Plastic Deformation
4.6. Low-Temperature Plasticity of the Present Alloy
5. Conclusions
- The most important types of dislocation defects in the lattice structure of the alloy;
- The types of barriers that prevent the movement of dislocation lines (strings);
- Adequate mechanisms of thermallyactivated movement of various elements of dislocation strings through barriers under conditions of moderate and deep cooling;
- Quantitative estimates for the most important characteristics of dislocations and their interaction with barriers.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yeh, J.; Chen, S.; Lin, S.; Gan, J.; Chin, T.; Shun, T.; Tsau, C.; Chang, S. Nanostructured High-Entropy Alloys with Multiple Principal Elements: Novel Alloy Design Concepts and Outcomes. Adv. Eng. Mater. 2004, 6, 299–303. [Google Scholar] [CrossRef]
- Cantor, B.; Chang, I.T.H.; Knight, P.; Vincent, A.J.B. Microstructural development in equi-atomic multicomponent alloys. Mater. Sci. Eng. A 2004, 375–377, 213–218. [Google Scholar] [CrossRef]
- Cantor, B. Multicomponent high-entropy Cantor alloys. Prog. Mater. Sci. 2021, 120, 100754. [Google Scholar] [CrossRef]
- Kao, Y.F.; Chen, T.J.; Chen, S.K.; Yeh, J.W. Microstructure and mechanical property of as-cast, homogenized and deformed AlxCoCrFeNi (0 ≤ x ≤ 2) high-entropy alloys. J. Alloys Compd. 2009, 488, 57–64. [Google Scholar] [CrossRef]
- Gludovatz, B.; Ritchie, R.O. Fracture properties of high-entropy alloys. MRS Bull. 2022, 47, 176–185. [Google Scholar] [CrossRef]
- Chen, S.; Oh, H.S.; Gludovatz, B.; Kim, S.J.; Park, E.S.; Zhang, Z.; Ritchie, R.O.; Yu, Q. Real-time observations of TRIP-induced ultrahigh strain hardening in a dual-phase CrMnFeCoNi high-entropy alloy. Nat. Commun. 2020, 11, 826. [Google Scholar] [CrossRef] [PubMed]
- Pogrebnjak, A.D.; Yakushchenko, I.V.; Bagdasaryan, A.A.; Bondar, O.V.; Krause-Rehberg, R.; Abadias, G.; Chartier, P.; Oyoshi, K.; Takeda, Y.; Beresnev, V.M.; et al. Microstructure, physical and chemical properties of nanostructured (Ti–Hf–Zr–V–Nb) N coatings under different deposition conditions. Mater. Chem. Phys. 2014, 147, 1079–1091. [Google Scholar] [CrossRef]
- Laktionova, M.A.; Tabchnikova, E.D.; Tang, Z.; Liaw, P.K. Mechanical properties of the high-entropy alloy Al0.5CoCrCuFeNi at temperatures of 4.2–300 K. Low Temp. Phys. 2013, 39, 630–632. [Google Scholar] [CrossRef]
- Naeem, M.; He, H.; Zhang, F.; Huang, H.; Harjo, S.; Kawasaki, T.; Wang, B.; Lan, S.; Wu, Z.; Wang, F.; et al. Cooperative deformation in high-entropy alloys at ultralow temperatures. Sci. Adv. 2020, 6, eaax4002. [Google Scholar] [CrossRef]
- Semerenko, Y.O.; Tabachnikova, O.D.; Tikhonovska, T.M.; Kolodiy, I.V.; Tortika, O.S.; Shumilin, S.E.; Laktionova, M.O. Temperature Dependence of the Acoustic and Mechanical Properties of Cast and Annealed High-Entropy Al0.5CoCuCrNiFe Alloy. Metallofiz. Noveishie Tekhnol. 2015, 37, 1527–1538. [Google Scholar] [CrossRef][Green Version]
- Tabachnikova, E.D.; Laktionova, M.A.; Semerenko, Y.A.; Shumilin, S.E.; Podolskiy, A.V.; Tikhonovsky, M.A.; Miskuf, J.; Csach, K. Mechanical properties of the high-entropy alloy Al0.5CoCrCuFeNi in various structural states at temperatures of 0.5–300 K. Low Temp. Phys. 2017, 43, 1108–1118. [Google Scholar] [CrossRef]
- Semerenko, Y.A.; Natsik, V.D. Low temperature peak of internal friction in high entropy Al0.5CoCrCuFeNi alloy. Low Temp. Phys. 2020, 46, 78–86. [Google Scholar] [CrossRef]
- Pickering, E.J.; Stone, H.J.; Jones, N.G. Fine-scale precipitation in the high-entropy alloy Al0.5CrFeCoNiCu. Mat. Sci. Eng. A 2015, 645, 65–71. [Google Scholar] [CrossRef]
- Natsik, V.D.; Semerenko, Y.A. Dislocation mechanisms of low-temperature acoustic relaxation in iron. Low Temp. Phys. 2019, 45, 551–567. [Google Scholar] [CrossRef]
- Varshni, Y.P. Temperature Dependence of the Elastic Constants. Phys. Rev. B 1970, 2, 3952. [Google Scholar] [CrossRef]
- Girifalco, L.A. Statistical Physics of Materials; Wiley: New York, NY, USA, 1973; p. 362. [Google Scholar]
- Schoeck, G.; Bisogni, E.; Shyne, J. The activation energy of high temperature internal friction. Acta Metall. 1964, 12, 1466–1468. [Google Scholar] [CrossRef]
- Natsik, V.D.; Semerenko, Y.A. Dislocation mechanisms of low-temperature internal friction in nanostructured materials. Low Temp. Phys. 2016, 42, 138–148. [Google Scholar] [CrossRef]
- Asgari, S.; El-Danaf, E.; Kalidindi, S.R.; Doherty, R.D. Strain hardening regimes and microstructural evolution during large strain compression of low stacking fault energy FCC alloys that form deformation twins. Metall. Mater. Trans. A 1997, 28, 1781–1795. [Google Scholar] [CrossRef]
- Evans, A.; Rawlings, R. The thermally activated deformation of crystalline materials. Phys. Status Solidi 1969, 34, 9–31. [Google Scholar] [CrossRef]
- Niblett, D.H. Bordoni Peak in Face-Centered Cubic Metals. In Physical Acoustics: Principles and Methods. Volume III, Part A. The Effect of Imperfections; Mason, W.P., Ed.; Academic Press: New York, NY, USA, 1966; p. 428. [Google Scholar]
- Chambers, R. Dislocation relaxations in Base-Centered Cubic Transition Metals. In Physical Acoustics, Volume III, Part A; Mason, W.P., Ed.; Academic Press: New York, NY, USA, 1964; p. 428. [Google Scholar]
- Bordoni, P.G. Elastic and anelastic behavior of some metals at very low temperatures. J. Acoust. Soc. Am. 1954, 26, 495–502. [Google Scholar] [CrossRef]
- Postnikov, V.S. Internal Friction in Metals and Alloys, 1st ed.; Springer: New York, NY, USA, 1967; p. 266. [Google Scholar]
- Seeger, A. On the theory of the low-temperature internal friction peak observed in metals. Phyl. Mag. 1956, 1, 651–662. [Google Scholar] [CrossRef]
- Seeger, A.; Schiller, P. Kinks in Dislocation Lines and theit Effects on the Internal Friction in Crystals. In Physical Acoustics, Volume III, Part A; Mason, W.P., Ed.; Academic Press: New York, NY, USA, 1964; p. 428. [Google Scholar]
- Seeger, A.; Wüthrich, C. Dislocation relaxation processes in body-centred cubic metals. Nuovo Cimento B 1976, 33, 38–75. [Google Scholar] [CrossRef]
- Friedel, J. Dislocations; Pergamon Press: Oxford, UK, 1964; p. 491. [Google Scholar]
- Anderson, P.M.; Hirth, J.P.; Lothe, J. Theory of Dislocations, 3rd ed.; Cambridge University Press: Cambridge, UK, 2017; p. 699. [Google Scholar]
- Koiwa, M.; Hasiguti, R.R. A theory of internal friction peak due to thermal unpinning of dislocations and its application to P1 peak in copper. Acta Met. 1965, 13, 1219–1230. [Google Scholar] [CrossRef]
- Fitzgerald, S.P. Kink pair production and dislocation motion. Sci. Rep. 2016, 6, 39708. [Google Scholar] [CrossRef]
- Utt, D.; Lee, S.; Xing, Y.; Jeong, H.; Stukowski, A.; Oh, S.H.; Dehm, G.; Albe, K. The origin of jerky dislocation motion in high-entropy alloys. Nat. Commun. 2022, 13, 4777. [Google Scholar] [CrossRef]
- Bulatov, O.S.; Klochko, V.S.; Korniyets, A.V.; Kolodiy, I.V.; Kondratov, O.O.; Tikhonovska, T.M. Low temperature elastic properties of Al0.5CoCrCuFeNi high-entropy alloy. Funct. Mater. 2021, 28, 492–496. [Google Scholar] [CrossRef]
- Pustovalov, V.V.; Fomenko, V.S. Plastic Deformation of Crystals at Low Temperatures; Naukova Dumka: Kiev, Ukraine, 2012; p. 356. [Google Scholar]
- Moskalenko, V.A.; Natsik, V.D.; Kovaleva, V.N. The role of Peierls relief in the low-temperature plasticity of pure α-Ti. Low Temp. Phys. 2005, 31, 907–914. [Google Scholar] [CrossRef]
- Kocks, U.F.; Argon, A.S.; Ashby, M.F. Thermodynamics and Kinetics of Slip, 1st ed.; Progress in Materials Science; Pergamon Press: Oxford, UK, 1975; p. 288. [Google Scholar]
- Schwarz, B.; Isaac, R.D.; Granato, A.V. Dislocation Inertial Effects in the Plastic Deformation of Dilute Alloys of Lead and Copper. Phys. Rew. Lett. 1977, 38, 554–557. [Google Scholar] [CrossRef]
- Schwarz, B.; Labusch, R.J. Dynamic simulation of solution hardening. J. Appl. Phys. 1978, 49, 5174–5187. [Google Scholar] [CrossRef]





| A1 | A2 | ||||||
|---|---|---|---|---|---|---|---|
| 3.34·103 s−1 | 236 GPa | 3.5·10−4 K−1 | 160 K | 0.33 GPa | 6·10−5 | 0.3 | 0.16 eV |
| (a) | ||||||
| 228 K | 213 K | 242 K | 1.5·10−4 | 7.6·10−6 K−1 | −9.1·10−6 K−1 | 0.83 |
| (b) | ||||||
| 190 K | 182.6 K | 202.0 K | 2.64·10−5 | 3.1·10−6 K−1 | −2.5·10−6 K−1 | 1.24 |
| 2 × 10−13 s | 0.43 eV | 0.01 eV | 4 × 10−4 | 4 × 10−11 s | 0.07 eV | 0.01 eV | 1 × 10−4 |
| , MPa | , MPa | , eV | , K | |||
|---|---|---|---|---|---|---|
| 0.6 | 1.1 | 170 | 104 | 0.65 | 400 | 19 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Semerenko, Y.O.; Natsik, V.D.; Tabachnikova, E.D.; Huang, Y.; Langdon, T.G. Mechanisms of Low-Temperature Dislocation Motion in High-Entropy Al0.5CoCrCuFeNi Alloy. Metals 2024, 14, 778. https://doi.org/10.3390/met14070778
Semerenko YO, Natsik VD, Tabachnikova ED, Huang Y, Langdon TG. Mechanisms of Low-Temperature Dislocation Motion in High-Entropy Al0.5CoCrCuFeNi Alloy. Metals. 2024; 14(7):778. https://doi.org/10.3390/met14070778
Chicago/Turabian StyleSemerenko, Yuri O., Vasilij D. Natsik, Elena D. Tabachnikova, Yi Huang, and Terence G. Langdon. 2024. "Mechanisms of Low-Temperature Dislocation Motion in High-Entropy Al0.5CoCrCuFeNi Alloy" Metals 14, no. 7: 778. https://doi.org/10.3390/met14070778
APA StyleSemerenko, Y. O., Natsik, V. D., Tabachnikova, E. D., Huang, Y., & Langdon, T. G. (2024). Mechanisms of Low-Temperature Dislocation Motion in High-Entropy Al0.5CoCrCuFeNi Alloy. Metals, 14(7), 778. https://doi.org/10.3390/met14070778








