Tensile Fracture Behavior of Progressively-Drawn Pearlitic Steels
Abstract
:1. Introduction
2. Experimental Program
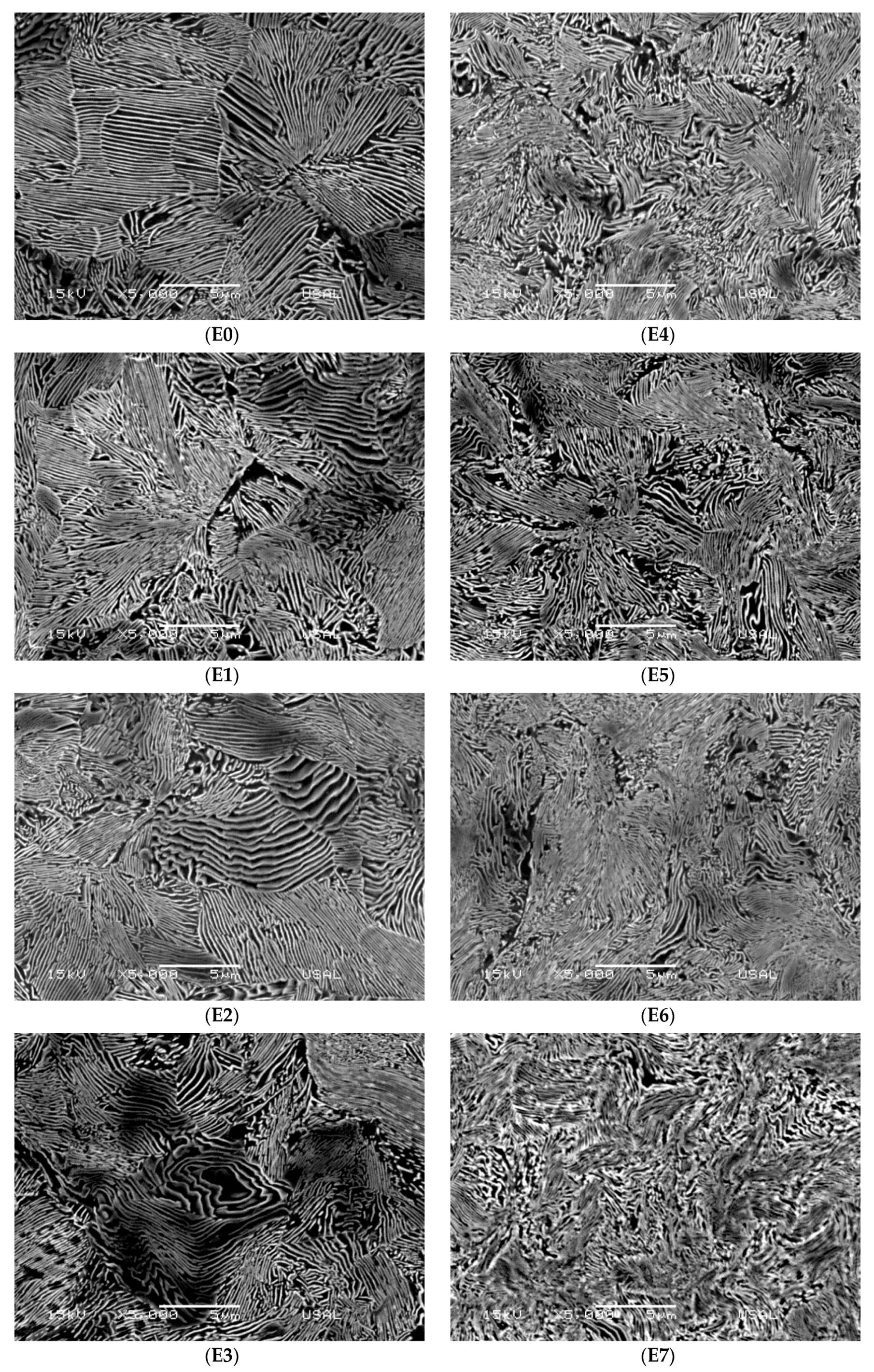
3. Microstructure of the Progressively-Drawn Wires
4. Mechanical Behavior of the Progressively-Drawn Wires
5. Fractographic Analysis
- Total fracture surface (SF).
- Surface of the FPZ (SFPZ).
- Radius of the FPZ (rFPZ).
- Surface of the external crown formed by MVC (SEC).
- Mean or average depth (xm), maximum depth (xmax) and minimum depth (xmin) of the external crown formed by MVC.
6. Discussion
7. Conclusions
- The fracture initiates at the central zone of each wire in which the fracture microscopic topography may be classified as micro-void coalescence (MVC) with fibrous aspect and propagates in a radial direction through the intermediate zone up to reaching the external ring.
- The intermediate zone shows a fractography by cleavage and MVC. The fracture surface by cleavage diminishes in favor of MVC in the last steps of the manufacturing process by cold drawing, so that the fracture process becomes more ductile as the drawing degree increases.
- Another important characteristic of such an intermediate zone is the presence of radial cracks oriented quasi-parallel to the drawing axis or cold drawing direction, embryos of anisotropic fracture behavior as a consequence of manufacture-induced microstructural orientation.
- With regard to the percentage values of the different parts in the fracture surface, the evolution with cold drawing of the central zone of the fibrous aspect (fracture process zone or FPZ) is similar in all analyzed steels and the same happens in the matter of the external ring (shear lip).
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| FPZ | Fracture Process Zone |
| SEM | Scanning Electron Microscopy |
| MFM | Micro-Fracture Map |
| MVC | Micro-Void Coalescence |
References
- Tao, Z. Mechanical properties of prestressing steel after fire exposure. Mater. Struct. 2015, 48, 3037–3047. [Google Scholar] [CrossRef]
- Hredil, M.I.; Toribio, J. Corrosion resistance of prestressing steel wires. Mater. Sci. 2015, 50, 665–670. [Google Scholar] [CrossRef]
- Jin, X.; Wen, Z.; Xiao, X.; Zhou, Z. A numerical method for prediction of curved rail wear. Multibody Syst. Dyn. 2007, 18, 531–557. [Google Scholar] [CrossRef]
- Ostash, O.P.; Andreiko, I.M.; Kulyk, V.V.; Vavrukh, V.I. Influence of braking on the microstructure and mechanical behavior of railroad wheel steels. Mater. Sci. 2013, 48, 569–574. [Google Scholar] [CrossRef]
- Christodoulou, I.A.; Kermanidis, A.T.; Haidemenopoulos, G.N. Fatigue and fracture behavior of pearlitic Grade 900A steel used in railway applications. Theor. Appl. Fract. Mech. 2016, 83, 51–59. [Google Scholar] [CrossRef]
- Gamboa, E.; Atrens, A. Material influence on the stress corrosion cracking of rock bolts. Eng. Fail. Anal. 2005, 12, 201–235. [Google Scholar] [CrossRef]
- Lee, S.K.; Ko, D.C.; Kim, B.M. Pass schedule of wire drawing process to prevent delamination for high strength steel cord wire. Mater. Des. 2009, 30, 2919–2927. [Google Scholar] [CrossRef]
- Bramfitt, B.L.; Mridha, S. Steels: Near Eutectoid. In Reference Module in Materials Science and Materials; Hashmi, M.S.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Toribio, J.; Lorenzo, M.; Vergara, D.; Kharin, V. Hydrogen degradation of cold-drawn wires: A numerical analysis of drawing-induced residual stresses and strains. Corrosion 2011. [Google Scholar] [CrossRef]
- Elices, M. Influence of residual stresses in the performance of cold-drawn pearlitic wires. J. Mater. Sci. 2004, 39, 3889–3899. [Google Scholar] [CrossRef]
- Toribio, J.; Kharin, V.; Lorenzo, M.; Vergara, D. Role of drawing-induced residual stresses and strains in the hydrogen embrittlement susceptibility of prestressing steels. Corros. Sci. 2011, 53, 3346–3355. [Google Scholar] [CrossRef]
- Toribio, J.; Ovejero, E. Microstructure evolution in a pearlitic steel subjected to progressive plastic deformation. Mater. Sci. Eng. A 1997, 234–236, 579–582. [Google Scholar] [CrossRef]
- Toribio, J.; Ovejero, E. Microstructure orientation in a pearlitic steel subjected to progressive plastic deformation. J. Mater. Sci. Lett. 1998, 17, 1037–1040. [Google Scholar] [CrossRef]
- Toribio, J.; Ovejero, E. Effect of cumulative cold drawing on the pearlite interlamellar spacing in eutectoid steel. Scr. Mater. 1998, 39, 323–328. [Google Scholar] [CrossRef]
- Toribio, J.; Ovejero, E. Effect of cold drawing on microstructure and corrosion performance of high-strength steel. Mech. Time-Depend. Mater. 1998, 1, 307–319. [Google Scholar] [CrossRef]
- Zelin, M. Microstructure evolution in pearlitic steels during wire drawing. Acta Mater. 2002, 50, 4431–4447. [Google Scholar] [CrossRef]
- Sauvage, X.; Guelton, N.; Blavette, D. Microstructure evolutions during drawing of a pearlitic steel containing 0.7 at. % copper. Scr. Mater. 2002, 46, 459–464. [Google Scholar] [CrossRef]
- Guo, N.; Luan, B.; Wang, B.; Liu, Q. Deformation bands in fully pearlitic steel during wire drawing. Sci. China Technol. Sci. 2014, 57, 796–803. [Google Scholar] [CrossRef]
- Fang, F.; Zhao, Y.; Liu, P.; Zhou, L.; Hu, X.J.; Zhou, X.; Xie, Z.H. Deformation of cementite in cold drawn pearlitic steel wire. Mater. Sci. Eng. A 2014, 608, 11–15. [Google Scholar] [CrossRef]
- Zhang, X.; Godfrey, A.; Huang, X.; Hansen, N.; Liu, Q. Microstructure and strengthening mechanisms in cold-drawn pearlitic steel wire. Acta Mater. 2011, 59, 3422–3430. [Google Scholar] [CrossRef]
- Fang, F.; Zhou, L.; Hu, X.; Zhou, X.; Tu, Y.; Xie, Z.; Jiang, J. Microstructure and mechanical properties of cold-drawn pearlitic wires affect by inherited texture. Mater. Des. 2015, 79, 60–67. [Google Scholar] [CrossRef]
- Rastegari, H.; Kermanpur, A.; Najafizadeh, A. Effect of initial microstructure on the work hardening behavior of plain eutectoid steel. Mater. Sci. Eng. A 2015, 632, 103–109. [Google Scholar] [CrossRef]
- Toribio, J.; Kharin, V.; Ayaso, F.J.; González, B.; Matos, J.C.; Vergara, D.; Lorenzo, M. Numerical and experimental analyses of the plasticity-induced fatigue crack growth in high-strength steels. Const. Build. Mater. 2011, 25, 3935–3940. [Google Scholar] [CrossRef]
- Toribio, J.; Matos, J.C.; González, B. A macro- and micro-approach to the anisotropic fatigue behaviour of hot-rolled and cold-drawn pearlitic steel. Eng. Fract. Mech. 2014, 123, 70–76. [Google Scholar] [CrossRef]
- Toribio, J.; Ayaso, F.J.; González, B.; Matos, J.C.; Vergara, D.; Lorenzo, M. Critical stress intensity factors in steel cracked wires. Mater. Des. 2011, 32, 4424–4429. [Google Scholar] [CrossRef]
- Toribio, J.; González, B.; Matos, J.C. Strength anisotropy and mixed mode fracture in heavily drawn pearlitic steel. Fat. Fract. Eng. Mater. Struct. 2013, 36, 1178–1186. [Google Scholar] [CrossRef]
- Toribio, J.; Vergara, D.; Lorenzo, M. Influence of loading rate on the hydrogen-assisted micro-damage in bluntly notched samples of pearlitic steel. Metals 2016, 6, 11. [Google Scholar] [CrossRef]
- Meseguer-Valdenebro, J.L.; Miguel, V.; Caravaca, M.; Portolés, A.; Gimeno, F. Teaching mechanical properties of different steels for engineering students. J. Mater. Educ. 2015, 37, 103–118. [Google Scholar]
- Toribio, J. On the intrinsic character of the stress-strain curve of a prestressing steel. J. Test. Eval. 1992, 20, 357–362. [Google Scholar] [CrossRef]
- Zhang, X.; Godfrey, A.; Hansen, N.; Huang, X.; Liu, W.; Liu, Q. Evolution of cementite morphology in pearlitic steel wire during wet wire drawing. Mater. Charact. 2010, 61, 65–72. [Google Scholar] [CrossRef]
- Zhang, X.; Godfrey, A.; Hansen, N.; Huang, X. Hierarchical structures in cold-drawn pearlitic steel wire. Acta Mater. 2013, 61, 4898–4909. [Google Scholar] [CrossRef] [Green Version]















| Element | Family of Steels | ||||
|---|---|---|---|---|---|
| A | B | C | D | E | |
| % C | 0.800 | 0.789 | 0.790 | 0.795 | 0.789 |
| % Mn | 0.690 | 0.698 | 0.670 | 0.624 | 0.681 |
| % Si | 0.230 | 0.226 | 0.200 | 0.224 | 0.210 |
| % P | 0.012 | 0.011 | 0.009 | 0.011 | 0.010 |
| % S | 0.009 | 0.005 | 0.009 | 0.008 | 0.008 |
| % Al | 0.004 | 0.003 | 0.003 | 0.003 | 0.003 |
| % Cr | 0.265 | 0.071 | 0.187 | 0.164 | 0.218 |
| % V | 0.060 | 0.078 | 0.053 | 0.064 | 0.061 |
| Drawing Step | Wire Diameter (mm) | ||||
|---|---|---|---|---|---|
| Family A | Family B | Family C | Family D | Family E | |
| 0 | 12.11 | 12.10 | 10.44 | 8.56 | 11.03 |
| 1 | 10.80 | 11.23 | 9.52 | 7.78 | 9.90 |
| 2 | 9.81 | 10.45 | 8.49 | 6.82 | 8.95 |
| 3 | 8.94 | 9.68 | 7.68 | 6.17 | 8.21 |
| 4 | 8.22 | 9.02 | 6.95 | 5.61 | 7.49 |
| 5 | 7.56 | 8.54 | 6.36 | 5.08 | 6.80 |
| 6 | 6.98 | 8.18 | 5.86 | 4.63 | 6.26 |
| 7 | - | 7.00 | 5.03 | 3.97 | 5.04 |
| Drawing Step | εP | ||||
|---|---|---|---|---|---|
| Family A | Family B | Family C | Family D | Family E | |
| 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | 0.229 | 0.149 | 0.184 | 0.191 | 0.216 |
| 2 | 0.421 | 0.293 | 0.414 | 0.454 | 0.418 |
| 3 | 0.607 | 0.446 | 0.614 | 0.655 | 0.591 |
| 4 | 0.775 | 0.588 | 0.814 | 0.845 | 0.774 |
| 5 | 0.942 | 0.697 | 0.991 | 1.044 | 0.967 |
| 6 | 1.102 | 0.800 | 1.155 | 1.229 | 1.133 |
| 7 | - | 1.095 | 1.460 | 1.537 | 1.566 |
| Steel | E (GPa) | σY (GPa) | σR (GPa) | εR |
|---|---|---|---|---|
| Family A | ||||
| A0 | 194 | 0.72 | 1.27 | 0.076 |
| A1 | 201 | 1.10 | 1.29 | 0.018 |
| A2 | 187 | 1.12 | 1.45 | 0.030 |
| A3 | 190 | 1.18 | 1.52 | 0.028 |
| A4 | 190 | 1.26 | 1.58 | 0.026 |
| A5 | 195 | 1.33 | 1.65 | 0.022 |
| A6 | 207 | 1.57 | 1.84 | 0.054 |
| Family B | ||||
| B0 | 202 | 0.72 | 1.27 | 0.066 |
| B1 | 204 | 0.84 | 1.34 | 0.057 |
| B2 | 204 | 0.88 | 1.37 | 0.061 |
| B3 | 203 | 0.95 | 1.43 | 0.052 |
| B4 | 203 | 1.01 | 1.49 | 0.042 |
| B5 | 201 | 1.09 | 1.55 | 0.038 |
| B6 | 201 | 1.12 | 1.58 | 0.035 |
| B7 | 205 | 1.58 | 1.84 | 0.052 |
| Family C | ||||
| C0 | 203 | 0.69 | 1.23 | 0.066 |
| C1 | 199 | 0.78 | 1.27 | 0.061 |
| C2 | 201 | 0.90 | 1.36 | 0.046 |
| C3 | 204 | 0.97 | 1.42 | 0.047 |
| C4 | 204 | 1.06 | 1.50 | 0.043 |
| C5 | 204 | 1.14 | 1.58 | 0.045 |
| C6 | 204 | 1.23 | 1.64 | 0.042 |
| C7 | 208 | 1.65 | 1.91 | 0.051 |
| Family D | ||||
| D0 | 194 | 0.68 | 1.23 | 0.072 |
| D1 | 192 | 0.84 | 1.32 | 0.056 |
| D2 | 189 | 0.99 | 1.42 | 0.038 |
| D3 | 194 | 1.00 | 1.49 | 0.045 |
| D4 | 200 | 1.07 | 1.55 | 0.048 |
| D5 | 202 | 1.16 | 1.63 | 0.051 |
| D6 | 202 | 1.25 | 1.69 | 0.047 |
| D7 | 206 | 1.65 | 1.88 | 0.057 |
| Family E | ||||
| E0 | 199 | 0.72 | 1.23 | 0.068 |
| E1 | 192 | 0.83 | 1.28 | 0.056 |
| E2 | 194 | 0.91 | 1.36 | 0.049 |
| E3 | 192 | 0.93 | 1.41 | 0.055 |
| E4 | 196 | 1.02 | 1.50 | 0.049 |
| E5 | 199 | 1.13 | 1.60 | 0.048 |
| E6 | 200 | 1.16 | 1.62 | 0.043 |
| E7 | 208 | 1.49 | 1.83 | 0.059 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Toribio, J.; Ayaso, F.-J.; González, B.; Matos, J.-C.; Vergara, D.; Lorenzo, M. Tensile Fracture Behavior of Progressively-Drawn Pearlitic Steels. Metals 2016, 6, 114. https://doi.org/10.3390/met6050114
Toribio J, Ayaso F-J, González B, Matos J-C, Vergara D, Lorenzo M. Tensile Fracture Behavior of Progressively-Drawn Pearlitic Steels. Metals. 2016; 6(5):114. https://doi.org/10.3390/met6050114
Chicago/Turabian StyleToribio, Jesús, Francisco-Javier Ayaso, Beatriz González, Juan-Carlos Matos, Diego Vergara, and Miguel Lorenzo. 2016. "Tensile Fracture Behavior of Progressively-Drawn Pearlitic Steels" Metals 6, no. 5: 114. https://doi.org/10.3390/met6050114
APA StyleToribio, J., Ayaso, F.-J., González, B., Matos, J.-C., Vergara, D., & Lorenzo, M. (2016). Tensile Fracture Behavior of Progressively-Drawn Pearlitic Steels. Metals, 6(5), 114. https://doi.org/10.3390/met6050114








