Triplet Test on Rubble Stone Masonry: Numerical Assessment of the Shear Mechanical Parameters
Abstract
:1. Introduction
2. The Triplet Test
2.1. Description of the Test
2.2. Description of the Experiments
3. Description of the Numerical Model
4. Simulating the Experimental Tests
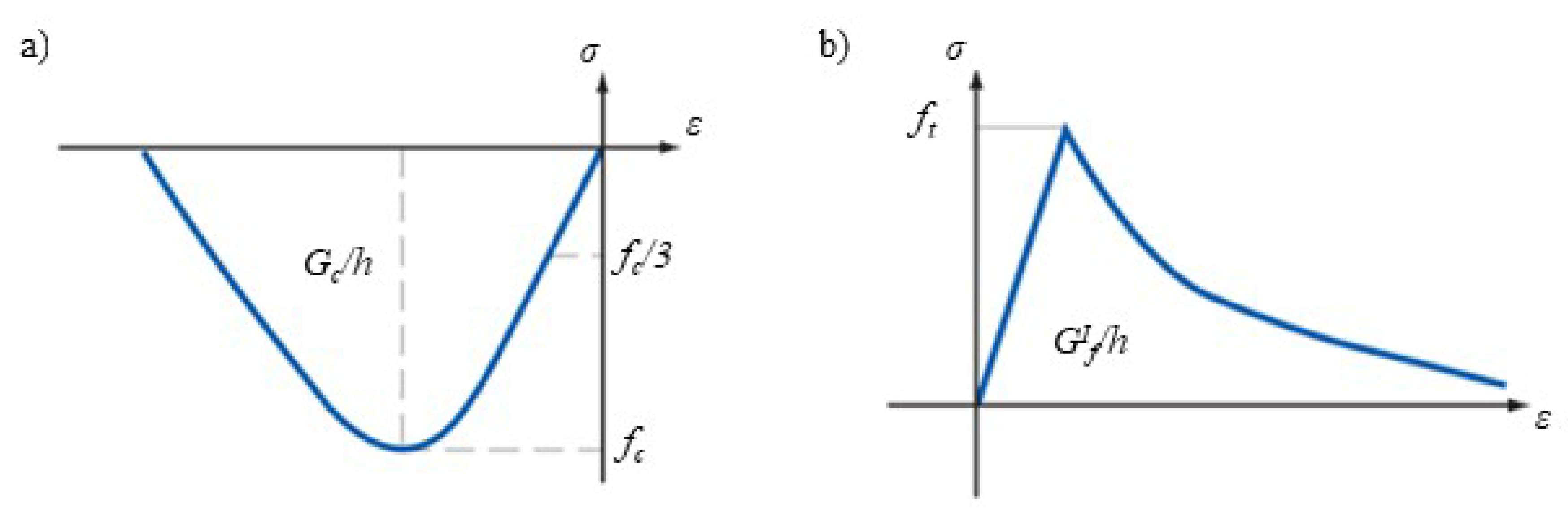
4.1. Calibration of the Material Parameters
4.2. Numerical Assessment of the Shear Mechanic Parameters
4.2.1. Confinement Effect
4.2.2. Bond Effect at the Masonry-Plate Interface
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Brandonisio, G.; Lucibello, G.; Mele, E.; De Luca, A. Damage and performance evaluation of masonry churches in the 2009 L’Aquila earthquake. Eng. Fail. Anal. 2013, 34, 693–714. [Google Scholar] [CrossRef]
- Milani, G.; Valente, M. Failure analysis of seven masonry churches severely damaged during the 2012 Emilia-Romagna (Italy) earthquake: Non-linear dynamic analyses vs conventional static approaches. Eng. Fail. Anal. 2015, 54, 13–56. [Google Scholar] [CrossRef]
- Azzaro, R.; Tertulliani, A.; Bernardini, F.; Camassi, R.; Del Mese, S.; Ercolani, E.; Graziani, L.; Locati, M.; Maramai, A.; Pessina, V.; et al. The 24 August 2016 Amatrice earthquake: Macroseismic survey in the damage area and EMS intensity assessment. Ann. Geophys. 2016. [Google Scholar] [CrossRef]
- Tinti, E.; Scognamiglio, L.; Michelini, A.; Cocco, M. Slip heterogeneity and directivity of the ML 6.0, 2016, Amatrice earthquake estimated with rapid finite-fault inversion. Geophys. Res. Lett. 2016, 43, 10–745. [Google Scholar] [CrossRef]
- Penna, A.; Calderini, C.; Sorrentino, L.; Carocci, C.F.; Cescatti, E.; Sisti, R.; Borri, A.; Modena, C.; Prota, A. Damage to churches in the 2016 central Italy earthquakes. Bull. Earthq. Eng. 2019, 17, 5763–5790. [Google Scholar] [CrossRef]
- Bosiljkov, V.; Page, A.; BOKAN, B.V. Performance Based Studies of in-Plane Loaded Unreinforced Masonry. Br. Mason. Soc. 2003, 16, 39–50. [Google Scholar]
- Vasconcelos, G.; Lourenço, P. Experimental characterization of stone masonry in shear and compression. Constr. Build. Mater. 2009, 23, 3337–3345. [Google Scholar] [CrossRef]
- Milosevic, J.; Gago, A.S.; Lopes, M.; Bento, R. Experimental assessment of shear strength parameters on rubble stone masonry specimens. Constr. Build. Mater. 2013, 47, 1372–1380. [Google Scholar] [CrossRef]
- Marino, M.; Neri, F.; De Maria, A.; Borri, A. Experimental data of friction coefficient for some types of masonry and its correlation with an Index of Quality Masonry (IQM). In Proceedings of the 2nd European Conference on Earthquake Engineering and Seismology, Instanbul, Turkey, 25–29 August 2014; Volume 1, pp. 25–29. [Google Scholar]
- Borri, A.; De Maria, A. Indice di Qualità Muraria (IQM): Correlazione con le caratteristiche meccaniche e livelli di conoscenza. Progett. Sismica 2015, 6, 45–63. [Google Scholar]
- Corradi, M.; Borri, A.; Vignoli, A. Experimental evaluation of in-plane shear behavior of masonry walls retrofitted using conventional and innovative methods. Mason. Int. 2008, 21, 29. [Google Scholar]
- Tomazevic, M. The Influence of Rigidity of Floors on the Seismic Resistance of of Old Masonry Buildings: Shaking-Table Tests of Stone-Masonry Houses: Summary Report; ZRMK: Ljubljana, Slovenia, 1992. [Google Scholar]
- Modena, C.; Bettio, C. Experimental characterisation and modeling of injected and jacketed masonry walls. In Proceedings of the Italian-French Symposium Strengthening and Repair of Structures in Seismic Area, Nizza, France, 17–19 October 1994; Volume 1, pp. 273–282. [Google Scholar]
- Modena, C. Interpretazione Dei Risultati Ottenuti Dalle Prove in Sito Nell’Ambito Delle Tre Convenzioni Con Gli Istituti Di Ricerca Di Firenze e Milano e Modellazione Del Comportamento Strutturale Dei Componenti Rinforzati. Convenzione di ricerca tra la Regione Toscana e il Dipartimento di Costruzioni e Trasporti dell’Università degli Studi di Padova 1999, 1, 1–89. [Google Scholar]
- Corradi, M.; Borri, A.; Vignoli, A. Experimental study on the determination of strength of masonry walls. Constr. Build. Mater. 2003, 17, 325–337. [Google Scholar] [CrossRef]
- Borri, A.; Castori, G.; Corradi, M.; Speranzini, E. Shear behavior of unreinforced and reinforced masonry panels subjected to in situ diagonal compression tests. Constr. Build. Mater. 2011, 25, 4403–4414. [Google Scholar] [CrossRef]
- Corradi, M.; Borri, A.; Castori, G.; Sisti, R. Shear strengthening of wall panels through jacketing with cement mortar reinforced by GFRP grids. Compos. Part B Eng. 2014, 64, 33–42. [Google Scholar] [CrossRef] [Green Version]
- Magenes, G.; Penna, A.; Galasco, A.; Rota, M. Experimental characterisation of stone masonry mechanical properties. In Proceedings of the 8th International Masonry Conference, Dresden, Germany, 4–7 July 2010; Volume 1, pp. 247–256. [Google Scholar]
- Silva, B.; Dalla Benetta, M.; da Porto, F.; Modena, C. Experimental assessment of in-plane behavior of three-leaf stone masonry walls. Constr. Build. Mater. 2014, 53, 149–161. [Google Scholar] [CrossRef]
- Binda, L.; Tiraboschi, C.; Abbaneo, S. Experimental research to characterise masonry materials. Mason. Int. 1997, 10, 92–101. [Google Scholar]
- Atkinson, R.; Amadei, B.; Saeb, S.; Sture, S. Response of masonry bed joints in direct shear. J. Struct. Eng. 1989, 115, 2276–2296. [Google Scholar] [CrossRef]
- Lee, H.; Park, Y.; Cho, T.; You, K. Influence of asperity degradation on the mechanical behavior of rough rock joints under cyclic shear loading. Int. J. Rock Mech. Min. Sci. 2001, 38, 967–980. [Google Scholar] [CrossRef]
- Huang, T.; Chang, C.; Chao, C. Experimental and mathematical modeling for fracture of rock joint with regular asperities. Eng. Fract. Mech. 2002, 69, 1977–1996. [Google Scholar] [CrossRef]
- Di Fabio, F.; Gregori, A.; Totani, M. Experimental and numerical investigations on historical masonry wall specimens tested in shear-compression configuration. Eng. Struct. Technol. 2015, 7, 177–188. [Google Scholar] [CrossRef]
- Vasconcelos, G. Experimental Investigations on the Mechanics of Stone Masonry: Characterization of Granites and Behavior of Ancient Masonry Shear Walls. Ph.D. Thesis, Universidade do Minho, Minho, Portugal, 2005. [Google Scholar]
- Beattie, G.; Molyneaux, T.; Gilbert, M.; Burnett, S. Masonry shear strength under impact loading. In Proceedings of the 9th Canadian Masonry Symposium, Fredericton, New Brunswick, AB, Canada, 4–6 June 2001; Volume 1, pp. 1–13. [Google Scholar]
- Jukes, P.; Riddington, J. Review of masonry joint shear strength test methods. Mason. Int. 1997, 11, 37–43. [Google Scholar]
- De Normalisation, C.E. EN 1052–3. Methods of Test for Masonry. Part 3: Determination of Initial Shear Strength. Br. Stand. Inst. 2002, 1, 1–18. [Google Scholar]
- Riddington, J.; Ghazali, M. Hypothesis for shear failure in masonry joints. Proc. Inst. Civ. Eng. 1990, 89, 89–102. [Google Scholar] [CrossRef]
- Mann, W.; Muller, H. Failure of shear-stressed masonry. An enlarged theory, tests and application to shear walls. Proc. Br. Ceram. Soc. 1982, 30, 223. [Google Scholar]
- Calvi, G.M.; Kingsley, G.R.; Magenes, G. Testing of masonry structures for seismic assessment. Earthq. Spectra 1996, 12, 145–162. [Google Scholar] [CrossRef]
- Dalla Benetta, M. Qualificazione di Murature Storiche: Procedure Speriementali in Sito e Calibrazioni in Laboratorio. Ph.D. Thesis, University of Padova, Padova, Italy, 2012. [Google Scholar]
- Binda, L.; Fontana, A.; Mirabella, G. Mechanical behavior and stress distribution in multiple-leaf stone walls. In Proceedings of the 10th International Brick Block Masonry Conference, Calgary, AB, Canada, 5–7 July 1994; Volume 45, pp. 51–60. [Google Scholar]
- Code-NTC18 Norme Tecniche Per Le Costruzioni in Zone Sismiche. Minist. Decree DM 2018, 1, 1–365.
- De Normalisation, C.E. Eurocode 6: Design of Masonry Structures—Part 1-1: General Rules for Reinforced and Unreinforced Masonry Structures; Comité Européen de Normalisation: Brussels, Belgium, 2005. [Google Scholar]
- Augenti, N.; Parisi, F. Teoria e Tecnica Delle Strutture in Muratura; Hoepli: Milan, Italy, 2019. [Google Scholar]
- Goodman, R.E. Introduction to Rock Mechanics; Wiley: New York, NY, USA, 1989; Volume 2. [Google Scholar]
- Lourenço, P.J.B.B.; Louren, P.J.B.B. Computational Strategies for Masonry Structures. Ph.D. Thesis, Technische Universiteit Delft, Delft, The Netherlands, January 1996. [Google Scholar]
- Sorrentino, L.; Cattari, S.; da Porto, F.; Magenes, G.; Penna, A. Seismic behavior of ordinary masonry buildings during the 2016 central Italy earthquakes. Bull. Earthq. Eng. 2019, 17, 5583–5607. [Google Scholar] [CrossRef] [Green Version]
- Roca, P.; Cervera, M.; Gariup, G. Structural analysis of masonry historical constructions. Classical and advanced approaches. Arch. Comput. Methods Eng. 2010, 17, 299–325. [Google Scholar] [CrossRef] [Green Version]
- Giamundo, V.; Sarhosis, V.; Lignola, G.; Sheng, Y.; Manfredi, G. Evaluation of different computational modeling strategies for the analysis of low strength masonry structures. Eng. Struct. 2014, 73, 160–169. [Google Scholar] [CrossRef] [Green Version]
- Asteris, P.G.; Chronopoulos, M.; Chrysostomou, C.; Varum, H.; Plevris, V.; Kyriakides, N.; Silva, V. Seismic vulnerability assessment of historical masonry structural systems. Eng. Struct. 2014, 62, 118–134. [Google Scholar] [CrossRef]
- Lourenço, P.B. Computations on historic masonry structures. Prog. Struct. Eng. Mater. 2002, 4, 301–319. [Google Scholar] [CrossRef]
- Roca, P.; Molins, C.; Marí, A.R. Strength capacity of masonry wall structures by the equivalent frame method. J. Struct. Eng. 2005, 131, 1601–1610. [Google Scholar] [CrossRef]
- Lagomarsino, S.; Penna, A.; Galasco, A.; Cattari, S. TREMURI program: An equivalent frame model for the nonlinear seismic analysis of masonry buildings. Eng. Struct. 2013, 56, 1787–1799. [Google Scholar] [CrossRef]
- Lourenço, P.B.; Rots, J.G.; Blaauwendraad, J. Continuum model for masonry: Parameter estimation and validation. J. Struct. Eng. 1998, 124, 642–652. [Google Scholar] [CrossRef]
- Faria, R.M.C.M.d. Avaliação do Comportamento Sísmico de Barragens de Betão Através de um Modelo de dano Contínuo. Ph.D. Thesis, University of Porto, Porto, Portugal, 1994. [Google Scholar]
- Faria, R.; Oliver, J.; Cervera, M. A strain-based plastic viscous-damage model for massive concrete structures. Int. J. Solids Struct. 1998, 35, 1533–1558. [Google Scholar] [CrossRef]
- Silva, B.; Guedes, J.M.; Arêde, A.; Costa, A. Calibration and application of a continuum damage model on the simulation of stone masonry structures: Gondar church as a case study. Bull. Earthq. Eng. 2012, 10, 211–234. [Google Scholar] [CrossRef]
- Di Nino, S.; Luongo, A. A simple homogenized orthotropic model for in-plane analysis of regular masonry walls. Int. J. Solids Struct. 2019, 167, 156–169. [Google Scholar] [CrossRef]
- Lourenco, P. Computational Strategies for Masonry Structures. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1996. [Google Scholar]
- Costa, A.A.; Arêde, A.; Costa, A.; Guedes, J.; Silva, B. Experimental testing, numerical modeling and seismic strengthening of traditional stone masonry: Comprehensive study of a real Azorian pier. Bull. Earthq. Eng. 2012, 10, 135–159. [Google Scholar] [CrossRef] [Green Version]
- Bolhassani, M.; Hamid, A.A.; Lau, A.C.; Moon, F. Simplified micro modeling of partially grouted masonry assemblages. Constr. Build. Mater. 2015, 83, 159–173. [Google Scholar] [CrossRef]
- Tarque, N.; Benedetti, A.; Camata, G.; Spacone, E. Numerical modeling of the in-plane behavior of rubble stone masonry. In Proceedings of the SAHC2014_9th International Conference on Structural Anlysis of Historical Constructions, Mexico City, Mexico, 14–17 October 2014; Volume 1, pp. 1–10. [Google Scholar]
- Sui, C.; Rafiq, M. Laterally Loaded Masonry Wall Panels: A Review of Numerical Methods. Mason. Int. 2009, 22, 47. [Google Scholar]
- Lotfi, H.R.; Shing, P.B. Interface model applied to fracture of masonry structures. J. Struct. Eng. 1994, 120, 63–80. [Google Scholar] [CrossRef]
- Pelà, L.; Cervera, M.; Roca, P. An orthotropic damage model for the analysis of masonry structures. Constr. Build. Mater. 2013, 41, 957–967. [Google Scholar] [CrossRef]
- Tarque, N.; Camata, G.; Spacone, E.; Varum, H.; Blondet, M. Nonlinear dynamic analysis of a full-scale unreinforced adobe model. Earthq. Spectra 2014, 30, 1643–1661. [Google Scholar] [CrossRef]
- Midas, FEA. Analysis and Algorithm. MIDAS Family Program 2018, 1, 1–463. [Google Scholar]
- De Witte, F. DIANA Finite Element User’s Manual: Material Library; Release 8.1; TNO Building and Construction: The Hague, The Netherlands, 2002; Volume 1. [Google Scholar]
- Vecchio, F.J.; Collins, M.P. The modified compression-field theory for reinforced concrete elements subjected to shear. ACI J. 1986, 83, 219–231. [Google Scholar]
- Selby, R.G. Three-Dimensional Constitutive Relations for Reinforced Concrete. Ph.D. Thesis, Publ. Dept of Civil Eng., Univ. of Toronto, Toronto, ON, Canada, 1993. [Google Scholar]
- Gattulli, V.; Lofrano, E.; Paolone, A.; Pirolli, G. Performances of FRP reinforcements on masonry buildings evaluated by fragility curves. Comput. Struct. 2017, 190, 150–161. [Google Scholar] [CrossRef]
- Rots, J.G. Smeared and discrete representations of localized fracture. In Current Trends in Concrete Fracture Research; Springer: Belin, Germany, 1991; pp. 45–59. [Google Scholar]
- Lourenço, P.B. Recent advances in masonry modeling: Micromodeling and homogenisation. In Multiscale Modeling in Solid Mechanics: Computational Approaches; World Scientific: Singapore, 2010; pp. 251–294. [Google Scholar]
- Gattulli, V.; Lampis, G.; Marcari, G.; Paolone, A. Simulations of FRP reinforcement in masonry panels and application to a historic facade. Eng. Struct. 2014, 75, 604–618. [Google Scholar] [CrossRef]
- Manfredi, G.; Lignola, G.P.; Voto, S. Military quarters in Nola, Italy—Caserma Principe Amedeo: Damage assessment and reconstruction of a partially collapsed XVIII century complex. Int. J. Archit. Herit. 2013, 7, 225–246. [Google Scholar] [CrossRef] [Green Version]
- Da Porto, F.; Guidi, G.; Garbin, E.; Modena, C. In-plane behavior of clay masonry walls: Experimental testing and finite-element modeling. J. Struct. Eng. 2010, 136, 1379–1392. [Google Scholar] [CrossRef]
- Ghiassi, B.; Oliveira, D.V.; Lourenço, P.B.; Marcari, G. Numerical study of the role of mortar joints in the bond behavior of FRP-strengthened masonry. Compos. Part B Eng. 2013, 46, 21–30. [Google Scholar] [CrossRef] [Green Version]
- Clementi, F.; Gazzani, V.; Poiani, M.; Antonio Mezzapelle, P.; Lenci, S. Seismic assessment of a monumental building through nonlinear analyses of a 3D solid model. J. Earthq. Eng. 2018, 22, 35–61. [Google Scholar] [CrossRef]
- Feenstra, P.H. Computational Aspects of Biaxial Stress in Plain and Reinforced Concrete. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1993. [Google Scholar]
- Thorenfeldt, E. Mechanical properties of high-strength concrete and applications in design. In Proceedings of the Symposium Proceedings, Utilization of High-Strength Concrete, Stavanger, Norway, 15–18 June 1987; Volume 22, pp. 35–61. [Google Scholar]
- Hube, M.A.; Mosalam, K.M. Experimental and Computational Evaluation of Current and Innovative In-Span Hinge Details in Reinforced Concrete Box-Girder Bridges Part 1: Experimental Findings and Pre-Test Analysis; Technical Report; California Department of Transportation Division of Research and Innovation: Sacramento, CA, USA, 2009; Volume 1, pp. 35–61.
- Lourenço, P.B.; Barros, J.; Oliveira, J.T. Shear testing of stack bonded masonry. Constr. Build. Mater. 2004, 18, 125–132. [Google Scholar] [CrossRef] [Green Version]
- CEB-FIP. CEB-FIP Model Code 1990: Design Code; Thomas Telford Publishing: London, UK, 1993. [Google Scholar]
- Ganz, H.R.; Thürlimann, B. Tests on Masonry Walls under Normal and Shear Loading; Report No. 7502-4; Institute of Structural Engineering, ETH Zurich: Zurich, Switzerland, 1984; Volume 7502, pp. 1–61. (In German) [Google Scholar]
- Marcari, G.; Manfredi, G.; Prota, A.; Pecce, M. In-plane shear performance of masonry panels strengthened with FRP. Compos. Part B Eng. 2007, 38, 887–901. [Google Scholar] [CrossRef]
- Milosevic, J.; Lopes, M.; Bento, R.; Gago, A. Triplet Test on Rubble Stone Masonry Panels; CIST, IST, Technical University of Lisbon: Lisbon, Portugal, 2012. [Google Scholar]
- Van der Pluijm, R. Shear Behaviour of Bed Joints. In Proceedings of the 6th North American Masonry Conference, Philadelphia, PA, USA, 6–9 June 1993; Hamid, A.A., Harris, H.G., Eds.; Technomic Publ. Co.: Lancaster, UK, 1993; Volume 38, pp. 125–136. [Google Scholar]








| E | G | ||||
|---|---|---|---|---|---|
| (MPa) | (MPa) | (MPa) | (N/mm) | (MPa) | (N/mm) |
| 100 | 45 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Angiolilli, M.; Gregori, A. Triplet Test on Rubble Stone Masonry: Numerical Assessment of the Shear Mechanical Parameters. Buildings 2020, 10, 49. https://doi.org/10.3390/buildings10030049
Angiolilli M, Gregori A. Triplet Test on Rubble Stone Masonry: Numerical Assessment of the Shear Mechanical Parameters. Buildings. 2020; 10(3):49. https://doi.org/10.3390/buildings10030049
Chicago/Turabian StyleAngiolilli, Michele, and Amedeo Gregori. 2020. "Triplet Test on Rubble Stone Masonry: Numerical Assessment of the Shear Mechanical Parameters" Buildings 10, no. 3: 49. https://doi.org/10.3390/buildings10030049
APA StyleAngiolilli, M., & Gregori, A. (2020). Triplet Test on Rubble Stone Masonry: Numerical Assessment of the Shear Mechanical Parameters. Buildings, 10(3), 49. https://doi.org/10.3390/buildings10030049





