Research on the Dynamic Response of a Slope Reinforced by a Pile-Anchor Structure under Seismic Loading
Abstract
:1. Introduction
2. Numerical Model
2.1. Methodology
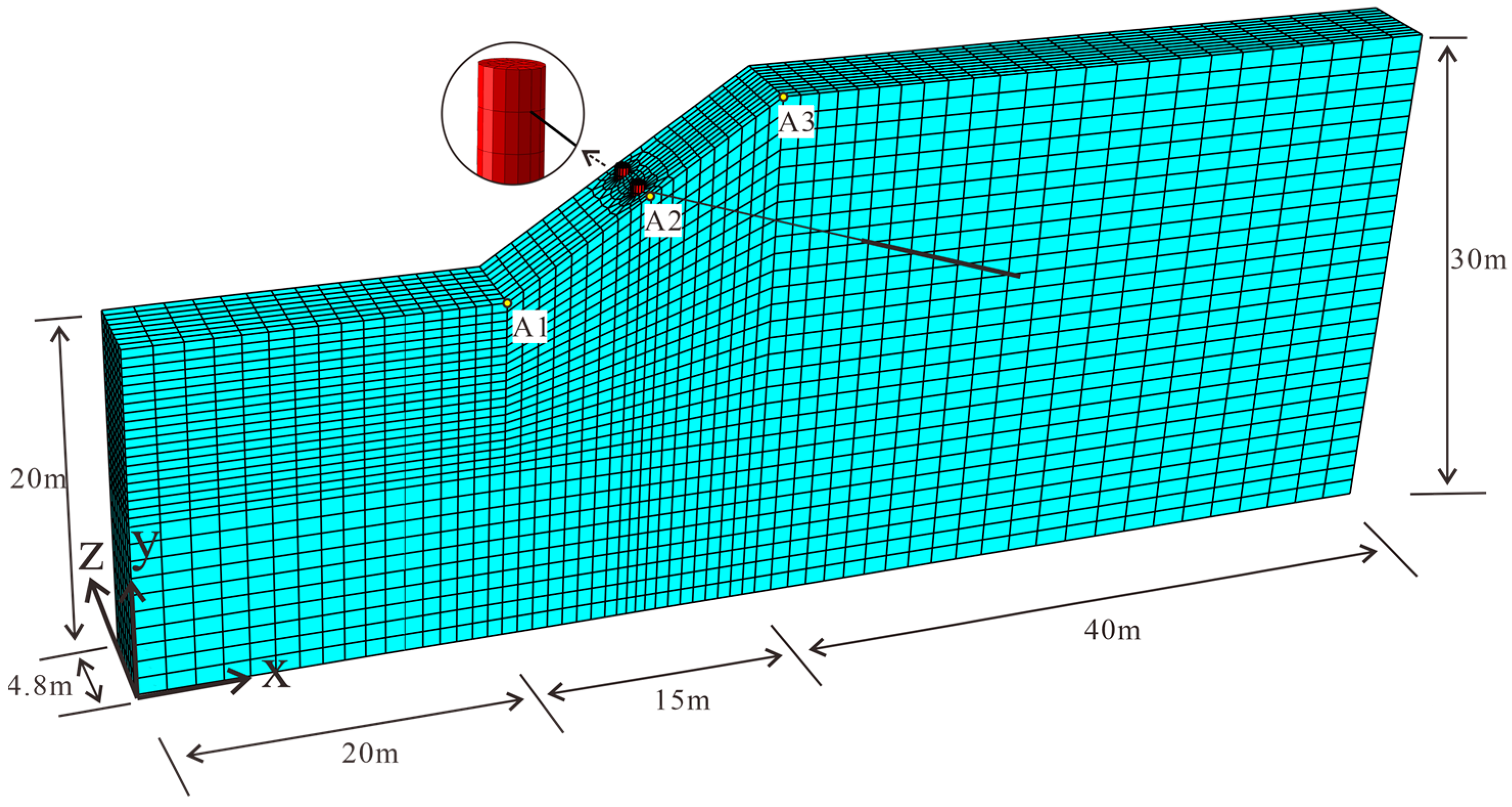
2.2. Model Description
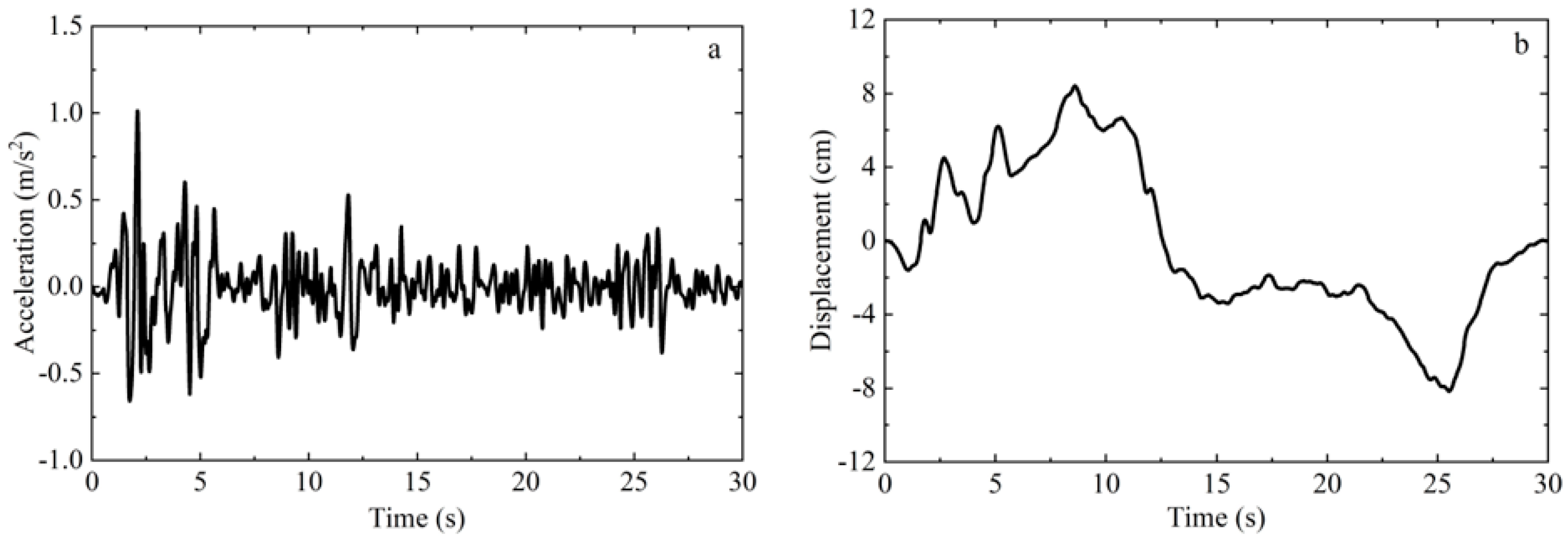
2.3. Seismic Input
2.4. Material Damping
2.5. Boundary Conditions
- (1)
- The bottom of the model is fixed, while the lateral boundaries are subjected to normal constraints. This allows for stress equilibrium within the model. Then, the entire model is subjected to gravity to simulate the self-weight load. As a result, nodal reactions at the left and right lateral boundaries can be obtained, providing valuable insights into the distribution of forces within the slope under gravity.
- (2)
- To achieve the conversion from static to dynamic boundary conditions, the horizontal constraints of the left and right boundaries of the model are released; meanwhile, the displacement in the vertical direction is restricted. Additionally, the reaction forces obtained from Step 1 are applied to the corresponding nodes at the left and right boundaries to maintain balance. This approach allows for a smooth transition from the static calculation to the dynamic analysis, ensuring consistency in the boundary conditions and enabling an accurate assessment of the dynamic response of the reinforced slope under seismic loading.
- (3)
- In the dynamic analysis, the horizontal constraint on the bottom of the model is released. Simultaneously, ground motion is applied in the horizontal direction. This setup allows for the investigation of the dynamic response characteristics of the reinforced slope under seismic loading.
2.6. Validation
- (1)
- Stability of the slope without reinforcement under gravity
- (2)
- Dynamic response of the slope without reinforcement under seismic loading
3. Dynamic Response of the Slope Model Reinforced by Different Structures
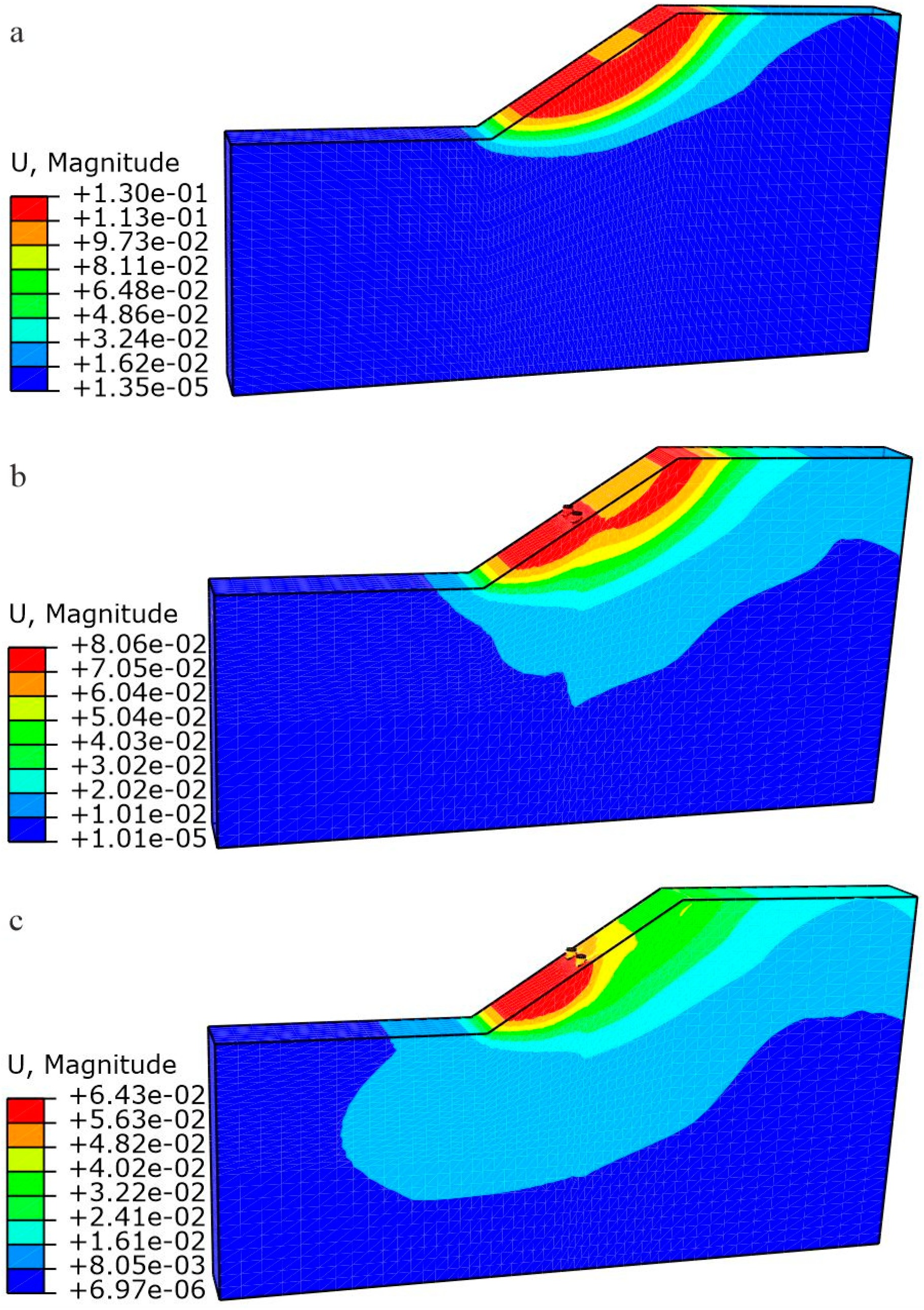
3.1. Dynamic Response of the Soil Slope
3.2. Dynamic Response of the Piles
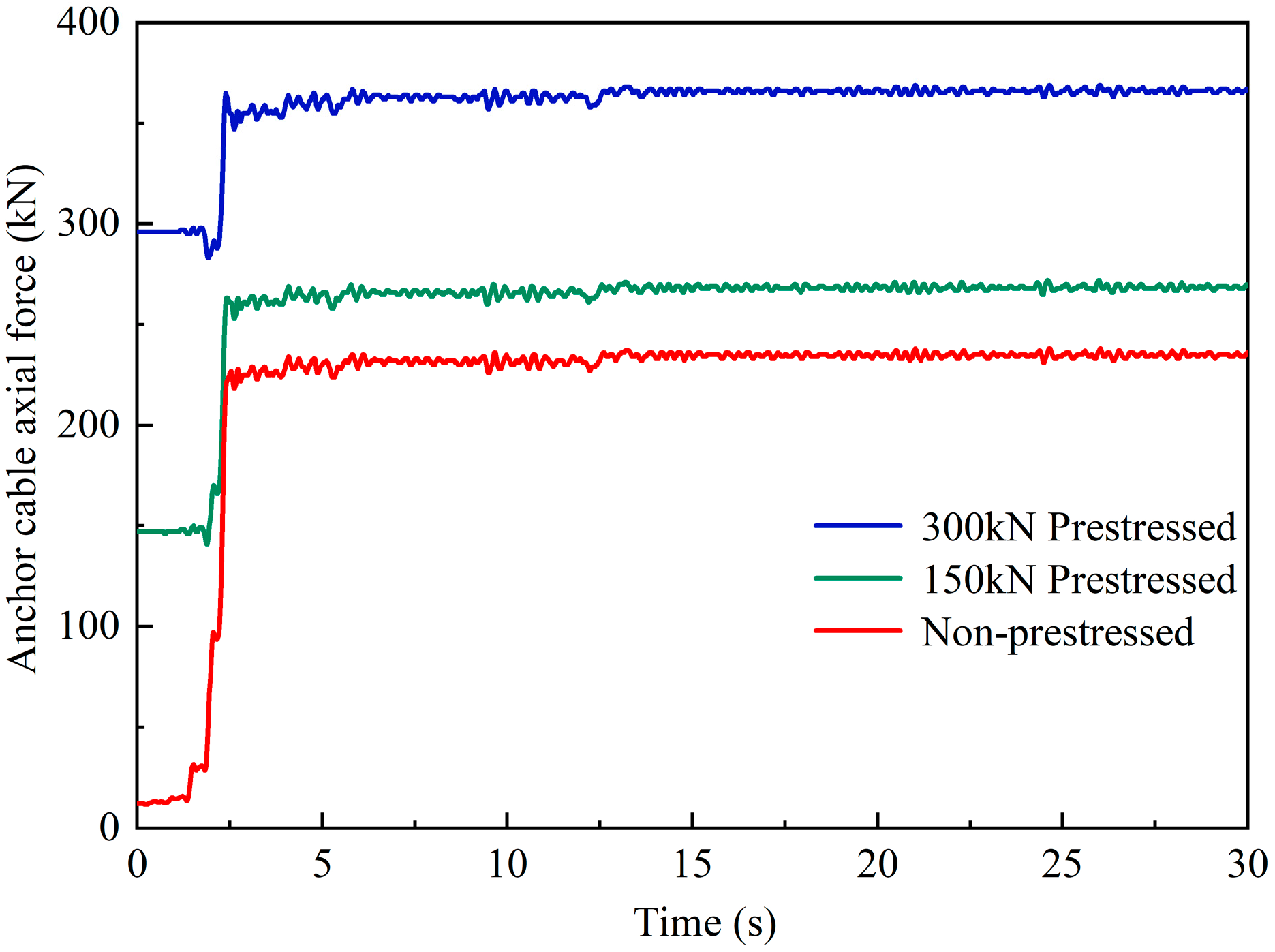
3.3. Dynamic Response of the Anchor
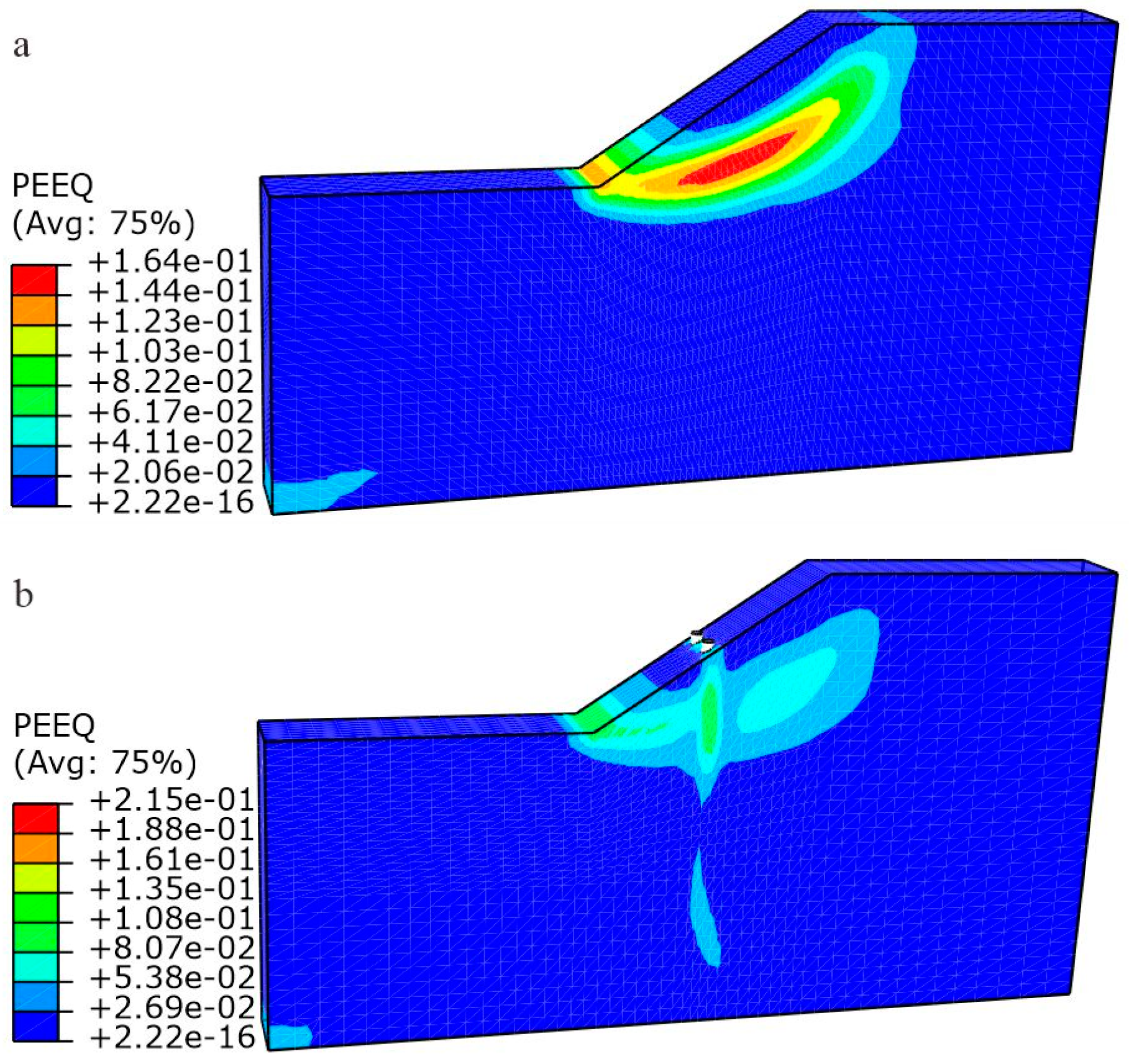
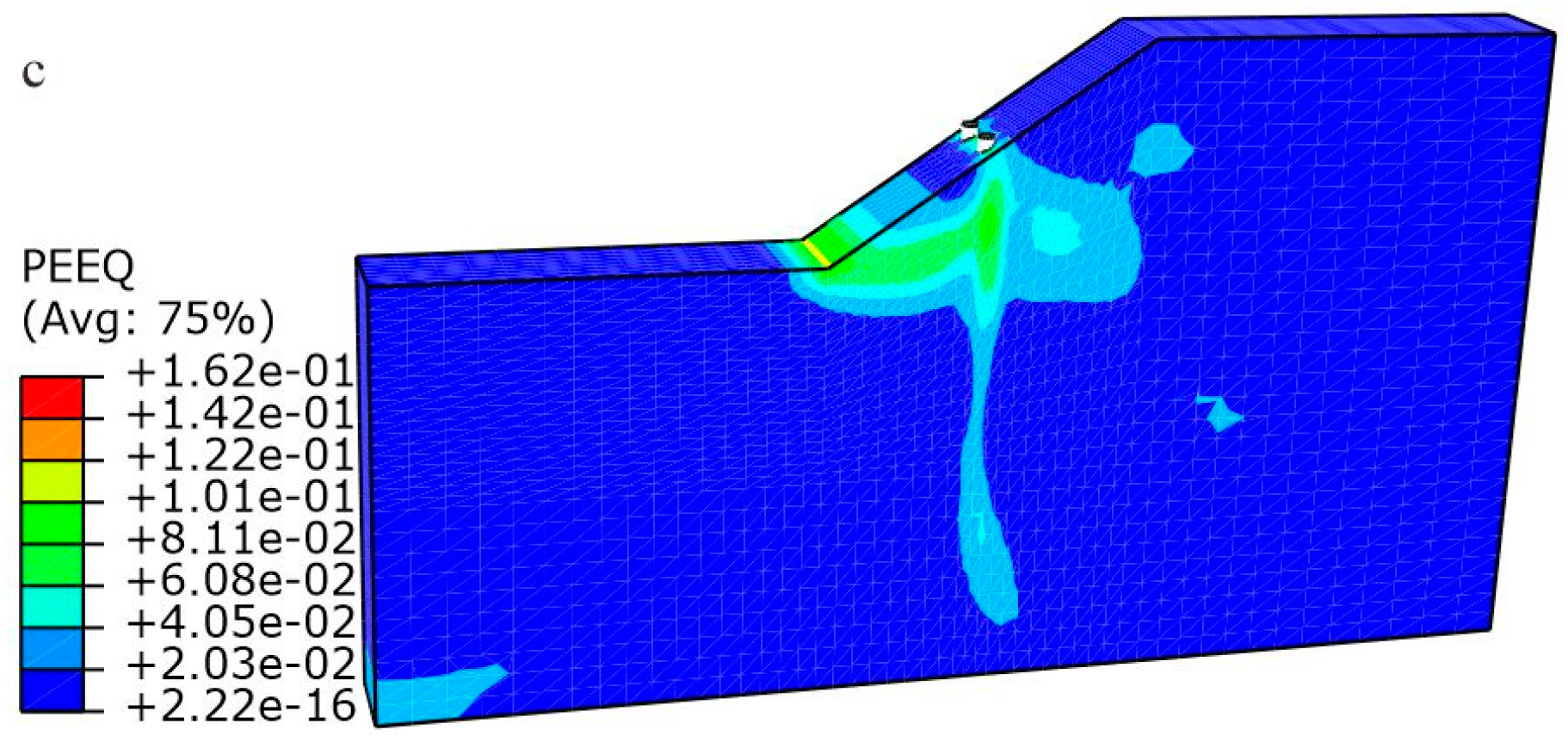
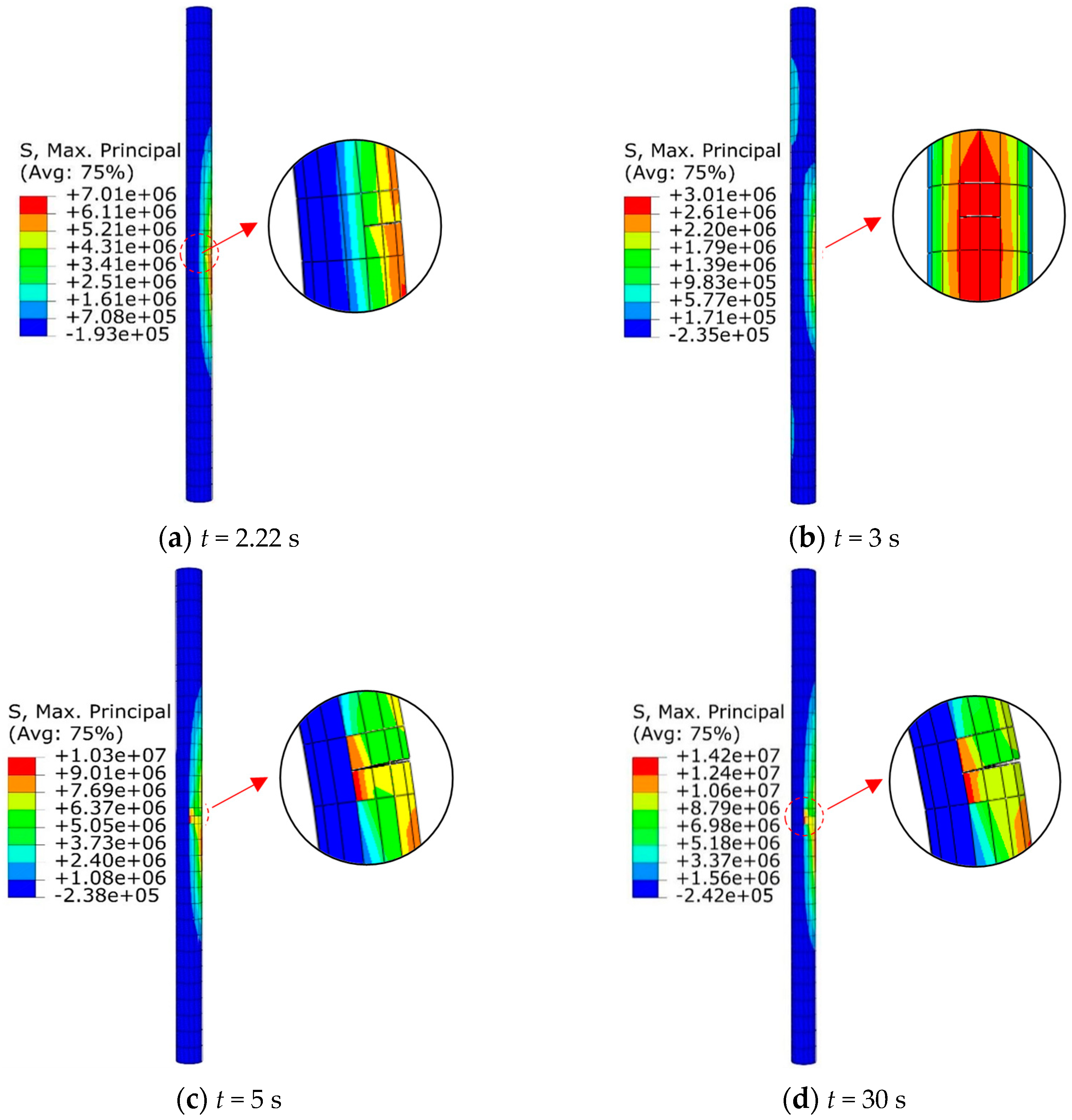
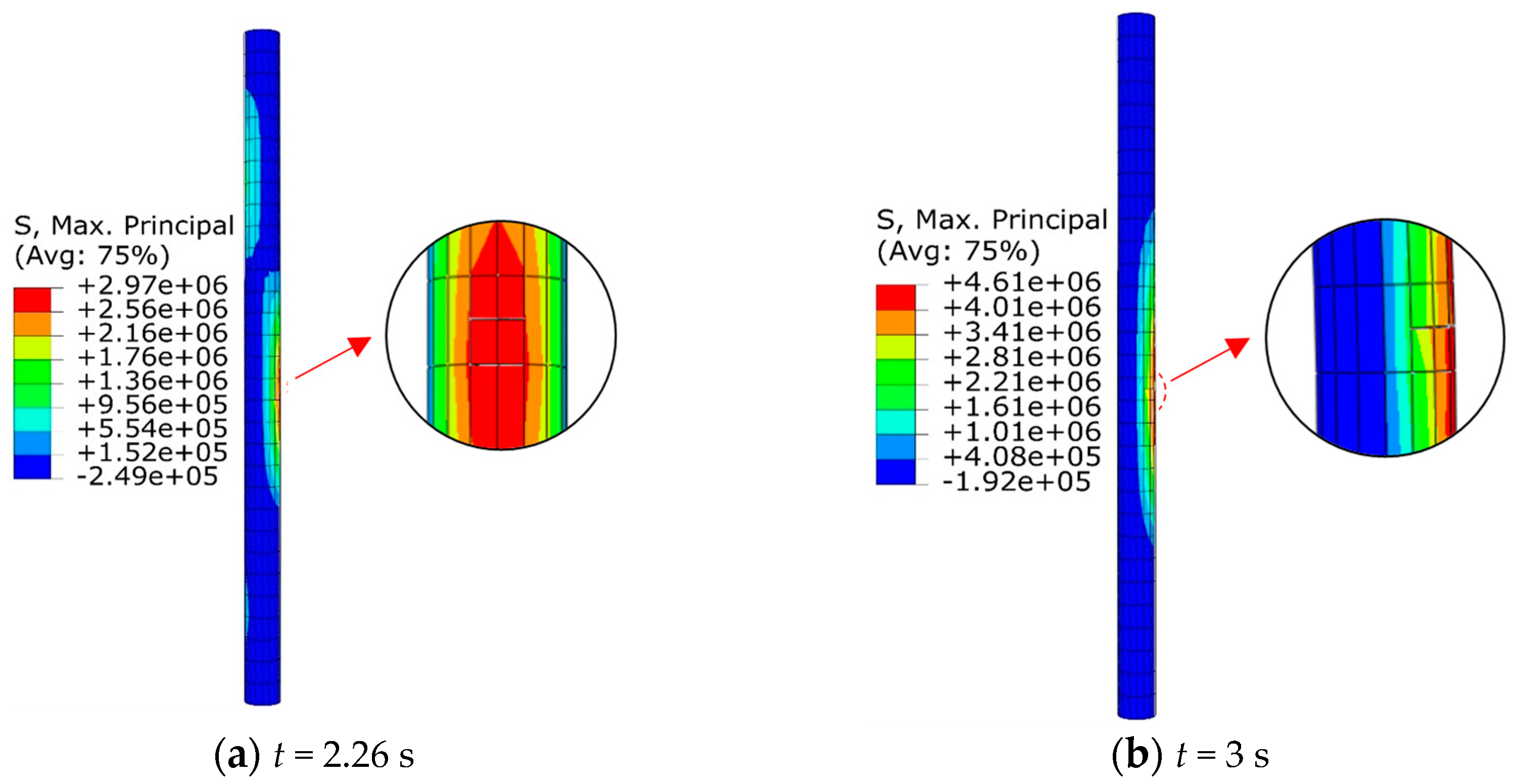
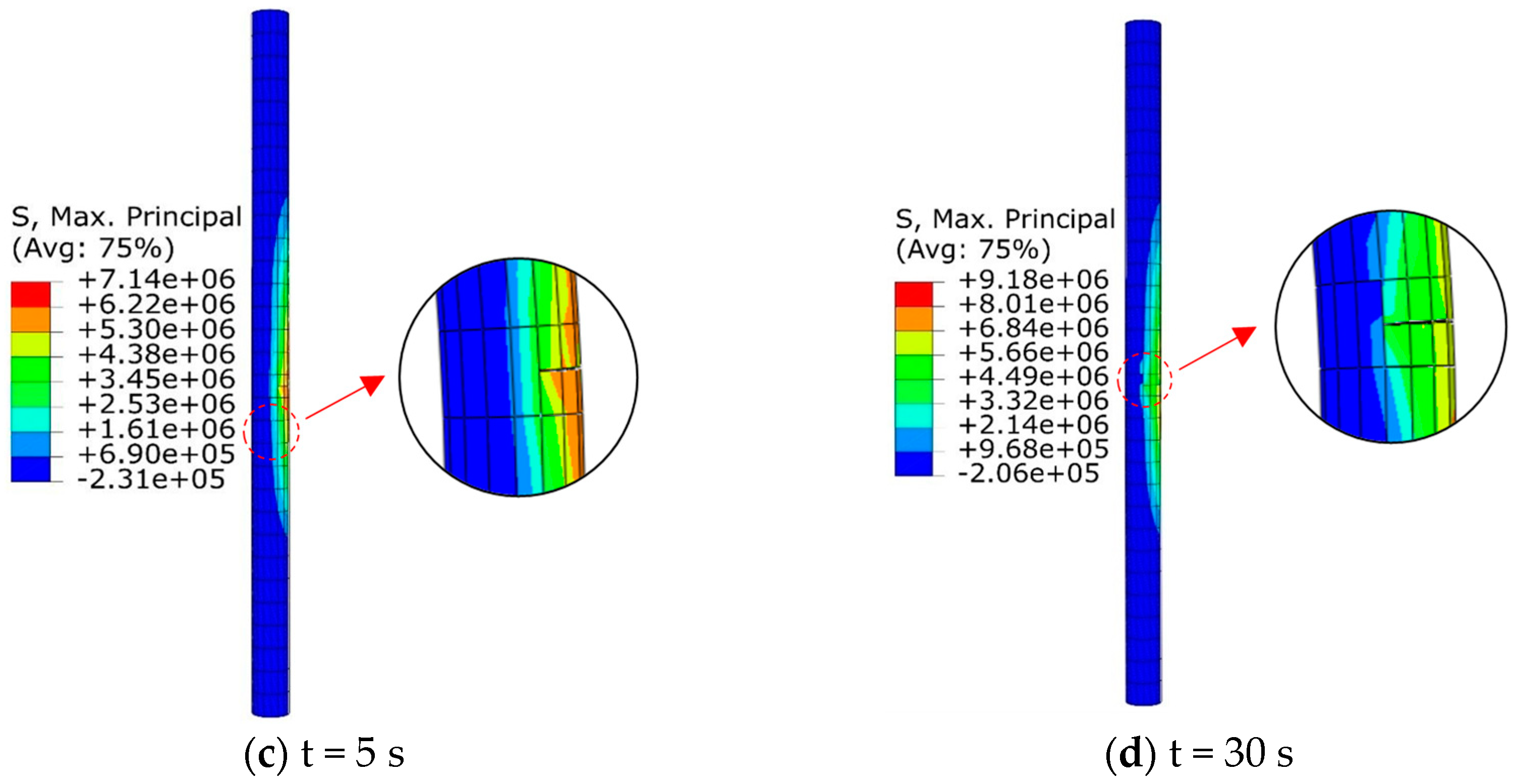
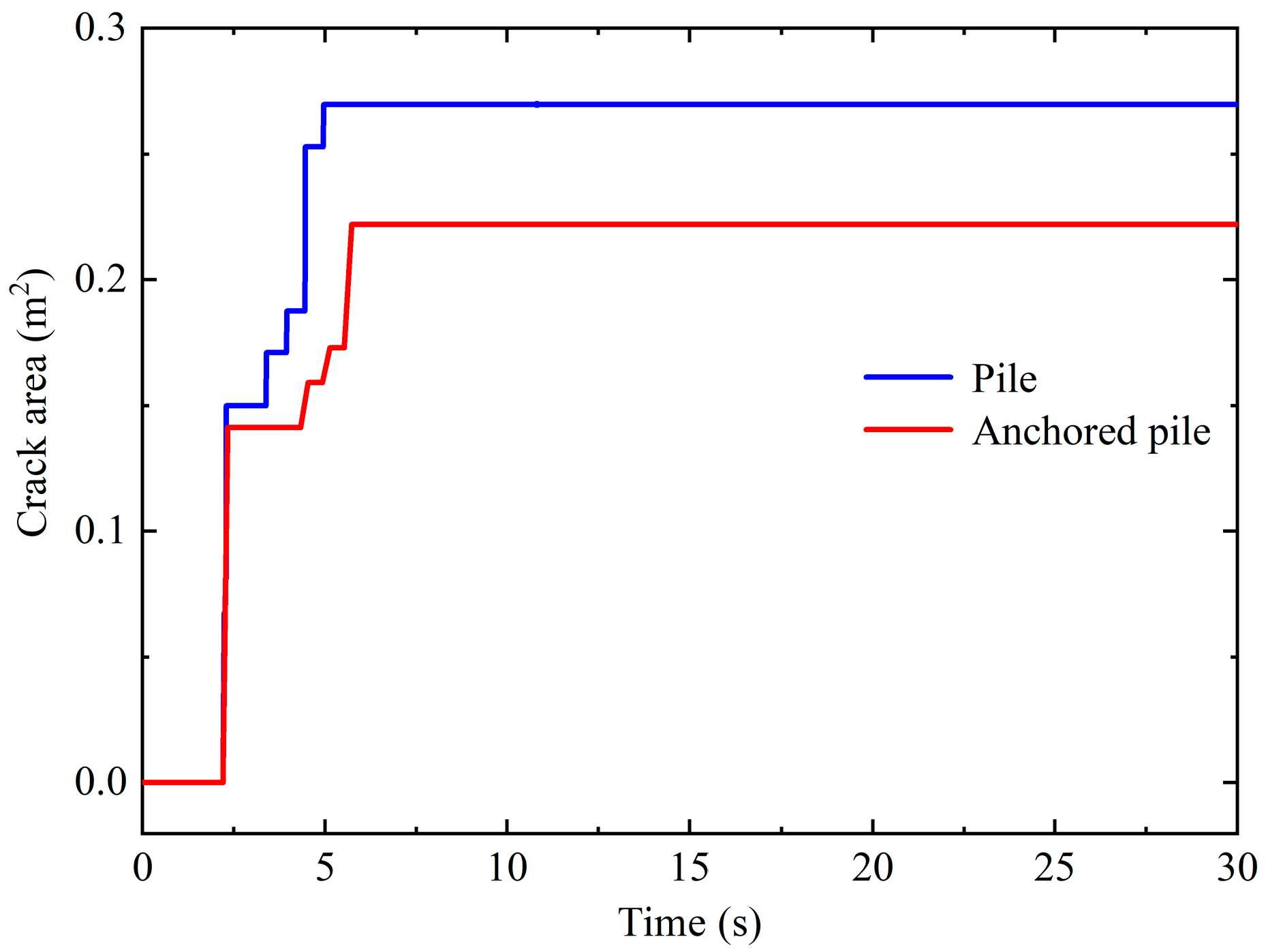
4. Progressive Failure Process of the Piles Subjected to Seismic Loading
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, Y.; Xu, X.; Mao, W. Numerical performance assessment of slope reinforcement using a pile-anchor structure under seismic loading. Soil Dyn. Earthq. Eng. 2020, 129, 105963. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, F.; Zhang, X. Application of small-diameter steel tube soldier piles in slope emergency works. Drill. Eng. 2022, 49, 152–157. [Google Scholar]
- Sun, J.; Fan, M.; Li, X. Combined supporting system of the slope protection pile and the compound soil nail wall with micro steel pipe piles. Drill. Eng. 2021, 48, 116–124. [Google Scholar]
- Xu, C. Landslide seismology geology: A sub-discipline of environmental earth sciences. Eng. Geol. 2018, 26, 207–222. [Google Scholar]
- Zhou, D.P.; Zhang, J.J.; Tang, Y. Seismic damage analysis of Roda slopes in Wenchuan earthquake. Chin. J. Rock Mech. Eng. 2010, 29, 565–576. [Google Scholar]
- Xu, X.; Huang, Y. Parametric study of structural parameters affecting seismic stability in slopes reinforced by pile-anchor structures. Soil Dyn. Earthq. Eng. 2021, 147, 106789. [Google Scholar] [CrossRef]
- Huang, Y.; Xu, X.; Liu, J.; Mao, W. Centrifuge modeling of seismic response and failure mode of a slope reinforced by a pile-anchor structure. Soil Dyn. Earthq. Eng. 2020, 131, 106037. [Google Scholar] [CrossRef]
- Hu, H.Q.; Huang, Y.; Xiong, M.; Zhao, L.Y. Investigation of seismic behavior of slope reinforced by anchored pile structures using shaking table tests. Soil Dyn. Earthq. Eng. 2021, 150, 106900. [Google Scholar] [CrossRef]
- Xu, M.; Tang, Y.; Liu, X.; Yang, H.; Luo, B. A shaking table model test on a rock slope anchored with adaptive anchor cables. Int. J. Rock Mech. Min. Sci. 2018, 112, 201–208. [Google Scholar] [CrossRef]
- Qu, H.L.; Luo, H.; Hu, H.G.; Jia, H.Y.; Zhang, D.Y. Dynamic response of anchored sheet pile wall under ground motion: Analytical model with experimental validation. Soil Dyn. Earthq. Eng. 2018, 115, 896–906. [Google Scholar] [CrossRef]
- Jahromi, H.F.; Jafarzadeh, F.; Zakaria, M.S. Experimental study of burial depth effect on embedded pipe deformations in sandy slopes under dynamic landsliding. Soil Dyn. Earthq. Eng. 2018, 114, 281–297. [Google Scholar] [CrossRef]
- Cheng, X.L.; Li, Y.F.; Wang, P.G.; Liu, Z.X.; Zhou, Y.D. Model tests and finite element analysis for vertically loaded anchors subjected to cyclic loads in soft clays. Comput. Geotech. 2020, 119, 103317. [Google Scholar] [CrossRef]
- Srilatha, N.; Latha, G.M.; Puttappa, C.G. Effect of frequency on seismic response of reinforced soil slopes in shaking table tests. Geotext. Geomembr. 2013, 36, 27–32. [Google Scholar] [CrossRef]
- Panah, A.K.; Eftekhari, Z. Shaking table tests on polymeric-strip reinforced-soil walls adjacent to a rock slope. Geotext. Geomembr. 2021, 49, 737–756. [Google Scholar] [CrossRef]
- Yang, Z.; Wang, J.; Xu, P. Stability analysis of deep fill slope under the influence of rainfall-surcharge coupling. Drill. Eng. 2023, 50, 94–102. [Google Scholar]
- Lin, Y.L.; Shi, F.; Yang, X.; Yang, G.L.; Li, L.M. Numerical analysis on seismic behavior of railway earth embankment: A case study. J. Cent. S. Univ. 2016, 23, 906–918. [Google Scholar] [CrossRef]
- Li, D.F.; Zhang, Z.J. The anti sliding mechanism of adjacent pile-anchor structure considering traffic load on slope top. Adv. Civ. Eng. 2021, 2021, 6615224. [Google Scholar] [CrossRef]
- Kiani, K. Nanomechanical sensors based on elastically supported double-walled carbon nanotubes. Appl. Math. Comput. 2015, 270, 216–241. [Google Scholar] [CrossRef]
- Peng, L.X.; Liew, K.M.; Kitipornchai, S. Buckling and free vibration analyses of stiffened plates using the FSDT mesh-free method. J. Sound Vib. 2006, 289, 421–449. [Google Scholar] [CrossRef]
- Zhang, L.W.; Zhang, Y.; Liew, K.M. Modeling of nonlinear vibration of graphene sheets using a meshfree method based on nonlocal elasticity theory. Appl. Math. Model. 2017, 49, 691–704. [Google Scholar] [CrossRef]
- Kiani, K. Column buckling of magnetically affected stocky nanowires carrying electric current. J. Phys. Chem. Solids. 2015, 83, 140–151. [Google Scholar] [CrossRef]
- Wang, L.; He, X.; Sun, Y.; Liew, K.M. A mesh-free vibration analysis of strain gradient nano-beams. Eng. Anal. Bound Elem. 2017, 84, 231–236. [Google Scholar] [CrossRef]
- Wu, Z.; Luo, G.; Yang, Z.; Guo, Y.; Li, K.; Xue, Y. A comprehensive review on deep learning approaches in wind forecasting applications. CAAI Trans. Intell. Technol. 2022, 7, 129–143. [Google Scholar] [CrossRef]
- Bong, T.; Kim, S.R.; Kim, B.I. Prediction of ultimate bearing capacity of aggregate pier reinforced clay using multiple regression analysis and deep learning. Appl. Sci. 2020, 10, 4580. [Google Scholar] [CrossRef]
- Gao, S.; Li, S. Bloody Mahjong playing strategy based on the integration of deep learning and XGBoost. CAAI Trans. Intell. Technol. 2021, 7, 95–106. [Google Scholar] [CrossRef]
- Cakiroglu, C.; Islam, K.; Bekdaş, G.; Nehdi, M.L. Data-driven ensemble learning approach for optimal design of cantilever soldier pile retaining walls. Structures 2023, 51, 1268–1280. [Google Scholar] [CrossRef]
- Gasparin, A.; Lukovic, S.; Alippi, C. Deep learning for time series forecasting: The electric load case. CAAI Trans. Intell. Technol. 2021, 7, 1–25. [Google Scholar] [CrossRef]
- Khan, J.; Lee, E.; Kim, K. A higher prediction accuracy–based alpha–beta filter algorithm using the feedforward artificial neural network. CAAI Trans. Intell. Technol. 2022, 1–16. [Google Scholar] [CrossRef]
- Hsiao, I.H.; Chung, C.Y. AI-infused semantic model to enrich and expand programming question generation. J. Artif. Intell. Res. 2022, 2, 47–54. [Google Scholar] [CrossRef]
- Benali, A.; Hachama, M.; Bounif, A.; Nechnech, A.; Karray, M. A TLBO-optimizedartificial neural network for modeling axial capacity of pile foundations. Eng. Comput. 2021, 37, 675–684. [Google Scholar] [CrossRef]
- Deng, Y.; Zeng, Z.; Jha, K.; Huang, D. Problem-Based Cybersecurity Lab with Knowledge Graph as Guidance. J. Artif. Intell. Res. 2022, 2, 55–61. [Google Scholar] [CrossRef]
- Jia, Z.; Wang, W.; Zhang, J.; Li, H. Contact High-Temperature Strain Automatic Calibration and Precision Compensation Research. J. Artif. Intell. Res. 2022, 2, 69–76. [Google Scholar]
- Cai, F.; Ugai, K. Numerical Analysis of the Stability of a Slope Reinforced with Piles. Soils Found. 2000, 1, 73–84. [Google Scholar] [CrossRef]
- Won, J.; You, K.; Jeong, S.; Kim, S. Coupled effects in stability analysis of pile–slope systems. Comput. Geotech. 2005, 32, 304–315. [Google Scholar] [CrossRef]
- Lin, Y.L.; Cheng, X.M.; Yang, G.L.; Li, Y. Seismic response of a sheet-pile wall with anchoring frame beam by numerical simulation and shaking table test. Soil Dyn. Earthq. Eng. 2018, 115, 352–364. [Google Scholar] [CrossRef]
- Joorabchi, A.E.; Liang, R.Y.; Li, L.; Liu, H.L. Yield acceleration and permanent displacement of a slope reinforced with a row of drilled shafts. Soil Dyn. Earthq. Eng. 2014, 57, 68–77. [Google Scholar] [CrossRef]
- Ye, S.; Fang, G.; Zhu, Y. Model establishment and response analysis of slope reinforced by frame with prestressed anchors under seismic considering the prestress. Soil Dyn. Earthq. Eng. 2019, 122, 228–234. [Google Scholar] [CrossRef]
- Yan, M.J.; Xia, Y.Y.; Liu, T.T.; Bowa, V.M. Limit analysis under seismic conditions of a slope reinforced with prestressed anchor cables. Comput. Geotech. 2019, 108, 226–233. [Google Scholar] [CrossRef]
- Chakraborty, R.; Dey, A. Probabilistic assessment of seismic response of toe-excavated hillslopes retained using anchored sheet-pile-wall. Ain Shams Eng. J. 2022, 13, 101736. [Google Scholar] [CrossRef]
- He, Z.; Qin, Y.; Zhang, Y.; Xu, Y.; Li, X. Engineering geological characteristics of completely weathered argillaceous limestone under the influence of water content and analysis of pile hole stability. Drill. Eng. 2023, 50, 84–93. [Google Scholar]
- Li, C.D.; Wang, X.Y.; Tang, H.M.; Lei, G.P.; Yan, J.F.; Zhang, Y.Q. A preliminary study on the location of the stabilizing piles for colluvial landslides with interbedding hard and soft bedrocks. Eng. Geol. 2017, 224, 15–28. [Google Scholar] [CrossRef]
- Xu, X.; Xing, Y.C.; Guo, Z.; Yu, H. Stability analysis of rainfall-triggered toe-cut slopes and effectiveness evaluation of pile-anchor structures. J. Earth Sci. 2021, 32, 1104–1112. [Google Scholar] [CrossRef]
- Mitani, Y.; Wang, F.; Okeke, A.C.; Qi, W.H. Dynamic analysis of earthquake amplification effect of slopes in different topographic and geological conditions by using ABAQUS. Prog. Geo Disaster Mitig. Technol. Asia 2012, 469–490. [Google Scholar]
- Shooshpasha, I.; Amirdehi, H.A. Evaluating the stability of slope reinforced with one row of free head piles. Arab. J. Geosci. 2015, 8, 2131–2141. [Google Scholar] [CrossRef]
- Wei, W.B.; Cheng, Y.M. Strength reduction analysis for slope reinforced with one row of piles. Comput. Geotech. 2009, 36, 1176–1185. [Google Scholar] [CrossRef]
- Haghani, M.; Neya, B.N.; Ahmadi, M.T.; Vaseghi, A.J. A new numerical approach in the seismic failure analysis of concrete gravity dams using extended finite element method. Eng. Fail. Anal. 2022, 132, 105835. [Google Scholar] [CrossRef]
- Huang, H.; Zeng, Z.C.; Zhang, F.; Zeng, Z.C. Mechanical behavior and influencing factors of different pile-anchor supporting structures. J. Highw. Transp. Res. Dev. 2015, 32, 58–66. [Google Scholar] [CrossRef]
- Liu, X.Y.; Cai, G.J.; Liu, L.L.; Zhou, Z.J. Investigation of internal force of anti-slide pile on landslides considering the actual distribution of soil resistance acting on anti-slide piles. Nat. Hazards 2020, 102, 1369–1392. [Google Scholar] [CrossRef]
- Ye, S.H.; Huang, A.P.; Fang, G.W. Dynamics of loess multi-level high fill slope under horizontal earthquake response law and stability analysis. Technol. Earthq. Disaster Prev. 2020, 1, 1–10. [Google Scholar]
- Lin, Y.L.; Li, Y.X.; Yang, G.L.; Li, Y. Experimental and numerical study on the seismic behavior of anchoring frame beam supporting soil slope on rock mass. Soil Dyn. Earthq. Eng. 2017, 98, 12–23. [Google Scholar] [CrossRef]
- Lin, Y.L.; Li, Y.X.; Zhao, L.H.; Yang, T.Y. Investigation on seismic response of a three-stage soil slope supported by anchor frame structure. J. Cent. South Univ. 2020, 27, 1290–1305. [Google Scholar] [CrossRef]
- Xie, J.; Duan, L.; Li, Y.T.; Yan, J.; Peng, J.J. Shock absorption analysis based on the tunnel-soil-surface building interaction system. J. Asian Archit. Build. 2022, 21, 1545–1560. [Google Scholar] [CrossRef]
- Zhuang, h.y.; Hu, z.h.; Wang, x.j.; Chen, G.X. Seismic responses of a large underground structure in liquefied soils by FEM numerical modelling. Bull. Earthq. Eng. 2015, 13, 3645–3668. [Google Scholar] [CrossRef]
- Machaček, J.; Triantafyllidis, T.H.; Staubach, P. Fully coupled simulation of an opencast mine subjected to earthquake loading. Soil Dyn. Earthq. Eng. 2018, 115, 853–867. [Google Scholar] [CrossRef]
- Li, Y.H.; Guo, Z.; Jing, H.B.; Deng, Q.C. Failure effect of seismic faults and the slope stability along highways under seismic hazards based on dynamic finite element analysis and genetic algorithm. Geotech. Geol. Eng. 2021, 39, 5191–5200. [Google Scholar]
- Xu, X.; Huang, Y.; Yashima, A.; Du, X. Failure evolution process of pile-anchor reinforced rock slope based on centrifuge shaking table tests. Eng. Geol. 2022, 311, 106920. [Google Scholar] [CrossRef]
- Li, X.J.; Wang, X.M.; Zhang, J.W. Method for judging seismic stability state of soil slopes. Chin. J. Geotech. 2018, 40, 2096–2102. [Google Scholar]
- Fries, T.P.; Baydoun, M. Crack propagation with the extended finite element method and a hybrid explicit-implicit crack description. Int. J. Numer. Methods Eng. 2012, 89, 1527–1558. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Meth. Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Wang, X.L.; Liu, C.; Wang, H.; Liu, H.; Wu, H.A. Comparison of consecutive and alternate hydraulic fracturing in horizontal wells using XFEM-based cohesive zone method. J. Pet. Sci. Eng. 2016, 143, 14–25. [Google Scholar] [CrossRef]
- Guo, J.C.; Luo, B.; Lu, C.; Lai, J.; Ren, J.C. Numerical investigation of hydraulic fracture propagation in a layered reservoir using the cohesive zone method. Eng. Fract. Mech. 2017, 186, 195–207. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Chu, Z.; Zhang, L.; He, Y. Research on the Dynamic Response of a Slope Reinforced by a Pile-Anchor Structure under Seismic Loading. Buildings 2023, 13, 2500. https://doi.org/10.3390/buildings13102500
Li Y, Chu Z, Zhang L, He Y. Research on the Dynamic Response of a Slope Reinforced by a Pile-Anchor Structure under Seismic Loading. Buildings. 2023; 13(10):2500. https://doi.org/10.3390/buildings13102500
Chicago/Turabian StyleLi, Yanyan, Zhuqiang Chu, Le Zhang, and Yujie He. 2023. "Research on the Dynamic Response of a Slope Reinforced by a Pile-Anchor Structure under Seismic Loading" Buildings 13, no. 10: 2500. https://doi.org/10.3390/buildings13102500
APA StyleLi, Y., Chu, Z., Zhang, L., & He, Y. (2023). Research on the Dynamic Response of a Slope Reinforced by a Pile-Anchor Structure under Seismic Loading. Buildings, 13(10), 2500. https://doi.org/10.3390/buildings13102500






