Calculation Theory of Shear Stress Distribution in Box Girder with Corrugated Steel Webs Based on the Energy Method
Abstract
:1. Introduction
2. The Calculation Theory of Shear Force Distribution of Variable Cross-Section Corrugated Steel Webs Based on the Energy Method
2.1. Deflection Curve and Deflection Surface
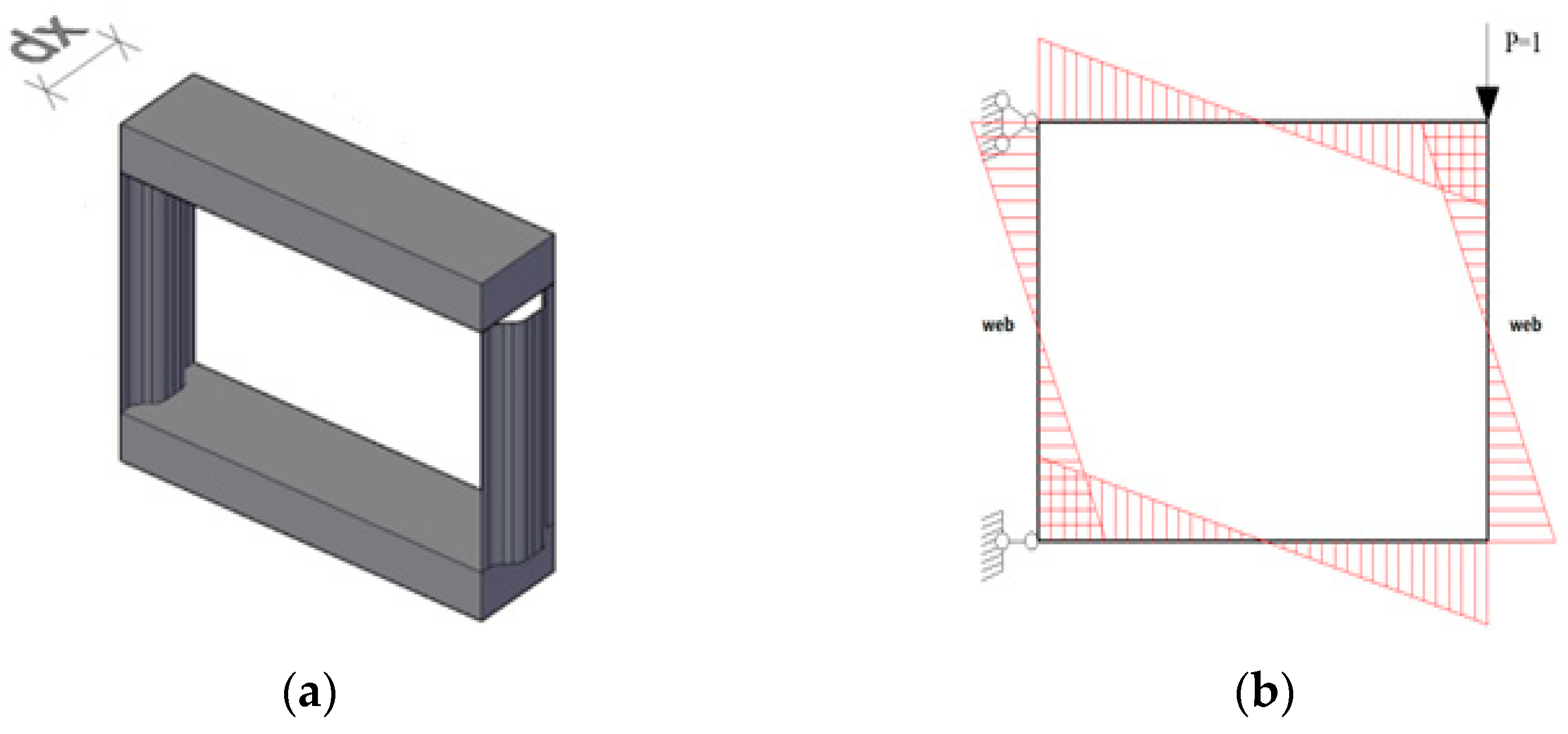
2.2. Longitudinal Bending Strain Energy
2.3. Bending Strain Energy in the Transverse Direction
2.4. Calculation of Work of External Force
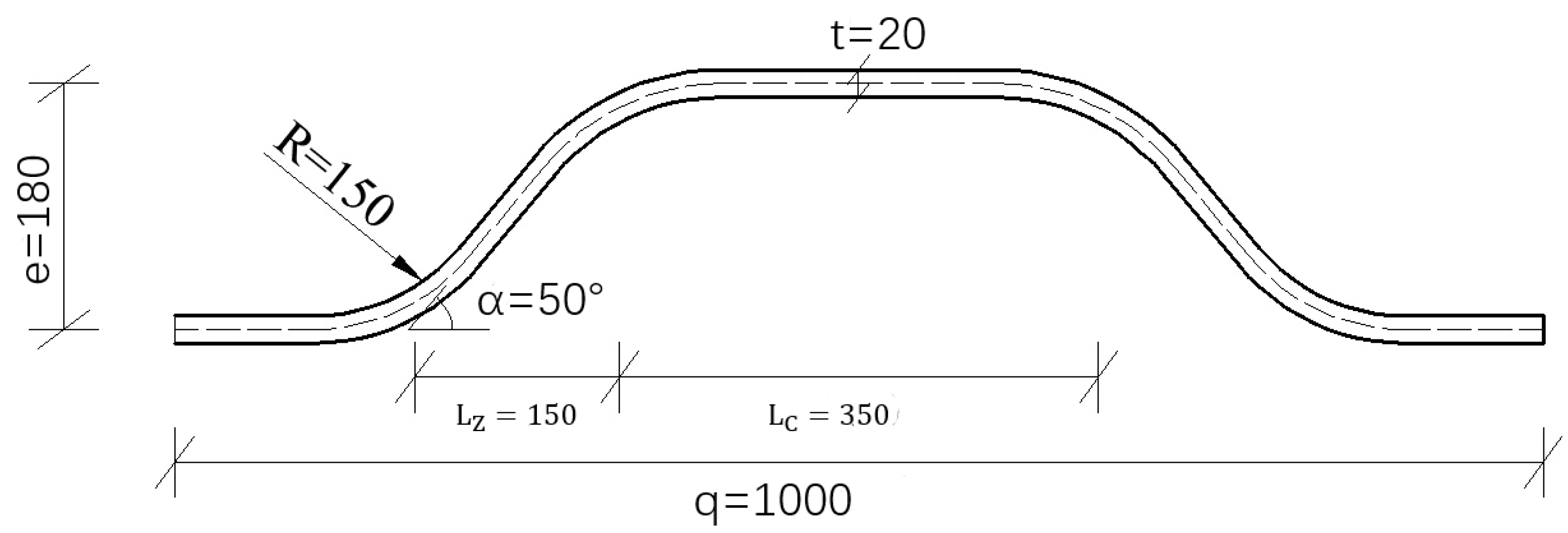
2.5. Parameter Calculation
2.6. Shear Distribution Coefficient
2.7. Shear Stress of Each Web
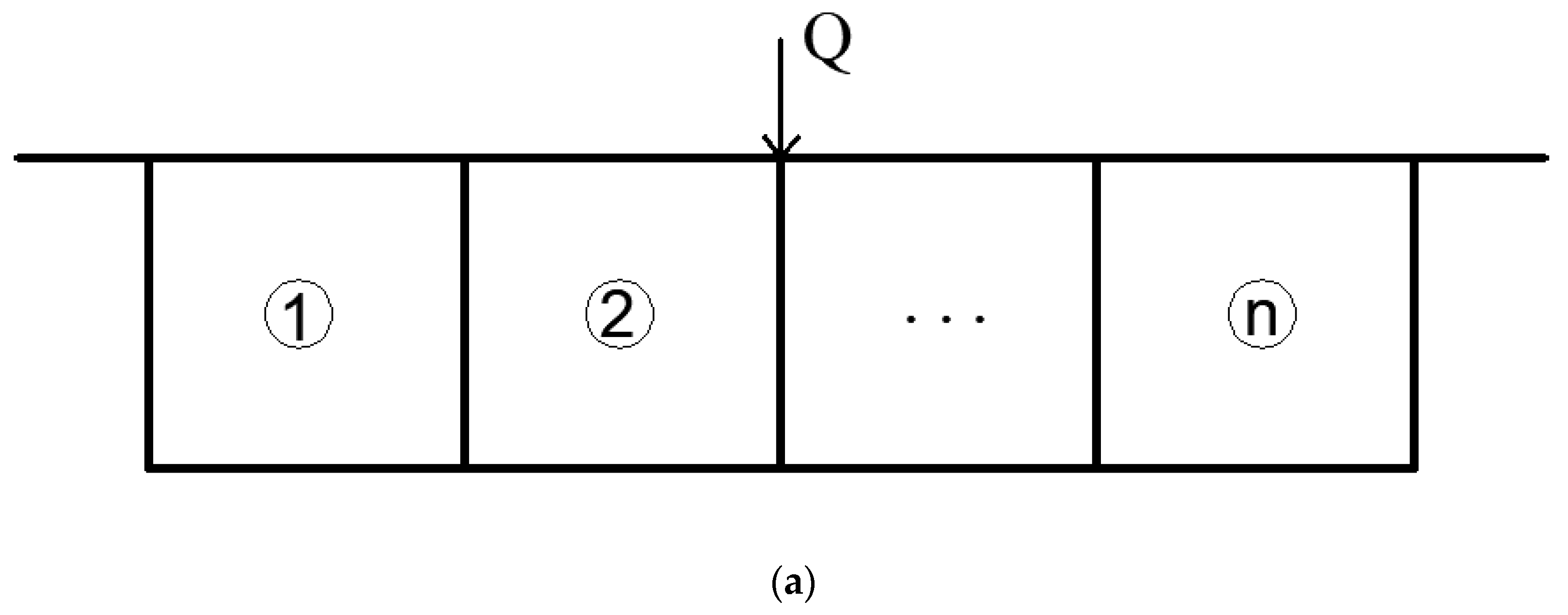
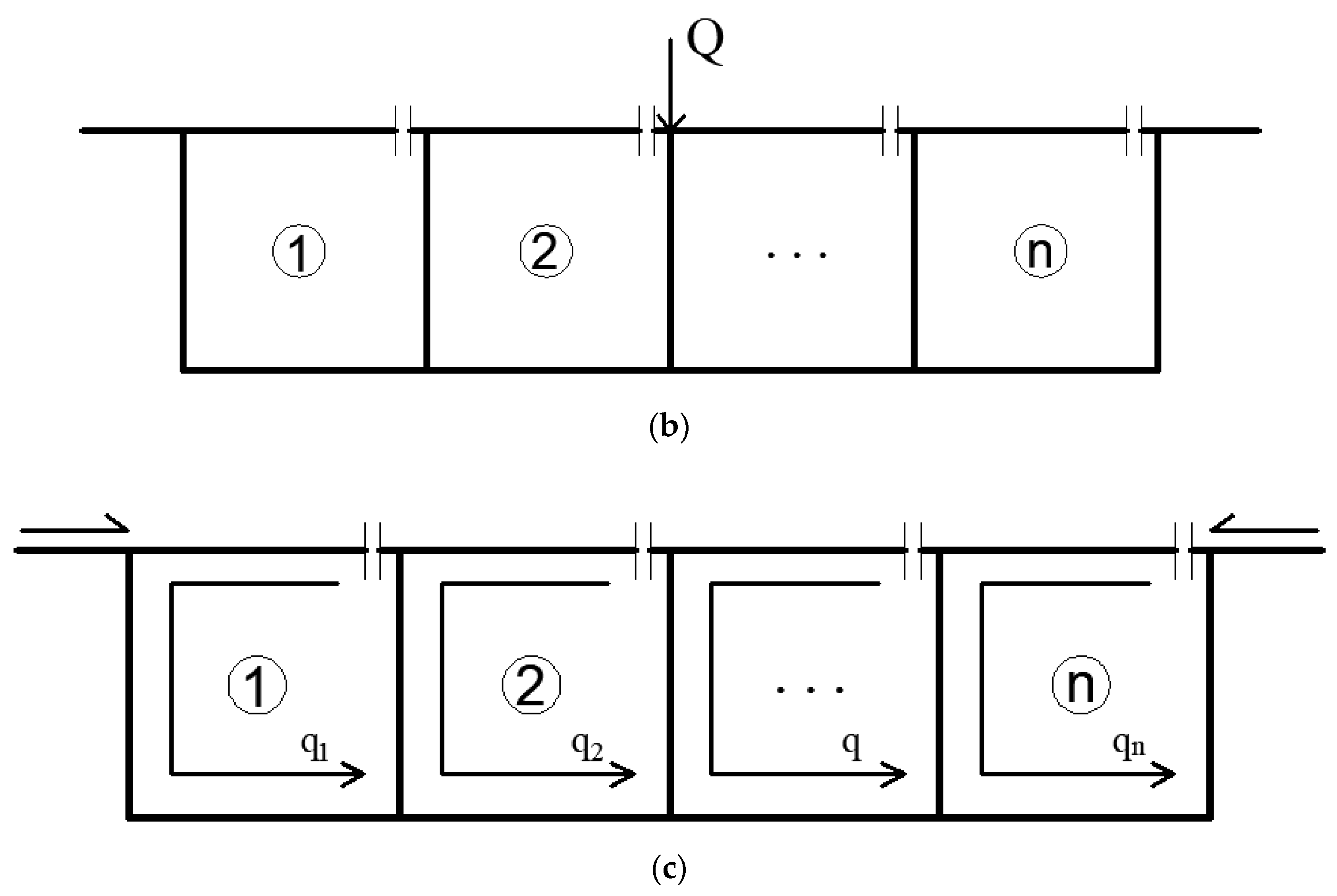
3. Shear Flow Theory
4. Finite Element Calculation Model
5. Result Analysis
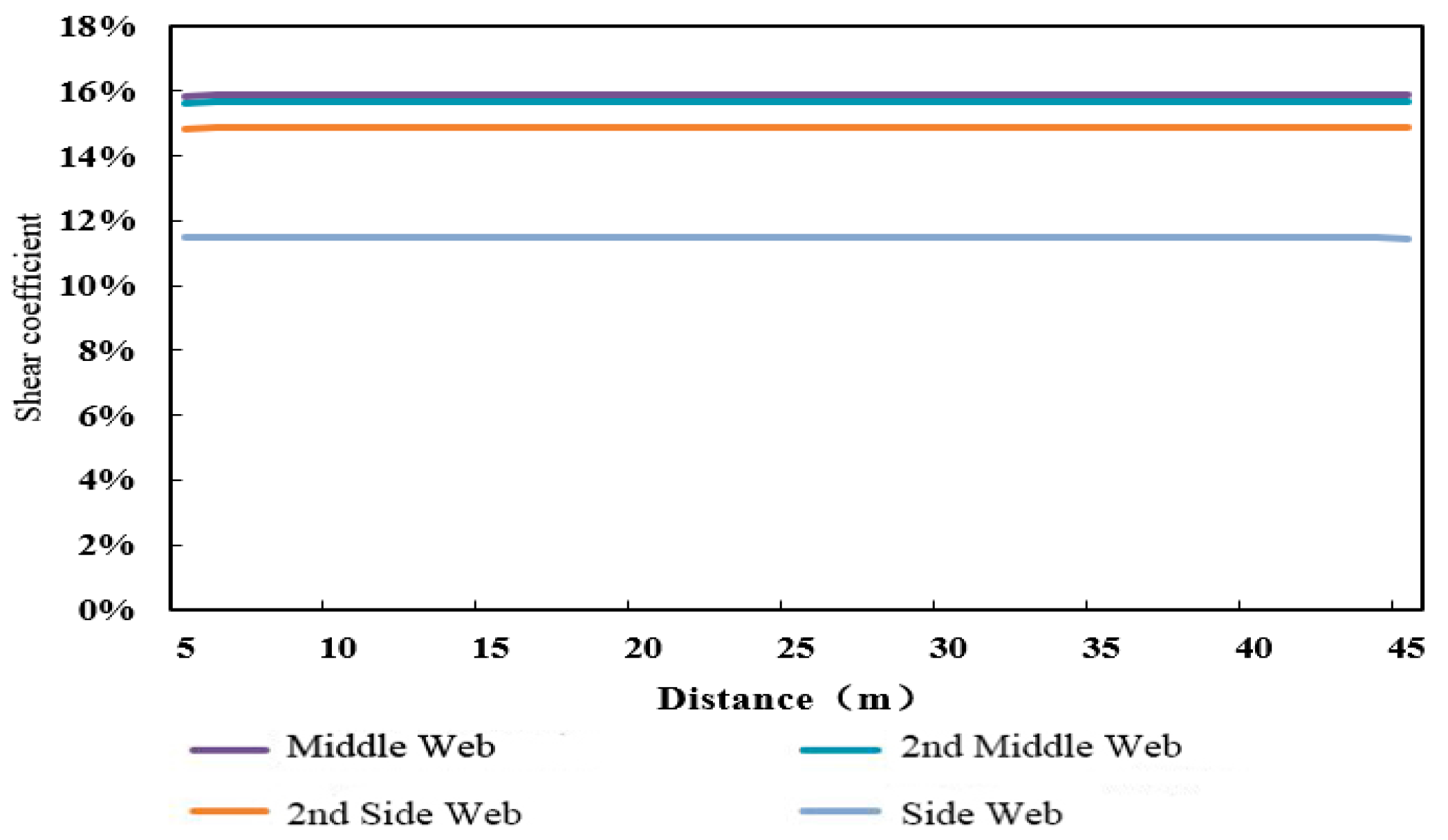
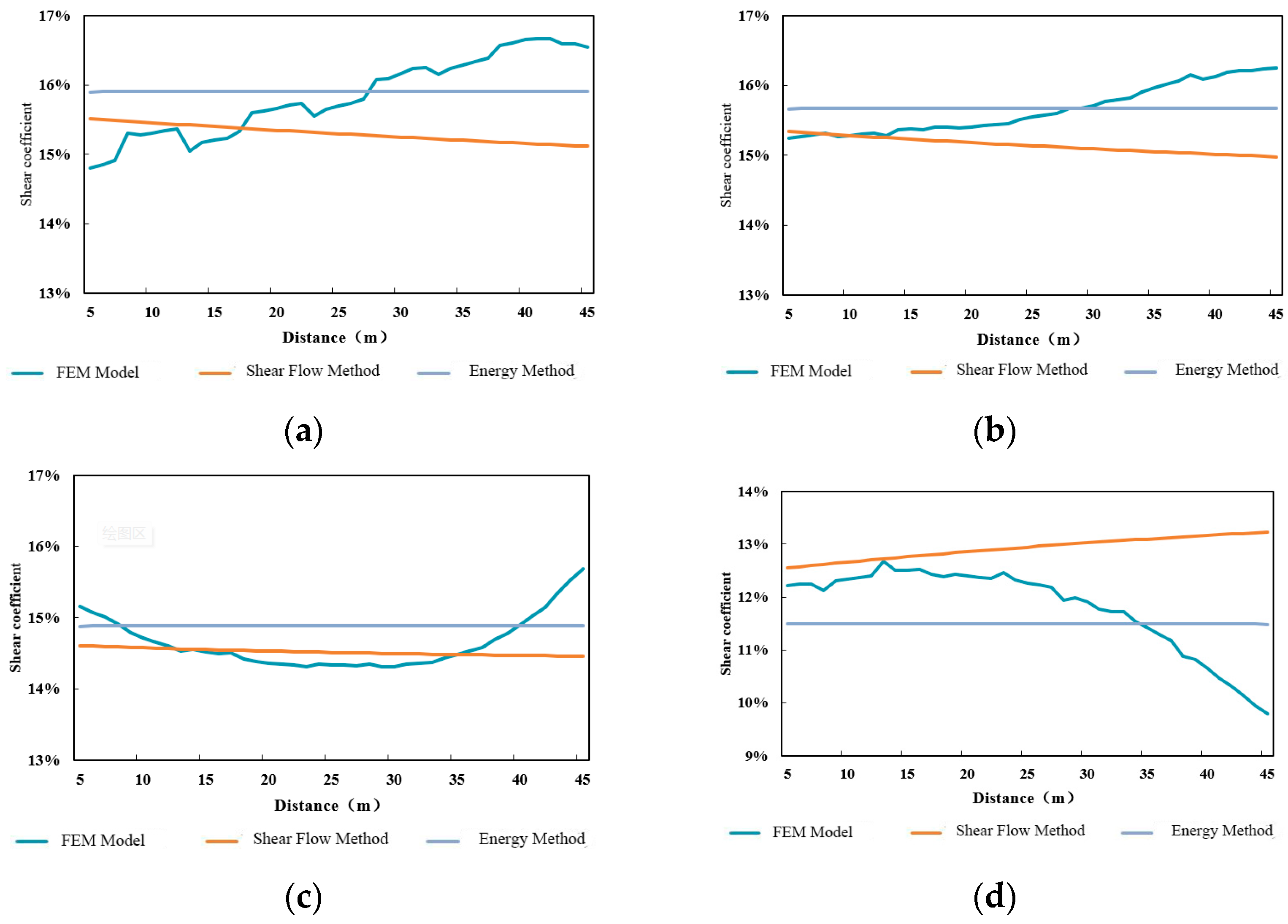
5.1. Comparative Analysis of Shear Distribution Coefficient
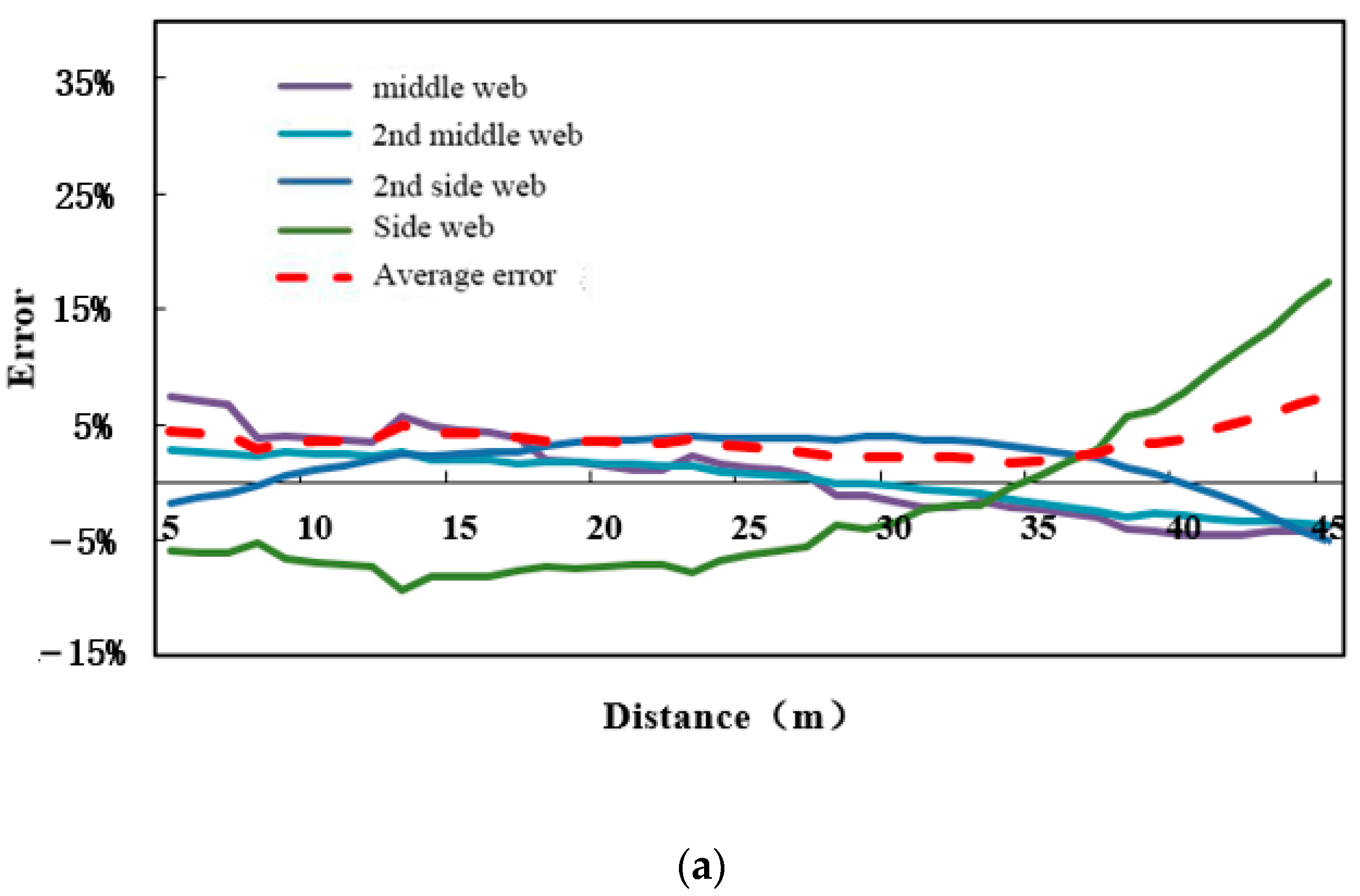
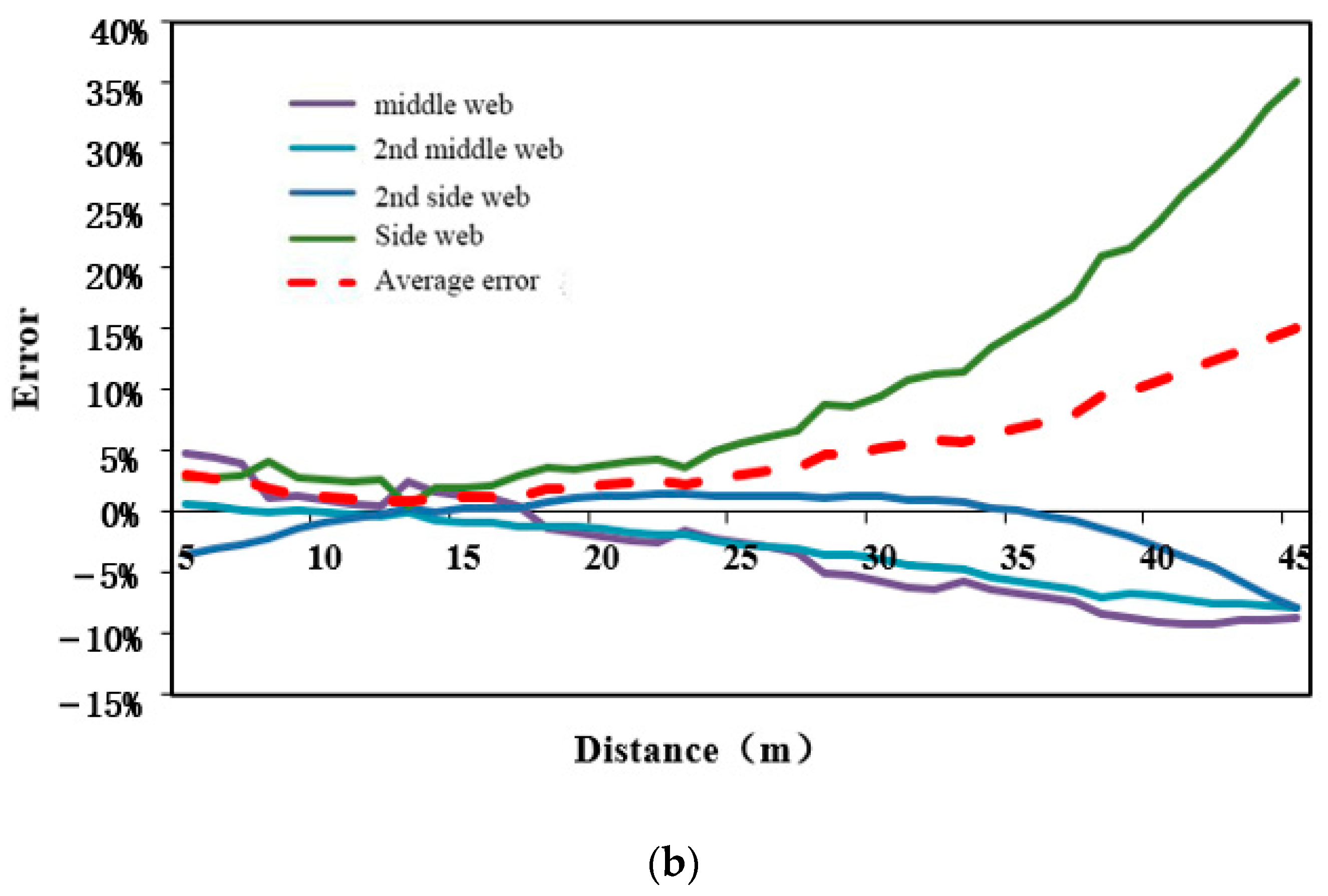
5.2. Error Analysis
6. Conclusions
- (1)
- Taking the shear force distribution coefficient as the calculation reference standard, according to the finite element results, two conclusions can be clearly drawn: (a) There is an obvious shear force distribution phenomenon between the webs of the single-box, multi-chamber section, as the shear force distribution coefficient gradually decreases from the middle web to the side web. (b) The shear force distribution coefficient is not constant, but it changes with the distance from the free end.
- (2)
- Comparing the finite element model with the two calculation theories, four conclusions can be drawn: (a) The law of the shear force distribution coefficient of each web of the shear flow method varies with the distance from the free end, which is contrary to the finite element result. The shear force distribution coefficient of each web in the method basically does not change with the distance from the free end. (b) Generally speaking, the energy method calculates the shear force distribution coefficient, the finite element error is small, and the error distribution has no obvious law. The error with the finite element is larger, and as the distance from the free end increases, the error becomes larger. (c) From the perspective of subdivided webs, the energy method has a larger error with the side webs, and the closer to the fixed end, the greater the error; the maximum error exceeds 15%, and the other webs have smaller errors, and the absolute value of the error is basically 5%. Within, the shear flow method has a large error with the side webs; the maximum error exceeds 35%, and there are also large errors with other webs; the maximum error is close to 10%.
- (3)
- Regardless of whether it is a single web or a whole, the energy method is more accurate than the shear flow method in calculating the shear distribution coefficient. The energy method, based on the principle of conservation of energy, assumes linear material behavior and small deformations. In the case of corrugated steel webs, these assumptions may not always hold true, particularly under high shear loads or complex stress conditions, leading to potential discrepancies. The shear flow method, often used for thin-walled structures, assumes uniform shear stress distribution across the thickness, which might not be consistently accurate for corrugated steel web structures, especially under high shear flow or when the web thickness varies. The finite element method, a numerical approach, is susceptible to errors due to approximations in the numerical solution, definitions of boundary conditions, or simplifications in the material behavior or geometry of the structure. The quality and density of the mesh used in the finite element analysis can also significantly impact the accuracy of the results.
- (4)
- In the present study, the influence of torsion and distortion on the shear stress of waveform webs was not considered, and the folding effect of waveform webs could not be calculated. Further research should focus on these two aspects and conduct corresponding experimental verification and analysis.
- (5)
- By using the energy method to study the distribution of shear stress, we contribute to a deeper understanding of the behavior of beams under different loading conditions. The knowledge gained from our research can be applied to improve the accuracy of structural models and calculations, particularly when dealing with beams with non-uniform conditions or complex geometries. By considering the actual distribution of shear stress, designers can optimize the structural performance, ensure adequate load-bearing capacity, and prevent potential failure modes such as shear deformation or stress concentration.
- (6)
- The focus of this paper is to introduce the calculation method of the shear stress distribution coefficient for the waveform steel web-plate. Therefore, a parametric analysis of the relevant parameters of the waveform steel web-plate box girder bridge was not conducted. In the future, it is necessary to perform parameter analysis and experimental verification of factors that influence the shear stress performance of the waveform steel web-plate, such as the thickness of the steel web-plate, the thickness of the top and bottom plates, and the waveform of the steel web-plate. This will provide references for improving the theoretical analysis and design of such structures.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hendy, C.R.; Johnson, R. Designers’ Guide to EN 1994-2 Eurocode 4: Design of Composite Steel and Concrete Structures; General Rules and Rules for Bridges; Thomas Telford: London, UK, 2006; ISBN 0727731610. [Google Scholar]
- Units, C.U.S.; Edition, T. AASHTO LRFD Bridge Design Specifications; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2010; ISBN 9781560514053. [Google Scholar]
- Abbas, H.H.; Sause, R.; Driver, R.G. Analysis of Flange Transverse Bending of Corrugated Web I-Girders under In-Plane Loads. J. Struct. Eng. 2007, 133, 347–355. [Google Scholar] [CrossRef]
- Elgaaly, M.; Hamilton, R.W.; Seshadri, A. Shear Strength of Beams with Corrugated Webs. J. Struct. Eng. 1996, 122, 390–398. [Google Scholar] [CrossRef]
- Elgaaly, M.; Seshadri, A. Girders with Corrugated Webs under Partial Compressive Edge Loading. J. Struct. Eng. 1997, 123, 783–791. [Google Scholar] [CrossRef]
- Elgaaly, M.; Seshadri, A. Depicting the behavior of girders with corrugated webs up to failure using non-linear finite element analysis. Adv. Eng. Softw. 1998, 29, 195–208. [Google Scholar] [CrossRef]
- Ibrahim, S.A.; El-Dakhakhni, W.W.; Elgaaly, M. Behavior of bridge girders with corrugated webs under monotonic and cyclic loading. Eng. Struct. 2006, 28, 1941–1955. [Google Scholar]
- Khalid, Y.A.; Chan, C.L.; Sahari, B.B.; Hamouda, A.M.S. Bending behaviour of corrugated web beams. J. Mater. Process. Technol. 2004, 150, 242–254. [Google Scholar] [CrossRef]
- Metwally, A.E.; Loov, R.E. Corrugated steel webs for prestressed concrete girders. Mater. Struct. 2003, 36, 127–134. [Google Scholar]
- Moon, J.; Yi, J.W.; Choi, B.H.; Lee, H.E. Lateral–torsional buckling of I-girder with corrugated webs under uniform bending. Thin-Walled Struct. 2009, 47, 21–30. [Google Scholar]
- Nguyen, N.D.; Han, S.R.; Kim, J.H.; Kim, S.N.; Kang, Y.J. Moment modification factors of I-girder with trapezoidal web corrugations under moment gradient. Thin Walled Struct. 2012, 57, 1–12. [Google Scholar]
- Nguyen, N.D.; Han, S.R.; Lee, G.S.; Kang, Y.J. Moment modification factor of I-girder with trapezoidal-web-corrugations considering concentrated load height effects. J. Constr. Steel Res. 2011, 67, 1773–1787. [Google Scholar]
- Nguyen, N.D.; Kim, S.N.; Han, S.R.; Kang, Y.J. Elastic lateral-torsional buckling strength of I-girder with trapezoidal web corrugations using a new warping constant under uniform moment. Eng. Struct. 2010, 32, 2157–2165. [Google Scholar] [CrossRef]
- Oh, J.-Y.; Lee, D.H.; Kim, K.S. Accordion effect of prestressed steel beams with corrugated webs. Thin Walled Struct. 2012, 57, 49–61. [Google Scholar]
- Romeijn, A.; Sarkhosh, R.; Hoop, H.D. Basic parametric study on corrugated web girders with cut outs. J. Constr. Steel Res. 2009, 65, 395–407. [Google Scholar]
- He, J.; Liu, Y.Q.; Wang, S.H.; Xin, H.; Chen, H.; Ma, C. Experimental Study on Structural Performance of Prefabricated Composite Box Girder with Corrugated Webs and Steel Tube Slab. J. Bridge Eng. 2019, 24, 04019047. [Google Scholar]
- Zhou, M.; Zhang, J.D.; Zhong, J.T.; Zhao, Y. Shear Stress Calculation and Distribution in Variable Cross Sections of Box Girders with Corrugated Steel Webs. J. Struct. Eng. 2016, 142, 04016022. [Google Scholar] [CrossRef]
- Deng, W.Q.; Mao, Z.L.; Liu, D.; Zhang, J.D. Analysis and Experimental Study on Torsion and Distortion of Single Box Three-Cell Cantilever Girder with Corrugated Steel Webs. J. Build. Struct. 2020, 41, 173–181. [Google Scholar]
- Liu, B.D.; Feng, W.Z.; Ren, H.W.; Li, P.F.; Mou, K. Experimental Study on Torsion and Distortion of Continuous Rigid Frame Bridge with Corrugated Steel Webs. China Railw. Sci. 2015, 36, 40–46. [Google Scholar]
- Ji, W.; Deng, L.; He, W. Vehicle-Bridge Coupled Vibration Analysis and Calculation of Dynamic Impact Factor for the PC Box-Girder Bridge with Corrugated Steel Webs. J. Vib. Eng. 2016, 29, 1041–1047. [Google Scholar]
- Yang, Z.Y.; Yang, M.; Rong, X.L.; Tian, L. Theoretical and Numerical Study on Dynamic Characteristics of Composite Trough Girder with Corrugated Steel Webs. J. Bridge Eng. 2021, 26, 0402100. [Google Scholar]
- He, J.; Liu, Y.Q.; Chen, A.R.; Wang, Q. Deflection Calculation of Composite Girder Bridge with Corrugated Steel Web with Consideration of Shear Deformation. J. Tongji Univ. Nat. Sci. Ed. 2009, 37, 440–444. [Google Scholar]
- Nie, J.G.; Li, F.X.; Fan, J.S. Effective Stiffness Method for Calculating Deflection of Corrugated Web Girder. Eng. Mech. 2012, 29, 71–79. [Google Scholar]
- Chen, X.C.; Bai, Z.Z.; Zeng, Y.; Jiang, R.J.; Au, F.T. Prestressed Concrete Bridges with Corrugated Steel Webs: Nonlinear Analysis and Experimental Investigation. Steel Compos. Struct. 2016, 21, 1045–1067. [Google Scholar] [CrossRef]
- Jia, Z.L.; Gao, S.Z.; Tao, M.X. Shear Design Method for Corrugated Steel Web of PC Compounding Box Girder. Highw. Eng. 2015, 40, 96–100+123. [Google Scholar]
- Chao, L.; Yuhao, H.; Zhan, G. Calculation of shear stress of corrugated steel web box girder with variable section. J. Tongji Univ. 2018, 47, 475–481. [Google Scholar]
- Department of Materials Mechanics, Tongji University. Mechanics of Materials; Tongji University Press: Shanghai, China, 1987. [Google Scholar]
- Li, J. Research on practical shear stress calculation of variable cross-section composited box-girder with corrugated steel webs. J. Railw. Sci. Eng. 2017, 14, 80–86. [Google Scholar]
- Luo, R.; Edlund, B. Shear capacity of plate girders with trapezoidally corrugated webs. Thin-Walled Struct. 1996, 26, 19–44. [Google Scholar] [CrossRef]
- Wei, N.; Zhang, Y.; Yao, X. Analysis on Restrained Torsional Shear Stresses of Box Girders with Corrugated Steel Webs. Appl. Math. Mech. 2020, 41, 386–395. [Google Scholar]
- Shi, L.; Yanlin, G. Study on the Shear Performance of Wave-folded Web Beam. J. Archit. Struct. 2001, 49–54. [Google Scholar] [CrossRef]
- Song, J.Y.; Zhang, S.R.; Lu, J.M. Simulation and Influence of Initial Defects in the Analysis of Shear Buckle of Corrugated Steel Web. Highw. Traffic Technol. 2004, 64–67. [Google Scholar]
- Abbas, H.H.; Sause, R.; Driver, R.G. Shear Strength and Stability of High Performance Steel Corrugated Web Girders. In Proceedings of the Annual Stability Conference, Research Council, San Antonio, TX, USA, 8–11 February 2006; pp. 1381–1392. [Google Scholar]
- Jianguo, N.; Liang, T. Nonlinear Analysis of Pure Torsion Property for Prestressed Concrete Composite Box Girders with Corrugated Steel Webs. Chin. J. Highw. 2007, 20, 71–77. [Google Scholar]
- Li, L.; Peng, K.; Wang, W. Theoretical and Experimental Study on Shear Lag Effect of Composite Box Girder with Corrugated Steel Webs. J. Highw. Transp. Res. Dev. 2009, 26, 78–83. [Google Scholar]
- Liu, B.; Ren, H.; Li, P. Deflection Analysis Considering the Characteristics of Box Girder with Corrugated Steel Webs. China Railw. Sci. 2011, 32, 21–26. (In Chinese) [Google Scholar]
- Ji, W.; Lin, P.Z.; Liu, S.Z. Deflection Calculation and Analysis of PC Box Girder Bridges with Corrugated Steel Webs. J. Southwest Jiaotong Univ. 2018, 53, 46–55. [Google Scholar]
- Cheng, J.; Yao, H. Simplified Method for Predicting the Deflections of Composite Box Girders. Eng. Struct. 2016, 128, 256–264. [Google Scholar] [CrossRef]
- Ma, C.; Liu, S.Z.; Wu, M.Q. Matrix Analysis of Composite Box Girder with Corrugated Steel Webs Considering Shear Deformation and Shear Lag Effect. China J. Highw. Transp. 2018, 31, 80–88. [Google Scholar]
- Zhang, Z.C.; Jin, X.; Gan, Y. Vertical Bending Mechanical Properties of Box Composite Girder with Corrugated Steel Webs. China Railw. Sci. 2019, 40, 52–59. [Google Scholar]
- Zhou, C.; Wang, J.Q.; Li, L.F. Bar Simulation Method for Shear Lag Analysis of Non-Prismatic Composite Box Girders with Corrugated Steel Webs. J. China Railw. Soc. 2022, 44, 143–152. [Google Scholar] [CrossRef]




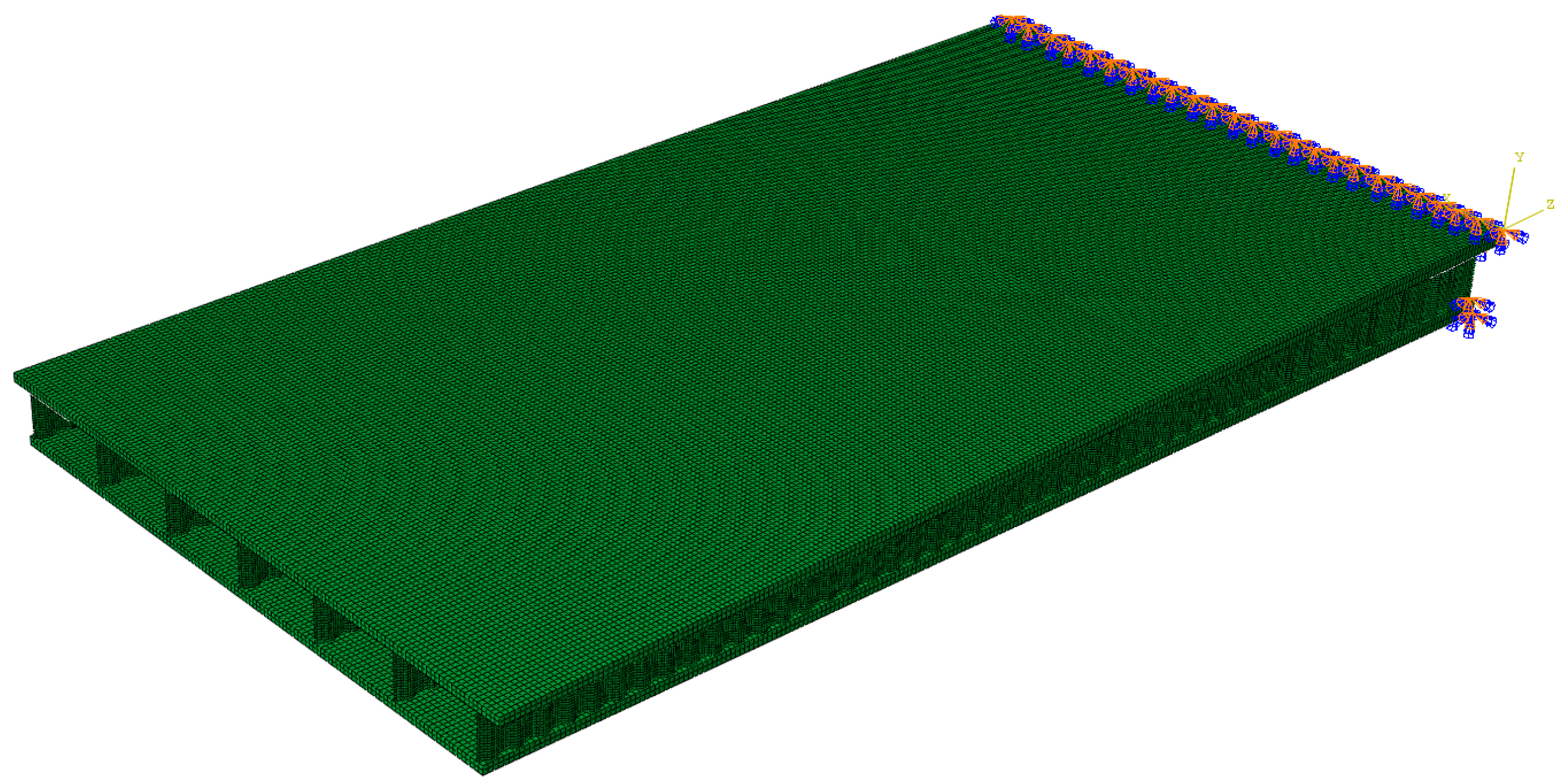
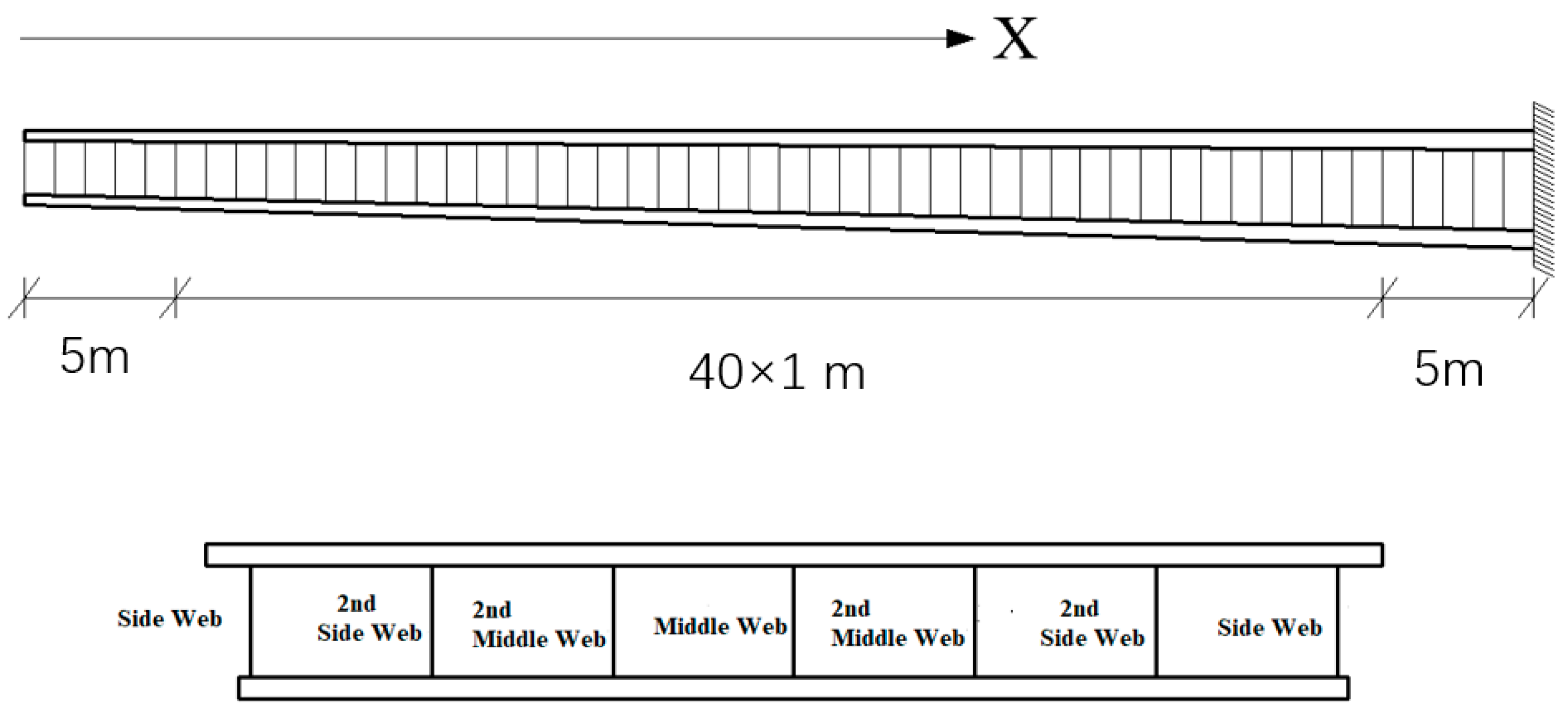
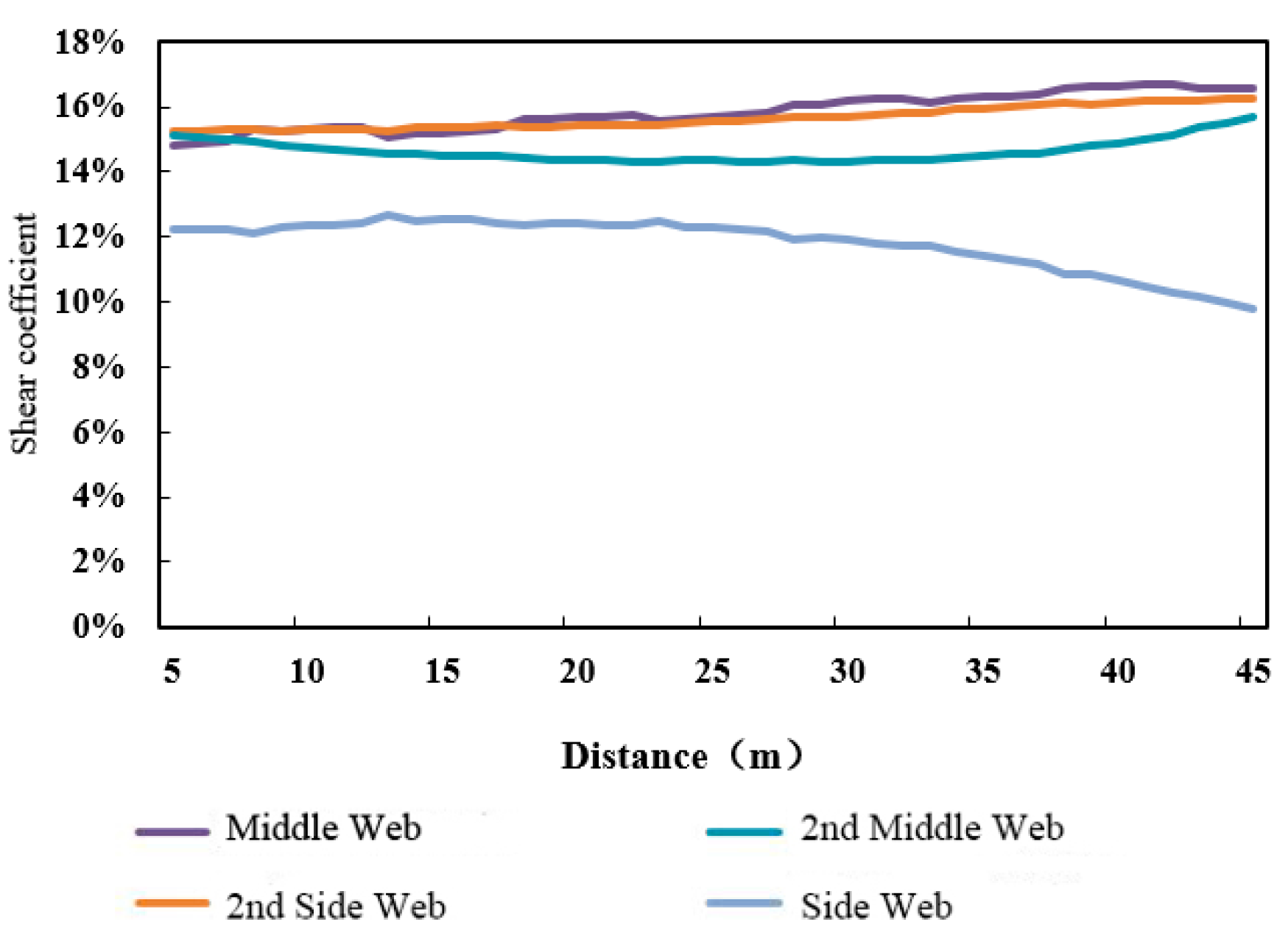
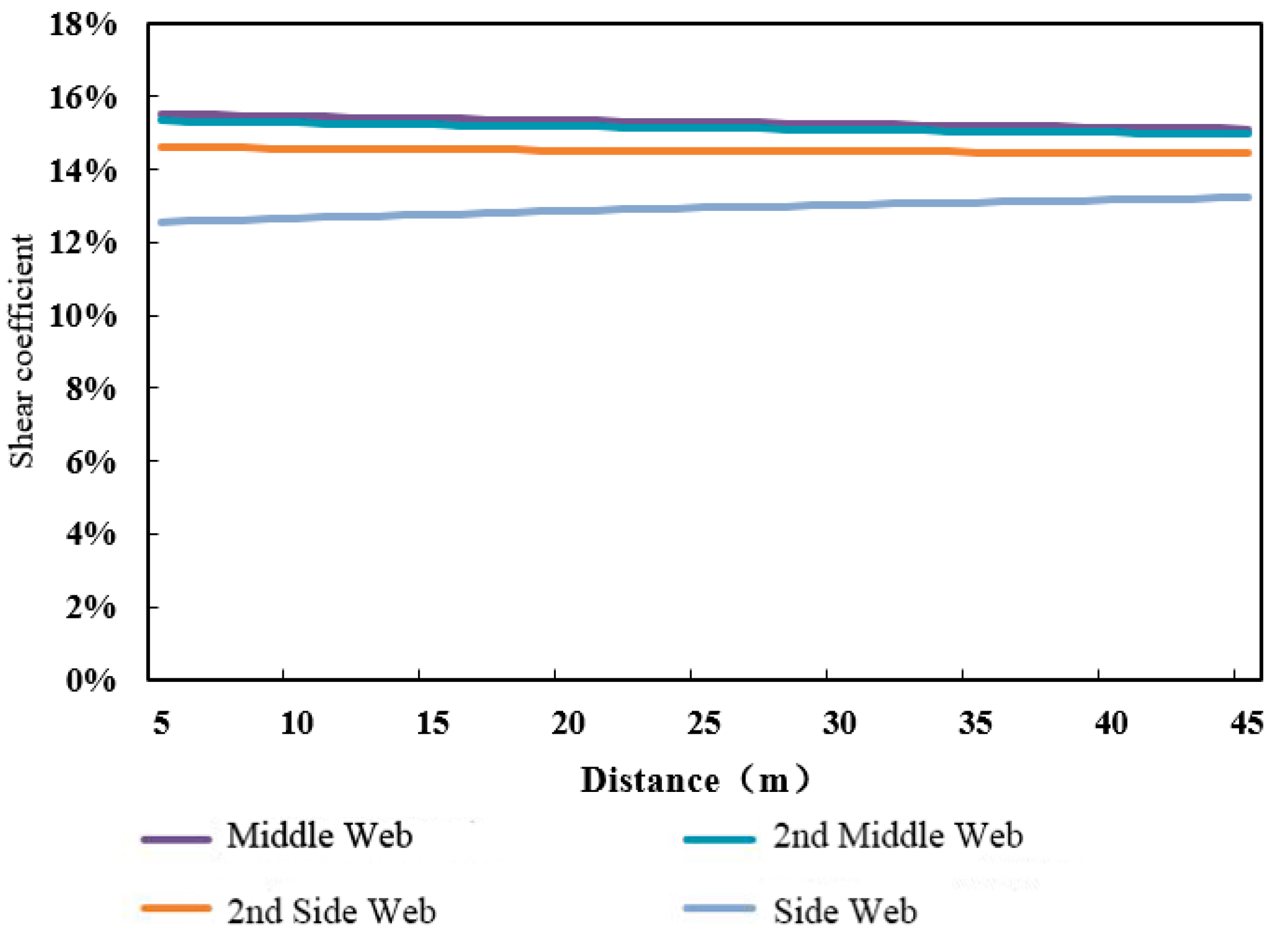
| Material | Density/kg·m−3 | Young’s Modulus/GPa | Possion’s Ratio | Element |
|---|---|---|---|---|
| Concrete | 2600 | 34.5 | 0.2 | C3D8(3D) |
| Steel | 7800 | 206.0 | 0.3 | S4R(2D) |
| Errors | Middle | Second Middle | Second Side | Side | Average | |
|---|---|---|---|---|---|---|
| Shear flow method | max | 4.8% | 0.6% | 1.4% | 35.2% | 14.9% |
| min | −9.1% | −7.8% | −7.8% | 0.4% | 0.8% | |
| average | −3.2% | −3.2% | −0.8% | 10.2% | 4.9% | |
| Energy method | max | 10.7% | 6.0% | 3.8% | 17.3% | 7.6% |
| min | −5.3% | −4.0% | −5.2% | −9.2% | 1.8% | |
| average | 0.2% | −0.2% | 1.3% | −2.5% | 3.6% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Sun, Z. Calculation Theory of Shear Stress Distribution in Box Girder with Corrugated Steel Webs Based on the Energy Method. Buildings 2023, 13, 2547. https://doi.org/10.3390/buildings13102547
Liu C, Sun Z. Calculation Theory of Shear Stress Distribution in Box Girder with Corrugated Steel Webs Based on the Energy Method. Buildings. 2023; 13(10):2547. https://doi.org/10.3390/buildings13102547
Chicago/Turabian StyleLiu, Chao, and Zizhou Sun. 2023. "Calculation Theory of Shear Stress Distribution in Box Girder with Corrugated Steel Webs Based on the Energy Method" Buildings 13, no. 10: 2547. https://doi.org/10.3390/buildings13102547
APA StyleLiu, C., & Sun, Z. (2023). Calculation Theory of Shear Stress Distribution in Box Girder with Corrugated Steel Webs Based on the Energy Method. Buildings, 13(10), 2547. https://doi.org/10.3390/buildings13102547





