Efficient Finite Element Approach to Four-Variable Power-Law Functionally Graded Plates
Abstract
:1. Introduction
2. Problem Description and Modeling
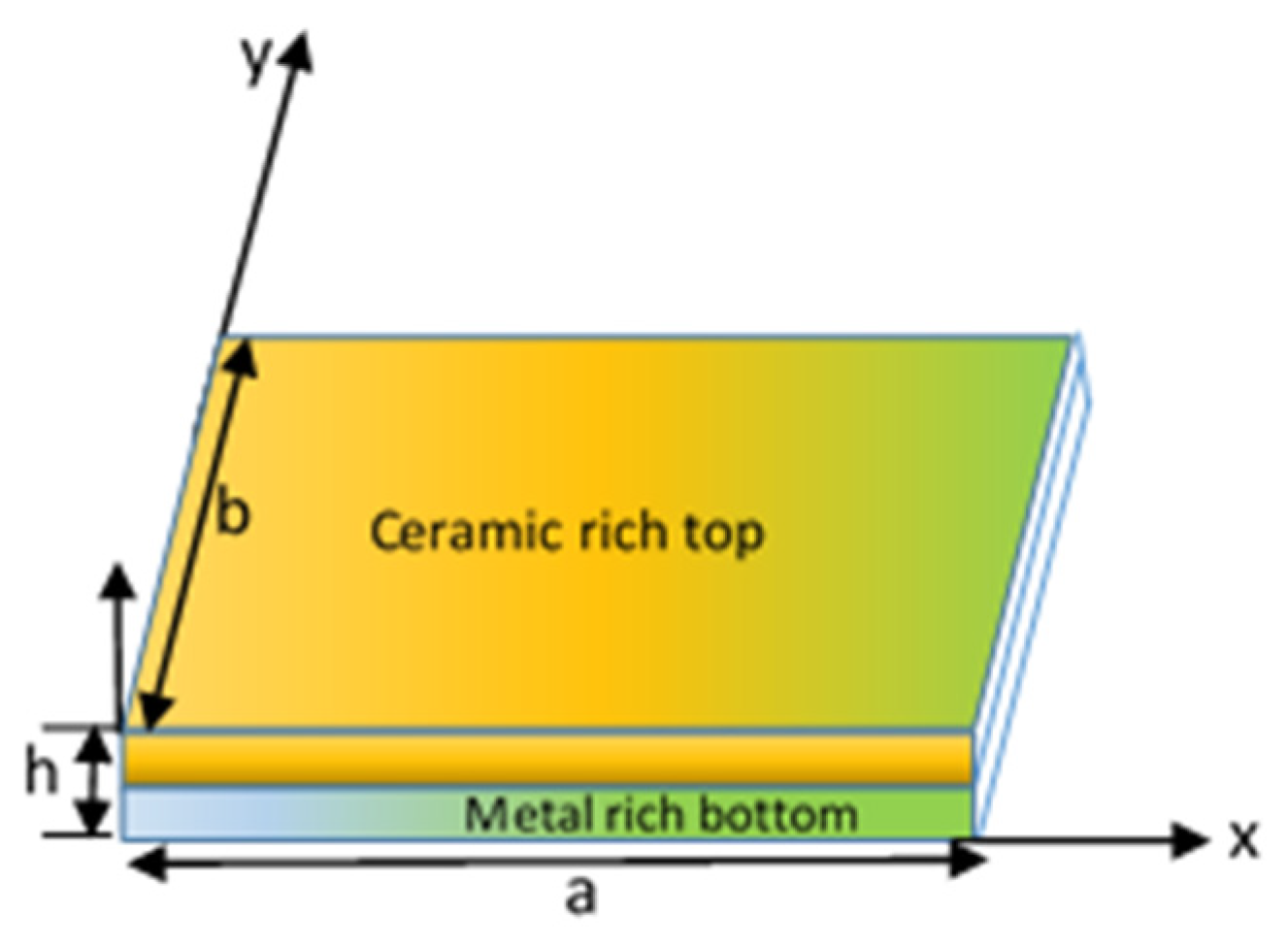
2.1. Modeling of Plates
2.2. Kinematic Field
3. Investigation of Numerical Problems
4. Conclusions
- A generalized power law variation led to different combinations of FGM constituents by means of appropriate choices of the material gradient parameters.
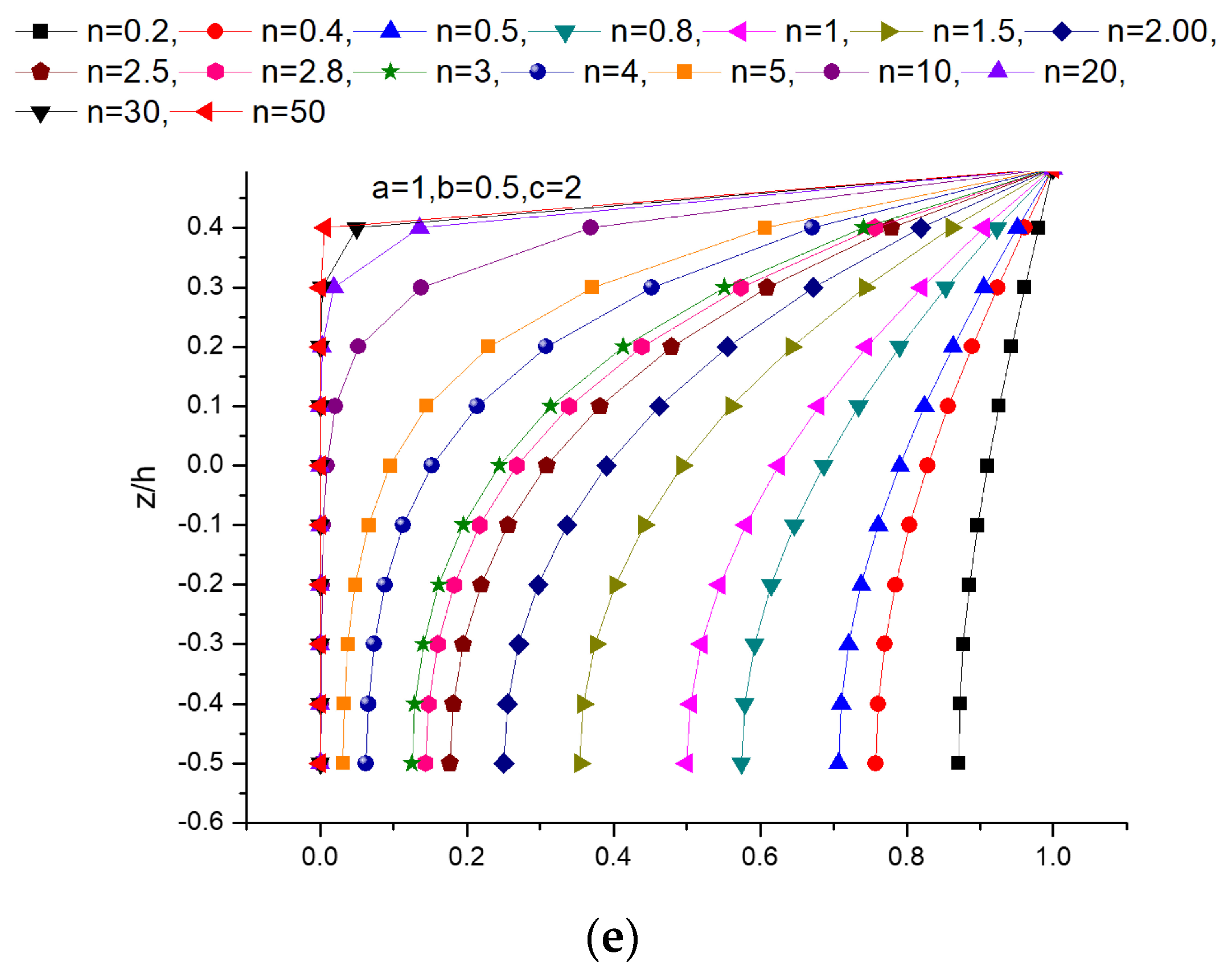
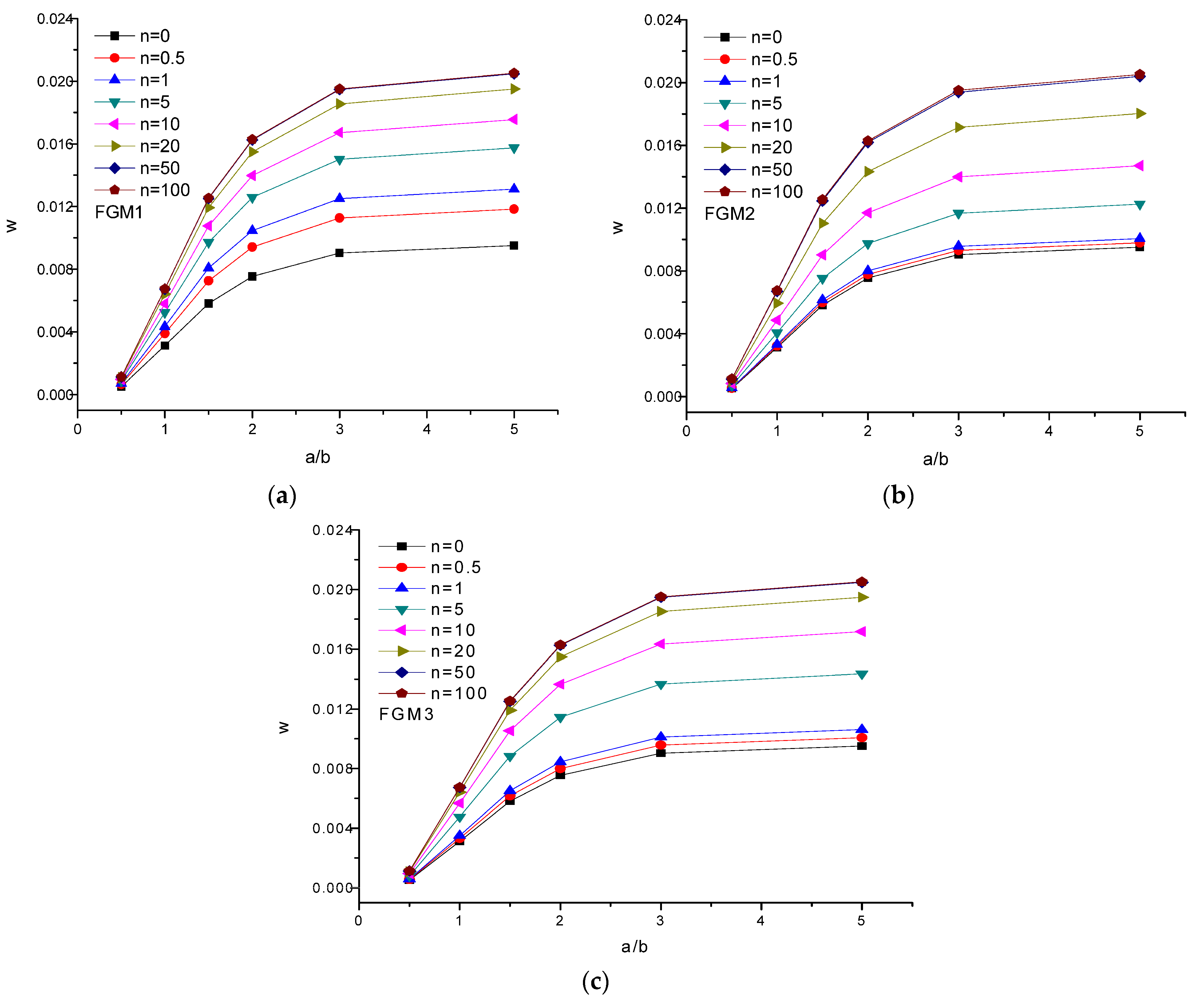
- Different FGM models incorporating various boundary conditions demonstrated marked bending responses for n > 5. Further, an asymptotic response was ensured when n reached a value greater than 50.
- It was witnessed that symmetric plates exhibited lower material gradient values, which, in turn, did not play a primary role in dictating the deflection parameter when the aspect ratio was varied.
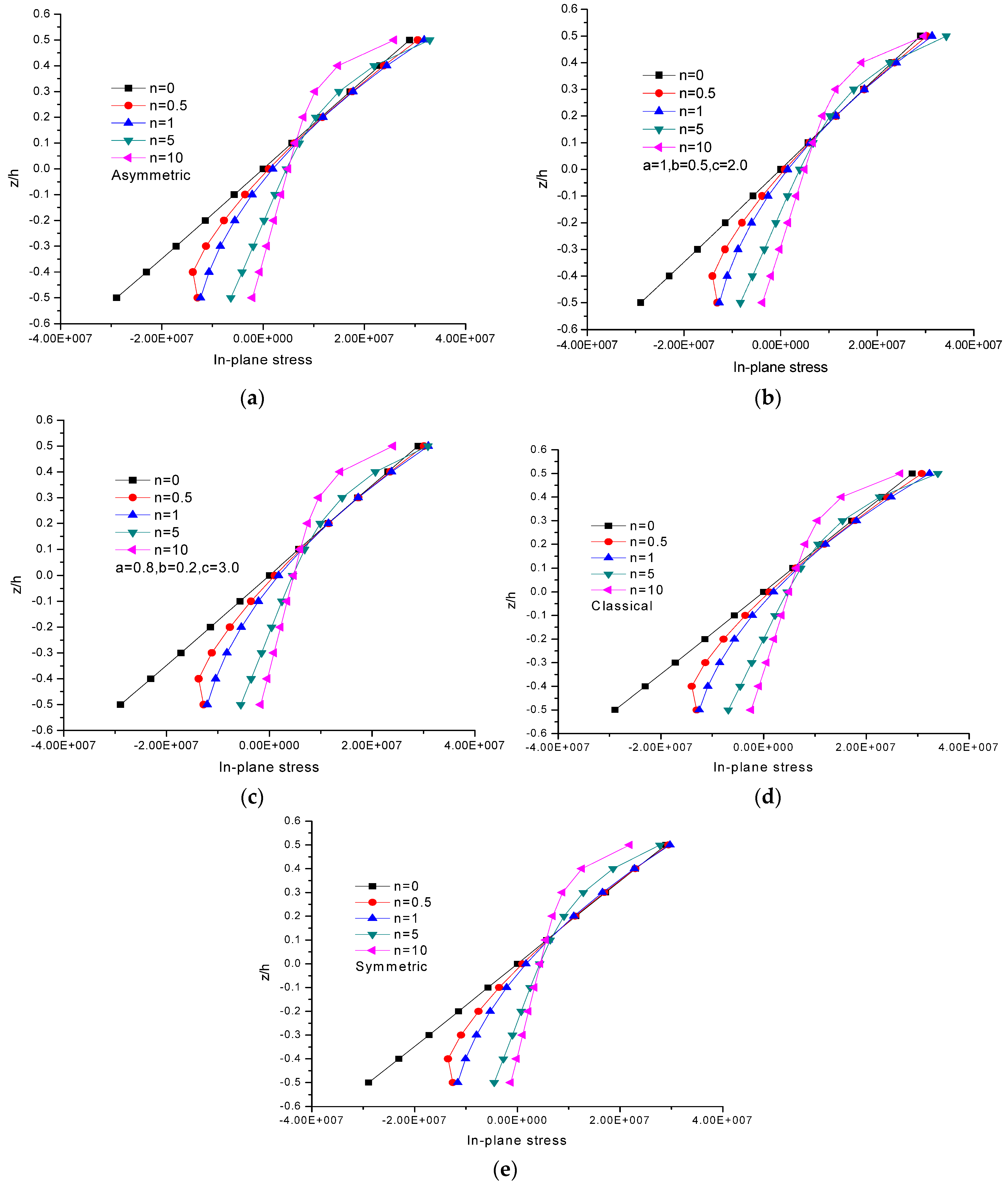
- Linear axial stress variations in the x direction were noticed for isotropic and graded plates, and the trend was not obvious when n assumed the numerical value of 10. Also, the neutral plane shifted toward the bottom segment of the plate when n approached 10 and remained unchanged.
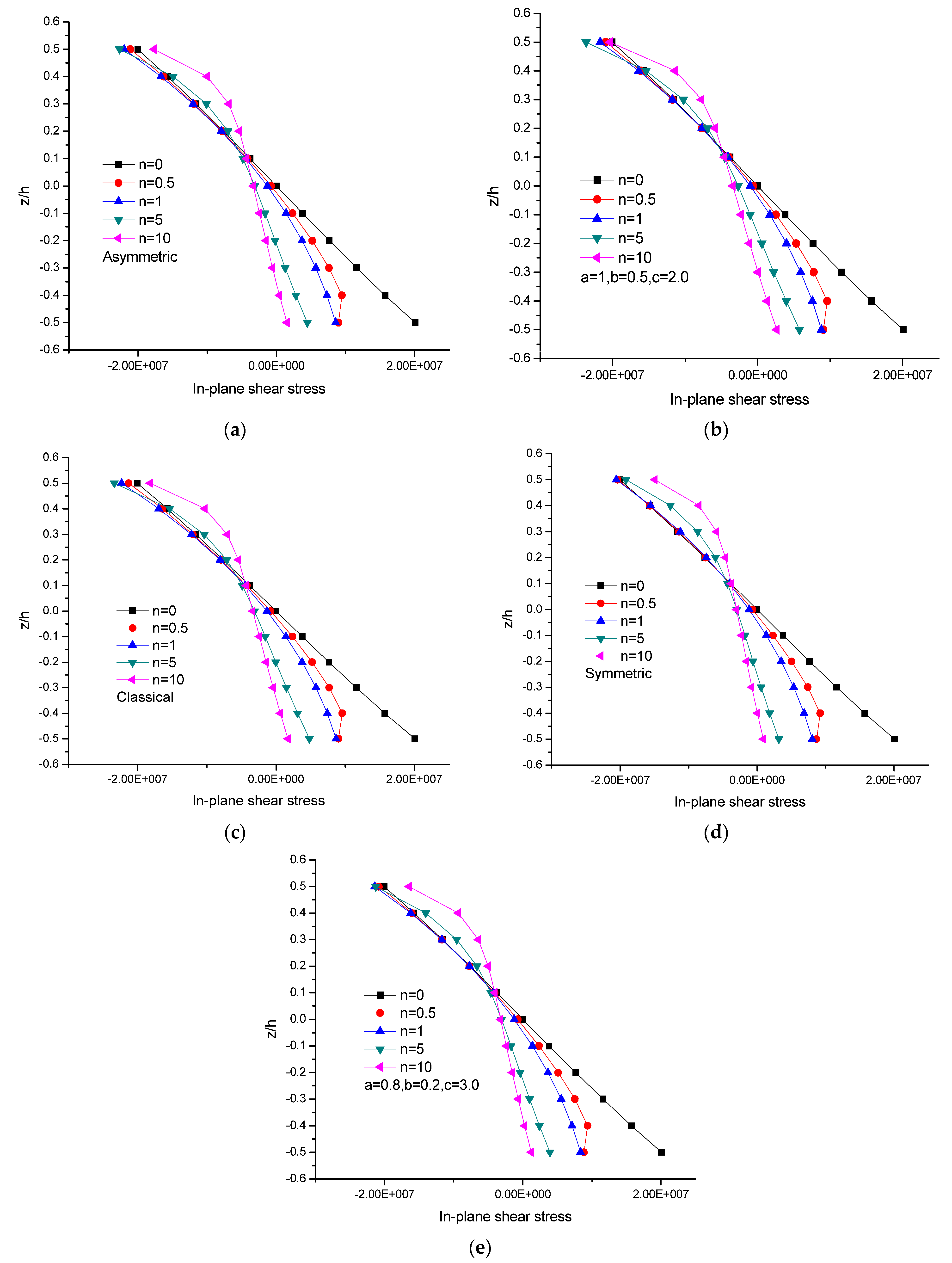
- A marked response was seen in the isotropic and graded FGM plates in the case of axial stress variations in the y direction, and this response was considerable in the bottom segment.
- The graded plate illustrated a noticeable response on par with that of the isotropic plates. The magnitudes of the tensile and compressive stresses were identical for the isotropic case, which was not true in the case of the graded plate.
- In all the cases, symmetric profiles were a better choice than other profiles for minimizing the deflection of the plate.
- As the mesh underwent refinement, the stress values tended to be elevated at the reentrant corners, which was a common observation.
- Based on the achieved results, the generalized power law containing four parameters provides structural designers with a flexible approach for multiple requirements.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Praveen, G.N.; Reddy, J.N. Non-linear transient thermo elastic analysis of functionally graded ceramic-metal plates. Int. J. Solids Struct. 1998, 35, 4457–4476. [Google Scholar] [CrossRef]
- Reddy, J.N. Analysis of functionally graded plate. Int. J. Numer. Methods Eng. 2000, 47, 663–684. [Google Scholar] [CrossRef]
- Vel, S.S.; Batra, R.C. Exact solution for thermo elastic deformation of functionally graded thick rectangular plates. J. Am. Inst. Aerosp. Astrol. 2002, 40, 20–24. [Google Scholar]
- Qian, L.F.; Batra, R.C.; Chen, L.M. Static and dynamic deformations of thick functionally graded elastic plates by using higher-order shear and normal deformable plate theory and meshless local Petroc-Galerkin method. Compos. Struct. Part B 2004, 35, 685–697. [Google Scholar] [CrossRef]
- Zenkour, A.M. Generalized shear deformation theory for bending analysis of functionally graded plates. Appl. Math. Model. 2006, 30, 67–84. [Google Scholar] [CrossRef]
- Lee, Y.Y.; Zhao, X.; Liew, K.M. Thermo elastic analysis of functionally graded plates using the element free kp-Ritz method. Smart Mater. Struct. 2009, 18, 035007–035022. [Google Scholar] [CrossRef]
- Talha, M.; Singh, B.N. Static response and free vibration analysis of FGM plates using higher order shear deformation theory. Appl. Math. Model. 2010, 34, 3991–4011. [Google Scholar] [CrossRef]
- Woodward, B.; Kashtalyan, M. 3D elasticity analysis of sandwich panels with graded core under distributed and concentrated loadings. Int. J. Mech. Sci. 2011, 53, 872–885. [Google Scholar] [CrossRef]
- Jha, D.K.; Tarun, K.; Singh, R.K. A critical review of recent research on functionally graded plates. Compos. Struct. 2012, 96, 833–849. [Google Scholar] [CrossRef]
- Tay, T.E.; Shen, F.; Lee, K.H.; Scaglione, A.; Di Sciuva, M. Mesh design in finite element analysis of post-buckled delamination in composite laminates. Compos. Struct. 1999, 47, 603–611. [Google Scholar] [CrossRef]
- Sheikh, A.H.; Chakrabarti, A. A new plate bending element based on higher order shear deformation theory for the analysis of composite plates. Finite Elem. Anal. Des. 2003, 39, 883–903. [Google Scholar] [CrossRef]
- Della Croce, L.; Venini, P. Finite elements for functionally graded Reissner-Mindlin plates. Comput. Methods Appl. Mech. Eng. 2004, 193, 705–725. [Google Scholar] [CrossRef]
- Chakrabarti, A.; Sengupta, D.; Sheikh, A. Analysis of skew composite plates using a new triangular element based on higher order shear deformation theory. Inst. Eng. 2004, 85, 77–83. [Google Scholar]
- Na, K.S.; Kim, J.H. Thermal post buckling investigations of functionally graded plates using 3-D finite element method. Finite Elem. Anal. Des. 2006, 42, 749–756. [Google Scholar] [CrossRef]
- Kulkarni, S.D.; Kapuria, S. A new discrete Kirchoff quadrilateral element based on the third order theory for composite plates. Comput. Mech. 2007, 39, 237–246. [Google Scholar] [CrossRef]
- Ayhan, A.O. Stress intensity factors for three-dimensional cracks in functionally graded materials using enriched finite elements. Int. J. Solids Struct. 2007, 44, 8579–8599. [Google Scholar] [CrossRef]
- Del Coz Díaz, J.J.; García Nieto, P.J.; Álvarez Rabanal, F.P. Finite element analysis of thin-walled composite two-span wood-based load bearing stressed skin roof panels and experimental validation. Thin-Walled Struct. 2008, 46, 276–289. [Google Scholar] [CrossRef]
- Panda, A.; Ray, M.C. Non-linear finite element analysis of functionally graded plates integrated with patches of piezoelectric fiber reinforced composite. Finite Elem. Anal. Des. 2008, 44, 493–504. [Google Scholar] [CrossRef]
- Almeida, F.S.; Awruch, A.M. Design optimization of composite laminated structures using genetic algorithms and finite element analysis. Compos. Struct. 2009, 88, 443–454. [Google Scholar] [CrossRef]
- Liu, P.F.; Hou, S.J.; Chu, J.K.; Hu, X.Y.; Zhou, C.L.; Liu, Y.L.; Zheng, J.Y.; Zhao, A.; Yan, L. Finite element analysis of post buckling and delamination of composite laminates using virtual crack closure technique. Compos. Struct. 2011, 93, 1549–1560. [Google Scholar] [CrossRef]
- Abdelaziz, H.H.; Atmane, H.A.; Mechab, I.; Boumia, L.; Tounsi, A.; El Abbas, A.B. Static analysis of functionally graded sandwich plates using an efficient and simple refined theory. Chin. J. Aeronaut. 2011, 24, 434–448. [Google Scholar] [CrossRef]
- Natarajan, S.; Manickam, G. Bending and vibration of functionally graded material sandwich plates using an accurate theory. Finite Elem. Anal. Des. 2002, 57, 32–42. [Google Scholar] [CrossRef]
- Gao, Z.; Lee, K.Y.; Zhou, Y. Coupled thermo-mechanical analysis of functionally gradient weak/micro discontinuous interface with graded finite element method. Acta Mech. Solida Sin. 2012, 25, 331–341. [Google Scholar] [CrossRef]
- Valizadeh, N.; Natarajan, S.; Gonzalez-Estrada, O.A.; Rabczuk, T.; Bui, T.Q.; Bordas, S.P. NURBS-based finite element analysis of functionally graded plates: Static bending, vibration, buckling and flutter. Compos. Struct. 2003, 99, 209–326. [Google Scholar] [CrossRef]
- Taj, G.; Chakrabarti, A. Static and dynamic analysis of functionally graded skew plates. ASCE J. Eng. Mech. 2013, 139, 848–857. [Google Scholar] [CrossRef]
- Taj, M.G.; Chakrabarti, A.; Sheikh, A.H. Analysis of functionally graded plates using higher order shear deformation theory. Appl. Math. Model. 2013, 37, 8484–8494. [Google Scholar]
- Tornabene, F. Free vibration analysis of functionally graded conical, cylindrical shell and annular plate structures with a four-parameter power-law distribution. Comput. Methods Appl. Mech. Eng. 2009, 198, 2911–2935. [Google Scholar] [CrossRef]
- Violoa, E.; Tornabene, F. Free vibrations of three parameter functionally graded parabolic panels of revolution. Mech. Res. Commun. 2009, 36, 587–594. [Google Scholar] [CrossRef]
- Matbuly, M.S.; Ragb, O.; Nassar, M. Natural frequencies of a functionally graded cracked beam using the differential quadrature method. Appl. Math. Comput. 2009, 215, 2307–2316. [Google Scholar] [CrossRef]
- Alibeigloo, A.; Nouri, V. Static analysis of functionally graded cylindrical shell with piezoelectric layers using differential quadrature method. Comput. Struct. 2010, 92, 1775–1785. [Google Scholar] [CrossRef]
- Hosseini-Hashemi, S.H.; Akhavan, H.; Rokni Damavandi Taher, H.; Daemi, N.; Alibeigloo, A. Differential quadrature analysis of functionally graded circular and annular sector plates on elastic foundation. Mater. Des. 2010, 31, 1871–1880. [Google Scholar] [CrossRef]
- Akbari Alashti, R.; Khorsand, M. Three-dimensional thermo-elastic analysis of a functionally graded cylindrical shell with piezoelectric layers by differential quadrature method. Int. J. Press. Vessels Pip. 2011, 88, 167–180. [Google Scholar] [CrossRef]
- Sobhani Aragh, B.; Hedayati, H.; Borzabadi Farahani, E. A novel 2-D six-parameter power-law distribution for free vibration and vibrational displacements of two-dimensional functionally graded fiber- reinforced curved panel. Eur. J. Mech. A Solids 2011, 30, 865–883. [Google Scholar] [CrossRef]
- Setoodeh, A.R.; Tahani, M.; Selahi, E. Hybrid layerwise-differential quadrature transient dynamic analysis of functionally graded axisymmetric cylindrical shells subjected to dynamic pressure. Comput. Struct. 2011, 93, 2663–2670. [Google Scholar] [CrossRef]
- Malekzadeh, P.; Heydarpour, Y.; Golbahar Haghighi, M.R. Transient response of rotating laminated functionally graded cylindrical shells in thermal environment. Int. J. Press. Vessels Pip. 2012, 92, 43–56. [Google Scholar] [CrossRef]
- Rajasekaran, S. Free vibration of centrifugally stiffened axially functionally graded tapered Timoshenko beams using differential transformation and quadrature methods. Appl. Math. Model. 2013, 37, 4440–4463. [Google Scholar] [CrossRef]
- Tornaben, F.; Violoa, E. Static analysis of functionally graded doubly-curved shells and panels of revolution. Meccanica 2013, 48, 901–930. [Google Scholar] [CrossRef]
- Liu, G.R.; Wang, J.G. A point interpolation meshless methods based on radial basis functions. Int. J. Numer. Methods Eng. 2002, 54, 1623–1648. [Google Scholar]
- Ferreira, A.J.M. A formulation of the multi quadricraial basis function method for the analysis of laminated composite plates. Compos. Struct. 2003, 59, 385–392. [Google Scholar] [CrossRef]
- Ferreira, A.J.M. Thick composite beam analysis using a global meshless approximation based on radial basis function. Mech. Adv. Mater. Struct. 2003, 10, 271–284. [Google Scholar] [CrossRef]
- Ferreira, A.J.M.; Rouque, C.M.C.; Pals, M. Analysis of composite plates using higher order shear deformation theory and a finite point formulation based on the multiquadric radial basis function method. Compos. Part B 2003, 34, 627–636. [Google Scholar] [CrossRef]
- Neves, A.M.A.; Ferreira, A.J.M.; Carrera, E. Static analysis of functionally graded sandwich plates according to a hyperbolic theory considering Zig-Zag and warping effects. Adv. Eng. Softw. 2012, 52, 30–43. [Google Scholar] [CrossRef]
- Neves, A.M.A.; Ferreira, A.J.M.; Carrera, E. A quasi 3d sinusoidal shear deformation theory for the static and free vibration analysis of functionally graded plates. Compos. Part B 2012, 43, 711–725. [Google Scholar] [CrossRef]
- Neves, A.M.A.; Ferreira, A.J.M.; Carrera, E. Static, free vibration and buckling analysis of isotropic and sandwich functionally graded plates using a quasi-3D higher-order shear deformation theory and a meshless technique. Compos. Part B 2013, 44, 657–674. [Google Scholar] [CrossRef]
- Carrera, E.; Brischetto, S.; Cinefra, M. Effects of thickness stretching in functionally graded plates and shells. Compos. Part B 2009, 42, 123–133. [Google Scholar] [CrossRef]
- Rychter, Z. Generalized displacements and the accuracy of classical plate theory. Int. J. Solids Struct. 1993, 30, 129–136. [Google Scholar] [CrossRef]
- Volokh, K.Y. On the classical theory of plates. J. Appl. Math. Mech. 1994, 58, 1101–1110. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Rastgo, A. An analytical study on the free vibration of smart circular thin FGM plate based on classical plate theory. Thin Walled Struct. 2008, 46, 1402–1408. [Google Scholar] [CrossRef]
- Aydogdu, M. Conditions for functionally graded plates to remain flat under in-plane loads by classical plate theory. Compos. Struct. 2008, 82, 155–157. [Google Scholar] [CrossRef]
- Reddy, J.N. Nonlocal nonlinear formulations for bending of classical and shear deformation theories of beams and plates. J. Eng. Sci. 2010, 48, 1507–1518. [Google Scholar] [CrossRef]
- Cheng, Z.Q.; Batra, R.C. Deflection relationships between the homogeneous Kirchhoff plate theory and different functionally graded plate theories. Arch. Mech. 2000, 52, 143–158. [Google Scholar]
- Qian, L.F.; Batra, R.C.; Chen, L.M. Elasto static deformations of a thick plate by using a higher-order shear and normal deformable plate theory and two meshless local Petrov-Galerkin (MLPG) methods. Comput. Model Eng. Sci. 2003, 4, 161–176. [Google Scholar]
- Qian, L.F.; Batra, R.C.; Chen, L.M. Free and forced vibrations of thick rectangular plates by using higher-order shear and normal deformable plate theory and meshless local Petrov-Galerkin (MLPG) method. Comput. Model Eng. Sci. 2003, 4, 519–534. [Google Scholar]
- Liu, T.M.; Thach, L.N.; Quoc, T.H. Finite element modeling for bending and vibration analysis of laminated and sandwich composite plates based on higher order theory. Comput. Mater. Sci. 2010, 49, 390–394. [Google Scholar]
- Cinefra, M.; Carrera, E.; Brischetto, S. Thermo-mechanical analysis of functionally graded shells. J. Therm. Stresses 2010, 33, 942–963. [Google Scholar] [CrossRef]
- Reddy, J.N. A generalization of two dimensional theories of laminated composite plates. Commun. Appl. Numer. Methods 1987, 3, 173–180. [Google Scholar] [CrossRef]
- Emat-alla, M. Reduction of thermal stresses by developing two-dimensional functionally graded material. Int. J. Solids Struct. 2003, 40, 7339–7356. [Google Scholar] [CrossRef]
- Qian, L.F.; Batra, R.C. Design of bidirectional functionally graded plate for optimal natural frequencies. J. Sound Vib. 2005, 280, 415–424. [Google Scholar] [CrossRef]
- Hedia, H.S. Comparison of one-dimensional and two-dimensional functionally graded materials for the backing shell of the cemented ace tabular cup. J. Biomed. Mater. Res. Part B Appl. Biomater. 2005, 74, 732–739. [Google Scholar] [CrossRef]
- Hedia, H.S.; Shabara, M.; El-midany, T.T. Improved design of cementless ship stems using two-dimensional functionally graded materials. J. Biomed. Mater. Res. Part B Appl. Biomater. 2006, 79, 42–49. [Google Scholar] [CrossRef]
- Asgari, M.; Akhlaghi, M.; Hosseini, S.M. Dynamic analysis of two-dimensional functionally graded thick hollow cylinder with finite length under impact loading. Acta Mech. 2009, 208, 163–180. [Google Scholar] [CrossRef]
- Alipour, M.M.; Shariyat, M.; Shaban, M. A semi analytical solution for free vibration of variable thickness two-directional-functionally graded plates on elastic foundations. Int. J. Mech. Mater. Des. 2010, 6, 293–304. [Google Scholar] [CrossRef]
- Suresh, S.; Mortensen, A. Fundamentals of Functionally Graded Materials, 1st ed.; IOM Communications: London, UK, 1998. [Google Scholar]
- Chung, Y.L.; Chi, S.H. The residual stress of functionally graded materials. J. Chin. Instit. Civil Hydraul. Eng. 2001, 13, 1–9. [Google Scholar]
- Jin, Z.H.; Batra, R.C. Stress intensity relaxation at the top of an edge crack in a functionally graded material subjected to a thermal shock. J. Therm. Stresses 1996, 19, 317–333. [Google Scholar] [CrossRef]
- Tornabene, F.; Violoa, E. Free vibrations of four-parameter functionally graded parabolic panels and shells of revolution. Eur. J. Mech. A Solids 2009, 28, 991–1013. [Google Scholar] [CrossRef]
- Yas, M.H.; Sobhani Arag, B. Elasticity solution for free vibration analysis of four-parameter functionally graded fiber orientation cylindrical panels using differential quadrature method. Eur. J. Mech. A Solids 2011, 30, 631–638. [Google Scholar] [CrossRef]
- Toorani, M.H.; Lakis, A.A. General equations of anisotropic plates and shells including transverse shear deformations, rotary inertia and initial curvature effects. J. Sound Vib. 2000, 237, 561–615. [Google Scholar] [CrossRef]
- Vidal, P.; Gallimard, O.; Polit, L.; Valot, E. Analysis of functionally graded plates based on a variable separation method. Mech. Adv. Mater. Struct. 2021, 29, 4890–4901. [Google Scholar] [CrossRef]
- Barbosa, J.A.T.; Ferreira, A.J.M. Geometrically nonlinear analysis of functionally graded plates and shells. Mech. Adv. Mater. Struct. 2010, 17, 40–48. [Google Scholar] [CrossRef]
- Turteltaub, S. Optimal control and optimization of functionally graded materials for thermomechanical processes. Int. J. Solids Struct. 2002, 39, 3175–3197. [Google Scholar] [CrossRef]
- Turteltaub, S. Optimal non-homogeneous composites for dynamic loading. Struct. Multidiscip. Optim. 2005, 30, 101–112. [Google Scholar] [CrossRef]
- Abdalla, H.M.A.; Casagrande, D.; De Bona, F. A Dynamic programming setting for functionally graded thick-walled cylinders. Materials 2020, 13, 3988. [Google Scholar] [CrossRef] [PubMed]
- Boussaa, D. Optimizing the composition profile of a functionally graded interlayer using a direct transcription method. Comput. Mech. 2006, 39, 59–71. [Google Scholar] [CrossRef]
- Abdalla, H.M.A.; Casagrande, D. An intrinsic material tailoring approach for functionally graded axisymmetric hollow bodies under plane elasticity. J. Elast. 2021, 144, 15–32. [Google Scholar] [CrossRef]
- Abdalla, H.M.A.; Boussaa, D.; Sburlati, R.; Casagrande, D. On the best volume fraction distributions for functionally graded cylinders, spheres and disks—A pseudospectral approach. Compos. Struct. 2023, 311, 116784. [Google Scholar] [CrossRef]
- Van Vinh, P.; Van Chinh, N.; Tounsi, A. Static bending and buckling analysis of bi-directional functionally graded porous plates using an improved first-order shear deformation theory and FEM. Eur. J. Mech. A Solids 2022, 96, 104743. [Google Scholar] [CrossRef]
- Salari, E.; Sadough Vanini, S.A. Investigation of thermal preloading and porosity effects on the nonlocal nonlinear instability of FG nanobeams with geometrical imperfection. Eur. J. Mech. Solid 2021, 86, 104183. [Google Scholar] [CrossRef]
- Priyanka, R.; Twinkle, C.M.; Pitchaimani, J. Stability and dynamic behavior of porous FGM beam: Influence of graded porosity, graphene platelets, and axially varying loads. Eng. Comput. 2021, 38, 4347–4366. [Google Scholar] [CrossRef]
- Keleshteri, M.M.; Jelovica, J. Nonlinear vibration analysis of bidirectional porous beams. Eng. Comput. 2021, 38, 5033–5049. [Google Scholar] [CrossRef]
- Xiaohuan, L.; Tian, W.; Fang, L.; Zhiwen, Z. Computer simulation of the nonlinear static behavior of axially functionally graded microtube with porosity. Adv. Nano Res. 2021, 11, 437–451. [Google Scholar]
- Zarabimanesh, Y.; Roodgar Saffari, P.; Roudgar Saffari, P.; Refahati, N. Hygro-thermo-mechanical vibration of two vertically aligned single-walled boron nitride nanotubes conveying fluid. J. Vib. Control 2021, 28, 2101–2120. [Google Scholar] [CrossRef]
- Mechab, I.; Mechab, B.; Benaissa, S. Static and dynamic analysis of functionally graded plates using four-variable refined plate theory by the new function. Compos. Part B Eng. 2013, 45, 748–757. [Google Scholar] [CrossRef]
- Attia, M.A.; Shanab, R.A. Vibration characteristics of two-dimensional FGM nanobeams with couple stress and surface energy under general boundary conditions. Aerosp. Sci. Technol. 2021, 111, 106552. [Google Scholar] [CrossRef]
- Kumar, L.; Harsha, S.P. Effect of carbon nanotubes on CNT reinforced FGM nano plate under thermo mechanical loading. Proc. Technol. 2016, 23, 130–137. [Google Scholar] [CrossRef]



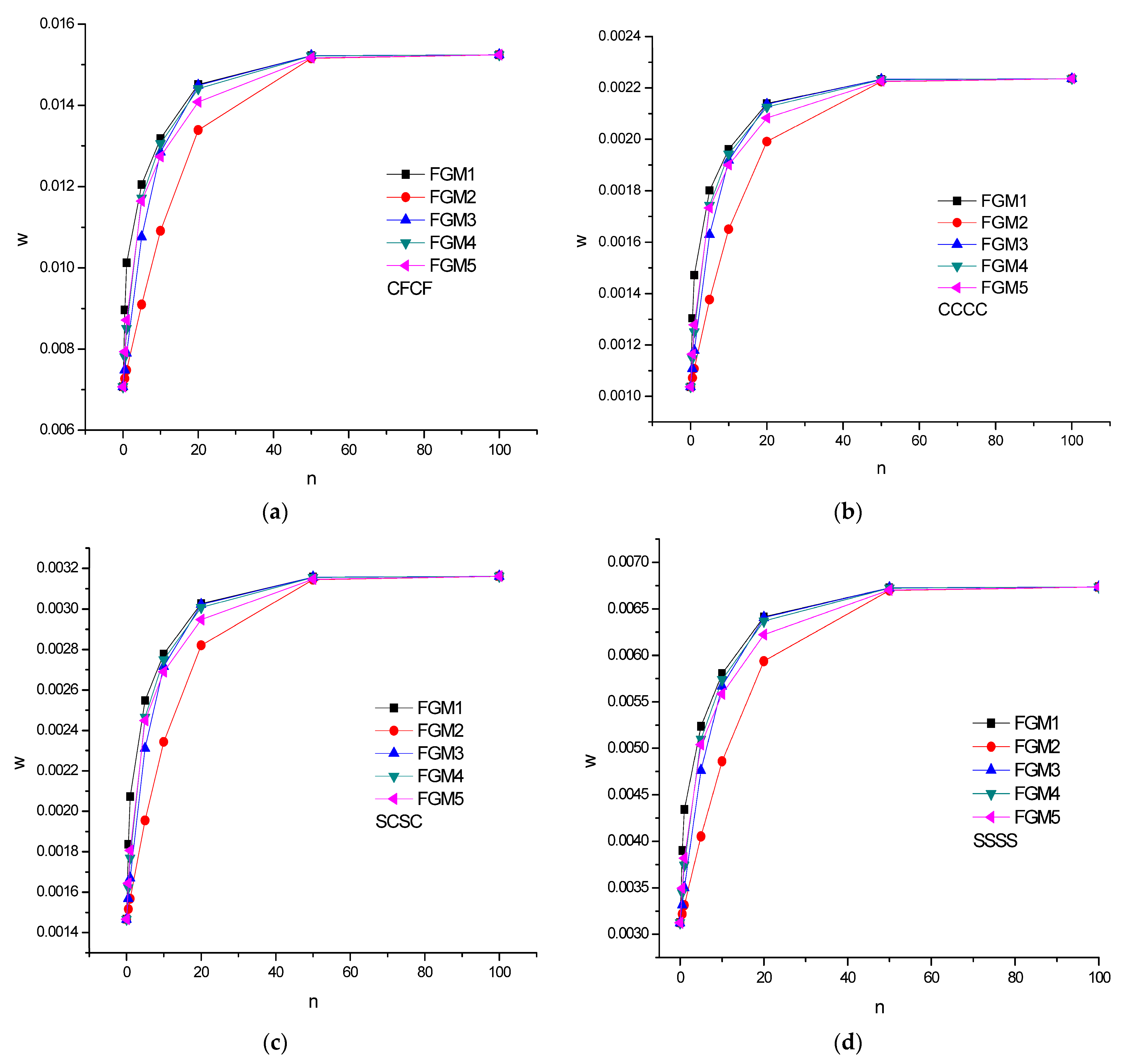





| Type | Parameter | Profile | Distribution |
|---|---|---|---|
| FGM 1 | a = 1, b = c = 0 | Classical | Metal and ceramic at the top and bottom, respectively |
| FGM 2 | a = 1, b = 1, c = 2 | Symmetric | Ceramic at the top and bottom |
| FGM 3 | a = 1, b = 1, c = 4 | Asymmetric | Ceramic at the top and bottom |
| FGM 4 | a = 1, b = 0.5, c = 2 | Asymmetric | Ceramic at the bottom/top and a combination of metal and ceramic at the top/bottom |
| FGM 5 | a = 0.8, b = 0.2, c = 3 | Asymmetric | Ceramic at the bottom/top and a combination of metal and ceramic at the top/bottom |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed Nabi Anwarbasha, G.T.; Chakrabarti, A.; Bahrami, A.; Venkatesan, V.; Vikram, A.S.V.; Subramanian, J.; Mahesh, V. Efficient Finite Element Approach to Four-Variable Power-Law Functionally Graded Plates. Buildings 2023, 13, 2577. https://doi.org/10.3390/buildings13102577
Mohammed Nabi Anwarbasha GT, Chakrabarti A, Bahrami A, Venkatesan V, Vikram ASV, Subramanian J, Mahesh V. Efficient Finite Element Approach to Four-Variable Power-Law Functionally Graded Plates. Buildings. 2023; 13(10):2577. https://doi.org/10.3390/buildings13102577
Chicago/Turabian StyleMohammed Nabi Anwarbasha, Gulshan Taj, Anupam Chakrabarti, Alireza Bahrami, Vasugi Venkatesan, Abdhullapuram Sachidhanandam Vijay Vikram, Jeyabharathi Subramanian, and Vutukuru Mahesh. 2023. "Efficient Finite Element Approach to Four-Variable Power-Law Functionally Graded Plates" Buildings 13, no. 10: 2577. https://doi.org/10.3390/buildings13102577








