Rolling Shear Strength of Cross Laminated Timber (CLT)—Testing, Evaluation, and Design
Abstract
:1. Introduction
1.1. General
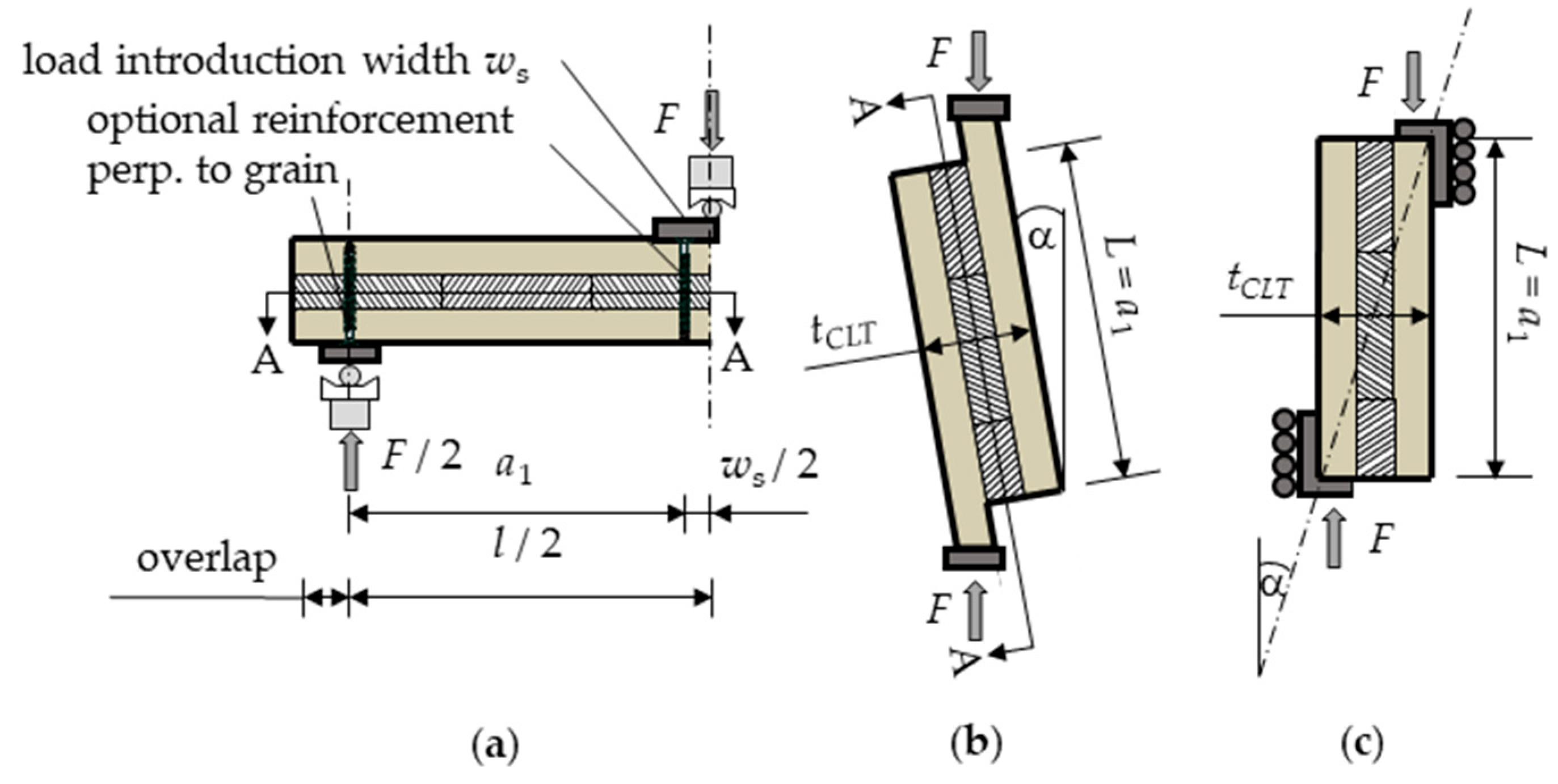
1.2. Common Setups for Testing the Rolling Shear Strength
1.3. Previous Research Regarding the Rolling Shear Strength
1.4. Scope
- a reliable and objective definition of the rolling shear failure and strength of CLT is not available;
- it is unclear whether different calculation methods anchored in various standards give comparable results;
- the comparability of test results gained by different test setups is uncertain;
- the interaction of rolling shear stresses with stresses perpendicular to the grain is not considered in current testing practice;
- although very likely, the dependency of the rolling shear strength on the specimen size was not yet considered nor quantified.
2. Material and Methods
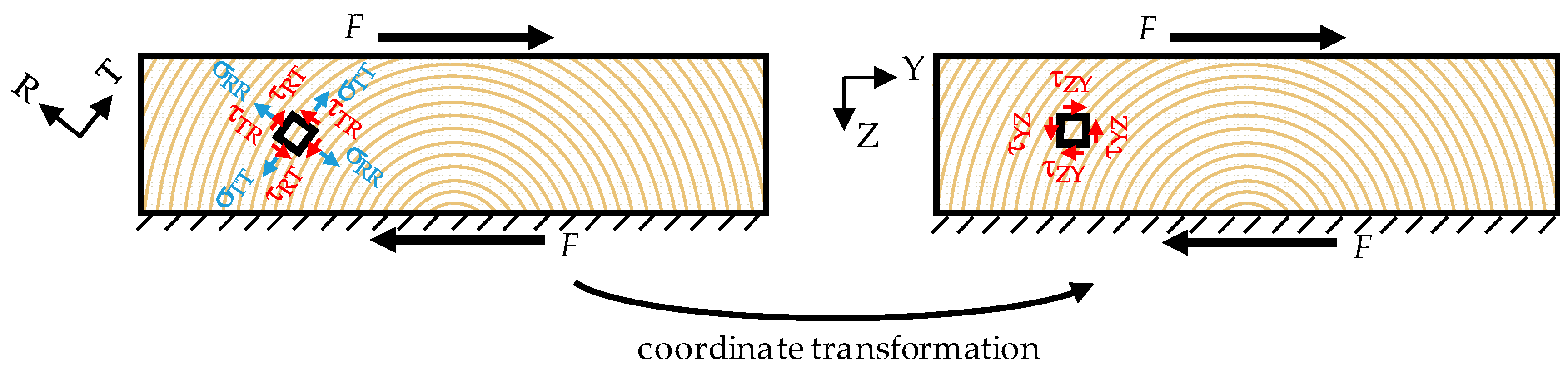
2.1. Proposal for the Definition of Rolling Shear Failure and Strength of CLT
- determination of the effective system stiffness Keff by linear regression of the force versus global displacement relationships between 10% and 40% of Fmax;
- intersection of the linear regression line at F = 0;
- construction of a second linear function with a slope of 0.9 Keff, which intersects with the linear regression line from Step 2 at F = 0;
- intersection of the linear function from Step 3 with the force–displacement curve of the tested specimen. Fr,0.9K, which is used to calculate fr, is then the maximum force, which occurred until this intersection.
2.2. Numerical Investigations
2.2.1. Comparison of Calculation Methods
2.2.2. Comparison of Test Setups
2.3. Experimental Tests
2.3.1. Parameter Variation
2.3.2. Tests of EAD Database
2.3.3. Interaction of Rolling Shear Stresses and Stresses Perpendicular to the Grain
3. Results and Discussion
3.1. Numerical Investigations
3.1.1. Comparison of Calculation Methods
3.1.2. Comparison of Test Setups
3.2. Experimental Program
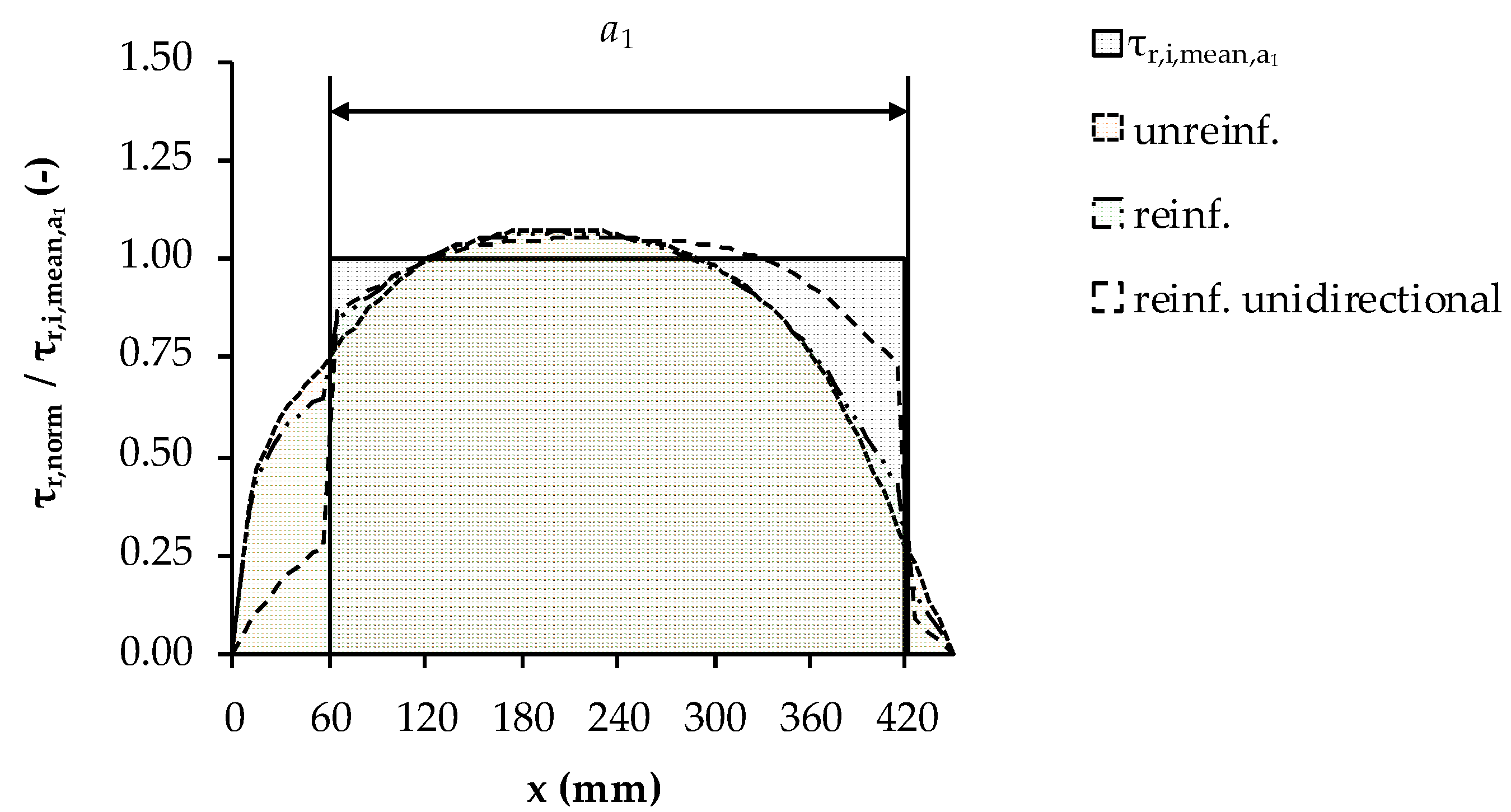
3.2.1. Reinforcement against Compression Perpendicular to the Grain
3.2.2. Specimen Width w
3.2.3. Test Configuration
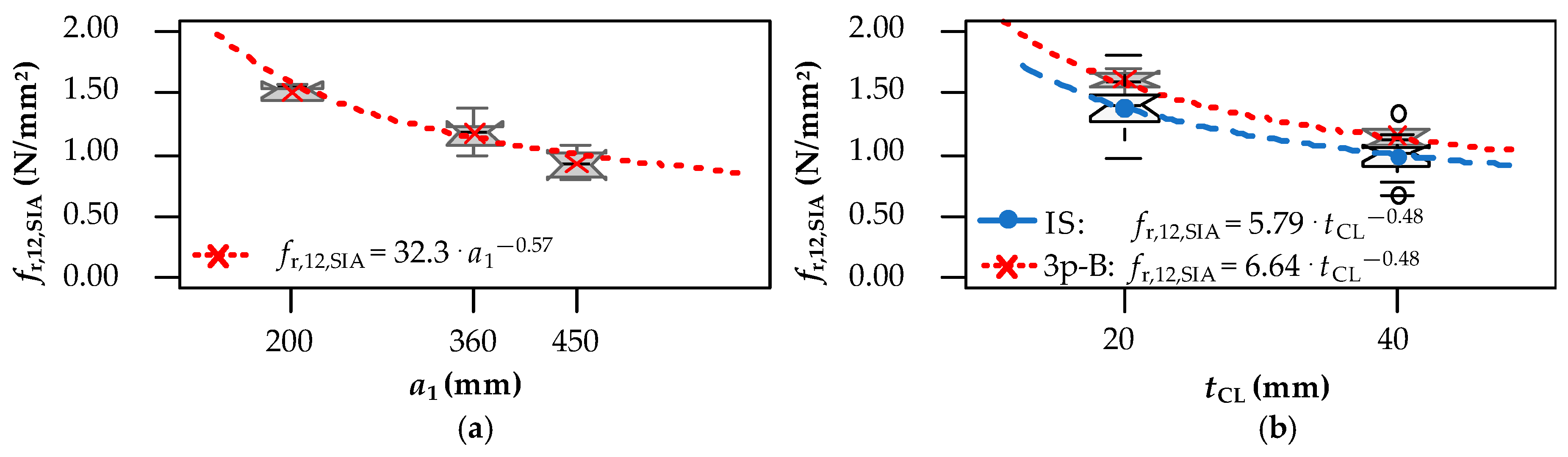
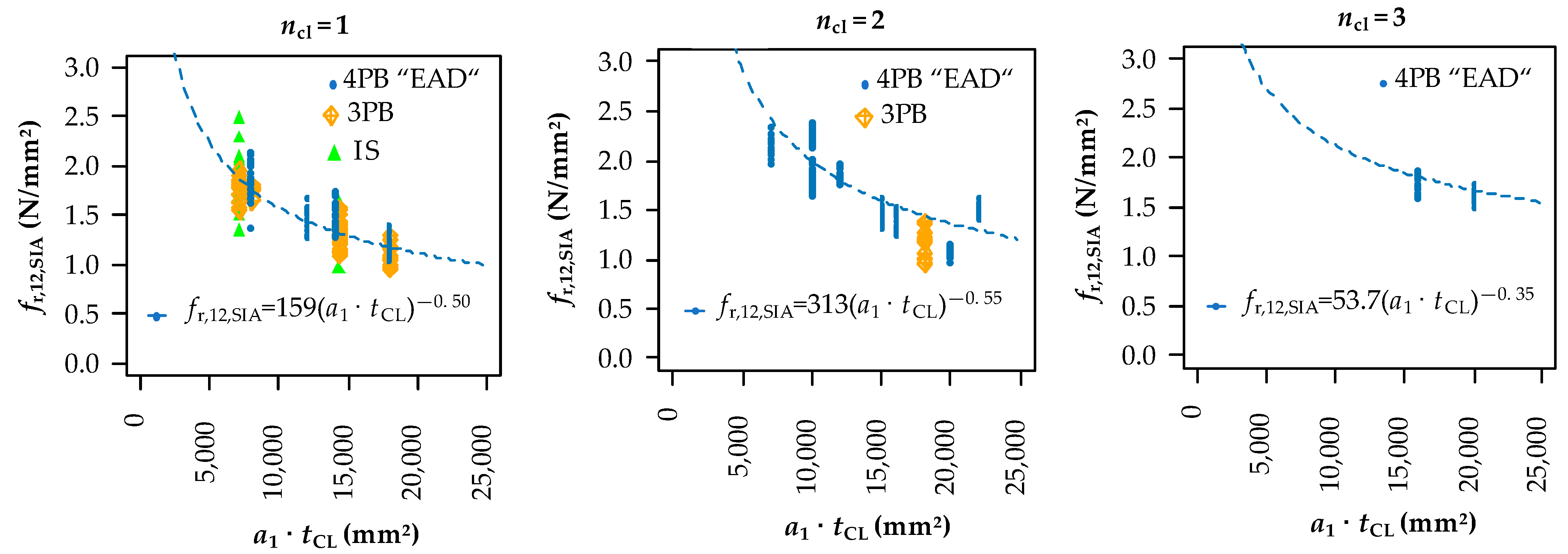
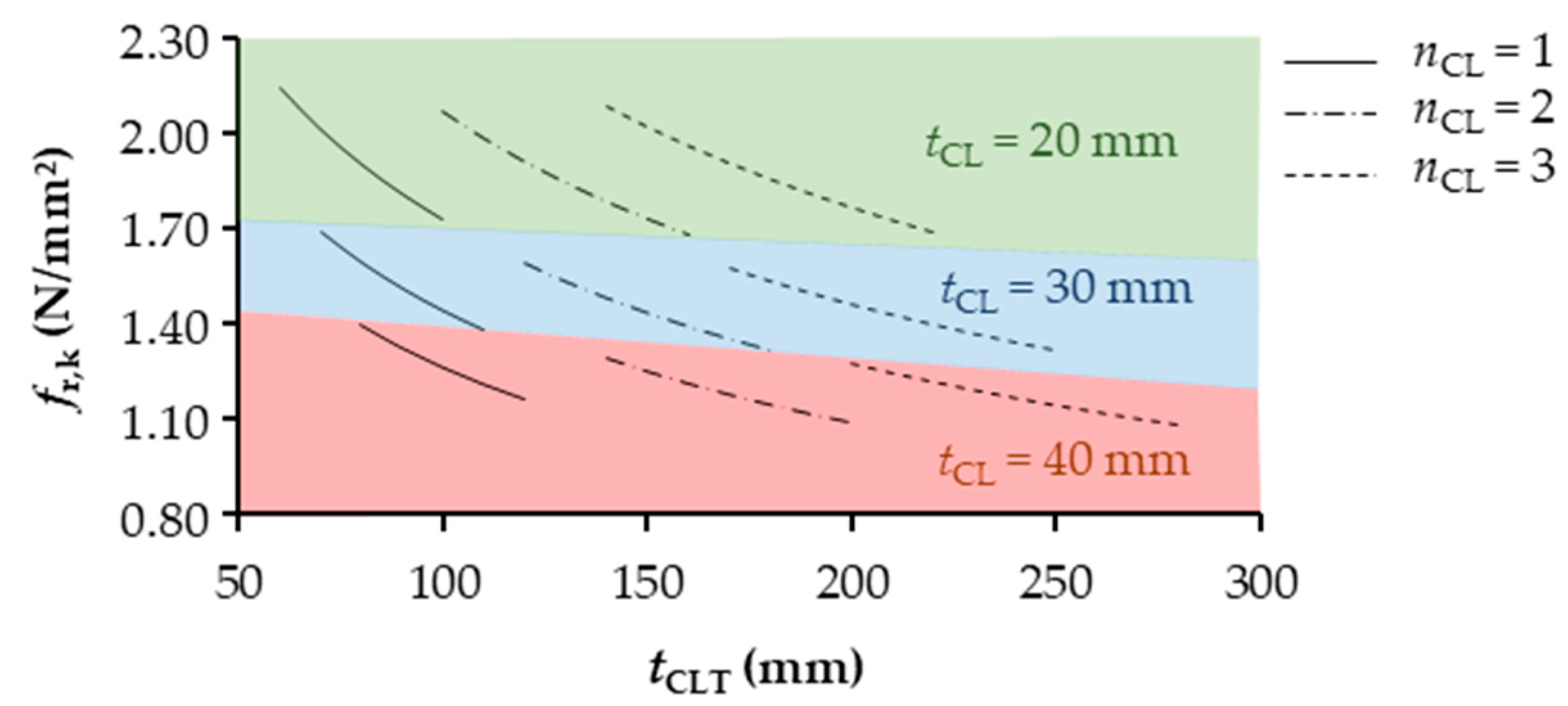
3.2.4. Shear Length a1 and the Thickness of the Cross Layer tCL
3.2.5. Layup
4. Rolling Shear Strength Model
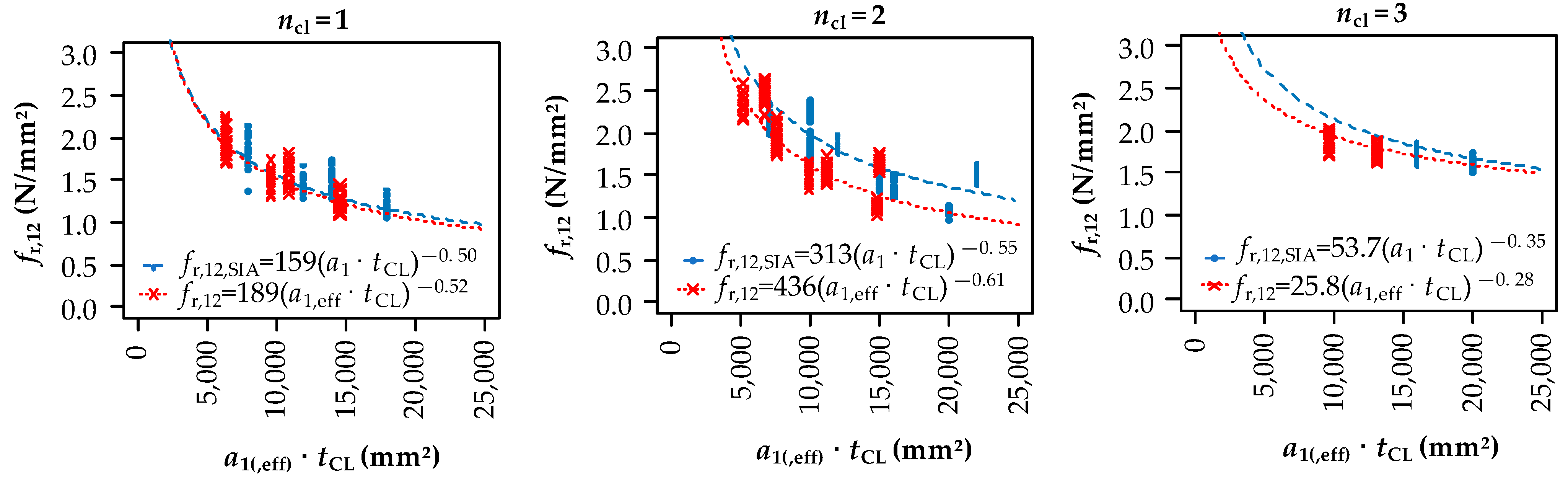
4.1. Derivation of a Basic Model to Predict the Test Data
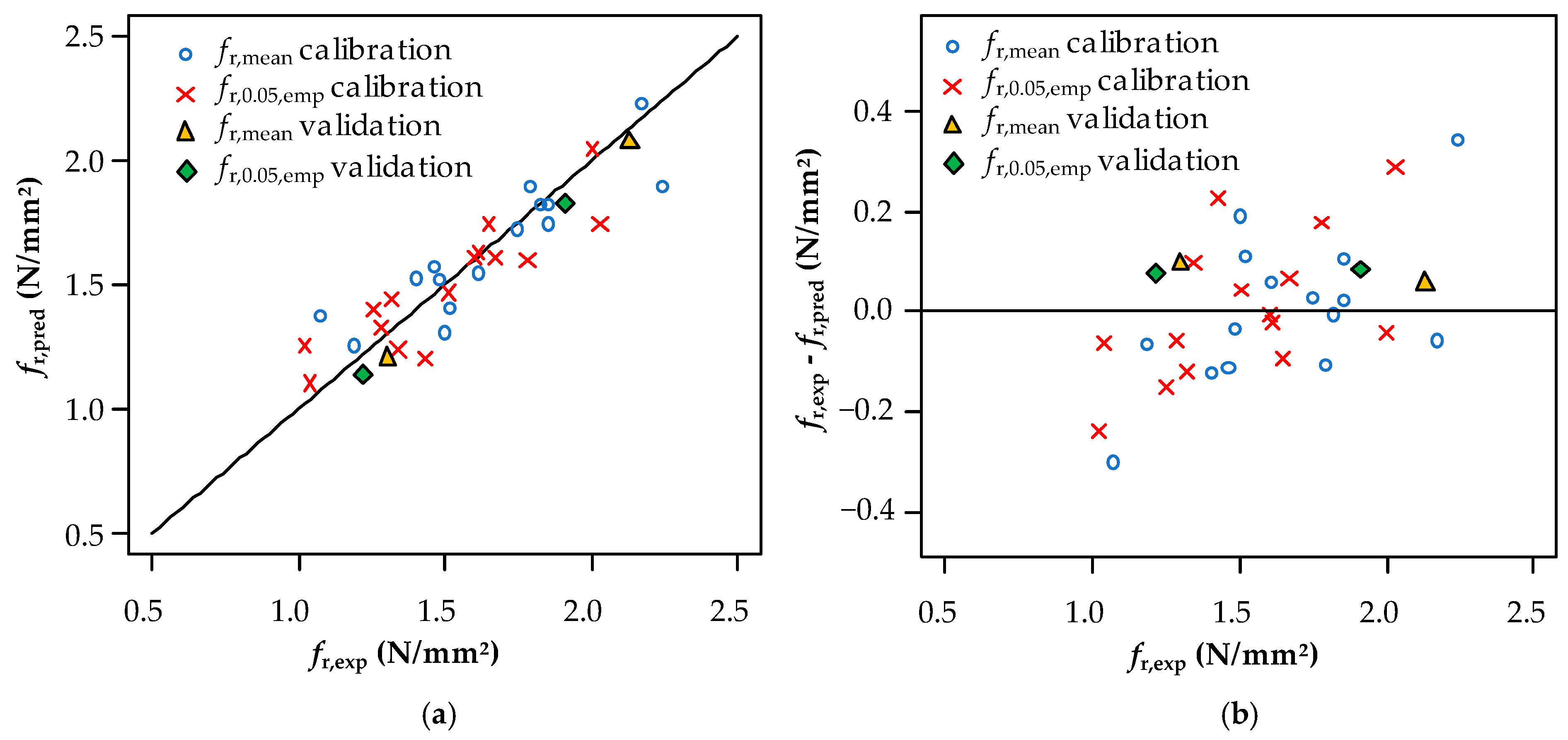
4.2. Model Verification and Validation
4.3. Proposal for a Normative Rolling Shear Strength Model
5. Summary and Conclusions
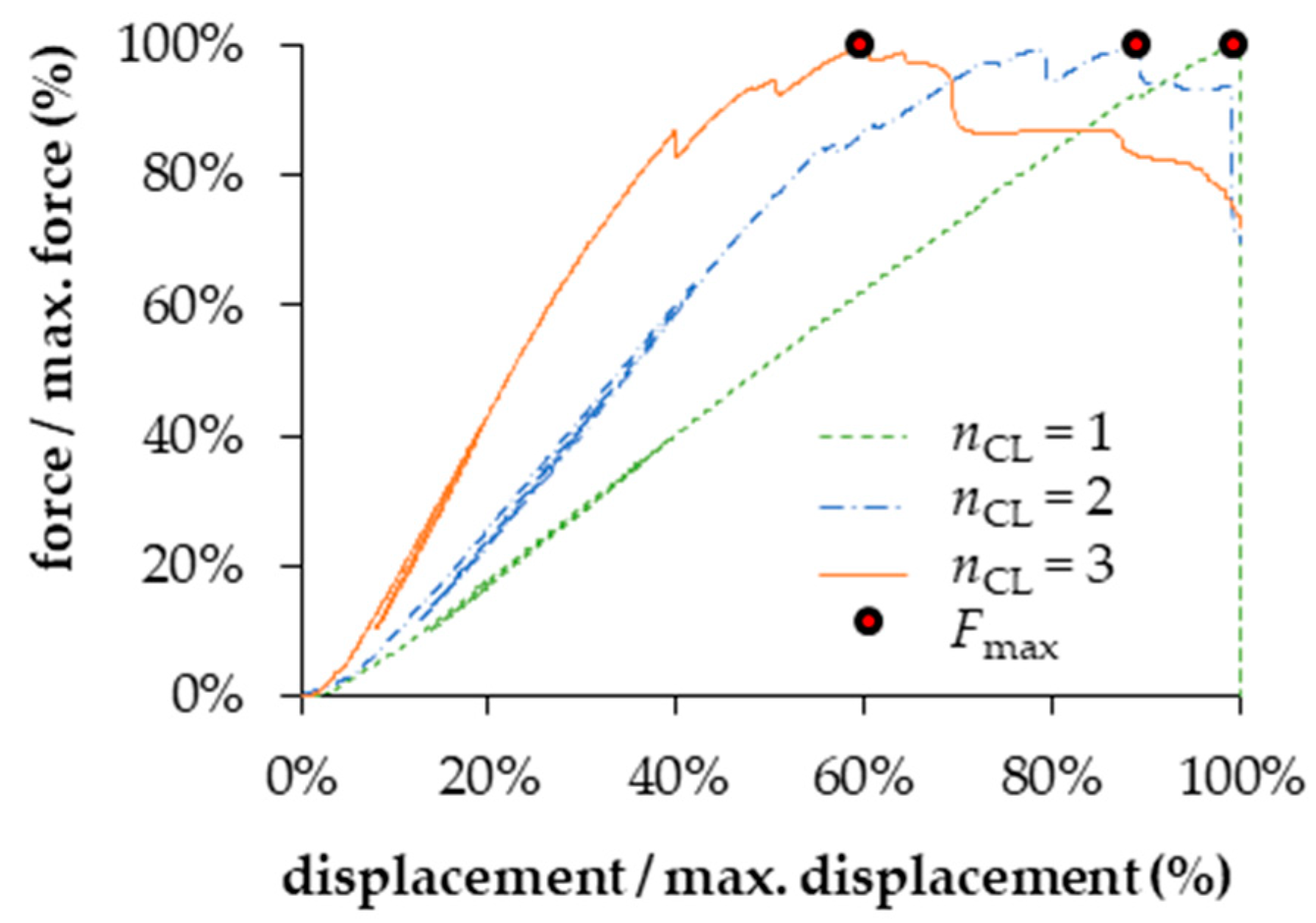
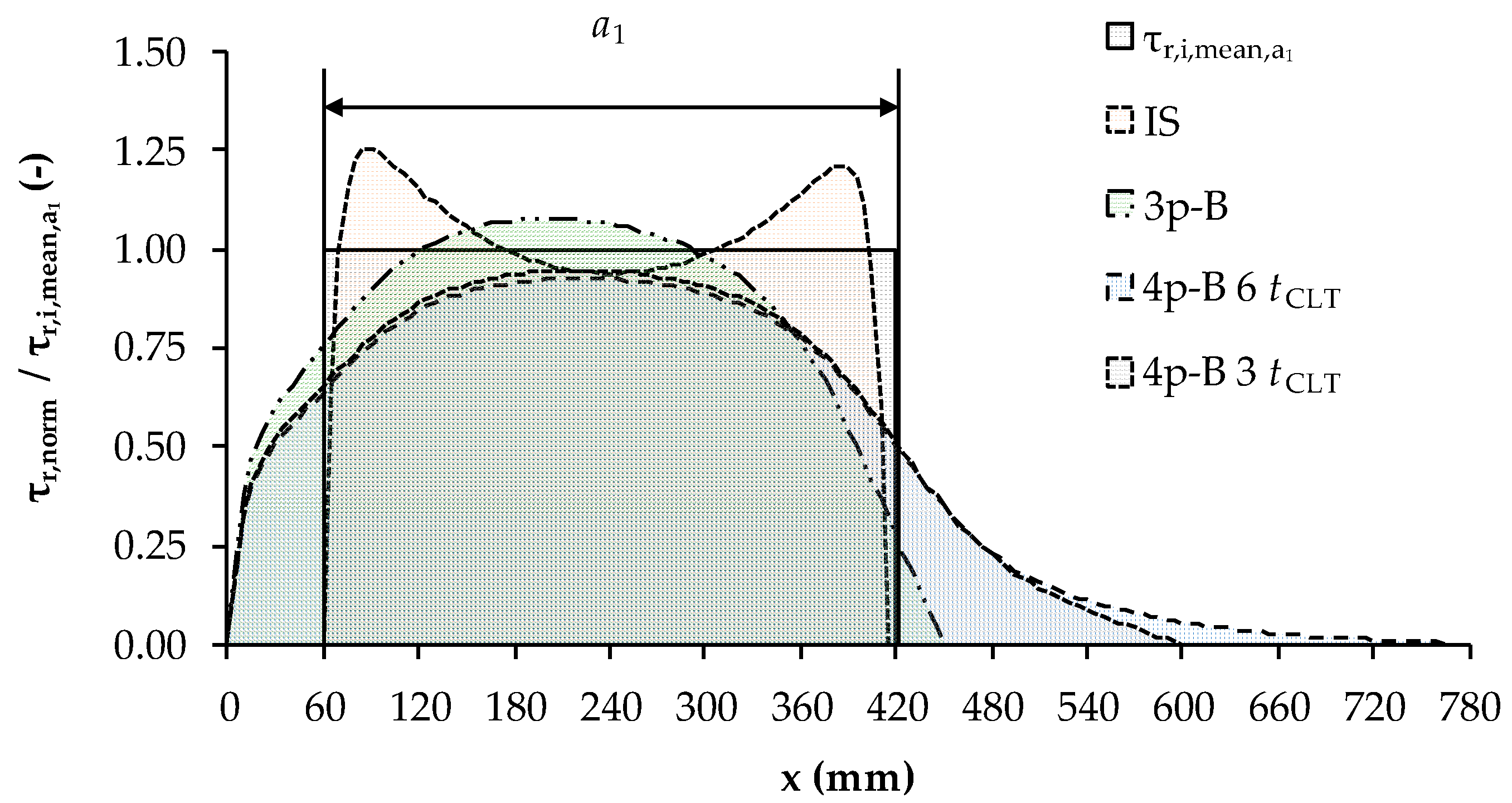
- there is an effect of the used test setup on the rolling shear strength. This is (beside a possible difference in tested volumes) attributed to the different resulting rolling shear stress distributions. Although material use is higher for out-of-plane bending tests as compared to shear tests, it is recommended to use 4PB tests according to EN 16351 [3] with a1 = 3 tCLT (cf. Figure 19), as this setup best approximates the shear stress distribution in common practical and relevant exposure scenarios;
- the comparison of the maximum resulting rolling shear stresses from different test setups showed differences of up to 35%, as illustrated in Table 4. This table provides a set of configuration factors to convert the results from different test setups. It would be worth expanding this set of factors also for other test setups;
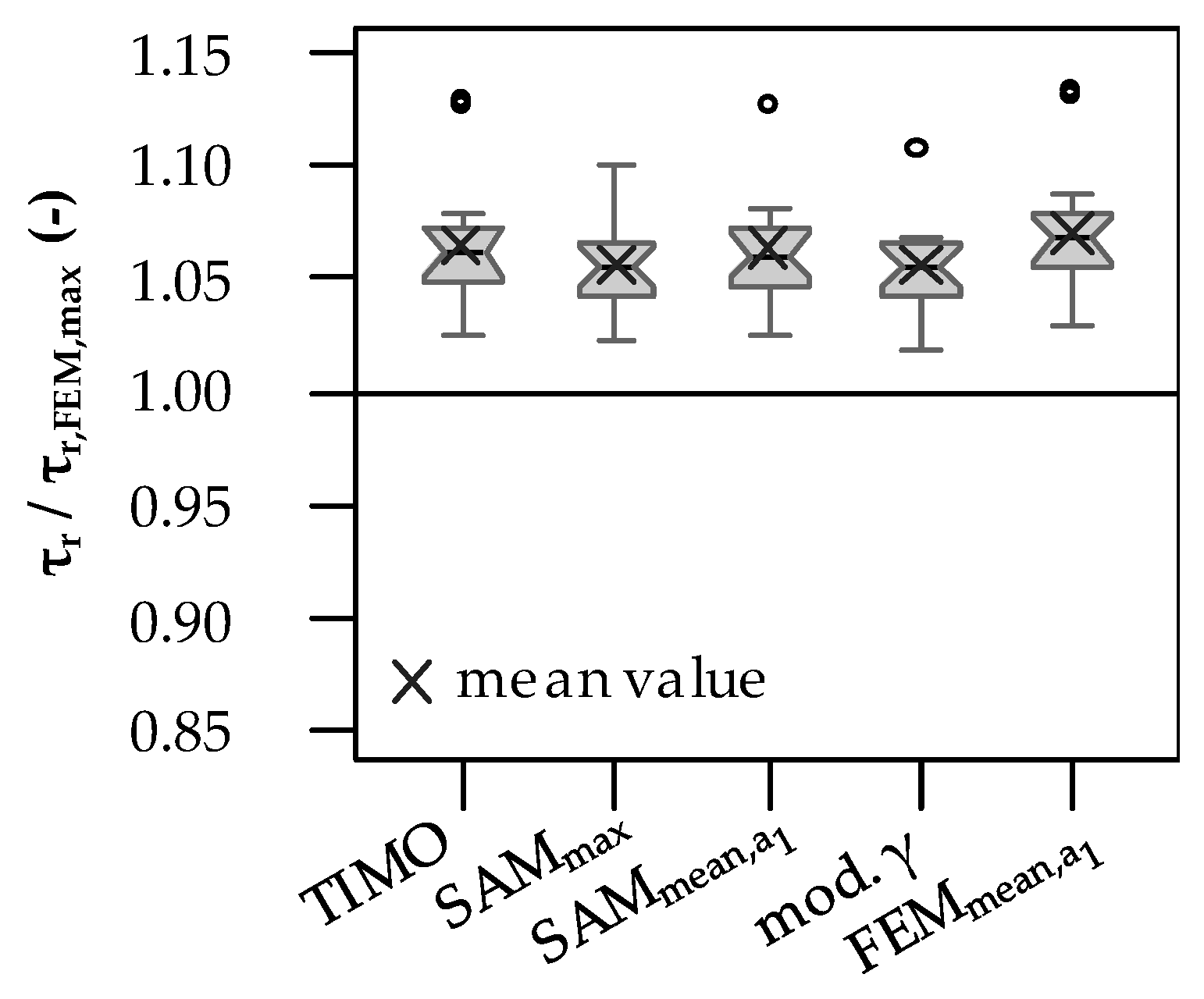
- between the used stress calculation methods, no significant differences were found for the rolling shear stresses averaged over the shear length. Due to its simplicity, the Timoshenko beam theory is recommended;
- the rolling shear strength is affected by the stresses perpendicular to the grain. As a consequence, the results of rolling shear from out-of-plane bending tests have to be corrected for this influence. It is proposed to do this either directly, with the method outlined in Section 2.3.3, or indirectly, by means of Equations (5) and (8);
- within the tested range, the rolling shear strength was not affected by the width w of the CLT slab, i.e., the number of lamellas in longitudinal layers. Consequently, a reduction of w to max {2 wb,LL; 300} mm is possible and recommended;
- the application of reinforcements against the stresses perpendicular to the grain at the load introductions and supports is not suited to provide a more homogeneous rolling shear stress field, as it was found for testing longitudinal shear in solid timber and structural timber products with unidirectional layups;
- for CLT without gaps or relief grooves, the parameters a1, tCL and nCL have been identified as the main influencing parameters on the rolling shear strength subjected to out-of-plane bending. The frequently discussed parameter wb/tb with a simultaneous occurrence of gaps and/or relief grooves could not be evaluated due to the lack of an adequate parameter range. However, this parameter could still be implemented in the form of reduction factors. This strength model could also be adapted for cross layers made of other timber products (e.g., veneers, split boards, recycled CLT, etc.) and different wood species (especially hardwood);
- a strength model under consideration of a1, tCL, and nCL was derived, based on approximately 300 4PB test results, and successfully validated by means of two recently and additionally conducted test series. For design practice, a simplified model was presented with Equation (9) based on the evaluation of the strength model for the most commonly used layups;
- the presented model is seen as a valuable candidate to substitute the current practice of extensive testing. According to the current EAD procedure, only for rolling shear is an amount of 25% of, in total, 40–160 m3 CLT needed to be destroyed to obtain an updated or new ETA. In contrast, the current regulations for glulam clearly point out the potential for significantly reduced test efforts by means of a set of load bearing models based on the properties of the base material, cf. EN 14080:2013 [41]. Using a similar approach to introduce a strength class system for CLT, combined with the harmonization of EN 16351 [3], would be a major step forward. The herein aimed load bearing model for the rolling shear of CLT is seen as an important contribution to this desirable evolution.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ehrhart, T.; Brandner, R. Test configurations for determining rolling shear properties with focus on cross laminated timber: A critical review. In Properties, Testing and Design of Cross Laminated Timber: COST Action FP1402/WG 2; Brandner, R., Tomasi, R., Moosbrugger, T., Serrano, E., Dietsch, P., Eds.; Shaker: Aachen, Germany, 2018; pp. 151–169. ISBN 978-3-8440-6143-7. [Google Scholar]
- EAD 130005-00-0304; Solid Wood Slab Element to Be Used as a Structural Element in Buildings. EOTA: Brussels, Belgium, 2015.
- EN 16351; Timber Structures—Cross Laminated Timber—Requirements. CEN: Brussels, Belgium, 2021.
- Aicher, S.; Reinhardt, H.W. Einfluss der Bauteilgrößer in der linearen und nichtlinearen (Holz-) Bruchmechanik. Holz Als Roh-Und Werkst. 1993, 51, 215–220. [Google Scholar] [CrossRef]
- Brandner, R.; Bogensperger, T.; Schickhofer, G. In-plane shear strength of cross laminated timber (CLT): Test configuration, quantification and influencing parameters. In Proceedings of the CIB-W18 Proceedings, Meeting 46, Vancouver, BC, Canada, 26–29 August 2013; Görlacher, R., Ed.; Timber Scientific Publishing KIT Holzbau und Baukonstruktionen: Karlsruhe, Germany, 2013; pp. 227–242. [Google Scholar]
- Brandner, R.; Gatternig, W.; Schickhofer, G. Determination of shear strength of structural and glued laminated timber. In Proceedings of the CIB-W18 Proceedings, Meeting 45, Växjö, Sweden, 27–30 August 2012; Görlacher, R., Ed.; Ingenieurholzbau und Baukonstruktionen: Karlsruhe, Germany, 2012. [Google Scholar]
- Brandner, R.; Jantscher, L. Out-of-plane tensile properties of cross laminated timber (CLT). Buildings 2022, 12, 135. [Google Scholar] [CrossRef]
- Colling, F. Influence of volume and stress distribution on the shear strength and tensile strength perpendicular to grain. In Proceedings of the CIB-W18 Proceedings Volume II, Meeting 19, Florence, Italy, 1–5 September 1986. [Google Scholar]
- Kreuzinger, H. Platten, Scheiben und Schalen: Ein Berechnungsmodell für gängige Statikprogramme. Bau. Mit Holz 1999, 1, 34–39. (In German) [Google Scholar]
- Mestek, P.; Kreuzinger, H.; Winter, S. Design concept for CLT—Reinforced with selftapping screws. In Proceedings of the CIB-W18 Proceedings, Meeting 44, Alghero, Italy, 29 August–1 September 2011; Görlacher, R., Ed.; Ingenieurholzbau und Baukonstruktionen: Karlsruhe, Germany, 2011. [Google Scholar]
- Mestek, P. Punktgestützte Flächentragwerke aus Brettsperrholz (BSP): Schubbemessung unter Berücksichtigung von Schubverstärkungen. Doctoral Thesis, Technische Universität München, München, Germany, 2011. (In German). [Google Scholar]
- Ehrhart, T.; Brandner, R. Rolling shear: Test configurations and properties of some European soft- and hardwood species. Eng. Struct. 2018, 172, 554–572. [Google Scholar] [CrossRef]
- EN 408; Structural Timber—Structural Timber and Glued Laminated Timber—Determination of Some Physical and Mechanical Properties. CEN: Brussels, Belgium, 2012.
- EN 789; Timber Structures—Test Methods—Determination of Mechanical Properties of Wood Based Panels. CEN: Brussels, Belgium, 2005.
- Aicher, S.; Hirsch, M.; Christian, Z. Hybrid cross laminated timber plates with beech wood cross layers. Constr. Build. Mater. 2016, 124, 1007–1018. [Google Scholar] [CrossRef]
- Silly, G. Auslotung und Aufbereitung der Möglichkeiten zu Einer Ressourcenschonenderen Produktion von BSP-Elementen–Rollschubeigenschaften; TU Graz University of Technology: Graz, Austria, 2013. (In German) [Google Scholar]
- CEN/TC 250/SC 5 T1. prEN 1995-1-1; SC5.T1 Working Draft of Design of Cross Laminated Timber in a Revised Eurocode 5-1-1. CEN: Brussels, Belgium, 2018.
- CEN/TC 250/SC 5 N 1488. prEN 1995-1-1; Consolidated Draft of prEN 1995-1-1. CEN: Brussels, Belgium, 2021.
- Li, M.; Dong, W.; Lim, H. Influence of lamination aspect ratios and test methods on rolling shear strength evaluation of cross laminated timber. J. Mater. Civ. Eng. 2019, 31, 4019310. [Google Scholar] [CrossRef]
- Nero, R.; Christopher, P.; Ngo, T. Investigation of rolling shear properties of cross laminated timber (CLT) and comparison of experimental approaches. Constr. Build. Mater. 2022, 316, 125897. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, J.; Dong, W.; Yao, Y.; Gong, M. Influence of technical characteristics on the rolling shear properties of cross laminated timber by modified planar shear tests. Maderas Cienc. Tecnol. 2018, 20, 469–478. [Google Scholar] [CrossRef]
- Gardner, C.; Davids, W.G.; Lopez-Anido, R.; Herzog, B.; Edgar, R.; Nagy, E.; Berube, K.; Shaler, S. The effect of edge gaps on shear strength and rolling shear modulus of cross laminated timber panels. Constr. Build. Mater. 2020, 259, 119710. [Google Scholar] [CrossRef]
- Zare, A. Influence of Cross layer Lamella Edge-Bonding/Adhesive-Type on Rolling Shear Properties of Different Cross laminated-Timber Lay-Ups. Master Thesis, University of Applied Sciences, Bern, Switzerland, 2022. [Google Scholar]
- O’Ceallaigh, C.; Sikora, K.; Harte, A. The influence of panel lay-up on the characteristic bending and rolling shear strength of CLT. Buildings 2018, 8, 114. [Google Scholar] [CrossRef]
- Akter, S.T.; Bader, T.K. Experimental assessment of failure criteria for the interaction of normal stress perpendicular to the grain with rolling shear stress in Norway spruce clear wood. Holz Als Roh-Und Werkst. 2020, 78, 1105–1123. [Google Scholar] [CrossRef]
- Hill, R. The Mathematical Theory of Plasticity, 1st ed.; Oxford University Press: Oxford, UK, 1950; ISBN 99-0013542-3. [Google Scholar]
- Hoffman, O. The brittle strength of orthotropic materials. J. Compos. Mater. 1967, 1, 200–206. [Google Scholar] [CrossRef]
- Steiger, R.; Gehri, E. Interaction of shear stresses and stresses perpendicular to the grain. In Proceedings of the CIB-W18 Proceedings, Meeting 44, Alghero, Italy, 29 August–1 September 2011; Görlacher, R., Ed.; Ingenieurholzbau und Baukonstruktionen: Karlsruhe, Germany, 2011. [Google Scholar]
- SIA 265; 2021 Timber Structures: Timber Structures. SIA: Zürich, Switzerland, 2021.
- Akter, S.T.; Binder, E.; Bader, T.K. Moisture and short-term time-dependent behavior of Norway spruce clear wood under compression perpendicular to the grain and rolling shear. Wood Mater. Sci. Eng. 2022, 18, 580–593. [Google Scholar] [CrossRef]
- Glasner, D.; Ringhofer, A.; Brandner, R.; Schickhofer, G. Contribution to the testing, evaluation and design of cross laminated timber (CLT) in respect to rolling shear. In Proceedings of the International Network on Timber Engineering Research, Meeting 55. INTER, Bad Aibling, Germany, 22–25 August 2022; Görlacher, R., Ed.; Timber Scientific Publishing KIT Holzbau und Baukonstruktionen: Karlsruhe, Germany, 2022; pp. 455–473. [Google Scholar]
- EN 338; Structural Timber—Strength Classes. CEN: Brussels, Belgium, 2016; 79.040 (EN 338:2016-06-01).
- Dlubal Software GmbH. RFEM, version 5.17; Dlubal Software GmbH: Tiefenbach, Germany, 2020. [Google Scholar]
- Dvorkin, E.N.; Bathe, K.-N. A continuum mechanics based four-node shell element for general non-linear analysis. Eng. Comput. 1984, 1, 77–88. [Google Scholar] [CrossRef]
- Fink, G.; Kohler, J.; Brandner, R. Application of European design principles to cross laminated timber. Eng. Struct. 2018, 171, 934–943. [Google Scholar] [CrossRef]
- Brandner, R. Cross laminated timber (CLT) in compression perpendicular to plane: Testing, properties, design and recommendations for harmonizing design provisions for structural timber products. Eng. Struct. 2018, 171, 944–960. [Google Scholar] [CrossRef]
- EN 14358; Timber Structures—Calculation and Verification of Characteristic Values. CEN: Brussels, Belgium, 2016.
- Bažant, Z.P. Size effect in blunt fracture: Concrete, rock, metal. J. Eng. Mech. 1984, 110, 518–535. [Google Scholar] [CrossRef]
- Weibull, W. A Statistical Theory of the Strength of Materials; Generalstabens Litografiska Anstalts Förlag: Stockholm, Sweden, 1939; ISBN 991-428078-1. [Google Scholar]
- ETA-14/0349 CLT – Cross Laminated Timber Massive plattenförmige Holzbauelemente für tragende Bauteile in Bauwerken; Stora Enso Wood Products OY Ltd, OIB: Vienna, Austria, 2020.
- EN 14080; Timber Structures—Glued Laminated Timber and Glued Solid Timber—Requirements. CEN: Brussels, Belgium, 2013.

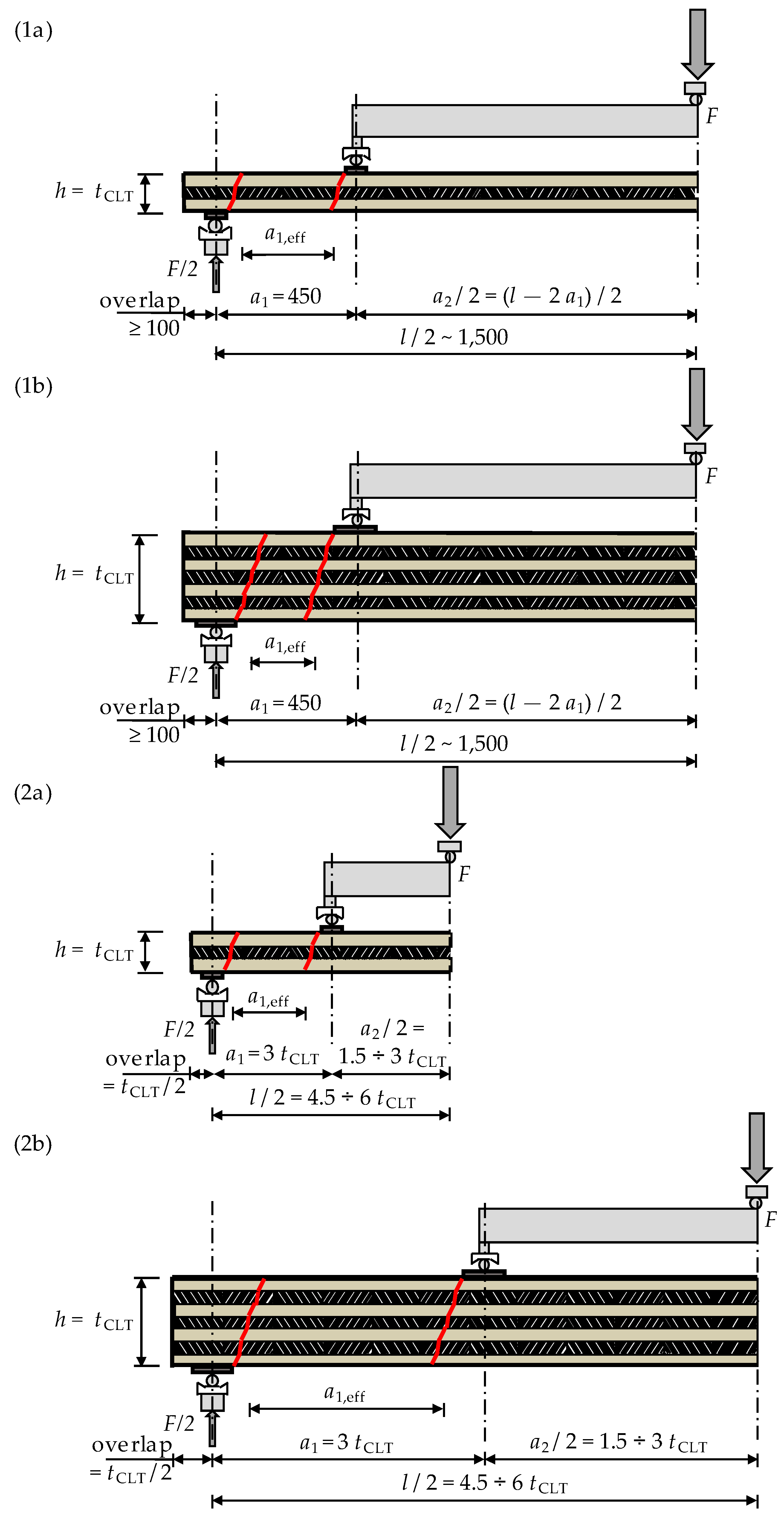





| Geometric Property | Variable | EAD | EN 16351 |
|---|---|---|---|
| Span | l | ~3000 | 12h + (9h *) |
| Measuring length for the modulus of elasticity (MoE) | l1 | 5h | 5h + (– *) |
| Distance between load application and support | a1 | 450 | 3h |
| Distance between load applications | a2 | l − 2a1 | 6h + (3h *) |
| Nominal width | w | ~800 | ≥600 |
| Nominal slab thickness | h | tCLT | tCLT |
| Overlap at supports | – | ≥100 | h/2 |
| Test Series No. | Manufacturer No. | Layup | tCLT | a1 | a2 | Overlap | l | ws |
|---|---|---|---|---|---|---|---|---|
| (-) | (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | |
| 01 | 01 | 40-40-40-40-40-40-40 | 280 | 500 | 2000 | 100 | 3000 | 100 |
| 02 | 01 | 40-40-20-20-20-40-40 | 220 | 500 | 2000 | 100 | 3000 | 100 |
| 03 | 01 | 40-20-40-20-40 | 160 | 350 | 2200 | 150 | 2900 | 50 |
| 04 | 01 | 40-20-40 | 100 | 400 | 2200 | 100 | 3000 | 50 |
| 05 | 02 | 30-40-30 | 100 | 350 | 2300 | 100 | 3000 | 50 |
| 06 | 02 | 40-30-40-30-40 | 180 | 400 | 2200 | 100 | 3000 | 100 |
| 07 | 02 | 30-40-30-40-30-40-30 | 240 | 400 | 2200 | 100 | 3000 | 100 |
| 08 | 03 | 40-20-40 | 100 | 400 | 2200 | 200 | 3000 | 45 |
| 09 | 03 | 40-30-40-30-40 | 180 | 500 | 2000 | 200 | 3000 | 80 |
| 10 | 03 | 40-40-40-40-40-40-40 | 280 | 550 | 1900 | 200 | 3000 | 100 |
| 11 | 04 | 30-30-30 | 90 | 400 | 2200 | 100 | 3000 | 50 |
| 12 | 04 | 40-20-40-20-40 | 160 | 500 | 2000 | 100 | 3000 | 80 |
| 13 | 04 | 40-40-40 | 120 | 450 | 2100 | 100 | 3000 | 50 |
| 14 | 04 | 40-40-40-40-40 | 200 | 500 | 2000 | 100 | 3000 | 80 |
| 15 | 05 | 40-40-40-40-40 | 200 | 400 | 1800 | 300 | 2600 | 100 |
| Test Series | Layup | tCLT | w | a1 | Reinforced Perp. to Grain | Overlap | l | ws | |
| (-) | (mm) | (mm) | (mm) | (mm) | (-) | (mm) | (mm) | (mm) | |
| 3PB | PS-100-300-360-V | 40-20-40 | 100 | 300 | 360 | yes | 60 | 780 | 60 |
| PS-200-300-450-V | 40-40-40-40-40 | 200 | 300 | 450 | yes | 100 | 1000 | 60 | |
| PS-120-300-360 | 40-40-40 | 120 | 300 | 360 | no | 60 | 780 | 60 | |
| PS-120-800-360 | 40-40-40 | 120 | 800 | 360 | no | 60 | 780 | 60 | |
| PS-120-500-200 | 40-40-40 | 120 | 500 | 200 | no | 60 | 460 | 60 | |
| PS-120-500-360 | 40-40-40 | 120 | 500 | 360 | no | 60 | 780 | 60 | |
| PS-120-500-450 | 40-40-40 | 120 | 800 | 450 | no | 60 | 960 | 60 | |
| PS-100-500-360-V | 40-20-40 | 100 | 500 | 360 | yes | 60 | 780 | 60 | |
| PS-120-500-360-V | 40-40-40 | 120 | 500 | 360 | yes | 60 | 780 | 60 | |
| PS-120-500-450-V | 40-40-40 | 120 | 500 | 450 | yes | 60 | 960 | 60 | |
| PS-200-500-450-V | 40-40-40-40-40 | 200 | 500 | 450 | yes | 100 | 1000 | 60 | |
| Test Series | Layup | tCLT | w | a1 | α | - | l | - | |
| (-) | (mm) | (mm) | (mm) | (mm) | (°) | (-) | (mm) | (-) | |
| IS | IS-100-500-360 | 40-20-40 | 100 | 500 | 360 | 10 | - | 360 | - |
| IS-120-500-360 | 40-40-40 | 120 | 500 | 360 | 10 | - | 360 | - |
| max(τr,norm,m)/ max(τr,norm,n) | m | τr,i,mean,a1 | IS | 3PB | 4PB 6 tCLT | 4PB 3 tCLT | |
|---|---|---|---|---|---|---|---|
| n | |||||||
| τr,i,mean,a1 | 1.00 | 1.25 | 1.08 | 0.93 | 0.95 | ||
| IS | 0.80 | 1.00 | 0.86 | 0.74 | 0.76 | ||
| 3PB | 0.93 | 1.17 | 1.00 | 0.86 | 0.88 | ||
| 4PB|a2 = 6 tCLT | 1.08 | 1.35 | 1.16 | 1.00 | 1.02 | ||
| 4PB|a2 = 3 tCLT | 1.06 | 1.32 | 1.14 | 0.98 | 1.00 | ||
| 3PB | IS | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Test Series | PS-100-300-360-V | PS-200-300-450-V | PS-120-300-360 | PS-120-800-360 | PS-120-500-200 | PS-120-500-360 | PS-120-500-450 | PS-100-500-360-V | PS-120-500-360-V | PS-120-500-450-V | PS-200-500-450-V | IS-100-500-360 | IS-120-500-360 | |
| No. of Specimens | 6 | 6 | 6 | 6 | 6(5) 1 | 6 | 6 | 6 | 6 | 6 | 6 | 15 | 12 | |
| u (%) | Mean | 10.9 | 10.9 | 11.0 | 10.9 | 11.0 | 10.9 | 10.3 | 10.9 | 11.0 | 10.6 | 11.2 | 11.3 | 11.5 |
| CoV (%) | 2.52 | 1.98 | 5.42 | 1.71 | 4.75 | 3.41 | 2.62 | 5.13 | 3.74 | 2.52 | 2.18 | 1.64 | 2.93 | |
| ρ12 (kg/m3) | Mean | 478 | 434 | 433 | 445 | 459 | 438 | 446 | 478 | 452 | 446 | 454 | 460 | 432 |
| Median | 478 | 432 | 432 | 447 | 461 | 432 | 446 | 482 | 456 | 452 | 457 | 460 | 433 | |
| CoV (%) | 3.60 | 2.98 | 1.63 | 2.83 | 1.14 | 3.20 | 1.42 | 4.01 | 2.46 | 5.12 | 2.67 | 1.58 | 3.39 | |
| fr,12 (N/mm2) | Mean | 1.53 | 1.02 | 1.21 | 1.09 | 1.72 | 1.22 | 0.96 | 1.64 | 1.18 | 0.99 | 1.09 | 1.43 | 1.00 |
| CoV (%) | 8.98 | 10.3 | 9.61 | 11.9 | 4.37 | 12.1 | 12.7 | 4.96 | 10.1 | 8.60 | 11.8 | 16.1 | 15.4 | |
| LN0.05 2 | 1.32 | 0.85 | 1.03 | 0.89 | 1.60 | 0.99 | 0.77 | 1.51 | 1.00 | 0.86 | 0.89 | 1.08 | 0.75 | |
| charEN14358 3 | 1.24 | 0.79 | 0.97 | 0.82 | 1.52 | 0.91 | 0.71 | 1.46 | 0.94 | 0.81 | 0.82 | 1.02 | 0.70 | |
| fr,12,SIA (N/mm2) | Mean | 1.50 | 1.00 | 1.16 | 1.04 | 1.51 | 1.17 | 0.93 | 1.60 | 1.16 | 0.98 | 1.07 | 1.37 | 0.98 |
| CoV (%) | 8.81 | 10.1 | 9.22 | 11.5 | 3.92 | 11.7 | 12.4 | 4.86 | 9.90 | 8.49 | 11.6 | 15.7 | 15.3 | |
| LN0.05 2 | 1.29 | 0.83 | 0.99 | 0.86 | 1.41 | 0.96 | 0.75 | 1.48 | 0.99 | 0.85 | 0.87 | 10.4 | 0.74 | |
| charEN14358 3 | 1.22 | 0.78 | 0.93 | 0.80 | 1.33 | 0.88 | 0.69 | 1.42 | 0.92 | 0.80 | 0.80 | 0.98 | 0.69 | |
| fr,12,Hoffman (N/mm2) | Mean | 1.51 | 1.00 | 1.18 | 1.06 | 1.60 | 1.19 | 0.94 | 1.61 | 1.16 | 0.98 | 1.08 | 1.41 | 0.99 |
| CoV (%) | 8.88 | 10.2 | 9.42 | 11.7 | 4.23 | 11.9 | 12.5 | 4.90 | 10.0 | 8.53 | 11.6 | 16.6 | 15.6 | |
| LN0.05 2 | 1.30 | 0.84 | 1.01 | 0.87 | 1.49 | 0.97 | 0.76 | 1.49 | 0.99 | 0.85 | 0.88 | 1.05 | 0.74 | |
| charEN14358 3 | 1.22 | 0.78 | 0.95 | 0.81 | 1.42 | 0.89 | 0.70 | 1.43 | 0.93 | 0.80 | 0.81 | 0.99 | 0.70 | |
| fr,12,Mestek (N/mm2) | Mean | 1.49 | 0.99 | 1.13 | 1.02 | 1.47 | 1.14 | 0.92 | 1.59 | 1.15 | 0.97 | 1.06 | 1.36 | 0.95 |
| CoV (%) | 8.75 | 10.0 | 9.13 | 11.3 | 4.12 | 11.6 | 12.2 | 4.82 | 9.82 | 8.45 | 11.5 | 15.7 | 15.1 | |
| LN0.05 2 | 1.29 | 0.83 | 0.98 | 0.85 | 1.37 | 0.94 | 0.75 | 1.47 | 0.98 | 0.85 | 0.87 | 1.03 | 0.72 | |
| charEN14358 3 | 1.21 | 0.77 | 0.92 | 0.79 | 1.30 | 0.87 | 0.69 | 1.41 | 0.92 | 0.80 | 0.80 | 0.97 | 0.67 | |
| Test Series | 01 | 02 | 03 | 04 | 05 | 06 | 07 | 08 | 09 | 10 | 11 | 12 | 13 | 14 | 15 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| No. of Specimens | 20 | 20 | 16 | 19 | 20 | 19 | 20 | 17 | 20 | 19 | 21 | 20 | 21 | 21 | 19 | |
| u (%) | Mean | 13.2 | 13.4 | 12.8 | 13.4 | 11.4 | 11.0 | 11.1 | 12.3 | 13.3 | 12.9 | 9.94 | 11.9 | 7.87 | 8.68 | 13.1 |
| CoV (%) | 1.78 | 3.43 | 3.06 | 2.47 | 2.46 | 2.27 | 2.01 | 5.22 | 2.44 | 3.64 | 12.2 1 | 3.22 | 9.34 | 3.28 | 5.23 | |
| ρ12 (kg/m3) | Mean | 445 | 442 | 437 | 444 | 446 | 445 | 446 | 444 | 446 | 444 | 472 | 465 | 457 | 461 | 456 |
| Median | 446 | 442 | 435 | 443 | 446 | 442 | 448 | 443 | 448 | 444 | 464 | 471 | 458 | 460 | 459 | |
| CoV (%) | 1.31 | 1.06 | 2.34 | 1.88 | 1.77 | 1.22 | 1.68 | 2.53 | 1.85 | 1.59 | 3.64 | 3.79 | 1.72 | 1.10 | 1.67 | |
| fr,12 (N/mm2) | Mean | 1.76 | 2.42 | 2.36 | 1.92 | 1.58 | 2.00 | 1.92 | 1.89 | 1.53 | 1.62 | 1.52 | 1.88 | 1.22 | 1.12 | 1.49 |
| CoV (%) | 4.53 | 5.45 | 5.39 | 11.0 | 8.84 | 3.18 | 5.09 | 5.89 | 6.12 | 3.66 | 7.04 | 5.69 | 8.66 | 4.31 | 6.54 | |
| LN0.05 | 1.63 | 2.21 | 2.16 | 1.58 | 1.36 | 1.90 | 1.77 | 1.71 | 1.38 | 1.52 | 1.35 | 1.71 | 1.06 | 1.04 | 1.34 | |
| charEN14358 | 1.59 | 2.17 | 2.11 | 1.53 | 1.33 | 1.81 | 1.74 | 1.68 | 1.36 | 1.47 | 1.32 | 1.68 | 1.03 | 1.09 | 1.31 | |
| fr,12,SIA (N/mm2) | Mean | 1.61 | 2.24 | 2.17 | 1.85 | 1.52 | 1.85 | 1.75 | 1.82 | 1.46 | 1.50 | 1.48 | 1.79 | 1.19 | 1.07 | 1.40 |
| CoV (%) | 4.22 | 5.08 | 5.08 | 10.7 | 8.55 | 2.99 | 4.71 | 5.71 | 5.89 | 3.43 | 6.87 | 5.46 | 8.44 | 4.16 | 6.20 | |
| LN0.05 | 1.50 | 2.05 | 1.99 | 1.53 | 1.31 | 1.76 | 1.61 | 1.66 | 1.32 | 1.42 | 1.31 | 1.63 | 1.03 | 1.00 | 1.26 | |
| charEN14358 | 1.46 | 2.02 | 1.95 | 1.48 | 1.28 | 1.67 | 1.59 | 1.63 | 1.30 | 1.36 | 1.29 | 1.61 | 1.01 | 0.97 | 1.23 | |
| fr,12,Hoffmann (N/mm2) | Mean | 1.67 | 2.33 | 2.27 | 1.88 | 1.54 | 1.92 | 1.82 | 1.85 | 1.49 | 1.55 | 1.49 | 1.83 | 1.20 | 1.09 | 1.44 |
| CoV (%) | 4.40 | 5.32 | 5.35 | 10.8 | 8.69 | 3.13 | 4.95 | 5.80 | 6.03 | 3.55 | 6.94 | 5.61 | 8.53 | 4.24 | 6.40 | |
| LN0.05 | 1.55 | 2.13 | 2.07 | 1.55 | 1.33 | 1.82 | 1.68 | 1.68 | 1.35 | 1.46 | 1.33 | 1.67 | 1.04 | 1.02 | 1.29 | |
| charEN14358 | 1.52 | 2.09 | 2.03 | 1.50 | 1.30 | 1.74 | 1.65 | 1.65 | 1.32 | 1.40 | 1.30 | 1.64 | 1.01 | 0.99 | 1.26 | |
| fr,12,Mestek (N/mm2) | Mean | 1.58 | 2.17 | 2.14 | 1.82 | 1.49 | 1.83 | 1.71 | 1.79 | 1.44 | 1.47 | 1.46 | 1.77 | 1.17 | 1.06 | 1.38 |
| CoV (%) | 4.30 | 5.16 | 5.10 | 10.6 | 8.42 | 3.03 | 4.84 | 5.64 | 5.92 | 3.43 | 6.78 | 5.50 | 8.33 | 4.19 | 6.25 | |
| LN0.05 | 1.47 | 1.99 | 1.97 | 1.51 | 1.30 | 1.74 | 1.57 | 1.63 | 1.31 | 1.39 | 1.30 | 1.61 | 1.02 | 0.99 | 1.24 | |
| charEN14358 | 1.43 | 1.96 | 1.93 | 1.46 | 1.26 | 1.65 | 1.55 | 1.61 | 1.28 | 1.33 | 1.27 | 1.59 | 1.00 | 0.96 | 1.21 | |
| fr,ref (N/mm2) | c0 (-) | c1 (-) | |
|---|---|---|---|
| fr,mean | 5.74 | 0.85 | 0.15 |
| fr,0.05,emp | 5.05 | 0.80 | 0.20 |
| 4PB ”Validation” | Test Series | Manufacturer No. | Layup | tCLT | w | a1 | a2 | Overlap | ws |
| (-) | (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | ||
| PS-100-600-300 | 04 | 40-20-40 | 100 | 600 | 300 | 600 | 100 | 50 | |
| PS-280-300-840 | 06 | 40-40-40-40-40-40-40 | 280 | 300 | 840 | 840 | 140 | 100 |
| Test Series | No. of Specimens | u (%) | ρ12 (kg/m3) | fr,12 (N/mm2) | fr,12,SIA (N/mm2) | |||||||||
| Mean | CoV (%) | Mean | Median | CoV (%) | Mean | CoV (%) | LN0.05 | charEN14358 | Mean | CoV (%) | LN0.05 | charEN14358 | ||
| PS-100-600-300 | 19 | 10.7 | 7.53 | 456 | 460 | 5.52 | 2.27 | 6.93 | 2.01 | 1.97 | 2.13 | 6.76 | 1.90 | 1.87 |
| PS-280-300-840 | 8 | 10.9 | 4.98 | 444 | 443 | 2.98 | 1.35 | 4.52 | 1.25 | 1.21 | 1.30 | 4.67 | 1.20 | 1.16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Glasner, D.; Ringhofer, A.; Brandner, R.; Schickhofer, G. Rolling Shear Strength of Cross Laminated Timber (CLT)—Testing, Evaluation, and Design. Buildings 2023, 13, 2831. https://doi.org/10.3390/buildings13112831
Glasner D, Ringhofer A, Brandner R, Schickhofer G. Rolling Shear Strength of Cross Laminated Timber (CLT)—Testing, Evaluation, and Design. Buildings. 2023; 13(11):2831. https://doi.org/10.3390/buildings13112831
Chicago/Turabian StyleGlasner, David, Andreas Ringhofer, Reinhard Brandner, and Gerhard Schickhofer. 2023. "Rolling Shear Strength of Cross Laminated Timber (CLT)—Testing, Evaluation, and Design" Buildings 13, no. 11: 2831. https://doi.org/10.3390/buildings13112831
APA StyleGlasner, D., Ringhofer, A., Brandner, R., & Schickhofer, G. (2023). Rolling Shear Strength of Cross Laminated Timber (CLT)—Testing, Evaluation, and Design. Buildings, 13(11), 2831. https://doi.org/10.3390/buildings13112831








