A Numerical Study on the Exergy Performance of a Hybrid Radiant Cooling System in an Office Building: Comparative Case Study and Analysis

Abstract

This research investigated the exergy enhancement performance of a hybrid radiant cooling system adapting to a hot and humid summer conditions through comparative case studies and analyses. This study suggested three cooling systems: a general all-air system (AAS), a conventional radiant cooling system (CRCS), and a hybrid radiant cooling system (HRCS). As a case study, an office building with cooling systems was examined in the summer season in four different cities: Beijing, Shanghai, Chengdu, and Guangzhou, China. This study utilized the building energy performance simulation program to analyze the cooling loads of office space in a building with numerical approaches. The comparison analysis using the four different weather datasets showed simple and rational exergy efficiency and the overall impact ratio. According to the results, the ambient conditions, i.e., the surrounding temperature and the humidity ratio, significantly impacted the cooling systems’ exergy efficiency ratio. On the basis of the calculated energetic and exergetic performance, the HRCS had a higher exergy efficiency and a higher overall impact ratio. The HRCS system released an additional 20–30% of cooling output, and it could adapt well in extreme hot and humid weather conditions compared to the AAS and the CRCS system. The overall cooling impact ratio of the HRCS with an airbox convector was approximately 185% higher than that of the AAS and 8.5% higher than that of the CRCS. This study can provide the design references for the hybrid radiant cooling system and other cooling systems in hot and humid summer conditions.

1. Introduction

Energy consumption in buildings is mainly responsible for climate change and global warming because the building sector emits about one-third of the total amount of global carbon dioxide (CO₂) [1,2]. In the building sector, heating, ventilation, and air-conditioning (HVAC) systems are responsible for the majority of the building energy consumption. In particular, global warming has affected the rise in cooling loads in hot and humid climates. Low-exergy technologies that have a high-temperature cooling system, e.g., radiant cooling systems [3,4], could minimize the actual environmental impact and maximize the energy efficiency of the systems due to the minimization of the exergy released and the CO₂ emissions [5,6].

Many studies have illustrated the exergy performance of space heating and cooling systems in buildings [7,8]. The studies described that an exergy-based analysis strategy could represent a high-performance system and optimize the exergy efficiency. Moreover, the exergy-based analysis accurately articulates energy source quality and flow [8,9]. The exergetic approach illustrates a better understanding of our surrounding built environment and the exergy flow for cooling and heating in buildings, as well as how exergy is constructed or deconstructed in the process [10]. This research newly investigates the exergy enhancement performance of a hybrid radiant cooling system to present the exergy potential and efficiency compared to three different cooling systems, adapting to a local environment as comparative analysis.

It is well known that the conventional radiant cooling system (CRCS) has limited adaptation to surrounding hot and humid conditions due to high air vapor condensation risk on the surface of a radiant cooling panel when unexpected humidity occurs, such as when opening windows or through the moisture generation of occupants. Moreover, it faces difficulty with increasing cooling outputs in extreme weather conditions. Recently, a hybrid radiant cooling system (HRCS) was newly designed, adapted to the hot and humid climate [11,12,13]. The novel HRCS comprised two units—a CRCS and an airbox convector. The major benefits of this system are that the HRCS can reduce vapor condensation risks on the radiant cooling panel surface due to dehumidification and the adjusted surface temperature using an airbox convector. Moreover, the HRCS can simplify space zoning for cooling in an occupied area due to adjusted air movement and directivity using an airbox convector, and it can improve indoor air quality due to using the air filters in an airbox convector [14].

Energy balance processing has been commonly used to investigate the calculation of energy load and efficiency and performance of the HRCS to adapt to hot and humid summer conditions; however, the balance methodology does not indicate the source’s energy quality and availability. Recently, exergy analysis combined the two major laws of thermodynamics applied to reflect the coupling of both energy potential and availability [7,15], e.g., using a conventional boiler in a heating center [16] and a novel desiccant cooling system [17]. According to exergy studies, exergy evaluation is defined by the value inherent in heat fluxes denoted throughout gradients of temperature difference [10,18]. Small temperature differences, hence, can minimize exergetic destruction or entropy generation and maximize energy reversibility. Therefore, the high-temperature cooling and low-temperature heating system minimizes the energetic value and the actual environmental impact of the systems [19,20]. For example, a fossil-fuel boiler releases high-temperature energy sources in the combustion process and generates high entropy [21]. However, a low-temperature heating and high-temperature cooling system with a heat pump system can use low-exergetic-energy sources, minimize entropy generation, and utilize energy reversibility with the least energy source added [22,23]. In the end, these leading approaches can improve exergy efficiency and reduce the actual environmental impact of systems [24,25].

Therefore, this study explored the energy and exergy potential performance analyses of a hybrid radiant cooling system compared with three cooling systems adapted to hot and humid summer conditions in four major cities, Beijing, Shanghai, Chengdu, and Guangzhou, located in China, as a comparative analysis. Consequently, the performance of each system was evaluated by exergetic approaches.

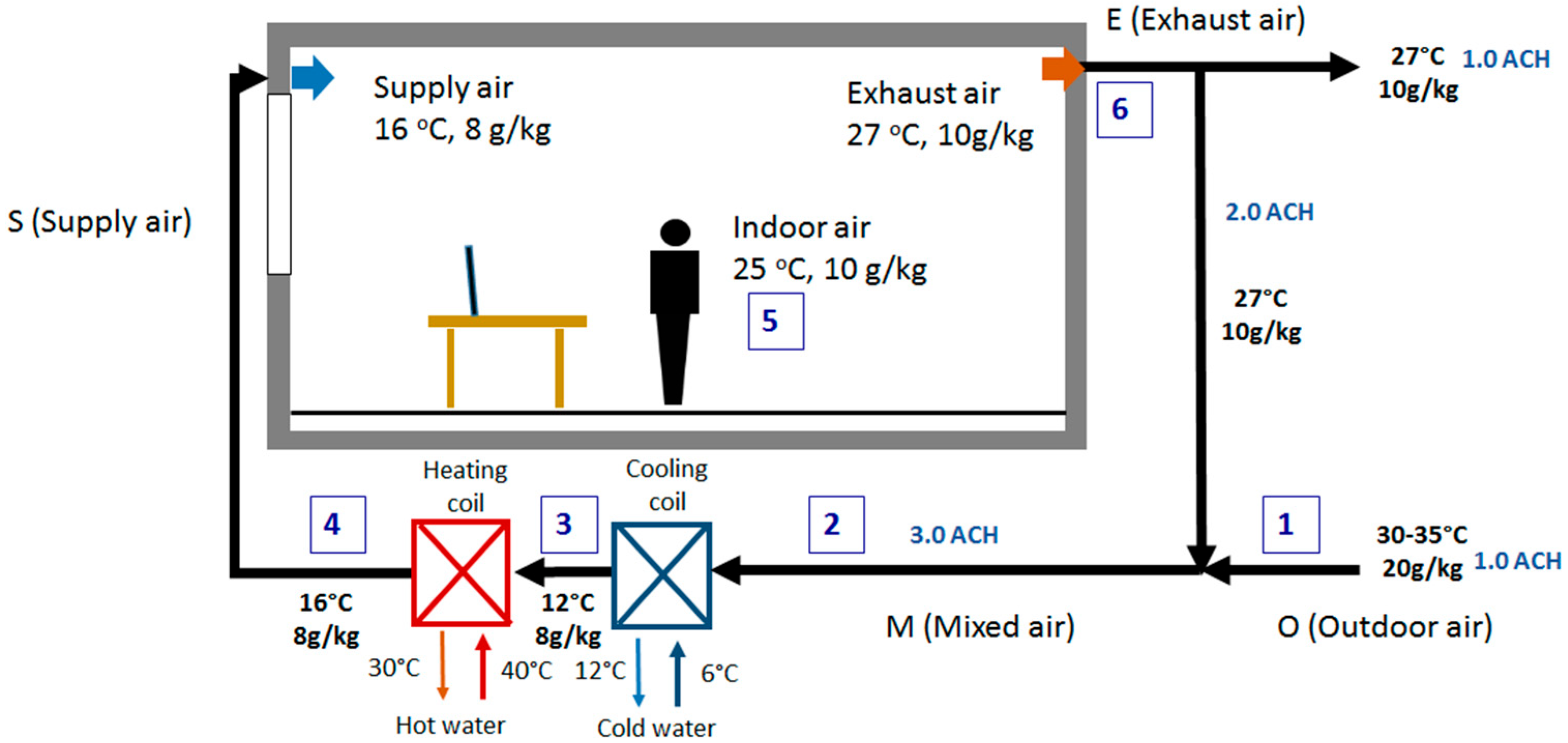
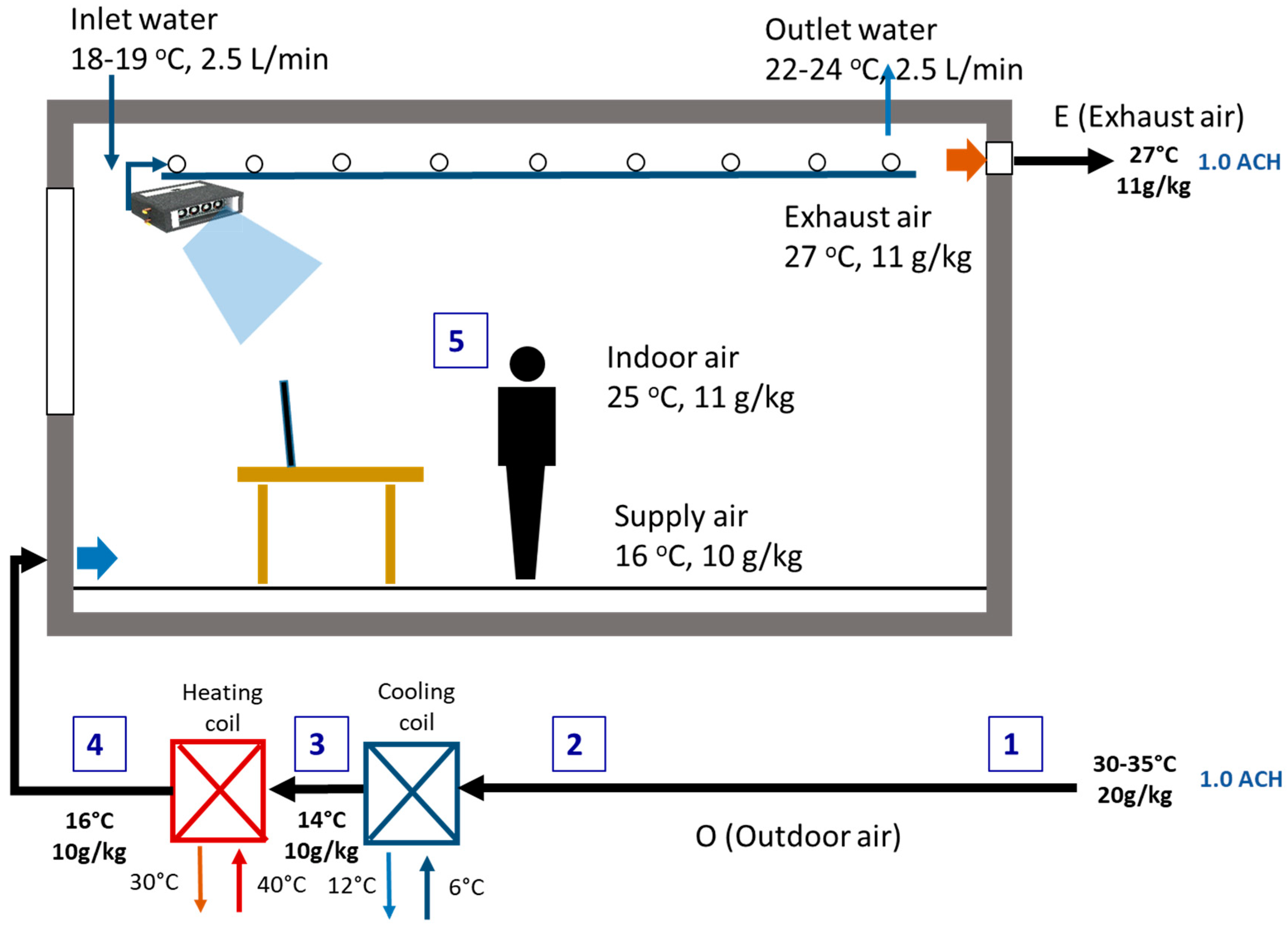
2. System Description and Methods

2.1. Cooling Systems in a Building

The common HVAC systems with ventilation can be classified into three types in buildings: an all-air system (AAS), a CRCS with air handing unit (AHU), and an HRCS with an AHU [26,27]. An AAS, which is currently very commonly used, supplies an amount of air to deliver fresh air to occupants, as well as heat transfer and cooling in a room [28,29]. Figure 1 presents the conceptual process of an AAS that supplies chilled and dehumidified outdoor air that is mixed with return air to reduce cooling energy loads. The system can simply adjust air volume depending on the thermal loads. However, it also consumes a large amount of electricity for fan energy and releases airflow noise while supplying a large volume of air.

A CRCS with an AHU consumes less energy than an AAS [30] because it minimizes the air volume supplied, and the high-temperature cooling system can minimize entropy generation and maximize the coefficient of performance (COP). The system is divided into the air ventilation and cooling tasks by employing radiant cooling panels to deal with the sensible cooling load while utilizing a fresh air supplying system [31,32]. Figure 2 illustrates a schematic of the CRCS with AHU. However, this system has limitations for use in certain places. High internal humidity gains cause moisture condensation on the chilled radiant panel surface. Therefore, the supply air should be dehumidified below 8 g/kg of the humidity ratio in case of internal humidity gains [33,34]. Compared with an AAS, a CRCS has a time delay for activation when cooling in a room [35,36,37]. Small airflow rates have difficulty improving indoor air quality in a highly polluted zone [30,38,39]. The CRCS also has difficulties adjusting the cooling outcome because a chilled water supply directly passing through the radiant cooling panel causes condensation, especially in hot and humid surrounding conditions [32,40,41,42]. Most buildings in China use the AAS because China weather conditions are hot and humid in the summer season, and the CRCS is limited to use in a building that combines natural ventilation system and a high infiltration rate with poor air tightness. However, the AAS has been widely used in residential and commercial buildings since it can simply adjust cooling outputs with a variable air volume control system.

Figure 3 shows the schematic HRCS with AHU. A compact air convector and a chilled radiant panel system are connected in series, adjusting and controlling the airflow for cooling and dehumidification in a convector and sensible cooling with a chilled radiant ceiling panel. An airbox convector consists of compact heat exchangers, electric fans, air filter, and a drain tube [43]. During the process in humid indoor conditions higher than a 12 g/kg humidity ratio, the water vapor is condensed in the compact air convector, and the condensed water is drained through a tube [14,44]. The supplied water temperature is increased by 1–2 °C after passing through the airbox unit. The system can simply adjust actual cooling output based on surrounding environments. In general, to prevent moisture condensation on the chilled radiant panel, the supplied water temperature must be around 18 °C and the supplied air should be dehumidified around 8–9 g/kg humidity ratio. However, a HRCS can allow the supplied air to be dehumidified up to 10 g/kg to save cooling energy since an airbox convector can dehumidify the indoor air to reduce moisture condensation risk and to improve thermal comfort in conditions of high internal moisture gain.

Figure 4 shows a psychrometric of the three systems. In the figure, the cooling and reheating processes of each system are clearly illustrated as a comparative analysis. We can estimate the quantitative cooling and reheating loads of each system using the psychrometric. However, the psychrometric analysis has a limitation in presenting exergetic performance. Actually, entropy generation and the energy reversibility utilized in the cooling and reheating processes affect the exergy performance. The psychrometric can reflect energy quantitatively but does not provide qualitative analysis.

2.2. Weather and Building Characteristics as Case Studies in China

This study selected four local China weather conditions, Beijing (northern China), Shanghai (southeastern China), Chengdu (northwestern China), and Guangzhou (southern China), to investigate the acceptable performance for the operation of AAS, CRCS, and HRCS in the hot and humid summer season. Beijing, the capital city of China, is located in northern China, and it has hot and humid summer conditions due to East Asian monsoons. Shanghai is located in the Yangtze River in southeastern China and shows a humid subtropical climate. Chengdu, located in northwestern China, has a humid subtropical climate with high relative humidity in the summer season. Guangzhou, located in southern China, has a hot and humid summer with a high heat index.

Figure 5 and Figure 6 illustrate the weather data (temperature and humidity) in the summer season (June–August) of four local cities in China. These places in the summer season are very hot and humid, requiring entirely high cooling and ventilation loads. The Beijing weather data illustrate high temperature variations between day and nighttime, as well as seasonal changes. Therefore, the temperatures oscillate largely in a day, but relatively lower humidity ratios are shown compared to other cities. The Guangzhou weather data show low temperature differences between daytime and nighttime and small seasonal changes, but high temperature and humidity ratios are illustrated in the overall years. Therefore, we estimated that Guangzhou weather was challenging due to high cooling and dehumidification loads. The Shanghai weather conditions present high temperature and humidity ratios in the summer and autumn seasons and a relatively low temperature and humidity ratio in the spring and winter. The Chengdu weather conditions have a similarity to the Beijing and Shanghai weather conditions.

This study selected a small office space in the four major cities for these case studies, which used a heat pump system for the cooling and air ventilation processes. The container-size office unit was used as a test room to evaluate the cooling and ventilation capacity of an AAS, a CRCS with an AHU, and an HRCS with an AHU. The building wall and window materials were selected and used for a case that was designed as a normal thermal performance case.

The thermal performance of the above systems was simulated using the TRNSYS program [45], which has been widely employed to simulate radiant cooling systems [46]. The multimode building module Type 56 was used to model the dynamic thermal performance by solving the energy balance equations. The heat balance equation considers the change rate of air node thermal energy, building envelope node heat transfer rate, and power out of the indoor heat source node, as shown in Equation (1).

$$c\dot{m}\left(T_{\mathrm{set}}-T_{\mathsf{\tau }}\right)={\dot{Q}}_{\mathrm{surf}}+{\dot{Q}}_{\mathrm{air}}+{\dot{Q}}_{\inf }+{\dot{Q}}_{\mathrm{int}}+{\dot{Q}}_{\mathrm{solar}},$$

(1)

where c is the specific heat capacity of air in kJ/(kg⸱K), $\dot{m}$ is the mass flow rate in kg/h, T_set indicates the indoor cooling set temperature in K, $T_{\mathsf{\tau }}$ is the indoor air temperature at the time of τ in K, ${\dot{Q}}_{\mathrm{surf}}$ is the radiative and convective gain from the surfaces in kJ/h, ${\dot{Q}}_{\mathrm{air}}$ is the heat gain due to air entering indoor in kJ/h, Q_inf is the infiltration gain in kJ/h, Q_int denotes the radiative and convective gains by the internal heat source in kJ/h, and Q_solar is the solar radiation gain through the windows in kJ/h.

Table 1. Simulation setting in the office room.

Settings	Values
Volume flow rate (m3/h)	43
Net floor area (m2)	15
Indoor air temperature (°C)	25
Ventilation rate (h−1)	1.0
Infiltration rate (h−1)	0.1
Occupant (W)	150 × 2
Computer (W)	100 × 2
Lighting (W)	10 × 2

presents the simulation setup of the building model in the software. This is an office room with a floor area of 5 m × 3 m and a south-facing window with a window-to-wall ratio of 0.33.

Table 2. U-values of the building envelope.

Structure	U-Values, W/(m2⸱K)
Ceiling	0.215
Long wall	0.215
Short wall	0.215
Ground	0.151
Window	1.432

shows the envelopes’ overall heat transfer coefficient (U-values). A recommended supplied air volume flow rate of 43 m³/h, with 1.0 air changes per hour (ACH), was selected according to international standards for normal air quality [47,48,49], and the space area of the office room was 14.3 m². This study estimated that two occupants worked in the room.

2.3. Energy and Exergy Performance of the Systems

2.3.1. Energy Load Calculation

The energy load for the heat transfer to supply air for cooling is expressed as follows [28,50,51]:

$$\dot{Q}={\dot{m}}_{\mathrm{air}}\Delta h,$$

(2)

where ${\dot{m}}_{\mathrm{air}}$ is the mass flow rate of the supply air (kg/h), and $\Delta h$ is the enthalpy change value (kJ/kg) in the air cooling and dehumidification process of the heat exchanger with an AHU.

$${\dot{Q}}_{\mathrm{total} }={\dot{Q}}_{\mathrm{sen}}+{\dot{Q}}_{\mathrm{lat}},$$

(3)

The total cooling energy load value of air, ${\dot{Q}}_{\mathrm{total} }$, is calculated from the sum value of the sensible (${\dot{Q}}_{\mathrm{sen}}$) and latent (${\dot{Q}}_{\mathrm{lat}}$) cooling energy load.

The sensible energy load value of air, ${\dot{Q}}_{\mathrm{sen}}$, is as follows:

$${\dot{Q}}_{\mathrm{sen}}={\dot{m}}_{\mathrm{air}}{c}_{\mathrm{p}.\mathrm{air}}\Delta T,$$

(4)

The latent energy load value of air, ${\dot{Q}}_{\mathrm{lat}}$, is as follows:

$${\dot{Q}}_{\mathrm{lat}}={\dot{m}}_{\mathrm{air}}{h}_{\mathrm{fg}}\Delta w.$$

(5)

The total energy load of the water side is determined from the equation below.

$${\dot{Q}}_{\mathrm{water}}={\dot{m}}_{\mathrm{water}}{c}_{\mathrm{p}.\mathrm{water}}\left(T_{\mathrm{out}.\mathrm{water}}-T_{\mathrm{in}.\mathrm{water}}\right),$$

(6)

where h_fg is the specific enthalpy value of the vaporization of water in kJ/kg, c_p.air is the specific heat capacity value of air in kJ/(kg⸱K), ${\dot{m}}_{\mathrm{air}}$ is the mass flow rate of air in kg/h, Δw is the change in the humidity ratio of air in kg/kg, ΔT is the temperature change value of air in K, Δh is the change in specific enthalpy in kJ/kg, ${\dot{m}}_{\mathrm{water}}$ is the mass flow rate of water in kg/h, and c_p.water is the specific heat capacity of water in kJ/(kg⸱K).

The total energy load of AAS for cooling and dehumidification only or for cooling, dehumidification, and reheating is presented as follows:

$${\dot{Q}}_{\mathrm{AAS}}={\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{mix}.\mathrm{air}}-h_{\mathrm{cooling} \mathrm{and} \mathrm{dehum}}\right)+{\dot{m}}_{\sup .\mathrm{air}}{C}_{\mathrm{p}.\mathrm{air}}\left(T_{\mathrm{reheat}}-T_{\mathrm{c}}\right).$$

(7)

The total energy load of the CRCS with an AHU is shown as follows:

$${\dot{Q}}_{\mathrm{CRCS}\_\mathrm{AHU}}={\dot{m}}_{\sup .\mathrm{water}}{C}_{\mathrm{p}.\mathrm{water}}\left(T_{\mathrm{out}}-T_{\mathrm{in}}\right)+{\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{out}}-h_{\mathrm{cooling} \mathrm{and} \mathrm{dehum}}\right)+{\dot{m}}_{\sup .\mathrm{air}}{C}_{\mathrm{p}.\mathrm{air}}\left(T_{\mathrm{reheat}}-T_{\mathrm{c}}\right).$$

(8)

The total energy load of the HRCS with an AHU is shown as follows:

$${\dot{Q}}_{\mathrm{HRCS}\_\mathrm{AHU}}={\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{out}}-h_{\mathrm{c}}\right)+{\dot{m}}_{\sup .\mathrm{water}}{C}_{\mathrm{p}.\mathrm{water}}\left(T_{\mathrm{out}}-T_{\mathrm{in}}\right)+P_{\mathrm{airbox}}.$$

(9)

The actual cooling capacities of the AAS, the CRCS with AHU, and the HRCS with AHU are as follows:

$${\dot{Q}}_{\mathrm{CC}\_\mathrm{AAS}}={\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{indoor}.\mathrm{air}}-h_{\sup .\mathrm{air}}\right),$$

(10)

$${\dot{Q}}_{\mathrm{CC}\_\mathrm{CRCS}\_\mathrm{AHU}}={\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{indoor}.\mathrm{air}}-h_{\sup .\mathrm{air}}\right)+{\dot{m}}_{\sup .\mathrm{water}}{C}_{\mathrm{p}.\mathrm{water}}\left(T_{\mathrm{out}}-T_{\mathrm{in}}\right),$$

(11)

$${\dot{Q}}_{\mathrm{CC}\_\mathrm{HRCS}\_\mathrm{AHU}}={\dot{m}}_{\sup .\mathrm{air}}\left(h_{\mathrm{indoor}.\mathrm{air}}-h_{\sup .\mathrm{air}}\right)+{\dot{m}}_{\sup .\mathrm{water}}{C}_{\mathrm{p}.\mathrm{water}}\left(T_{\mathrm{out}}-T_{\mathrm{in}}\right).$$

(12)

2.3.2. Exergy Load Calculation

In general, the heat output of a system is a combination of the exergy input ($e{x}_{\mathrm{input}}$) and anergy input ($A{n}_{\mathrm{input}}$, corresponding to the waste energy) [52,53].

$${\dot{Q}}_{\mathrm{ex}.\mathrm{an}}=e{x}_{\mathrm{input}}+A{n}_{\mathrm{input}}.$$

(13)

According to the Carnot COP, the heat output is maximized by minimizing the temperature lifting in the thermal process of systems.

$$COP=\frac{{\dot{Q}}_{\max }}{W_{\mathrm{input}}}=T_{\mathrm{hot}}/\left(T_{\mathrm{hot}}-T_{\mathrm{cold}}\right).$$

(14)

Exergy presents the energy availability to convert the maximum theoretical work in the energy generated or transferred [52,53]. The exergy is zero when the system’s state is in equilibrium with the surrounding environment, the so-called ambient condition [22,52,53]. The ambient condition is defined in terms of the temperature, humidity ratio, pressure, and chemical potentials. In terms of the exergy load evaluation and analysis, the exergy load is classified into two exergy parts, the chemical and physical exergy areas. The chemical exergy is defined by the chemical potential μ_i with the pressure (p_o), temperature (T_o), and chemical potential (μ_o) [53], and the physical exergy is defined by the pressure (p) and temperature (T) of the system. The detailed formulas were illustrated in [22,53,54].

The total specific exergy of a supply air system is shown as follows:

$$e{x}_{\mathrm{tot}}=e{x}_{\mathrm{phys}}+e{x}_{\mathrm{chem}}.$$

(15)

The physical exergy of air with humidity is shown as follows [54,55]:

$$e{x}_{\mathrm{phys}.\mathrm{air}}=\left(C_{\mathrm{p}.\mathrm{air}}+\omega C_{\mathrm{p}.\mathrm{vapor}}\right)\left[\left(T-T_{\mathrm{o}}\right)-T_{\mathrm{o}}ln\frac{T}{T_{\mathrm{o}}}\right]+(1+\mathrm{ῶ})R_{\mathrm{air}}{T}_{\mathrm{o}}ln\frac{p}{p_{\mathrm{o}}}.$$

(16)

The chemical exergy of air with humidity variation is expressed as follows [55,56]:

$$e{x}_{\mathrm{chem}.\mathrm{air}}=R_{\mathrm{air}}{T}_{\mathrm{o}}\left[\left(1+\mathrm{ῶ}\right)ln\frac{1+{\mathrm{ῶ}}_{\mathrm{o}}}{1+\mathrm{ῶ}}+\mathrm{ῶ}ln\frac{\mathrm{ῶ}}{{\mathrm{ῶ}}_{\mathrm{o}}}\right],$$

(17)

where R_air is the specific ideal gas constant in J/(kg⸱K), T_o is the outdoor air temperature in K, ω is the indoor air humidity ratio in kg/kg, ῶ is mole fraction ratio, ω_o is the outdoor air humidity ratio in kg/kg, c_p.vapor is the specific heat capacity of water vapor in kJ/(kg⸱K), and p_o is the outdoor air pressure in Pa.

The liquid water physical specific exergy passing through a ceiling panel for cooling is as follows:

$$e{x}_{\mathrm{phys}.\mathrm{water}}=C_{\mathrm{p}.\mathrm{water}}\left[\left(T-T_{\mathrm{o}}\right)-T_{\mathrm{o}}ln\frac{T}{T_{\mathrm{o}}}\right]+\nu \left(p-p_{\mathrm{o}}\right).$$

(18)

The chemical exergy of liquid water is shown as follows [54,55]:

$$e{x}_{\mathrm{chem}.\mathrm{water}}=\left(p-p_{\mathrm{sat}}\right)\nu -R_{\mathrm{w}}{T}_{\mathrm{o}}ln{\varphi }_{\mathrm{o}},$$

(19)

where p_sat is the saturated water vapor pressure in Pa, and ϕ_o is the outdoor air relative humidity as a percentage.

To calculate the dynamic total exergy loads with physical and chemical compositions, in this study, a reference environment was used as the actual variable hourly weather data surrounding a building in four major cities, Beijing, Shanghai, Chengdu, and Guangzhou, in China.

Additionally, pump and fan energy represents one of the main parts of an HVAC system. The electric power of the pump and fan energy of the HVAC system is 100% exergy [54]. The calculation of fan and pump power is given as follows [28,51]:

$$P_{\mathrm{fan}}={\dot{V}}_{\mathrm{fan}}\Delta p/\left(3600{\eta }_{\mathrm{fan}}\right),$$

(20)

$$P_{\mathrm{pump}}={\dot{V}}_{\mathrm{pump}}\Delta p/\left(3600{\eta }_{\mathrm{pump}}\right),$$

(21)

where ${\dot{V}}_{\mathrm{fan}}$ and ${\dot{V}}_{\mathrm{pump}}$ are the air and water volumetric flow rate in m³/h, respectively, $\Delta p$ represents the total pressure differences in Pa, and ${\eta }_{\mathrm{fan}}$ and ${\eta }_{\mathrm{pump}}$ are the fan and pump system efficiencies of units as a percentage, respectively.

2.3.3. Exergy Efficiencies

Exergy analysis shows a methodology to evaluate the impact of a building energy system on the environment [57]. The method accesses a system to present the interactions between building systems, as well as its surroundings, from an exergetic view [57]. Relatively low exergy destruction means low entropy generation; therefore, it can maximize high energy availability in a thermal system [7,11,18]. The reference literature describes the exergy performance efficiencies of a system that is determined as the ratio between the input required or consumed and the output obtained or generated for the exergy in systems [58,59]. In general, two types of exergy efficiencies are defined and explained: “simple or universal” (${\psi }_{\mathrm{sim}}$) and “rational and functional” (${\psi }_{\mathrm{rat}}$) [22,59]. The mathematical approaches are shown below.

$${\psi }_{\mathrm{sim}}=\frac{e{x}_{\mathrm{out}}}{e{x}_{\mathrm{in}}}.$$

(22)

$${\psi }_{\mathrm{rat}}=\frac{e{x}_{\mathrm{desired},\mathrm{out}}}{e{x}_{\mathrm{in}}}.$$

(23)

Many researchers have investigated the exergy efficiencies using different approaches [11,54,59]. The simple exergy ratio describes the estimation of differences between the actual involved processes in a system and the ideal performance. The rational exergy ratio presents how much potential is consumed to generate the required output in a system. Generally, exergy losses have been explained with the rational efficiency because the ratio presents both the unconsumed output exergy and the irreversible processes released [22,59].

Simple ratios for different systems are shown as follows:

$${\psi }_{\mathrm{sim}\_\mathrm{AAS}}=\frac{{\dot{m}}_{3\mathrm{a}}e{x}_4+{\dot{m}}_{\mathrm{cw}}e{x}_{\mathrm{cw}}+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\mathrm{re}}+{\dot{m}}_{\mathrm{hc}}e{x}_{\mathrm{hot}.\mathrm{re}}}{{\dot{m}}_{1\mathrm{a}}e{x}_1+{\dot{m}}_{2\mathrm{a}}e{x}_6+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\sup }+{\dot{m}}_{\mathrm{hc}}e{x}_{\mathrm{hot}.\sup }},$$

(24)

$${\psi }_{\mathrm{sim}\_\mathrm{CRCS}\_\mathrm{AHU}}=\frac{{\dot{m}}_{\mathrm{a}}e{x}_4+{\dot{m}}_{\mathrm{cw}}e{x}_{\mathrm{cw}}+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\mathrm{re}}+{\dot{m}}_{\mathrm{hc}}e{x}_{\mathrm{hot}.\mathrm{re}}+{\dot{m}}_{\mathrm{chc}}e{x}_{\mathrm{ceil}.\mathrm{re}}}{{\dot{m}}_{a}e{x}_1+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\sup }+{\dot{m}}_{\mathrm{hc}}e{x}_{\mathrm{hot}.\sup }+{\dot{m}}_{\mathrm{chc}}e{x}_{\mathrm{ceil}.\sup }},$$

(25)

$${\psi }_{\mathrm{sim}\_\mathrm{HRCS}\_\mathrm{AHU}}=\frac{{\dot{m}}_{\mathrm{a}}e{x}_4+{\dot{m}}_{\mathrm{cw}}e{x}_{\mathrm{cw}}+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\mathrm{re}}+{\dot{m}}_{\mathrm{chc}}e{x}_{\mathrm{ceil}.\mathrm{re}}}{{\dot{m}}_{\mathrm{a}}e{x}_1+{\dot{m}}_{\mathrm{cc}}e{x}_{\mathrm{cool}.\sup }+{\dot{m}}_{\mathrm{chc}}e{x}_{\mathrm{ceil}.\sup }}.$$

(26)

Rational ratios for different systems are shown as follows:

$${\psi }_{\mathrm{rat}\_\mathrm{AAS}}=\frac{{\dot{m}}_{1\mathrm{a}}(e{x}_4-E{x}_1)+{\dot{m}}_{2\mathrm{a}}(e{x}_4-e{x}_6)}{{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{hc}}\left(e{x}_{\mathrm{hot}.\sup }-e{x}_{\mathrm{hot}.\mathrm{re}}\right)+{\dot{m}}_{chc}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})},$$

(27)

$${\psi }_{\mathrm{rat}\_\mathrm{CRCS}\_\mathrm{AHU}}=\frac{{\dot{m}}_{\mathrm{a}}(e{x}_4-e{x}_1)+{\dot{m}}_{\mathrm{a}}(e{x}_4-e{x}_6)}{{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{hc}\left(e{x}_{\mathrm{hot}.\sup }-e{x}_{\mathrm{hot}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{chc}}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})},$$

(28)

$${\psi }_{\mathrm{rat}\_\mathrm{HRCS}\_\mathrm{AHU}}=\frac{{\dot{m}}_{\mathrm{a}}(e{x}_4-e{x}_1)+{\dot{m}}_{\mathrm{a}}(e{x}_4-e{x}_6)}{{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{chc}}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})}.$$

(29)

The overall impact ratio for the cooling process is designed to present a system potential that combines the energetic analysis with exergetic analysis, as the ratio between the actual cooling energy output obtained for the air and water supplied and the total specific exergy consumed in a system, including the fan and pump exergy consumption. In general, the electric pump and fan energy is 100% of the exergy because the main energy source of the fan and pump is electricity [10]. The overall cooling impact ratio (CIR) is defined as follows:

$$CI{R}_{\mathrm{system}}=\frac{{\dot{Q}}_{\mathrm{acc}}}{e{x}_{\mathrm{e}.\mathrm{c}.}},$$

(30)

$$CI{R}_{\mathrm{AAS}}=\frac{{\dot{Q}}_{\mathrm{acc}\_\mathrm{AAS}}}{\begin{array}{c}{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{hc}\left(e{x}_{\mathrm{hot}.\sup }-e{x}_{\mathrm{hot}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{chc}}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})\\ +P_{\mathrm{fan}.\mathrm{AHU}}+P_{\mathrm{pump}.\mathrm{AHU}}\end{array}},$$

(31)

$$CI{R}_{\mathrm{CRCS}\_\mathrm{AHU}}=\frac{{\dot{Q}}_{\mathrm{acc}\_\mathrm{CRCS}\_\mathrm{AHU}}}{\begin{array}{c}{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{hc}}\left(e{x}_{\mathrm{hot}.\sup }-e{x}_{\mathrm{hot}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{chc}}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})\\ +P_{\mathrm{pump}.\mathrm{ceil}}+P_{\mathrm{fan}.\mathrm{AHU}}+P_{\mathrm{pump}.\mathrm{AHU}}\end{array}},$$

(32)

$$CI{R}_{\mathrm{HRCS}\_\mathrm{AHU}}=\frac{{\dot{Q}}_{\mathrm{acc}\_\mathrm{HRCS}\_\mathrm{AHU}}}{\begin{array}{c}{\dot{m}}_{\mathrm{cc}}\left(e{x}_{\mathrm{cool}.\sup }-e{x}_{\mathrm{cool}.\mathrm{re}}\right)+{\dot{m}}_{hc}\left(e{x}_{\mathrm{hot}.\sup }-E{x}_{\mathrm{hot}.\mathrm{re}}\right)+{\dot{m}}_{\mathrm{chc}}(e{x}_{\mathrm{ceil}.\sup }-e{x}_{\mathrm{ceil}.\mathrm{re}})\\ +P_{\mathrm{fan}.\mathrm{Airbox}}+P_{\mathrm{pump}.\mathrm{ceil}}+P_{\mathrm{fan}.\mathrm{AHU}}+P_{\mathrm{pump}.\mathrm{AHU}}\end{array}},$$

(33)

where ${\dot{m}}_{\mathrm{cc}}$ is the cooling coil mass flow rate in kg/h, ${\dot{m}}_{\mathrm{cc}}$ is the heating coil mass flow rate in kg/h, ${\dot{m}}_{\mathrm{chc}}$ is the chilled ceiling mass flow rate in kg/h, ${\dot{Q}}_{\mathrm{acc}}$ is the actual cooling capacity, kJ/h, ex_cool.sup is the specific exergy for cooling supply fluid in kJ/kg, ex_cool.re is the specific exergy for cooling return fluid in kJ/kg, ex_ceil.sup is the specific exergy for ceiling supply fluid in kJ/kg, ex_ceil.re is the specific exergy for ceiling return fluid in kJ/kg, P_pump is the pump electricity in kJ/h, and P_fan is the fan electricity in kJ/h.

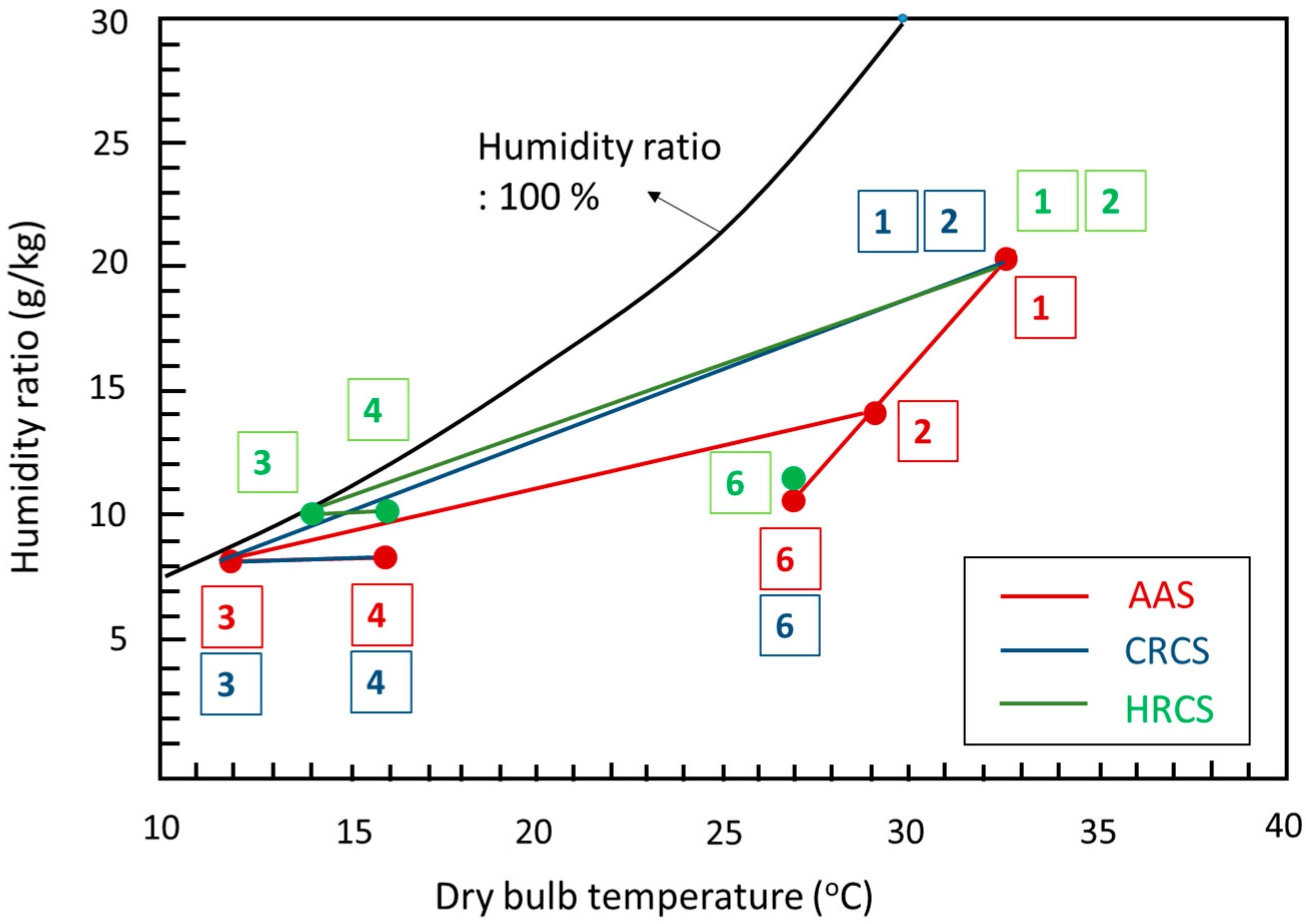
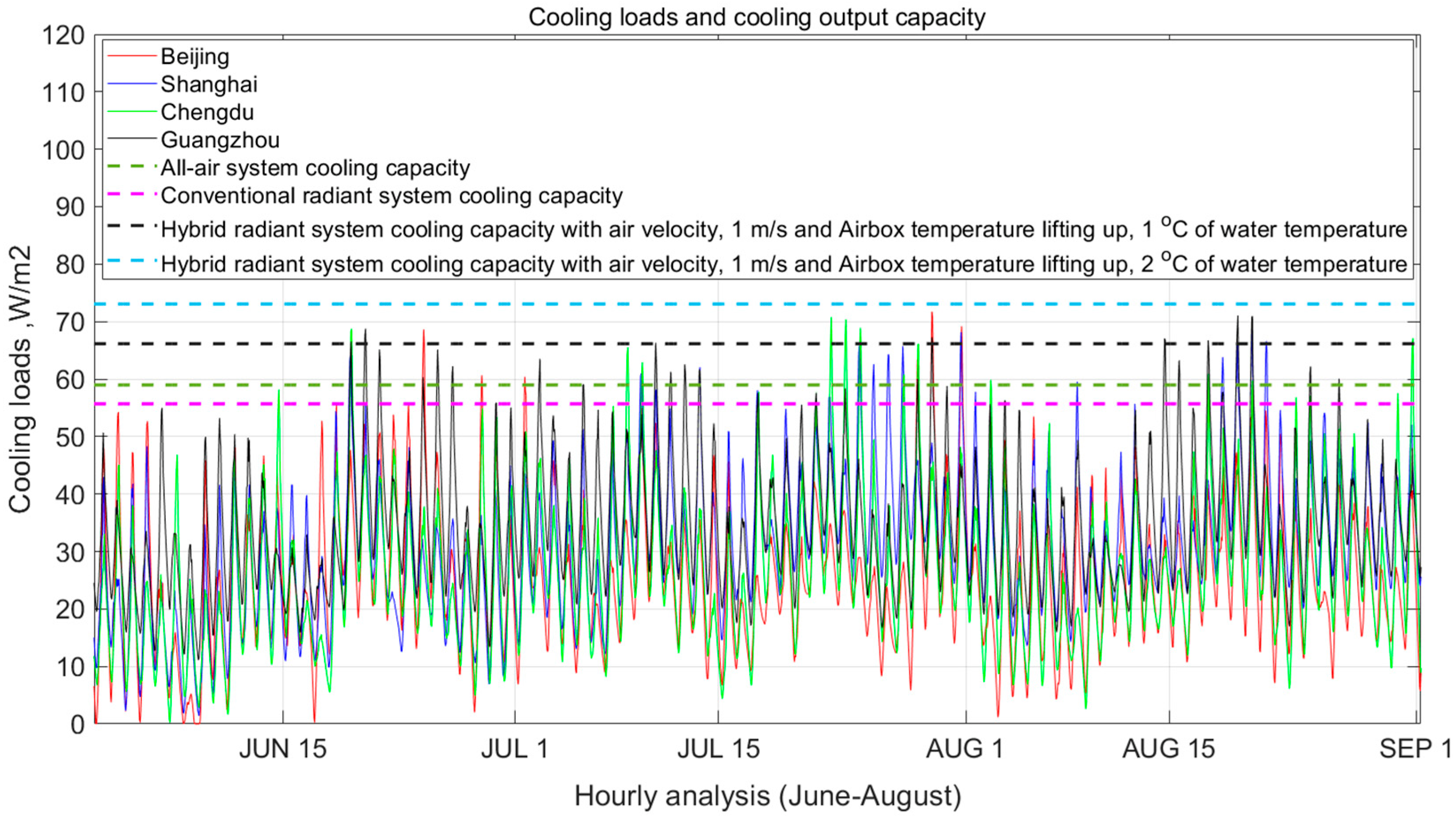
3. Results

3.1. Energy Performance of Systems

This study classified three main cooling systems and analyzed them numerically to evaluate the energy performance: an AAS, a CRCS with an AHU, and a HRCS with an AHU. Typically, an AAS uses a large amount air volume for cooling, and the air supplied reuses around 67% of the return air to reduce the cooling load. Compared to a typical radiant cooling system, an AAS consumes much volume space to supply a large air volume and has higher air pressure losses. A CRCS with an AHU can minimize the air volume supplied but also requires installing a hydronic pipe and a radiant panel. The HRCS connects a compact air convector to a radiant cooling panel hydronically in series. The convector can control the indoor air movement and the cooling capacity for the occupied zone efficiently. It can also reduce the condensation risks on the radiant panel surface and minimize the time delay in operating the chilled radiant cooling system in space.

This study used TRNSYS energy simulation software to analyze the cooling energy load in each building located in four main cities. Figure 7 presents the cooling load of the office room located in four cities in the summer season and the cooling capacities of the three systems. A CRCS has approximately the same cooling capacity as an AAS. However, it is limited to increasing the cooling output using lower-temperature supplied water under hot and humid surrounding conditions. The cooling capacities of an AAS and CRCS in 5–10 days of extreme weather conditions were insufficient to dominate the actual cooling loads; therefore, the systems needed additional cooling output to dominate the simulated cooling loads. Typically, an AAS can simply adjust the supplied airflow rate to increase the cooling output. However, a CRCS is limited to increasing the cooling output with lower temperature water supplied using a chilled radiant panel because the lower supplied water temperature causes moisture condensation risk on the surface of the radiant panel in an extreme hot and humid summer condition. The moisture condensation risk is the main limitation of the use of a chilled radiant cooling panel system in a hot and humid summer condition instead of using an AAS. However, the HRCS has a 20–30% relatively higher cooling capacity than the other two systems. The cooling output of the HRCS can be adjusted depending on the weather conditions using the heat exchanger capacity and fan speed of an airbox convector. The cooling output of the hybrid system, using the airbox increasing 1–2 °C of supplied water temperature and 1 m/s of air speed, is shown in Figure 7.

It can be concluded that the HRCS with an AHU adapted well to four different locations in the extreme hot and humid surrounding condition using even the same supply-water temperature, 18 °C, since the heat exchangers in the compact air convector and the mixed natural and mechanical forced convection effect enhanced the additional cooling potential outputs [43]. The occupants could simply turn on or turn off the airbox convector. Therefore, the indoor thermal comfort could be adjusted by the air convector’s distributed air movement and directivity.

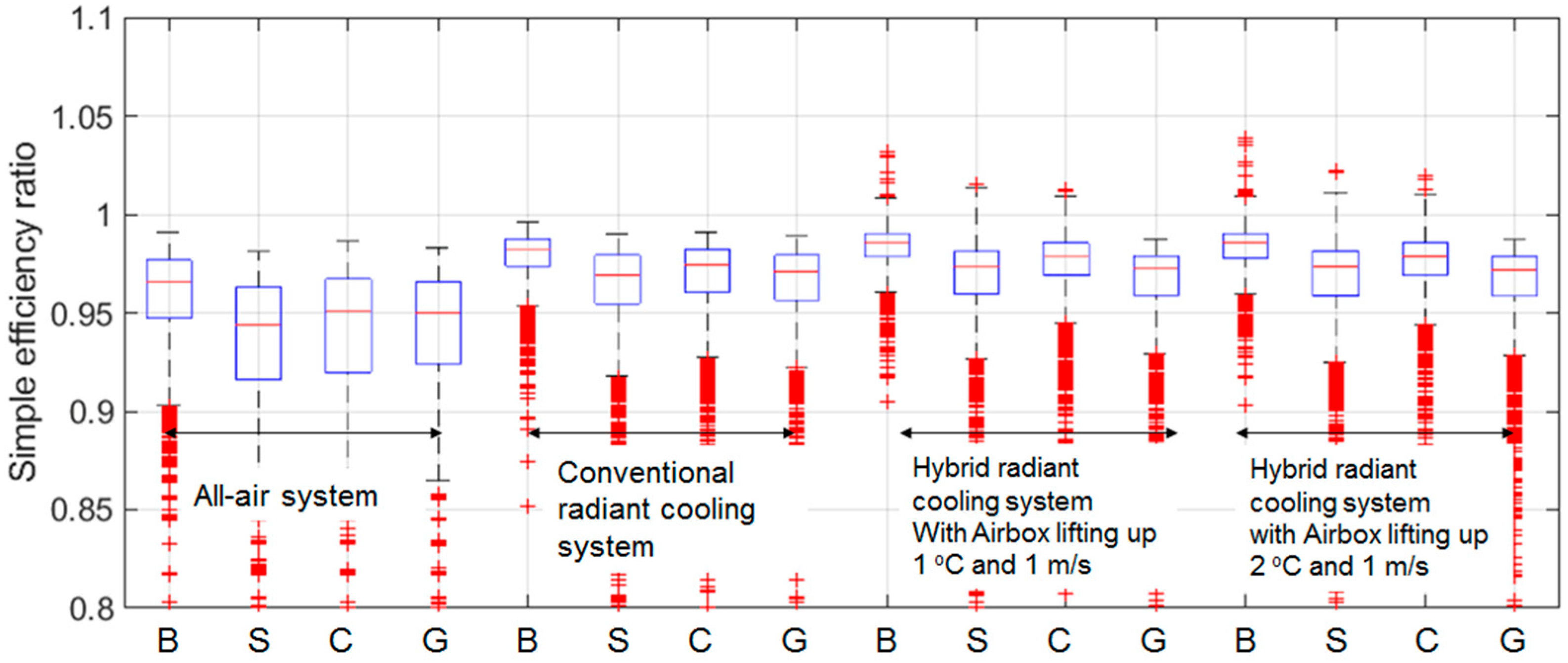
3.2. Exergy Analysis

In this study, the exergy performances of three cooling systems were analyzed and evaluated on the basis of the local historical hourly weather database of four cities in the summer season (June–August). The ambient environments are illustrated as shown in Figure 5 and Figure 6 to determine the exergy performance of three cooling systems. Two main exergy efficiencies and the simple and rational ratios of the three systems were calculated and compared. Figure 8 presents the simple exergy efficiency ratio. There are no significant differences among the results. However, the simple exergy efficiency ratios of the HRCS and the CRCS were 2.72% and 2.20% higher than those of the AAS. Specifically, the ratio had the highest value in Beijing and the lowest value in Guangzhou because the Guangzhou climate in the summer was much more challenging than the weather conditions of the other cities.

Figure 9 illustrates the rational exergy efficiencies of the three main systems. The HRCS with the AHU had the highest rational exergy efficiency compared with the other systems because the high-temperature cooling system maximized the actual exergy efficiency, especially in extreme weather conditions. The rational exergy efficiency ratio of the HRCS was 83%, 18% higher than the ratios of the AAS and the CRCS.

Moreover, the rational exergy efficiencies of the AAS were highly influenced by the ambient conditions compared with other radiant cooling systems, as shown in Figure 9. The maximum rational exergy efficiency in Beijing was much higher than that in Guangzhou, but the average value showed no significant differences because the temperature in Beijing deeply oscillates in the daytime and nighttime. Therefore, the ambient condition affected the efficiency of the AAS. It should be noted that the simple exergy efficiency ratio evaluates how close the process of the systems is to the ideal performance, and the rational exergy efficiency ratio presents how much potential the systems lose in generating specific outputs [56,60]. These two exergy efficiency ratios represent how the ambient environmental conditions affect the potentials of the systems.

Figure 10 presents the overall cooling impact ratios (CIRs) of the three systems between the actual obtained energetic cooling potentials of the three systems and the overall exergy consumed in the systems combined with the exergetic consumption of the fan and pump. We concluded that the HRCS had the highest cooling output and relatively low exergy consumptions with respect to the other two systems. The overall CIR of the HRCS, with an airbox convector increasing 2 °C of the temperature and 1 m/s of the air velocity, was approximately 185% higher than that of the AAS, 8.5% higher than that of the CRCS, and 2% higher than that of the HRCS increasing 1 °C of the temperature and 1 m/s of the air velocity.

Figure 11 illustrates the overall CIR using the weather conditions of four cities, Beijing, Shanghai, Chengdu, and Guangzhou. The high temperature and high humidity ratio of the ambient conditions affected the high exergy consumption. Therefore, these factors reduced the overall CIR. The impact ratio in Beijing had the highest value. The results varied more dramatically due to the high temperature differences between daytime and nighttime. However, the results at Guangzhou show that the relatively lower temperature differences between daytime and nighttime compared with other cities had less of an influence on the variation of the CIR results. The HRCS showed strong performance for the overall weather conditions compared with the other systems. It could maximize the potential for mild conditions with a relatively lower temperature and humidity ratio difference between the ambient and indoor conditions.

These numerical energetic and exergetic results indicate that the HRCS system with an AHU had better performance compared to the three cooling systems adapting to hot and humid conditions. This is because the HRCS could produce additional cooling output with a compact air convector and simply adjust the indoor thermal condition and humidity ratio using the airbox convector when a space had extra humidity gains. The low-exergy/high-temperature cooling source could improve the system performance and minimize the exergy destruction.

However, this study also had some limitations. The application potential of the radiant cooling systems is affected by different climatic conditions [61]. Therefore, the meteorological parameters in severe cold, cold, hot summer and cold winter, hot summer and warm winter, and temperate climatic zones should be evaluated considering the control strategies. Moreover, this study suggested an airbox convector to minimize moisture condensation risks on the surface of radiant cooling panels and to adjust airflow rates depending on the indoor thermal condition. However, the control strategy needs to be verified via experimental analysis [62,63]. The total energy and exergy analysis should also consider the initial construction and operating cost.

Nevertheless, further studies should consider evaluating the performance with actual experimental results to launch the system in extreme weather conditions successfully. Additionally, the thermal comfort in a room should be investigated using different cooling systems to verify the occupants’ satisfaction in the future.

4. Conclusions

This study explored an energetic and exergetic analysis to evaluate the performance of three main air-cooling systems (AAS, CRCS, and HRCS) in an office building adapted to hot and humid summer conditions in four main cities in China: Beijing, Shanghai, Chengdu, and Guangzhou. Compared with a CRCS, the HRCS with an AHU could generate higher cooling outputs with an air convector due to an enhanced natural and mechanical forced convection effect. The system could minimize exergy losses in the process involved with cooling in buildings. This study found that the HRCS had a higher overall impact ratio, and that lower temperature differences could minimize exergy destruction in the process of cooling and dehumidification. A summary of this study is described below.

• In the hot and humid summer season, the HRCS was the most efficient cooling strategy due to the extra cooling output provided by the compact convector and the relatively low exergy destruction in the cooling and dehumidification process with higher-temperature cooling sources.
• The HRCS released an additional 20–30% of cooling output, and it could adapt well in extreme hot and humid weather conditions.
• The comparison analysis using the four different weather datasets showed simple and rational exergy efficiency; as a result, the ambient condition, temperature, and humidity ratio significantly impacted the exergy efficiency ratio.
• The overall CIR of the HRCS with an airbox convector was approximately 185% higher than that of the AAS and 8.5% higher than that of the CRCS.
• The HRCS presented the most efficient characteristic in reducing the environmental impact and increasing the benefits compared with the AAS and CRCS in hot and humid summer conditions.