Investigation of the Effect of Air Layer Thickness on the Thermal Performance of the PCM Integrated Roof
Abstract
:1. Introduction
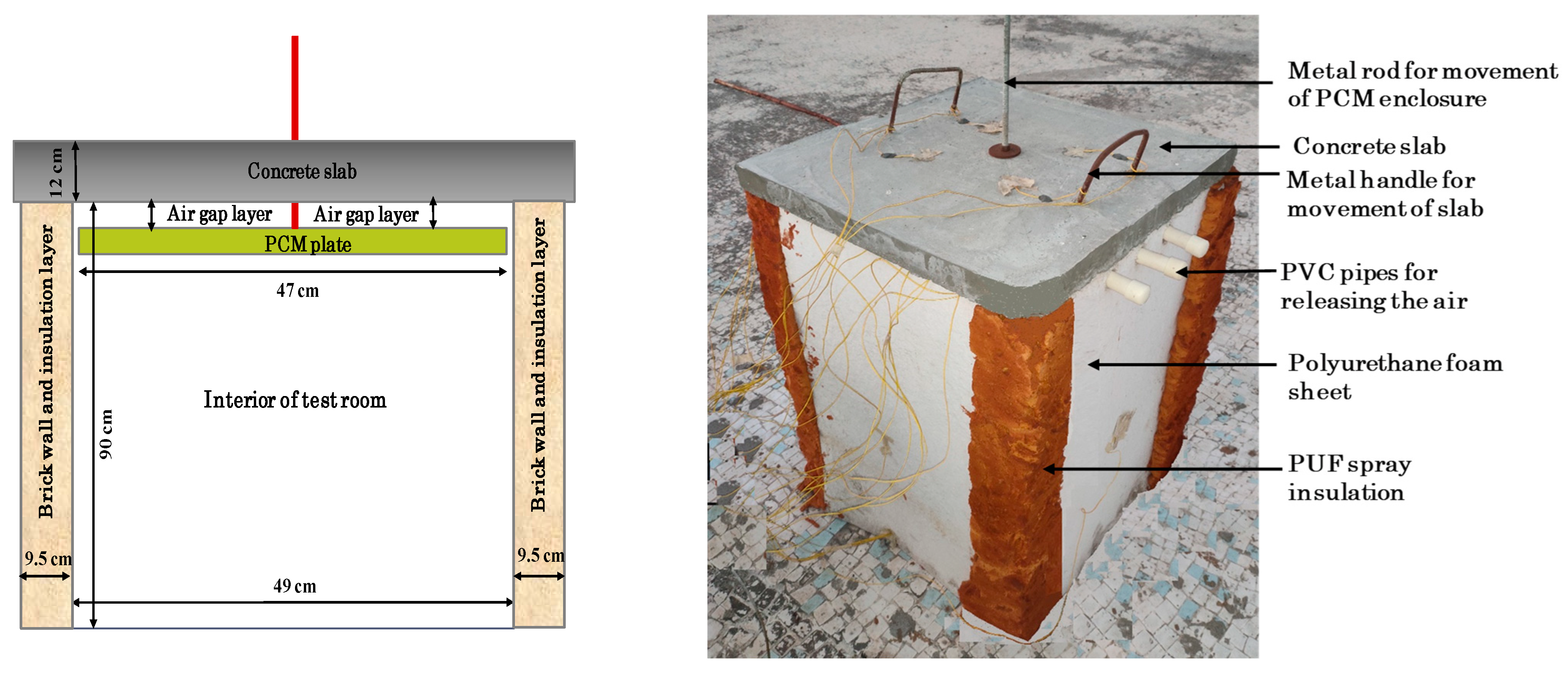
2. Experimental Setup
2.1. Materials and Testing
2.2. Instrumentation and Measurement
2.3. Climatic Data Deduction
3. Results and Discussion
Effect on Room Temperature Variation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| f | Time lag (hr) |
| Dimensionless time lag | |
| Fsky | Shape factor for horizontal surface (=1 for the rooftop slab and sky) |
| h | Heat transfer coefficient, (W/m2 K) |
| I | Solar radiation, (W/m2) |
| PCM | Phase Change Materials |
| q | Heat flux, (W/m2) |
| Q | Solar heat gain, (W/m2) |
| T | Temperature, (K) |
| V | Velocity, (m/s) |
| Vo | Volume, (m3) |
| Greek Letters | |
| α | |
| ε | Emissivity of rooftop slab |
| ϕ | Decrement factor |
| Dimensionless decrement factor | |
| σ | Stefan Boltzmann constant, (W/m2 K4) |
| Subscript | |
| B | Brick |
| C | Concrete |
| combined | Combined radiative and convective conditions |
| ceiling | Ceiling conditions |
| d | Direct solar radiation |
| i | Indoor conditions |
| o | Ambient or outdoor conditions |
| P | PCM |
| roof | Roof conditions |
| sky | Clear sky conditions |
| sol-air | Solar air |
Appendix A


References
- International Energy Agency. CO2 Emissions From Fuel Combustion: Highlights; IEA Publication: Paris, France, 2012. [Google Scholar]
- 2013 April. Annual Energy Outlook 2013 with Projections to 2040; Energy Information Administration: Washington, DC, USA, 2013.
- Lei, J.; Yang, J.; Yang, E.-H. Energy performance of building envelopes integrated with phase change materials for cooling load reduction in tropical Singapore. Appl. Energy 2016, 162, 207–217. [Google Scholar] [CrossRef]
- Dehkordi, B.S.; Afrand, M. Energy-saving owing to using PCM into buildings: Considering of hot and cold climate region. Sustain. Energy Technol. Assess. 2022, 52, 102112. [Google Scholar]
- BBland, A.; Khzouz, M.; Statheros, T.; Gkanas, E.I. PCMs for Residential Building Applications: A Short Review Focused on Disadvantages and Proposals for Future Development. Buildings 2017, 7, 78. [Google Scholar] [CrossRef]
- Al-Sanea, S.A.; Zedan, M.; Al-Ajlan, S.A.; Hadi, A.S.A. Heat Transfer Characteristics and Optimum Insulation Thickness for Cavity Walls. J. Therm. Envel. Build. Sci. 2003, 26, 285–307. [Google Scholar] [CrossRef]
- Jamal, B.; Boukendil, M.; Abdelbaki, A.; Zrikem, Z. Numerical simulation of coupled heat transfer through double solid walls separated by an air layer. Int. J. Therm. Sci. 2020, 156, 106461. [Google Scholar] [CrossRef]
- Mahlia, T.; Iqbal, A. Cost benefits analysis and emission reductions of optimum thickness and air gaps for selected insulation materials for building walls in Maldives. Energy 2010, 35, 2242–2250. [Google Scholar] [CrossRef]
- Mavromatidis, L.E.; Bykalyuk, A.; El Mankibi, M.; Michel, P.; Santamouris, M. Numerical estimation of air gaps’ influence on the insulating performance of multilayer thermal insulation. Build. Environ. 2012, 49, 227–237. [Google Scholar] [CrossRef]
- Kurt, H. The usage of air gap in the composite wall for energy saving and air pollution. Environ. Prog. Sustain. Energy 2010, 30, 450–458. [Google Scholar] [CrossRef]
- Bhamare, D.K.; Rathod, M.K.; Banerjee, J. Passive cooling techniques for building and their applicability in different climatic zones—The state of art. Energy Build. 2019, 198, 467–490. [Google Scholar] [CrossRef]
- Rathod, M.K.; Banerjee, J. Thermal stability of phase change materials used in latent heat energy storage systems: A review. Renew. Sustain. Energy Rev. 2013, 18, 246–258. [Google Scholar] [CrossRef]
- Saffari, M.; Roe, C.; Finn, D.P. Improving the building energy flexibility using PCM-enhanced envelopes. Appl. Therm. Eng. 2022, 217, 119092. [Google Scholar] [CrossRef]
- Ascione, F.; Bianco, N.; De Masi, R.F.; de’ Rossi, F.; Vanoli, G.P. Energy refurbishment of existing buildings through the use of phase change materials: Energy savings and indoor comfort in the cooling season. Appl. Energy 2014, 113, 990–1007. [Google Scholar] [CrossRef]
- Alshuraiaan, B. Efficient utilization of PCM in building envelope in a hot environment condition. Int. J. Thermofluids 2022, 16, 100205. [Google Scholar] [CrossRef]
- Li, D.; Zheng, Y.; Liu, C.; Wu, G. Numerical analysis on thermal performance of roof contained PCM of a single residential building. Energy Convers. Manag. 2015, 100, 147–156. [Google Scholar] [CrossRef]
- Tokuç, A.; Başaran, T.; Yesügey, S.C. An experimental and numerical investigation on the use of phase change materials in building elements: The case of a flat roof in Istanbul. Energy Build. 2015, 102, 91–104. [Google Scholar] [CrossRef]
- Saffari, M.; de Gracia, A.; Ushak, S.; Cabeza, L.F. Economic impact of integrating PCM as passive system in buildings using Finger comfort model. Energy Build. 2016, 112, 159–172. [Google Scholar] [CrossRef]
- Singh, S.; Bhat, V. Performance evaluation of dual phase change material gypsum board for the reduction of temperature swings in a building prototype in composite climate. Energy Build. 2018, 159, 191–200. [Google Scholar] [CrossRef]
- Arıcı, M.; Bilgin, F.; Nižetić, S.; Karabay, H. PCM integrated to external building walls: An optimization study on maximum activation of latent heat. Appl. Therm. Eng. 2019, 165, 114560. [Google Scholar] [CrossRef]
- Jin, X.; Medina, M.; Zhang, X. On the importance of the location of PCMs in building walls for enhanced thermal performance. Appl. Energy 2013, 106, 72–78. [Google Scholar] [CrossRef]
- Khedher, B. Numerical Study of the Thermal Behavior of a Composite Phase Change Material (PCM) Room. Eng. Technol. Appl. Sci. Res. 2018, 8, 2663–2667. [Google Scholar] [CrossRef]
- Lee, K.O.; Medina, M.A.; Raith, E.; Sun, X. Assessing the integration of a thin phase change material (PCM) layer in a residential building wall for heat transfer reduction and management. Appl. Energy 2015, 137, 699–706. [Google Scholar] [CrossRef]
- Lagou, A.; Kylili, A.; Šadauskienė, J.; Fokaides, P.A. Numerical investigation of phase change materials (PCM) optimal melting properties and position in building elements under diverse conditions. Constr. Build. Mater. 2019, 225, 452–464. [Google Scholar] [CrossRef]
- Pasupathy, A.; Velraj, R. Effect of double layer phase change material in building roof for year round thermal management. Energy Build. 2007, 40, 193–203. [Google Scholar] [CrossRef]
- Jin, X.; Zhang, X. Thermal analysis of a double layer phase change material floor. Appl. Therm. Eng. 2011, 31, 1576–1581. [Google Scholar] [CrossRef]
- Bhamare, D.K.; Rathod, M.K.; Banerjee, J. Numerical model for evaluating thermal performance of residential building roof integrated with inclined phase change material (PCM) layer. J. Build. Eng. 2019, 28, 101018. [Google Scholar] [CrossRef]
- Bhamare, D.K.; Rathod, M.K.; Banerjee, J. Proposal of a unique index for selection of optimum phase change material for effective thermal performance of a building envelope. Sol. Energy 2021, 218, 129–141. [Google Scholar] [CrossRef]
- Available online: https://www.pluss.co.in/technical-datasheets/Doc304-TDS-HS-29.pdf (accessed on 7 February 2023).
- Tchinda, R. Thermal behaviour of solar air heater with compound parabolic concentrator. Energy Convers. Manag. 2008, 49, 529–540. [Google Scholar] [CrossRef]
- EnergyPlus, Engineering References. 2013. Available online: http://apps1.eere.energy.gov/buildings/energyplus/energyplus_documentation.cfm (accessed on 7 February 2023).
- Bhamare, D.K.; Rathod, M.K.; Banerjee, J. Selection of phase change material and establishment of thermophysical properties of phase change material integrated with roof of a building using Measure of Key Response index: Proposal of a new parameter. J. Energy Storage 2020, 32, 101812. [Google Scholar] [CrossRef]
- Kong, X.; Lu, S.; Li, Y.; Huang, J.; Liu, S. Numerical study on the thermal performance of building wall and roof incorporating phase change material panel for passive cooling application. Energy Build. 2014, 81, 404–415. [Google Scholar] [CrossRef]



| Authors | PCM Used in | Climatic Conditions | Parameters Studied | Remarks |
|---|---|---|---|---|
| Ascione et al. [14] | wall | Five Mediterranean climatic cities | PCM layer thickness | Maximum cooling energy savings are attained for a PCM plaster of 3 cm thickness. |
| Li et al. [16] | Roof | Northeast and cold regions of China | Increasing PCM layer thickness decreases the peak time of the average temperature of a base layer and upper surface heat flux. | |
| Tokuc et al. [17] | Roof | Mediterranean climate of İstanbul, Turkey | Optimum PCM layer thickness is suitable for achieving energy conservation in flat roofs of the city of Istanbul. | |
| Saffari et al. [18] | Wall | Warm and temperate Climate of the city of Madrid | PCM layer thickness of 10 mm provides greater energy savings and a payback period. | |
| Singh and Bhat [19] | Roof | Hot and dry climate of the city of Indore, India | Optimum PCM layer thickness of 16 mm can reduce the cooling load in the building. | |
| Arici et al. [20] | Wall | Erzurum, Diyarbakır, and Konya cities, Turkey. | Optimum PCM layer thickness varies from 1 to 20 mm depending on the climatic zone. | |
| Jin et al. [21] | Wall | Dynamic wall simulator | PCM layer location | Optimal position of the PCM layer is approximately 0.2 times the total length of the wall from the internal surface of the wall. The optimal position of the PCM layer shows 41% of peak heat flux reduction. |
| Nidhal Ben Khedher [22] | Wall | Tropical climate of Tunisia | The PCM n-octadecane performs best for maintaining an indoor temperature close to 27 °C for the test room. | |
| Lee et al. [23] | Wall | Warm and temperate Climate of the city of Lawrence, KS, USA | Optimal PCM location on the south wall is 2.54 cm, whereas, for a west wall, it is 1.27 cm from the location of the wallboard. Peak heat flux reduction for these optimal locations is 29.7% and 51.3% for the west and south, respectively. | |
| Lagou et al. [24] | Wall | Southern, central, and northern European climatic conditions | PCM layer placement should be on the inside of the building for yearly energy saving. | |
| Pasupathy and Velraj [25] | Roof | Hot and humid climate of the city of Chennai, India | Single and double-layer PCM | Double-layer PCM narrows indoor temperature swings. |
| Jin and Zhang [26] | Floor | Humid subtropical climate of the city of Nanjing, China | PCM layer should be located near the cold source and floor surface in the case of the cooling system and heating system, respectively. | |
| Bhamare et al. [27] | Roof | Hot and humid climate of the city of Chennai, India | PCM layer Inclination | Maximum reduction in heat gain savings is obtained for a PCM slab inclination of 2°. |
| ƒ (hr) | ϕ | (Wh/m2) | ƒ′ | ϕ′ | MKR Index | ||
|---|---|---|---|---|---|---|---|
| Non-PCM | 1.50 | 0.87 | 2211.54 | - | - | - | - |
| PCM with 0 cm air layer | 2.00 | 0.36 | 599.27 | 1.33 | 0.41 | 0.27 | 5.40 |
| PCM with 2 cm air layer | 3.00 | 0.35 | 557.45 | 2.00 | 0.40 | 0.25 | 8.83 |
| PCM with 4 cm air layer | 2.00 | 0.38 | 622.89 | 1.33 | 0.43 | 0.28 | 5.07 |
| PCM with 6 cm air layer | 2.00 | 0.36 | 628.09 | 1.33 | 0.41 | 0.28 | 5.17 |
| Configuration | (W hr/m2) | (W hr/m2) | (%) | |
|---|---|---|---|---|
| PCM with 0 cm air layer | 599.27 | 2211.54 | 0.27 | 73 |
| PCM with 2 cm air layer | 557.45 | 2211.54 | 0.25 | 75 |
| PCM with 4 cm air layer | 622.89 | 2211.54 | 0.28 | 72 |
| PCM with 6 cm air layer | 628.09 | 2211.54 | 0.28 | 72 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhamare, D.K.; Rathod, M.K.; Banerjee, J.; Arıcı, M. Investigation of the Effect of Air Layer Thickness on the Thermal Performance of the PCM Integrated Roof. Buildings 2023, 13, 488. https://doi.org/10.3390/buildings13020488
Bhamare DK, Rathod MK, Banerjee J, Arıcı M. Investigation of the Effect of Air Layer Thickness on the Thermal Performance of the PCM Integrated Roof. Buildings. 2023; 13(2):488. https://doi.org/10.3390/buildings13020488
Chicago/Turabian StyleBhamare, Dnyandip K., Manish K. Rathod, Jyotirmay Banerjee, and Müslüm Arıcı. 2023. "Investigation of the Effect of Air Layer Thickness on the Thermal Performance of the PCM Integrated Roof" Buildings 13, no. 2: 488. https://doi.org/10.3390/buildings13020488
APA StyleBhamare, D. K., Rathod, M. K., Banerjee, J., & Arıcı, M. (2023). Investigation of the Effect of Air Layer Thickness on the Thermal Performance of the PCM Integrated Roof. Buildings, 13(2), 488. https://doi.org/10.3390/buildings13020488








