Algorithm-Aided Design for Composite Bridges
Abstract
1. Introduction
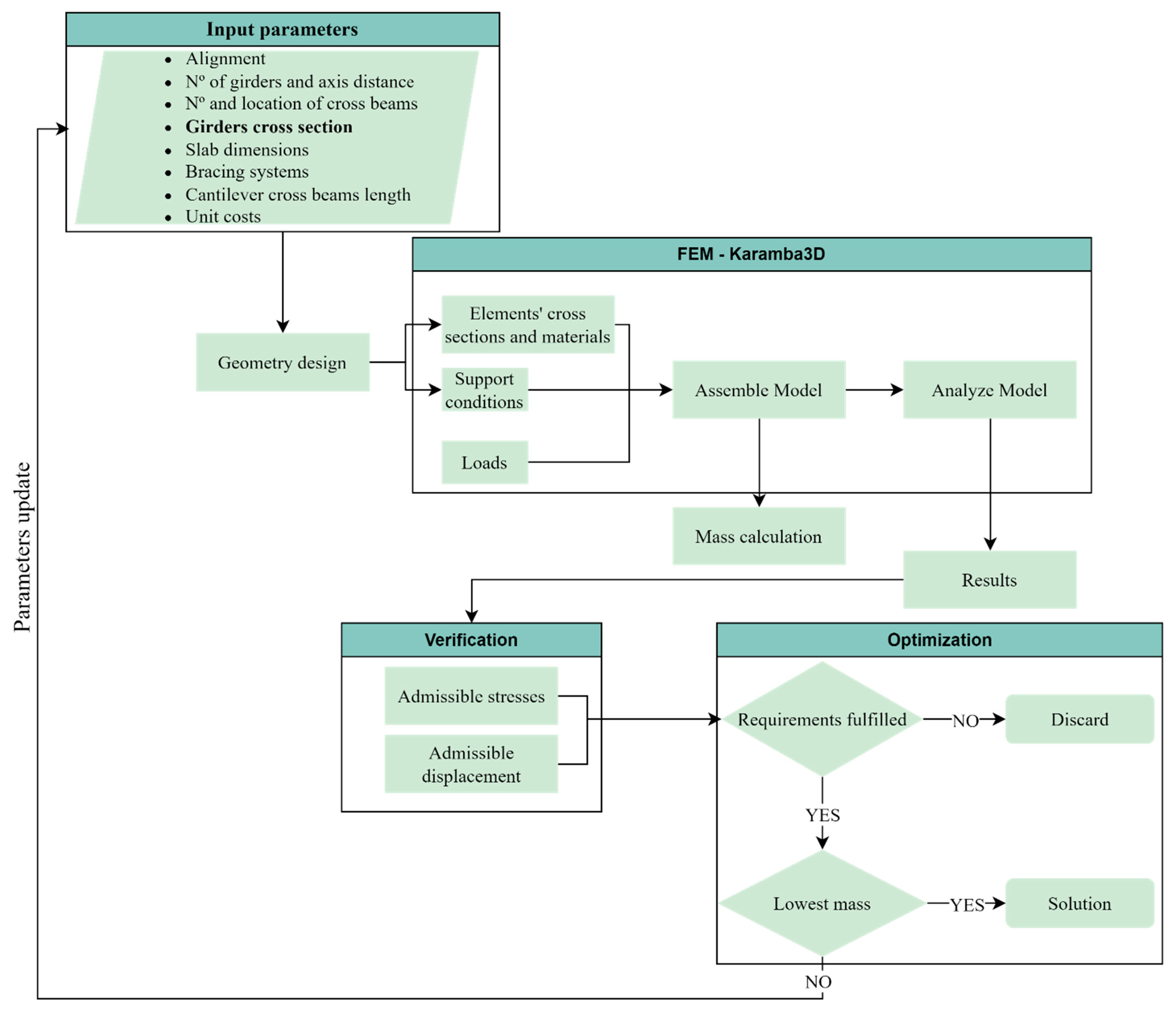
2. Methodology and Model Development
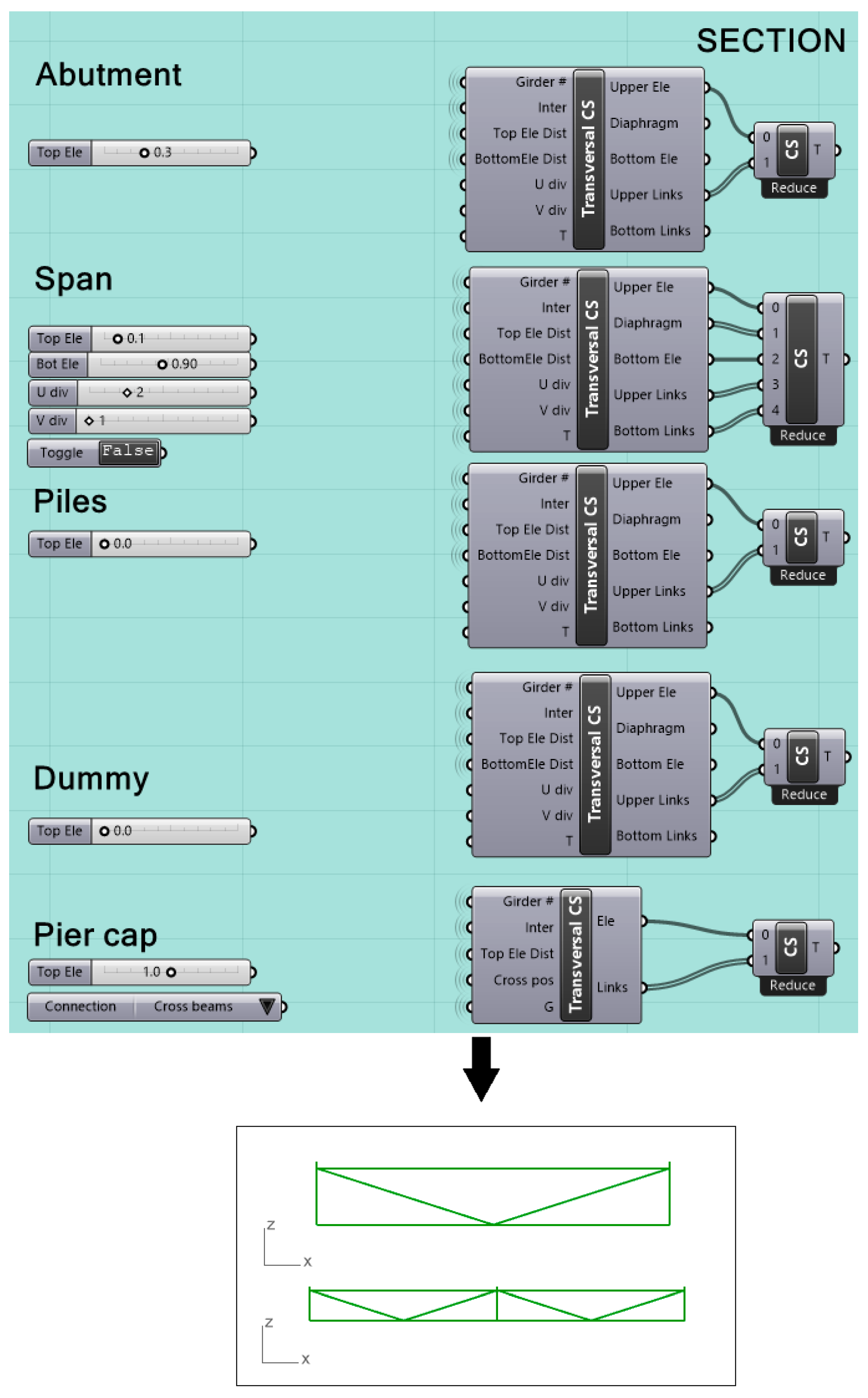
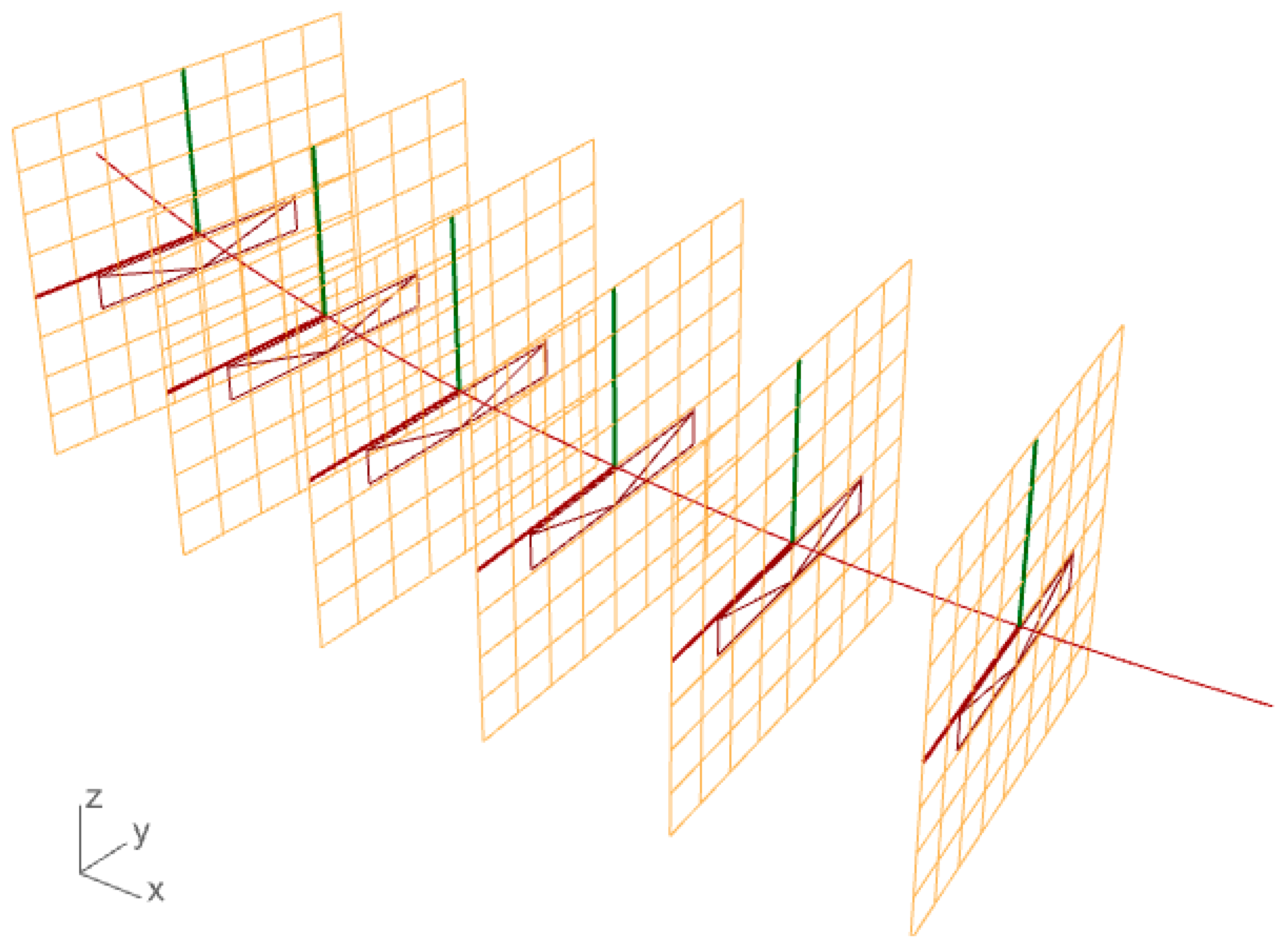
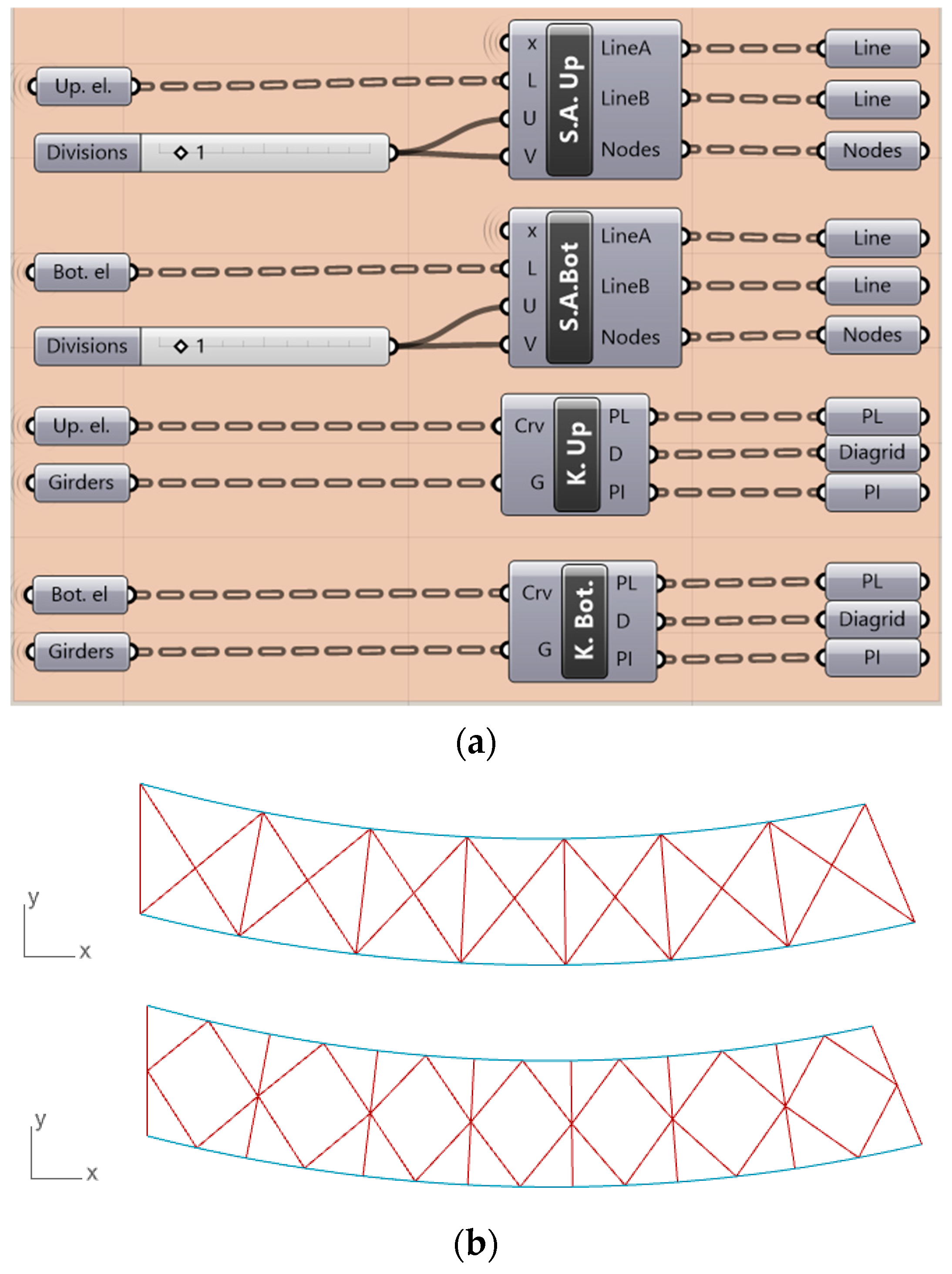
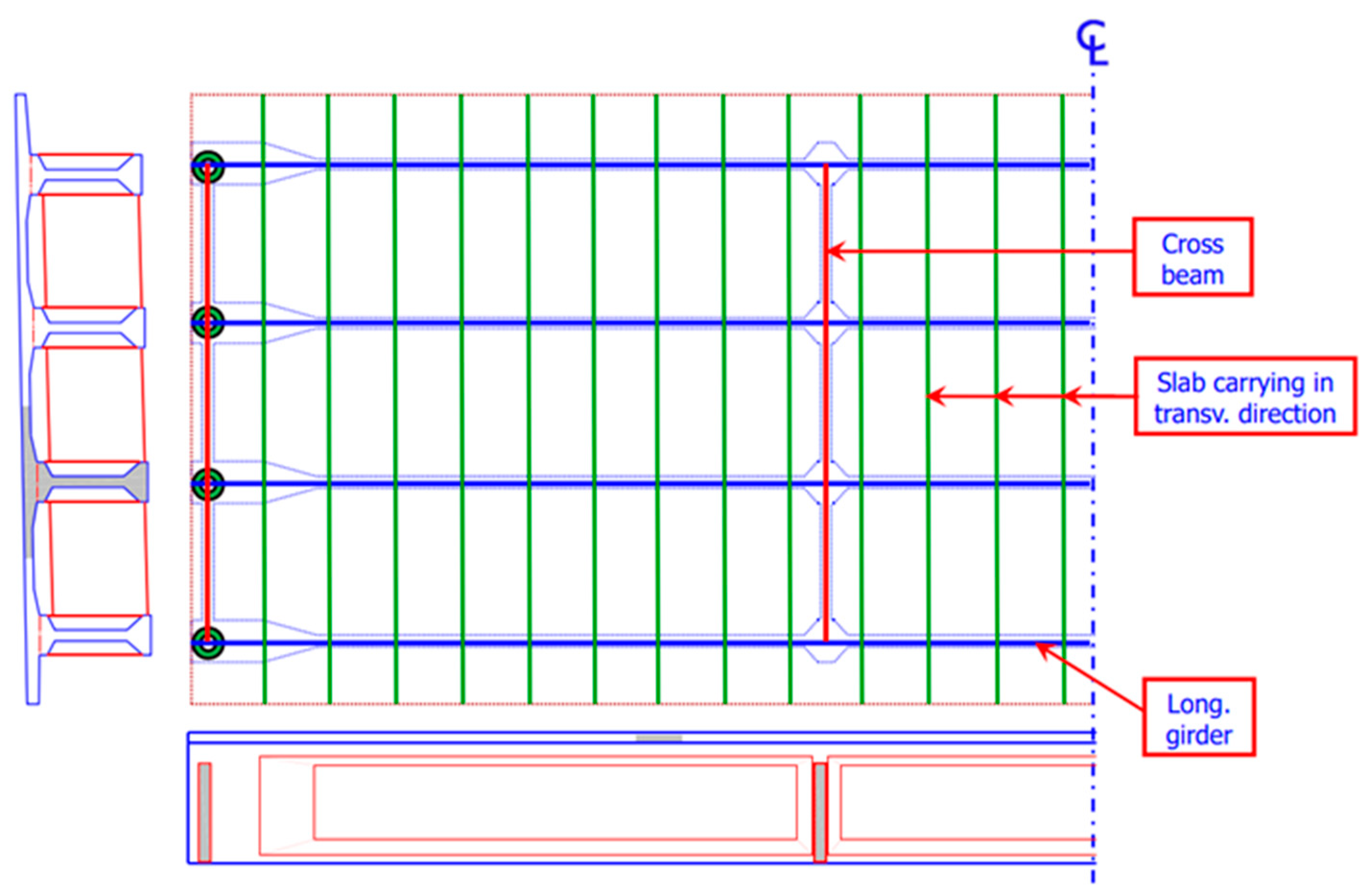
2.1. Parametric Design: Geometry Development
- Bridge alignment;
- Number of girders and axis distance;
- Number and location of cross-beams;
- Girder cross-section;
- Slab dimensions;
- Bracing systems, axis distance, and vertical position;
- Cantilever cross-beams length.
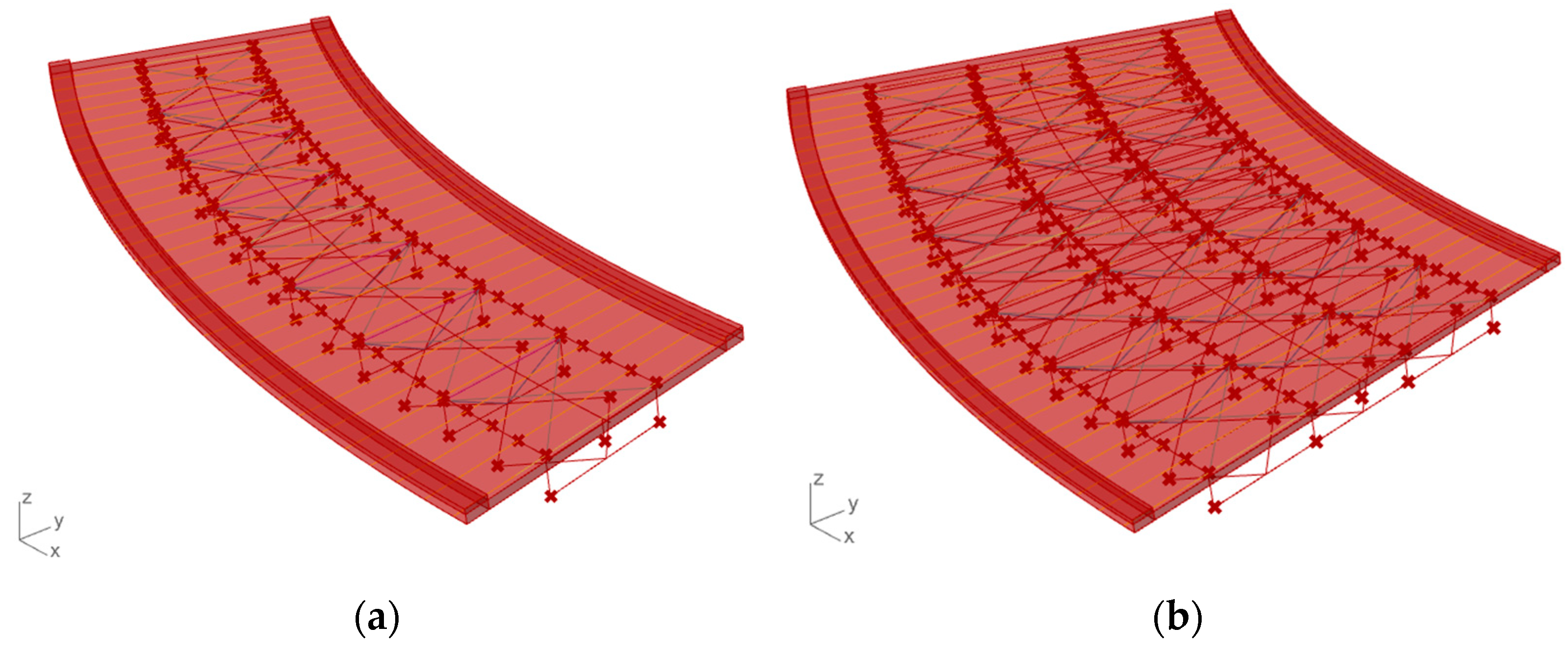
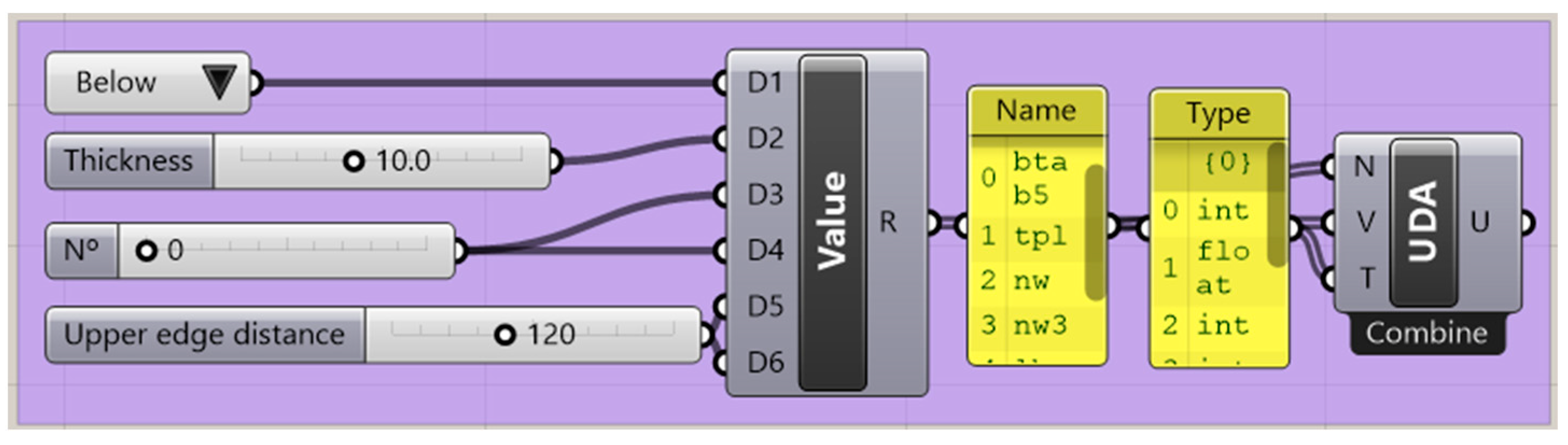
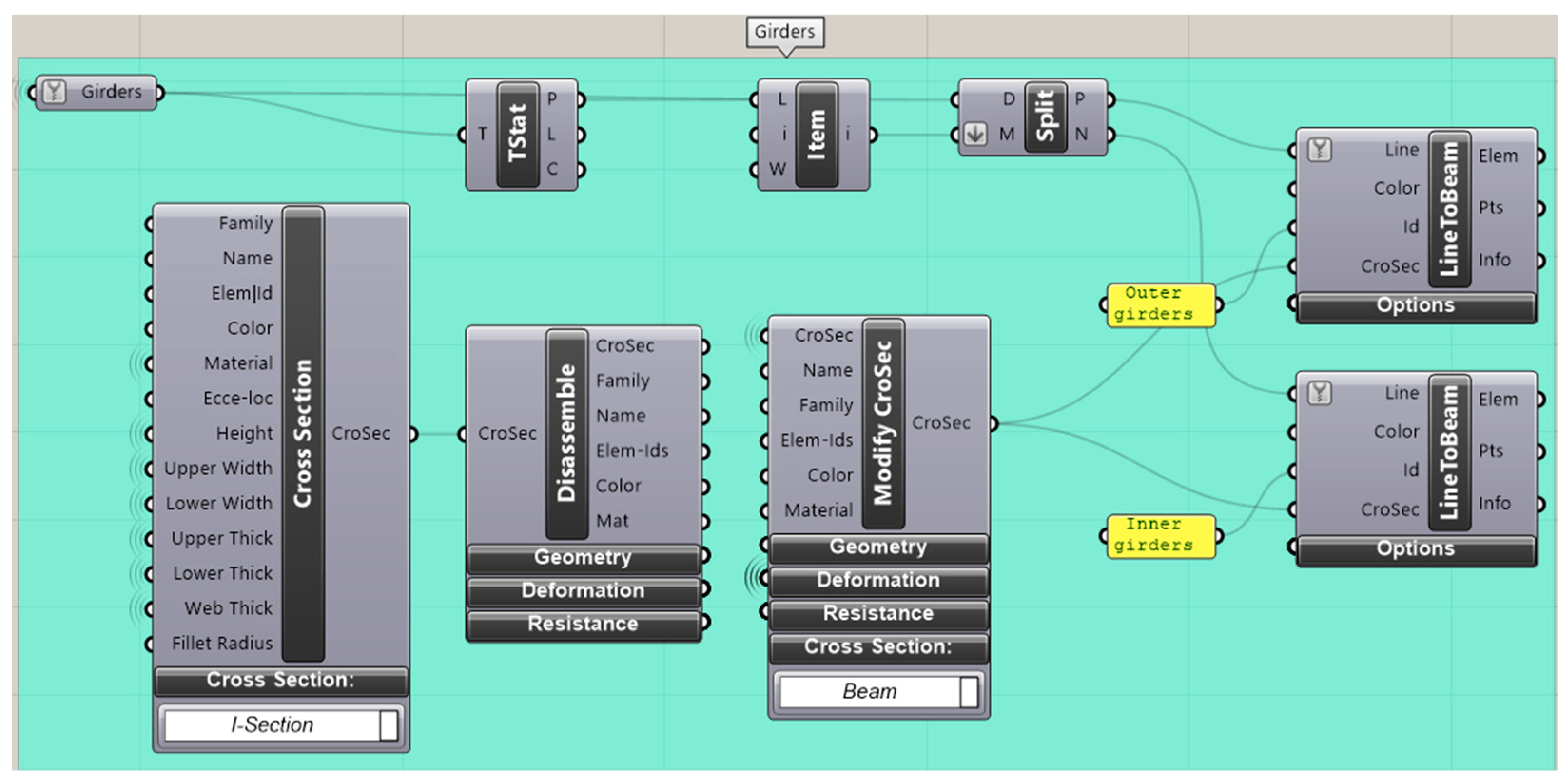
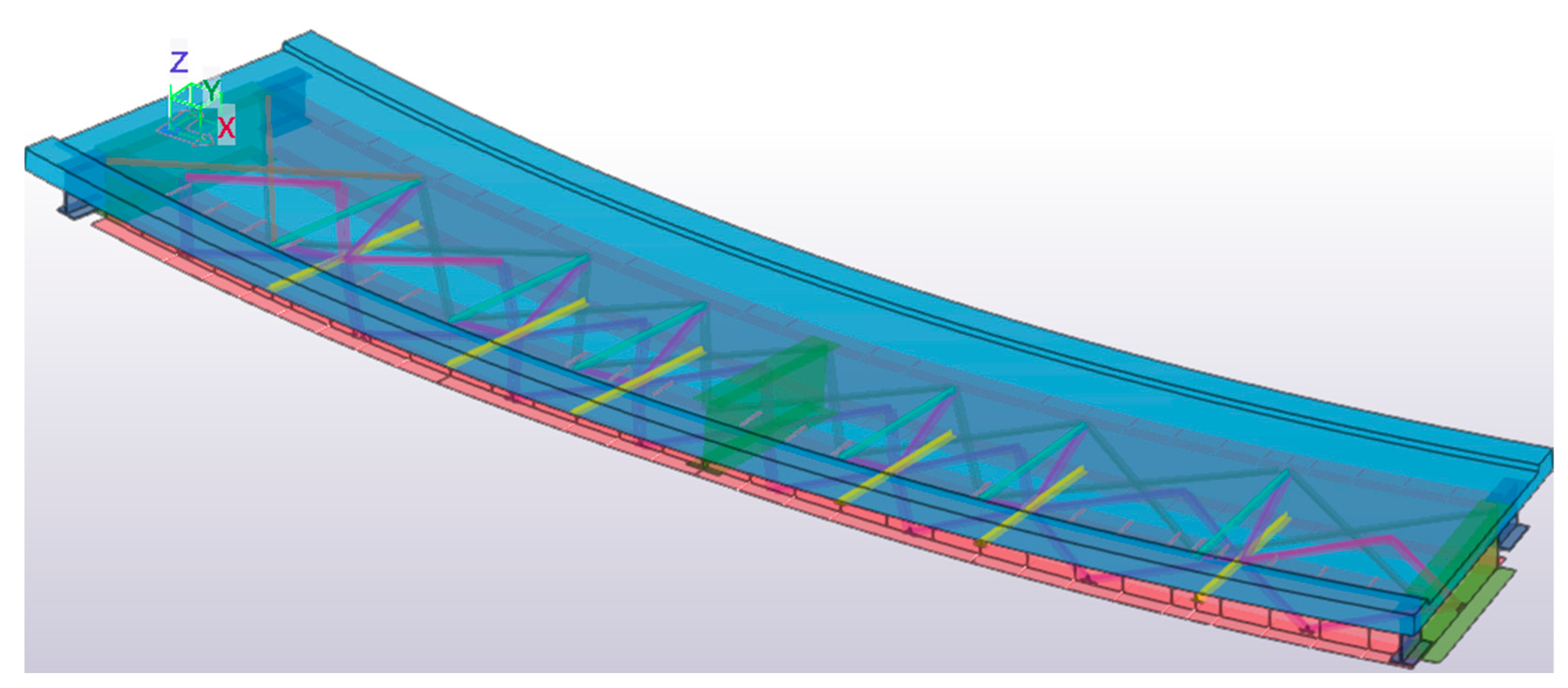
2.2. Three-Dimensional Modelling for FEA Development
2.3. Structural Model: Finite Element Analysis Development
3. Optimization Strategy
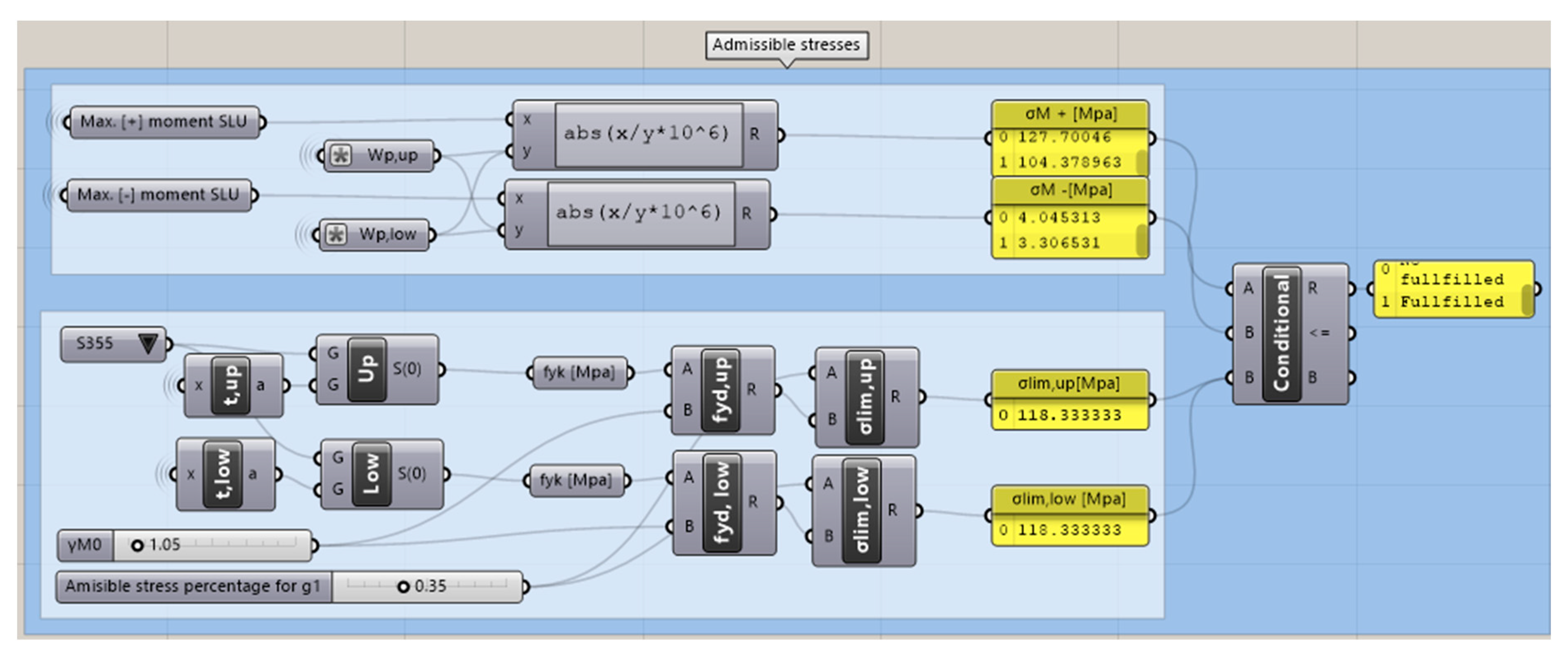
- Flanges’ admissible stress under permanent loads;
- Maximum displacement allowed.
4. Optimal Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Azhar, S.; Nadeem, A.; Mok, J.Y.; Leung, B.H. Building Information Modeling (BIM): A new paradigm for visual interactive modeling and simulation for construction projects. In Proceedings of the First International Conference on Construction in Developing Countries, Loughborough, UK, 14–16 May 2008; pp. 435–446. [Google Scholar]
- Svoboda, L.; Novák, J.; Kurilla, L.; Zeman, J. A framework for integrated design of algorithmic architectural forms. Adv. Eng. Softw. 2014, 72, 109–118. [Google Scholar] [CrossRef]
- Sardone, L.; Greco, R.; Fiore, A.; Moccia, C.; De Tommasi, D.; Lagaros, N.D. A preliminary study on a variable section beam through Algorithm-Aided Design: A way to connect architectural shape and structural optimization. Procedia Manuf. 2020, 44, 497–504. [Google Scholar] [CrossRef]
- Frangedaki, E.; Sardone, L.; Marano, G.C.; Lagaros, N.D. Optimisation-driven design in the architectural, engineering and construction industry. Proc. ICE Struct. Build. 2023, 0, 1–12. [Google Scholar] [CrossRef]
- Girardet, A.; Boton, C. A parametric BIM approach to foster bridge project design and analysis. Autom. Constr. 2021, 126, 103679. [Google Scholar] [CrossRef]
- Konia Company Ltd. Overcoming Complexity and Driving Efficiency in Bridge Design with Parametric Design. Available online: Storekonia.com (accessed on 21 November 2022).
- Ji, Y.; Beetz, J.; Bonsma, P.; Nisbet, N.; Katz, C.; Borrmann, A. Integration of Parametric Geometry into IFC-Bridge. In Proceedings of the 23rd European Conference Forum Bauinformatik, Cork, Ireland, 12–14 September 2011. [Google Scholar]
- Havelia, P.A. Ground Structure Method to Optimize Topology and Sizing of Steel Frame Structures to Minimize Material, Fabrication and Erection Cost. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2016. [Google Scholar]
- Sardone, L.; Fiore, A.; Manuello, A.; Greco, R. Performative Structural Design Optimization: Generative Algorithm for a Preliminary Study of a Voided Beam. Appl. Sci. 2022, 12, 8663. [Google Scholar] [CrossRef]
- Frangedaki, E.; Sardone, L.; Lagaros, N.D. Design Optimization of Tree-Shaped Structural Systems and Sustainable Architecture Using Bamboo and Earthen Materials. J. Archit. Eng. 2021, 27, 04021033. [Google Scholar] [CrossRef]
- Anwar, N. The Impact and Future Role of Computations and Software in Bridge Modeling, Analysis and Design. In Proceedings of the China Bridge Congress Chongqing, Chongqing, China, 29 March 2007. [Google Scholar]
- Giordano, L. Girder bridges, Bridge construction and design. PowerPoint slides for PoliTo Courses, DISEG. 2021. Available online: https://file.didattica.polito.it/download/MATDID/33329945 (accessed on 10 January 2023).
- Sardone, L.; Fiore, A.; Greco, R.; Moccia, C.; Lagaros, N.D.; De Tommasi, D. Algorithm-aided structural-optimization strategies for the design of variable cross-section beams. In Proceedings of the Fib Symposium, Attisholz, Switzerland, 16–18 September 2021; pp. 485–492. [Google Scholar] [CrossRef]
- Giordano, L.; Gino, D. Actions on road bridges, Bridge construction and design, PowerPoint slides for PoliTo Courses, DISEG. 2021. Available online: https://file.didattica.polito.it/download/MATDID/33335856 (accessed on 10 January 2023).
- Sotiropoulos, S.; Lagaros, N.D. A two-stage structural optimization-based design procedure of structural systems. Struct. Des. Tall Spec. Build. 2021, 31, e1909. [Google Scholar] [CrossRef]
- Preisinger, C. Linking Structure and Parametric Geometry. Archit. Des. 2013, 83, 110–113. [Google Scholar] [CrossRef]
- Corne, D.W.; Lones, A. Evolutionary Algorithms. In Handbook of Heuristics; Springer International Publishing: Berlin/Heidelberg, Germany, 2018; pp. 1–22. [Google Scholar]
- Sotiropoulos, S.; Lagaros, N.D. Optimum topological bracing design of tall steel frames subjected to dynamic loading. Comput. Struct. 2022, 259, 106705. [Google Scholar] [CrossRef]
- Stromberg, L.L.; Beghini, A.; Baker, W.F.; Paulino, G.H. Topology optimization for braced frames: Combining continuum and beam/column elements. Eng. Struct. 2012, 37, 106–124. [Google Scholar] [CrossRef]
- EN 1993-1-1; Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings. Authority: The European Union Per Regulation 305/2011, Directive 98/34/EC, Directive 2004/18/EC. BSI: London, UK, 2005. (In English)
- Flöry, S. Goat. Free Optimization Solver Component for Rhino’s Grasshopper. Available online: https://www.rechenraum.com/en/goat.html (accessed on 3 January 2023).
- Rutten, D. Galapagos: On the Logic and Limitations of Generic Solvers. J. Archit. Des. 2013, 83, 132–135. [Google Scholar] [CrossRef]
- Tomasetti, T.; Dambron, O.; Peng, M.; Sun, H.; Colibri. A Design Space Exporter for Grasshopper. Published on GitHub Library under MIT License. 2017. Available online: https://github.com/bhowes-tt/colibri.grasshopper (accessed on 9 January 2023).
- Tomasetti, T. Design Explorer 2. Published Open Source under Thornton Tomasetti CORE Studio License. 2019. Available online: http://tt-acm.github.io/DesignExplorer/?GFOLDER=0B8secD5h7wUFanYtbEFWSjBLWFk (accessed on 9 January 2023).






| Max. Displacement [cm] | Percentage Variation [%] | |
|---|---|---|
| Midas | Karamba 3D | |
| 5.2 | 5.3 | +1.5% |
| Girder Forces | Midas | Karamba 3D | Percentage Variation [%] |
|---|---|---|---|
| Max. My [kNm] | 4749.5 | 4750.2 | 0.0% |
| Min. My [kNm] | −158.0 | −157.5 | −0.3% |
| Max. Mz [kNm] | 323.9 | 310.5 | −4.1% |
| Min. Mz [kNm] | −100.7 | −97.7 | −2.9% |
| Max. Mt [kNm] | 55.7 | 53.7 | −3.5% |
| Min. Mt [kNm] | −52.5 | −53.7 | 2.1% |
| Max.Vz [kN] | 671.3 | 670.8 | −0.1% |
| Min. Vz[kN] | −671.3 | −670.8 | −0.1% |
| Max.Vy [kN] | 132.8 | 128.6 | −3.2% |
| Min. Vy[kN] | −132.6 | −128.5 | −3.0% |
| Max. N [kN] | 112.3 | 108.6 | −3.3% |
| Min. N [kN] | −164.4 | −160.8 | −2.2% |
| Permanent loads | |
| Self-weight steel | 78.5 kN/m3 |
| Self-weight concrete | 25 kN/m3 |
| Other permanent loads | |
| Pavement | 3 kN/m2 |
| Curbs | 25 kN/m3 |
| VRS | 2 kN/m |
| Parapet | 1 kN/m |
| Live loads | |
| Traffic load | LM1 |
| Location | Tandem System | UDL System |
|---|---|---|
| Axle Loads Qik [kN] | qik [kN/m2] | |
| Notional lane 1 | 300 | 9 |
| Notional lane 2 | 200 | 2.5 |
| Notional lane 3 | 100 | 2.5 |
| Other lanes | 0 | 2.5 |
| Remaining area | 0 | 2.5 |
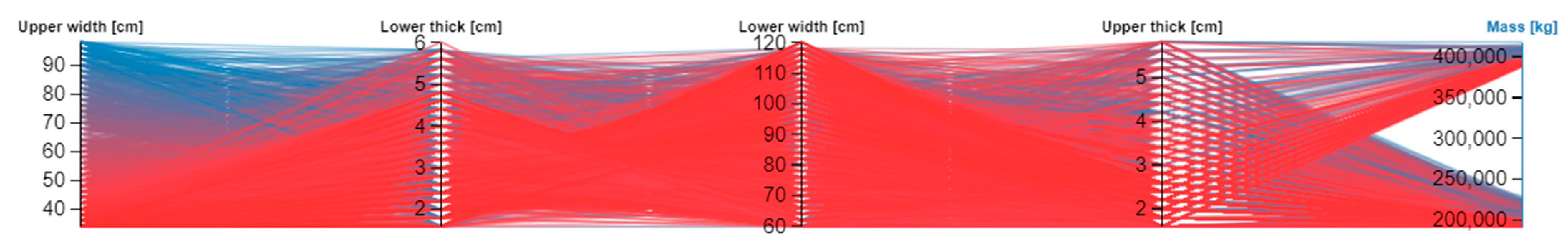
| Variable | Bounds | Increments |
|---|---|---|
| Upper width | [35, 100] cm | 2 cm |
| Upper thickness | [1.6, 6] cm | 0.2 cm |
| Lower width | [60, 120] cm | 2 cm |
| Lower thickness | [1.6, 6] cm | 0.2 cm |
| Steel Grade to EN 10025 | Nominal Thickness of the Element t in mm | |||
|---|---|---|---|---|
| t ≤ 40 mm | 40 mm < t ≤ 80 mm | |||
| fyk [Mpa] | ftk [Mpa] | fyk [Mpa] | ftk [Mpa] | |
| S235 | 235 | 360 | 215 | 360 |
| S275 | 275 | 430 | 255 | 410 |
| S355 | 355 | 510 | 335 | 470 |
| S450 | 450 | 550 | 430 | 550 |
| Variable | Input Data | Results | Percentage Variation [%] |
|---|---|---|---|
| Upper width [cm] | 60.0 | 68.0 | +13.3 |
| Upper thickness [cm] | 4.0 | 3.0 | −25 |
| Lower width [cm] | 80.0 | 78.0 | −2.5 |
| Lower thickness [cm] | 4.0 | 2.6 | −35 |
| Total mass [kg] | 206,199.3 | 198,983.6 | −3.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boretti, V.; Sardone, L.; Bohórquez Graterón, L.A.; Masera, D.; Marano, G.C.; Domaneschi, M. Algorithm-Aided Design for Composite Bridges. Buildings 2023, 13, 865. https://doi.org/10.3390/buildings13040865
Boretti V, Sardone L, Bohórquez Graterón LA, Masera D, Marano GC, Domaneschi M. Algorithm-Aided Design for Composite Bridges. Buildings. 2023; 13(4):865. https://doi.org/10.3390/buildings13040865
Chicago/Turabian StyleBoretti, Valentina, Laura Sardone, Luis Alberto Bohórquez Graterón, Davide Masera, Giuseppe Carlo Marano, and Marco Domaneschi. 2023. "Algorithm-Aided Design for Composite Bridges" Buildings 13, no. 4: 865. https://doi.org/10.3390/buildings13040865
APA StyleBoretti, V., Sardone, L., Bohórquez Graterón, L. A., Masera, D., Marano, G. C., & Domaneschi, M. (2023). Algorithm-Aided Design for Composite Bridges. Buildings, 13(4), 865. https://doi.org/10.3390/buildings13040865








