Collapse Assessment of Mid-Rise RC Dual Wall-Frame Buildings Subjected to Subduction Earthquakes
Abstract
:1. Introduction
2. Collapse Assessment Methodology
2.1. Estimation of Sa(T1) Intensities That Trigger Collapse
2.2. Estimation of Collapse Fragility Functions
2.3. Collapse Performance Metrics: P(C|Sa(T1)MCE), CMR, λc, and Pc(50)
2.4. Deaggregation of λc
3. Methodology to Define Code-Conforming Archetype Buildings
3.1. Statistical Evaluation of Chilean RC Buildings
3.2. Structural Layout and Code-Conforming Design Procedure of the Archetype Buildings
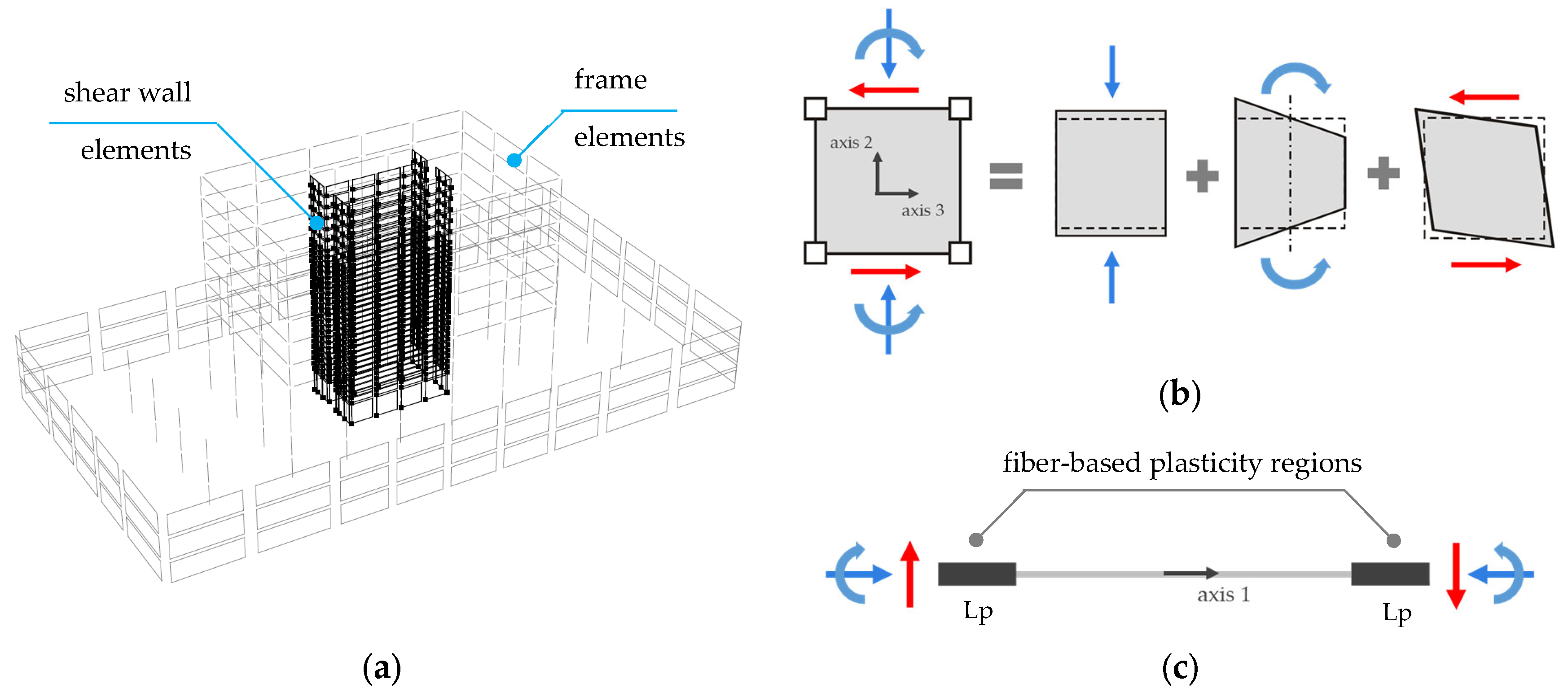
3.3. Modeling Details
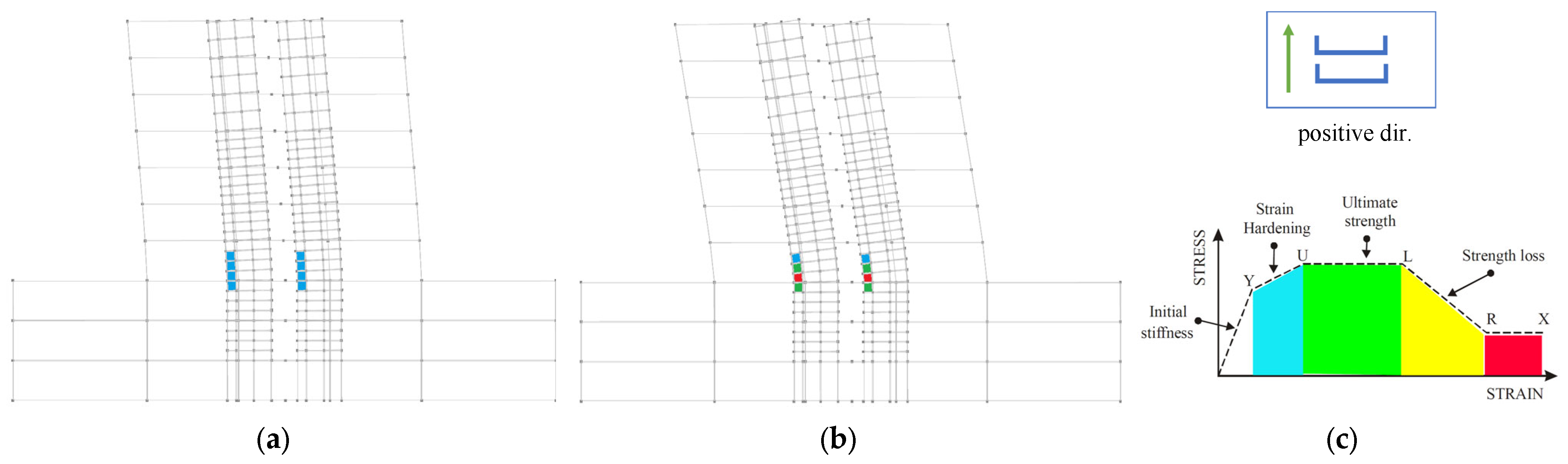
3.4. Seismic Collapse Criteria
3.5. Pushover Analyses
4. Seismic Hazard Analyses and Selection of Subduction Ground Motions
4.1. Seismic Hazard Analyses
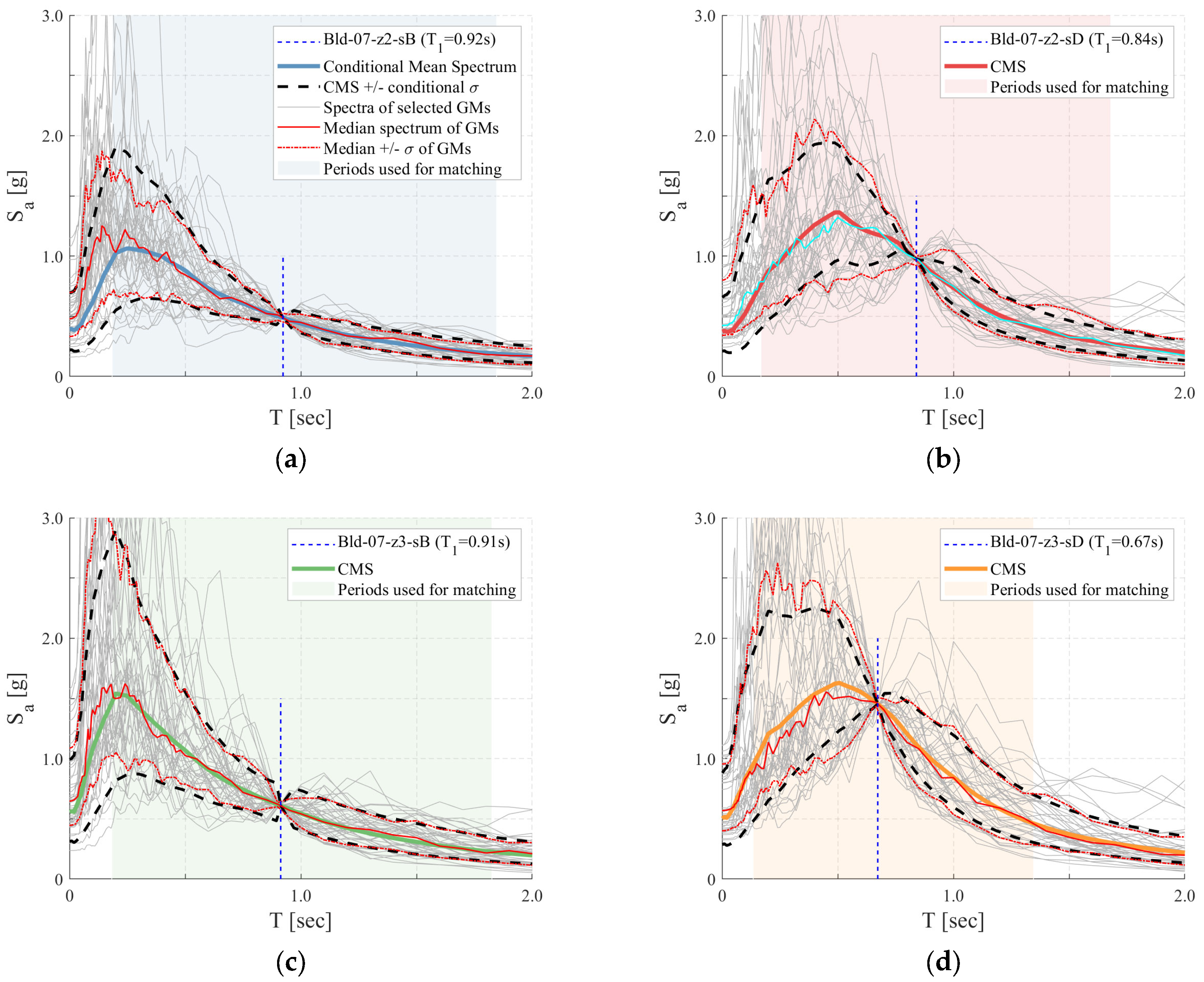
4.2. Selection of Ground Motions
5. Response and Collapse Assessment Results
5.1. IDAs, Collapse Fragility Functions, P(C|Sa(T1)MCE), and CMR
5.2. Values of λc and Pc(50)
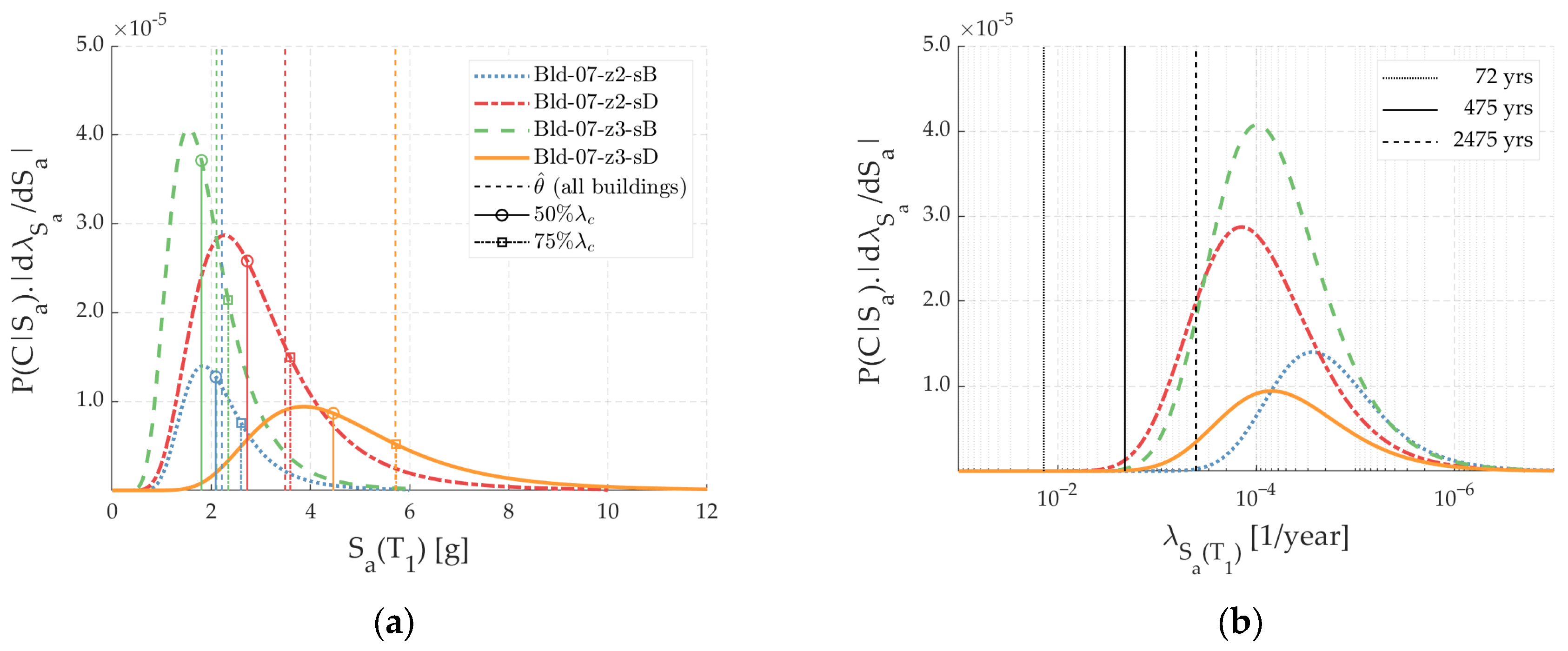
5.3. Deaggregation Values of λc
6. Summary and Closing Remarks
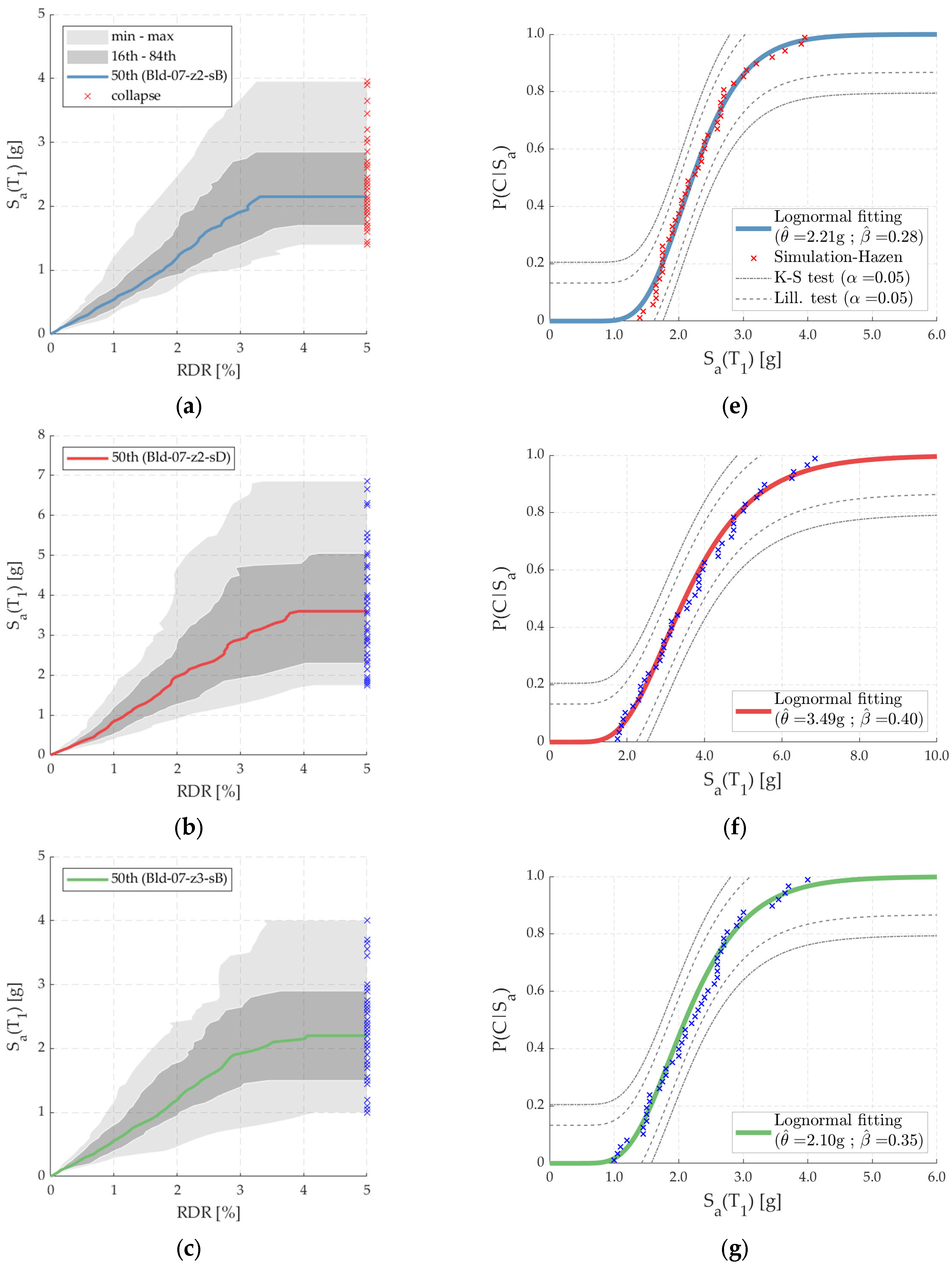
- The lognormal distribution is an adequate representation of the collapse fragility function of the archetype buildings since each collapse fragility function passed the Kolmogorov–Smirnov and Lilliefors goodness-of-fit tests, both at a 5% significance level. Other distribution functions can be tested in further studies.
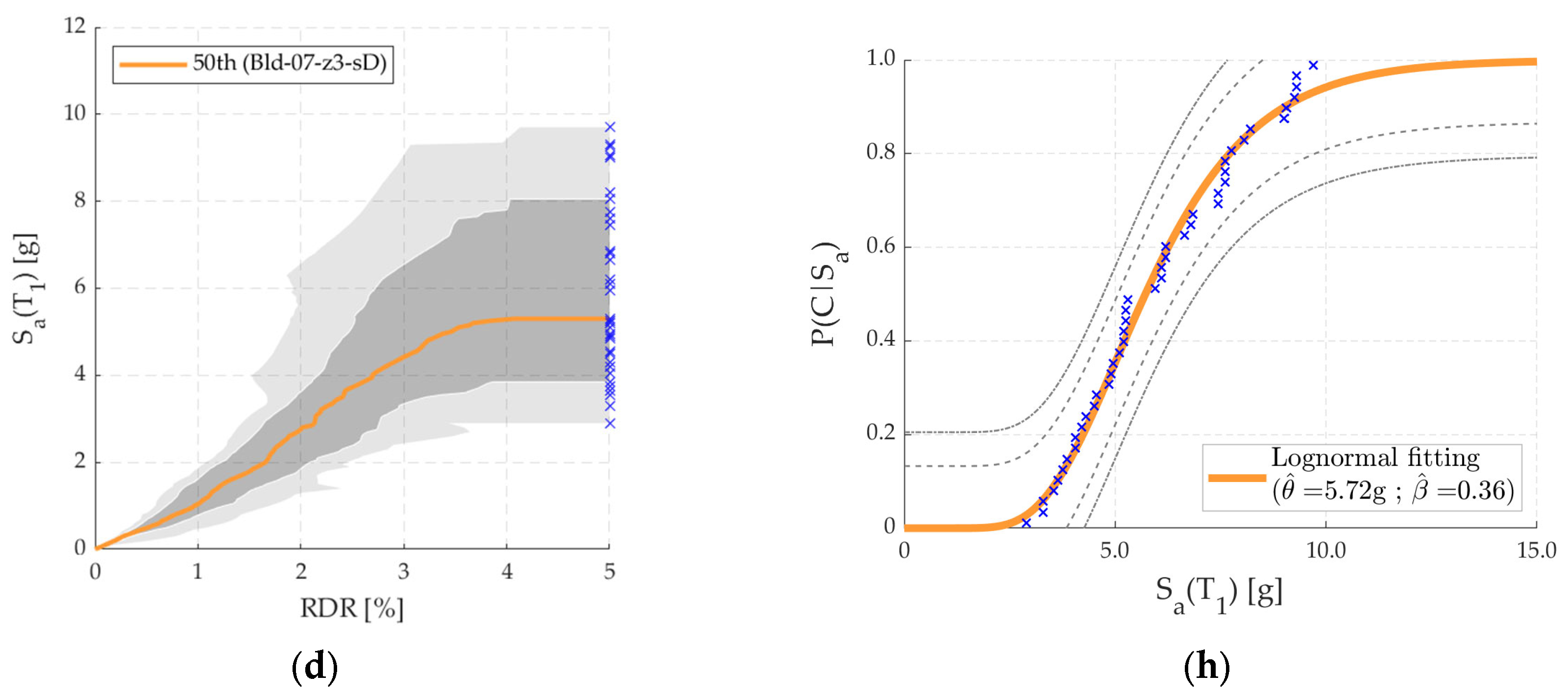
- The estimated median collapse () and logarithmic dispersion () values were 2.21 g, 3.49 g, 2.10 g, and 5.72 g, and 0.28, 0.40, 0.35, and 0.36, for archetype buildings Bld-07-z2-sB, Bld-07-z2-sD, Bld-07-z3-sB, and Bld-07-z3-sD, respectively.
- The analysis and comparison of the collapse fragilities based on λSa(T1) (as an alternative to Sa(T1)) generated meaningful information since adequate direct comparisons of the conditional collapse probability can be obtained at different hazard levels such as the SLE, 50%—50 years; DBE, 10%—50 years; and MCE, 2%—50 years. Specifically, all archetype buildings had a negligible collapse probability at the SLE and DBE levels. At the MCE level, the archetype buildings Bld-07-z2-sD and Bld-07-z3-sB had non-negligible collapse probabilities (2.78% and 1.58%, respectively). However, all these MCE collapse probabilities were smaller than the 10% target defined in ASCE 7-22. Consequently, current Chilean seismic design standards seem to provide adequate levels of collapse prevention, which is in agreement with the observed performance of modern RC buildings in recent earthquakes.
- The calculated values of the CMR (i.e., divided by the MCE intensity) ranged from 2.1 to 2.7, with a mean of 2.3. This mean value was higher (i.e., lower collapse risk) than the values stated in previous studies on RC frame buildings designed using US seismic codes and subjected to crustal ground motions. No clear influence of the seismic zone and soil type on the CMR was identified.
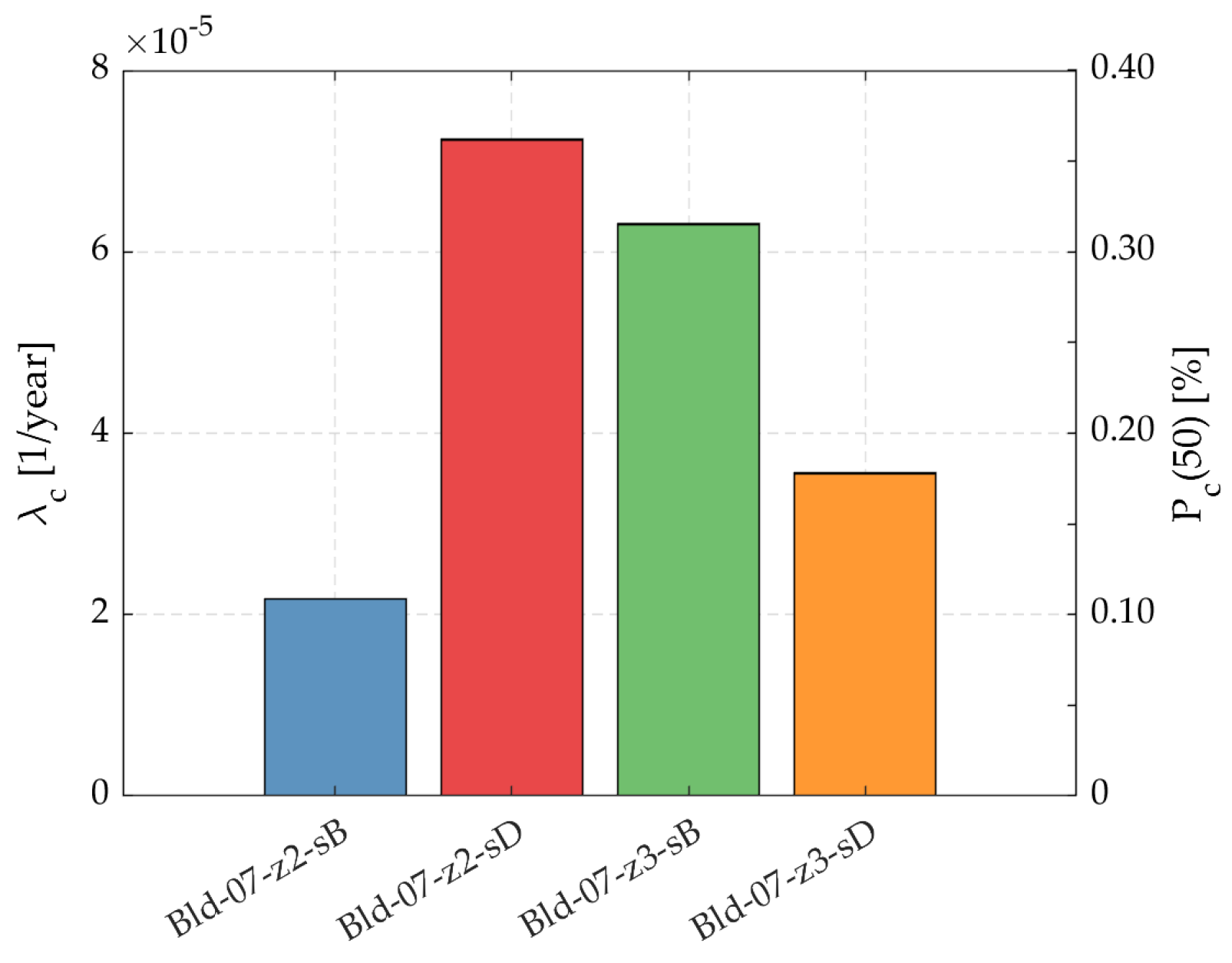
- The estimated values of λc and Pc(50) were 2.17 × 10−5, 7.24 × 10−5, 6.31 × 10−5, and 3.56 × 10−5, and 0.11%, 0.36%, 0.31%, and 0.18% for archetypes Bld-07-z2-sB, Bld-07-z2-sD, Bld-07-z3-sB, and Bld-07-z3-sD, respectively. The values of Pc(50) largely fulfilled the maximum 1% target collapse probability in 50 years stated by ASCE 7-22, indicating, once more, the adequate level of collapse prevention provided by current Chilean seismic design standards.
- Non-negligible differences were found between the values of Pc(50) for the different archetype buildings but no clear influence of the seismic zone and soil type on Pc(50) was observed. Although Chilean seismic design codes are mostly prescriptive and do not include explicit PBEE design targets, the collapse risk should nevertheless be more uniform, suggesting that current Chilean seismic design codes (particularly the design spectra) might require a revision.
- Lastly, the deaggregation of λc (as a function of λSa(T1), which allows adequate direct comparisons between the deaggregation functions) showed that the values of were always higher than the Sa(T1) values at 50% of λc. In other words, in terms of the collapse risk, the contribution of the Sa(T1) values at the Sa(T1) < range was greater than that of the Sa(T1) values at the Sa(T1) > range. This observation means that, contrary to intuition, the accurate characterization of collapse fragilities is more important at the Sa(T1) < range than at the Sa(T1) > range.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ruiz, S.; Madariaga, R. Historical and recent large megathrust earthquakes in Chile. Tectonophysics 2018, 733, 37–56. [Google Scholar] [CrossRef]
- Massone, L.M.; Bonelli, P.; Lagos, R. Seismic design and construction practices for RC structural wall buildings. Earthq. Spectra 2012, 28, S245–S256. [Google Scholar] [CrossRef]
- EERI. The Mw 8.8 Chile Earthquake of 27 February 2010; Earthquake Engineering Research Institute: Oakland, CA, USA, 2010. [Google Scholar]
- Zareian, F.; Krawinkler, H. Assessment of probability of collapse and design for collapse safety. Earthq. Eng. Struct. Dyn. 2007, 36, 1901–1914. [Google Scholar] [CrossRef]
- Tena-Colunga, A.; Sanchez-Ballinas, D. The collapse of Alvaro Obregon 286 building in Mexico City during the September 19, 2017 earthquake. A case study. J. Build. Eng. 2022, 49, 104060. [Google Scholar] [CrossRef]
- EERI. Mw 8.2 Iquique, Chile Earthquake and Tsunami: Preliminary Reconnaissance Observations; Earthquake Engineering Research Institute: Oakland, CA, USA, 2014. [Google Scholar]
- GEER. Geotechnical Reconnaissance of the 2015 M8.3 Illapel, Chile Earthquake; Geotechnical Extreme Events Reconnaissance (GEER) Association, Report No. GEER-043; GEER, 2015. [Google Scholar]
- INN. Norma Chilena Oficial NCh433 Of. 1996 Mod. 2009 Diseño Sismico de Edificios; Instituto Nacional de Normalizacion: Santiago, Chile, 2009. (In Spanish) [Google Scholar]
- MINVU. DS 61 Diseño Sismico de Edificios; Ministerio de Vivienda y Urbanismo: Santiago, Chile, 2011. (In Spanish) [Google Scholar]
- ASCE. Minimum Design Loads and Associated Criteria for Buildings and Other Structures ASCE/SEI 7-22; American Association of Civil Engineers: Reston, VA, USA, 2022. [Google Scholar]
- MINVU. DS 60 Requisitos de Diseño y Calculo Para el Hormigon Armado; Ministerio de Vivienda y Urbanismo: Santiago, Chile, 2011. (In Spanish) [Google Scholar]
- Lagos, R.; Lafontaine, M.; Bonelli, P.; Boroschek, R.; Guendelman, T.; Massone, L.; Saragoni, R.; Rojas, F.; Yañez, F. The quest for resilience: The Chilean practice of seismic design for reinforced concrete buildings. Earthq. Spectra 2021, 37, 26–45. [Google Scholar] [CrossRef]
- FEMA. Seismic Performance Assessment of Buildings FEMA P-58; Federal Emergency Management Agency: Hyattsville, MD, USA, 2018. [Google Scholar]
- Araya-Letelier, G.; Parra, P.F.; Lopez-Garcia, D.; Garcia-Valdes, A.; Candia, G.; Lagos, R. Collapse risk assessment of a Chilean dual wall-frame reinforced concrete office building. Eng. Struct. 2019, 183, 770–779. [Google Scholar] [CrossRef]
- Cando, M.A.; Hube, M.A.; Parra, P.F.; Arteta, C.A. Effect of stiffness on the seismic performance of code-conforming reinforced concrete shear wall buildings. Eng. Struct. 2020, 219, 110724. [Google Scholar] [CrossRef]
- FEMA. Quantification of Building Seismic Performance Factors FEMA P695; Federal Emergency Management Agency: Hyattsville, MD, USA, 2009. [Google Scholar]
- Gogus, A.; Wallace, J.W. Seismic safety evaluation of reinforced concrete walls through FEMA P695 methodology. J. Struct. Eng. 2015, 141, 04015002. [Google Scholar] [CrossRef]
- Arabzadeh, H.; Galal, K. Seismic collapse risk assessment and FRP retrofitting of RC coupled C-shaped core walls using the FEMA P695 methodology. J. Struct. Eng. 2017, 143, 04017096. [Google Scholar] [CrossRef]
- Dabaghi, M.; Saad, G.; Allhassania, N. Seismic collapse fragility analysis of reinforced concrete shear wall buildings. Earthq. Spectra 2019, 35, 383–404. [Google Scholar] [CrossRef]
- Terzic, V.; Kolozvari, K.; Saldana, D. Implications of modeling approaches on seismic performance of low- and mid-rise office and hospital shear wall buildings. Eng. Struct. 2019, 189, 129–146. [Google Scholar] [CrossRef]
- Marafi, N.A.; Ahmed, K.A.; Lehman, D.E.; Lowes, L.N. Variability in seismic collapse probabilities of solid- and coupled-wall buildings. J. Struct. Eng. 2019, 145, 04019047. [Google Scholar] [CrossRef]
- Raghunandan, M.; Liel, A.; Luco, N. Collapse risk of buildings in the Pacific northwest region due to subduction earthquakes. Earthq. Spectra 2015, 31, 2087–2115. [Google Scholar] [CrossRef]
- Chandramohan, R.; Baker, J.; Deierlein, G. Impact of hazard-consistent ground motion duration in structural collapse risk assessment. Earthq. Eng. Struct. Dyn. 2016, 45, 1357–1379. [Google Scholar] [CrossRef]
- Medalla, M.; Lopez-Garcia, D.; Zareian, F. Seismic characterization of steel special moment frames subjected to megathrust earthquakes. Earthq. Spectra 2020, 36, 2033–2057. [Google Scholar] [CrossRef]
- Marafi, N.A.; Makdisi, A.J.; Eberhard, M.O.; Berman, J.W. Impacts of an M9 Cascadia subduction zone earthquake and Seattle basin on performance of RC core wall buildings. J. Struct. Eng. 2020, 146, 04019201. [Google Scholar] [CrossRef]
- Vamvatsikos, D.; Cornell, C.A. Incremental dynamic analysis. Earthq. Eng. Struct. Dyn. 2002, 31, 491–514. [Google Scholar] [CrossRef]
- Monjardin-Quevedo, J.G.; Valenzuela-Beltran, F.; Reyes-Salazar, A.; Leal-Graciano, J.M.; Torres-Carrillo, X.G.; Gaxiola-Camacho, J.R. Probabilistic assessment of buildings subjected to multi-level earthquake loading based on the PBSD concept. Buildings 2022, 12, 1942. [Google Scholar] [CrossRef]
- Kostinakis, K.; Fontara, I.; Athanatopoulou, A. Scalar structure-specific ground motion intensity measures for assessing the seismic performance of structures: A review. J. Earthq. Eng. 2018, 22, 630–665. [Google Scholar] [CrossRef]
- Rong, X.; Yang, J.; Jun, L.; Zhang, Y.; Zheng, S.; Dong, L. Optimal ground motion intensity measure for seismic assessment of high-rise reinforced concrete structures. Case Stud. Constr. Mater. 2023, 18, e01678. [Google Scholar] [CrossRef]
- Shome, N. Probabilistic Seismic Demand Analysis of Nonlinear Structures. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1999. [Google Scholar]
- Ibarra, L.F.; Krawinkler, H. Global Collapse of Frame Structures under Seismic Excitations; Technical Report 152; John A. Blume Earthquake Engineering Center: Stanford, CA, USA, 2005. [Google Scholar]
- Eads, L.; Miranda, E.; Krawinkler, H.; Lignos, D. An efficient method for estimating the collapse risk of structures in seismic regions. Earthq. Eng. Struct. Dyn. 2013, 42, 25–41. [Google Scholar] [CrossRef]
- Baker, J.W. Efficient analytical fragility function fitting using dynamic structural analysis. Earthq. Spectra 2015, 31, 579–599. [Google Scholar] [CrossRef]
- Massey, F.J., Jr. The Kolmogorov-Smirnov test for goodness of fit. J. Am. Stat. Assoc. 1951, 46, 68–78. [Google Scholar] [CrossRef]
- Lilliefors, H. On the Kolmogorov-Smirnov test for normality with mean and variance unknown. J. Am. Stat. Assoc. 1967, 62, 399–402. [Google Scholar] [CrossRef]
- INE. Base de Datos de Permisos de Edificación; Instituto Nacional de Estadística: Santiago, Chile, 2020. (In Spanish) [Google Scholar]
- IBC. 2021 International Building Code; International Code Council: Country Club Hills, IL, USA, 2021. [Google Scholar]
- Araya-Letelier, G. Design of Building Structural Systems and Enhanced Partition Walls to Improve the Life Cycle Costs Associated with Risk of Earthquake Damage. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2014. [Google Scholar]
- Porcu, M.C.; Vielma Pérez, J.C.; Pais, G.; Osorio Bravo, D.; Vielma Quintero, J.C. Some issues in the seismic assessment of shear-wall buildings through code-compliant dynamic analyses. Buildings 2022, 12, 694. [Google Scholar] [CrossRef]
- CSI. ETABS v16 User’s Guide Manual; Computers & Structures: Berkeley, CA, USA, 2016. [Google Scholar]
- Akcelyan, S.; Lignos, D.G. Seismic Assessment and retrofit of pre-Northridge high rise steel moment resisting frame buildings with bilinear oil dampers. Buildings 2023, 13, 139. [Google Scholar] [CrossRef]
- ACI. Building Code Requirements for Structural Concrete and Commentary ACI 318-08; American Concrete Institute: Farmington Hills, MI, USA, 2008. [Google Scholar]
- CSI. PERFORM 3D 7.0.0 Manual; Computer & Structures: Berkeley, CA, USA, 2017. [Google Scholar]
- NIST. Nonlinear Structural Analysis for Seismic Design: A Guide for Practicing Engineers NIST GCR 10-917-5; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2010. [Google Scholar]
- ATC. Modeling and Acceptance Criteria for Seismic Design and Analysis of Tall Buildings PEER/ATC 72-1; Applied Technology Council: Redwood City, CA, USA, 2010. [Google Scholar]
- NIST. Recommended Modeling Parameters and Acceptance Criteria for Nonlinear Analysis in Support of Seismic Evaluation, Retrofit, and Design NIST GCR 17-917-45; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2017. [Google Scholar]
- NIST. Guidelines for Nonlinear Structural Analysis for Design of Buildings Part I—General, NIST GCR 17-917-46v1; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2017. [Google Scholar]
- ACHISINA. Alternative Procedure for the Seismic Analysis and Design of Tall Buildings; Asociacion Chilena de Sismologia e Ingenieria Antisismica: Santiago, Chile, 2017. (In Spanish) [Google Scholar]
- TBI. Guidelines for Performance-Based Seismic Design of Tall Buildings; PEER Report 2017-06; Pacific Earthquake Engineering Research Center: Berkeley, CA, USA, 2017. [Google Scholar]
- LATBSDC. An Alternative Procedure for Seismic Analysis and Design of Tall Buildings Located in the Los Angeles Region; Los Angeles Tall Buildings Structural Design Council: Los Angeles, CA, USA, 2020. [Google Scholar]
- Ugalde, D.; Lopez-Garcia, D. Analysis of the seismic capacity of Chilean residential RC shear wall buildings. J. Build. Eng. 2020, 31, 101369. [Google Scholar] [CrossRef]
- Massone, L.M.; Bedecarratz, E.; Rojas, F.; Lafontaine, M. Nonlinear modeling of a damaged reinforced concrete building and design improvement behavior. J. Build. Eng. 2021, 41, 102766. [Google Scholar] [CrossRef]
- Lowes, L.N.; Lehman, D.E.; Baker, C. Recommendations for modeling the nonlinear response of slender reinforced concrete walls using PERFORM-3D. In Proceedings of the SEAOC Convention, Maui, HI, USA, 12–15 October 2016. [Google Scholar]
- Kolozvari, K.; Arteta, C.; Fischinger, M.; Gavridou, S.; Hube, M.; Isakovic, T.; Lowes, L.; Orakcal, K.; Vásquez, J.; Wallace, J. Comparative study of state-of-the-art macroscopic models for planar reinforced concrete walls. ACI Struct. J. 2018, 115, 1637–1657. [Google Scholar] [CrossRef]
- Coleman, J.; Spacone, E. Localization issues in force-based frame elements. J. Struct. Eng. 2001, 127, 1257–1265. [Google Scholar] [CrossRef]
- Dazio, A.; Beyer, K.; Bachmann, H. Quasi-static cyclic tests and plastic hinge analysis of RC structural walls. Eng. Struct. 2009, 31, 1556–1571. [Google Scholar] [CrossRef]
- Thomsen, J.H.; Wallace, J.W. Displacement-Based Design of Reinforced Concrete Structural Walls: An Experimental Investigation of Walls with Rectangular and T-Shaped Cross-Sections; Tech. Rep. No. CU/CEE-95/06; Department of Civil Engineering, Clarkson University: Postdam, NY, USA, 1995. [Google Scholar]
- Ugalde, D.; Parra, P.F.; Lopez-Garcia, D. Assessment of the seismic capacity of tall wall buildings using nonlinear finite element modeling. Bull. Earthq. Eng. 2019, 17, 6565–6589. [Google Scholar] [CrossRef]
- Paulay, T.; Priestley, M.J.N. Seismic Design of Reinforced Concrete and Masonry Buildings; Wiley: Hoboken, NJ, USA, 1992. [Google Scholar]
- Yang, T.Y.; Moehle, J.P.; Bozorgnia, Y.; Zareian, F.; Wallace, J.W. Performance assessment of tall concrete core-wall building designed using two alternative approaches. Earthq. Eng. Struct. Dyn. 2012, 45, 1515–1531. [Google Scholar] [CrossRef]
- Odabasi, O.; Kohrangi, M.; Bazzurro, P. Seismic collapse risk of reinforced concrete tall buildings in Istanbul. Bull. Earthq. Eng. 2021, 19, 6545–6571. [Google Scholar] [CrossRef]
- Kim, T.; Foutch, D.A. Application of FEMA methodology to RC shear wall buildings governed by flexure. Eng. Struct. 2007, 29, 2514–2522. [Google Scholar] [CrossRef]
- ASCE. Seismic Evaluation and Retrofit of Existing Buildings ASCE/SEI 41-17; American Association of Civil Engineers: Reston, VA, USA, 2017. [Google Scholar]
- Poulos, A.; Monsalve, M.; Zamora, N.; de la Llera, J.C. An updated recurrence model for Chilean subduction seismicity and statistical validation of its Poisson nature. Bull. Seismol. Soc. Am. 2019, 109, 66–74. [Google Scholar] [CrossRef]
- Montalva, G.A.; Bastías, N.; Rodriguez-Marek, A. Ground-motion prediction equation for the Chilean subduction zone. Bull. Seismol. Soc. Am. 2017, 107, 901–911. [Google Scholar] [CrossRef]
- Idini, B.; Rojas, F.; Ruiz, S.; Pasten, C. Ground motion prediction equations for the Chilean subduction zone. Bull. Earthq. Eng. 2017, 15, 1853–1880. [Google Scholar] [CrossRef]
- Candia, G.; Macedo, J.; Jaimes, M.A.; Magna-Verdugo, C. A new state-of-the-art platform for probabilistic and deterministic seismic hazard assessment. Seismol. Res. Lett. 2019, 90, 226–2275. [Google Scholar] [CrossRef]
- Baker, J.W. Conditional mean spectrum: Tool for ground motion selection. J. Struct. Eng. 2011, 137, 322–331. [Google Scholar] [CrossRef]
- Candia, G.; Poulos, A.; de la Llera, J.C.; Crempien, J.; Macedo, J. Correlations of spectral accelerations in the Chilean subduction zone. Earthq. Spectra 2020, 36, 788–805. [Google Scholar] [CrossRef]
- Lin, T.; Harmsen, S.C.; Baker, J.W.; Luco, N. Conditional spectrum computation incorporating multiple causal earthquakes and ground motion prediction models. Bull. Seismol. Soc. Am. 2013, 103, 1103–1116. [Google Scholar] [CrossRef]
- Castro, S.; Benavente, R.; Crempien, J.G.F.; Candia, G.; De la Llera, J.C. A consistently processed strong-motion database for Chilean earthquakes. Seismol. Res. Lett. 2022, 93, 2700–2718. [Google Scholar] [CrossRef]
- Baker, J.W.; Lee, C. An improved algorithm for selecting ground motions to match a conditional spectrum. J. Earthq. Eng. 2018, 22, 708–723. [Google Scholar] [CrossRef]
- NIST. Selecting and Scaling Earthquake Ground Motions for Performing Response-History Analyses NIST GCR 11-917-15; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2011. [Google Scholar]
- Davalos, H.; Miranda, E. Evaluation of bias on the probability of collapse from amplitude scaling using spectral-shape-matched records. Earthq. Eng. Struct. Dyn. 2019, 48, 970–986. [Google Scholar] [CrossRef]
- Davalos, H.; Miranda, E. Evaluation of the scaling factor bias influence on the probability of collapse using Sa(T1) as the intensity measure. Earthq. Spectra 2019, 35, 679–702. [Google Scholar] [CrossRef]









| Archetype | Transverse Direction | Longitudinal Direction | Cmin | ||||||
|---|---|---|---|---|---|---|---|---|---|
| T (s) | R* | Reff | C (%) | T (s) | R* | Reff | C (%) | (%) | |
| Bld-07-z2-sB | 0.923 | 8.6 | 6.5 | 5.0 | 0.465 | 6.2 | 6.2 | 6.0 | 5.0 |
| Bld-07-z2-sD | 0.835 | 6.1 | 6.1 | 7.0 | 0.426 | 3.7 | 3.7 | 11.2 | 6.0 |
| Bld-07-z3-sB | 0.913 | 8.6 | 6.5 | 6.7 | 0.464 | 6.2 | 6.2 | 8.1 | 6.7 |
| Bld-07-z3-sD | 0.673 | 5.9 | 5.9 | 9.8 | 0.357 | 3.6 | 3.6 | 15.7 | 8.0 |
| Archetype | Core Walls | Beams | Columns | ||||
|---|---|---|---|---|---|---|---|
| Flanges | Webs | (b × h) | (b × h) | ||||
| l | t | l | l | us3–s2° | s3°–s7° | ||
| Bld-07-z2-sB | 4.2 | 0.35 | 16.0 | 0.25 | 0.6 × 0.5 | 0.7 × 0.7 | 0.6 × 0.6 |
| Bld-07-z2-sD | 4.2 | 0.45 | 16.0 | 0.30 | 0.6 × 0.5 | 0.7 × 0.7 | 0.6 × 0.6 |
| Bld-07-z3-sB | 4.2 | 0.35 | 16.0 | 0.25 | 0.6 × 0.5 | 0.7 × 0.7 | 0.6 × 0.6 |
| Bld-07-z3-sD | 4.2 | 0.55 | 16.0 | 0.40 | 0.7 × 0.6 | 1.0 × 1.0 | 0.8 × 0.8 |
| Archetype | Vdes | Vdes/W | Vmax+ | Vmax+/W | Ω+ | Vmax− | Vmax−/W | Ω− |
|---|---|---|---|---|---|---|---|---|
| (tonf) | (%) | (tonf) | (%) | (tonf) | (%) | |||
| Bld-07-z2-sB | 348 | 4.7 | 1524 | 20.4 | 4.4 | 1283 | 17.2 | 3.7 |
| Bld-07-z2-sD | 826 | 10.7 | 2036 | 26.3 | 2.5 | 2038 | 26.3 | 2.5 |
| Bld-07-z3-sB | 464 | 6.2 | 1827 | 24.4 | 3.9 | 1495 | 20.0 | 3.2 |
| Bld-07-z3-sD | 1231 | 15.1 | 2918 | 35.7 | 2.4 | 3149 | 38.5 | 2.6 |
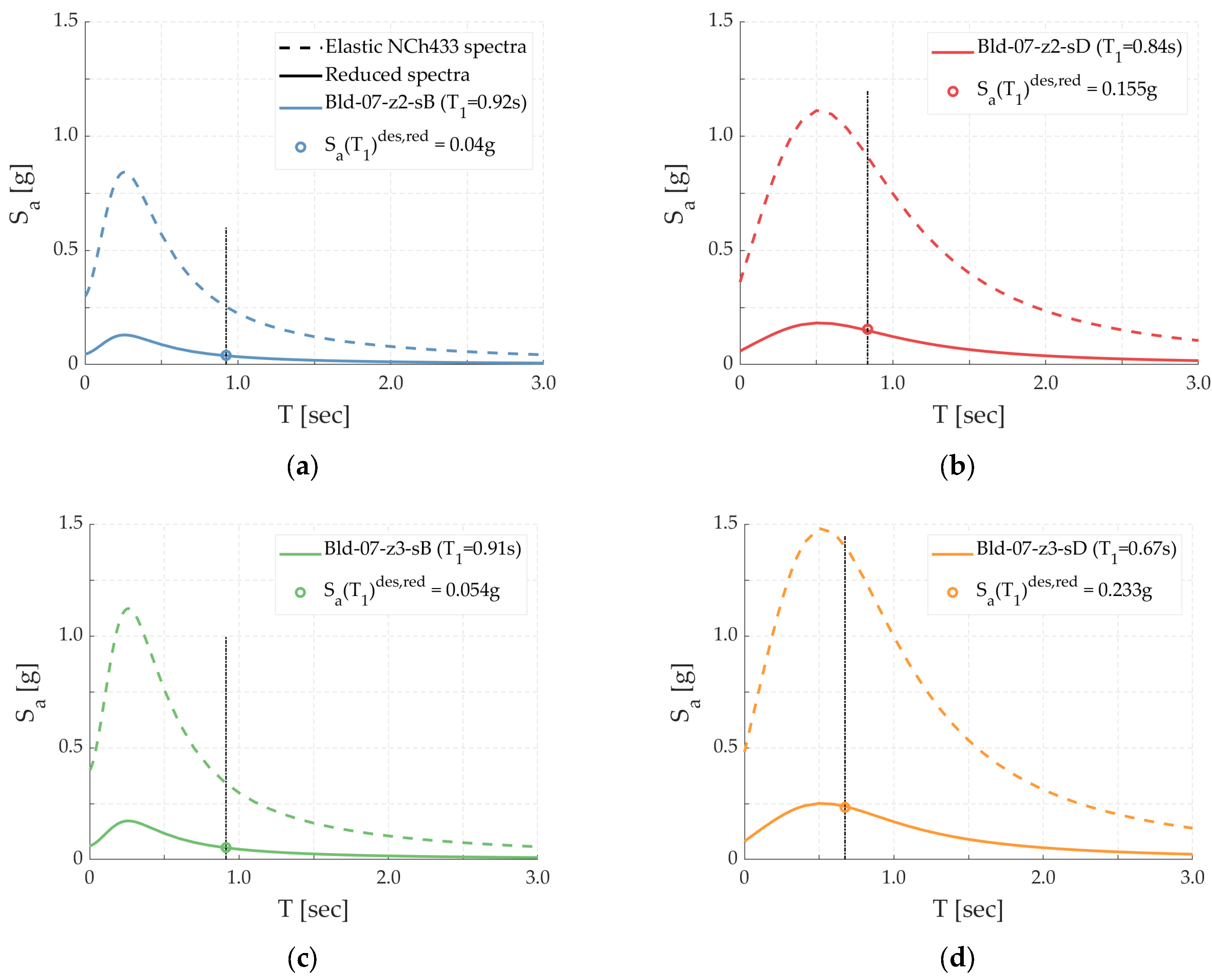
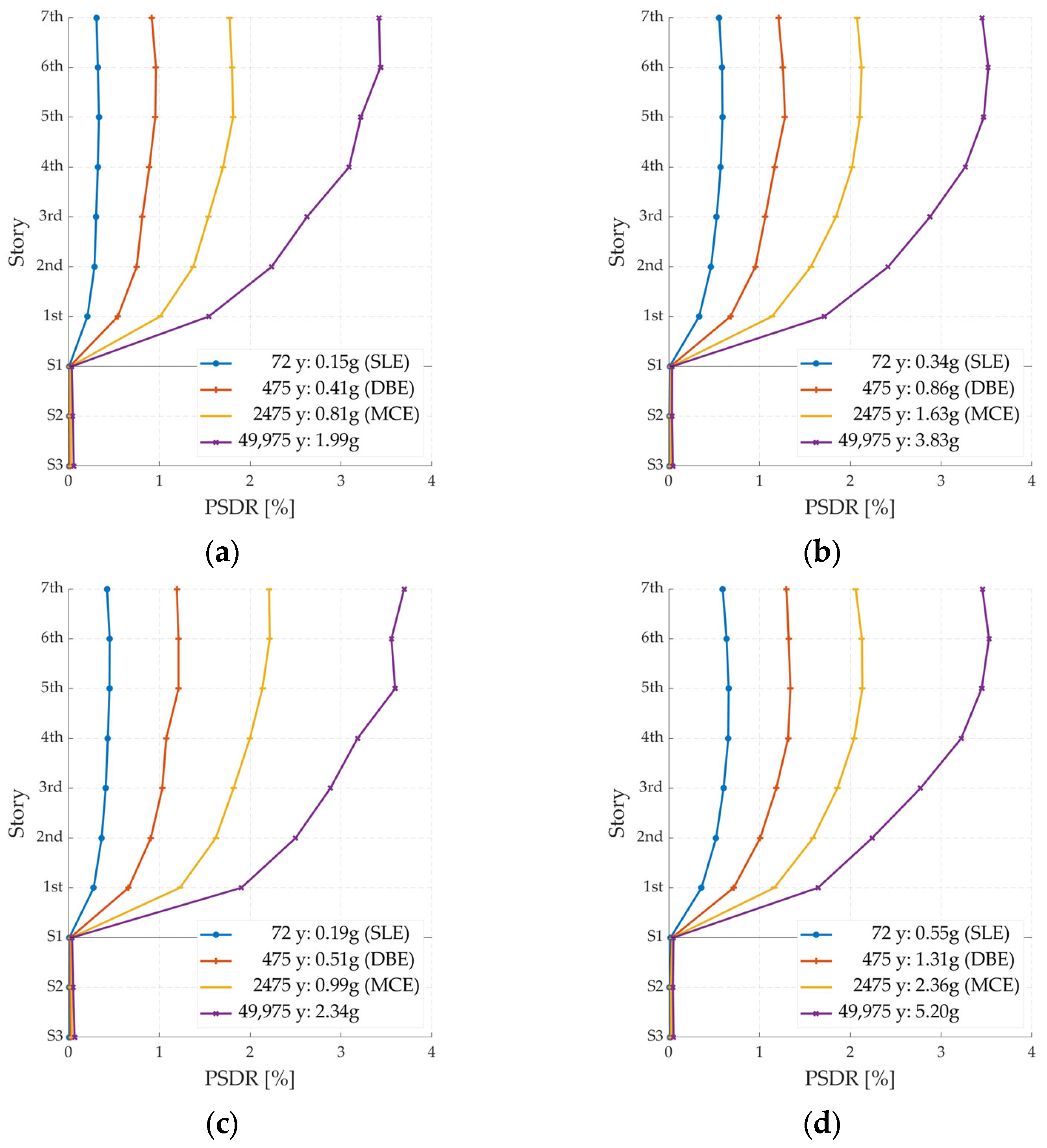
| Archetype | Sa(T1)SLE (g) | Sa(T1)DBE (g) | Sa(T1)MCE (g) | Sa(T1)des,red (g) | Sa(T1)des,e (g) | P(%) in 50 y | Tr (y) |
|---|---|---|---|---|---|---|---|
| Bld-07-z2-sB | 0.16 | 0.41 | 0.81 | 0.040 | 0.261 | 24 | 180 |
| Bld-07-z2-sD | 0.34 | 0.86 | 1.63 | 0.155 | 0.943 | 8 | 600 |
| Bld-07-z3-sB | 0.19 | 0.52 | 0.99 | 0.054 | 0.348 | 22 | 200 |
| Bld-07-z3-sD | 0.55 | 1.31 | 2.36 | 0.233 | 1.377 | 9 | 530 |
| Archetype | K–S | Lilliefors | P(C|Sa(T1)MCE) | CMR | ||
|---|---|---|---|---|---|---|
| (g) | Test? | Test? | (%) | |||
| Bld-07-z2-sB | 2.21 | 0.28 | Pass | Pass | 0.02 | 2.7 |
| Bld-07-z2-sD | 3.49 | 0.40 | Pass | Pass | 2.78 | 2.1 |
| Bld-07-z3-sB | 2.10 | 0.35 | Pass | Pass | 1.58 | 2.1 |
| Bld-07-z3-sD | 5.72 | 0.36 | Pass | Pass | 0.66 | 2.4 |
| Archetype | λc (1/Year) | Pc(50) (%) | λc(λSa(T1) = 10−4)/λc (%) |
|---|---|---|---|
| Bld-07-z2-sB | 2.17 × 10−5 | 0.11 | 5.4 |
| Bld-07-z2-sD | 7.24 × 10−5 | 0.36 | 42.1 |
| Bld-07-z3-sB | 6.31 × 10−5 | 0.31 | 32.6 |
| Bld-07-z3-sD | 3.56 × 10−5 | 0.18 | 25.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gallegos, M.F.; Araya-Letelier, G.; Lopez-Garcia, D.; Parra, P.F. Collapse Assessment of Mid-Rise RC Dual Wall-Frame Buildings Subjected to Subduction Earthquakes. Buildings 2023, 13, 880. https://doi.org/10.3390/buildings13040880
Gallegos MF, Araya-Letelier G, Lopez-Garcia D, Parra PF. Collapse Assessment of Mid-Rise RC Dual Wall-Frame Buildings Subjected to Subduction Earthquakes. Buildings. 2023; 13(4):880. https://doi.org/10.3390/buildings13040880
Chicago/Turabian StyleGallegos, Marco F., Gerardo Araya-Letelier, Diego Lopez-Garcia, and Pablo F. Parra. 2023. "Collapse Assessment of Mid-Rise RC Dual Wall-Frame Buildings Subjected to Subduction Earthquakes" Buildings 13, no. 4: 880. https://doi.org/10.3390/buildings13040880
APA StyleGallegos, M. F., Araya-Letelier, G., Lopez-Garcia, D., & Parra, P. F. (2023). Collapse Assessment of Mid-Rise RC Dual Wall-Frame Buildings Subjected to Subduction Earthquakes. Buildings, 13(4), 880. https://doi.org/10.3390/buildings13040880













